Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-PAS-HIG-20-011 | ||
| Combination of searches for nonresonant Higgs boson pair production in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | ||
| CMS Collaboration | ||
| 2024-11-15 | ||
| Abstract: This note presents a comprehensive overview and statistical combination of searches for the nonresonant production of Higgs boson pairs (HH) using data from proton-proton collisions collected by the CMS experiment at the LHC from 2016 to 2018 at a centre-of-mass energy of 13 TeV, corresponding to a total integrated luminosity of 138 fb$ ^{-1} $. Upper limits at 95% confidence level are set on the rate of the HH production. The observed (expected) upper limit on the inclusive production cross section relative to the standard model expectation is found to be 3.5 (2.5). Assuming all other Higgs boson couplings are equal to their values in the standard model, we exclude HH production at 95% confidence level for values of the Higgs boson trilinear self-coupling modifier $ \kappa_{\lambda} $ outside the range between $-$1.39 and 7.02. Similarly, for the coupling modifier $ \kappa_{2\mathrm{V}} $ affecting the interaction between two vector bosons and two Higgs bosons, we exclude HH production for values outside the range between 0.62 and 1.42. This work also studies HH production in new physics scenarios, using the Higgs effective field theory parametrisation. An extrapolation of the results to the integrated luminosity expected after the high-luminosity upgrade of the LHC is also presented. | ||
| Links: CDS record (PDF) ; CADI line (restricted) ; | ||
| Figures | |
png pdf |
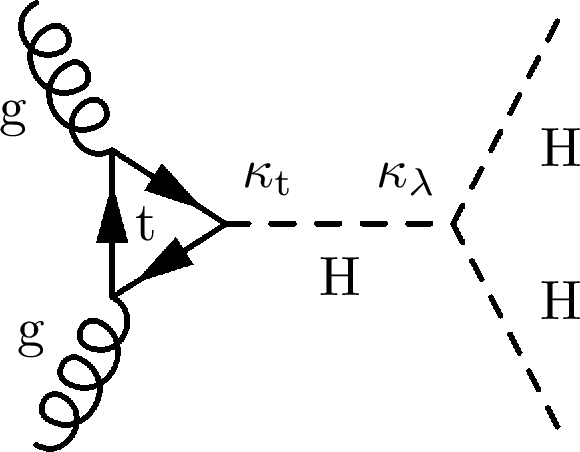
Figure 1:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via gluon-gluon fusion in the standard model. The modifiers of the Higgs boson coupling with the top quark and the Higgs boson trilinear self-coupling are shown as $ \kappa_{\mathrm{t}} $ and $ \kappa_{\lambda} $, respectively. |
png pdf |
Figure 1-a:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via gluon-gluon fusion in the standard model. The modifiers of the Higgs boson coupling with the top quark and the Higgs boson trilinear self-coupling are shown as $ \kappa_{\mathrm{t}} $ and $ \kappa_{\lambda} $, respectively. |
png pdf |
Figure 1-b:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via gluon-gluon fusion in the standard model. The modifiers of the Higgs boson coupling with the top quark and the Higgs boson trilinear self-coupling are shown as $ \kappa_{\mathrm{t}} $ and $ \kappa_{\lambda} $, respectively. |
png pdf |
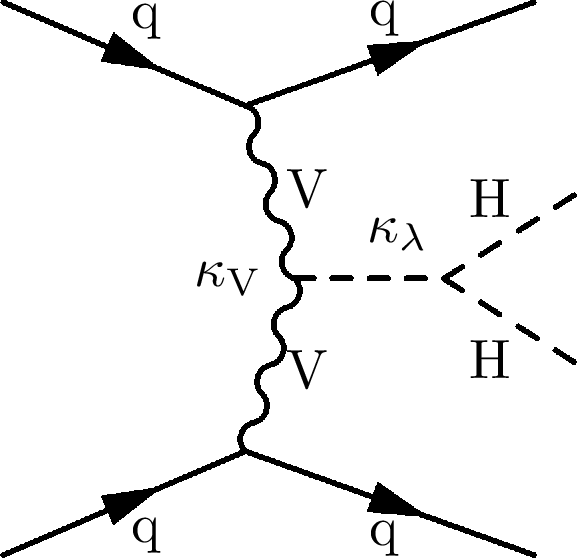
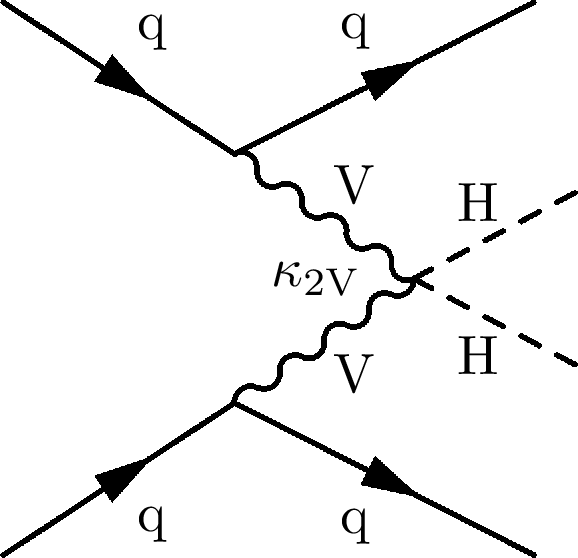
Figure 2:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via vector boson fusion in the standard model. The modifiers of the Higgs boson coupling with a vector boson, the Higgs boson trilinear self-coupling, and the coupling between two Higgs bosons and two vector bosons are shown as $ \kappa_{V} $, $ \kappa_{\lambda} $, and $ \kappa_{2\mathrm{V}} $, respectively. |
png pdf |
Figure 2-a:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via vector boson fusion in the standard model. The modifiers of the Higgs boson coupling with a vector boson, the Higgs boson trilinear self-coupling, and the coupling between two Higgs bosons and two vector bosons are shown as $ \kappa_{V} $, $ \kappa_{\lambda} $, and $ \kappa_{2\mathrm{V}} $, respectively. |
png pdf |
Figure 2-b:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via vector boson fusion in the standard model. The modifiers of the Higgs boson coupling with a vector boson, the Higgs boson trilinear self-coupling, and the coupling between two Higgs bosons and two vector bosons are shown as $ \kappa_{V} $, $ \kappa_{\lambda} $, and $ \kappa_{2\mathrm{V}} $, respectively. |
png pdf |
Figure 2-c:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via vector boson fusion in the standard model. The modifiers of the Higgs boson coupling with a vector boson, the Higgs boson trilinear self-coupling, and the coupling between two Higgs bosons and two vector bosons are shown as $ \kappa_{V} $, $ \kappa_{\lambda} $, and $ \kappa_{2\mathrm{V}} $, respectively. |
png pdf |
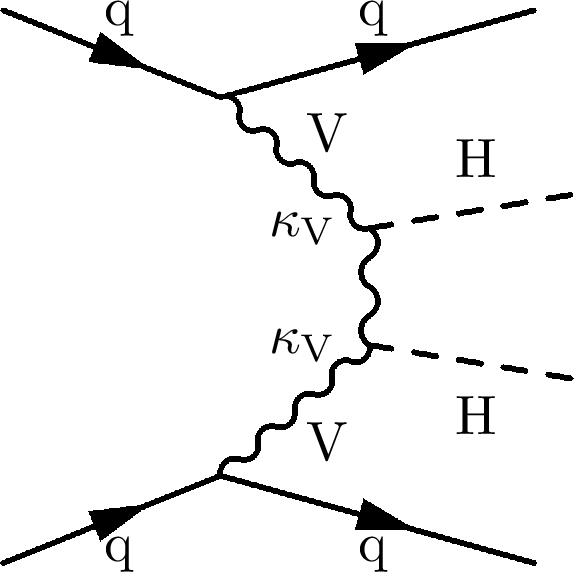
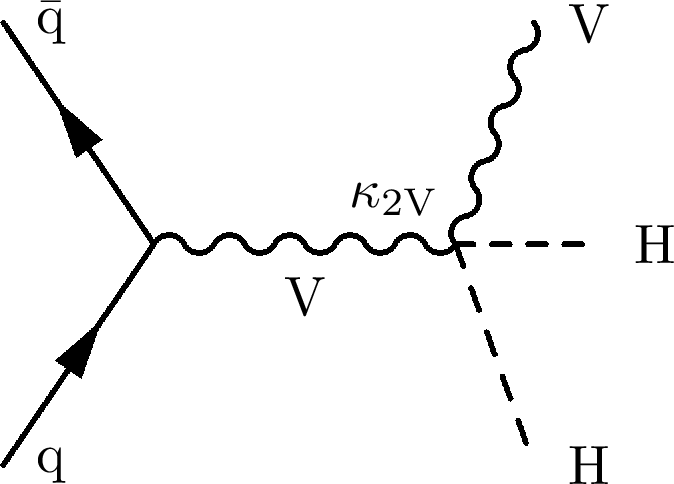
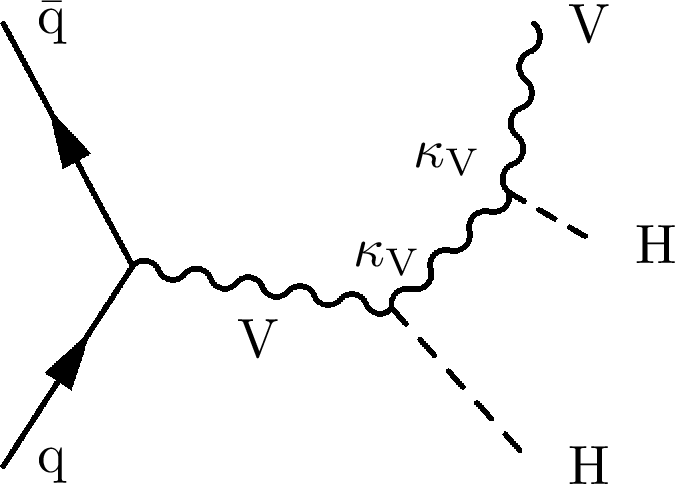
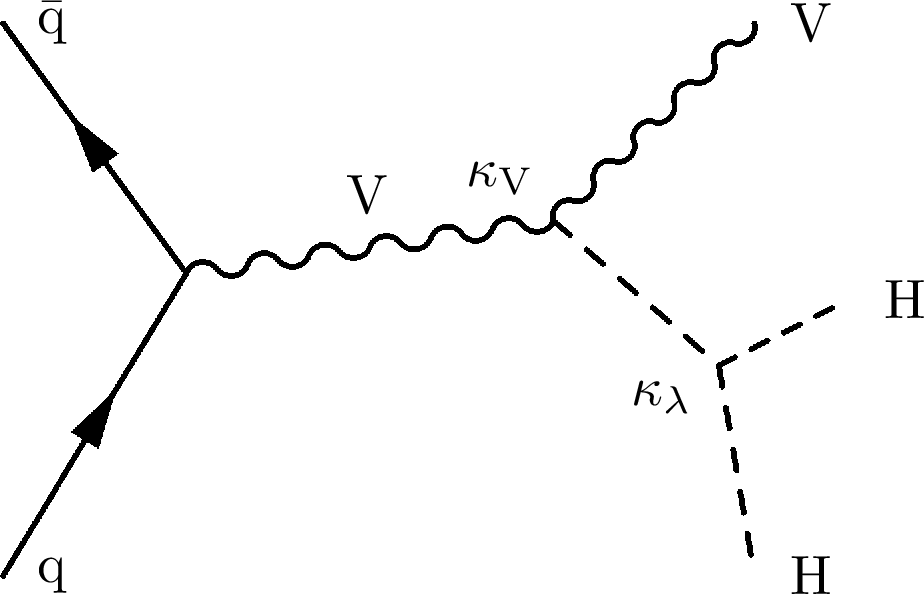
Figure 3:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via associated production with a vector boson in the standard model. The modifiers of the Higgs boson coupling with a vector boson, the Higgs boson trilinear self-coupling, and the coupling between two Higgs bosons and two vector bosons are shown as $ \kappa_{V} $, $ \kappa_{\lambda} $, and $ \kappa_{2\mathrm{V}} $ respectively. |
png pdf |
Figure 3-a:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via associated production with a vector boson in the standard model. The modifiers of the Higgs boson coupling with a vector boson, the Higgs boson trilinear self-coupling, and the coupling between two Higgs bosons and two vector bosons are shown as $ \kappa_{V} $, $ \kappa_{\lambda} $, and $ \kappa_{2\mathrm{V}} $ respectively. |
png pdf |
Figure 3-b:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via associated production with a vector boson in the standard model. The modifiers of the Higgs boson coupling with a vector boson, the Higgs boson trilinear self-coupling, and the coupling between two Higgs bosons and two vector bosons are shown as $ \kappa_{V} $, $ \kappa_{\lambda} $, and $ \kappa_{2\mathrm{V}} $ respectively. |
png pdf |
Figure 3-c:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via associated production with a vector boson in the standard model. The modifiers of the Higgs boson coupling with a vector boson, the Higgs boson trilinear self-coupling, and the coupling between two Higgs bosons and two vector bosons are shown as $ \kappa_{V} $, $ \kappa_{\lambda} $, and $ \kappa_{2\mathrm{V}} $ respectively. |
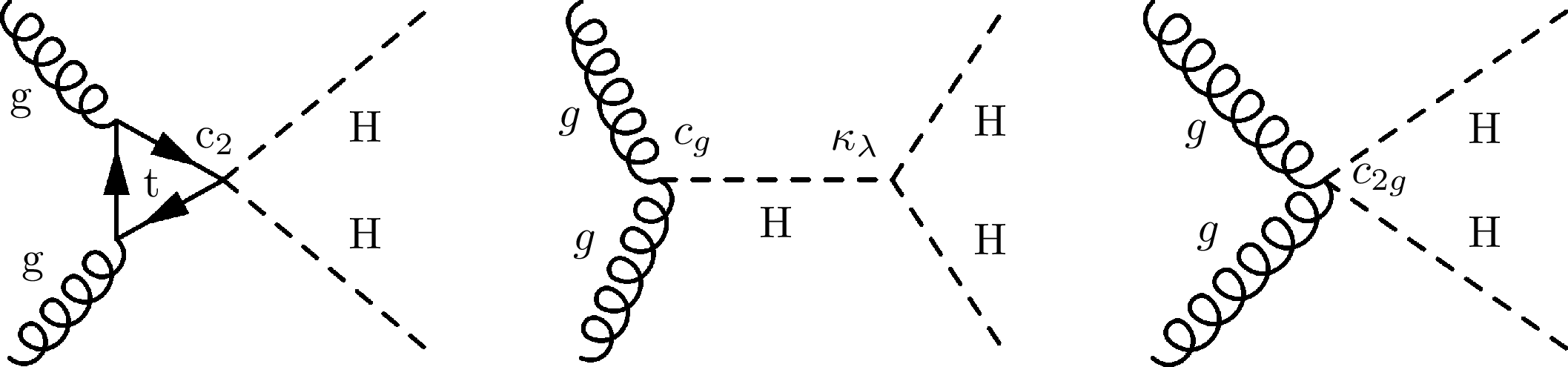
png pdf |
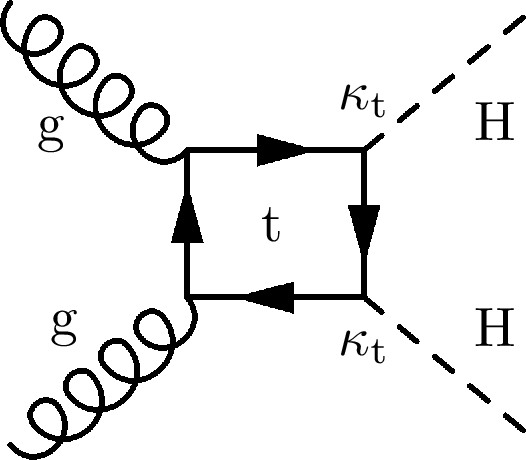
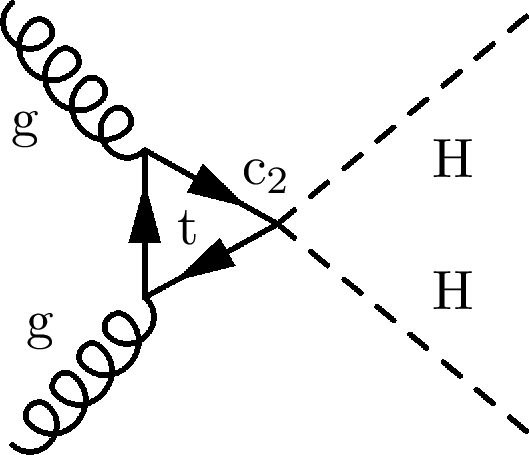
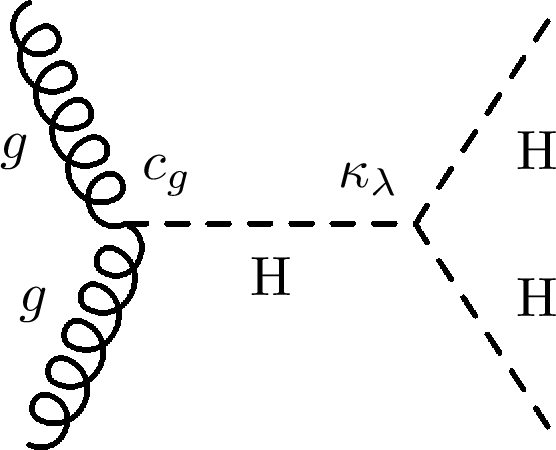
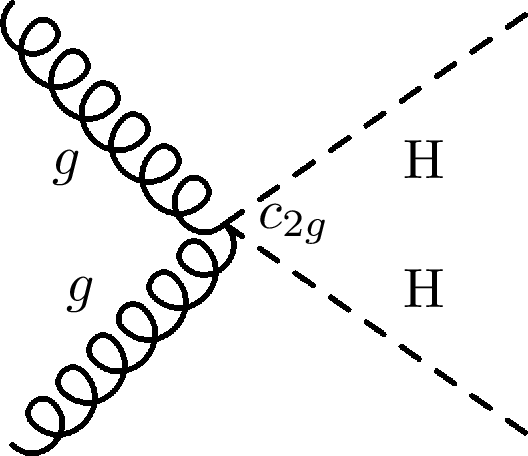
Figure 4:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via gluon-gluon fusion with anomalous Higgs boson couplings $ c_{2} $, $ c_{\mathrm{g}} $, and $ c_{2\mathrm{g}} $. The Higgs boson trilinear self-coupling modifier is shown as $ \kappa_{\lambda} $. |
png pdf |
Figure 4-a:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via gluon-gluon fusion with anomalous Higgs boson couplings $ c_{2} $, $ c_{\mathrm{g}} $, and $ c_{2\mathrm{g}} $. The Higgs boson trilinear self-coupling modifier is shown as $ \kappa_{\lambda} $. |
png pdf |
Figure 4-b:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via gluon-gluon fusion with anomalous Higgs boson couplings $ c_{2} $, $ c_{\mathrm{g}} $, and $ c_{2\mathrm{g}} $. The Higgs boson trilinear self-coupling modifier is shown as $ \kappa_{\lambda} $. |
png pdf |
Figure 4-c:
Leading-order Feynman diagrams of nonresonant Higgs boson pair production via gluon-gluon fusion with anomalous Higgs boson couplings $ c_{2} $, $ c_{\mathrm{g}} $, and $ c_{2\mathrm{g}} $. The Higgs boson trilinear self-coupling modifier is shown as $ \kappa_{\lambda} $. |
png pdf |
Figure 5:
The 95% CL upper limits on the inclusive signal strength $ r = \sigma_{\mathrm{H}\mathrm{H}} /\sigma_{\mathrm{H}\mathrm{H}} ^\text{SM} $ for each channel and their combination. The inner (green) band and the outer (yellow) band indicate the 68 and 95% CL intervals, respectively, under the background-only hypothesis. The $ \mathrm{b}\overline{\mathrm{b}}\mathrm{b}\overline{\mathrm{b}} $ and $ \mathrm{b}\overline{\mathrm{b}}\mathrm{W}\mathrm{W} $ contributions have been combined in order to simplify the presentation of results. |
png pdf |
Figure 6:
The 95% CL upper limits on the VBF signal strength $ r_{{\text{VBF}} {\mathrm{H}\mathrm{H}}} = \sigma_{{\text{VBF}} \mathrm{H}\mathrm{H}} /\sigma_{{\text{VBF}} \mathrm{H}\mathrm{H}} ^\text{SM} $ for each channel and their combination. The inner (green) band and the outer (yellow) band indicate the 68 and 95% CL intervals, respectively, under the background-only hypothesis. Not all of the eight channels are used to extract the combined VBF limit. The contributing channels are indicated in the figure. The $ \mathrm{b}\overline{\mathrm{b}}\mathrm{b}\overline{\mathrm{b}} $ and $ \mathrm{b}\overline{\mathrm{b}}\mathrm{W}\mathrm{W} $ contributions have been combined in order to simplify the presentation of results. |
png pdf |
Figure 7:
The 95% CL upper limits on the inclusive HH cross section as a function of $ \kappa_{\lambda} $ (left) and $ \kappa_{2\mathrm{V}} $ (right), respectively. All other couplings are set to the values predicted by the SM. The theoretical uncertainties in the HH ggF and VBF signal cross sections are not considered because here we directly constrain the measured cross section. The inner (green) band and the outer (yellow) band indicate the 68 and 95% CL intervals, respectively, under the background-only hypothesis. The star shows the limit at the SM value for $ \kappa_{\lambda} $ and $ \kappa_{2\mathrm{V}} $, respectively. |
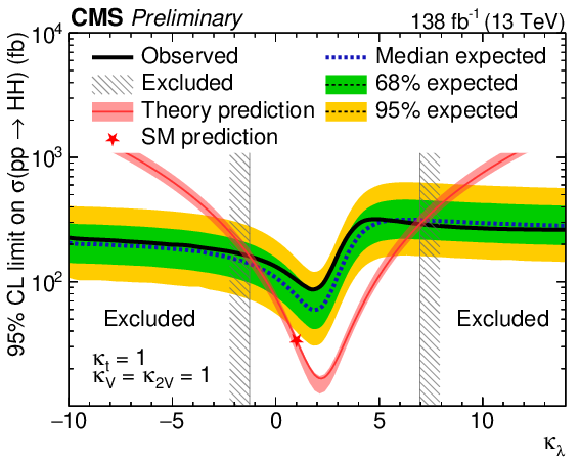
png pdf |
Figure 7-a:
The 95% CL upper limits on the inclusive HH cross section as a function of $ \kappa_{\lambda} $ (left) and $ \kappa_{2\mathrm{V}} $ (right), respectively. All other couplings are set to the values predicted by the SM. The theoretical uncertainties in the HH ggF and VBF signal cross sections are not considered because here we directly constrain the measured cross section. The inner (green) band and the outer (yellow) band indicate the 68 and 95% CL intervals, respectively, under the background-only hypothesis. The star shows the limit at the SM value for $ \kappa_{\lambda} $ and $ \kappa_{2\mathrm{V}} $, respectively. |
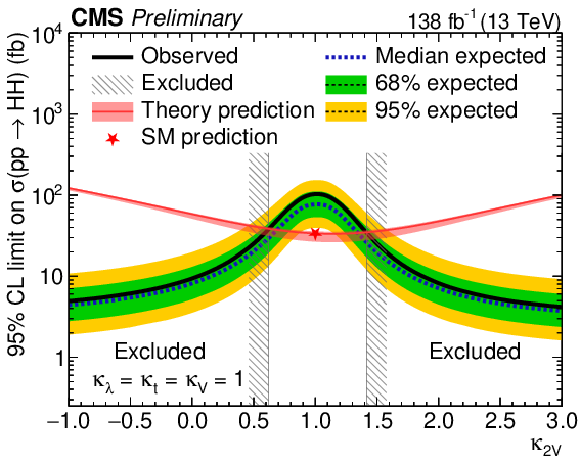
png pdf |
Figure 7-b:
The 95% CL upper limits on the inclusive HH cross section as a function of $ \kappa_{\lambda} $ (left) and $ \kappa_{2\mathrm{V}} $ (right), respectively. All other couplings are set to the values predicted by the SM. The theoretical uncertainties in the HH ggF and VBF signal cross sections are not considered because here we directly constrain the measured cross section. The inner (green) band and the outer (yellow) band indicate the 68 and 95% CL intervals, respectively, under the background-only hypothesis. The star shows the limit at the SM value for $ \kappa_{\lambda} $ and $ \kappa_{2\mathrm{V}} $, respectively. |
png pdf |
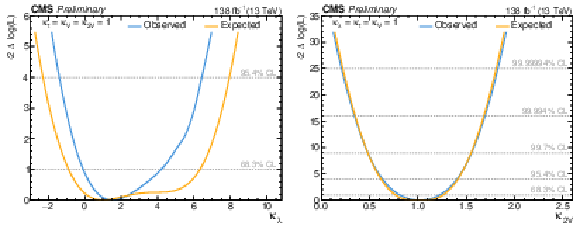
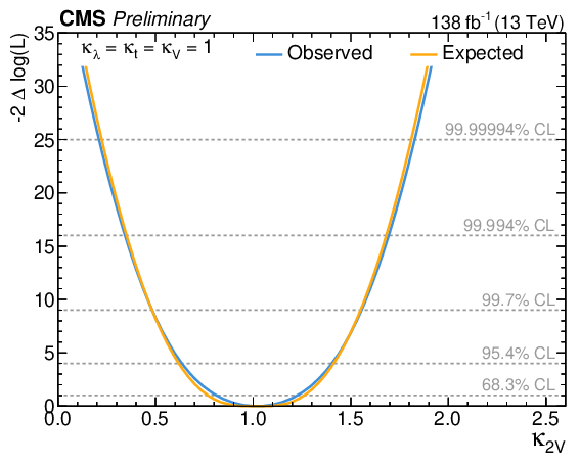
Figure 8:
The profile likelihood ratio test statistic $ -2\Delta\log(L) $ as a function of coupling modifiers $ \kappa_{\lambda} $ (left) and $ \kappa_{2\mathrm{V}} $ (right) for the combination of all channels. |
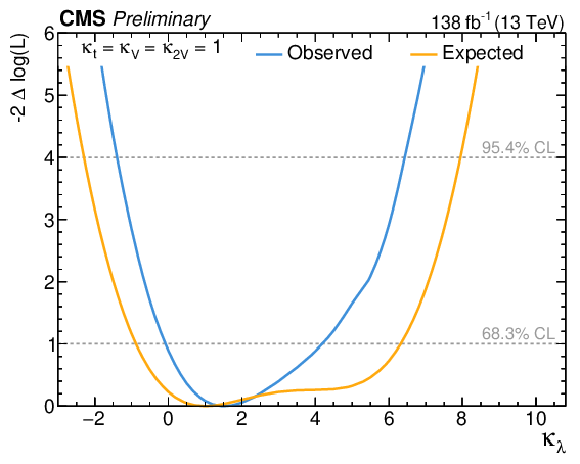
png pdf |
Figure 8-a:
The profile likelihood ratio test statistic $ -2\Delta\log(L) $ as a function of coupling modifiers $ \kappa_{\lambda} $ (left) and $ \kappa_{2\mathrm{V}} $ (right) for the combination of all channels. |
png pdf |
Figure 8-b:
The profile likelihood ratio test statistic $ -2\Delta\log(L) $ as a function of coupling modifiers $ \kappa_{\lambda} $ (left) and $ \kappa_{2\mathrm{V}} $ (right) for the combination of all channels. |
png pdf |
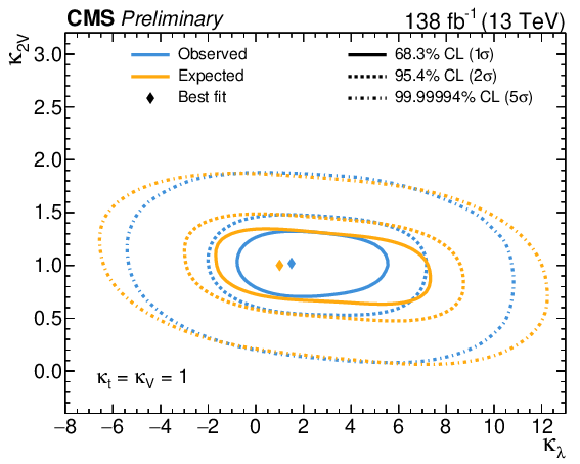
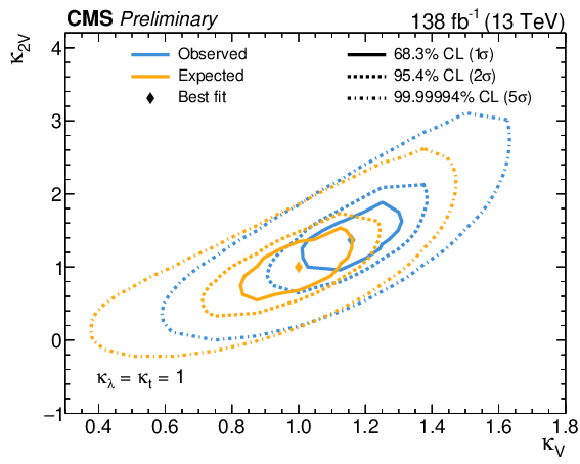
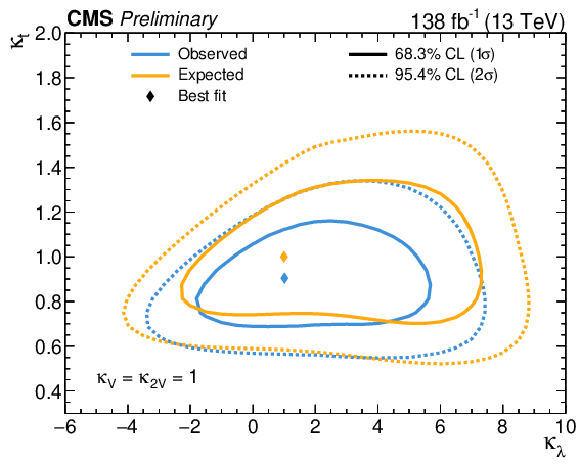
Figure 9:
Profile likelihood ratio test statistic $ -2\Delta\log(L) $ scans as a function of pairs of coupling modifiers ( $ \kappa_{\lambda} $, $ \kappa_{2\mathrm{V}} $) (top left), ($ \kappa_{V} $, $ \kappa_{2\mathrm{V}} $) (top right), and ( $ \kappa_{\lambda} $, $ \kappa_{\mathrm{t}} $) (bottom) for the combination of all channels when all the other parameters are fixed to their SM value. |
png pdf |
Figure 9-a:
Profile likelihood ratio test statistic $ -2\Delta\log(L) $ scans as a function of pairs of coupling modifiers ( $ \kappa_{\lambda} $, $ \kappa_{2\mathrm{V}} $) (top left), ($ \kappa_{V} $, $ \kappa_{2\mathrm{V}} $) (top right), and ( $ \kappa_{\lambda} $, $ \kappa_{\mathrm{t}} $) (bottom) for the combination of all channels when all the other parameters are fixed to their SM value. |
png pdf |
Figure 9-b:
Profile likelihood ratio test statistic $ -2\Delta\log(L) $ scans as a function of pairs of coupling modifiers ( $ \kappa_{\lambda} $, $ \kappa_{2\mathrm{V}} $) (top left), ($ \kappa_{V} $, $ \kappa_{2\mathrm{V}} $) (top right), and ( $ \kappa_{\lambda} $, $ \kappa_{\mathrm{t}} $) (bottom) for the combination of all channels when all the other parameters are fixed to their SM value. |
png pdf |
Figure 9-c:
Profile likelihood ratio test statistic $ -2\Delta\log(L) $ scans as a function of pairs of coupling modifiers ( $ \kappa_{\lambda} $, $ \kappa_{2\mathrm{V}} $) (top left), ($ \kappa_{V} $, $ \kappa_{2\mathrm{V}} $) (top right), and ( $ \kappa_{\lambda} $, $ \kappa_{\mathrm{t}} $) (bottom) for the combination of all channels when all the other parameters are fixed to their SM value. |
png pdf |
Figure 10:
Upper limits on the HH production cross section at 95% CL for the two sets of HEFT benchmarks. The theoretical uncertainties in the HH ggF signal cross section are not considered because we directly constrain the measured cross section. |
png pdf |
Figure 11:
On the left, upper limits on the HH cross section as a function of the $ c_{2} $ coupling modifier. The theoretical uncertainties in the HH ggF signal cross section are not considered because we directly constrain the measured cross section. On the right, the profile likelihood ratio test statistic $ -2\Delta\log(L) $ as a function of the $ c_{2} $ coupling modifier. |
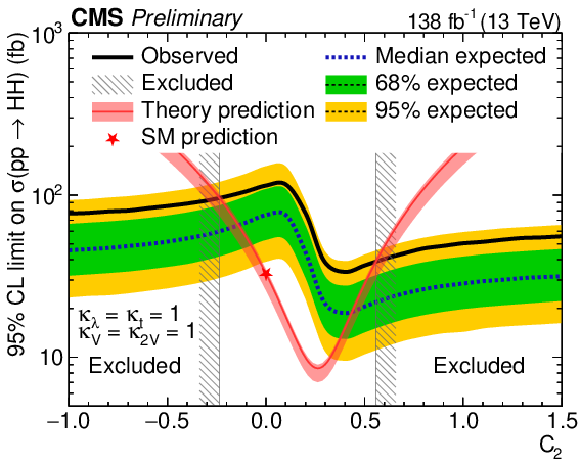
png pdf |
Figure 11-a:
On the left, upper limits on the HH cross section as a function of the $ c_{2} $ coupling modifier. The theoretical uncertainties in the HH ggF signal cross section are not considered because we directly constrain the measured cross section. On the right, the profile likelihood ratio test statistic $ -2\Delta\log(L) $ as a function of the $ c_{2} $ coupling modifier. |
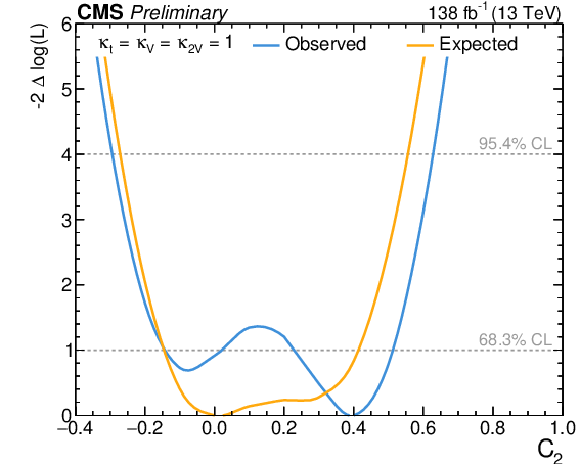
png pdf |
Figure 11-b:
On the left, upper limits on the HH cross section as a function of the $ c_{2} $ coupling modifier. The theoretical uncertainties in the HH ggF signal cross section are not considered because we directly constrain the measured cross section. On the right, the profile likelihood ratio test statistic $ -2\Delta\log(L) $ as a function of the $ c_{2} $ coupling modifier. |
png pdf |
Figure 12:
Expected upper limits on the HH signal strength from the combination of all the considered channels at different integrated luminosities (left), and under different assumptions on the systematic uncertainties for an integrated luminosity of 3000 fb$ ^{-1} $ (right). |
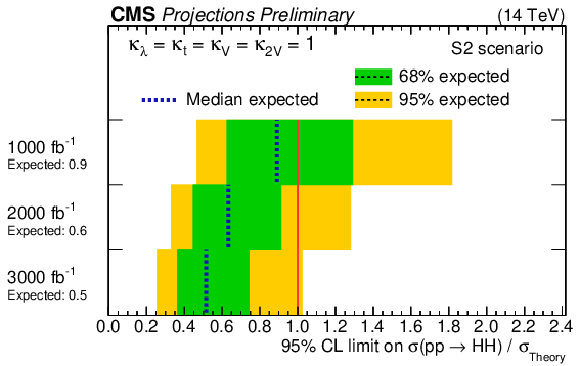
png pdf |
Figure 12-a:
Expected upper limits on the HH signal strength from the combination of all the considered channels at different integrated luminosities (left), and under different assumptions on the systematic uncertainties for an integrated luminosity of 3000 fb$ ^{-1} $ (right). |
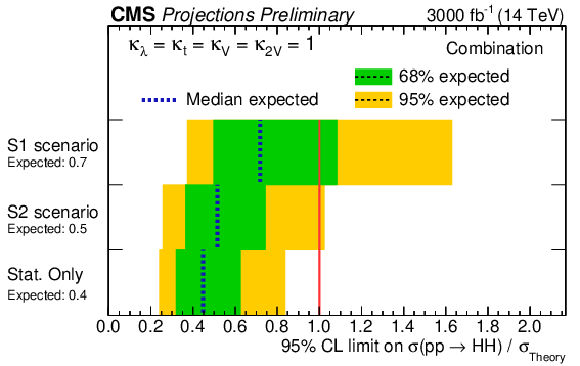
png pdf |
Figure 12-b:
Expected upper limits on the HH signal strength from the combination of all the considered channels at different integrated luminosities (left), and under different assumptions on the systematic uncertainties for an integrated luminosity of 3000 fb$ ^{-1} $ (right). |
png pdf |
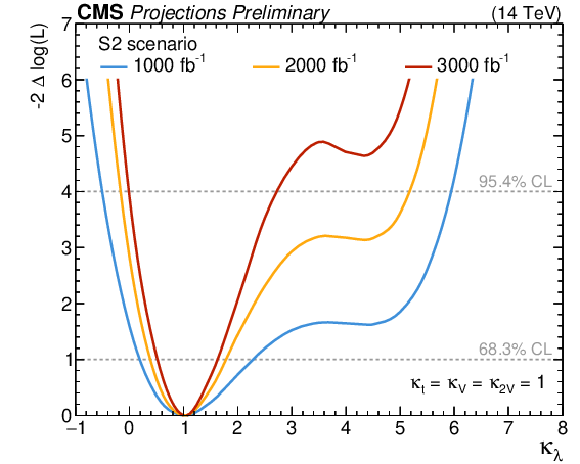
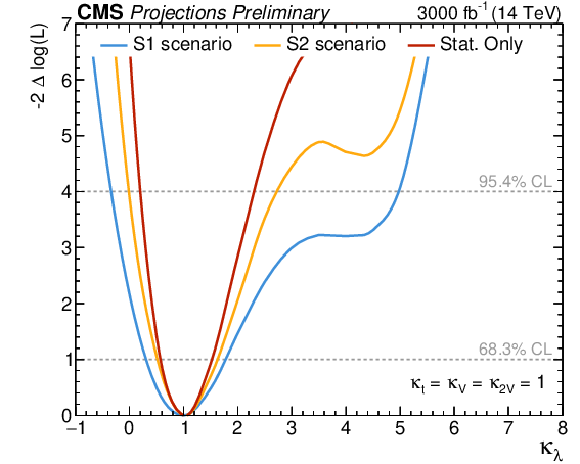
Figure 13:
Expected $ \kappa_{\lambda} $ likelihood scan from the combination of all the considered channels projected at different integrated luminosities (left), and under different assumptions on the systematic uncertainties for an integrated luminosity of 3000 fb$ ^{-1} $ (right). |
png pdf |
Figure 13-a:
Expected $ \kappa_{\lambda} $ likelihood scan from the combination of all the considered channels projected at different integrated luminosities (left), and under different assumptions on the systematic uncertainties for an integrated luminosity of 3000 fb$ ^{-1} $ (right). |
png pdf |
Figure 13-b:
Expected $ \kappa_{\lambda} $ likelihood scan from the combination of all the considered channels projected at different integrated luminosities (left), and under different assumptions on the systematic uncertainties for an integrated luminosity of 3000 fb$ ^{-1} $ (right). |
png pdf |
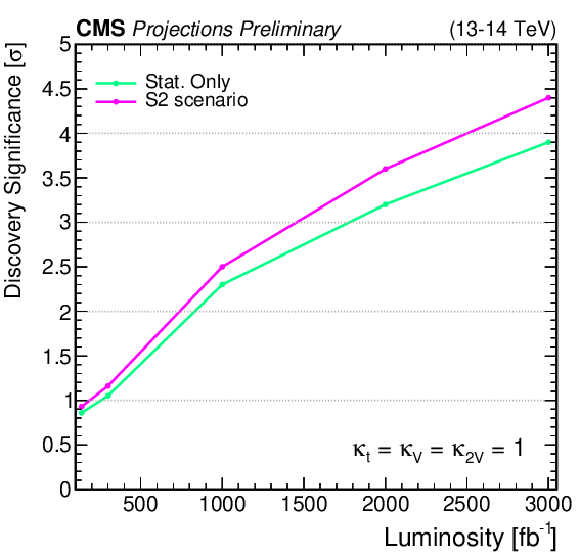
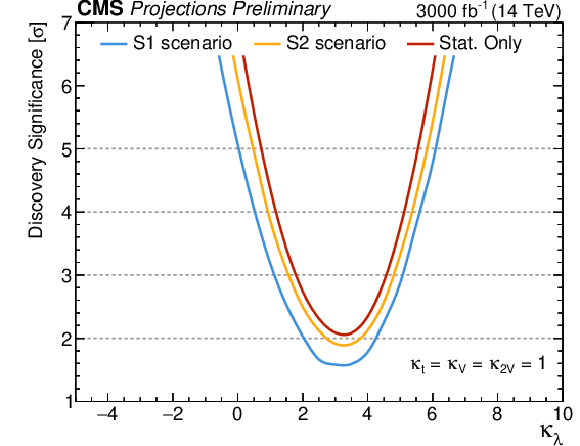
Figure 14:
Expected signal significance as a function of integrated luminosity for the nominal scenario of systematic uncertainties S2 and for the scenario with statistical uncertainties only (left). Expected signal significance as a function of $ \kappa_{\lambda} $ under different assumptions on the systematic uncertainties for an integrated luminosity of 3000 fb$ ^{-1} $ (right). |
png pdf |
Figure 14-a:
Expected signal significance as a function of integrated luminosity for the nominal scenario of systematic uncertainties S2 and for the scenario with statistical uncertainties only (left). Expected signal significance as a function of $ \kappa_{\lambda} $ under different assumptions on the systematic uncertainties for an integrated luminosity of 3000 fb$ ^{-1} $ (right). |
png pdf |
Figure 14-b:
Expected signal significance as a function of integrated luminosity for the nominal scenario of systematic uncertainties S2 and for the scenario with statistical uncertainties only (left). Expected signal significance as a function of $ \kappa_{\lambda} $ under different assumptions on the systematic uncertainties for an integrated luminosity of 3000 fb$ ^{-1} $ (right). |
| Tables | |
png pdf |
Table 1:
Values of the effective Lagrangian couplings for the Higgs Effective field theory benchmarks proposed in Ref. [33]. |
png pdf |
Table 2:
Values of the effective Lagrangian couplings for the Higgs effective field theory benchmarks proposed in Ref. [34]. |
png pdf |
Table 3:
Summary of results for the HH analyses included in this combination. The second column is the observed (expected) 95% CL upper limit on the inclusive signal strength $ r $. The third (fourth) column is the allowed 68% CL interval for the coupling modifier $ \kappa_{\lambda} $ ($ \kappa_{2\mathrm{V}} $). The last column indicates whether the analysis is included in the results using the HEFT parametrisation. |
png pdf |
Table 4:
Treatment of most important common systematic uncertainties in the S2 scenario. |
png pdf |
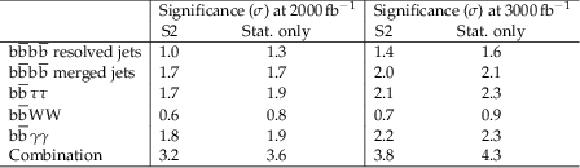
Table 5:
Expected significance for the HH signal projected to 2000 or 3000 fb$ ^{-1} $ under different assumptions of systematic uncertainties. |
| Summary |
| A combined search for nonresonant Higgs boson pair (HH) production is performed using the proton-proton collision data set produced by the LHC at $ \sqrt{s} = $ 13 TeV, and collected by the CMS detector from 2016 to 2018 (Run 2), which corresponds to an integrated luminosity of 138 fb$ ^{-1} $. Searches for HH production via gluon-gluon (ggF) and vector boson fusion (VBF) production, are carried out in the $ \mathrm{b}\overline{\mathrm{b}}\gamma\gamma $, $ \mathrm{b}\overline{\mathrm{b}}\tau\tau $, $ \mathrm{b}\overline{\mathrm{b}}\mathrm{b}\overline{\mathrm{b}} $, $ \mathrm{b}\overline{\mathrm{b}}\mathrm{W}\mathrm{W} $, and multilepton channels. Additionally, the gluon-gluon fusion Higgs boson pair production is also searched for in the $ \mathrm{b}\overline{\mathrm{b}}\mathrm{Z}\mathrm{Z} $ with ZZ decaying to four leptons, $ \mathrm{W}\mathrm{W}\gamma\gamma $, and $ \tau\tau\gamma\gamma $ final states, which have clean signatures but relatively small branching fractions. The associated production mechanism with a vector boson is searched for in the $ \mathrm{b}\overline{\mathrm{b}}\mathrm{b}\overline{\mathrm{b}} $ final state with the largest branching fraction. The analyses of these channels are combined to probe the Higgs boson trilinear self-coupling, the quartic coupling between two vector bosons and two Higgs bosons (VVHH) and to search for beyond the standard model physics scenarios in the Higgs effective field theory approach. The observed and expected upper limits at 95% confidence level (CL) on the cross section of gluon-gluon fusion Higgs boson pair production are found to be 3.5 and 2.5 times the standard model expectations. For the vector boson fusion production, the observed and expected upper limit at 95% CL are 79 and 91 times the standard model expectations. One-dimensional scans of coupling modifiers are performed. When all other parameters are assumed to be as expected from the SM, we (expect to) exclude HH production at 95% CL when the Higgs boson trilinear self-coupling modifier $ \kappa_{\lambda} $ is outside the range from $- $1.39 to 7.02 ($-$1.02 to 7.19). Equivalently, HH production is excluded when the VVHH coupling modifier $ \kappa_{2\mathrm{V}} $ is outside the range from 0.62 to 1.42 (0.69 to 1.35 expected). Two-dimensional measurements are also performed, including simultaneous measurements of $ \kappa_{\lambda} $ and $ \kappa_{2\mathrm{V}} $, $ \kappa_{\lambda} $ and the modifier of the Higgs boson coupling to the top quark ($ \kappa_{\mathrm{t}} $), and $ \kappa_{2\mathrm{V}} $ and the modifier of the Higgs boson coupling to vector bosons ($ \kappa_{\mathrm{V}} $). The results are in agreement with the standard model predictions. Under an effective field theory framework, the cross section of the nonresonant ggF HH pair production is parameterised as a function of anomalous couplings of the Higgs boson, involving the contact interactions between two Higgs and two top quarks, between two gluons and two Higgs bosons, and between two gluons and a Higgs boson. We perform searches for benchmark signals under different anomalous coupling scenarios and set upper limits on their cross sections at 95% CL. We (expect to) exclude HH production at 95% CL when the coupling of the contact interaction between two Higgs and two top quarks is outside the range between $-$0.28 to 0.59 ($-$0.17 to 0.47). These results present the most stringent limits and constraints obtained from the searches for nonresonant Higgs boson pair production using the LHC Run 2 data set collected by the CMS detector. Extrapolating our current results to the luminosity of HL-LHC it can be expected to see first evidence for Higgs boson pair production with $ \approx $2000 fb$ ^{-1} $ of data. |
| References | ||||
| 1 | CMS Collaboration | Observation of a new boson at a mass of 125 GeV with the CMS experiment at the LHC | PLB 716 (2012) 30 | CMS-HIG-12-028 1207.7235 |
| 2 | CMS Collaboration | Observation of a new boson with mass near 125 GeV in pp collisions at $ \sqrt{s} = $ 7 and 8 TeV | JHEP 06 (2013) 081 | CMS-HIG-12-036 1303.4571 |
| 3 | ATLAS Collaboration | Observation of a new particle in the search for the Standard Model Higgs boson with the ATLAS detector at the LHC | PLB 716 (2012) 1 | 1207.7214 |
| 4 | CMS Collaboration | Combined measurements of Higgs boson couplings in proton--proton collisions at $ \sqrt{s}= $ 13 TeV | EPJC 79 (2019) 421 | CMS-HIG-17-031 1809.10733 |
| 5 | ATLAS Collaboration | Combined measurements of Higgs boson production and decay using up to 80 fb$ ^{-1} $ of proton-proton collision data at $ \sqrt{s}= $ 13 TeV collected with the ATLAS experiment | PRD 101 (2020) 012002 | 1909.02845 |
| 6 | A. Papaefstathiou and G. White | The electro-weak phase transition at colliders: confronting theoretical uncertainties and complementary channels | JHEP 05 (2021) 099 | 2010.00597 |
| 7 | A. Mazumdar and G. White | Review of cosmic phase transitions: their significance and experimental signatures | Rept. Prog. Phys. 82 (2019) 076901 | 1811.01948 |
| 8 | V. Brigljevic et al. | $ \mathrm{H}\mathrm{H}\mathrm{H} $ Whitepaper | 2407.03015 | |
| 9 | A. Dainese et al. | Report from Working Group 2: Higgs physics at the HL-LHC and HE-LHC | CERN Yellow Rep. Monogr. 7 (2019) 221 | 1902.00134 |
| 10 | T. Plehn and M. Rauch | The quartic higgs coupling at hadron colliders | PRD 72 (2005) 053008 | hep-ph/0507321 |
| 11 | S. Borowka et al. | Higgs boson pair production in gluon fusion at next-to-leading order with full top-quark mass dependence | PRL 117 (2016) 012001 | 1604.06447 |
| 12 | J. Baglio et al. | Gluon fusion into Higgs pairs at NLO QCD and the top mass scheme | EPJC 79 (2019) 459 | 1811.05692 |
| 13 | F. A. Dreyer and A. Karlberg | Vector-boson fusion Higgs pair production at N$ ^3 $LO | PRD 98 (2018) 114016 | 1811.07906 |
| 14 | L. Alasfar et al. | Effective field theory descriptions of Higgs boson pair production | SciPost Phys. Comm. Rep. 2, 2024 | 2304.01968 |
| 15 | ATLAS Collaboration | Combination of searches for Higgs boson pair production in pp collisions at $ \sqrt{s}= $ 13 TeV with the ATLAS detector | PRL 133 (2024) 101801 | 2406.09971 |
| 16 | CMS Collaboration | A portrait of the Higgs boson by the CMS experiment ten years after the discovery | Nature 607 (2022) 60 | CMS-HIG-22-001 2207.00043 |
| 17 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | |
| 18 | CMS Collaboration | Development of the CMS detector for the CERN LHC Run 3 | JINST 19 (2024) P05064 | |
| 19 | CMS Collaboration | Performance of the CMS Level-1 trigger in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JINST 15 (2020) P10017 | CMS-TRG-17-001 2006.10165 |
| 20 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 21 | CMS Collaboration | Performance of the CMS high-level trigger during LHC Run 2 | Submitted to JINST, 2024 | CMS-TRG-19-001 2410.17038 |
| 22 | CMS Collaboration | Electron and photon reconstruction and identification with the CMS experiment at the CERN LHC | JINST 16 (2021) P05014 | CMS-EGM-17-001 2012.06888 |
| 23 | CMS Collaboration | Performance of the CMS muon detector and muon reconstruction with proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JINST 13 (2018) P06015 | CMS-MUO-16-001 1804.04528 |
| 24 | CMS Collaboration | Description and performance of track and primary-vertex reconstruction with the CMS tracker | JINST 9 (2014) P10009 | CMS-TRK-11-001 1405.6569 |
| 25 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 26 | CMS Collaboration | Technical proposal for the Phase-II upgrade of the Compact Muon Solenoid | CMS Technical Proposal CERN-LHCC-2015-010, CMS-TDR-15-02, 2015 CDS |
|
| 27 | CMS Collaboration | Performance of missing transverse momentum reconstruction in proton-proton collisions at $ \sqrt{s} = $ 13 TeV using the CMS detector | JINST 14 (2019) P07004 | CMS-JME-17-001 1903.06078 |
| 28 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_{\mathrm{T}} $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 29 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 30 | G. Heinrich et al. | Probing the trilinear Higgs boson coupling in di-Higgs production at NLO QCD including parton shower effects | JHEP 06 (2019) 066 | 1903.08137 |
| 31 | D. de Florian et al. | Anomalous couplings in Higgs-boson pair production at approximate NNLO QCD | JHEP 09 (2021) 161 | 2106.14050 |
| 32 | A. Carvalho et al. | On the reinterpretation of non-resonant searches for Higgs boson pairs | JHEP 02 (2021) 049 | 1710.08261 |
| 33 | A. Carvalho et al. | Higgs pair production: Choosing benchmarks with cluster analysis | JHEP 04 (2016) 126 | 1507.02245 |
| 34 | M. Capozi and G. Heinrich | Exploring anomalous couplings in Higgs boson pair production through shape analysis | JHEP 03 (2020) 091 | 1908.08923 |
| 35 | P. Nason | A new method for combining NLO QCD with shower Monte Carlo algorithms | JHEP 11 (2004) 040 | hep-ph/0409146 |
| 36 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with parton shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 37 | S. Alioli, P. Nason, C. Oleari, and E. Re | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX | JHEP 06 (2010) 043 | 1002.2581 |
| 38 | S. Amoroso et al. | Les Houches 2019: Physics at TeV Colliders: Standard Model Working Group Report | in 11th Les Houches Workshop on Physics at \TeV Colliders: PhysTeV Les Houches, 2020 | 2003.01700 |
| 39 | G. Heinrich, J. Lang, and L. Scyboz | SMEFT predictions for $ gg \to hh $ at full NLO QCD and truncation uncertainties | JHEP 08 (2022) 079 | 2204.13045 |
| 40 | E. Bagnaschi, G. Degrassi, and R. Gröber | Higgs boson pair production at NLO in the POWHEG approach and the top quark mass uncertainties | EPJC 83 (2023) 1054 | 2309.10525 |
| 41 | F. A. Dreyer and A. Karlberg | Fully differential Vector-Boson Fusion Higgs Pair Production at Next-to-Next-to-Leading Order | PRD 99 (2019) 074028 | 1811.07918 |
| 42 | J. Baglio et al. | The measurement of the Higgs self-coupling at the LHC: theoretical status | JHEP 04 (2013) 151 | 1212.5581 |
| 43 | T. Sjöstrand, S. Mrenna, and P. Z. Skands | A brief introduction to PYTHIA 8.1 | Comput. Phys. Commun. 178 (2008) 852 | 0710.3820 |
| 44 | CMS Collaboration | Search for nonresonant Higgs boson pair production in final states with two bottom quarks and two photons in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JHEP 03 (2021) 257 | CMS-HIG-19-018 2011.12373 |
| 45 | CMS Collaboration | Search for Higgs boson pair production in the $ \mathrm{b}\mathrm{b}\tau\tau $ final state in proton-proton collisions at $ \sqrt{s}= $ 8 TeV | PRD 96 (2017) 072004 | CMS-HIG-15-013 1707.00350 |
| 46 | CMS Collaboration | Search for Higgs boson pair production in the four b quark final state in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | PRL 129 (2022) 081802 | CMS-HIG-20-005 2202.09617 |
| 47 | CMS Collaboration | Search for nonresonant pair production of highly energetic Higgs bosons decaying to bottom quarks | PRL 131 (2023) 041803 | 2205.06667 |
| 48 | CMS Collaboration | Search for Higgs boson pair production with one associated vector boson in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JHEP 10 (2024) 061 | CMS-HIG-22-006 2404.08462 |
| 49 | CMS Collaboration | Search for Higgs boson pair production in the $ \textrm{b}\overline{\textrm{b}}{\textrm{W}}^{+}{\textrm{W}}^{-} $ decay mode in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JHEP 07 (2024) 293 | CMS-HIG-21-005 2403.09430 |
| 50 | CMS Collaboration | Search for highly energetic double Higgs boson production in the two bottom quark and two vector boson all-hadronic final state | CMS Physics Analysis Summary, 2024 CMS-PAS-HIG-23-012 |
CMS-PAS-HIG-23-012 |
| 51 | CMS Collaboration | Search for Higgs boson pairs decaying to $ \mathrm{W}\mathrm{W}^\ast\mathrm{W}\mathrm{W}^\ast $, $ \mathrm{W}\mathrm{W}^\ast\tau\tau $, and $ \tau\tau\tau\tau $ in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JHEP 07 (2023) 095 | CMS-HIG-21-002 2206.10268 |
| 52 | CMS Collaboration | Search for nonresonant Higgs boson pair production in the $ \mathrm{W}\mathrm{W}\gamma\gamma $ channel in pp collisions at $ \sqrt{s} = $ 13 TeV | CMS Physics Analysis Summary, 2022 CMS-PAS-HIG-21-014 |
CMS-PAS-HIG-21-014 |
| 53 | CMS Collaboration | Search for nonresonant Higgs boson pair production in the four leptons plus twob jets final state in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JHEP 06 (2023) 130 | CMS-HIG-20-004 2206.10657 |
| 54 | CMS Collaboration | Search for the nonresonant and resonant production of a Higgs boson in association with an additional scalar boson in the $ \gamma\gamma\tau\tau $ final state | CMS Physics Analysis Summary, 2024 CMS-PAS-HIG-22-012 |
CMS-PAS-HIG-22-012 |
| 55 | A. J. Larkoski, S. Marzani, G. Soyez, and J. Thaler | Soft drop | JHEP 05 (2014) 146 | 1402.2657 |
| 56 | I. Henrion et al. | Neural message passing for jet physics | in Deep Learning for Physical Sciences Workshop at 31st Conf. on Neural Information Processing Systems. Long Beach, CA, 2017 | |
| 57 | H. Qu and L. Gouskos | Jet tagging via particle clouds | PRD 101 (2020) 056019 | 1902.08570 |
| 58 | E. A. Moreno et al. | JEDI-net: a jet identification algorithm based on interaction networks | EPJC 80 (2020) 58 | 1908.05318 |
| 59 | E. A. Moreno et al. | Interaction networks for the identification of boosted $ \mathrm{H}\to\mathrm{b}\overline{\mathrm{b}} $ decays | PRD 102 (2020) 012010 | 1909.12285 |
| 60 | CMS Collaboration | Performance of heavy-flavour jet identification in boosted topologies in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | CMS Physics Analysis Summary, 2023 CMS-PAS-BTV-22-001 |
CMS-PAS-BTV-22-001 |
| 61 | CMS Collaboration | Mass regression of highly-boosted jets using graph neural networks | CMS Detector Performance Note CMS-DP-2021-017, 2021 CDS |
|
| 62 | CMS Collaboration | Search for a massive resonance decaying to a pair of Higgs bosons in the four b quark final state in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | PLB 781 (2018) 244 | 1710.04960 |
| 63 | CMS Collaboration | Search for production of Higgs boson pairs in the four b quark final state using large-area jets in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JHEP 01 (2019) 040 | 1808.01473 |
| 64 | CMS Collaboration | Inclusive search for highly boosted Higgs bosons decaying to bottom quark-antiquark pairs in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JHEP 12 (2020) 85 | CMS-HIG-19-003 2006.13251 |
| 65 | CMS Collaboration | Identification of heavy, energetic, hadronically decaying particles using machine-learning techniques | JINST 15 (2020) P06005 | CMS-JME-18-002 2004.08262 |
| 66 | CMS Collaboration | Identification of hadronic tau lepton decays using a deep neural network | JINST 17 (2022) P07023 | CMS-TAU-20-001 2201.08458 |
| 67 | L. Bianchini et al. | Reconstruction of the higgs mass in events with higgs bosons decaying into a pair of leptons using matrix element techniques | Nucl. Instr. Meth. Phys. Res. 862 (2017) 54 | 1603.05910 |
| 68 | E. Bols et al. | Jet flavour classification using DeepJet | JINST 15 (2020) P12012 | 2008.10519 |
| 69 | CMS Collaboration | Evidence for associated production of a Higgs boson with a top quark pair in final states with electrons, muons, and hadronically decaying $ \tau $ leptons at $ \sqrt{s} = $ 13 TeV | JHEP 08 (2018) 066 | CMS-HIG-17-018 1803.05485 |
| 70 | H. Qu, C. Li, and S. Qian | Particle transformer for jet tagging | in Proceedings of the 39th International Conference on Machine Learning, K. Chaudhuri et al., eds., volume 162, 2022 link |
2202.03772 |
| 71 | CMS Collaboration | Performance of heavy-flavour jet identification in boosted topologies in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | CMS Physics Analysis Summary, 2023 CMS-PAS-BTV-22-001 |
CMS-PAS-BTV-22-001 |
| 72 | CMS Collaboration | Lund plane reweighting for jet substructure correction | CMS Detector Performance Note CMS-DP-2023-046, 2023 CDS |
|
| 73 | F. A. Dreyer, G. P. Salam, and G. Soyez | The Lund jet plane | JHEP 12 (2018) 064 | 1807.04758 |
| 74 | CMS Collaboration | Measurement of the Higgs boson production rate in association with top quarks in final states with electrons, muons, and hadronically decaying tau leptons at $ \sqrt{s} = $ 13 TeV | EPJC 81 (2021) 378 | CMS-HIG-19-008 2011.03652 |
| 75 | S. Manzoni et al. | Taming a leading theoretical uncertainty in $ {\mathrm{H}\mathrm{H}} $ measurements via accurate simulations for $ \mathrm{b}\overline{\mathrm{b}}\mathrm{H} $ production | JHEP 09 (2023) 179 | 2307.09992 |
| 76 | J. Baglio et al. | $ gg\to HH $: Combined uncertainties | PRD 103 (2021) 056002 | 2008.11626 |
| 77 | L.-S. Ling et al. | NNLO QCD corrections to Higgs pair production via vector boson fusion at hadron colliders | PRD 89 (2014) 073001 | 1401.7754 |
| 78 | D. de Florian, I. Fabre, and J. Mazzitelli | Higgs boson pair production at NNLO in QCD including dimension 6 operators | JHEP 10 (2017) 215 | 1704.05700 |
| 79 | CMS Collaboration | Precision luminosity measurement in proton-proton collisions at $ \sqrt{s} = $ 13 TeV in 2015 and 2016 at CMS | EPJC 81 (2021) 800 | CMS-LUM-17-003 2104.01927 |
| 80 | CMS Collaboration | CMS luminosity measurement for the 2017 data-taking period at $ \sqrt{s} = $ 13 TeV | CMS Physics Analysis Summary, 2018 CMS-PAS-LUM-17-004 |
CMS-PAS-LUM-17-004 |
| 81 | CMS Collaboration | CMS luminosity measurement for the 2018 data-taking period at $ \sqrt{s} = $ 13 TeV | CMS Physics Analysis Summary, 2019 CMS-PAS-LUM-18-002 |
CMS-PAS-LUM-18-002 |
| 82 | R. Barlow and C. Beeston | Fitting using finite Monte Carlo samples | Comput. Phys. Commun. 77 (1993) 219 | |
| 83 | ATLAS and CMS Collaborations, and LHC Higgs Combination Group | Procedure for the LHC Higgs boson search combination in Summer 2011 | Technical Report CMS-NOTE-2011-005, ATL-PHYS-PUB-2011-11, 2011 | |
| 84 | G. Cowan, K. Cranmer, E. Gross, and O. Vitells | Asymptotic formulae for likelihood-based tests of new physics | EPJC 71 (2011) 1554 | 1007.1727 |
| 85 | A. L. Read | Presentation of search results: the $ \text{CL}_\text{s} $ technique | JPG 28 (2002) 2693 | |
| 86 | T. Junk | Confidence level computation for combining searches with small statistics | NIM A 434 (1999) 435 | hep-ex/9902006 |
| 87 | CMS Collaboration | The CMS statistical analysis and combination tool: Combine | Comput. Softw. Big Sci. 8 (2024) 19 | CMS-CAT-23-001 2404.06614 |
| 88 | W. Verkerke and D. P. Kirkby | The RooFit toolkit for data modeling | physics/0306116 | |
| 89 | L. Moneta et al. | The RooStats Project | PoS ACAT 057, 2010 | 1009.1003 |
| 90 | I. Zurbano Fernandez et al. | High-Luminosity Large Hadron Collider (HL-LHC): Technical design report | CERN Yellow Rep. Monogr. 10 (2020) | |
| 91 | CMS Collaboration | The Phase-2 upgrade of the CMS level-1 trigger | CMS Technical Design Report CERN-LHCC-2020-004, CMS-TDR-021, 2020 CDS |
|
| 92 | CMS Collaboration | The Phase-2 upgrade of the CMS data acquisition and high level trigger | CMS Technical Design Report CERN-LHCC-2021-007, CMS-TDR-022, 2021 CDS |
|
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|