

Compact Muon Solenoid
LHC, CERN
| CMS-PAS-HIG-22-012 | ||
| Search for the nonresonant and resonant production of a Higgs boson in association with an additional scalar boson in the γγττγγττ final state | ||
| CMS Collaboration | ||
| 26 March 2024 | ||
| Abstract: Proton-proton interactions resulting in final states with two photons and two tau leptons are studied in a search for the production of two scalar bosons. The search considers both nonresonant production of a Higgs boson pair, HH, and resonant production via a new boson X which decays either to HH or to H and a new scalar Y. The analysis uses 138 fb−1 of data collected at a center-of-mass energy of 13 TeV with the CMS detector at the LHC from 2016 to 2018. An observed (expected) upper limit at the 95% confidence level (CL) on the HH production cross section is found to be 930 (740) fb, corresponding to 33 (26) times the standard model prediction. The observed (expected) constraint on the Higgs boson self-coupling is −13(−11)<κλ< 18 (16) at the 95% CL. Observed (expected) upper limits at the 95% CL for the X→HH cross section are found to be within 140 to 2200 (200 to 1700) fb depending on mX. In the X→YH scenario, the most significant excess is found for mX= 525 GeV and mY= 115 GeV in the Y→γγ decay channel and has a local (global) significance of 3.4 (0.1) standard deviations. In the Y→ττ decay channel, an excess at mX= 320 GeV and mY= 60 GeV is found with a local (global) significance of 2.6 (2.2) standard deviations. | ||
| Links: CDS record (PDF) ; CADI line (restricted) ; | ||
| Figures & Tables | Summary | Additional Figures | References | CMS Publications |
|---|
| Figures | |

png pdf |
Figure 1:
Leading order Feynman diagrams of the nonresonant HH production via ggF. The two diagrams in the upper row correspond to SM processes, involving the top Yukawa coupling yt and the trilinear Higgs boson self-coupling λHHH. The diagrams in the lower row correspond to BSM processes involving contact interactions introduced in the effective field theory, namely c2, c2g and cg. |

png pdf |
Figure 2:
Feynman diagram of the resonant production of a pair of SM Higgs bosons (X→HH) or a SM Higgs boson and a new scalar particle (X→YH). |

png pdf |
Figure 3:
Distribution of the BDT scores used for the nonresonant analysis event categorization from data (black points) and predictions from MC simulation (coloured histograms). The ``H'' process includes ggH, VBF, VH, and t¯tH. The background histograms are stacked, while the signal distribution is shown separately. The normalization of the signal distribution is set to 25 times the SM prediction and the background MC simulation is normalized to data. The ratio of the data to the sum of the background predictions is shown in the lower panel. Statistical MC uncertainties for the background are represented by the grey-shaded bands. The grey dotted lines represent the boundaries that define the analysis categories. |

png pdf |
Figure 4:
Transformed output of the pNN used in the X(2)→HH search, evaluated at mX= 260 GeV (left) and mX= 800 GeV (right). The filled histograms represent the background simulation, and the data are shown by the black points. The ``H'' process includes ggH, VBF, VH, and t¯tH. The targeted signal distributions for which the pNN is evaluated are shown by the black unfilled histograms. The background MC simulation is normalized to data and the signal is normalized to an arbitrary cross section for representation purposes. The ratio of the data to the sum of the background predictions is shown in the lower panel. Statistical MC uncertainties for the background are represented by the grey-shaded bands. |

png pdf |
Figure 4-a:
Transformed output of the pNN used in the X(2)→HH search, evaluated at mX= 260 GeV (left) and mX= 800 GeV (right). The filled histograms represent the background simulation, and the data are shown by the black points. The ``H'' process includes ggH, VBF, VH, and t¯tH. The targeted signal distributions for which the pNN is evaluated are shown by the black unfilled histograms. The background MC simulation is normalized to data and the signal is normalized to an arbitrary cross section for representation purposes. The ratio of the data to the sum of the background predictions is shown in the lower panel. Statistical MC uncertainties for the background are represented by the grey-shaded bands. |

png pdf |
Figure 4-b:
Transformed output of the pNN used in the X(2)→HH search, evaluated at mX= 260 GeV (left) and mX= 800 GeV (right). The filled histograms represent the background simulation, and the data are shown by the black points. The ``H'' process includes ggH, VBF, VH, and t¯tH. The targeted signal distributions for which the pNN is evaluated are shown by the black unfilled histograms. The background MC simulation is normalized to data and the signal is normalized to an arbitrary cross section for representation purposes. The ratio of the data to the sum of the background predictions is shown in the lower panel. Statistical MC uncertainties for the background are represented by the grey-shaded bands. |

png pdf |
Figure 5:
Transformed output of the pNNs used in the X(0)→HH (top left), X(2)→HH (top right), X→Y(ττ)H(γγ) (middle), low-mass X→Y(γγ)H(ττ) (bottom left) and high-mass X→Y(γγ)H(ττ) (bottom right) searches. The pNNs are evaluated at the mass points where the largest excess with respect to the background-only hypothesis is observed. If the MC simulation at this mass point is not available, then the sample produced at a mass point closest to the excess is shown. The filled histograms represent the background simulation, and the data are shown by the black points. The ``H'' process includes ggH, VBF, VH, and t¯tH. The targeted signal distributions for which the pNN is evaluated are shown by the black unfilled histograms. The background MC simulation is normalized to data and the signal is normalized to an arbitrary cross section for representation purposes. The ratio of the data to the sum of the background predictions is shown in the lower panel. Statistical MC uncertainties for the background are represented by the grey-shaded bands. |

png pdf |
Figure 5-a:
Transformed output of the pNNs used in the X(0)→HH (top left), X(2)→HH (top right), X→Y(ττ)H(γγ) (middle), low-mass X→Y(γγ)H(ττ) (bottom left) and high-mass X→Y(γγ)H(ττ) (bottom right) searches. The pNNs are evaluated at the mass points where the largest excess with respect to the background-only hypothesis is observed. If the MC simulation at this mass point is not available, then the sample produced at a mass point closest to the excess is shown. The filled histograms represent the background simulation, and the data are shown by the black points. The ``H'' process includes ggH, VBF, VH, and t¯tH. The targeted signal distributions for which the pNN is evaluated are shown by the black unfilled histograms. The background MC simulation is normalized to data and the signal is normalized to an arbitrary cross section for representation purposes. The ratio of the data to the sum of the background predictions is shown in the lower panel. Statistical MC uncertainties for the background are represented by the grey-shaded bands. |
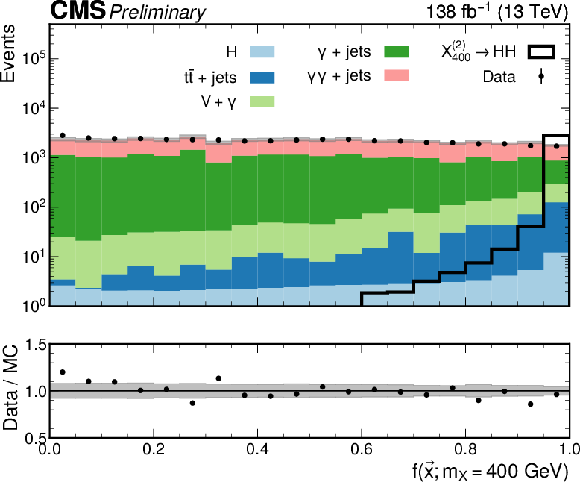
png pdf |
Figure 5-b:
Transformed output of the pNNs used in the X(0)→HH (top left), X(2)→HH (top right), X→Y(ττ)H(γγ) (middle), low-mass X→Y(γγ)H(ττ) (bottom left) and high-mass X→Y(γγ)H(ττ) (bottom right) searches. The pNNs are evaluated at the mass points where the largest excess with respect to the background-only hypothesis is observed. If the MC simulation at this mass point is not available, then the sample produced at a mass point closest to the excess is shown. The filled histograms represent the background simulation, and the data are shown by the black points. The ``H'' process includes ggH, VBF, VH, and t¯tH. The targeted signal distributions for which the pNN is evaluated are shown by the black unfilled histograms. The background MC simulation is normalized to data and the signal is normalized to an arbitrary cross section for representation purposes. The ratio of the data to the sum of the background predictions is shown in the lower panel. Statistical MC uncertainties for the background are represented by the grey-shaded bands. |

png pdf |
Figure 5-c:
Transformed output of the pNNs used in the X(0)→HH (top left), X(2)→HH (top right), X→Y(ττ)H(γγ) (middle), low-mass X→Y(γγ)H(ττ) (bottom left) and high-mass X→Y(γγ)H(ττ) (bottom right) searches. The pNNs are evaluated at the mass points where the largest excess with respect to the background-only hypothesis is observed. If the MC simulation at this mass point is not available, then the sample produced at a mass point closest to the excess is shown. The filled histograms represent the background simulation, and the data are shown by the black points. The ``H'' process includes ggH, VBF, VH, and t¯tH. The targeted signal distributions for which the pNN is evaluated are shown by the black unfilled histograms. The background MC simulation is normalized to data and the signal is normalized to an arbitrary cross section for representation purposes. The ratio of the data to the sum of the background predictions is shown in the lower panel. Statistical MC uncertainties for the background are represented by the grey-shaded bands. |

png pdf |
Figure 5-d:
Transformed output of the pNNs used in the X(0)→HH (top left), X(2)→HH (top right), X→Y(ττ)H(γγ) (middle), low-mass X→Y(γγ)H(ττ) (bottom left) and high-mass X→Y(γγ)H(ττ) (bottom right) searches. The pNNs are evaluated at the mass points where the largest excess with respect to the background-only hypothesis is observed. If the MC simulation at this mass point is not available, then the sample produced at a mass point closest to the excess is shown. The filled histograms represent the background simulation, and the data are shown by the black points. The ``H'' process includes ggH, VBF, VH, and t¯tH. The targeted signal distributions for which the pNN is evaluated are shown by the black unfilled histograms. The background MC simulation is normalized to data and the signal is normalized to an arbitrary cross section for representation purposes. The ratio of the data to the sum of the background predictions is shown in the lower panel. Statistical MC uncertainties for the background are represented by the grey-shaded bands. |

png pdf |
Figure 5-e:
Transformed output of the pNNs used in the X(0)→HH (top left), X(2)→HH (top right), X→Y(ττ)H(γγ) (middle), low-mass X→Y(γγ)H(ττ) (bottom left) and high-mass X→Y(γγ)H(ττ) (bottom right) searches. The pNNs are evaluated at the mass points where the largest excess with respect to the background-only hypothesis is observed. If the MC simulation at this mass point is not available, then the sample produced at a mass point closest to the excess is shown. The filled histograms represent the background simulation, and the data are shown by the black points. The ``H'' process includes ggH, VBF, VH, and t¯tH. The targeted signal distributions for which the pNN is evaluated are shown by the black unfilled histograms. The background MC simulation is normalized to data and the signal is normalized to an arbitrary cross section for representation purposes. The ratio of the data to the sum of the background predictions is shown in the lower panel. Statistical MC uncertainties for the background are represented by the grey-shaded bands. |

png pdf |
Figure 6:
Signal pdfs for the nonresonant search analysis categories, shown for each year of simulated data, and for the sum of all years together. The pdfs are normalized to 25 times the expected event yields in the SM. The open squares represent the weighted simulation events and the blue line is the corresponding pdf. The grey shaded areas correspond to the σeff, defined as half the width of the narrowest interval containing 68.3% of the mγγ distribution. The contribution from each year of data-taking is illustrated with the dotted lines. |
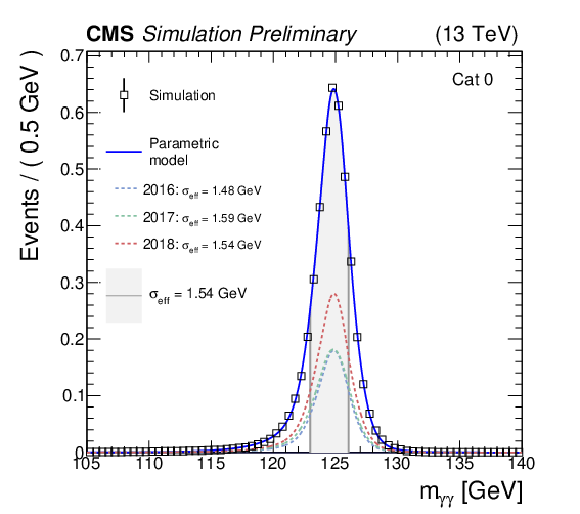
png pdf |
Figure 6-a:
Signal pdfs for the nonresonant search analysis categories, shown for each year of simulated data, and for the sum of all years together. The pdfs are normalized to 25 times the expected event yields in the SM. The open squares represent the weighted simulation events and the blue line is the corresponding pdf. The grey shaded areas correspond to the σeff, defined as half the width of the narrowest interval containing 68.3% of the mγγ distribution. The contribution from each year of data-taking is illustrated with the dotted lines. |

png pdf |
Figure 6-b:
Signal pdfs for the nonresonant search analysis categories, shown for each year of simulated data, and for the sum of all years together. The pdfs are normalized to 25 times the expected event yields in the SM. The open squares represent the weighted simulation events and the blue line is the corresponding pdf. The grey shaded areas correspond to the σeff, defined as half the width of the narrowest interval containing 68.3% of the mγγ distribution. The contribution from each year of data-taking is illustrated with the dotted lines. |

png pdf |
Figure 7:
Signal efficiency, ϵ, and interpolated DCB shape parameters, Δmγγ and σ, for the highest purity analysis category in the X(2)→HH search, as functions of mX (left). The first shape parameter, Δmγγ, is defined as ¯mγγ− 125. Signal efficiency in the (mX,mY) plane for the highest purity analysis category in the X→Y(ττ)H(γγ) search (right). |
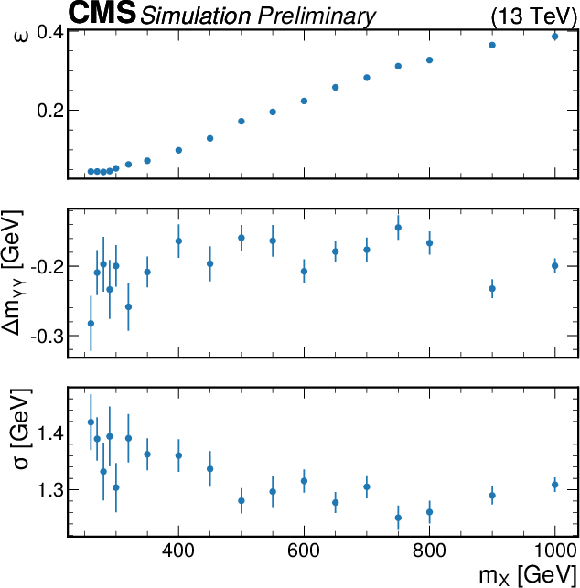
png pdf |
Figure 7-a:
Signal efficiency, ϵ, and interpolated DCB shape parameters, Δmγγ and σ, for the highest purity analysis category in the X(2)→HH search, as functions of mX (left). The first shape parameter, Δmγγ, is defined as ¯mγγ− 125. Signal efficiency in the (mX,mY) plane for the highest purity analysis category in the X→Y(ττ)H(γγ) search (right). |

png pdf |
Figure 7-b:
Signal efficiency, ϵ, and interpolated DCB shape parameters, Δmγγ and σ, for the highest purity analysis category in the X(2)→HH search, as functions of mX (left). The first shape parameter, Δmγγ, is defined as ¯mγγ− 125. Signal efficiency in the (mX,mY) plane for the highest purity analysis category in the X→Y(ττ)H(γγ) search (right). |

png pdf |
Figure 8:
Data points (black) and signal-plus-background models for the most sensitive analysis category in each search channel are shown: nonresonant (top left), X(0)→HH (top right), X(2)→HH (middle left), X→Y(ττ)H(γγ) (middle right), low-mass X→Y(γγ)H(ττ) (bottom left) and the high-mass X→Y(γγ)H(ττ) (bottom right). The analysis categories for the resonant search channels correspond to the mass hypotheses where the largest excesses with respect to the background-only hypothesis are observed. The one (green) standard deviation and two (yellow) standard deviation bands show the uncertainties in the background component of the fit. The solid red line shows the sum of the fitted signal and background components, the solid blue line shows the continuum background and the background from single H production together, and the dashed black line shows only the continuum background component. The lower panel in each plot shows the residual signal yield after subtraction of the background. |

png pdf |
Figure 8-a:
Data points (black) and signal-plus-background models for the most sensitive analysis category in each search channel are shown: nonresonant (top left), X(0)→HH (top right), X(2)→HH (middle left), X→Y(ττ)H(γγ) (middle right), low-mass X→Y(γγ)H(ττ) (bottom left) and the high-mass X→Y(γγ)H(ττ) (bottom right). The analysis categories for the resonant search channels correspond to the mass hypotheses where the largest excesses with respect to the background-only hypothesis are observed. The one (green) standard deviation and two (yellow) standard deviation bands show the uncertainties in the background component of the fit. The solid red line shows the sum of the fitted signal and background components, the solid blue line shows the continuum background and the background from single H production together, and the dashed black line shows only the continuum background component. The lower panel in each plot shows the residual signal yield after subtraction of the background. |

png pdf |
Figure 8-b:
Data points (black) and signal-plus-background models for the most sensitive analysis category in each search channel are shown: nonresonant (top left), X(0)→HH (top right), X(2)→HH (middle left), X→Y(ττ)H(γγ) (middle right), low-mass X→Y(γγ)H(ττ) (bottom left) and the high-mass X→Y(γγ)H(ττ) (bottom right). The analysis categories for the resonant search channels correspond to the mass hypotheses where the largest excesses with respect to the background-only hypothesis are observed. The one (green) standard deviation and two (yellow) standard deviation bands show the uncertainties in the background component of the fit. The solid red line shows the sum of the fitted signal and background components, the solid blue line shows the continuum background and the background from single H production together, and the dashed black line shows only the continuum background component. The lower panel in each plot shows the residual signal yield after subtraction of the background. |

png pdf |
Figure 8-c:
Data points (black) and signal-plus-background models for the most sensitive analysis category in each search channel are shown: nonresonant (top left), X(0)→HH (top right), X(2)→HH (middle left), X→Y(ττ)H(γγ) (middle right), low-mass X→Y(γγ)H(ττ) (bottom left) and the high-mass X→Y(γγ)H(ττ) (bottom right). The analysis categories for the resonant search channels correspond to the mass hypotheses where the largest excesses with respect to the background-only hypothesis are observed. The one (green) standard deviation and two (yellow) standard deviation bands show the uncertainties in the background component of the fit. The solid red line shows the sum of the fitted signal and background components, the solid blue line shows the continuum background and the background from single H production together, and the dashed black line shows only the continuum background component. The lower panel in each plot shows the residual signal yield after subtraction of the background. |

png pdf |
Figure 8-d:
Data points (black) and signal-plus-background models for the most sensitive analysis category in each search channel are shown: nonresonant (top left), X(0)→HH (top right), X(2)→HH (middle left), X→Y(ττ)H(γγ) (middle right), low-mass X→Y(γγ)H(ττ) (bottom left) and the high-mass X→Y(γγ)H(ττ) (bottom right). The analysis categories for the resonant search channels correspond to the mass hypotheses where the largest excesses with respect to the background-only hypothesis are observed. The one (green) standard deviation and two (yellow) standard deviation bands show the uncertainties in the background component of the fit. The solid red line shows the sum of the fitted signal and background components, the solid blue line shows the continuum background and the background from single H production together, and the dashed black line shows only the continuum background component. The lower panel in each plot shows the residual signal yield after subtraction of the background. |

png pdf |
Figure 8-e:
Data points (black) and signal-plus-background models for the most sensitive analysis category in each search channel are shown: nonresonant (top left), X(0)→HH (top right), X(2)→HH (middle left), X→Y(ττ)H(γγ) (middle right), low-mass X→Y(γγ)H(ττ) (bottom left) and the high-mass X→Y(γγ)H(ττ) (bottom right). The analysis categories for the resonant search channels correspond to the mass hypotheses where the largest excesses with respect to the background-only hypothesis are observed. The one (green) standard deviation and two (yellow) standard deviation bands show the uncertainties in the background component of the fit. The solid red line shows the sum of the fitted signal and background components, the solid blue line shows the continuum background and the background from single H production together, and the dashed black line shows only the continuum background component. The lower panel in each plot shows the residual signal yield after subtraction of the background. |

png pdf |
Figure 8-f:
Data points (black) and signal-plus-background models for the most sensitive analysis category in each search channel are shown: nonresonant (top left), X(0)→HH (top right), X(2)→HH (middle left), X→Y(ττ)H(γγ) (middle right), low-mass X→Y(γγ)H(ττ) (bottom left) and the high-mass X→Y(γγ)H(ττ) (bottom right). The analysis categories for the resonant search channels correspond to the mass hypotheses where the largest excesses with respect to the background-only hypothesis are observed. The one (green) standard deviation and two (yellow) standard deviation bands show the uncertainties in the background component of the fit. The solid red line shows the sum of the fitted signal and background components, the solid blue line shows the continuum background and the background from single H production together, and the dashed black line shows only the continuum background component. The lower panel in each plot shows the residual signal yield after subtraction of the background. |
png pdf |
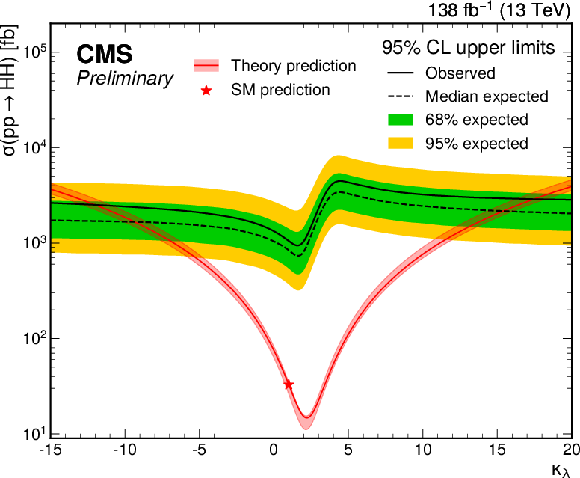
Figure 9:
Expected and observed upper limits on the nonresonant HH production cross section at the 95% CL, obtained for different values of κλ. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. The theoretical prediction with the uncertainty of the cross section as a function of κλ is shown by the red band. |

png pdf |
Figure 10:
Expected and observed upper limits on the nonresonant HH production cross section at the 95% CL, for different thirteen BSM benchmark scenarios which consider different values of the couplings, κλ, κt, c2g, cg and c2 (defined in Table 1). The green and yellow bands represent the one and two standard deviations for the expected limits, respectively. |

png pdf |
Figure 11:
Expected and observed 95% CL upper limit on the resonant production cross section, σ(pp→X→HH) for the spin-0 X(0)→HH search (top) and spin-2 X(2)→HH search (bottom). The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. The red lines show the theoretical predictions with different energy scales and couplings [82]. |

png pdf |
Figure 11-a:
Expected and observed 95% CL upper limit on the resonant production cross section, σ(pp→X→HH) for the spin-0 X(0)→HH search (top) and spin-2 X(2)→HH search (bottom). The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. The red lines show the theoretical predictions with different energy scales and couplings [82]. |

png pdf |
Figure 11-b:
Expected and observed 95% CL upper limit on the resonant production cross section, σ(pp→X→HH) for the spin-0 X(0)→HH search (top) and spin-2 X(2)→HH search (bottom). The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. The red lines show the theoretical predictions with different energy scales and couplings [82]. |

png pdf |
Figure 12:
The top plot shows the expected and observed 95% CL upper limit on σ(pp→X→YH→γγττ) for the X→Y(ττ)H(γγ) search. The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. Limits are scaled by orders of 10, labeled in the plot, depending on mX (left) or mY (right). The bottom plot shows the observed upper limits in the 2D (mX,mY) plane. The values of the limits are shown by the colour scale. |

png pdf |
Figure 12-a:
The top plot shows the expected and observed 95% CL upper limit on σ(pp→X→YH→γγττ) for the X→Y(ττ)H(γγ) search. The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. Limits are scaled by orders of 10, labeled in the plot, depending on mX (left) or mY (right). The bottom plot shows the observed upper limits in the 2D (mX,mY) plane. The values of the limits are shown by the colour scale. |

png pdf |
Figure 12-b:
The top plot shows the expected and observed 95% CL upper limit on σ(pp→X→YH→γγττ) for the X→Y(ττ)H(γγ) search. The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. Limits are scaled by orders of 10, labeled in the plot, depending on mX (left) or mY (right). The bottom plot shows the observed upper limits in the 2D (mX,mY) plane. The values of the limits are shown by the colour scale. |

png |
Figure 12-c:
The top plot shows the expected and observed 95% CL upper limit on σ(pp→X→YH→γγττ) for the X→Y(ττ)H(γγ) search. The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. Limits are scaled by orders of 10, labeled in the plot, depending on mX (left) or mY (right). The bottom plot shows the observed upper limits in the 2D (mX,mY) plane. The values of the limits are shown by the colour scale. |
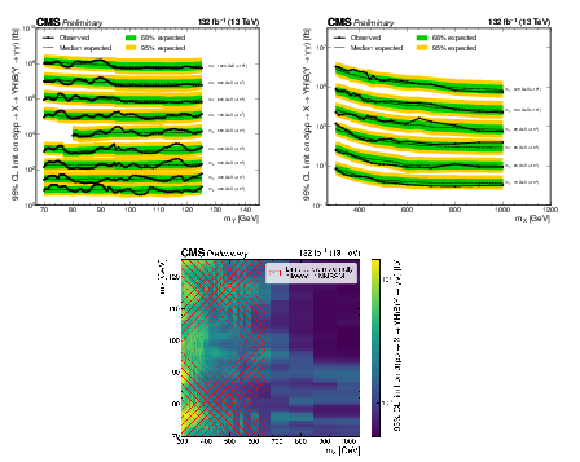
png pdf |
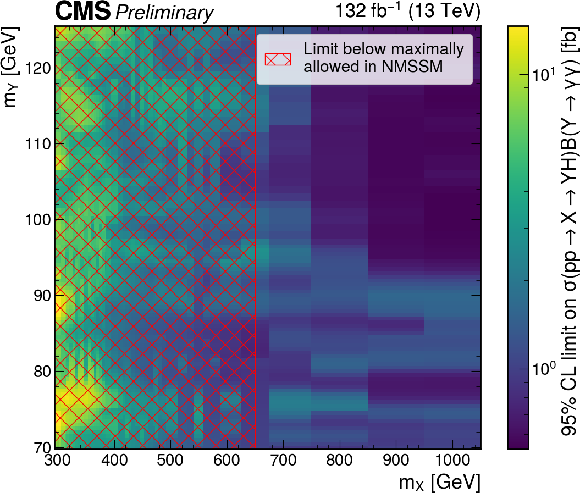
Figure 13:
The top plot shows the expected and observed 95% CL upper limit on σ(pp→X→YH)B(Y→γγ) for the low-mass X→Y(γγ)H(ττ) search. The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. Limits are scaled by orders of 10, labeled in the plot, depending on mX (left) or mY (right). The bottom plot shows the observed upper limits in the 2D (mX,mY) plane. The values of the limits are shown by the colour scale. The red-hatched region indicates masses for which the observed limits are below the maximally allowed limits in the NMSSM taken from Ref. [83]. |

png pdf |
Figure 13-a:
The top plot shows the expected and observed 95% CL upper limit on σ(pp→X→YH)B(Y→γγ) for the low-mass X→Y(γγ)H(ττ) search. The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. Limits are scaled by orders of 10, labeled in the plot, depending on mX (left) or mY (right). The bottom plot shows the observed upper limits in the 2D (mX,mY) plane. The values of the limits are shown by the colour scale. The red-hatched region indicates masses for which the observed limits are below the maximally allowed limits in the NMSSM taken from Ref. [83]. |

png pdf |
Figure 13-b:
The top plot shows the expected and observed 95% CL upper limit on σ(pp→X→YH)B(Y→γγ) for the low-mass X→Y(γγ)H(ττ) search. The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. Limits are scaled by orders of 10, labeled in the plot, depending on mX (left) or mY (right). The bottom plot shows the observed upper limits in the 2D (mX,mY) plane. The values of the limits are shown by the colour scale. The red-hatched region indicates masses for which the observed limits are below the maximally allowed limits in the NMSSM taken from Ref. [83]. |
png |
Figure 13-c:
The top plot shows the expected and observed 95% CL upper limit on σ(pp→X→YH)B(Y→γγ) for the low-mass X→Y(γγ)H(ττ) search. The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. Limits are scaled by orders of 10, labeled in the plot, depending on mX (left) or mY (right). The bottom plot shows the observed upper limits in the 2D (mX,mY) plane. The values of the limits are shown by the colour scale. The red-hatched region indicates masses for which the observed limits are below the maximally allowed limits in the NMSSM taken from Ref. [83]. |

png pdf |
Figure 14:
The top plot shows the expected and observed 95% CL upper limit on σ(pp→X→YH)B(Y→γγ) for the high-mass X→Y(γγ)H(ττ) search. The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. Limits are scaled by orders of 100 (left) or 10 (right), labeled in the plot, depending on mX (left) or mY (right). In the top-left plot, there is a discontinuity in the limits for mX= 650 GeV which is due to the chosen limit granularity in mX and mY. For the range mY∈[274,388]Ge\hspace{-.08em}V, there are limits placed at mX= 600, 633, 666 and 700 GeV instead of 600, 650 and 700 GeV. The bottom plot shows the observed upper limits in the 2D (mX,mY) plane. The values of the limits are shown by the colour scale. |

png pdf |
Figure 14-a:
The top plot shows the expected and observed 95% CL upper limit on σ(pp→X→YH)B(Y→γγ) for the high-mass X→Y(γγ)H(ττ) search. The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. Limits are scaled by orders of 100 (left) or 10 (right), labeled in the plot, depending on mX (left) or mY (right). In the top-left plot, there is a discontinuity in the limits for mX= 650 GeV which is due to the chosen limit granularity in mX and mY. For the range mY∈[274,388]Ge\hspace{-.08em}V, there are limits placed at mX= 600, 633, 666 and 700 GeV instead of 600, 650 and 700 GeV. The bottom plot shows the observed upper limits in the 2D (mX,mY) plane. The values of the limits are shown by the colour scale. |

png pdf |
Figure 14-b:
The top plot shows the expected and observed 95% CL upper limit on σ(pp→X→YH)B(Y→γγ) for the high-mass X→Y(γγ)H(ττ) search. The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. Limits are scaled by orders of 100 (left) or 10 (right), labeled in the plot, depending on mX (left) or mY (right). In the top-left plot, there is a discontinuity in the limits for mX= 650 GeV which is due to the chosen limit granularity in mX and mY. For the range mY∈[274,388]Ge\hspace{-.08em}V, there are limits placed at mX= 600, 633, 666 and 700 GeV instead of 600, 650 and 700 GeV. The bottom plot shows the observed upper limits in the 2D (mX,mY) plane. The values of the limits are shown by the colour scale. |

png |
Figure 14-c:
The top plot shows the expected and observed 95% CL upper limit on σ(pp→X→YH)B(Y→γγ) for the high-mass X→Y(γγ)H(ττ) search. The dashed and solid black lines represent the expected and observed limits, respectively. The green and yellow bands represent the one and two standard deviations for the expected limit, respectively. Limits are scaled by orders of 100 (left) or 10 (right), labeled in the plot, depending on mX (left) or mY (right). In the top-left plot, there is a discontinuity in the limits for mX= 650 GeV which is due to the chosen limit granularity in mX and mY. For the range mY∈[274,388]Ge\hspace{-.08em}V, there are limits placed at mX= 600, 633, 666 and 700 GeV instead of 600, 650 and 700 GeV. The bottom plot shows the observed upper limits in the 2D (mX,mY) plane. The values of the limits are shown by the colour scale. |
| Tables | |

png pdf |
Table 1:
Parameter values of nonresonant BSM benchmark hypotheses. The first column corresponds to the SM sample, while the next 13 correspond to the benchmark hypotheses identified using the method from Refs. [24,25]. |

png pdf |
Table 2:
Additional photon requirements for barrel and endcap photons at different ranges of R9, intended to mimic the HLT requirements. |

png pdf |
Table 3:
Number of expected events in each category for each analysis. There is one last category in addition to the ones shown here that contains the remainder of the events. |
| Summary |
| A search for the production of two scalar bosons in the γγττ final state is presented. The search uses data from proton-proton collisions collected by the CMS experiment at the LHC in 2016--2018 at a centre-of-mass energy of 13 TeV, corresponding to 138 fb−1 of integrated luminosity. In total, five search channels are considered. One channel targets the nonresonant HH production via gluon-fusion, where no significant deviation from the background-only hypothesis is observed. Upper limits at the 95% CL on the HH production cross section are extracted for production in the SM and in several BSM scenarios. The observed upper limit for the SM production is found to be 930 fb, corresponding to 33 times the SM prediction, whilst the expected upper limit is 740 fb, corresponding to 26 times the SM prediction. The limit is also derived as a function of the Higgs boson self-coupling modifier, κλ, assuming all other Higgs boson couplings are as predicted in the SM. The self-coupling modifier, κλ, is constrained within the range −13 <κλ< 18 at the 95% CL. In addition, the limit is extracted for numerous BSM benchmark scenarios. The results are consistent with the SM predictions. This analysis also targets the resonant production of two scalar bosons. Two channels are constructed to search for a resonance X decaying to a SM Higgs boson pair, X→HH, for both the spin-0 resonance and spin-2 resonance scenarios. No significant deviation from the background-only hypothesis is observed. Furthermore, the analysis targets the X→YH process, where Y is an additional, lighter (than X) scalar particle. Three search channels are constructed, namely the X→Y(ττ)H(γγ), low-mass X→Y(γγ)H(ττ) and high-mass X→Y(γγ)H(ττ) channels, which target the different decay chains in different mass regimes. The largest local significance across these channels is 3.4 standard deviations, which once considering the look-elsewhere effect, has a global significance of 0.1 standard deviations. Therefore, this analysis, as a standalone result, does not present any significant deviation from the standard model. However, when put into the context of recent excesses in CMS at resonance masses of 650 GeV and 95 GeV, the local significance of 2.3 at mX= 650 GeV and mY= 95 GeV in the low-mass X→Y(γγ)H(ττ) analysis is interesting and warrants further measurements. |
| Additional Figures | |

png pdf |
Additional Figure 1:
Profile likelihood scan as a function of κλ for the observed data (black solid line). The expected result (red dashed line) assuming the SM hypothesis, derived from an Asimov data set with κλ= 1 is also shown. The best-fit values and 68% CL intervals for κλ from the observed and expected scans are shown in the legend. |

png pdf |
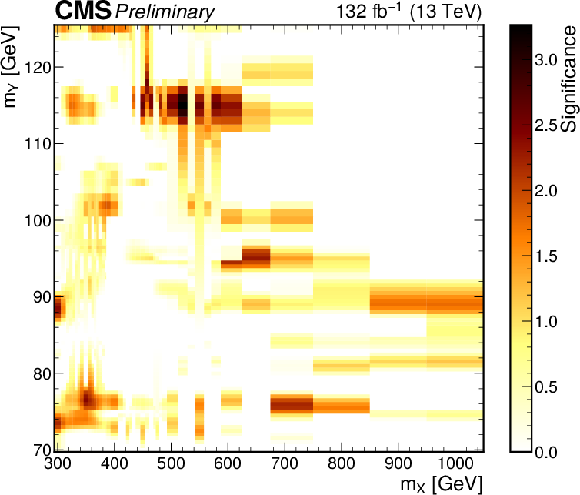
Additional Figure 2:
Local significances of signals in the 2D (mX,mY) plane in the high-mass X→Y(ττ)H(γγ) search. The values of significance are shown by the colour scale. |
png pdf |
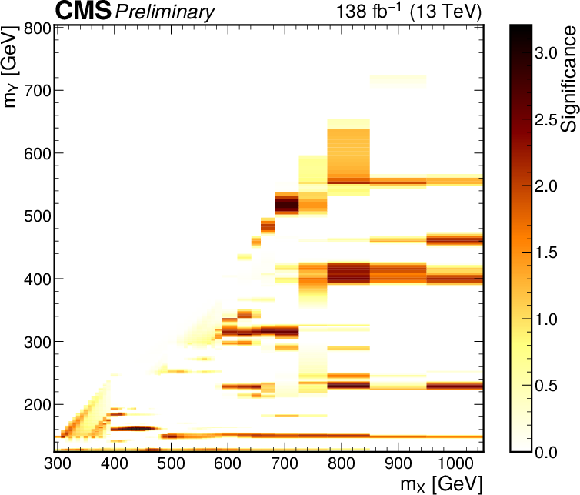
Additional Figure 3:
Local significances of signals in the 2D (mX,mY) plane in the low-mass X→Y(γγ)H(ττ) search. The values of significance are shown by the colour scale. |
png pdf |
Additional Figure 4:
Local significances of signals in the 2D (mX,mY) plane in the high-mass X→Y(γγ)H(ττ) search. The values of significance are shown by the colour scale. |
| References | ||||
| 1 | ATLAS Collaboration | Observation of a new particle in the search for the standard model Higgs boson with the atlas detector at the LHC | PLB 716 (2012) 1 | 1207.7214 |
| 2 | CMS Collaboration | Observation of a new boson at a mass of 125 GeV with the CMS experiment at the LHC | PLB 716 (2012) 30 | CMS-HIG-12-028 1207.7235 |
| 3 | CMS Collaboration | Observation of a new boson with mass near 125 GeV in pp collisions at √s= 7 and 8 TeV | JHEP 06 (2013) 081 | CMS-HIG-12-036 1303.4571 |
| 4 | M. Grazzini et al. | Higgs boson pair production at NNLO with top quark mass effects | JHEP 05 (2018) 059 | 1803.02463 |
| 5 | S. Dawson, S. Dittmaier, and M. Spira | Neutral Higgs boson pair production at hadron colliders: QCD corrections | PRD 58 (1998) 115012 | hep-ph/9805244 |
| 6 | S. Borowka et al. | Higgs boson pair production in gluon fusion at next-to-leading order with full top-quark mass dependence | PRL 117 (2016) 012001 | 1604.06447 |
| 7 | J. Baglio et al. | Gluon fusion into Higgs pairs at NLO QCD and the top mass scheme | EPJC 79 (2019) 459 | 1811.05692 |
| 8 | D. de Florian and J. Mazzitelli | Higgs boson pair production at next-to-next-to-leading order in QCD | PRL 111 (2013) 201801 | 1309.6594 |
| 9 | D. Y. Shao, C. S. Li, H. T. Li, and J. Wang | Threshold resummation effects in Higgs boson pair production at the LHC | JHEP 07 (2013) 169 | 1301.1245 |
| 10 | D. de Florian and J. Mazzitelli | Higgs pair production at next-to-next-to-leading logarithmic accuracy at the LHC | JHEP 09 (2015) 053 | 1505.07122 |
| 11 | J. Baglio et al. | gg→hh: Combined uncertainties | PRD 103 (2021) 056002 | 2008.11626 |
| 12 | CMS Collaboration | A portrait of the Higgs boson by the CMS experiment ten years after the discovery | Nature 607 (2022) 60 | CMS-HIG-22-001 2207.00043 |
| 13 | ATLAS Collaboration | Constraints on the Higgs boson self-coupling from single- and double-Higgs production with the ATLAS detector using pp collisions at s=13 TeV | PLB 843 (2023) 137745 | 2211.01216 |
| 14 | LHC Higgs Cross Section Working Group | Handbook of LHC Higgs cross sections: 4. Deciphering the nature of the Higgs sector | CERN Report CERN-2017-002-M, 2016 link |
1610.07922 |
| 15 | F. Goertz, A. Papaefstathiou, L. L. Yang, and J. Zurita | Higgs boson pair production in the D=6 extension of the SM | JHEP 04 (2015) 167 | 1410.3471 |
| 16 | L. Randall and R. Sundrum | A large mass hierarchy from a small extra dimension | PRL 83 (1999) 3370 | hep-ph/9905221 |
| 17 | W. D. Goldberger and M. B. Wise | Modulus stabilization with bulk fields | PRL 83 (1999) 4922 | hep-ph/9907447 |
| 18 | H. Davoudiasl, J. L. Hewett, and T. G. Rizzo | Phenomenology of the Randall-Sundrum gauge hierarchy model | PRL 84 (2000) 2080 | hep-ph/9909255 |
| 19 | A. Djouadi | The anatomy of electro-weak symmetry breaking. II. the Higgs bosons in the minimal supersymmetric model | Phys. Rept. 459 (2008) 1 | hep-ph/0503173 |
| 20 | CMS Collaboration | Search for a new resonance decaying into two spin-0 bosons in a final state with two photons and two bottom quarks in proton-proton collisions at √s= 13 TeV | CMS Physics Analysis Summary , CERN-EP-2023-132, 2023 CMS-PAS-HIG-21-011 |
CMS-PAS-HIG-21-011 |
| 21 | CMS Collaboration | Search for high mass resonances decaying into W+W− in the dileptonic final state with 138 fb−1 of proton-proton collisions at √s= 13 TeV | CMS Physics Analysis Summary, 2022 CMS-PAS-HIG-20-016 |
CMS-PAS-HIG-20-016 |
| 22 | CMS Collaboration | Searches for additional Higgs bosons and for vector leptoquarks in ττ final states in proton-proton collisions at √s= 13 TeV | JHEP 07 (2023) 073 | CMS-HIG-21-001 2208.02717 |
| 23 | CMS Collaboration | Search for a standard model-like Higgs boson in the mass range between 70 and 110GeV in the diphoton final state in proton-proton collisions at √s= 13 TeV | CMS Physics Analysis Summary, 2023 CMS-PAS-HIG-20-002 |
CMS-PAS-HIG-20-002 |
| 24 | A. Carvalho et al. | Higgs pair production: Choosing benchmarks with cluster analysis | JHEP 04 (2016) 126 | 1507.02245 |
| 25 | G. Buchalla et al. | Higgs boson pair production in non-linear effective field theory with full mt-dependence at NLO QCD | JHEP 09 (2018) 057 | 1806.05162 |
| 26 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | |
| 27 | CMS Collaboration | Performance of the CMS level-1 trigger in proton-proton collisions at √s= 13 TeV | JINST 15 (2020) P10017 | CMS-TRG-17-001 2006.10165 |
| 28 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 29 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 30 | CMS Collaboration | Electron and photon reconstruction and identification with the CMS experiment at the CERN LHC | JINST 16 (2021) P05014 | CMS-EGM-17-001 2012.06888 |
| 31 | CMS Collaboration | Performance of photon reconstruction and identification with the CMS detector in proton-proton collisions at √s= 8 TeV | JINST 10 (2015) P08010 | CMS-EGM-14-001 1502.02702 |
| 32 | CMS Collaboration | A measurement of the Higgs boson mass in the diphoton decay channel | PLB 805 (2020) 135425 | CMS-HIG-19-004 2002.06398 |
| 33 | CMS Collaboration | ECAL 2016 refined calibration and Run2 summary plots | CMS Detector Performance Summary CMS-DP-2020-021, 2020 CDS |
|
| 34 | CMS Collaboration | Performance of the CMS muon detector and muon reconstruction with proton-proton collisions at √s= 13 TeV | JINST 13 (2018) P06015 | CMS-MUO-16-001 1804.04528 |
| 35 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-kT jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 36 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 37 | CMS Collaboration | Pileup mitigation at CMS in 13 TeV data | JINST 15 (2020) P09018 | CMS-JME-18-001 2003.00503 |
| 38 | CMS Collaboration | Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV | JINST 12 (2017) P02014 | CMS-JME-13-004 1607.03663 |
| 39 | CMS Collaboration | Performance of reconstruction and identification of τ leptons decaying to hadrons and ντ in pp collisions at √s= 13 TeV | JINST 13 (2018) P10005 | CMS-TAU-16-003 1809.02816 |
| 40 | CMS Collaboration | Identification of hadronic tau lepton decays using a deep neural network | JINST 17 (2022) P07023 | CMS-TAU-20-001 2201.08458 |
| 41 | CMS Collaboration | Performance of missing transverse momentum reconstruction in proton-proton collisions at √s= 13 TeV using the CMS detector | JINST 14 (2019) P07004 | CMS-JME-17-001 1903.06078 |
| 42 | P. Baldi et al. | Parameterized neural networks for high-energy physics | EPJC 76 (2016) 235 | 1601.07913 |
| 43 | S. Choi and H. Oh | Improved extrapolation methods of data-driven background estimations in high energy physics | EPJC 81 (2021) 643 | 1906.10831 |
| 44 | CMS Collaboration | Precision luminosity measurement in proton-proton collisions at √s= 13 TeV in 2015 and 2016 at CMS | EPJC 81 (2021) 800 | CMS-LUM-17-003 2104.01927 |
| 45 | CMS Collaboration | CMS luminosity measurement for the 2017 data-taking period at √s= 13 TeV | CMS Physics Analysis Summary, 2018 CMS-PAS-LUM-17-004 |
CMS-PAS-LUM-17-004 |
| 46 | CMS Collaboration | CMS luminosity measurement for the 2018 data-taking period at √s= 13 TeV | CMS Physics Analysis Summary, 2019 CMS-PAS-LUM-18-002 |
CMS-PAS-LUM-18-002 |
| 47 | E. Bagnaschi, G. Degrassi, P. Slavich, and A. Vicini | Higgs production via gluon fusion in the POWHEG approach in the SM and in the MSSM | JHEP 02 (2012) 088 | 1111.2854 |
| 48 | G. Heinrich et al. | NLO predictions for Higgs boson pair production with full top quark mass dependence matched to parton showers | JHEP 08 (2017) 088 | 1703.09252 |
| 49 | G. Heinrich et al. | Probing the trilinear Higgs boson coupling in di-Higgs production at NLO QCD including parton shower effects | JHEP 06 (2019) 066 | 1903.08137 |
| 50 | S. Jones and S. Kuttimalai | Parton shower and NLO-matching uncertainties in Higgs boson pair production | JHEP 02 (2018) 176 | 1711.03319 |
| 51 | G. Heinrich, S. P. Jones, M. Kerner, and L. Scyboz | A non-linear EFT description of gg→hh at NLO interfaced to POWHEG | JHEP 10 (2020) 021 | 2006.16877 |
| 52 | P. Nason | A new method for combining NLO QCD with shower Monte Carlo algorithms | JHEP 11 (2004) 040 | hep-ph/0409146 |
| 53 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with parton shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 54 | S. Alioli, P. Nason, C. Oleari, and E. Re | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX | JHEP 06 (2010) 043 | 1002.2581 |
| 55 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 56 | E. Bothmann et al. | Event generation with SHERPA 2.2 | SciPost Phys. 7 (2019) 34 | 1905.09127 |
| 57 | T. Sjöstrand et al. | An introduction to PYTHIA 8.2 | Comput. Phys. Commun. 191 (2015) 159 | 1410.3012 |
| 58 | J. Alwall et al. | Comparative study of various algorithms for the merging of parton showers and matrix elements in hadronic collisions | EPJC 53 (2008) 473 | 0706.2569 |
| 59 | R. Frederix and S. Frixione | Merging meets matching in MC@NLO | JHEP 12 (2012) 061 | 1209.6215 |
| 60 | CMS Collaboration | Event generator tunes obtained from underlying event and multiparton scattering measurements | EPJC 76 (2016) 155 | CMS-GEN-14-001 1512.00815 |
| 61 | CMS Collaboration | Extraction and validation of a new set of CMS PYTHIA8 tunes from underlying-event measurements | EPJC 80 (2020) 4 | CMS-GEN-17-001 1903.12179 |
| 62 | NNPDF Collaboration | Parton distributions from high-precision collider data | EPJC 77 (2017) 663 | 1706.00428 |
| 63 | GEANT4 Collaboration | GEANT 4 --- a simulation toolkit | NIM A 506 (2003) 250 | |
| 64 | CMS Collaboration | Performance of electron reconstruction and selection with the CMS detector in proton-proton collisions at \ensuremath\sqrts = 8 TeV | JINST 10 (2015) P06005 | CMS-EGM-13-001 1502.02701 |
| 65 | T. Chen and C. Guestrin | XGBoost: A scalable tree boosting system | in Proc. 22nd ACM SIGKDD Intern. Conf. on Knowledge Discovery and Data Mining, New York, 2016 Proc. 2 (2016) 785 |
|
| 66 | CMS Collaboration | Performance of the DeepJet b tagging algorithm using 41.9 fb−1 of data from proton-proton collisions at 13 TeV with Phase-1 CMS detector | CMS Detector Performance Note CMS-DP-2018-058, 2018 CDS |
|
| 67 | L. Bianchini, J. Conway, E. K. Friis, and C. Veelken | Reconstruction of the Higgs mass in h→ττ events by dynamical likelihood techniques | J. Phys. Conf. Ser. 513 (2014) 022035 | |
| 68 | P. D. Dauncey, M. Kenzie, N. Wardle, and G. J. Davies | Handling uncertainties in background shapes: the discrete profiling method | JINST 10 (2015) P04015 | 1408.6865 |
| 69 | R. A. Fisher | On the interpretation of χ2 from contingency tables, and the calculation of p | Journal of the Royal Statistical Society 85 (1922) 87 | |
| 70 | CMS Collaboration | Search for narrow resonances in the b-tagged dijet mass spectrum in proton-proton collisions at √s= 13TeV | PRD 108 (2023) 012009 | CMS-EXO-20-008 2205.01835 |
| 71 | CMS Collaboration | Measurements of Higgs boson production cross sections and couplings in the diphoton decay channel at √s= 13 TeV | JHEP 07 (2021) 027 | CMS-HIG-19-015 2103.06956 |
| 72 | J. Butterworth et al. | PDF4LHC recommendations for LHC Run II | JPG 43 (2016) 023001 | 1510.03865 |
| 73 | LHC Higgs Cross Section Working Group | Handbook of LHC Higgs cross sections: 3. Higgs properties | CERN Report CERN-2013-004, 2013 link |
1307.1347 |
| 74 | CMS Collaboration | Measurement of the inclusive W and Z production cross sections in pp collisions at √s= 7 TeV | JHEP 10 (2011) 132 | CMS-EWK-10-005 1107.4789 |
| 75 | CMS Collaboration | Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV | JINST 13 (2018) P05011 | CMS-BTV-16-002 1712.07158 |
| 76 | A. L. Read | Presentation of search results: The CLs technique | JPG 28 (2002) 2693 | |
| 77 | ATLAS and CMS Collaborations, and LHC Higgs Combination Group | Procedure for the LHC Higgs boson search combination in Summer 2011 | Technical Report CMS-NOTE-2011-005, ATL-PHYS-PUB-2011-11, 2011 | |
| 78 | T. Junk | Confidence level computation for combining searches with small statistics | NIM A 434 (1999) 435 | hep-ex/9902006 |
| 79 | G. Cowan, K. Cranmer, E. Gross, and O. Vitells | Asymptotic formulae for likelihood-based tests of new physics | EPJC 71 (2011) 1554 | 1007.1727 |
| 80 | F. Maltoni, D. Pagani, A. Shivaji, and X. Zhao | Trilinear Higgs coupling determination via single-Higgs differential measurements at the LHC | EPJC 77 (2017) 887 | 1709.08649 |
| 81 | CMS Collaboration | Search for nonresonant Higgs boson pair production in final states with two bottom quarks and two photons in proton-proton collisions at √s= 13 TeV | JHEP 03 (2021) 257 | CMS-HIG-19-018 2011.12373 |
| 82 | A. Carvalho | Gravity particles from warped extra dimensions, predictions for LHC | technical report, 2014 link |
1404.0102 |
| 83 | U. Ellwanger and C. Hugonie | Benchmark planes for Higgs-to-Higgs decays in the NMSSM | EPJC 82 (2022) 406 | 2203.05049 |
| 84 | U. Ellwanger, J. F. Gunion, and C. Hugonie | NMHDECAY: A Fortran code for the Higgs masses, couplings and decay widths in the NMSSM | JHEP 02 (2005) 066 | hep-ph/0406215 |
| 85 | U. Ellwanger and C. Hugonie | NMHDECAY 2.1: An updated program for sparticle masses, Higgs masses, couplings and decay widths in the NMSSM | Computer Physics Communications 175 (2006) 290 | |
| 86 | G. Belanger, F. Boudjema, A. Pukhov, and A. Semenov | micrOMEGAs_3: A program for calculating dark matter observables | Comput. Phys. Commun. 185 (2014) 960 | 1305.0237 |

|
Compact Muon Solenoid LHC, CERN |

|

|

|

|

|

|