

Compact Muon Solenoid
LHC, CERN
| CMS-HIG-19-008 ; CERN-EP-2020-200 | ||
| Measurement of the Higgs boson production rate in association with top quarks in final states with electrons, muons, and hadronically decaying tau leptons at √s= 13 TeV | ||
| CMS Collaboration | ||
| 7 November 2020 | ||
| Eur. Phys. J. C 81 (2021) 378 | ||
| Abstract: The rate for Higgs (H) bosons production in association with either one (tH) or two (tˉtH) top quarks is measured in final states containing multiple electrons, muons, or tau leptons decaying to hadrons and a neutrino, using proton-proton collisions recorded at a center-of-mass energy of 13 TeV by the CMS experiment. The analyzed data correspond to an integrated luminosity of 137 fb−1. The analysis is aimed at events that contain H→WW, H→ττ, or H→ZZ decays and each of the top quark(s) decays either to lepton+jets or all-jet channels. Sensitivity to signal is maximized by including ten signatures in the analysis, depending on the lepton multiplicity. The separation among the tH, the tˉtH, and the backgrounds is enhanced through machine-learning techniques and matrix-element methods. The measured production rates for the tˉtH and tH signals correspond to 0.92 ± 0.19 (stat) +0.17−0.13 (syst) and 5.7 ± 2.7 (stat) ± 3.0 (syst) of their respective standard model (SM) expectations. The corresponding observed (expected) significance amounts to 4.7 (5.2) standard deviations for tˉtH, and to 1.4 (0.3) for tH production. Assuming that the Higgs boson coupling to the tau lepton is equal in strength to its expectation in the SM, the coupling yt of the Higgs boson to the top quark divided by its SM expectation, κt=yt/ySMt, is constrained to be within −0.9<κt<−0.7 or 0.7<κt<1.1, at 95% confidence level. This result is the most sensitive measurement of the tˉtH production rate to date. | ||
| Links: e-print arXiv:2011.03652 [hep-ex] (PDF) ; CDS record ; inSPIRE record ; HepData record ; CADI line (restricted) ; | ||
| Figures | |

png pdf |
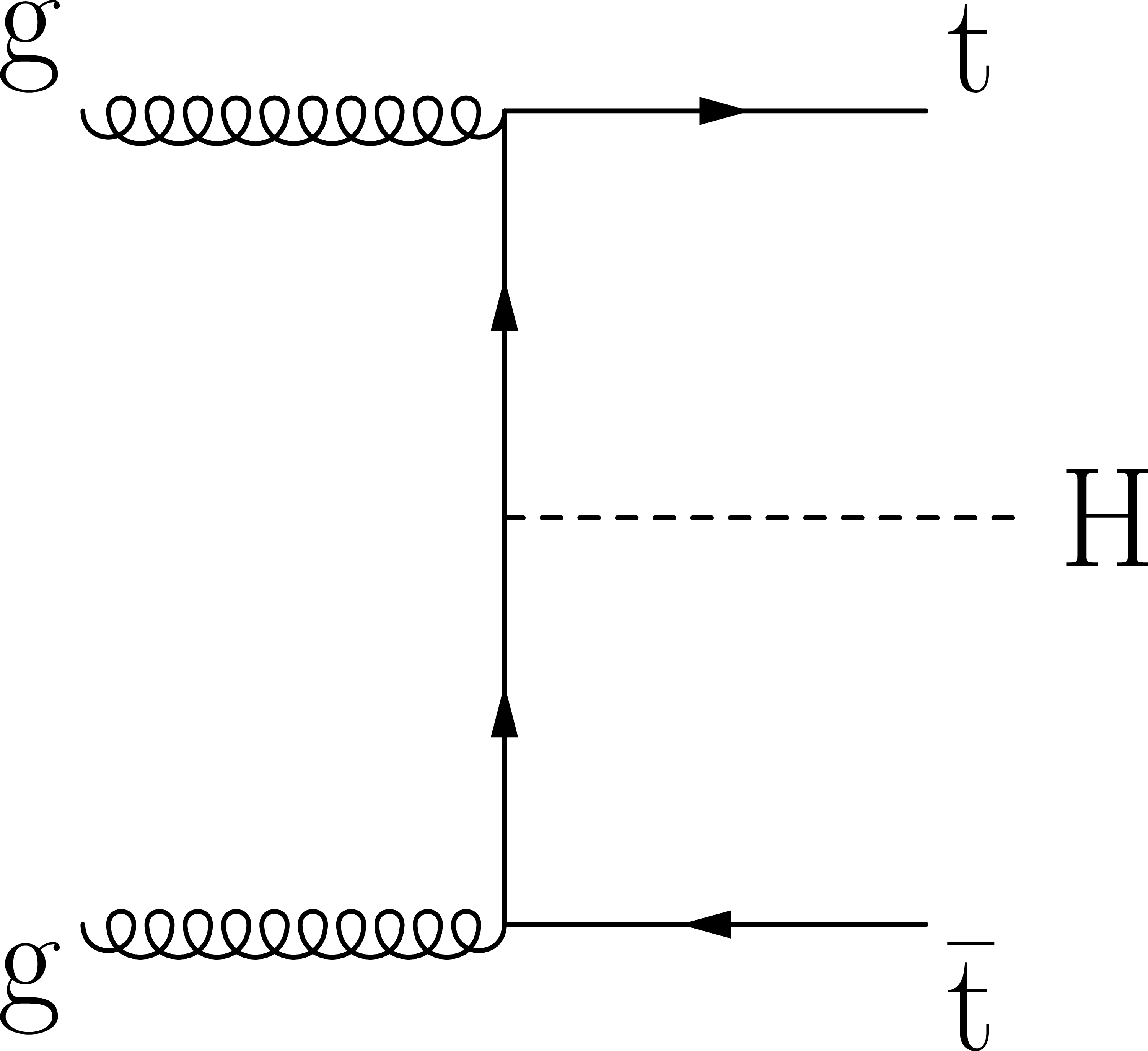
Figure 1:
Feynman diagrams at LO for tˉtH production. |
png pdf |
Figure 1-a:
Feynman diagram at LO for tˉtH production. |

png pdf |
Figure 1-b:
Feynman diagram at LO for tˉtH production. |
png pdf |
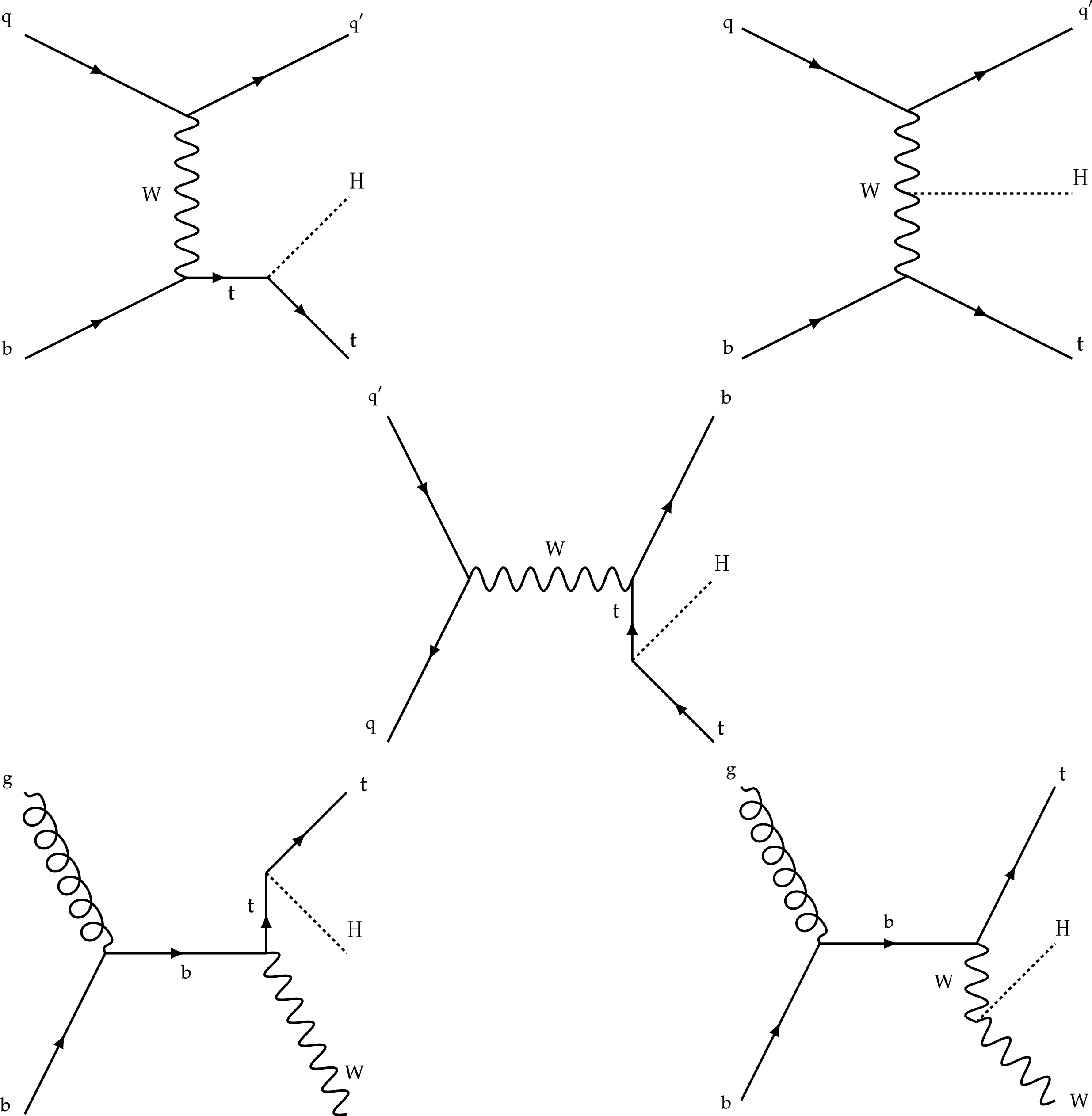
Figure 2:
Feynman diagrams at LO for tH production via the t-channel (tHq in upper left and upper right) and s-channel (middle) processes, and for associated production of a Higgs boson with a single top quark and a W boson (tHW in lower left and lower right). The tHq and tHW production processes are shown for the five-flavor scheme. |

png pdf |
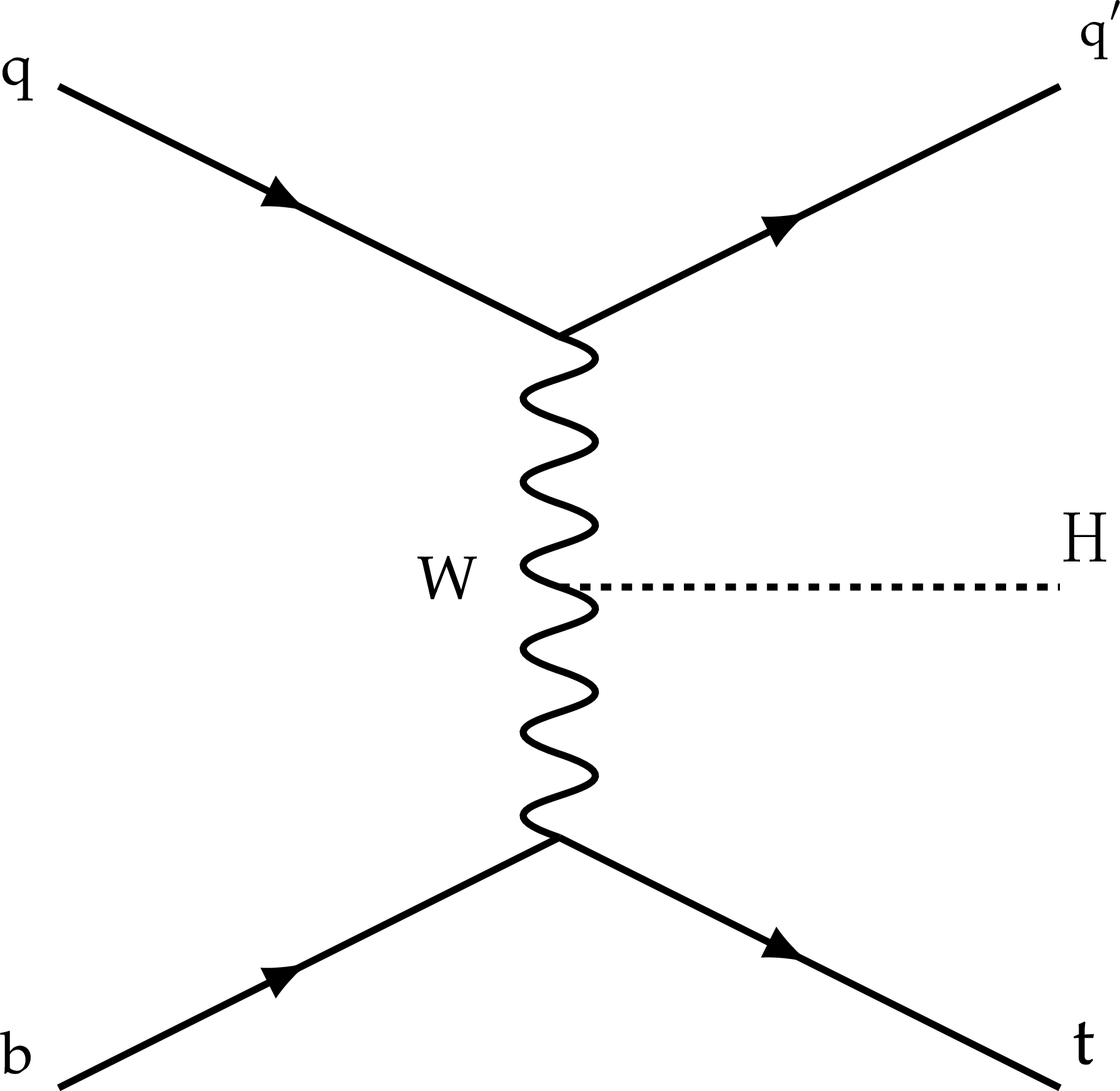
Figure 2-a:
Feynman diagram at LO for the tH production via the t-channel tHq process. This production processes is shown for the five-flavor scheme. |
png pdf |
Figure 2-b:
Feynman diagram at LO for the tH production via the t-channel tHq process. This production processes is shown for the five-flavor scheme. |

png pdf |
Figure 2-c:
Feynman diagram at LO for the tH production via the s-channel process. |

png pdf |
Figure 2-d:
Feynman diagram at LO for the associated production of a Higgs boson with a single top quark and a W boson tHW. This production processes is shown for the five-flavor scheme. |

png pdf |
Figure 2-e:
Feynman diagram at LO for the associated production of a Higgs boson with a single top quark and a W boson tHW. This production processes is shown for the five-flavor scheme. |
png pdf |
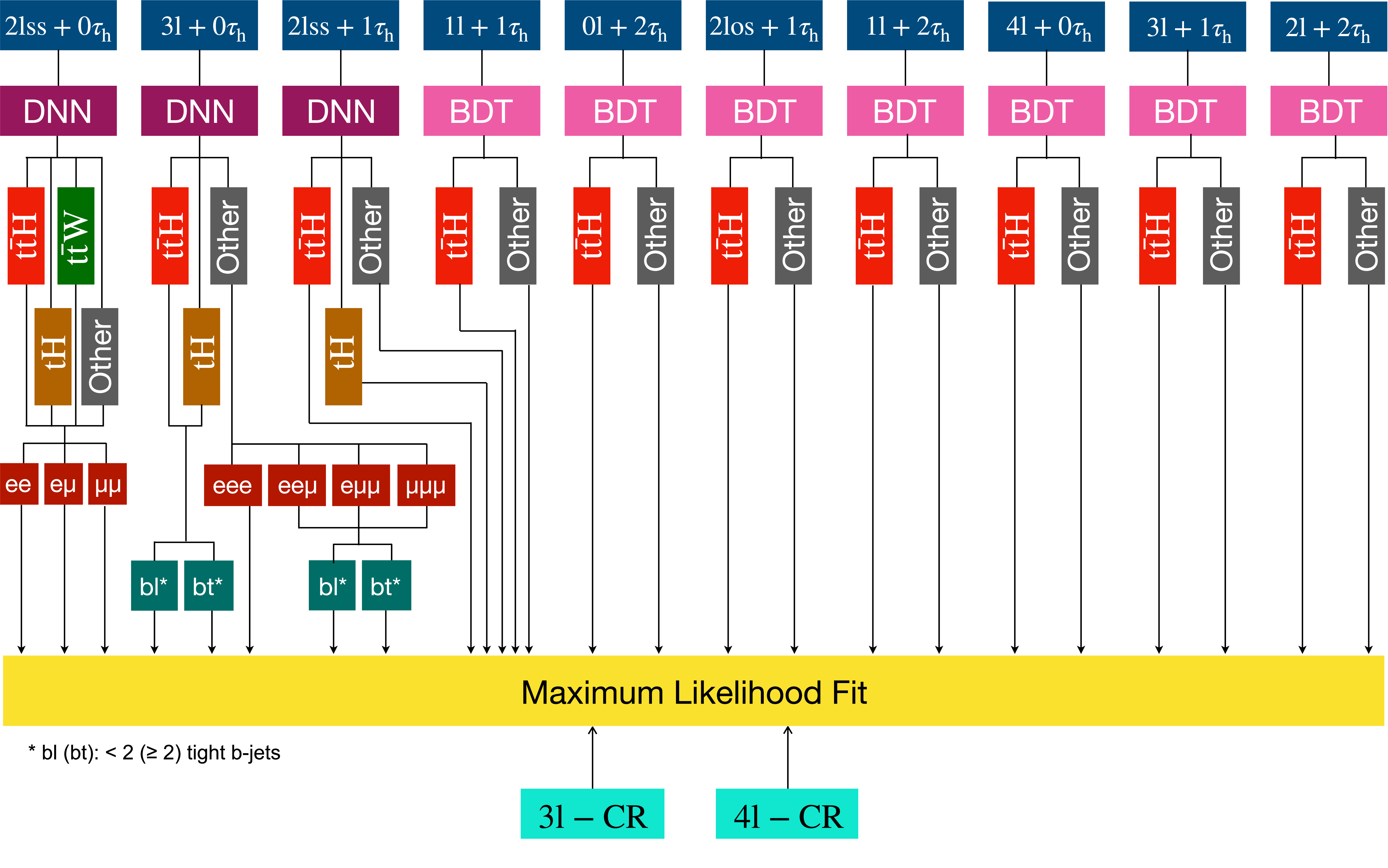
Figure 3:
Diagram showing the categorization strategy used for the signal extraction, making use of MVA-based algorithms and topological variables. In addition to the ten channels, the ML fit receives input from two control regions (CRs) defined in Section 7.3. |

png pdf |
Figure 4:
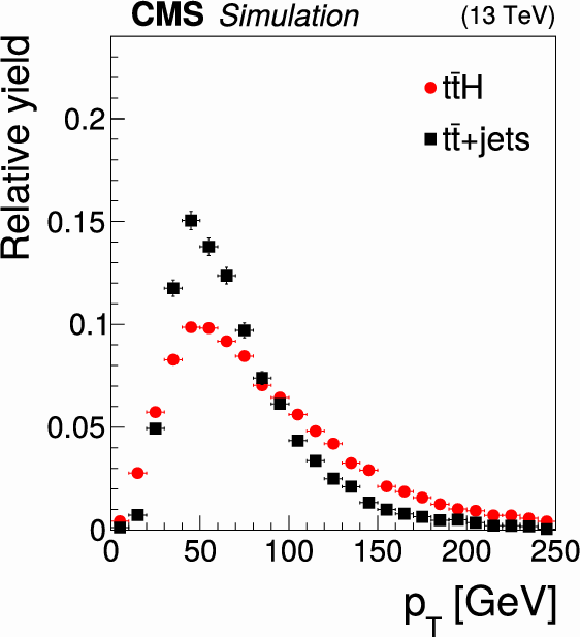
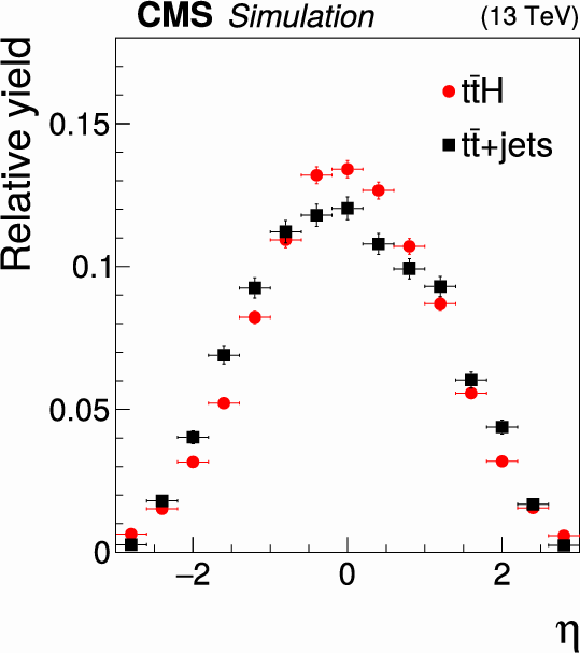
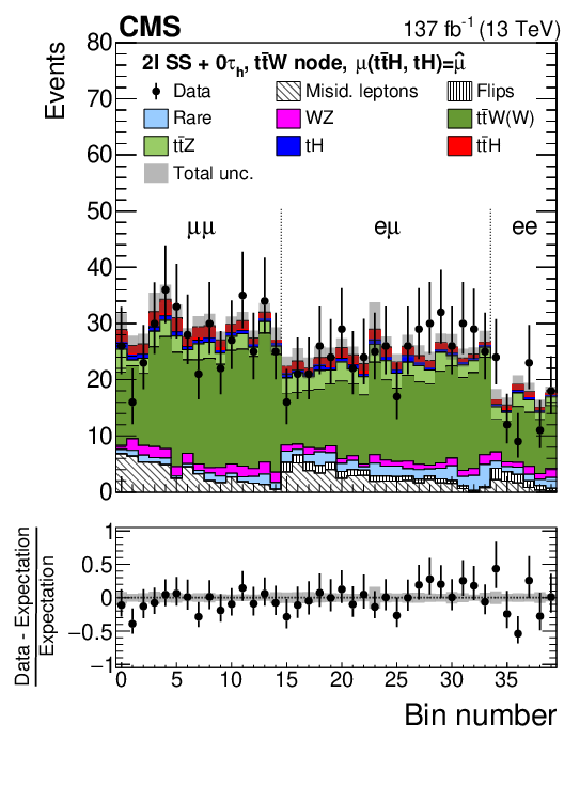
Transverse momentum (left) and pseudorapidity (middle) distributions of bottom quarks produced in top quark decays in tˉtH signal events compared to tˉt+jets background events, and multiplicity of jets passing tight b jet identification criteria (right). The latter distribution is shown separately for tˉt+jets background events in which a nonprompt lepton is misidentified as a prompt lepton and for those background events in which all reconstructed leptons are prompt leptons. The events are selected in the 2ℓSS+0τh channel. |
png pdf |
Figure 4-a:
Transverse momentum distribution of bottom quarks produced in top quark decays in tˉtH signal events compared to tˉt+jets background events. The events are selected in the 2ℓSS+0τh channel. |
png pdf |
Figure 4-b:
Pseudorapidity distribution of bottom quarks produced in top quark decays in tˉtH signal events compared to tˉt+jets background events. The events are selected in the 2ℓSS+0τh channel. |

png pdf |
Figure 4-c:
Multiplicity of jets passing tight b jet identification criteria. The distribution is shown separately for tˉt+jets background events in which a nonprompt lepton is misidentified as a prompt lepton and for those background events in which all reconstructed leptons are prompt leptons. The events are selected in the 2ℓSS+0τh channel. |
png pdf |
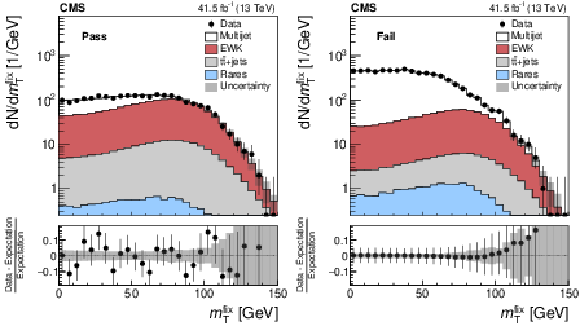
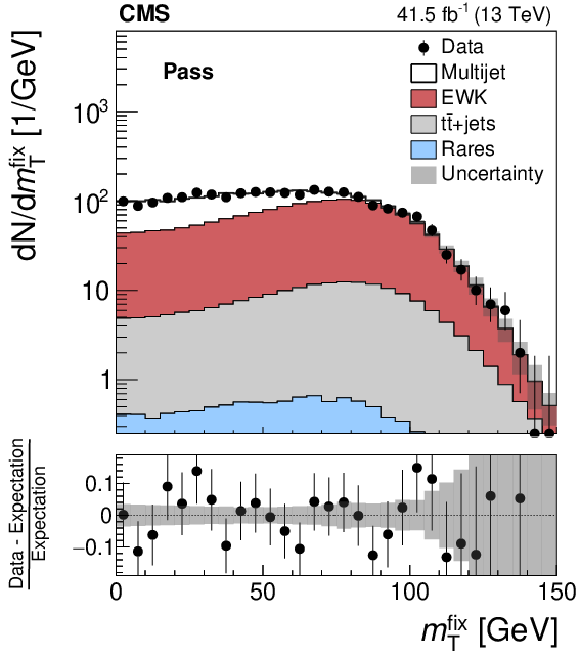
Figure 5:
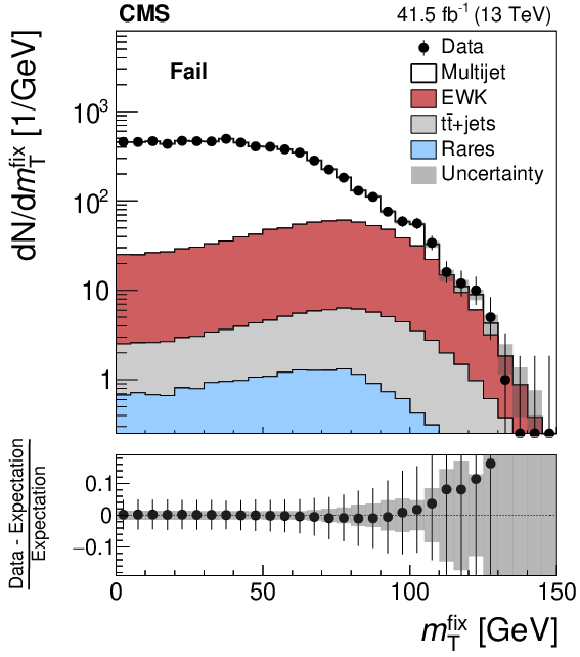
Distributions of mTfix for events containing an electron candidate of 25 <pT< 35 GeV in the ECAL barrel, which (left) passes the nominal selection and (right) passes the relaxed, but fails the nominal selection. The "electroweak'' (EWK) background refers to the sum of W+jets, DY, and diboson production. The "rare'' backgrounds are defined in the text. The data in the fail sample agrees with the sum of multijet, EWK, tˉt+jets, and rare backgrounds by construction, as the number of multijet events in the fail sample is computed by subtracting the sum of EWK, tˉt+jets, and rare background contributions from the data. The misidentification probabilities are derived separately for each era: this figure shows, as an example, the results obtained with the 2017 data set. The uncertainty band represents the total uncertainty after the fit has been performed. |
png pdf |
Figure 5-a:
Distribution of mTfix for events containing an electron candidate of 25 <pT< 35 GeV in the ECAL barrel, which passes the nominal selection. The "electroweak'' (EWK) background refers to the sum of W+jets, DY, and diboson production. The "rare'' backgrounds are defined in the text. The misidentification probabilities are derived separately for each era: this figure shows, as an example, the results obtained with the 2017 data set. The uncertainty band represents the total uncertainty after the fit has been performed. |
png pdf |
Figure 5-b:
Distribution of mTfix for events containing an electron candidate of 25 <pT< 35 GeV in the ECAL barrel, which passes the relaxed, but fails the nominal selection. The "electroweak'' (EWK) background refers to the sum of W+jets, DY, and diboson production. The "rare'' backgrounds are defined in the text. The data in this sample agrees with the sum of multijet, EWK, tˉt+jets, and rare backgrounds by construction, as the number of multijet events in the fail sample is computed by subtracting the sum of EWK, tˉt+jets, and rare background contributions from the data. The misidentification probabilities are derived separately for each era: this figure shows, as an example, the results obtained with the 2017 data set. The uncertainty band represents the total uncertainty after the fit has been performed. |

png pdf |
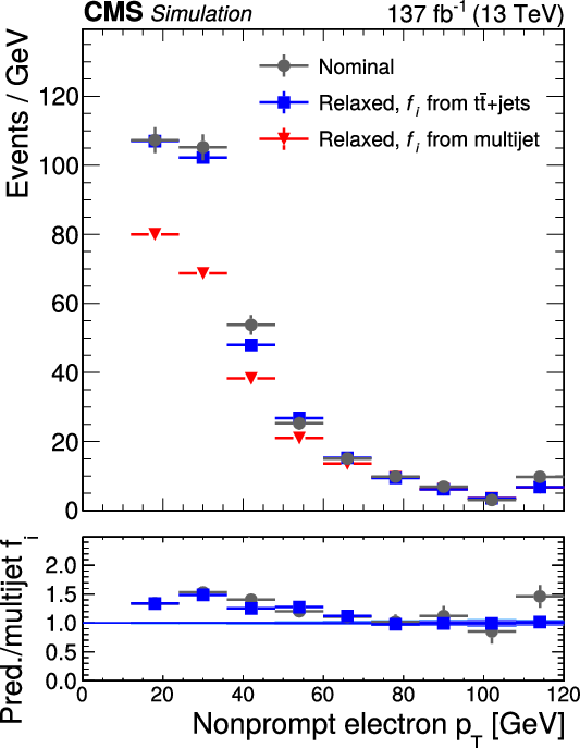
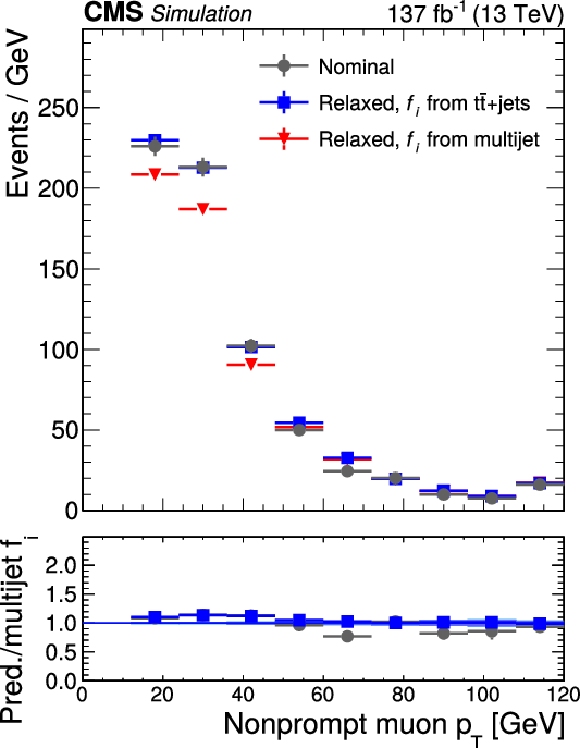
Figure 6:
Transverse momentum distributions of nonprompt (left) electrons and (right) muons in simulated tˉt+jets events, for the three cases "nominal'', "relaxed, fi from tˉt+jets'', and "relaxed, fi from multijet'' discussed in text. The figure illustrates that a nonclosure correction needs to be applied to the probabilities fi measured for electrons in data, while no such correction is needed for muons. |
png pdf |
Figure 6-a:
Transverse momentum distributions of nonprompt electrons in simulated tˉt+jets events, for the three cases "nominal'', "relaxed, fi from tˉt+jets'', and "relaxed, fi from multijet'' discussed in text. The figure illustrates that a nonclosure correction needs to be applied to the probabilities fi measured for electrons in data. |
png pdf |
Figure 6-b:
Transverse momentum distributions of nonprompt muons in simulated tˉt+jets events, for the three cases "nominal'', "relaxed, fi from tˉt+jets'', and "relaxed, fi from multijet'' discussed in text. The figure illustrates that no nonclosure correction needs to be applied to the probabilities fi measured for muons in data. |
png pdf |
Figure 7:
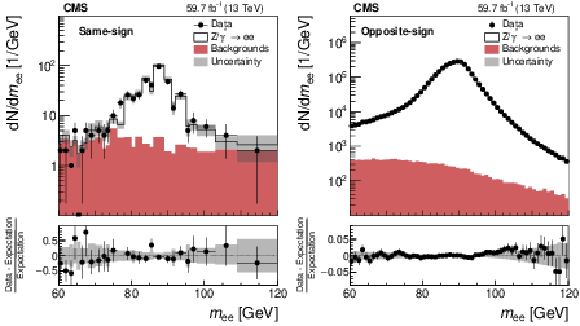
Distributions of mee for (left) SS and (right) OS electron pairs in Z/γ∗→ee candidate events in which both electrons are in the ECAL barrel and have transverse momenta within the range 25 <pT< 50 GeV, for data recorded in 2018, compared to the expectation. Uncertainties shown are statistical only. |
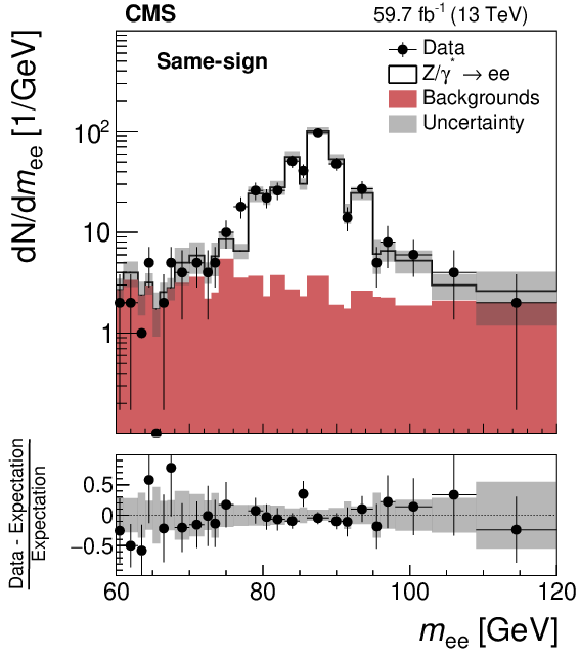
png pdf |
Figure 7-a:
Distribution of mee for SS electron pairs in Z/γ∗→ee candidate events in which both electrons are in the ECAL barrel and have transverse momenta within the range 25 <pT< 50 GeV, for data recorded in 2018, compared to the expectation. Uncertainties shown are statistical only. |

png pdf |
Figure 7-b:
Distribution of mee for OS electron pairs in Z/γ∗→ee candidate events in which both electrons are in the ECAL barrel and have transverse momenta within the range 25 <pT< 50 GeV, for data recorded in 2018, compared to the expectation. Uncertainties shown are statistical only. |
png pdf |
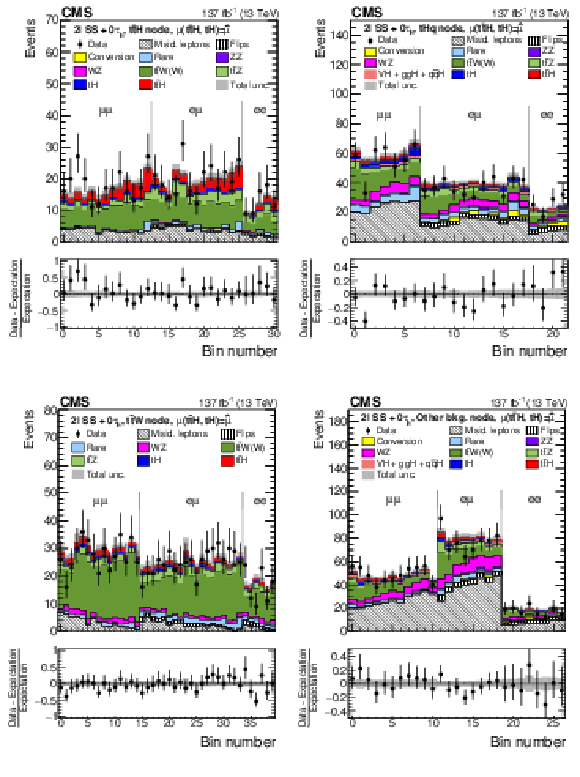
Figure 8:
Distributions of the activation value of the ANN output node with the highest activation value for events selected in the 2ℓSS+0τh channel and classified as tˉtH signal (upper left), tH signal (upper right), tˉtW background (lower left), and other backgrounds (lower right). The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
Figure 8-a:
Distribution of the activation value of the ANN output node with the highest activation value for events selected in the 2ℓSS+0τh channel and classified as tˉtH signal. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
Figure 8-b:
Distribution of the activation value of the ANN output node with the highest activation value for events selected in the 2ℓSS+0τh channel and classified as tH signal. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
Figure 8-c:
Distribution of the activation value of the ANN output node with the highest activation value for events selected in the 2ℓSS+0τh channel and classified as tˉtW background. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |

png pdf |
Figure 8-d:
Distribution of the activation value of the ANN output node with the highest activation value for events selected in the 2ℓSS+0τh channel and classified as other backgrounds. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
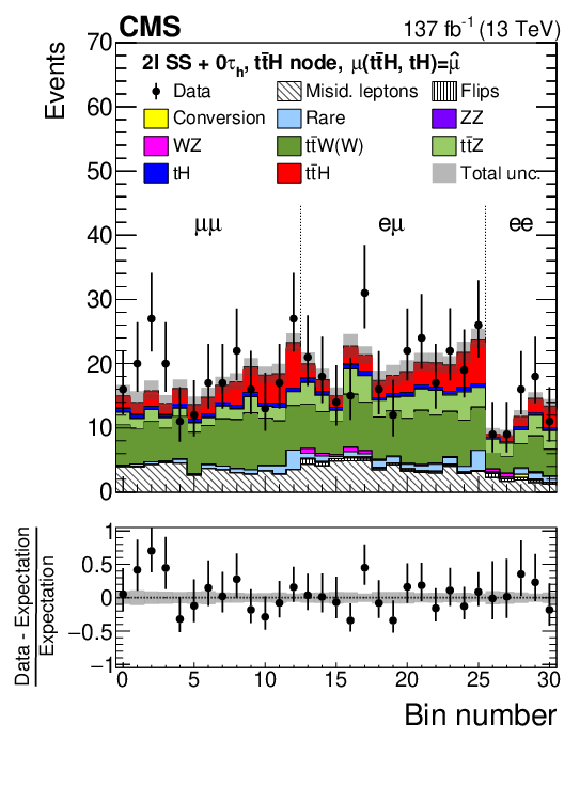
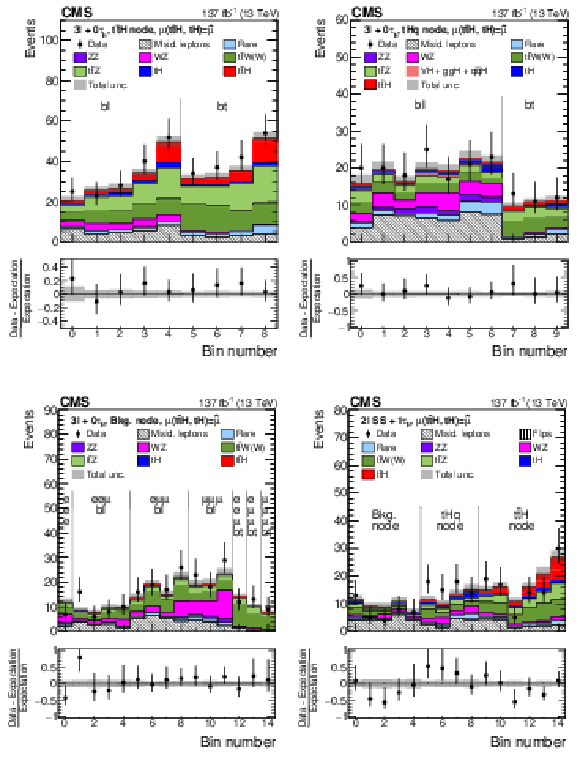
Figure 9:
Distributions of the activation value of the ANN output node with the highest activation value for events selected in the 3ℓ+0τh channel and classified as tˉtH signal (upper left), tH signal (upper right), and background (lower left), and for events selected in the 2ℓSS+1τh channel (lower right). In case of the 2ℓSS+1τh channel, the activation value of the ANN output nodes for tˉtH signal, tH signal, and background are shown together in a single histogram, concatenating histogram bins as appropriate and enumerating the bins by a monotonously increasing number. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |

png pdf |
Figure 9-a:
Distributions of the activation value of the ANN output node with the highest activation value for events selected in the 3ℓ+0τh channel and classified as tˉtH signal. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
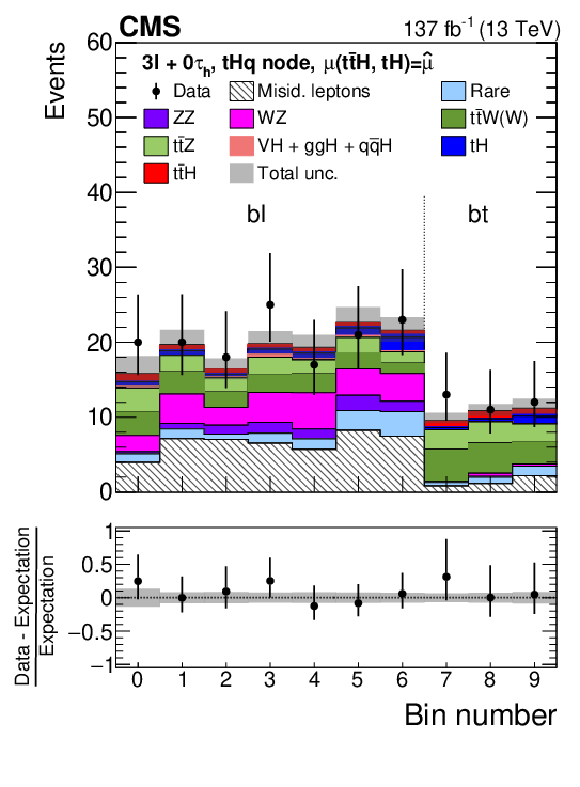
png pdf |
Figure 9-b:
Distributions of the activation value of the ANN output node with the highest activation value for events selected in the 3ℓ+0τh channel and classified as tH signal. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
Figure 9-c:
Distributions of the activation value of the ANN output node with the highest activation value for events selected in the 3ℓ+0τh channel and classified as background. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
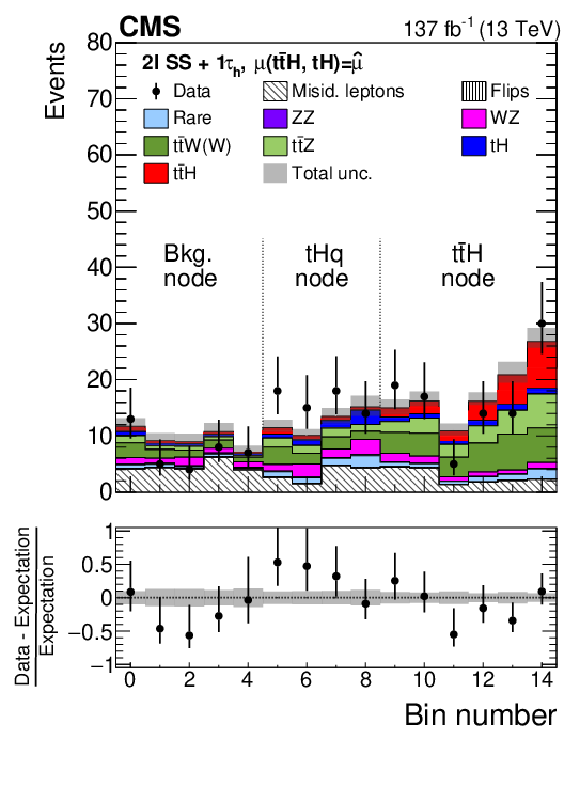
Figure 9-d:
Distributions of the activation value of the ANN output node with the highest activation value for events selected in the 2ℓSS+1τh channel. The activation value of the ANN output nodes for tˉtH signal, tH signal, and background are shown together in a single histogram, concatenating histogram bins as appropriate and enumerating the bins by a monotonously increasing number. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
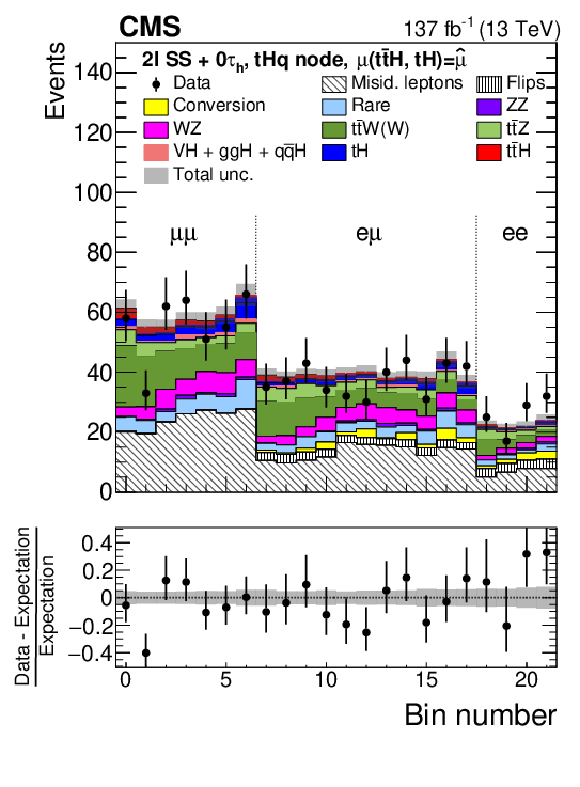
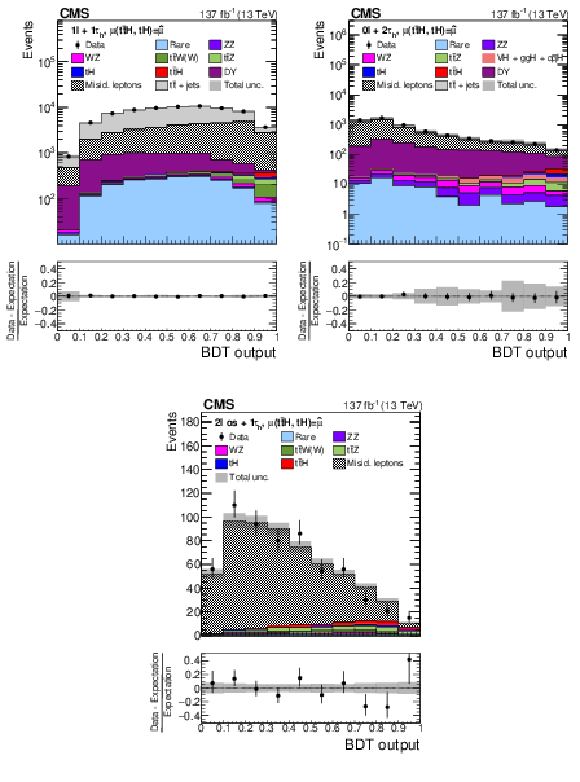
Figure 10:
Distributions of the BDT output for events selected in the 1ℓ+1τh (upper left), 0ℓ+2τh (upper right), and 2ℓOS+1τh (lower) channels. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
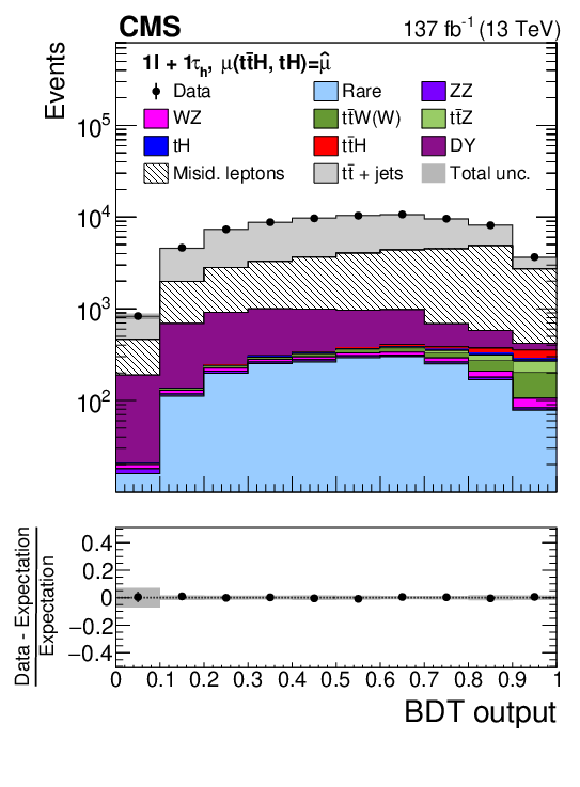
Figure 10-a:
Distributions of the BDT output for events selected in the 1ℓ+1τh channel. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
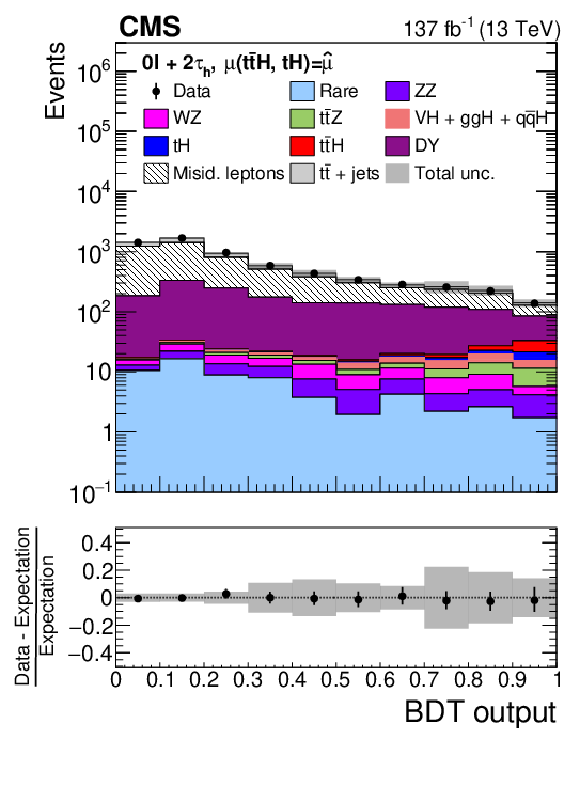
Figure 10-b:
Distributions of the BDT output for events selected in the 0ℓ+2τh channel. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |

png pdf |
Figure 10-c:
Distributions of the BDT output for events selected in the 2ℓOS+1τh channel. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
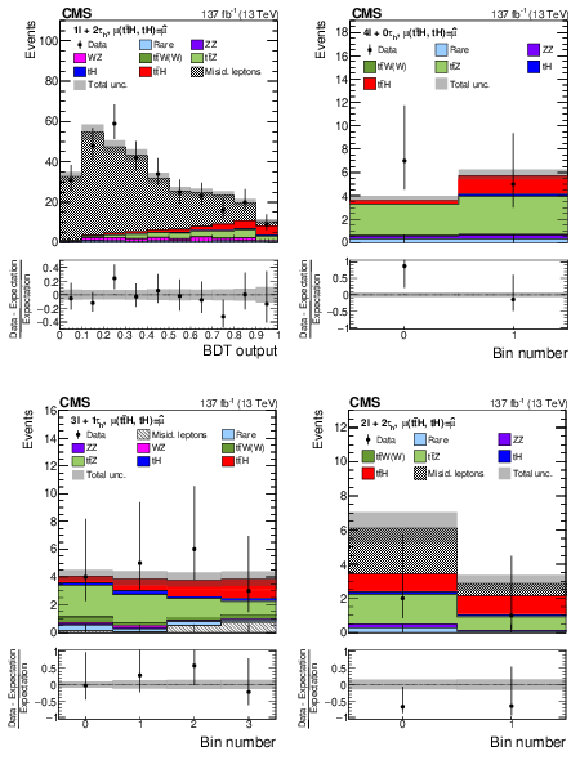
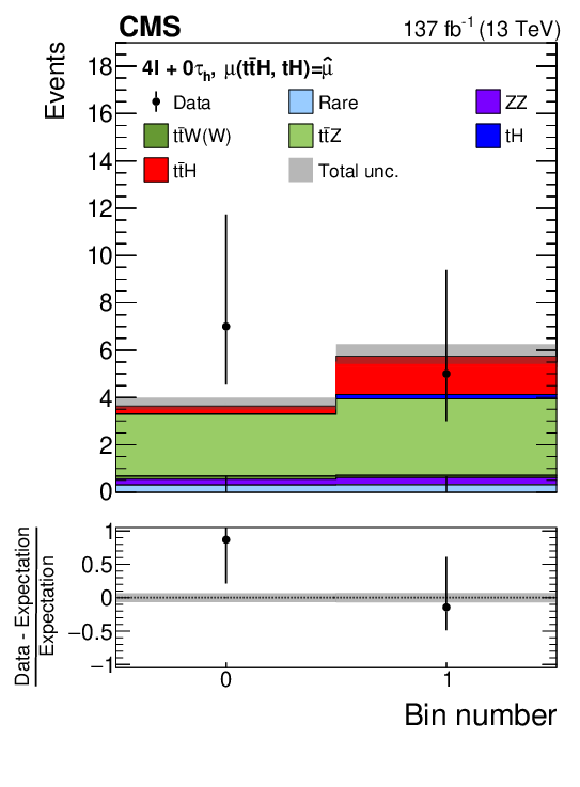
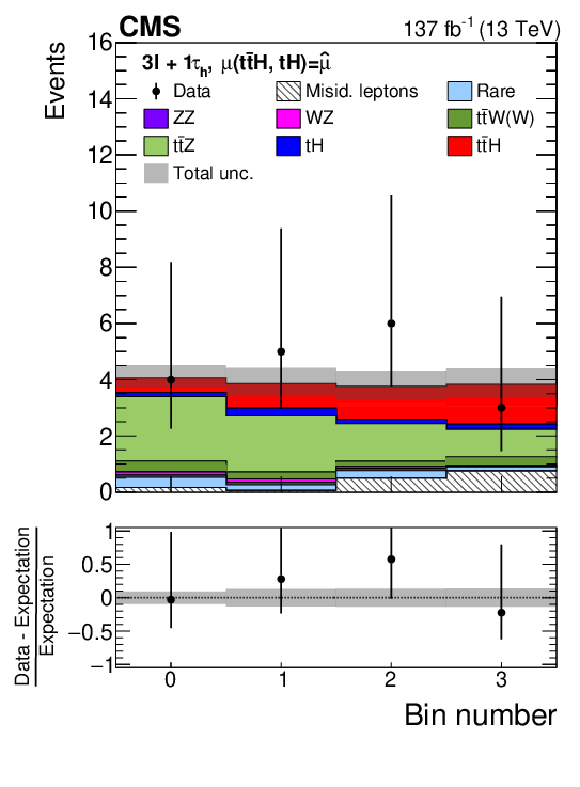
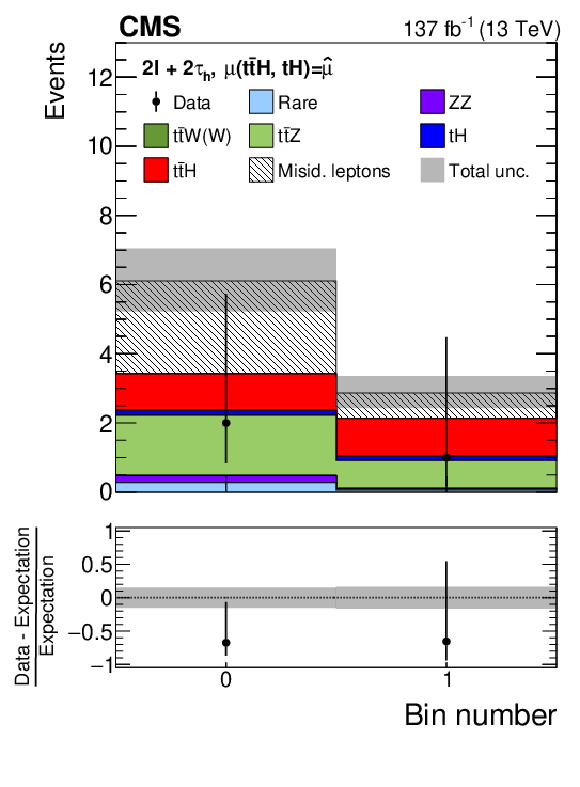
Figure 11:
Distributions of the BDT output used for the signal extraction in the 1ℓ+2τh (upper left), 4ℓ+0τh (upper right), 3ℓ+1τh (lower left), and 2ℓ+2τh (lower right) channels. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |

png pdf |
Figure 11-a:
Distributions of the BDT output used for the signal extraction in the 1ℓ+2τh channel. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
Figure 11-b:
Distributions of the BDT output used for the signal extraction in the 4ℓ+0τh channel. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
Figure 11-c:
Distributions of the BDT output used for the signal extraction in the 3ℓ+1τh channel. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
Figure 11-d:
Distributions of the BDT output used for the signal extraction in the 2ℓ+2τh channel. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |

png pdf |
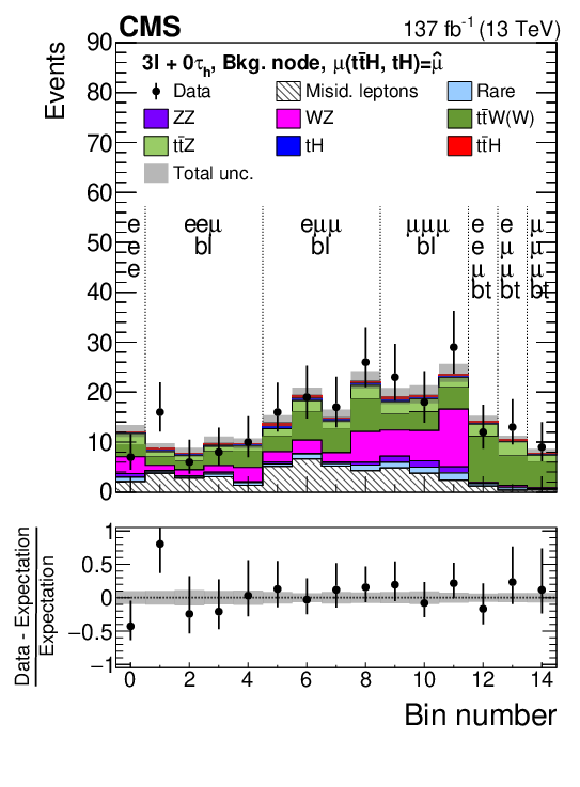
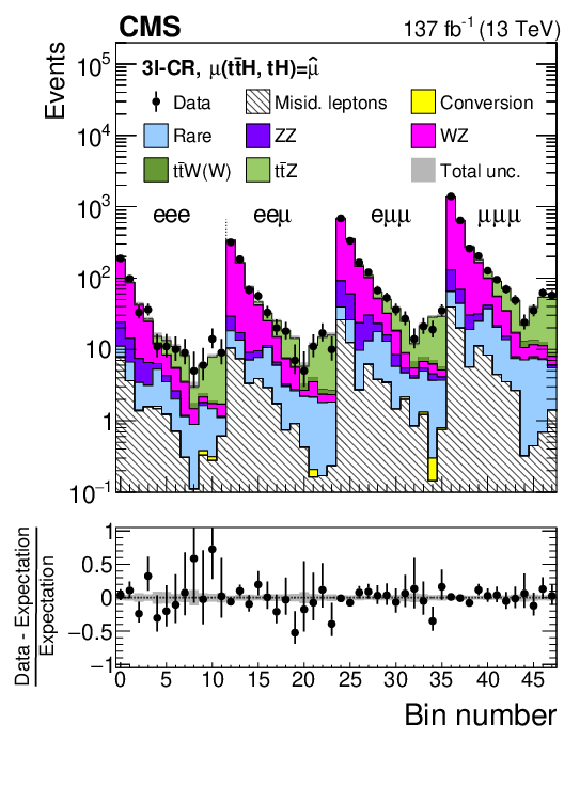
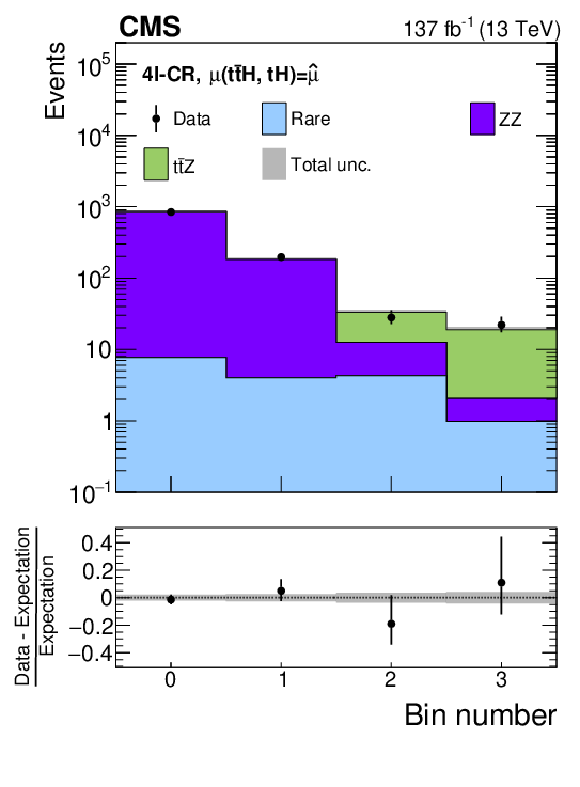
Figure 12:
Distributions of discriminating observables in the 3ℓ+0τh (left) and 4ℓ+0τh (right) control region. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
Figure 12-a:
Distributions of discriminating observables in the 3ℓ+0τh control region. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
Figure 12-b:
Distributions of discriminating observables in the 4ℓ+0τh control region. The distributions expected for the tˉtH and tH signals and for background processes are shown for the values of the parameters of interest and of the nuisance parameters obtained from the ML fit. The best fit value of the tˉtH and tH production rates amounts to ˆμtˉtH= 0.92 and ˆμtH= 5.7 times the rates expected in the SM. |
png pdf |
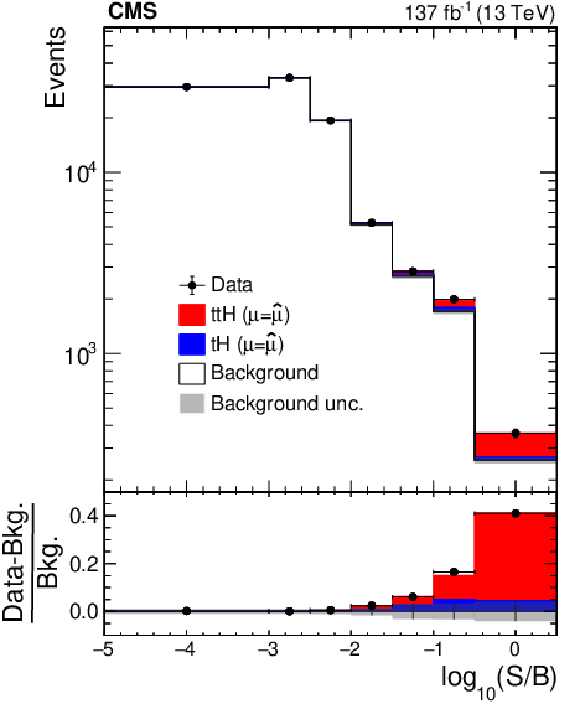
Figure 13:
Distribution of the decimal logarithm of the ratio between the expected tˉtH+tH signal and the expected sum of background contributions in each bin of the 105 distributions that are included in the ML fit used for the signal extraction. The distributions expected for signal and background processes are computed for ˆμtˉtH= 0.92, ˆμtH= 5.7, and the values of nuisance parameters obtained from the ML fit. |
png pdf |
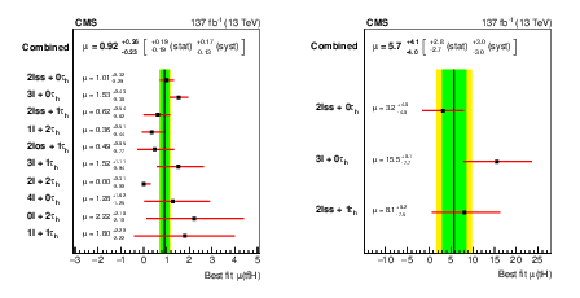
Figure 14:
Production rate ˆμtˉtH of the tˉtH signal (left) and ˆμtH of tH signal (right), in units of their rate of production expected in the SM, measured in each of the ten channels individually and for the combination of all channels. The central value of the signal strength in the 2ℓ+2τh is constrained to be greater than zero. |

png pdf |
Figure 14-a:
Production rate ˆμtˉtH of the tˉtH signal, in units of their rate of production expected in the SM, measured in each of the ten channels individually and for the combination of all channels. The central value of the signal strength in the 2ℓ+2τh is constrained to be greater than zero. |
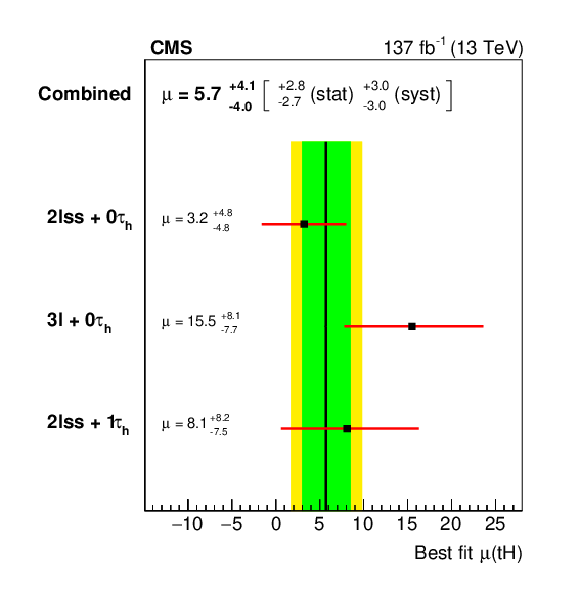
png pdf |
Figure 14-b:
Production rate ˆμtˉtH of the ˆμtH of tH signal, in units of their rate of production expected in the SM, measured in each of the three channels individually and for the combination of all channels. |

png pdf |
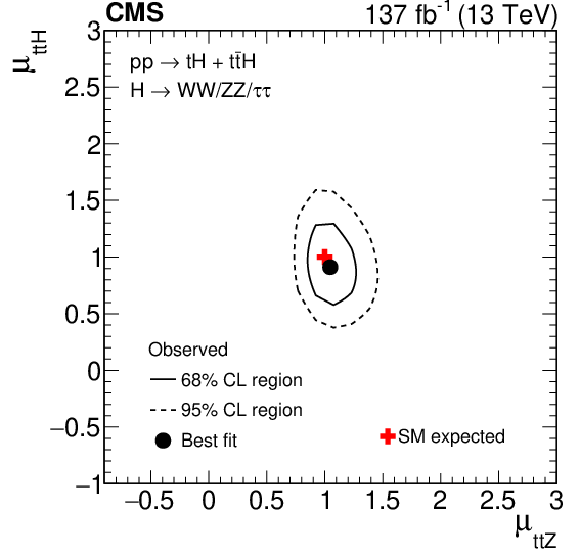
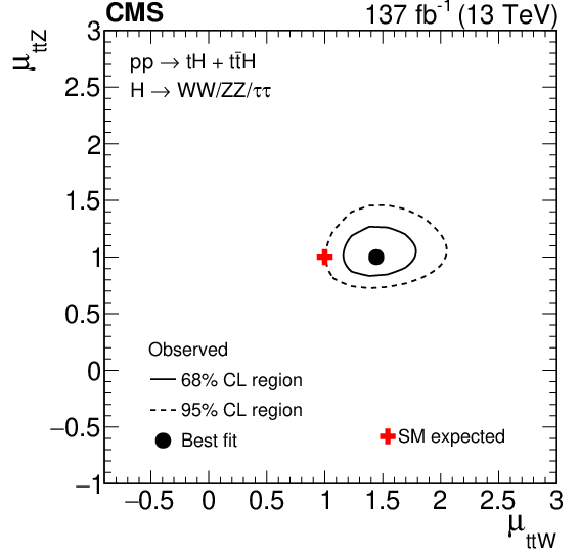
Figure 15:
Two-dimensional contours of the likelihood function L, given by Eq. (3), as a function of the production rates of the tˉtH and tH signals (μtˉtH and μtH) and of the tˉtZ and tˉtW backgrounds (μtˉtZ and μtˉtW). The two production rates that are not shown on either the x or the y axis are profiled such that the function L attains its minimum at each point in the x-y plane. |

png pdf |
Figure 15-a:
Two-dimensional contours of the likelihood function L, given by Eq. (3) (μtˉtH vs μtH). The production rates that are not shown on either the x or the y axis are profiled such that the function L attains its minimum at each point in the x-y plane. |
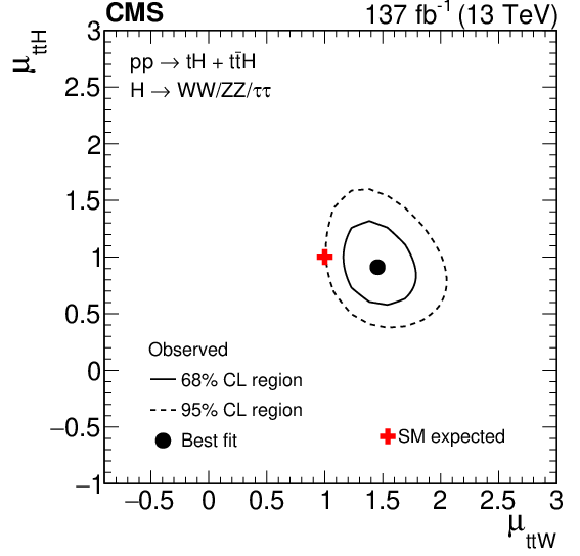
png pdf |
Figure 15-b:
Two-dimensional contours of the likelihood function L, given by Eq. (3) (μtˉtH vs μtˉtW). The production rates that are not shown on either the x or the y axis are profiled such that the function L attains its minimum at each point in the x-y plane. |
png pdf |
Figure 15-c:
Two-dimensional contours of the likelihood function L, given by Eq. (3) (μtˉtH vs μtˉtZ). The production rates that are not shown on either the x or the y axis are profiled such that the function L attains its minimum at each point in the x-y plane. |
png pdf |
Figure 15-d:
Two-dimensional contours of the likelihood function L, given by Eq. (3) (μtˉtZ vs μtˉtW). The production rates that are not shown on either the x or the y axis are profiled such that the function L attains its minimum at each point in the x-y plane. |

png pdf |
Figure 16:
Probability for tH signal events produced by the tHq (left) and tHW (right) production process to pass the event selection criteria for the 2ℓSS+0τh, 3ℓ+0τh, and 2ℓSS+1τh channels in each of the Higgs boson decay modes as a function of the ratio κt/κV of the Higgs boson couplings to the top quark and to the W boson. |

png pdf |
Figure 16-a:
Probability for tH signal events produced by the tHq production process to pass the event selection criteria for the 2ℓSS+0τh, 3ℓ+0τh, and 2ℓSS+1τh channels in each of the Higgs boson decay modes as a function of the ratio κt/κV of the Higgs boson couplings to the top quark and to the W boson. |
png pdf |
Figure 16-b:
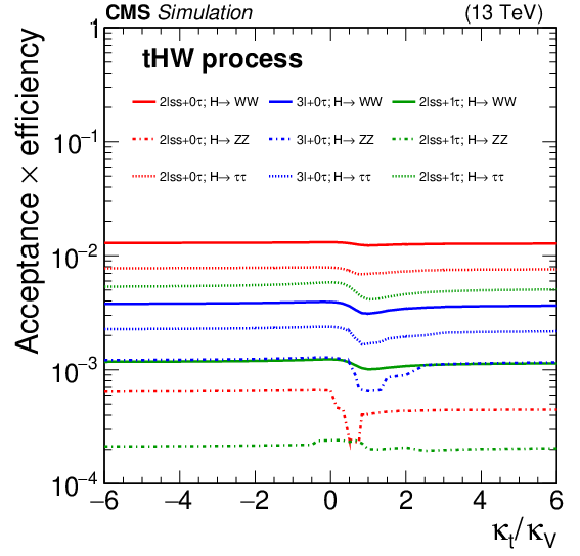
Probability for tH signal events produced by the tHW production process to pass the event selection criteria for the 2ℓSS+0τh, 3ℓ+0τh, and 2ℓSS+1τh channels in each of the Higgs boson decay modes as a function of the ratio κt/κV of the Higgs boson couplings to the top quark and to the W boson. |
png pdf |
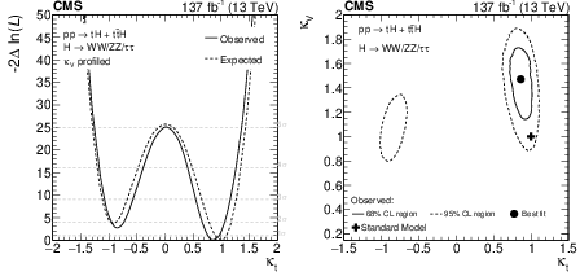
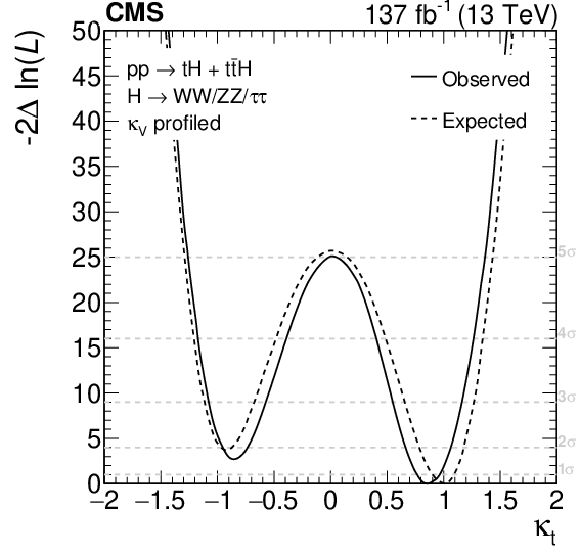
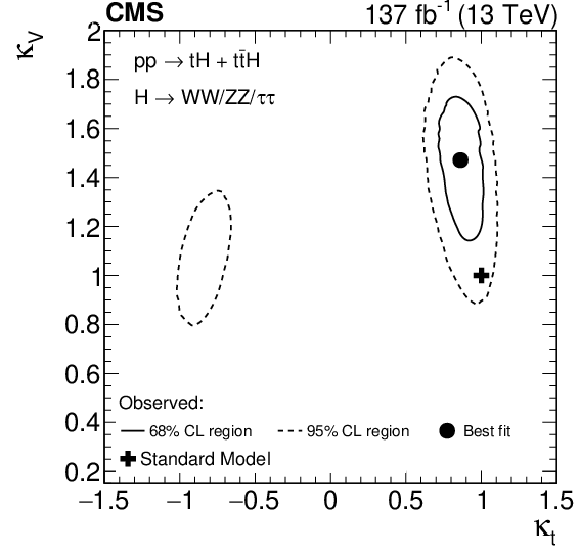
Figure 17:
Dependence of the likelihood function L in Eq. (3), as a function of κt, profiling over κV (left), and as a function of κt and κV (right). |
png pdf |
Figure 17-a:
Dependence of the likelihood function L in Eq. (3), as a function of κt, profiling over κV. |
png pdf |
Figure 17-b:
Dependence of the likelihood function L in Eq. (3), as a function of κt and κV. |
| Tables | |
png pdf |
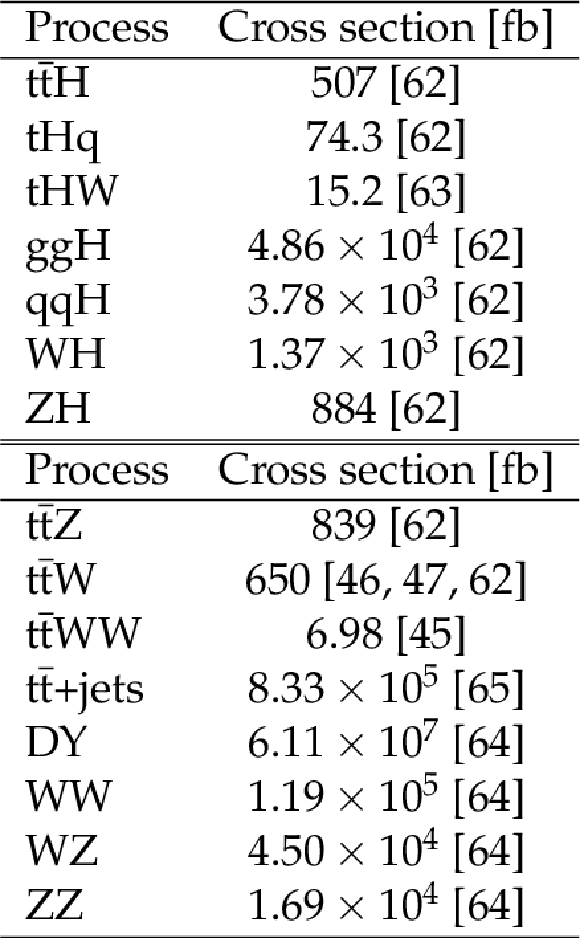
Table 1:
Standard model cross sections for the tˉtH and tH signals as well as for the most relevant background processes. The cross sections are quoted for pp collisions at √s= 13 TeV. The quoted value for DY production includes a generator-level requirement of mZ/γ∗> 50 GeV. |

png pdf |
Table 2:
Event selections applied in the 2ℓSS+0τh, 2ℓSS+1τh, 3ℓ+0τh, and 3ℓ+1τh channels. The pT thresholds applied to the lepton of highest, second-highest, and third-highest pT are separated by slashes. The symbol "--'' indicates that no requirement is applied. |

png pdf |
Table 3:
Event selections applied in the 0ℓ+2τh, 1ℓ+1τh, 1ℓ+2τh, and 2ℓ+2τh channels. The pT thresholds applied to the lepton and to the τh of highest and second-highest pT are separated by slashes. The symbol "--'' indicates that no requirement is applied. |
png pdf |
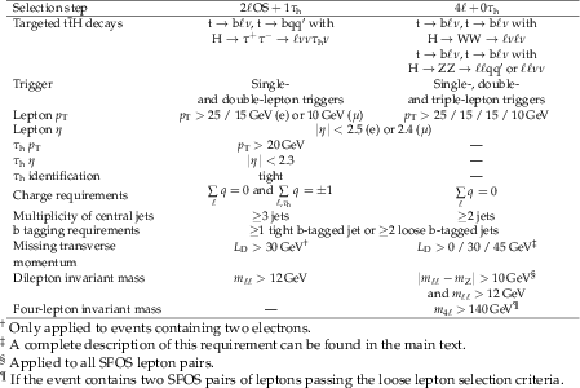
Table 4:
Event selections applied in the 2ℓOS+1τh and 4ℓ+0τh channels. The symbol "--'' indicates that no requirement is applied. |
png pdf |
Table 5:
Input variables to the multivariant discriminants in each of the ten analysis channels. The symbol "--'' indicates that the variable is not used. |
png pdf |
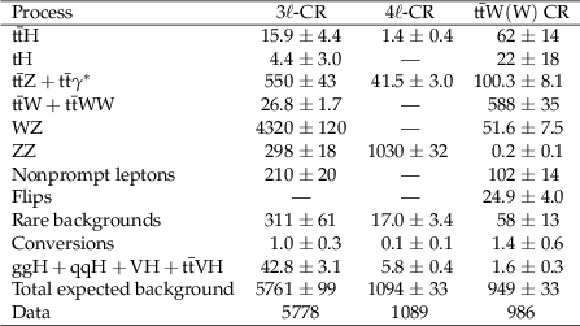
Table 6:
Number of events selected in the 3ℓ- and 4ℓ-CRs and in the CR for the tˉtW(W) background, compared to the event yields expected from different types of background and from the tˉtH and tH signals, after the fit to data is performed as described in Section 9. Uncertainties shown include all systematic components. The symbol "--'' indicates that the corresponding background does not apply. |

png pdf |
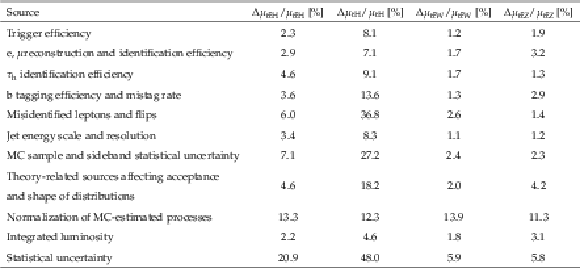
Table 7:
Summary of the sources of systematic and statistical uncertainties and their impact on the measurement of the tˉtH plus tH signal rate μtˉtH+tH, and the measured value of the unconstrained nuisance parameters. The quantity Δμx/μx corresponds to the change in uncertainty when fixing the nuisance parameters associated with that uncertainty in the fit. Under the label "MC and sideband statistical uncertainty'' are the uncertainties associated with the limited number of simulated MC events and the amount of data events in the application region of the MP method. |
| Summary |
| The rate for Higgs boson production in association with either one or two top quarks has been measured in events containing multiple electrons, muons, and hadronically decaying tau leptons, using data recorded by the CMS experiment in pp collisions at √s= 13 TeV in 2016, 2017, and 2018. The analyzed data corresponds to an integrated luminosity of 137 fb−1. Ten different experimental signatures are considered in the analysis, differing by the multiplicity of electrons, muons, and hadronically decaying tau leptons, and targeting events in which the Higgs boson decays via H→WW, H→ττ, or H→ZZ, whereas the top quark(s) decay either semi-leptonically or hadronically. The measured production rates for the tˉtH and tH signals amount to 0.92 ± 0.19 (stat) +0.17−0.13 (syst) and 5.7 ± 2.7 (stat) ± 3.0 (syst) times their respective standard model (SM) expectations. The corresponding observed (expected) significance amounts to 4.7 (5.2) standard deviations for tˉtH, and to 1.4 (0.3) for tH production. Assuming that the Higgs boson coupling to the tau lepton is equal in strength to the values expected in the SM, the coupling yt of the Higgs boson to the top quark divided by its SM expectation, κt=yt/ySMt, is constrained to be within −0.9<κt<−0.7 or 0.7<κt<1.1, at 95% confidence level. This result is the most sensitive measurement of the tˉtH production rate to date. |
| References | ||||
| 1 | ATLAS Collaboration | Observation of a new particle in the search for the standard model Higgs boson with the ATLAS detector at the LHC | PLB 716 (2012) 1 | 1207.7214 |
| 2 | CMS Collaboration | Observation of a new boson at a mass of 125 GeV with the CMS experiment at the LHC | PLB 716 (2012) 30 | CMS-HIG-12-028 1207.7235 |
| 3 | CMS Collaboration | Observation of a new boson with mass near 125 GeV in pp collisions at √s= 7 and 8 TeV | JHEP 06 (2013) 081 | CMS-HIG-12-036 1303.4571 |
| 4 | F. Englert and R. Brout | Broken symmetry and the mass of gauge vector mesons | PRL 13 (1964) 321 | |
| 5 | P. W. Higgs | Broken symmetries, massless particles and gauge fields | PL12 (1964) 132 | |
| 6 | P. W. Higgs | Broken symmetries and the masses of gauge bosons | PRL 13 (Oct, 1964) 508 | |
| 7 | G. S. Guralnik, C. R. Hagen, and T. W. B. Kibble | Global conservation laws and massless particles | PRL 13 (1964) 585 | |
| 8 | Particle Data Group, P. A. Zyla et al. | Review of particle physics | Prog. Theor. Exp. Phys. 2020 (2020) 083C01 | |
| 9 | B. A. Dobrescu and C. T. Hill | Electroweak symmetry breaking via top condensation seesaw | PRL 81 (1998) 2634 | hep-ph/9712319 |
| 10 | R. S. Chivukula, B. A. Dobrescu, H. Georgi, and C. T. Hill | Top quark seesaw theory of electroweak symmetry breaking | PRD 59 (1999) 075003 | hep-ph/9809470 |
| 11 | D. Delepine, J. M. Gerard, and R. Gonzalez Felipe | Is the standard Higgs scalar elementary? | PLB 372 (1996) 271 | hep-ph/9512339 |
| 12 | ATLAS and CMS Collaborations | Measurements of the Higgs boson production and decay rates and constraints on its couplings from a combined ATLAS and CMS analysis of the LHC pp collision data at √s= 7 and 8 TeV | JHEP 08 (2016) 045 | 1606.02266 |
| 13 | S. Biswas, E. Gabrielli, F. Margaroli, and B. Mele | Direct constraints on the top-Higgs coupling from the 8 TeV LHC data | JHEP 07 (2013) 073 | 1304.1822 |
| 14 | B. Hespel, F. Maltoni, and E. Vryonidou | Higgs and Z boson associated production via gluon fusion in the SM and the 2HDM | JHEP 06 (2015) 065 | 1503.01656 |
| 15 | CMS Collaboration | Search for the associated production of the Higgs boson with a top quark pair | JHEP 09 (2014) 087 | CMS-HIG-13-029 1408.1682 |
| 16 | ATLAS Collaboration | Search for Higgs→gammagamma produced in association with top quarks and constraints on the Yukawa coupling between the top quark and the Higgs boson using data taken at 7 TeV and 8 TeV with the ATLAS detector | PLB 740 (2015) 222 | 1409.3122 |
| 17 | ATLAS Collaboration | Search for the standard model Higgs boson produced in association with top quarks and decaying into bˉb in pp collisions at √s= 8 TeV with the ATLAS detector | EPJC 75 (2015) 349 | 1503.05066 |
| 18 | ATLAS Collaboration | Search for the associated production of the Higgs boson with a top quark pair in multilepton final states with the ATLAS detector | PLB 749 (2015) 519 | 1506.05988 |
| 19 | ATLAS Collaboration | Search for the standard model Higgs boson decaying into bˉb produced in association with top quarks decaying hadronically in pp collisions at √s= 8 TeV with the ATLAS detector | JHEP 05 (2016) 160 | 1604.03812 |
| 20 | CMS Collaboration | Measurements of properties of the Higgs boson decaying into the four-lepton final state in pp collisions at √s= 13 TeV | JHEP 11 (2017) 047 | CMS-HIG-16-041 1706.09936 |
| 21 | ATLAS Collaboration | Evidence for the associated production of the Higgs boson and a top quark pair with the ATLAS detector | PRD 97 (2018) 072003 | 1712.08891 |
| 22 | ATLAS Collaboration | Search for the standard model Higgs boson produced in association with top quarks and decaying into a bˉb pair in pp collisions at √s= 13 TeV with the ATLAS detector | PRD 97 (2018) 072016 | 1712.08895 |
| 23 | CMS Collaboration | Evidence for associated production of a Higgs boson with a top quark pair in final states with electrons, muons, and hadronically decaying τ leptons at √s= 13 TeV | JHEP 08 (2018) 066 | CMS-HIG-17-018 1803.05485 |
| 24 | CMS Collaboration | Search for tˉtH production in the all-jet final state in proton-proton collisions at √s= 13 TeV | JHEP 06 (2018) 101 | CMS-HIG-17-022 1803.06986 |
| 25 | CMS Collaboration | Measurements of Higgs boson properties in the diphoton decay channel in proton-proton collisions at √s= 13 TeV | JHEP 11 (2018) 185 | CMS-HIG-16-040 1804.02716 |
| 26 | CMS Collaboration | Search for tˉtH production in the Higgs→bˉb decay channel with leptonic tˉt decays in proton-proton collisions at √s= 13 TeV | JHEP 03 (2019) 026 | CMS-HIG-17-026 1804.03682 |
| 27 | CMS Collaboration | Observation of tˉtH production | PRL 120 (2018) 231801 | CMS-HIG-17-035 1804.02610 |
| 28 | ATLAS Collaboration | Observation of Higgs boson production in association with a top quark pair at the LHC with the ATLAS detector | PLB 784 (2018) 173 | 1806.00425 |
| 29 | CMS Collaboration | Search for the associated production of a Higgs boson with a single top quark in proton-proton collisions at √s= 8 TeV | JHEP 06 (2016) 177 | CMS-HIG-14-027 1509.08159 |
| 30 | CMS Collaboration | Search for associated production of a Higgs boson and a single top quark in proton-proton collisions at √s= 13 TeV | PRD 99 (2019) 092005 | CMS-HIG-18-009 1811.09696 |
| 31 | ATLAS Collaboration | CP properties of Higgs boson interactions with top quarks in the tˉtH and tH processes using H→γγ with the ATLAS detector | PRL 125 (2020) 061802 | 2004.04545 |
| 32 | H. Voss, A. Hocker, J. Stelzer, and F. Tegenfeldt | TMVA, the toolkit for multivariate data analysis with ROOT | in XIth International Workshop on Advanced Computing and Analysis Techniques in Physics Research (ACAT), p. 40 2007 [PoS(ACAT)040] | physics/0703039 |
| 33 | T. Chen and C. Guestrin | XGBoost: a scalable tree boosting system | in Proceedings of the 22nd ACM SIGKDD International Conference on Knowledge Discovery and Data Mining 2016 | |
| 34 | F. Pedregosa et al. | Scikit-learn: machine learning in python | JMLR 12 (2011) 2825 | |
| 35 | K. Kondo | Dynamical likelihood method for reconstruction of events with missing momentum. 1: method and toy models | J. Phys. Soc. Jap. 57 (1988) 4126 | |
| 36 | K. Kondo | Dynamical likelihood method for reconstruction of events with missing momentum. 2: mass spectra for 2→2 processes | J. Phys. Soc. Jap. 60 (1991) 836 | |
| 37 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 38 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | CMS-00-001 |
| 39 | CMS Collaboration | CMS luminosity measurements for the 2016 data-taking period | ||
| 40 | CMS Collaboration | CMS luminosity measurement for the 2017 data-taking period at √s= 13 TeV | ||
| 41 | CMS Collaboration | CMS luminosity measurement for the 2018 data-taking period at √s= 13 TeV | ||
| 42 | J. Alwall et al. | Comparative study of various algorithms for the merging of parton showers and matrix elements in hadronic collisions | EPJC 53 (2008) 473 | 0706.2569 |
| 43 | P. Artoisenet, R. Frederix, O. Mattelaer, and R. Rietkerk | Automatic spin-entangled decays of heavy resonances in Monte Carlo simulations | JHEP 03 (2013) 015 | 1212.3460 |
| 44 | R. Frederix and S. Frixione | Merging meets matching in MC@NLO | JHEP 12 (2012) 061 | 1209.6215 |
| 45 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 46 | R. Frederix and I. Tsinikos | Subleading EW corrections and spin-correlation effects in tˉtW multi-lepton signatures | EPJC 80 (2020) 803 | 2004.09552 |
| 47 | J. A. Dror, M. Farina, E. Salvioni, and J. Serra | Strong tW Scattering at the LHC | JHEP 01 (2016) 071 | 1511.03674 |
| 48 | P. Nason | A new method for combining NLO QCD with shower Monte Carlo algorithms | JHEP 11 (2004) 040 | hep-ph/0409146 |
| 49 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with parton shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 50 | S. Alioli, P. Nason, C. Oleari, and E. Re | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG | JHEP 06 (2010) 043 | 1002.2581 |
| 51 | M. Czakon et al. | Top-pair production at the LHC through NNLO QCD and NLO EW | JHEP 10 (2017) 186 | 1705.04105 |
| 52 | T. Sjostrand et al. | An introduction to PYTHIA 8.2 | CPC 191 (2015) 159 | 1410.3012 |
| 53 | NNPDF Collaboration | Unbiased global determination of parton distributions and their uncertainties at NNLO and at LO | NPB 855 (2012) 153 | 1107.2652 |
| 54 | NNPDF Collaboration | Parton distributions with QED corrections | NPB 877 (2013) 290 | 1308.0598 |
| 55 | NNPDF Collaboration | Parton distributions for the LHC Run 2 | JHEP 04 (2015) 040 | 1410.8849 |
| 56 | NNPDF Collaboration | Parton distributions from high-precision collider data | EPJC 77 (2017) 663 | 1706.00428 |
| 57 | F. Maltoni, G. Ridolfi, and M. Ubiali | b-initiated processes at the LHC: a reappraisal | JHEP 07 (2012) 022 | 1203.6393 |
| 58 | F. Demartin, F. Maltoni, K. Mawatari, and M. Zaro | Higgs production in association with a single top quark at the LHC | EPJC 75 (2015) 267 | 1504.00611 |
| 59 | CMS Collaboration | Extraction and validation of a new set of CMS PYTHIA 8 tunes from underlying-event measurements | EPJC 80 (2020) 4 | CMS-GEN-17-001 1903.12179 |
| 60 | CMS Collaboration | Investigations of the impact of the parton shower tuning in PYTHIA 8 in the modelling of tˉt at √s= 8 and 13 TeV | CMS-PAS-TOP-16-021 | CMS-PAS-TOP-16-021 |
| 61 | CMS Collaboration | Event generator tunes obtained from underlying event and multiparton scattering measurements | EPJC 76 (2016) 155 | CMS-GEN-14-001 1512.00815 |
| 62 | LHC Higgs Cross Section Working Group | Handbook of LHC Higgs cross sections: 4. deciphering the nature of the Higgs sector | CERN (2016) | 1610.07922 |
| 63 | F. Demartin et al. | tWH associated production at the LHC | EPJC 77 (2017) 34 | 1607.05862 |
| 64 | J. M. Campbell, R. K. Ellis, and C. Williams | Vector boson pair production at the LHC | JHEP 07 (2011) 018 | 1105.0020 |
| 65 | M. Czakon and A. Mitov | Top++: a program for the calculation of the top-pair cross section at hadron colliders | CPC 185 (2014) 2930 | 1112.5675 |
| 66 | Y. Li and F. Petriello | Combining QCD and electroweak corrections to dilepton production in FEWZ | PRD 86 (2012) 094034 | 1208.5967 |
| 67 | J. S. Gainer et al. | Exploring theory space with Monte Carlo reweighting | JHEP 10 (2014) 078 | 1404.7129 |
| 68 | O. Mattelaer | On the maximal use of Monte Carlo samples: re-weighting events at NLO accuracy | EPJC 76 (2016) 674 | 1607.00763 |
| 69 | GEANT4 Collaboration | GEANT4--a simulation toolkit | NIMA 506 (2003) 250 | |
| 70 | J. Allison et al. | Recent developments in Geant4 | NIMA 835 (2016) 186 | |
| 71 | CMS Collaboration | Measurements of inclusive W and Z cross sections in pp collisions at √s= 7 TeV | JHEP 01 (2011) 080 | CMS-EWK-10-002 1012.2466 |
| 72 | CMS Collaboration | Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV | JINST 12 (2017) P02014 | CMS-JME-13-004 1607.03663 |
| 73 | CMS Collaboration | Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV | JINST 13 (2018) P05011 | CMS-BTV-16-002 1712.07158 |
| 74 | CMS Collaboration | Performance of reconstruction and identification of τ leptons decaying to hadrons and nuτ in pp collisions at √s= 13 TeV | JINST 13 (2018) P10005 | CMS-TAU-16-003 1809.02816 |
| 75 | CMS Collaboration | Performance of missing transverse momentum reconstruction in proton-proton collisions at √s= 13 TeV using the CMS detector | JINST 14 (2019) P07004 | CMS-JME-17-001 1903.06078 |
| 76 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 77 | CMS Collaboration | Performance of electron reconstruction and selection with the CMS detector in proton-proton collisions at √s= 8 TeV | JINST 10 (2015) P06005 | CMS-EGM-13-001 1502.02701 |
| 78 | CMS Collaboration | Performance of the CMS muon detector and muon reconstruction with proton-proton collisions at √s= 13 TeV | JINST 13 (2018), no. 06, P06015 | CMS-MUO-16-001 1804.04528 |
| 79 | K. Rehermann and B. Tweedie | Efficient identification of boosted semileptonic top quarks at the LHC | JHEP 03 (2011) 059 | 1007.2221 |
| 80 | CMS Collaboration | Search for new physics in same-sign dilepton events in proton-proton collisions at √s= 13 TeV | EPJC 76 (2016) 439 | CMS-SUS-15-008 1605.03171 |
| 81 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-kT jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 82 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 83 | CMS Collaboration | Performance of the DeepTau algorithm for the discrimination of taus against jets, electrons, and muons | CMS-DP-2019-033 | |
| 84 | Y. Lecun | Generalization and network design strategies | of the University of Toronto, CRG-TR-89-4 | |
| 85 | M. Cacciari, G. P. Salam, and G. Soyez | The catchment area of jets | JHEP 04 (2008) 005 | 0802.1188 |
| 86 | M. Cacciari and G. P. Salam | Pileup subtraction using jet areas | PLB 659 (2008) 119 | 0707.1378 |
| 87 | CMS Collaboration | Jet algorithms performance in 13 TeV data | CMS-PAS-JME-16-003 | CMS-PAS-JME-16-003 |
| 88 | CMS Collaboration | CMS Phase 1 heavy flavour identification performance and developments | CMS-DP-2017-013 | |
| 89 | L. Bianchini et al. | Reconstruction of the Higgs mass in events with Higgs bosons decaying into a pair of τ leptons using matrix element techniques | NIMA 862 (2017) 54 | 1603.05910 |
| 90 | J. S. Bridle | Training stochastic model recognition algorithms as networks can lead to maximum mutual information estimation of parameters | in Proceedings, conference on advances in neural information processing systems 2 (NIPS 1989), p. 211 1990 | |
| 91 | X. Glorot, A. Bordes, and Y. Bengio | Deep sparse rectifier neural networks | in Proceedings, 14th international conference on artificial intelligence and statistics (AISTATS 2011), volume 15, p. 315 2011 | |
| 92 | M. Abadi et al. | TensorFlow: large-scale machine learning on heterogeneous distributed systems | 1603.04467 | |
| 93 | F. Chollet et al. | Keras | GitHub | |
| 94 | I. Goodfellow, Y. Bengio, and A. Courville | Deep learning | MIT Press | |
| 95 | A. N. Tikhonov | Solution of incorrectly formulated problems and the regularization method | Soviet Math. Dokl. 4 (1963) 1035 | |
| 96 | N. Srivastava et al. | Dropout: a simple way to prevent neural networks from overfitting | JMLR 15 (2014) 1929 | |
| 97 | CMS Collaboration | Measurement of the inelastic proton-proton cross section at √s= 13 TeV | JHEP 07 (2018) 161 | CMS-FSQ-15-005 1802.02613 |
| 98 | M. Cacciari et al. | The tˉt cross-section at 1.8 TeV and 1.96 TeV: a study of the systematics due to parton densities and scale dependence | JHEP 04 (2004) 068 | hep-ph/0303085 |
| 99 | S. Catani, D. de Florian, M. Grazzini, and P. Nason | Soft gluon resummation for Higgs boson production at hadron colliders | JHEP 07 (2003) 028 | hep-ph/0306211 |
| 100 | R. Frederix et al. | Four-lepton production at hadron colliders: MadGraph5+MCatNLO predictions with theoretical uncertainties | JHEP 02 (2012) 099 | 1110.4738 |
| 101 | J. Butterworth et al. | PDF4LHC recommendations for LHC Run 2 | JPG 43 (2016) 023001 | 1510.03865 |
| 102 | R. Gavin, Y. Li, F. Petriello, and S. Quackenbush | FEWZ 2.0: a code for hadronic Z production at next-to-next-to-leading order | CPC 182 (2011) 2388 | 1011.3540 |
| 103 | J. Neyman | Outline of a theory of statistical estimation based on the classical theory of probability | Phil. Trans. Roy. Soc. Lond. A 236 (1937) 333 | |
| 104 | J. S. Conway | Incorporating nuisance parameters in likelihoods for multisource spectra | in Proceedings, workshop on statistical issues related to discovery claims in search experiments and unfolding (PHYSTAT 2011), p. 115 2011 | 1103.0354 |
| 105 | R. J. Barlow and C. Beeston | Fitting using finite Monte Carlo samples | CPC 77 (1993) 219 | |
| 106 | The ATLAS Collaboration, The CMS Collaboration, The LHC Higgs Combination Group | Procedure for the LHC Higgs boson search combination in Summer 2011 | CMS-NOTE-2011-005 | |
| 107 | CMS Collaboration | Combined results of searches for the standard model Higgs boson in pp collisions at √s= 7 TeV | PLB 710 (2012) 26 | CMS-HIG-11-032 1202.1488 |

|
Compact Muon Solenoid LHC, CERN |

|

|

|

|

|

|