Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-EXO-19-016 ; CERN-EP-2023-144 | ||
| Search for a third-generation leptoquark coupled to a $ \tau $ lepton and a b quark through single, pair, and nonresonant production in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | ||
| CMS Collaboration | ||
| 15 August 2023 | ||
| JHEP 05 (2024) 311 | ||
| Abstract: A search is presented for a third-generation leptoquark (LQ) coupled exclusively to a $ \tau $ lepton and a b quark. The search is based on proton-proton collision data at a center-of-mass energy of 13 TeV recorded with the CMS detector, corresponding to an integrated luminosity of 138 fb$ ^{-1} $. Events with $ \tau $ leptons and a varying number of jets originating from b quarks are considered, targeting the single and pair production of LQs, as well as nonresonant $ t $-channel LQ exchange. An excess is observed in the data with respect to the background expectation in the combined analysis of all search regions. For a benchmark LQ mass of 2 TeV and an LQ-b-$\tau $ coupling strength of 2.5, the excess reaches a local significance of up to 2.8 standard deviations. Upper limits at the 95% confidence level are placed on the LQ production cross section in the LQ mass range 0.5--2.3 TeV, and up to 3 TeV for $ t $-channel LQ exchange. Leptoquarks are excluded below masses of 1.22--1.88 TeV for different LQ models and varying coupling strengths up to 2.5. The study of nonresonant $ \tau\tau $ production through $ t $-channel LQ exchange allows lower limits on the LQ mass of up to 2.3 TeV to be obtained. | ||
| Links: e-print arXiv:2308.07826 [hep-ex] (PDF) ; CDS record ; inSPIRE record ; HepData record ; CADI line (restricted) ; | ||
| Figures | |
png pdf |
Figure 1:
Example Feynman diagrams of signal processes at leading order: single (left) and pair (center) LQ production, as well as nonresonant production of two $ \tau $ leptons via $ t $-channel LQ exchange (right). |
png pdf |
Figure 1-a:
Example Feynman diagram at leading order of single LQ production. |
png pdf |
Figure 1-b:
Example Feynman diagram at leading order of pair LQ production. |
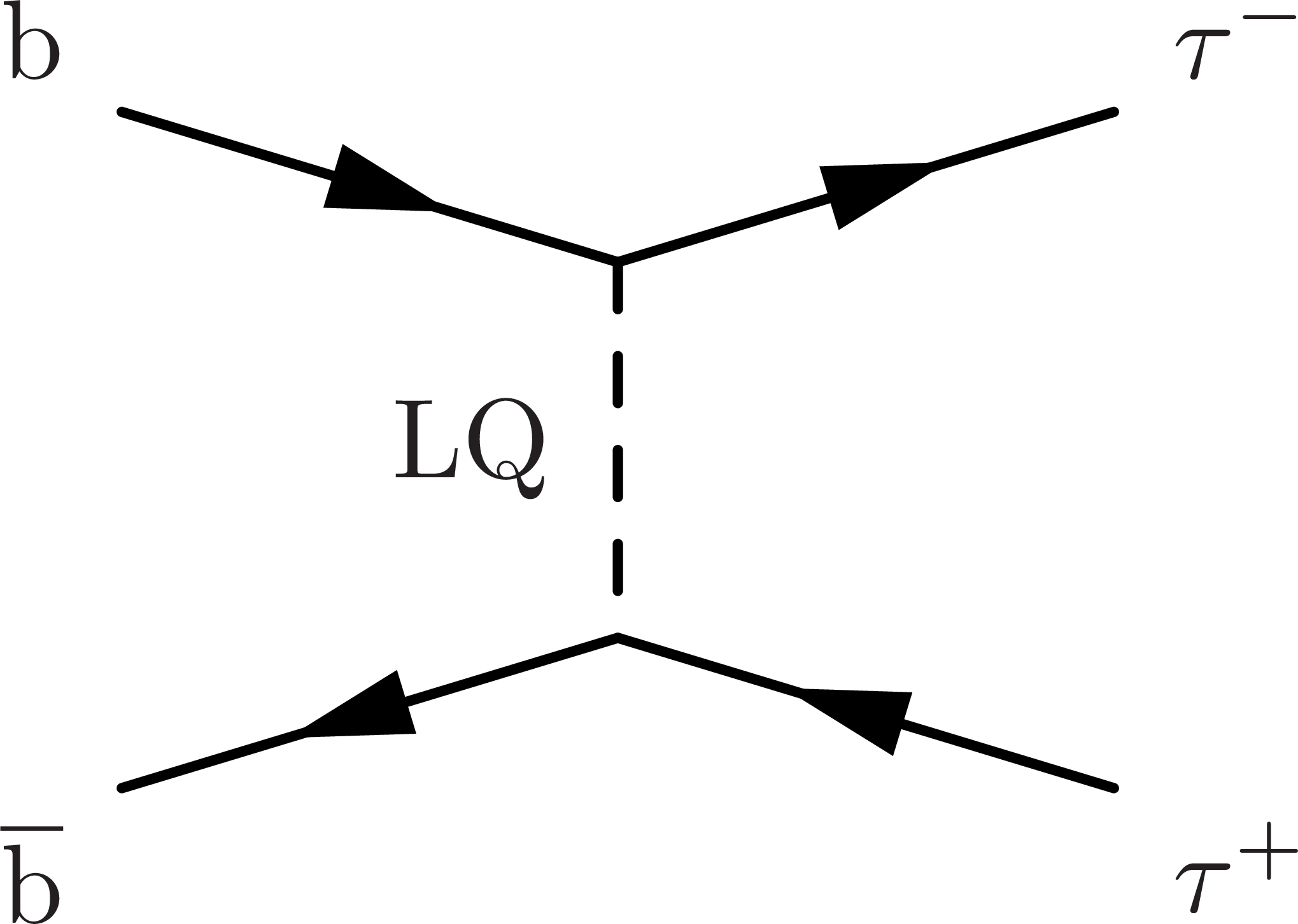
png pdf |
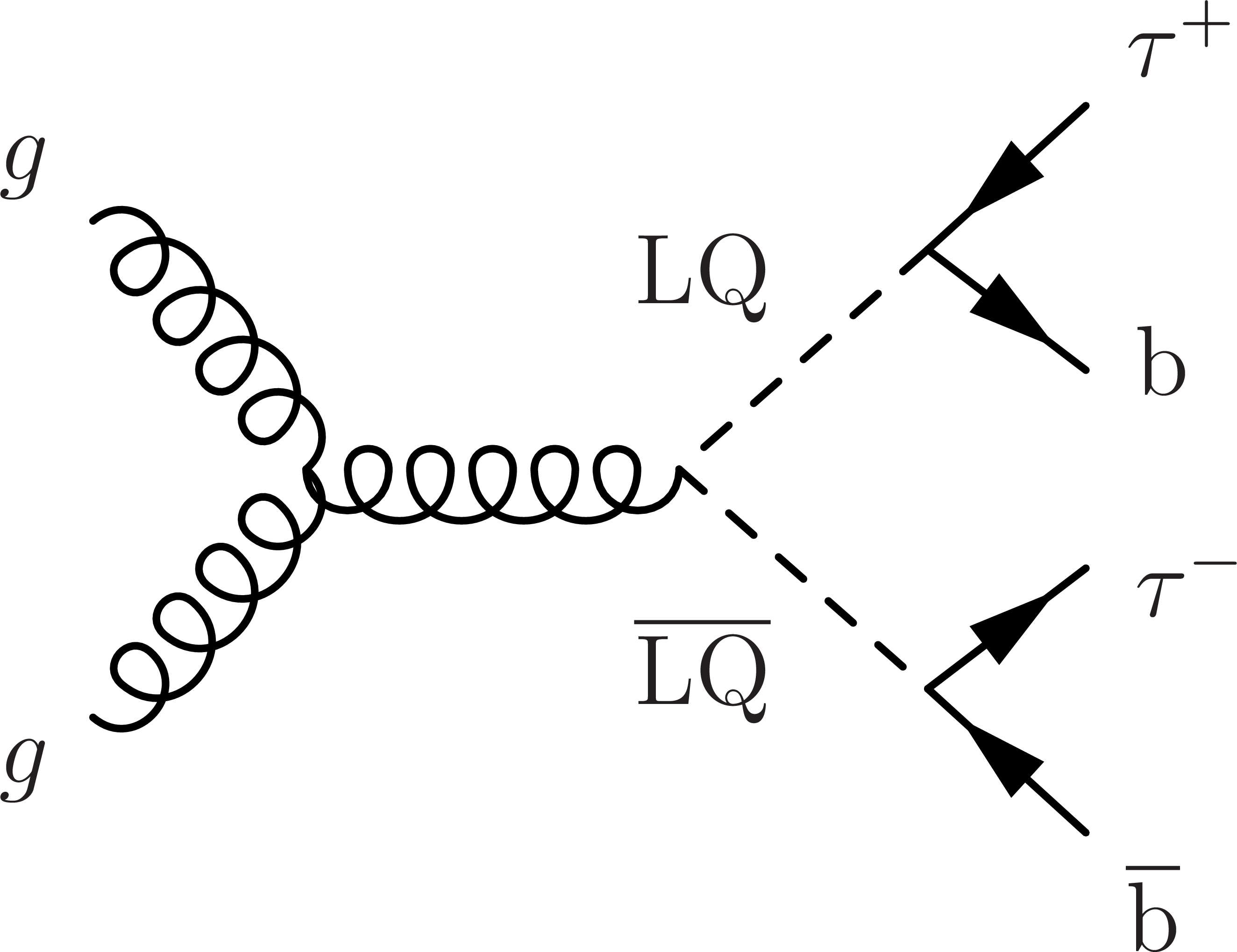
Figure 1-c:
Example Feynman diagram at leading order of nonresonant production of two $ \tau $ leptons via $ t $-channel LQ exchange. |
png pdf |
Figure 2:
Product of acceptance and efficiency for a vector LQ signal in the $ \tau_\mathrm{h}\tau_\mathrm{h} $ (left) and $ \mu\tau_\mathrm{h} $ (right) channels of the 0b and $ \geq $1b (upper), and the 0j categories (lower). The resonant LQ signals are neglected in the 0j category. Vertical bars (only just visible) indicate the statistical uncertainty. |
png pdf |
Figure 2-a:
Product of acceptance and efficiency for a vector LQ signal in the $ \tau_\mathrm{h}\tau_\mathrm{h} $ channel of the 0b and $ \geq $1b categories. Vertical bars (only just visible) indicate the statistical uncertainty. |
png pdf |
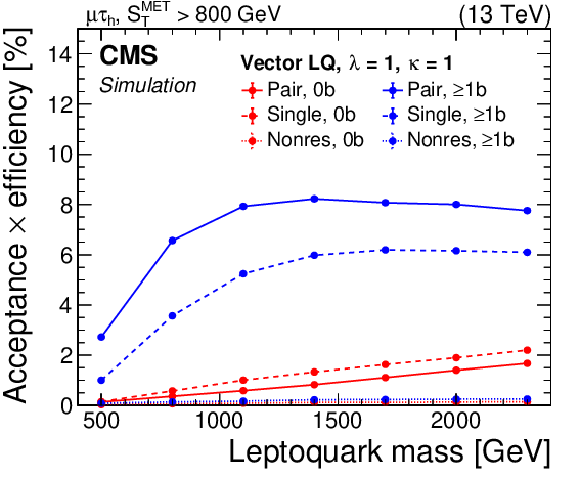
Figure 2-b:
Product of acceptance and efficiency for a vector LQ signal in the $ \mu\tau_\mathrm{h} $ channel of the 0b and $ \geq $1b categories. Vertical bars (only just visible) indicate the statistical uncertainty. |
png pdf |
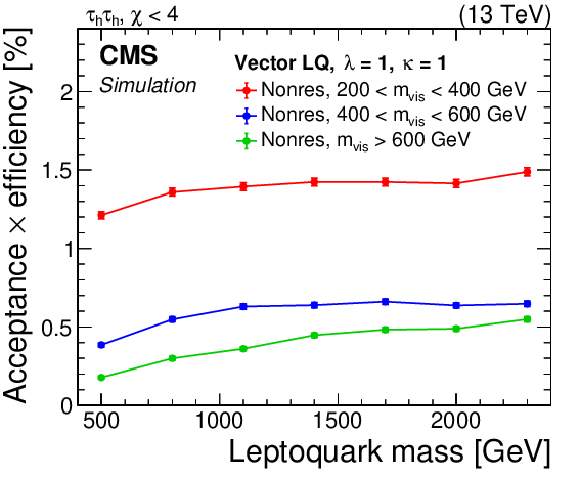
Figure 2-c:
Product of acceptance and efficiency for a vector LQ signal in the $ \tau_\mathrm{h}\tau_\mathrm{h} $ channel of the 0j category. The resonant LQ signals are neglected. Vertical bars (only just visible) indicate the statistical uncertainty. |
png pdf |
Figure 2-d:
Product of acceptance and efficiency for a vector LQ signal in the $ \mu\tau_\mathrm{h} $ channel of the 0j category. The resonant LQ signals are neglected. Vertical bars (only just visible) indicate the statistical uncertainty. |
png pdf |
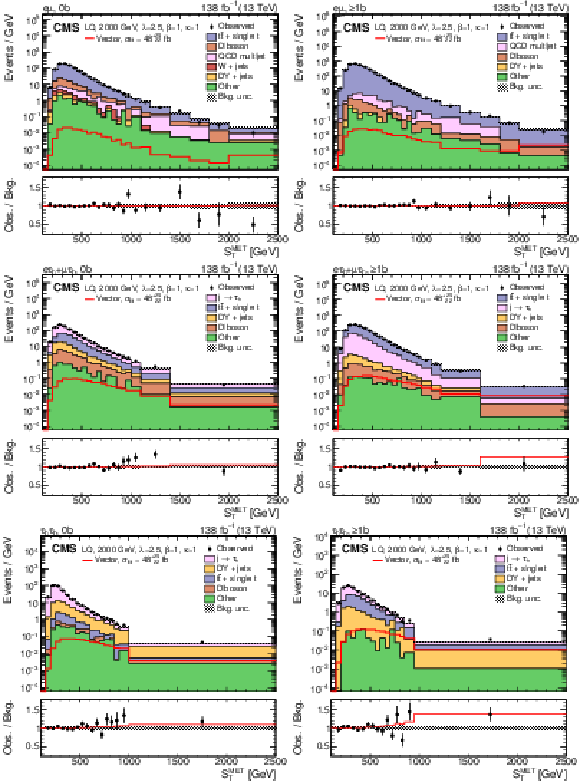
Figure 3:
Postfit distributions of $ S_\mathrm{T}^\text{MET} $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \mathrm{e}\mu $ (top), $ \ell\tau_\mathrm{h} $ (center), and $ \tau_\mathrm{h}\tau_\mathrm{h} $ (bottom) channels in the 0b (left) and $ \geq $1b (right) categories are shown. The fitted signal distribution for the total vector LQ signal (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. In each distribution, the lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
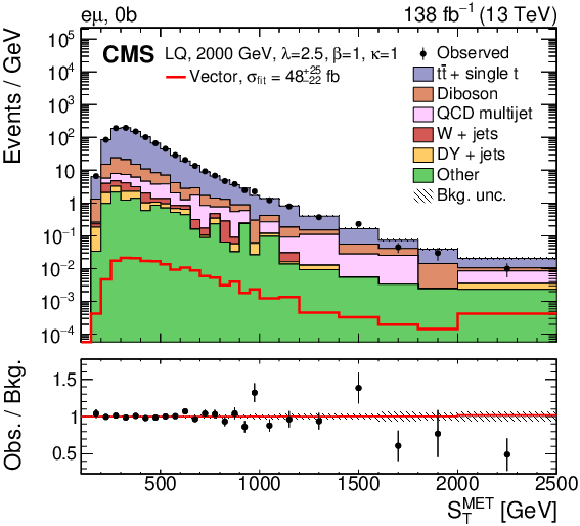
Figure 3-a:
Postfit distribution of $ S_\mathrm{T}^\text{MET} $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \mathrm{e}\mu $ channel in the 0b category is shown. The fitted signal distribution for the total vector LQ signal (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
Figure 3-b:
Postfit distribution of $ S_\mathrm{T}^\text{MET} $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \mathrm{e}\mu $ channel in the $ \geq $1b category is shown. The fitted signal distribution for the total vector LQ signal (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
Figure 3-c:
Postfit distribution of $ S_\mathrm{T}^\text{MET} $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \ell\tau_\mathrm{h} $ channel in the 0b category is shown. The fitted signal distribution for the total vector LQ signal (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
Figure 3-d:
Postfit distribution of $ S_\mathrm{T}^\text{MET} $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \ell\tau_\mathrm{h} $ channel in the $ \geq $1b category is shown. The fitted signal distribution for the total vector LQ signal (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
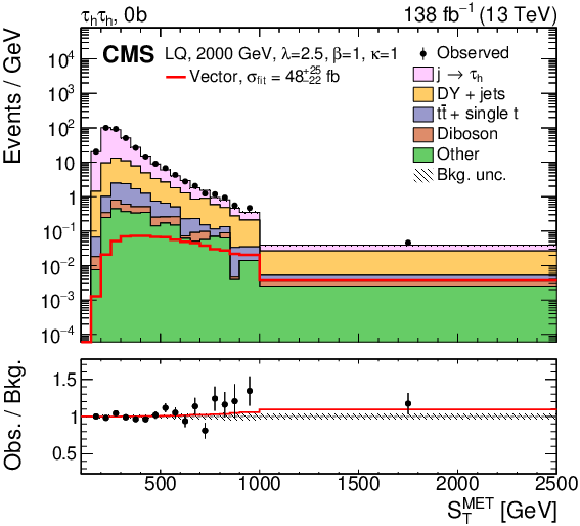
Figure 3-e:
Postfit distribution of $ S_\mathrm{T}^\text{MET} $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \tau_\mathrm{h}\tau_\mathrm{h} $ channel in the 0b category is shown. The fitted signal distribution for the total vector LQ signal (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
Figure 3-f:
Postfit distribution of $ S_\mathrm{T}^\text{MET} $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \tau_\mathrm{h}\tau_\mathrm{h} $ channel in the $ \geq $1b category is shown. The fitted signal distribution for the total vector LQ signal (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
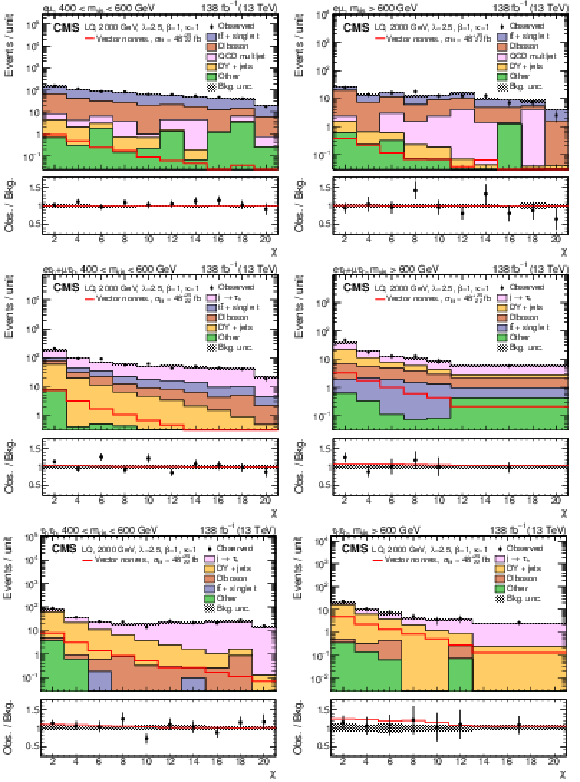
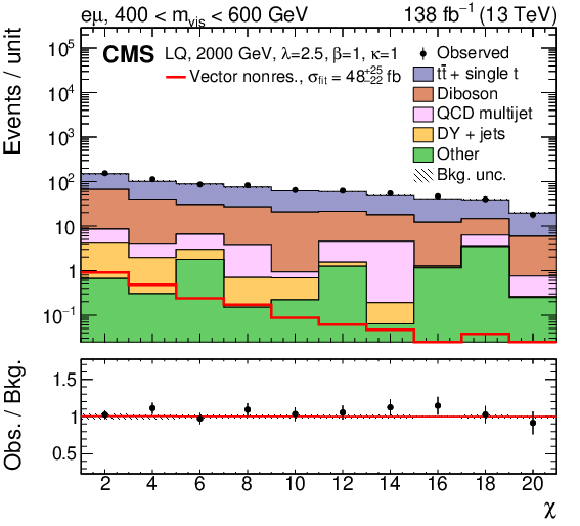
Figure 4:
Postfit distributions of $ \chi $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \mathrm{e}\mu $ (top), $ \ell\tau_\mathrm{h} $ (center), and $ \tau_\mathrm{h}\tau_\mathrm{h} $ (bottom) channels in the 400 $ < m_{\text{vis}} < $ 600 GeV (left) and $ m_{\text{vis}} > $ 600 GeV (right) categories are shown. The fitted signal distribution for the nonresonant vector LQ model (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The contribution from resonant LQ production is neglected. In each distribution, the lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
Figure 4-a:
Postfit distributions of $ \chi $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \mathrm{e}\mu $ channel in the 400 $ < m_{\text{vis}} < $ 600 GeV category is shown. The fitted signal distribution for the nonresonant vector LQ model (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The contribution from resonant LQ production is neglected. The lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
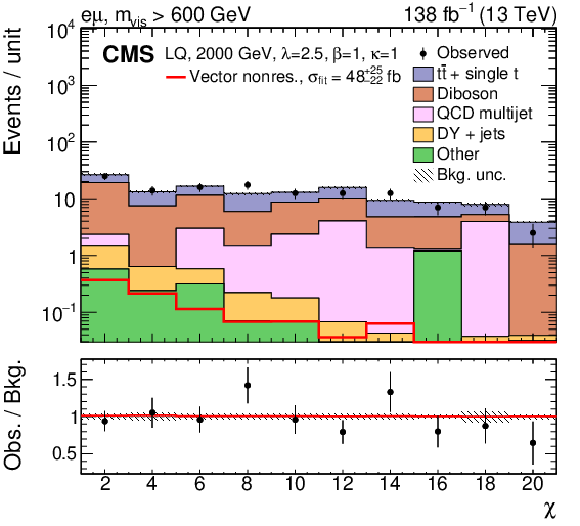
Figure 4-b:
Postfit distributions of $ \chi $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \mathrm{e}\mu $ channel in the $ m_{\text{vis}} > $ 600 GeV category is shown. The fitted signal distribution for the nonresonant vector LQ model (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The contribution from resonant LQ production is neglected. The lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
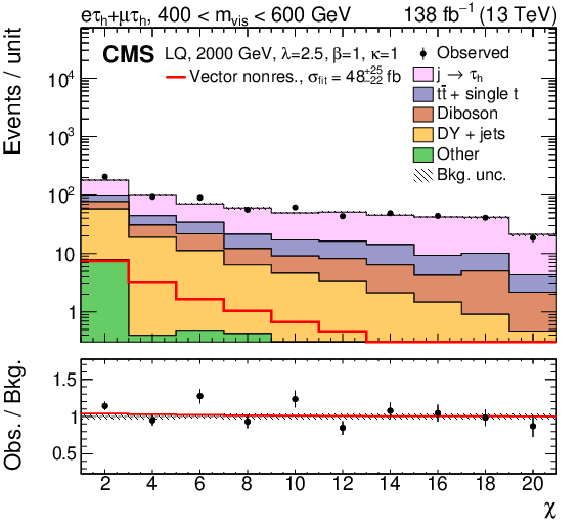
Figure 4-c:
Postfit distributions of $ \chi $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \ell\tau_\mathrm{h} $ channel in the 400 $ < m_{\text{vis}} < $ 600 GeV category is shown. The fitted signal distribution for the nonresonant vector LQ model (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The contribution from resonant LQ production is neglected. The lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
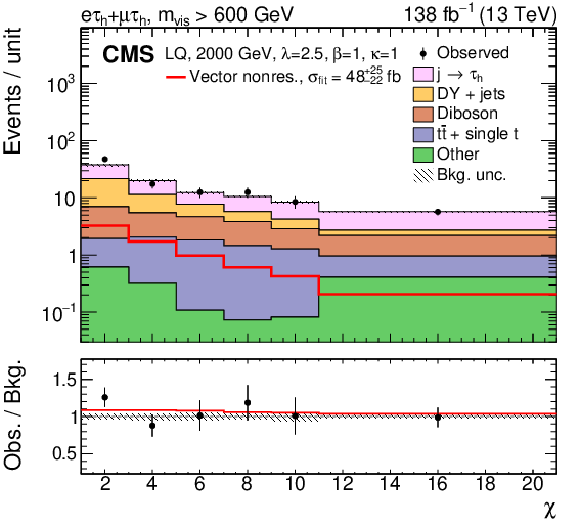
Figure 4-d:
Postfit distributions of $ \chi $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \ell\tau_\mathrm{h} $ channel in the $ m_{\text{vis}} > $ 600 GeV category is shown. The fitted signal distribution for the nonresonant vector LQ model (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The contribution from resonant LQ production is neglected. The lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
Figure 4-e:
Postfit distributions of $ \chi $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \tau_\mathrm{h}\tau_\mathrm{h} $ channel in the 400 $ < m_{\text{vis}} < $ 600 GeV category is shown. The fitted signal distribution for the nonresonant vector LQ model (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The contribution from resonant LQ production is neglected. The lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
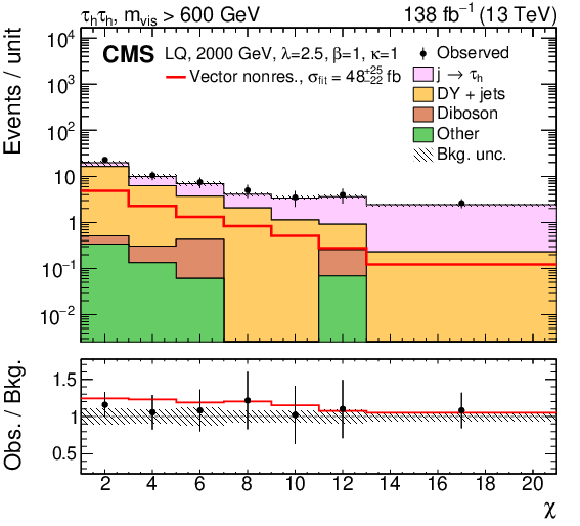
Figure 4-f:
Postfit distributions of $ \chi $ for the combined 2016-2018 data set after a simultaneous fit of the background and vector LQ signal to the data. The last bin includes the overflow. The $ \tau_\mathrm{h}\tau_\mathrm{h} $ channel in the $ m_{\text{vis}} > $ 600 GeV category is shown. The fitted signal distribution for the nonresonant vector LQ model (red line) with a mass of 2000 GeV, $ \lambda = $ 2.5, and $ \kappa= $ 1 is overlaid. The contribution from resonant LQ production is neglected. The lower panel shows the ratio of the data (black markers) or the sum of the postfit signal and background (red line) to the postfit background. The hatched band indicates the total postfit uncertainty in the background. |
png pdf |
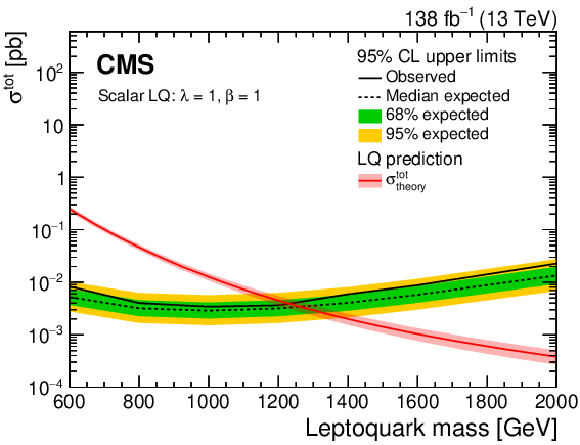
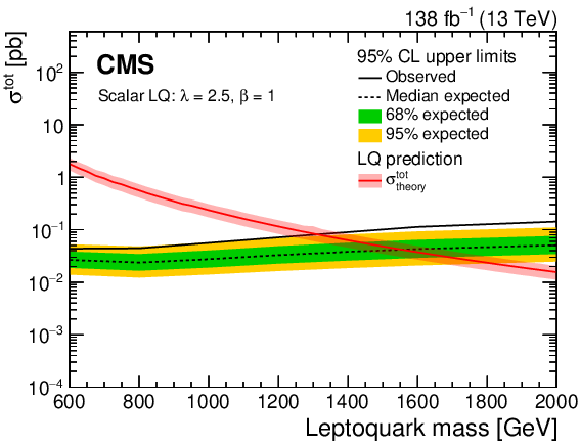
Figure 5:
Observed and expected upper limit on the total cross section of a scalar LQ signal with $ \lambda= $ 1 (left) and 2.5 (right) at the 95% CL under the assumption of exclusive LQ couplings to b quarks and $ \tau $ leptons. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The red line shows the cross section with the shaded band indicating the theoretical uncertainties. |
png pdf |
Figure 5-a:
Observed and expected upper limit on the total cross section of a scalar LQ signal with $ \lambda= $ 1 at the 95% CL under the assumption of exclusive LQ couplings to b quarks and $ \tau $ leptons. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The red line shows the cross section with the shaded band indicating the theoretical uncertainties. |
png pdf |
Figure 5-b:
Observed and expected upper limit on the total cross section of a scalar LQ signal with $ \lambda= $ 2.5 at the 95% CL under the assumption of exclusive LQ couplings to b quarks and $ \tau $ leptons. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The red line shows the cross section with the shaded band indicating the theoretical uncertainties. |
png pdf |
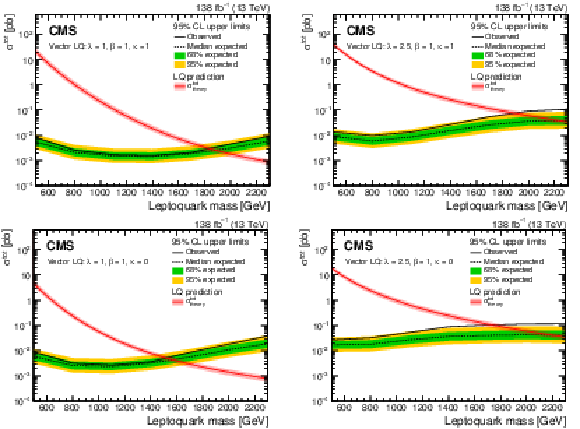
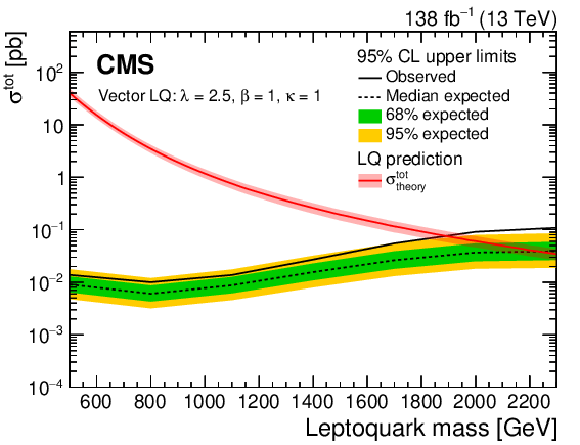
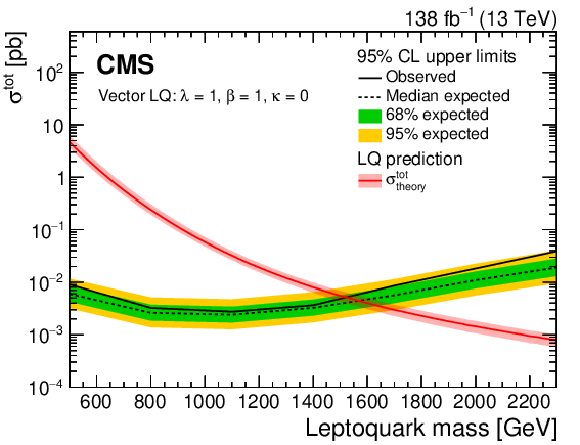
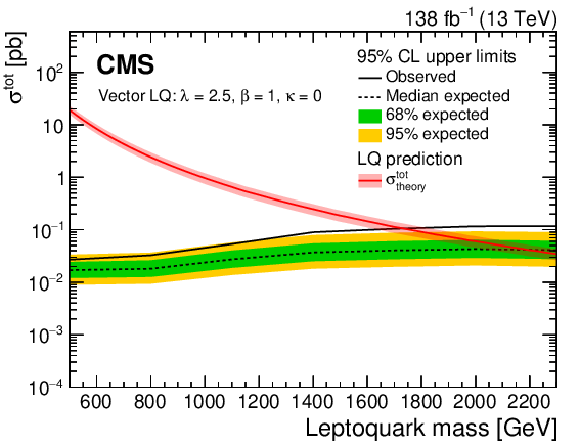
Figure 6:
Observed and expected upper limit on the total cross section of a vector LQ signal with $ \lambda= $ 1 (left) and 2.5 (right) at the 95% CL under the assumption of exclusive LQ couplings to b quarks and $ \tau $ leptons. The upper (lower) row assumes a coupling parameter $ \kappa= $ 1 (0). The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The red line shows the cross section calculated at LO with the shaded band indicating the theoretical uncertainties. |
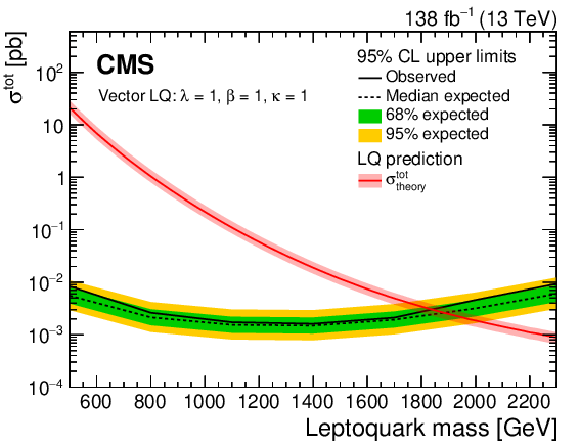
png pdf |
Figure 6-a:
Observed and expected upper limit on the total cross section of a vector LQ signal with $ \lambda= $ 1 at the 95% CL under the assumption of exclusive LQ couplings to b quarks and $ \tau $ leptons. A coupling parameter $ \kappa= $ 1 is assumed. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The red line shows the cross section calculated at LO with the shaded band indicating the theoretical uncertainties. |
png pdf |
Figure 6-b:
Observed and expected upper limit on the total cross section of a vector LQ signal with $ \lambda= $ 2.5 at the 95% CL under the assumption of exclusive LQ couplings to b quarks and $ \tau $ leptons. A coupling parameter $ \kappa= $ 1 is assumed. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The red line shows the cross section calculated at LO with the shaded band indicating the theoretical uncertainties. |
png pdf |
Figure 6-c:
Observed and expected upper limit on the total cross section of a vector LQ signal with $ \lambda= $ 1 at the 95% CL under the assumption of exclusive LQ couplings to b quarks and $ \tau $ leptons. A coupling parameter $ \kappa= $ 0 is assumed. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The red line shows the cross section calculated at LO with the shaded band indicating the theoretical uncertainties. |
png pdf |
Figure 6-d:
Observed and expected upper limit on the total cross section of a vector LQ signal with $ \lambda= $ 2.5 at the 95% CL under the assumption of exclusive LQ couplings to b quarks and $ \tau $ leptons. A coupling parameter $ \kappa= $ 0 is assumed. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The red line shows the cross section calculated at LO with the shaded band indicating the theoretical uncertainties. |
png pdf |
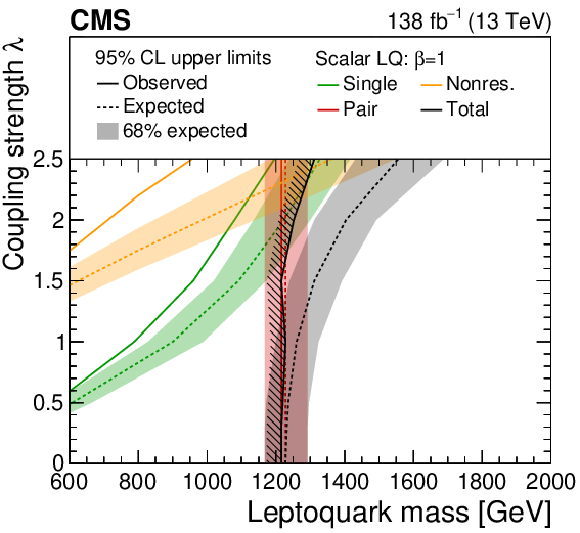
Figure 7:
Observed and expected upper limit at the 95% CL on the coupling strength $ \lambda $ of a scalar LQ under the assumption of exclusive LQ couplings to b quarks and $ \tau $ leptons. The limits derived for the single (green), pair (red), nonresonant (orange), and total LQ production (black) are shown. The shaded bands around the expected limit lines correspond to the regions containing 68% of the distribution of limits expected under the background-only hypothesis. The hatches indicate the excluded side of the parameter space with respect to the combined observed limit. |
png pdf |
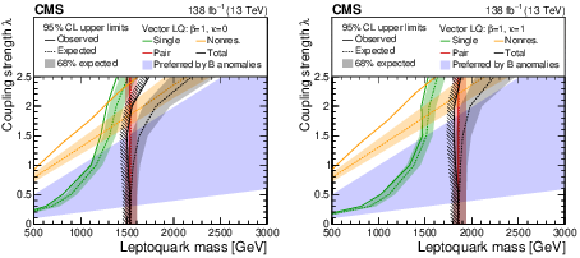
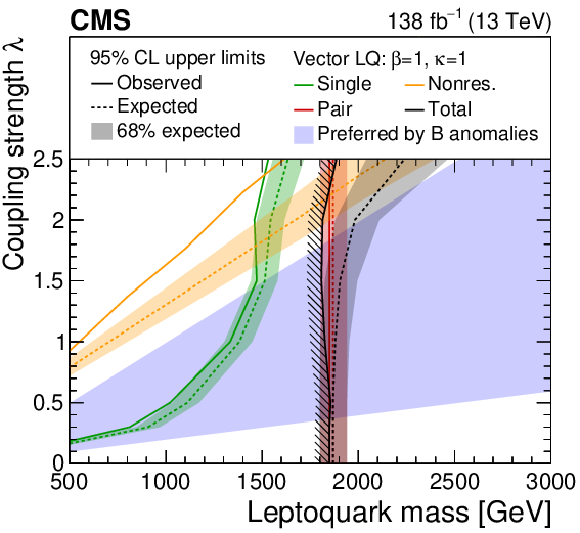
Figure 8:
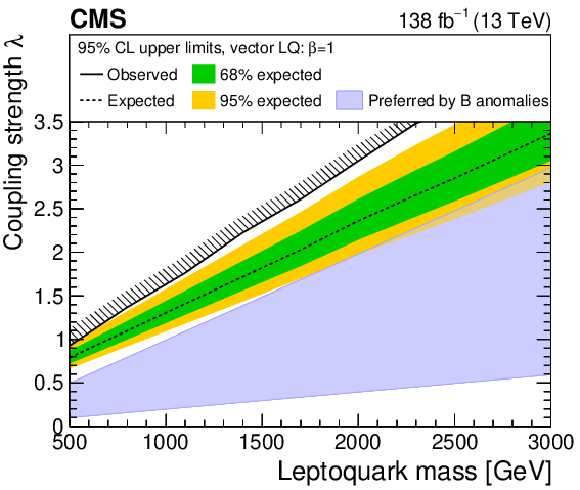
Observed and expected upper limit at the 95% CL on the coupling strength $ \lambda $ of a vector LQ model with $ \kappa= $ 0 (left) and $ \kappa= $ 1 (right) under the assumption of exclusive LQ couplings to b quarks and $ \tau $ leptons. The limits derived for the single (green), pair (red), nonresonant (orange), and total LQ production (black) are shown. The shaded bands around the expected limit lines correspond to the regions containing 68% of the distribution of limits expected under the background-only hypothesis. The hatches indicate the excluded side of the parameter space with respect to the combined observed limit. The region with blue shading shows the parameter space preferred by one of the models proposed to explain the B physics anomalies [61]. |
png pdf |
Figure 8-a:
Observed and expected upper limit at the 95% CL on the coupling strength $ \lambda $ of a vector LQ model with $ \kappa= $ 0 under the assumption of exclusive LQ couplings to b quarks and $ \tau $ leptons. The limits derived for the single (green), pair (red), nonresonant (orange), and total LQ production (black) are shown. The shaded bands around the expected limit lines correspond to the regions containing 68% of the distribution of limits expected under the background-only hypothesis. The hatches indicate the excluded side of the parameter space with respect to the combined observed limit. The region with blue shading shows the parameter space preferred by one of the models proposed to explain the B physics anomalies [61]. |
png pdf |
Figure 8-b:
Observed and expected upper limit at the 95% CL on the coupling strength $ \lambda $ of a vector LQ model with $ \kappa= $ 1 under the assumption of exclusive LQ couplings to b quarks and $ \tau $ leptons. The limits derived for the single (green), pair (red), nonresonant (orange), and total LQ production (black) are shown. The shaded bands around the expected limit lines correspond to the regions containing 68% of the distribution of limits expected under the background-only hypothesis. The hatches indicate the excluded side of the parameter space with respect to the combined observed limit. The region with blue shading shows the parameter space preferred by one of the models proposed to explain the B physics anomalies [61]. |
png pdf |
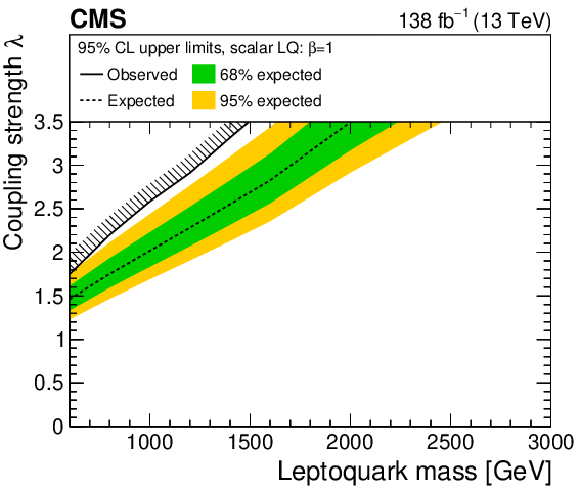
Figure 9:
Observed and expected upper limit at the 95% CL on the coupling strength $ \lambda $ of a scalar (left) and vector LQ model (right) determined by considering only the nonresonant production of two $ \tau $ leptons through $ t $-channel LQ exchange. Exclusive LQ couplings to b quarks and $ \tau $ leptons are assumed. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The hatches indicate the excluded side of the parameter space with respect to the observed limit. The region with blue shading shows the parameter space preferred by one of the models proposed to explain the B physics anomalies [61]. |
png pdf |
Figure 9-a:
Observed and expected upper limit at the 95% CL on the coupling strength $ \lambda $ of a scalar LQ model determined by considering only the nonresonant production of two $ \tau $ leptons through $ t $-channel LQ exchange. Exclusive LQ couplings to b quarks and $ \tau $ leptons are assumed. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The hatches indicate the excluded side of the parameter space with respect to the observed limit. The region with blue shading shows the parameter space preferred by one of the models proposed to explain the B physics anomalies [61]. |
png pdf |
Figure 9-b:
Observed and expected upper limit at the 95% CL on the coupling strength $ \lambda $ of a vector LQ model determined by considering only the nonresonant production of two $ \tau $ leptons through $ t $-channel LQ exchange. Exclusive LQ couplings to b quarks and $ \tau $ leptons are assumed. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The hatches indicate the excluded side of the parameter space with respect to the observed limit. The region with blue shading shows the parameter space preferred by one of the models proposed to explain the B physics anomalies [61]. |
| Tables | |
png pdf |
Table 1:
The sources of uncertainty considered, categorized as to whether they affect the rate or shape of the distributions. ``s.d.'' refers to the standard deviation of the input variable and ``(mis)ID'' stands for ``(mis)identification''. |
png pdf |
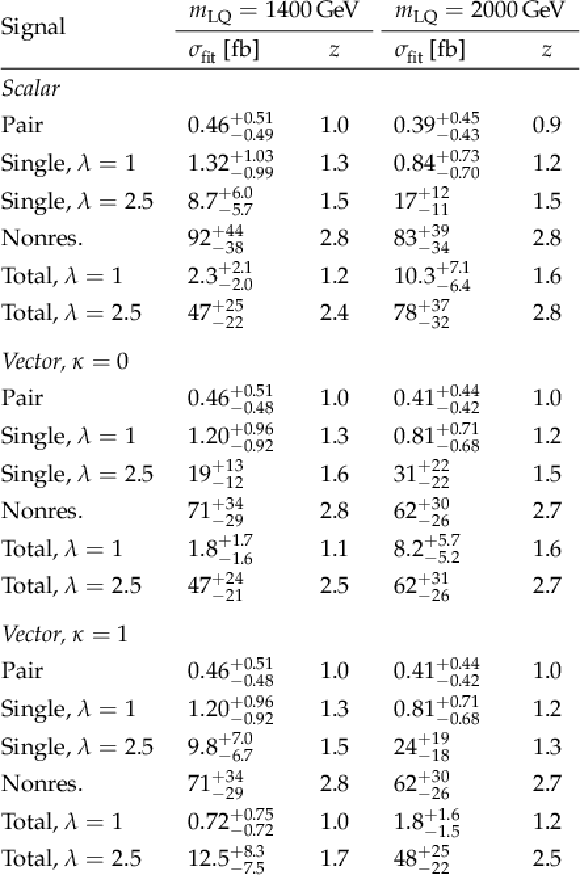
Table 2:
Best-fit LQ cross sections $ \sigma_\text{fit} $ for various masses and coupling strengths $ \lambda $, and the corresponding local significance $ z $ (given in standard deviations) for different production modes individually, as well as their combination. The look-elsewhere effect is negligible. |
| Summary |
| A search has been presented for a third-generation leptoquark (LQ) decaying to a $ \tau $ lepton and a b quark. Events with $ \tau $ leptons and a varying number of jets originating from b quarks are considered, targeting the single and pair production of the LQs, as well as the nonresonant production of two $ \tau $ leptons through $ t $-channel LQ exchange. The search uses proton-proton collision data at a center-of-mass energy of 13 TeV recorded with the CMS detector and corresponding to an integrated luminosity of 138 fb$ ^{-1} $. Upper limits are set on third-generation scalar and vector LQ production cross sections as a function of LQ mass, and results are compared with theoretical predictions to obtain lower limits on the LQ mass. At 95% confidence level, third-generation LQs decaying to a $ \tau $ lepton and a b quark with unit coupling ($ \lambda= $ 1) are excluded for masses below 1.22 TeV for a scalar model, and below 1.50 (1.82) TeV for a vector model with a coupling parameter $ \kappa= $ 0 (1). For $ \lambda= $ 2.5 the lower limits are 1.31 TeV for a scalar model, and 1.73 (1.88) TeV for a vector model with $ \kappa= $ 0 (1). The study of nonresonant $ \tau\tau $ production through $ t $-channel LQ exchange allows lower limits on the LQ mass of up to 2.3 TeV to be obtained. Upper limits are also set on the coupling strengths of scalar and vector LQs as functions of their mass. The observed data are found to agree with the standard model expectation within 2 standard deviations below a coupling strength of $ \lambda= $ 1.5. For a benchmark LQ model with a mass of 2 TeV and a coupling strength of 2.5, the data show an excess with a local significance of 2.8 standard deviations above the standard model expectation. Consequently, the observed upper limits on the LQ production cross section are about three times larger than expected for this benchmark. The present excess is driven by events with at least one highly energetic jet but no b-tagged jets, indicating the need for future similar analyses to consider alternative signal models. |
| References | ||||
| 1 | ATLAS Collaboration | Observation of a new particle in the search for the standard model Higgs boson with the ATLAS detector at the LHC | PLB 716 (2012) 1 | 1207.7214 |
| 2 | CMS Collaboration | Observation of a new boson at a mass of 125 GeV with the CMS experiment at the LHC | PLB 716 (2012) 30 | CMS-HIG-12-028 1207.7235 |
| 3 | CMS Collaboration | Observation of a new boson with mass near 125 GeV in pp collisions at $ \sqrt{s} $ = 7 and 8 TeV | JHEP 06 (2013) 081 | CMS-HIG-12-036 1303.4571 |
| 4 | BaBar Collaboration | Evidence for an excess of $ \bar{B} \to D^{(*)} \tau^-\bar{\nu}_\tau $ decays | PRL 109 (2012) 101802 | 1205.5442 |
| 5 | BaBar Collaboration | Measurement of an excess of $ \bar{B} \to D^{(*)}\tau^- \bar{\nu}_\tau $ decays and implications for charged Higgs bosons | PRD 88 (2013) 072012 | 1303.0571 |
| 6 | Belle Collaboration | Observation of $ B^0 \to D^{*-} \tau^+ \nu_\tau $ decay at Belle | PRL 99 (2007) 191807 | 0706.4429 |
| 7 | Belle Collaboration | Observation of $ B^+ \to \bar{D}^{*0} \tau^+ \nu_\tau $ and evidence for $ B^+ \to \bar{D}^0 \tau^+ \nu_\tau $ at Belle | PRD 82 (2010) 072005 | 1005.2302 |
| 8 | Belle Collaboration | Measurement of the branching ratio of $ \bar{B} \to D^{(\ast)} \tau^- \bar{\nu}_\tau $ relative to $ \bar{B} \to D^{(\ast)} \ell^- \bar{\nu}_\ell $ decays with hadronic tagging at Belle | PRD 92 (2015) 072014 | 1507.03233 |
| 9 | Belle Collaboration | Measurement of the branching ratio of $ \bar{B}^0 \rightarrow D^{*+} \tau^- \bar{\nu}_{\tau} $ relative to $ \bar{B}^0 \rightarrow D^{*+} \ell^- \bar{\nu}_{\ell} $ decays with a semileptonic tagging method | PRD 94 (2016) 072007 | 1607.07923 |
| 10 | Belle Collaboration | Measurement of the $ \tau $ lepton polarization and $ R(D^*) $ in the decay $ \bar{B} \to D^* \tau^- \bar{\nu}_\tau $ | PRL 118 (2017) 211801 | 1612.00529 |
| 11 | Belle Collaboration | Measurement of the $ \tau $ lepton polarization and $ R(D^*) $ in the decay $ \bar{B} \rightarrow D^* \tau^- \bar{\nu}_\tau $ with one-prong hadronic $ \tau $ decays at Belle | PRD 97 (2018) 012004 | 1709.00129 |
| 12 | LHCb Collaboration | Measurement of the ratio of branching fractions $ \mathcal{B}(\bar{B}^0 \to D^{*+}\tau^{-}\bar{\nu}_{\tau})/\mathcal{B}(\bar{B}^0 \to D^{*+}\mu^{-}\bar{\nu}_{\mu}) $ | PRL 115 (2015) 111803 | 1506.08614 |
| 13 | LHCb Collaboration | Measurement of the ratio of the $ B^0 \to D^{*-} \tau^+ \nu_{\tau} $ and $ B^0 \to D^{*-} \mu^+ \nu_{\mu} $ branching fractions using three-prong $ \tau $-lepton decays | PRL 120 (2018) 171802 | 1708.08856 |
| 14 | LHCb Collaboration | Test of lepton flavor universality by the measurement of the $ B^0 \to D^{*-} \tau^+ \nu_{\tau} $ branching fraction using three-prong $ \tau $ decays | PRD 97 (2018) 072013 | 1711.02505 |
| 15 | LHCb Collaboration | Measurement of the ratios of branching fractions $ \mathcal{R}(D^{*}) $ and $ \mathcal{R}(D^{0}) $ | Submitted to PRL, 2023 | 2302.02886 |
| 16 | LHCb Collaboration | Test of lepton flavor universality using $ B^0 \rightarrow D^{*-}\tau^+\nu_\tau $ decays with hadronic $ \tau $ channels | PRD 108 (2023) 012018 | 2305.01463 |
| 17 | HFLAV Collaboration | Averages of b-hadron, c-hadron, and $\tau$-lepton properties as of 2021 | PRD 107 (2023) 052008 | 2206.07501 |
| 18 | J. C. Pati and A. Salam | Unified lepton-hadron symmetry and a gauge theory of the basic interactions | PRD 8 (1973) 1240 | |
| 19 | H. Georgi and S. L. Glashow | Unity of all elementary particle forces | PRL 32 (1974) 438 | |
| 20 | J. C. Pati and A. Salam | Lepton number as the fourth color | PRD 10 (1974) 275 | |
| 21 | H. Fritzsch and P. Minkowski | Unified interactions of leptons and hadrons | Annals Phys. 93 (1975) 193 | |
| 22 | G. Senjanovic and A. Sokorac | Light leptoquarks in SO(10) | Z. Phys. C 20 (1983) 255 | |
| 23 | P. H. Frampton and B.-H. Lee | SU(15) grand unification | PRL 64 (1990) 619 | |
| 24 | P. H. Frampton and T. W. Kephart | Higgs sector and proton decay in SU(15) grand unification | PRD 42 (1990) 3892 | |
| 25 | H. Murayama and T. Yanagida | A viable SU(5) GUT with light leptoquark bosons | Mod. Phys. Lett. A 7 (1992) 147 | |
| 26 | S. Dimopoulos and L. Susskind | Mass without scalars | NPB 155 (1979) 237 | |
| 27 | S. Dimopoulos | Technicolored signatures | NPB 168 (1980) 69 | |
| 28 | E. Farhi and L. Susskind | Technicolor | Phys. Rept. 74 (1981) 277 | |
| 29 | L. F. Abbott and E. Farhi | Are the weak interactions strong? | PLB 101 (1981) 69 | |
| 30 | B. Schrempp and F. Schrempp | Light leptoquarks | PLB 153 (1985) 101 | |
| 31 | J. Wudka | Composite leptoquarks | PLB 167 (1986) 337 | |
| 32 | M. Tanaka and R. Watanabe | New physics in the weak interaction of $ \bar B \to D^{(*)}\tau\bar\nu $ | PRD 87 (2013) 034028 | 1212.1878 |
| 33 | Y. Sakaki, M. Tanaka, A. Tayduganov, and R. Watanabe | Testing leptoquark models in $ \bar B \to D^{(*)} \tau \bar\nu $ | PRD 88 (2013) 094012 | 1309.0301 |
| 34 | I. Doršner, S. Fajfer, N. Košnik, and I. Nišandžić | Minimally flavored colored scalar in $ \bar B \to D^{(*)} \tau \bar \nu $ and the mass matrices constraints | JHEP 11 (2013) 084 | 1306.6493 |
| 35 | B. Gripaios, M. Nardecchia, and S. A. Renner | Composite leptoquarks and anomalies in $ B $-meson decays | JHEP 05 (2015) 006 | 1412.1791 |
| 36 | R. Alonso, B. Grinstein, and J. Martin Camalich | Lepton universality violation and lepton flavor conservation in $ B $-meson decays | JHEP 10 (2015) 184 | 1505.05164 |
| 37 | L. Calibbi, A. Crivellin, and T. Ota | Effective field theory approach to $ b\to s\ell\ell^{(')} $, $ B\to K^{(*)}\nu\overline{\nu} $ and $ B\to D^{(*)}\tau\nu $ with third generation couplings | PRL 115 (2015) 181801 | 1506.02661 |
| 38 | M. Bauer and M. Neubert | Minimal leptoquark explanation for the $ R_{D^{(*)}} $, $ R_K $, and $ (g-2)_\mu $ anomalies | PRL 116 (2016) 141802 | 1511.01900 |
| 39 | R. Barbieri, G. Isidori, A. Pattori, and F. Senia | Anomalies in $ B $-decays and $ U(2) $ flavour symmetry | EPJC 76 (2016) 67 | 1512.01560 |
| 40 | I. Doršner et al. | Physics of leptoquarks in precision experiments and at particle colliders | Phys. Rept. 641 (2016) 1 | 1603.04993 |
| 41 | B. Dumont, K. Nishiwaki, and R. Watanabe | LHC constraints and prospects for $ S_1 $ scalar leptoquark explaining the $ \bar B \to D^{(*)} \tau \bar\nu $ anomaly | PRD 94 (2016) 034001 | 1603.05248 |
| 42 | E. Coluccio Leskow, G. DAmbrosio, A. Crivellin, and D. Muller | $ (g-2)_\mu $, lepton flavor violation, and Z decays with leptoquarks: correlations and future prospects | PRD 95 (2017) 055018 | 1612.06858 |
| 43 | D. Bečirević and O. Sumensari | A leptoquark model to accommodate $ R_K^\mathrm{exp} < R_K^\mathrm{SM} $ and $ R_{K^\ast}^\mathrm{exp} < R_{K^\ast}^\mathrm{SM} $ | JHEP 08 (2017) 104 | 1704.05835 |
| 44 | A. Crivellin, D. Müller, and T. Ota | Simultaneous explanation of $ R(D^{(*)}) $ and $ \mathrm{b}\to\mathrm{s}\mu^{+}\mu^{-} $: the last scalar leptoquarks standing | JHEP 09 (2017) 040 | 1703.09226 |
| 45 | D. Buttazzo, A. Greljo, G. Isidori, and D. Marzocca | B-physics anomalies: a guide to combined explanations | JHEP 11 (2017) 044 | 1706.07808 |
| 46 | G. Hiller and I. Nišandžić | $ R_K $ and $ R_{K^{\ast}} $ beyond the standard model | PRD 96 (2017) 035003 | 1704.05444 |
| 47 | I. Doršner, S. Fajfer, D. A. Faroughy, and N. Košnik | The role of the $ S_3 $ GUT leptoquark in flavor universality and collider searches | JHEP 10 (2017) 188 | 1706.07779 |
| 48 | L. Di Luzio, A. Greljo, and M. Nardecchia | Gauge leptoquark as the origin of B-physics anomalies | PRD 96 (2017) 115011 | 1708.08450 |
| 49 | L. Calibbi, A. Crivellin, and T. Li | Model of vector leptoquarks in view of the $ B $-physics anomalies | PRD 98 (2018) 115002 | 1709.00692 |
| 50 | M. Bordone, C. Cornella, J. Fuentes-Martín, and G. Isidori | A three-site gauge model for flavor hierarchies and flavor anomalies | PLB 779 (2018) 317 | 1712.01368 |
| 51 | G. Hiller, D. Loose, and I. Nišandžić | Flavorful leptoquarks at hadron colliders | PRD 97 (2018) 075004 | 1801.09399 |
| 52 | D. Bečirević et al. | Scalar leptoquarks from grand unified theories to accommodate the $ B $-physics anomalies | PRD 98 (2018) 055003 | 1806.05689 |
| 53 | L. Di Luzio et al. | Maximal flavour violation: a Cabibbo mechanism for leptoquarks | JHEP 11 (2018) 081 | 1808.00942 |
| 54 | R. Barbieri and A. Tesi | $ B $-decay anomalies in Pati-Salam SU(4) | EPJC 78 (2018) 193 | 1712.06844 |
| 55 | D. Marzocca | Addressing the B-physics anomalies in a fundamental composite Higgs model | JHEP 07 (2018) 121 | 1803.10972 |
| 56 | A. Angelescu, D. Bečirević , D. A. Faroughy, and O. Sumensari | Closing the window on single leptoquark solutions to the $ B $-physics anomalies | JHEP 10 (2018) 183 | 1808.08179 |
| 57 | J. Kumar, D. London, and R. Watanabe | Combined explanations of the $ b \to s \mu^+ \mu^- $ and $ b \to c \tau^- {\bar\nu} $ anomalies: a general model analysis | PRD 99 (2019) 015007 | 1806.07403 |
| 58 | M. J. Baker, J. Fuentes-Martín, G. Isidori, and M. König | High-$ p_{\mathrm{T}} $ signatures in vector-leptoquark models | EPJC 79 (2019) 334 | 1901.10480 |
| 59 | C. Cornella, J. Fuentes-Martín, and G. Isidori | Revisiting the vector leptoquark explanation of the B-physics anomalies | JHEP 07 (2019) 168 | 1903.11517 |
| 60 | A. Angelescu et al. | Single leptoquark solutions to the B-physics anomalies | PRD 104 (2021) 055017 | 2103.12504 |
| 61 | C. Cornella et al. | Reading the footprints of the B-meson flavor anomalies | JHEP 08 (2021) 050 | 2103.16558 |
| 62 | G. Isidori, D. Lancierini, P. Owen, and N. Serra | On the significance of new physics in $ b \rightarrow s \ell^{+} \ell^{-} $ decays | PLB 822 (2021) 136644 | 2104.05631 |
| 63 | J. Aebischer et al. | Confronting the vector leptoquark hypothesis with new low- and high-energy data | EPJC 83 (2023) 153 | 2210.13422 |
| 64 | LHCb Collaboration | Differential branching fractions and isospin asymmetries of $ B \to K^{(*)} \mu^+ \mu^- $ decays | JHEP 06 (2014) 133 | 1403.8044 |
| 65 | LHCb Collaboration | Measurements of the S-wave fraction in $ B^{0}\rightarrow K^{+}\pi^{-}\mu^{+}\mu^{-} $ decays and the $ B^{0}\rightarrow K^{\ast}(892)^{0}\mu^{+}\mu^{-} $ differential branching fraction | JHEP 11 (2016) 047 | 1606.04731 |
| 66 | LHCb Collaboration | Angular analysis and differential branching fraction of the decay $ B^0_s\to\phi\mu^+\mu^- $ | JHEP 09 (2015) 179 | 1506.08777 |
| 67 | LHCb Collaboration | Angular analysis of the $ B^{0} \to K^{*0} \mu^{+} \mu^{-} $ decay using 3 fb$ ^{-1} $ of integrated luminosity | JHEP 02 (2016) 104 | 1512.04442 |
| 68 | LHCb Collaboration | Measurement of $ CP $-averaged observables in the $ B^{0}\rightarrow K^{*0}\mu^{+}\mu^{-} $ decay | PRL 125 (2020) 011802 | 2003.04831 |
| 69 | LHCb Collaboration | Angular analysis of the $ B^{+}\rightarrow K^{\ast+}\mu^{+}\mu^{-} $ decay | PRL 126 (2021) 161802 | 2012.13241 |
| 70 | Belle Collaboration | Lepton-flavor-dependent angular analysis of $ B\to K^\ast \ell^+\ell^- $ | PRL 118 (2017) 111801 | 1612.05014 |
| 71 | W. Buchmuller, R. Ruckl, and D. Wyler | Leptoquarks in lepton-quark collisions | PLB 191 (1987) 442 | |
| 72 | J. Blümlein, E. Boos, and A. Kryukov | Leptoquark pair production in hadronic interactions | Z. Phys. C 76 (1997) 137 | hep-ph/9610408 |
| 73 | I. Doršner and A. Greljo | Leptoquark toolbox for precision collider studies | JHEP 05 (2018) 126 | 1801.07641 |
| 74 | D. A. Faroughy, A. Greljo, and J. F. Kamenik | Confronting lepton flavor universality violation in B decays with high-$ p_{\mathrm{T}} $ tau lepton searches at LHC | PLB 764 (2017) 126 | 1609.07138 |
| 75 | M. Schmaltz and Y.-M. Zhong | The leptoquark hunter's guide: large coupling | JHEP 01 (2019) 132 | 1810.10017 |
| 76 | ATLAS Collaboration | Search for pair production of third-generation leptoquarks decaying into a bottom quark and a $ \tau $-lepton with the ATLAS detector | Submitted to EPJC, 2023 | 2303.01294 |
| 77 | ATLAS Collaboration | Search for leptoquarks decaying into the b$ \tau $ final state in $ pp $ collisions at $ \sqrt{s}= $ 13 TeV with the ATLAS detector | Submitted to JHEP, 2023 | 2305.15962 |
| 78 | CMS Collaboration | Search for a singly produced third-generation scalar leptoquark decaying to a $ \tau $ lepton and a bottom quark in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JHEP 07 (2018) 115 | CMS-EXO-17-029 1806.03472 |
| 79 | CMS Collaboration | Searches for physics beyond the standard model with the $ M_\mathrm{T2} $ variable in hadronic final states with and without disappearing tracks in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | EPJC 80 (2020) 3 | CMS-SUS-19-005 1909.03460 |
| 80 | CMS Collaboration | Search for singly and pair-produced leptoquarks coupling to third-generation fermions in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | PLB 819 (2021) 136446 | CMS-EXO-19-015 2012.04178 |
| 81 | ATLAS Collaboration | Search for pair production of third-generation scalar leptoquarks decaying into a top quark and a $ \tau $-lepton in $ pp $ collisions at $ \sqrt{s} $ = 13 TeV with the ATLAS detector | JHEP 06 (2021) 179 | 2101.11582 |
| 82 | ATLAS Collaboration | Search for new phenomena in $ pp $ collisions in final states with tau leptons, b-jets, and missing transverse momentum with the ATLAS detector | PRD 104 (2021) 112005 | 2108.07665 |
| 83 | CMS Collaboration | HEPData record for this analysis | link | |
| 84 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | |
| 85 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 86 | CMS Collaboration | Performance of the CMS level-1 trigger in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JINST 15 (2020) P10017 | CMS-TRG-17-001 2006.10165 |
| 87 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 88 | J. Alwall et al. | Comparative study of various algorithms for the merging of parton showers and matrix elements in hadronic collisions | EPJC 53 (2008) 473 | 0706.2569 |
| 89 | T. Sjöstrand et al. | An introduction to PYTHIA 8.2 | Comput. Phys. Commun. 191 (2015) 159 | 1410.3012 |
| 90 | J. M. Campbell and R. K. Ellis | An update on vector boson pair production at hadron colliders | PRD 60 (1999) 113006 | hep-ph/9905386 |
| 91 | J. M. Campbell, R. K. Ellis, and C. Williams | Vector boson pair production at the LHC | JHEP 07 (2011) 18 | 1105.0020 |
| 92 | J. M. Campbell, R. K. Ellis, and W. T. Giele | A multi-threaded version of MCFM | EPJC 75 (2015) 246 | 1503.06182 |
| 93 | P. Nason | A new method for combining NLO QCD with shower Monte Carlo algorithms | JHEP 11 (2004) 040 | hep-ph/0409146 |
| 94 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with parton shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 95 | S. Alioli, P. Nason, C. Oleari, and E. Re | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX | JHEP 06 (2010) 043 | 1002.2581 |
| 96 | S. Frixione, P. Nason, and G. Ridolfi | A positive-weight next-to-leading-order Monte Carlo for heavy flavour hadroproduction | JHEP 09 (2007) 126 | 0707.3088 |
| 97 | J. M. Campbell, R. K. Ellis, P. Nason, and E. Re | Top-pair production and decay at NLO matched with parton showers | JHEP 04 (2015) 114 | 1412.1828 |
| 98 | S. Alioli, P. Nason, C. Oleari, and E. Re | NLO single-top production matched with shower in POWHEG: $ s $- and $ t $-channel contributions | JHEP 09 (2009) 111 | 0907.4076 |
| 99 | E. Re | Single-top Wt-channel production matched with parton showers using the POWHEG method | EPJC 71 (2011) 1547 | 1009.2450 |
| 100 | Y. Li and F. Petriello | Combining QCD and electroweak corrections to production in FEWZ | PRD 86 (2012) 094034 | 1208.5967 |
| 101 | M. Czakon and A. Mitov | Top++: a program for the calculation of the top-pair cross-section at hadron colliders | Comput. Phys. Commun. 185 (2014) 2930 | 1112.5675 |
| 102 | P. Kant et al. | HatHor for single top-quark production: updated predictions and uncertainty estimates for single top-quark production in hadronic collisions | Comput. Phys. Commun. 191 (2015) 74 | 1406.4403 |
| 103 | F. Maltoni and T. Stelzer | MadEvent: automatic event generation with MadGraph | JHEP 02 (2003) 027 | hep-ph/0208156 |
| 104 | M. Krämer, T. Plehn, M. Spira, and P. M. Zerwas | Pair production of scalar leptoquarks at the Tevatron | PRL 79 (1997) 341 | hep-ph/9704322 |
| 105 | M. Krämer, T. Plehn, M. Spira, and P. M. Zerwas | Pair production of scalar leptoquarks at the CERN LHC | PRD 71 (2005) 057503 | hep-ph/0411038 |
| 106 | C. Borschensky, B. Fuks, A. Kulesza, and D. Schwartländer | Scalar leptoquark pair production at hadron colliders | PRD 101 (2020) 115017 | 2002.08971 |
| 107 | CMS Collaboration | Event generator tunes obtained from underlying event and multiparton scattering measurements | EPJC 76 (2016) 155 | CMS-GEN-14-001 1512.00815 |
| 108 | CMS Collaboration | Extraction and validation of a new set of CMS PYTHIA8 tunes from underlying-event measurements | EPJC 80 (2020) 4 | CMS-GEN-17-001 1903.12179 |
| 109 | CMS Collaboration | Investigations of the impact of the parton shower tuning in Pythia 8 in the modelling of $ \mathrm{t\overline{t}} $ at $ \sqrt{s}= $ 8 and 13 TeV | CMS Physics Analysis Summary, 2016 CMS-PAS-TOP-16-021 |
CMS-PAS-TOP-16-021 |
| 110 | R. D. Ball et al. | Parton distributions for the LHC Run II | JHEP 15 (2015) 40 | 1410.8849 |
| 111 | NNPDF Collaboration | Parton distributions from high-precision collider data | EPJC 77 (2017) 663 | 1706.00428 |
| 112 | GEANT4 Collaboration | GEANT 4---a simulation toolkit | NIM A 506 (2003) 250 | |
| 113 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 114 | CMS Collaboration | Technical proposal for the Phase-II upgrade of the Compact Muon Solenoid | CMS Technical Proposal CERN-LHCC-2015-010, CMS-TDR-15-02, 2015 CDS |
|
| 115 | CMS Collaboration | ECAL 2016 refined calibration and Run2 summary plots | CMS Detector Performance Summary CMS-DP-2020-021, 2020 CDS |
|
| 116 | CMS Collaboration | Electron and photon reconstruction and identification with the CMS experiment at the CERN LHC | JINST 16 (2021) P05014 | CMS-EGM-17-001 2012.06888 |
| 117 | H. Voss, A. Höcker, J. Stelzer, and F. Tegenfeldt | TMVA, the toolkit for multivariate data analysis with ROOT | in XI Int. Workshop on Advanced Computing and Analysis Techniques in Physics Research. . . PoS ACAT:040, 2007 link |
physics/0703039 |
| 118 | CMS Collaboration | Performance of the CMS muon detector and muon reconstruction with proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JINST 13 (2018) P06015 | CMS-MUO-16-001 1804.04528 |
| 119 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_{\mathrm{T}} $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 120 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 121 | CMS Collaboration | Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV | JINST 12 (2017) P02014 | CMS-JME-13-004 1607.03663 |
| 122 | D. Guest et al. | Jet flavor classification in high-energy physics with deep neural networks | PRD 94 (2016) 112002 | 1607.08633 |
| 123 | CMS Collaboration | Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV | JINST 13 (2018) P05011 | CMS-BTV-16-002 1712.07158 |
| 124 | CMS Collaboration | Identification of b-quark jets with the CMS experiment | JINST 8 (2013) P04013 | CMS-BTV-12-001 1211.4462 |
| 125 | CMS Collaboration | Performance of reconstruction and identification of $ \tau $ leptons decaying to hadrons and $ \nu_\tau $ in pp collisions at $ \sqrt{s}= $ 13 TeV | JINST 13 (2018) P10005 | CMS-TAU-16-003 1809.02816 |
| 126 | CMS Collaboration | Identification of hadronic tau lepton decays using a deep neural network | JINST 17 (2022) P07023 | CMS-TAU-20-001 2201.08458 |
| 127 | CMS Collaboration | Performance of missing transverse momentum reconstruction in proton-proton collisions at $ \sqrt{s} = $ 13 TeV using the CMS detector | JINST 14 (2019) P07004 | CMS-JME-17-001 1903.06078 |
| 128 | B. L. Combridge and C. J. Maxwell | Untangling large-$ p_\mathrm{T} $ hadronic reactions | NPB 239 (1984) 429 | |
| 129 | CMS Collaboration | Search for additional neutral MSSM Higgs bosons in the $ \tau\tau $ final state in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JHEP 09 (2018) 007 | CMS-HIG-17-020 1803.06553 |
| 130 | CMS Collaboration | Measurement of the $ \mathrm{Z}\gamma^{*} \to \tau\tau $ cross section in pp collisions at $ \sqrt{s} = $ 13 TeV and validation of $ \tau $ lepton analysis techniques | EPJC 78 (2018) 708 | CMS-HIG-15-007 1801.03535 |
| 131 | CMS Collaboration | Precision luminosity measurement in proton-proton collisions at $ \sqrt{s} = $ 13 TeV in 2015 and 2016 at CMS | EPJC 81 (2021) 800 | CMS-LUM-17-003 2104.01927 |
| 132 | CMS Collaboration | CMS luminosity measurement for the 2017 data-taking period at $ \sqrt{s} $ = 13 TeV | CMS Physics Analysis Summary, 2018 link |
CMS-PAS-LUM-17-004 |
| 133 | CMS Collaboration | CMS luminosity measurement for the 2018 data-taking period at $ \sqrt{s} $ = 13 TeV | CMS Physics Analysis Summary, 2019 link |
CMS-PAS-LUM-18-002 |
| 134 | CMS Collaboration | Observation of the Higgs boson decay to a pair of $ \tau $ leptons with the CMS detector | PLB 779 (2018) 283 | CMS-HIG-16-043 1708.00373 |
| 135 | CMS Collaboration | Performance of reconstruction and identification of tau leptons in their decays to hadrons and tau neutrino in LHC Run-2 | CMS Physics Analysis Summary, 2016 CMS-PAS-TAU-16-002 |
CMS-PAS-TAU-16-002 |
| 136 | CMS Collaboration | Measurement of the inclusive W and Z production cross sections in pp collisions at $ \sqrt{s}= $ 7 TeV | JHEP 10 (2011) 132 | CMS-EWK-10-005 1107.4789 |
| 137 | CMS Collaboration | Determination of jet energy calibration and transverse momentum resolution in CMS | JINST 6 (2011) P11002 | CMS-JME-10-011 1107.4277 |
| 138 | CMS Collaboration | Measurement of differential $ {\mathrm{t}\overline{\mathrm{t}}} $ production cross sections in the full kinematic range using lepton+jets events from proton-proton collisions at $ \sqrt {s} $ = 13 TeV | PRD 104 (2021) 092013 | CMS-TOP-20-001 2108.02803 |
| 139 | ATLAS Collaboration | Measurements of differential cross-sections in top-quark pair events with a high transverse momentum top quark and limits on beyond the standard model contributions to top-quark pair production with the ATLAS detector at $ \sqrt{s} = $ 13 TeV | JHEP 06 (2022) 063 | 2202.12134 |
| 140 | ATLAS Collaboration | Differential $ {\mathrm{t}\overline{\mathrm{t}}} $ cross-section measurements using boosted top quarks in the all-hadronic final state with 139 fb$ ^{-1} $ of ATLAS data | JHEP 04 (2023) 080 | 2205.02817 |
| 141 | CMS Collaboration | Search for a heavy resonance decaying to a top quark and a W boson at $ \sqrt{s} $ = 13 TeV in the fully hadronic final state | JHEP 12 (2021) 106 | 2104.12853 |
| 142 | J. Butterworth et al. | PDF4LHC recommendations for LHC Run II | JPG 43 (2016) 023001 | 1510.03865 |
| 143 | R. J. Barlow and C. Beeston | Fitting using finite Monte Carlo samples | Comput. Phys. Commun. 77 (1993) 219 | |
| 144 | J. S. Conway | Incorporating nuisance parameters in likelihoods for multisource spectra | PHYSTAT 201 (2011) 115 | 1103.0354 |
| 145 | ATLAS and CMS Collaborations | Procedure for the LHC Higgs boson search combination in summer 2011 | Technical Report CMS-NOTE-2011-005, ATL-PHYS-PUB-2011-011, 2011 | |
| 146 | G. Cowan, K. Cranmer, E. Gross, and O. Vitells | Asymptotic formulae for likelihood-based tests of new physics | EPJC 71 (2011) 1554 | 1007.1727 |
| 147 | CMS Collaboration | Searches for additional Higgs bosons and for vector leptoquarks in $ \tau\tau $ final states in proton-proton collisions at $ \sqrt{s} $ = 13 TeV | JHEP 07 (2023) 073 | CMS-HIG-21-001 2208.02717 |
| 148 | T. Junk | Confidence level computation for combining searches with small statistics | NIM A 434 (1999) 435 | hep-ex/9902006 |
| 149 | A. L. Read | Presentation of search results: the CL$ _\text{s} $ technique | JPG 28 (2002) 2693 | |
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|