

Compact Muon Solenoid
LHC, CERN
| CMS-HIG-17-020 ; CERN-EP-2018-026 | ||
| Search for additional neutral MSSM Higgs bosons in the ττ final state in proton-proton collisions at √s= 13 TeV | ||
| CMS Collaboration | ||
| 17 March 2018 | ||
| JHEP 09 (2018) 007 | ||
| Abstract: A search is presented for additional neutral Higgs bosons in the ττ final state in proton-proton collisions at the LHC. The search is performed in the context of the minimal supersymmetric extension of the standard model (MSSM), using the data collected with the CMS detector in 2016 at a center-of-mass energy of 13 TeV, corresponding to an integrated luminosity of 35.9 fb−1. To enhance the sensitivity to neutral MSSM Higgs bosons, the search includes production of the Higgs boson in association with b quarks. No significant deviation above the expected background is observed. Model-independent limits at 95% confidence level (CL) are set on the product of the branching fraction for the decay into τ leptons and the cross section for the production via gluon fusion or in association with b quarks. These limits range from 18 pb at 90 GeV to 3.5 fb at 3.2 TeV for gluon fusion and from 15 pb (at 90 GeV) to 2.5 fb (at 3.2 TeV) for production in association with b quarks. In the mmod+h scenario these limits translate into a 95% CL exclusion of tanβ> 6 for neutral Higgs boson masses below 250 GeV, where tanβ is the ratio of the vacuum expectation values of the neutral components of the two Higgs doublets. The 95% CL exclusion contour reaches 1.6 TeV for tanβ= 60. | ||
| Links: e-print arXiv:1803.06553 [hep-ex] (PDF) ; CDS record ; inSPIRE record ; HepData record ; CADI line (restricted) ; | ||
| Figures & Tables | Summary | Additional Figures & Tables & Material | References | CMS Publications |
|---|
| Figures | |
png pdf |
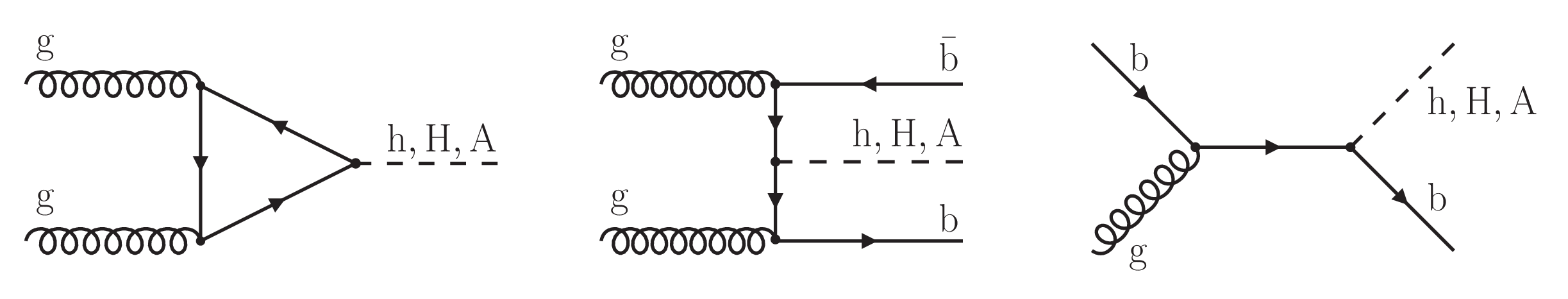
Figure 1:
Diagrams for the production of neutral Higgs bosons (left) via gluon fusion and (middle and right) in association with b quarks. In supersymmetric extensions of the SM, the super-partners also contribute to the fermion loop, shown in the left panel. In the middle panel a pair of b quarks is produced from two gluons (the LO process in the four-flavor scheme). In the right panel the Higgs boson is radiated from a b quark in the proton (the LO process in the five-flavor scheme). |
png pdf |
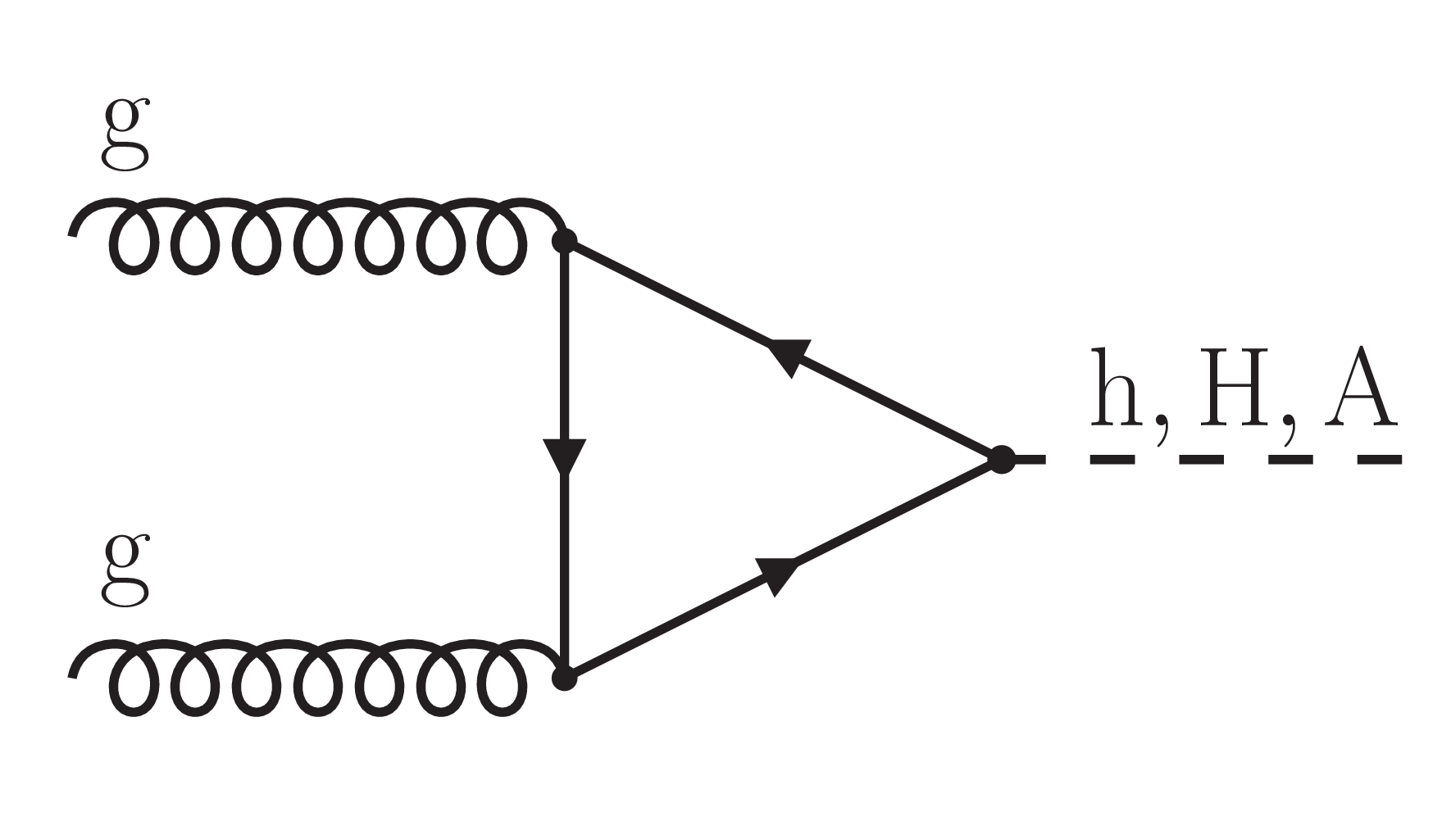
Figure 1-a:
Diagram for the production of neutral Higgs bosons via gluon fusion. In supersymmetric extensions of the SM, the super-partners also contribute to the fermion loop. |
png pdf |
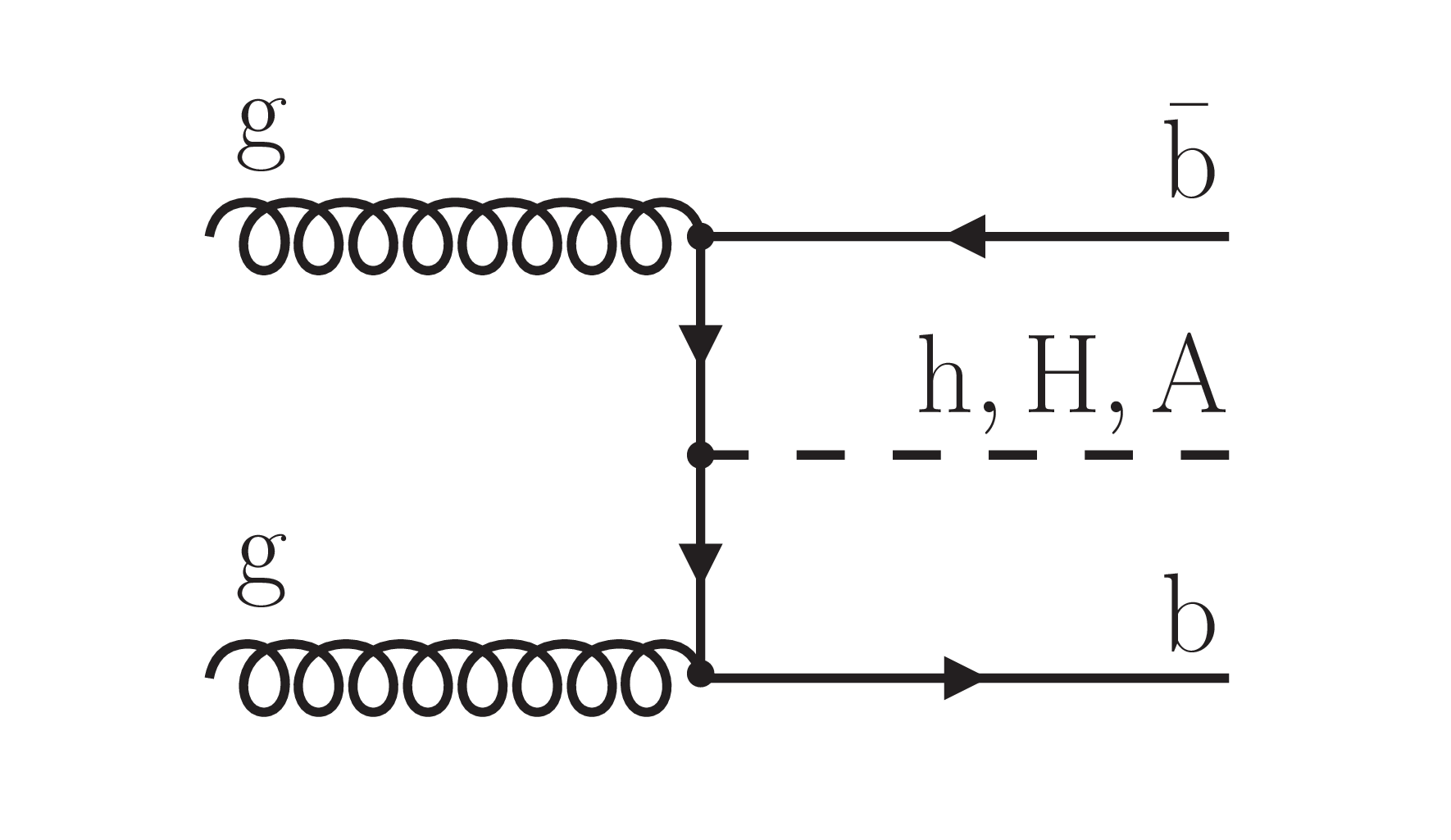
Figure 1-b:
Diagram for the production of neutral Higgs bosons in association with b quarks. A pair of b quarks is produced from two gluons (the LO process in the four-flavor scheme). |
png pdf |
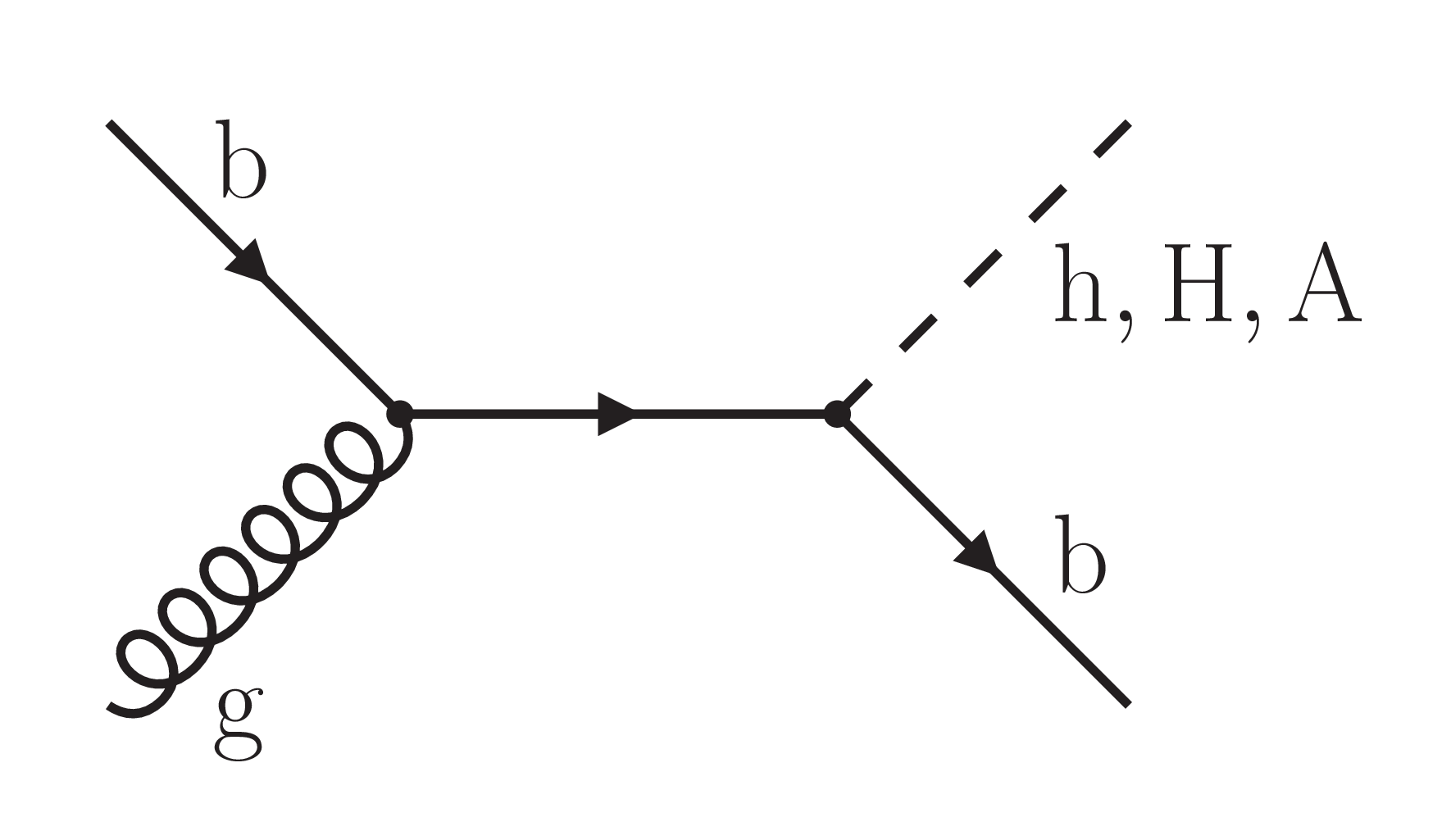
Figure 1-c:
Diagram for the production of neutral Higgs bosons in association with b quarks. The Higgs boson is radiated from a b quark in the proton (the LO process in the five-flavor scheme). |
png pdf |
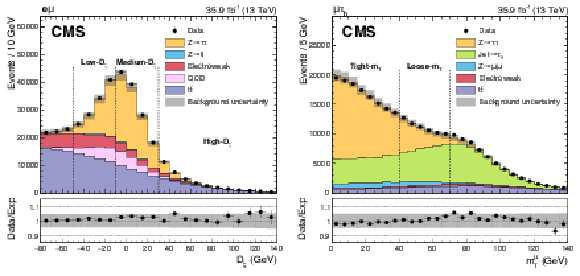
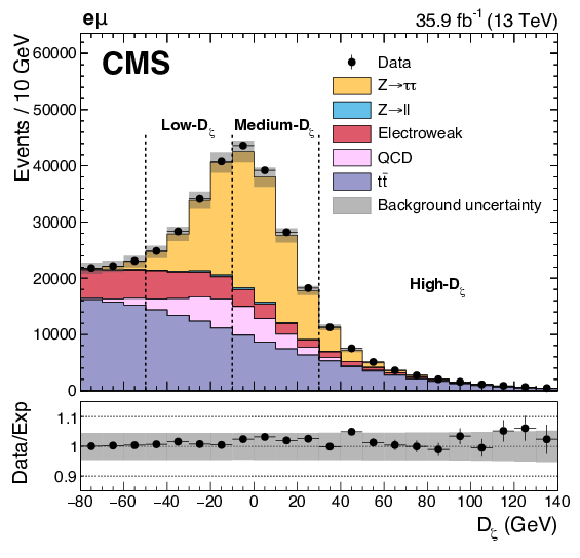
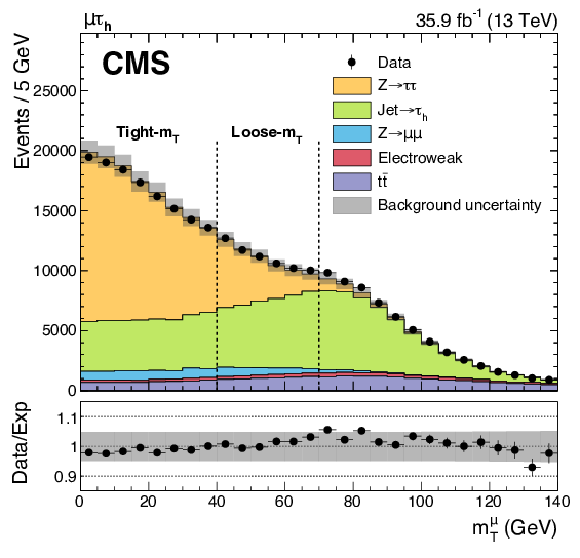
Figure 2:
Observed and expected distributions of (left) Dζ in the eμ final state and (right) mμT in the μτh final state. The dashed vertical lines indicate the definition of the subcategories in each final state. The label "jet→τh'' indicates events with jets misidentified as hadronic τ lepton decays, e.g. W+jets events, which are estimated from data as described in Section 5.2. A detailed description of the composition of the expected background is given in Section 6. The distributions are shown before any event categorization and prior to the fit used for the signal extraction. For these figures no uncertainties that affect the shape of the distributions have been included in the uncertainty model. |
png pdf |
Figure 2-a:
Observed and expected distribution of Dζ in the eμ final state. The dashed vertical lines indicate the definition of the subcategories in each final state. The label "jet→τh'' indicates events with jets misidentified as hadronic τ lepton decays, e.g. W+jets events, which are estimated from data as described in Section 5.2. A detailed description of the composition of the expected background is given in Section 6. The distributions are shown before any event categorization and prior to the fit used for the signal extraction. For this figure no uncertainties that affect the shape of the distributions have been included in the uncertainty model. |
png pdf |
Figure 2-b:
Observed and expected distribution of mμT in the μτh final state. The dashed vertical lines indicate the definition of the subcategories in each final state. The label "jet→τh'' indicates events with jets misidentified as hadronic τ lepton decays, e.g. W+jets events, which are estimated from data as described in Section 5.2. A detailed description of the composition of the expected background is given in Section 6. The distributions are shown before any event categorization and prior to the fit used for the signal extraction. For this figure no uncertainties that affect the shape of the distributions have been included in the uncertainty model. |
png pdf |
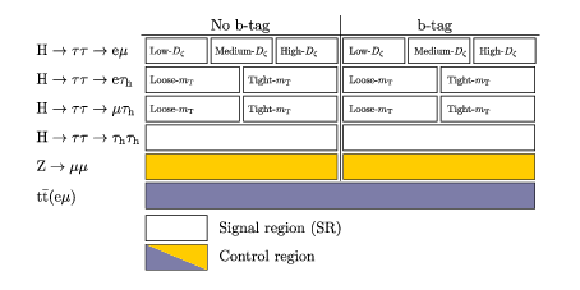
Figure 3:
Overview of all event subcategories that enter the statistical analysis. Sixteen signal categories are complemented by three background control regions in the main analysis as described in Section 5. |
png pdf |
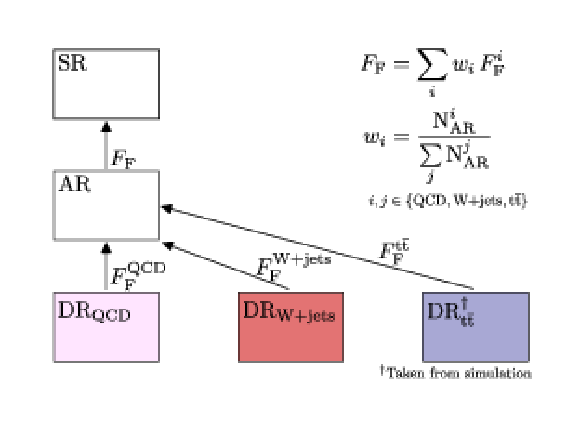
Figure 4:
Schematic view of the determination and application of the FFi and FF for the estimation of the background from QCD multijet, W+jets, and t¯t events due to the misidentification of jets as hadronic τ lepton decays. Note that DRt¯t is taken from simulation. |
png pdf |
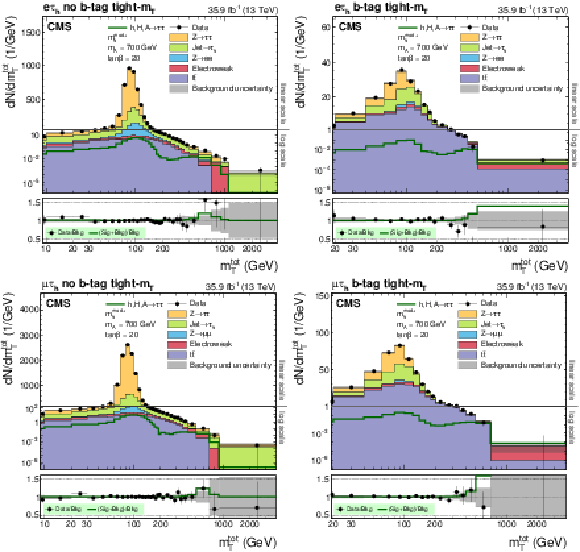
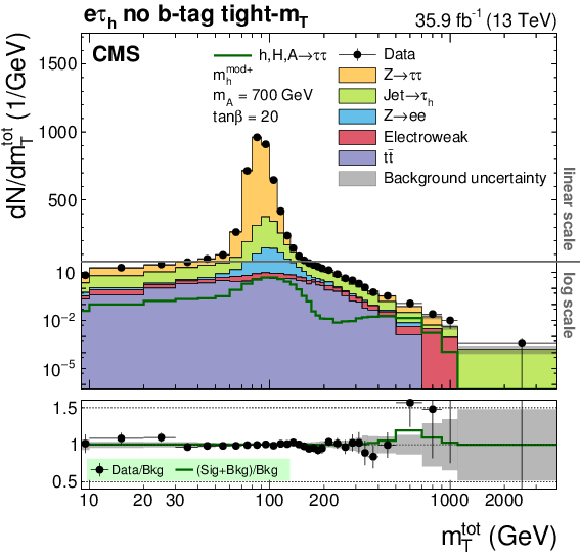
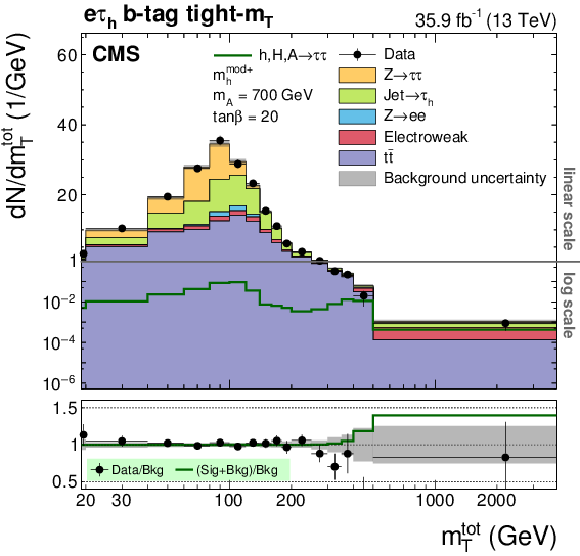
Figure 5:
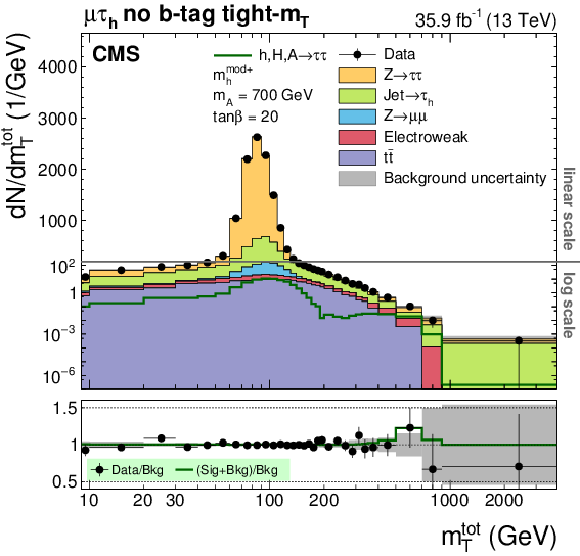
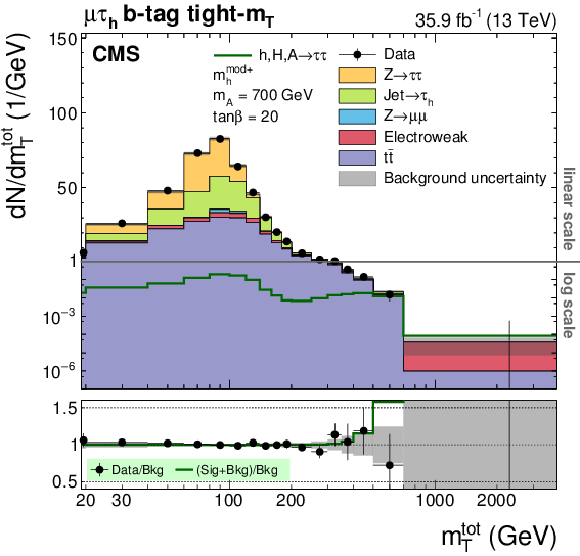
Distribution of mtotT in the global no b-tag (left) and b-tag (right) categories in the eτh (upper row) and μτh (lower row) final states. In all cases the most sensitive tight-mT event subcategory is shown. The gray horizontal line in the upper panel of each subfigure indicates the change from logarithmic to linear scale on the vertical axis. |
png pdf |
Figure 5-a:
Distribution of mtotT in the global no b-tag category in the eτh final state. The most sensitive tight-mT event subcategory is shown. The gray horizontal line in the upper panel indicates the change from logarithmic to linear scale on the vertical axis. |
png pdf |
Figure 5-b:
Distribution of mtotT in the global b-tag category in the eτh final state. The most sensitive tight-mT event subcategory is shown. The gray horizontal line in the upper panel indicates the change from logarithmic to linear scale on the vertical axis. |
png pdf |
Figure 5-c:
Distribution of mtotT in the global no b-tag category in the μτh final state. The most sensitive tight-mT event subcategory is shown. The gray horizontal line in the upper panel indicates the change from logarithmic to linear scale on the vertical axis. |
png pdf |
Figure 5-d:
Distribution of mtotT in the global b-tag category in the μτh final state. The most sensitive tight-mT event subcategory is shown. The gray horizontal line in the upper panel indicates the change from logarithmic to linear scale on the vertical axis. |

png pdf |
Figure 6:
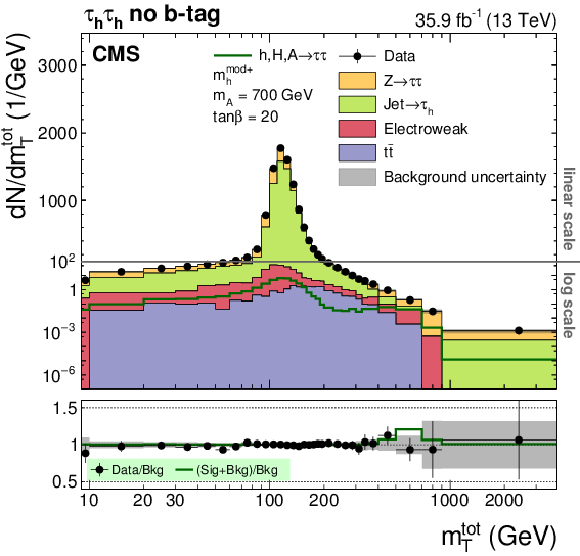
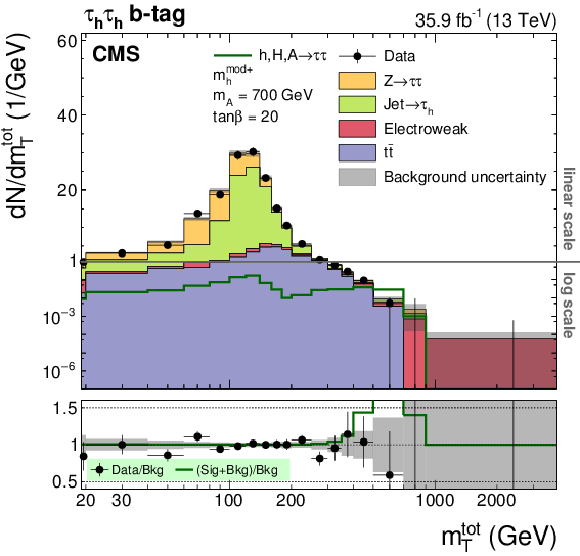
Distribution of mtotT in the global no b-tag (left) and b-tag (right) categories in the τhτh (upper row) and eμ (lower row) final states. For the eμ final state the most sensitive medium-Dζ event subcategory is shown. The gray horizontal line in the upper panel of each subfigure indicates the change from logarithmic to linear scale on the vertical axis. |
png pdf |
Figure 6-a:
Distribution of mtotT in the global no b-tag category in the τhτh final state. The gray horizontal line in the upper panel indicates the change from logarithmic to linear scale on the vertical axis. |
png pdf |
Figure 6-b:
Distribution of mtotT in the global b-tag category in the τhτh final state. The gray horizontal line in the upper panel indicates the change from logarithmic to linear scale on the vertical axis. |
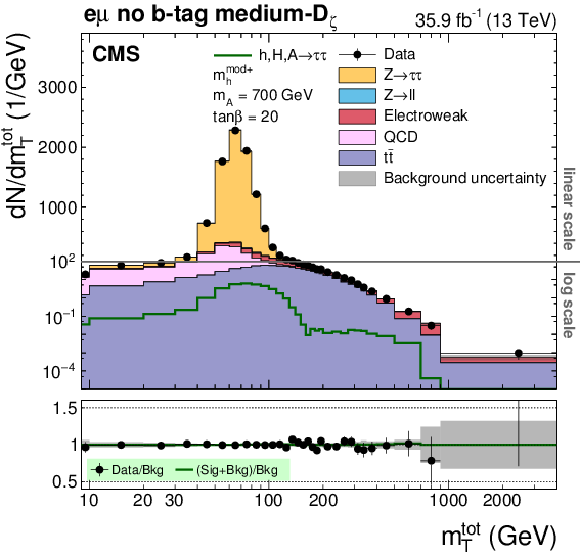
png pdf |
Figure 6-c:
Distribution of mtotT in the global no b-tag category in the eμ final state. The most sensitive medium-Dζ event subcategory is shown. The gray horizontal line in the upper panel indicates the change from logarithmic to linear scale on the vertical axis. |
png pdf |
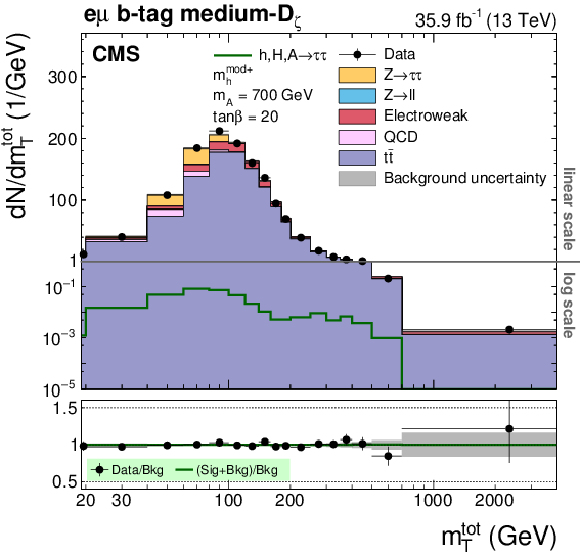
Figure 6-d:
Distribution of mtotT in the global b-tag category in the eμ final state. The most sensitive medium-Dζ event subcategory is shown. The gray horizontal line in the upper panel indicates the change from logarithmic to linear scale on the vertical axis. |
png pdf |
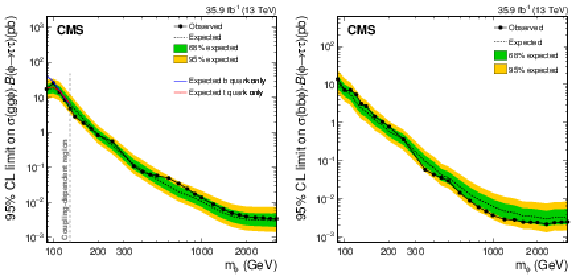
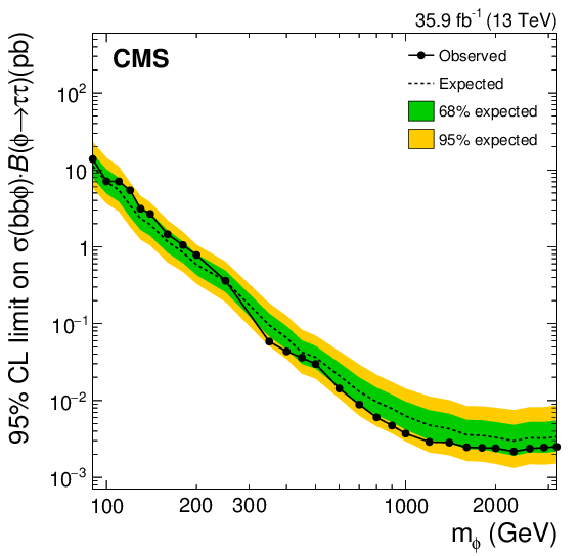
Figure 7:
Expected and observed 95% CL upper limits for the production of a single narrow resonance, ϕ, with a mass between 90 GeV and 3.2 TeV in the ττ final state (left) for the production via gluon fusion (ggϕ) and (right) in association with b quarks (bbϕ). The expected median of the exclusion limit is shown by the dashed line. The dark green and bright yellow bands indicate the 68 and 95% confidence intervals for the variation of the expected exclusion limit. The black dots correspond to the observed limits. In the left panel the expected exclusion limits for the cases where (blue continuous line) only the b quark and (red continuous line) only the t quark are taken into account in the fermion loop are also shown. Left of the dashed vertical line the two different assumptions lead to visible differences in the expected exclusion limit. |
png pdf |
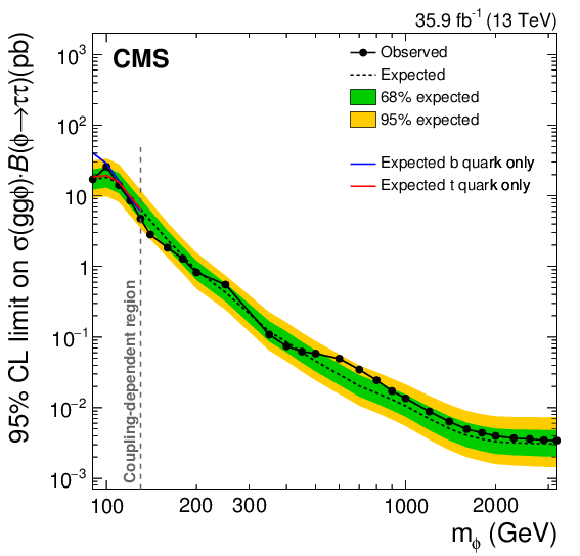
Figure 7-a:
Expected and observed 95% CL upper limits for the production of a single narrow resonance, ϕ, with a mass between 90 GeV and 3.2 TeV in association with b quarks (bbϕ). The expected median of the exclusion limit is shown by the dashed line. The dark green and bright yellow bands indicate the 68 and 95% confidence intervals for the variation of the expected exclusion limit. The black dots correspond to the observed limits. The expected exclusion limits for the cases where (blue continuous line) only the b quark and (red continuous line) only the t quark are taken into account in the fermion loop are also shown. Left of the dashed vertical line the two different assumptions lead to visible differences in the expected exclusion limit. |
png pdf |
Figure 7-b:
Expected and observed 95% CL upper limits for the production of a single narrow resonance, ϕ, with a mass between 90 GeV and 3.2 TeV in association with b quarks (bbϕ). The expected median of the exclusion limit is shown by the dashed line. The dark green and bright yellow bands indicate the 68 and 95% confidence intervals for the variation of the expected exclusion limit. The black dots correspond to the observed limits. |
png pdf |
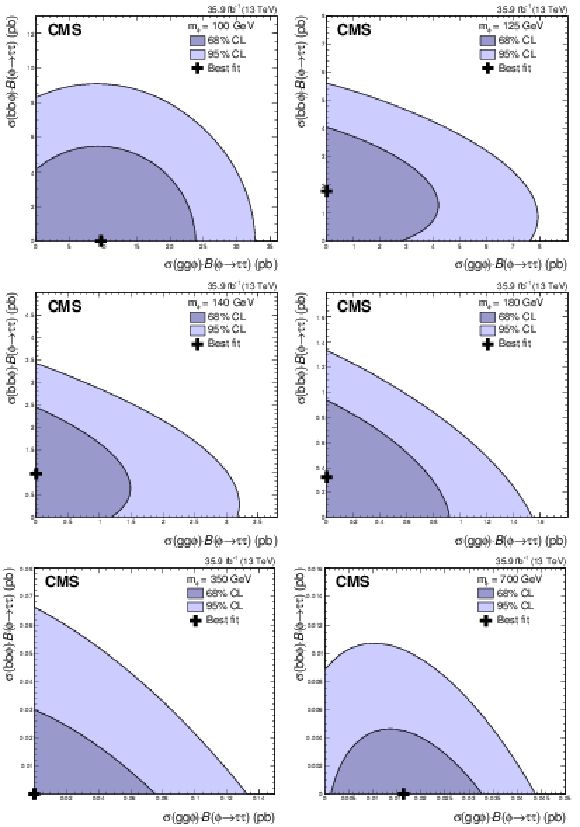
Figure 8:
Scan of the likelihood function for the search in the ττ final state for a single narrow resonance, ϕ, produced via gluon fusion (ggϕ) or in association with b quarks (bbϕ). A representative subset of the mass points tested at (upper left) 100 GeV, (upper right) 125 GeV, (middle left) 140 GeV, (middle right) 180 GeV, (lower left) 350 GeV, and (lower right) 700 GeV is shown. Note that in the fits the signal strengths are not allowed to become negative. |
png pdf |
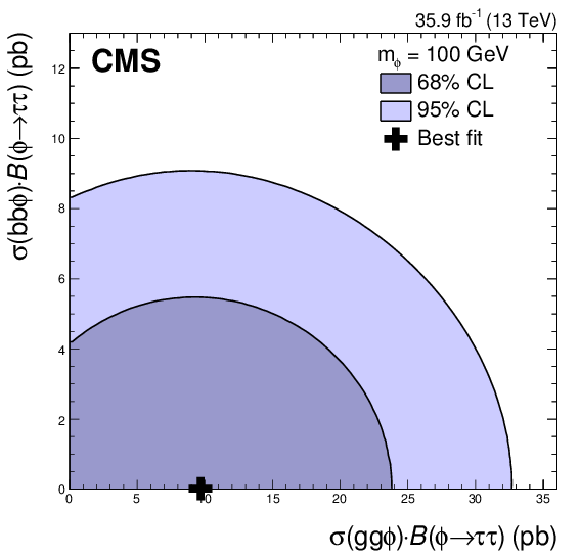
Figure 8-a:
Scan of the likelihood function for the search in the ττ final state for a single narrow resonance, ϕ, produced via gluon fusion (ggϕ) or in association with b quarks (bbϕ). The result is shown at mass point 100 GeV. Note that in the fit the signal strengths are not allowed to become negative. |
png pdf |
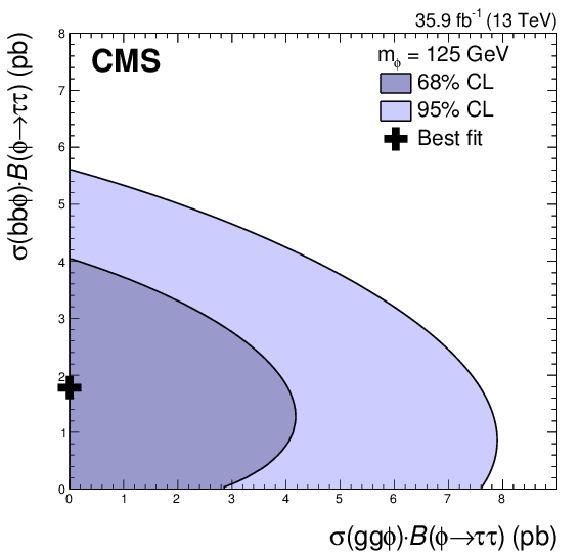
Figure 8-b:
Scan of the likelihood function for the search in the ττ final state for a single narrow resonance, ϕ, produced via gluon fusion (ggϕ) or in association with b quarks (bbϕ). The result is shown at mass point 125 GeV. Note that in the fit the signal strengths are not allowed to become negative. |
png pdf |
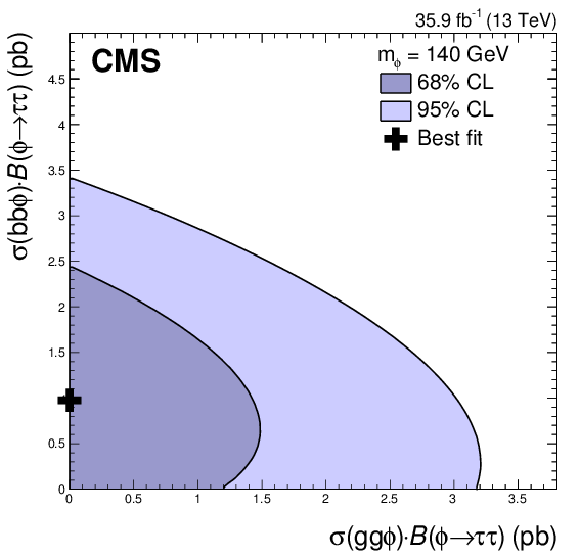
Figure 8-c:
Scan of the likelihood function for the search in the ττ final state for a single narrow resonance, ϕ, produced via gluon fusion (ggϕ) or in association with b quarks (bbϕ). The result is shown at mass point 140 GeV. Note that in the fit the signal strengths are not allowed to become negative. |
png pdf |
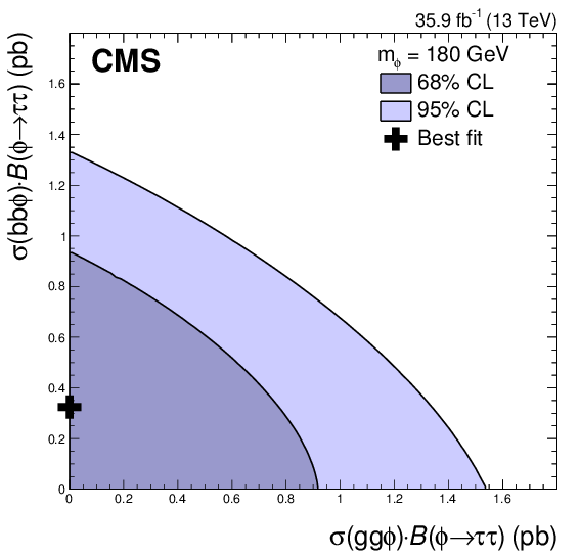
Figure 8-d:
Scan of the likelihood function for the search in the ττ final state for a single narrow resonance, ϕ, produced via gluon fusion (ggϕ) or in association with b quarks (bbϕ). The result is shown at mass point 180 GeV. Note that in the fit the signal strengths are not allowed to become negative. |
png pdf |
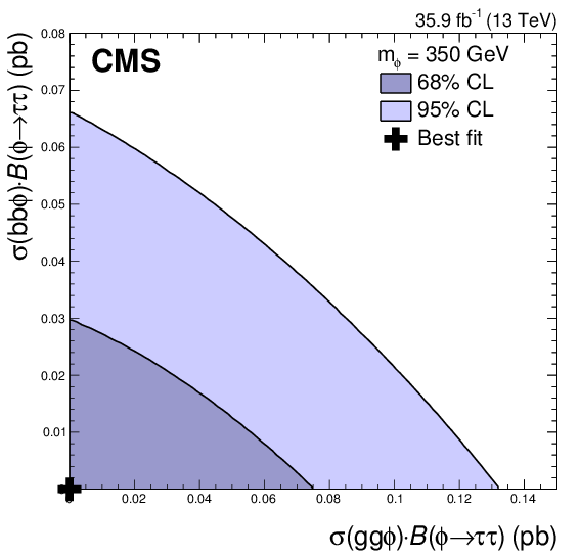
Figure 8-e:
Scan of the likelihood function for the search in the ττ final state for a single narrow resonance, ϕ, produced via gluon fusion (ggϕ) or in association with b quarks (bbϕ). The result is shown at mass point 350 GeV. Note that in the fit the signal strengths are not allowed to become negative. |

png pdf |
Figure 8-f:
Scan of the likelihood function for the search in the ττ final state for a single narrow resonance, ϕ, produced via gluon fusion (ggϕ) or in association with b quarks (bbϕ). The result is shown at mass point 700 GeV. Note that in the fit the signal strengths are not allowed to become negative. |
png pdf |
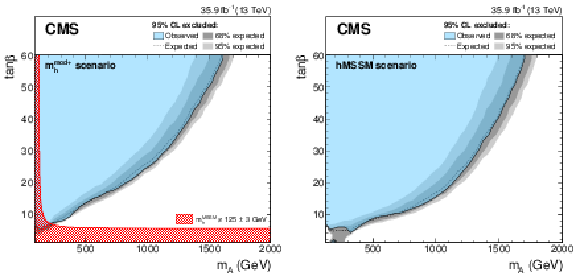
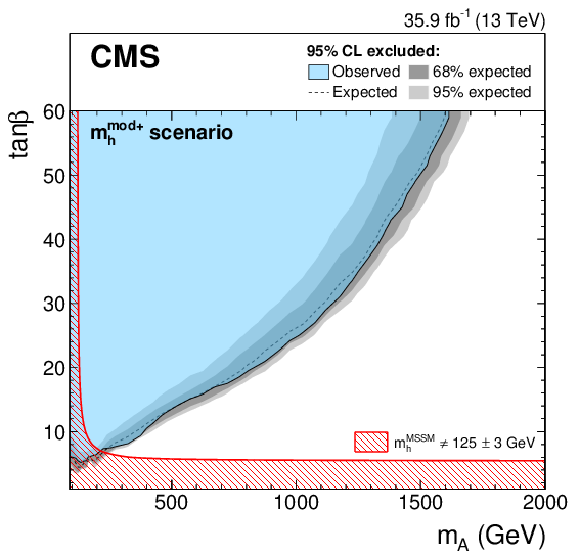
Figure 9:
Expected and observed 95% CL exclusion contour (left) in the MSSM mmod+h and (right) in the hMSSM scenarios. The expected median is shown as a dashed black line. The dark and bright gray bands indicate the 68 and 95% confidence intervals for the variation of the expected exclusion. The observed exclusion contour is indicated by the colored blue area. For the mmod+h scenario, those parts of the parameter space, where mh deviates by more then ±3 GeV from the mass of the observed Higgs boson at 125 GeV are indicated by a red hatched area. |
png pdf |
Figure 9-a:
Expected and observed 95% CL exclusion contour in the MSSM mmod+h scenario. The expected median is shown as a dashed black line. The dark and bright gray bands indicate the 68 and 95% confidence intervals for the variation of the expected exclusion. The observed exclusion contour is indicated by the colored blue area. Those parts of the parameter space, where mh deviates by more then ±3 GeV from the mass of the observed Higgs boson at 125 GeV are indicated by a red hatched area. |

png pdf |
Figure 9-b:
Expected and observed 95% CL exclusion contour in the hMSSM scenario. The expected median is shown as a dashed black line. The dark and bright gray bands indicate the 68 and 95% confidence intervals for the variation of the expected exclusion. The observed exclusion contour is indicated by the colored blue area. |
| Tables | |

png pdf |
Table 1:
Kinematic selection of the τ lepton decay products in the eμ, eτh, μτh, and τhτh final states. The expression "First (Second) object'' refers to the final state label used in the first column. |
png pdf |
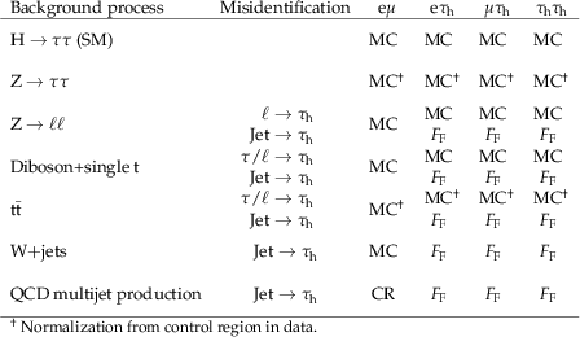
Table 2:
Background processes contributing to the event selection, as given in Section wwwww. The first row corresponds to the SM Higgs boson in the ττ final state, which is also taken into account in the statistical analysis. The further splitting of the processes in the second column refers only to final states that contain a τh candidate. The label "MC'' implies that the process is taken from simulation; the label "FF'' implies that the process is determined from data using the fake factor method, as described in Section 5.2. The label "CR'' implies that both the shape and normalization of QCD multijet events are estimated from control regions in data. The symbol ℓ corresponds to an electron or muon. |
png pdf |
Table 3:
Observed number of selected events (Ndata) and the relative contribution of the expected backgrounds in all event categories in the eμ, eτh, μτh, and τhτh final states. The relative contribution of the expected backgrounds is given in %, including the contribution of an SM Higgs boson with a mass of 125 GeV, and prior to the fit used for the signal extraction. In all but the eμ final state, processes in which a jet is misidentified as a hadronic τ lepton decay are subsumed into a common jet→τh background class which is estimated from data. |

png pdf |
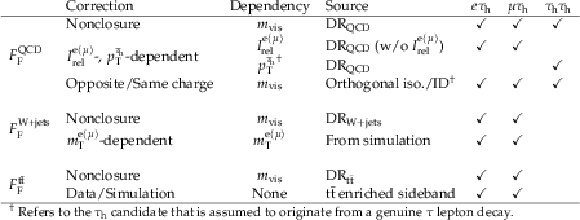
Table 4:
Corrections applied to the FFQCD, FFW+jets, and FFt¯t as described in the text. In the fourth column the source is indicated from which the correction is derived. The dependency pTτh in the third line refers to the pT of the τh candidate that is assumed to originate from a genuine τ lepton decay. |
| Summary |
| A search for additional heavy neutral Higgs bosons in the decay into two τ leptons in the context of the minimal supersymmetric standard model (MSSM) has been presented. This search has been performed in the most sensitive eμ, eτh, μτh, and τhτh final states of the ττ pair, where τh indicates a hadronic τ lepton decay. No signal has been found. Model-independent limits at 95% confidence level have been set for the production of a single narrow resonance decaying into a pair of τ leptons. These range from 18 pb at 90 GeV to 3.5 fb at 3.2 TeV for production via gluon fusion and from 15 pb (at 90 GeV) to 2.5 fb (at 3.2 TeV) for production in association with b quarks. Finally 95% confidence level exclusion contours have been provided for two representative benchmark scenarios, namely the mmod+h and the hMSSM scenarios. In these two scenarios the presence of a neutral heavy MSSM Higgs boson up to mA<250GeV is excluded for tanβ values above 6. The exclusion contour reaches 1.6 TeV for tanβ= 60. |
| Additional Figures | |
png pdf |
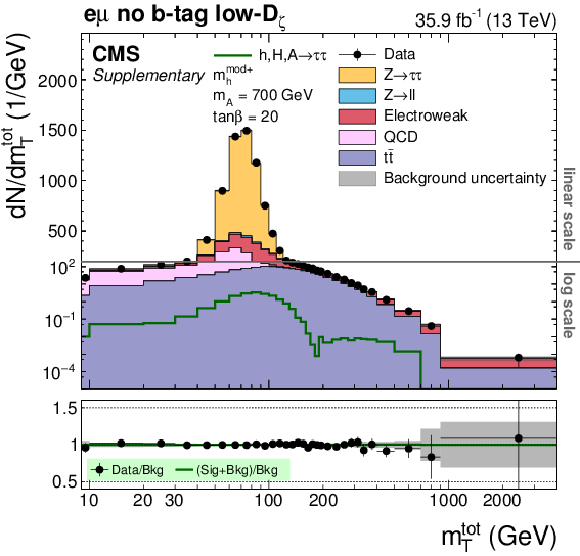
Additional Figure 1:
Distribution of mtotT in the no b-tag low-Dζ event category in the eμ final state. |
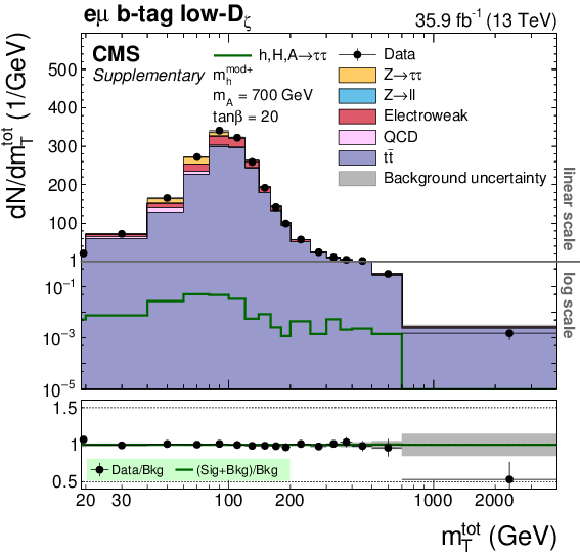
png pdf |
Additional Figure 2:
Distribution of mtotT in the b-tag low-Dζ event category in the eμ final state. |
png pdf |
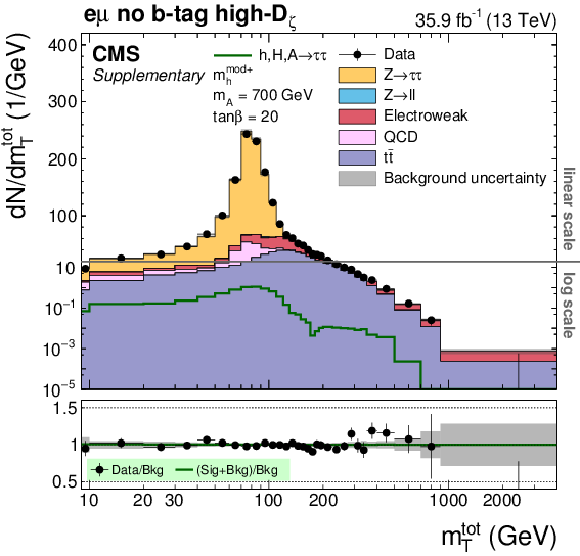
Additional Figure 3:
Distribution of mtotT in the no b-tag high-Dζ event category in the eμ final state. |
png pdf |
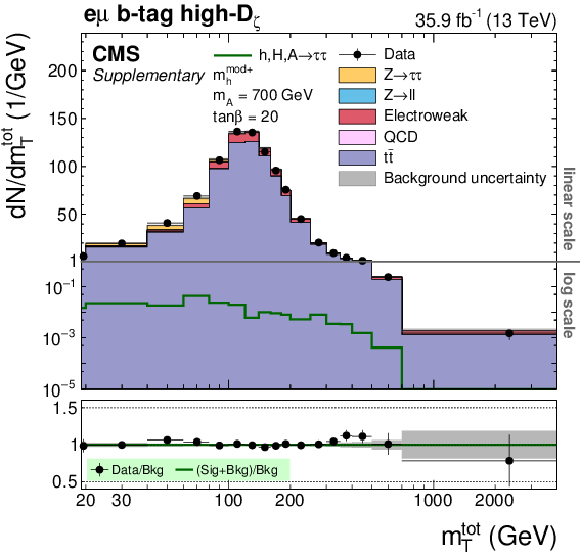
Additional Figure 4:
Distribution of mtotT in the b-tag high-Dζ event category in the eμ final state. |
png pdf |
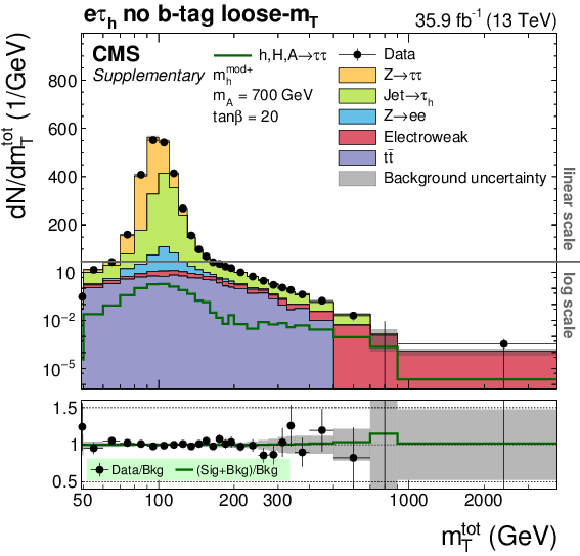
Additional Figure 5:
Distribution of mtotT in the no b-tag loose-mT event category in the eτh final state. |
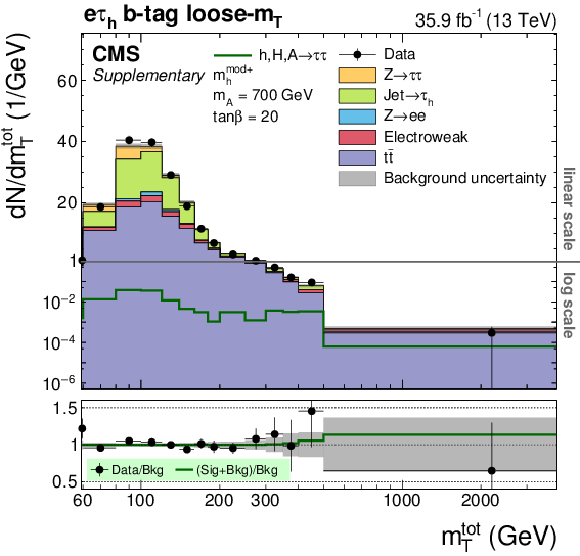
png pdf |
Additional Figure 6:
Distribution of mtotT in the b-tag loose-mT event category in the eτh final state. |
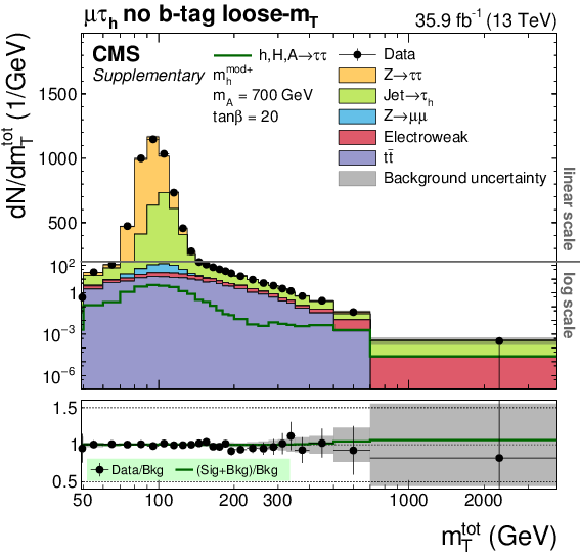
png pdf |
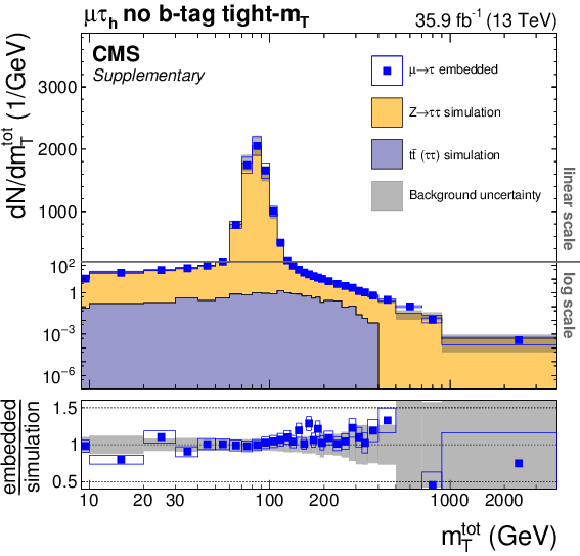
Additional Figure 7:
Distribution of mtotT in the no b-tag loose-mT event category in the μτh final state. |
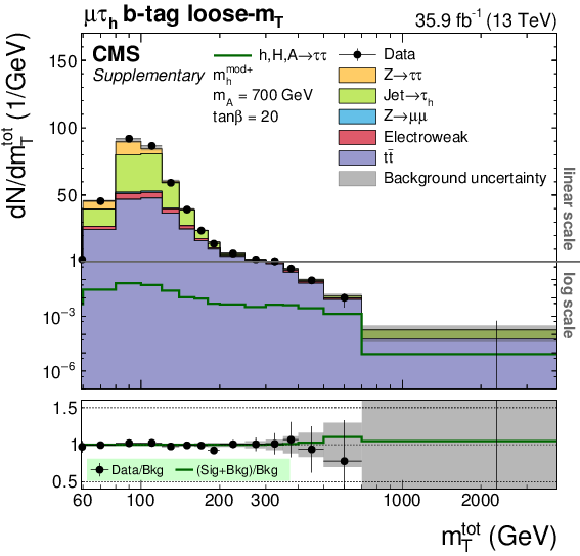
png pdf |
Additional Figure 8:
Distribution of mtotT in the b-tag loose-mT event category in the μτh final state. |
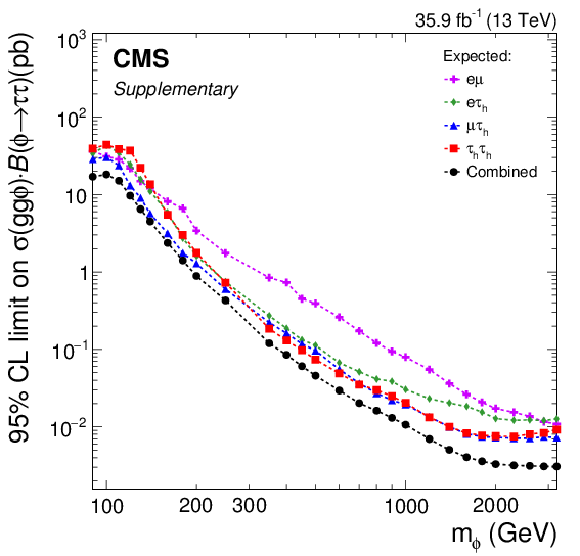
png pdf |
Additional Figure 9:
Expected 95% CL upper limits for the production of a single narrow resonance ϕ with a mass between 90 GeV and 3.2 TeV via gluon-fusion (ggϕ) in the ττ final sate. The individual contributions to the combined analysis have been split by the decay modes of the final state τ leptons. For these limits the SM Higgs boson has been added to the non-Higgs SM background. |
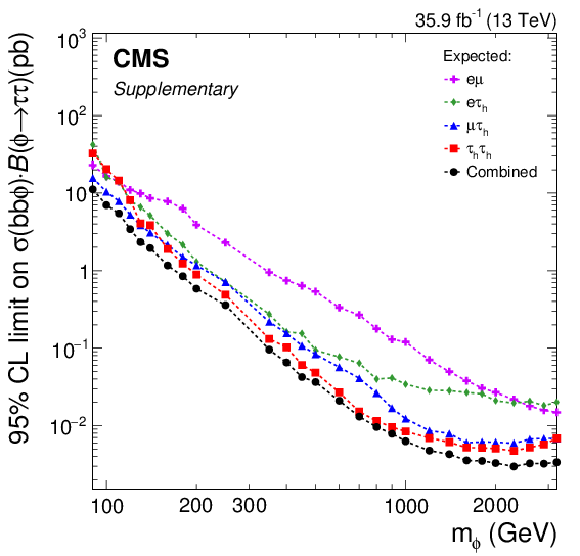
png pdf |
Additional Figure 10:
Expected 95% CL upper limits for the production of a single narrow resonance ϕ with a mass between 90 GeV and 3.2 TeV in association with b quarks (bbϕ) in the ττ final state. The individual contributions to the combined analysis have been split by the decay modes of the final state τ leptons. For these limits the SM Higgs boson has been added to the non-Higgs SM background. |
png pdf |
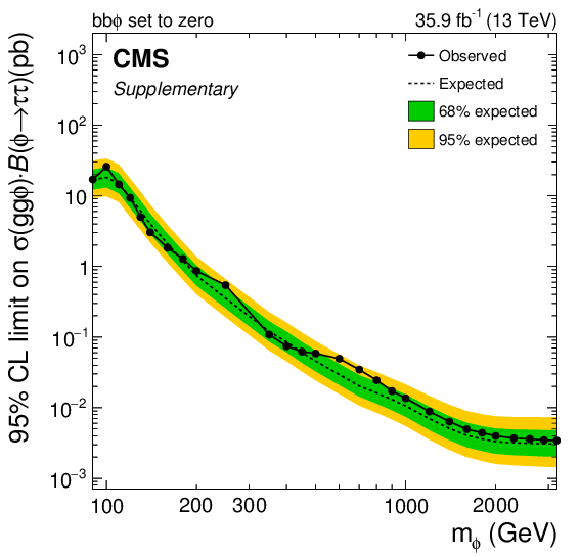
Additional Figure 11:
Expected and observed 95% CL upper limits for the production of a single narrow resonance ϕ with a mass between 90 GeV and 3.2 TeV via gluon-fusion (ggϕ) in the ττ final state. For these limits the cross section for the production in association with b quarks (bbϕ) has been set to zero; the SM Higgs boson has been added to the non-Higgs SM background. |
png pdf |
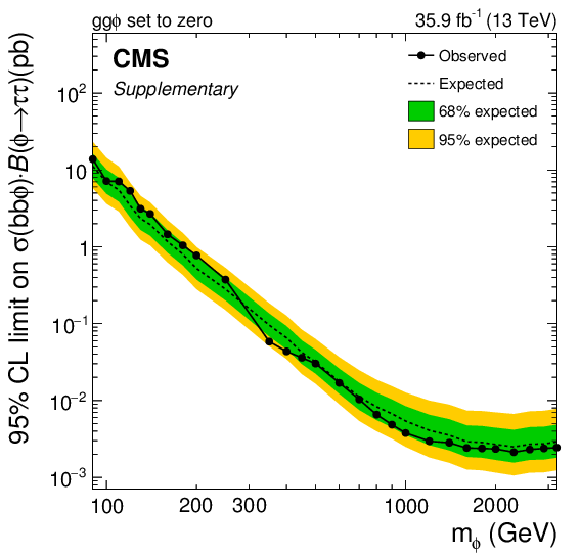
Additional Figure 12:
Expected and observed 95% CL upper limits for the production of a single narrow resonance ϕ with a mass between 90 GeV and 3.2 TeV in association with b quarks (bbϕ) in the ττ final state. For these limits the cross section for the production via gluon-fusion has been set to zero; the SM Higgs boson has been added to the non-Higgs SM background. |

png pdf |
Additional Figure 13:
Expected and observed 95% CL upper limits for the production of a single narrow resonance ϕ with a mass between 90 GeV and 3.2 TeV via gluon-fusion (ggϕ) in the ττ final state. For these limits only b quarks have been considered in the fermion loop; the SM Higgs boson has been added to the non-Higgs SM background. |

png pdf |
Additional Figure 14:
Expected and observed 95% CL upper limits for the production of a single narrow resonance ϕ with a mass between 90 GeV and 3.2 TeV via gluon-fusion (ggϕ) in the ττ final state. For these limits only t quarks have been considered in the fermion loop; the SM Higgs boson has been added to the non-Higgs SM background. |
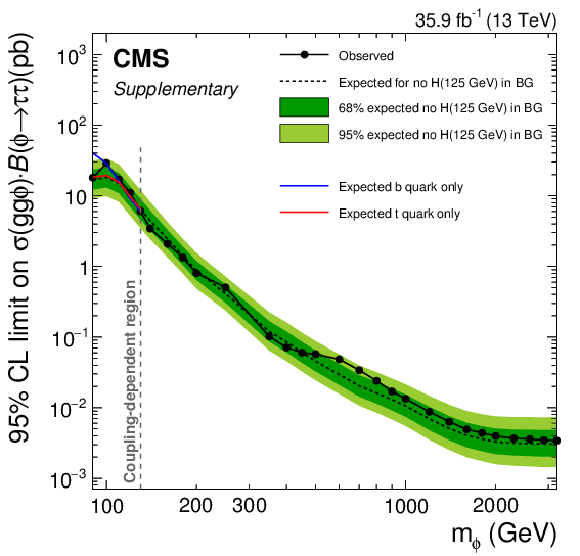
png pdf |
Additional Figure 15:
Expected and observed 95% CL upper limits for the production of a single narrow resonance ϕ with a mass between 90 GeV and 3.2 TeV via gluon-fusion (ggϕ) in the ττ final state. For these limits the SM Higgs boson has not been included in the background model. The expected exclusion limits for the cases where (blue continuous line) only the b quark and (red continuous line) only the t quark are taken into account in the fermion loop are also shown. |
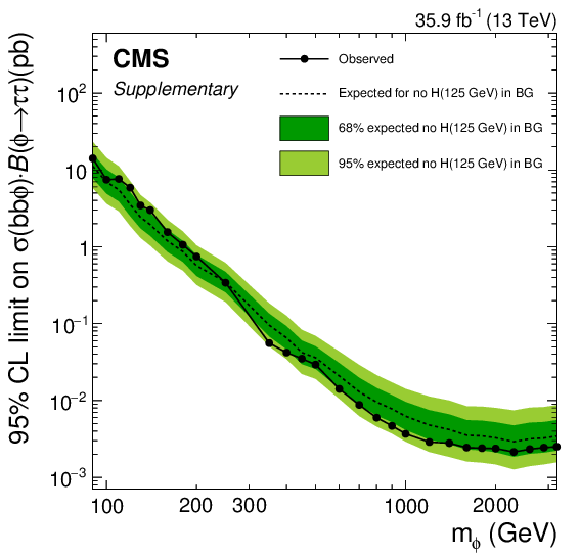
png pdf |
Additional Figure 16:
Expected and observed 95% CL upper limits for the production of a single narrow resonance ϕ with a mass between 90 GeV and 3.2 TeV in association with b quarks (bbϕ) in the ττ final state. For these limits the SM Higgs boson has not been included in the background model. |

png pdf |
Additional Figure 17:
Expected and observed 95% CL upper limits for the production of a single narrow resonance ϕ with a mass between 90 GeV and 3.2 TeV via gluon-fusion (ggϕ) in the ττ final state. For these limits only b quarks have been considered in the fermion loop; the SM Higgs boson has not been included in the background model. |

png pdf |
Additional Figure 18:
Expected and observed 95% CL upper limits for the production of a single narrow resonance ϕ with a mass between 90 GeV and 3.2 TeV via gluon-fusion (ggϕ) in the ττ final state. For these limits only t quarks have been considered in the fermion loop; the SM Higgs boson has not been included in the background model. |
png pdf |
Additional Figure 19:
Scan of the likelihood function used for the search for a single narrow resonance ϕ, with a mass of 100 GeV, produced via gluon-fusion or in association with b quarks, in the ττ final state. For this scan the SM Higgs boson has not been included in the background model. Instead the expected signal for a SM Higgs boson at 125 GeV is indicated by a red diamond. |
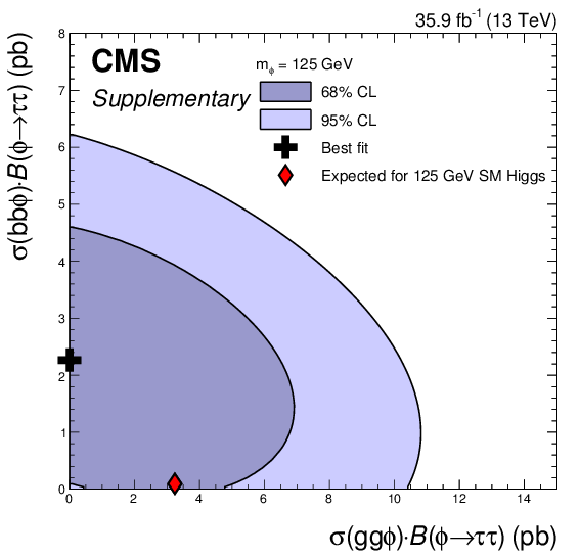
png pdf |
Additional Figure 20:
Scan of the likelihood function used for the search for a single narrow resonance ϕ, with a mass of 125 GeV, produced via gluon-fusion or in association with b quarks, in the ττ final state. For this scan the SM Higgs boson has not been included in the background model. Instead the expected signal for a SM Higgs boson at 125 GeV is indicated by a red diamond. |
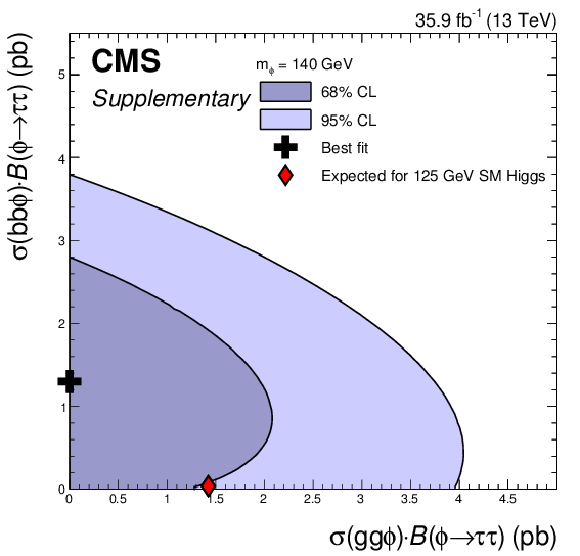
png pdf |
Additional Figure 21:
Scan of the likelihood function used for the search for a single narrow resonance ϕ, with a mass of 140 GeV, produced via gluon-fusion or in association with b quarks, in the ττ final state. For this scan the SM Higgs boson has not been included in the background model. Instead the expected signal for a SM Higgs boson at 125 GeV is indicated by a red diamond. |
png pdf |
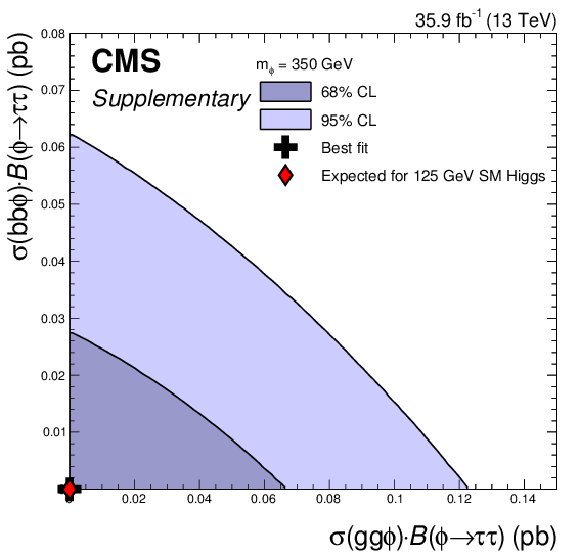
Additional Figure 22:
Scan of the likelihood function used for the search for a single narrow resonance ϕ, with a mass of 180 GeV, produced via gluon-fusion or in association with b quarks, in the ττ final state. For this scan the SM Higgs boson has not been included in the background model. Instead the expected signal for a SM Higgs boson at 125 GeV is indicated by a red diamond. |
png pdf |
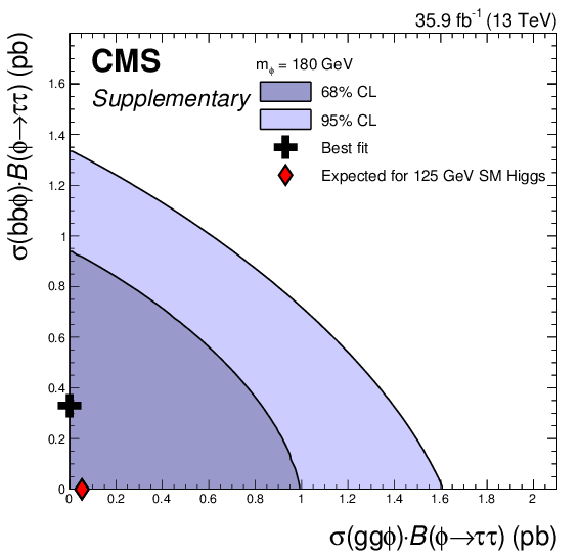
Additional Figure 23:
Scan of the likelihood function used for the search for a single narrow resonance ϕ, with a mass of 350 GeV, produced via gluon-fusion or in association with b quarks, in the ττ final state. For this scan the SM Higgs boson has not been included in the background model. Instead the expected signal for a SM Higgs boson at 125 GeV is indicated by a red diamond. |

png pdf |
Additional Figure 24:
Scan of the likelihood function used for the search for a single narrow resonance ϕ, with a mass of 700 GeV, produced via gluon-fusion or in association with b quarks, in the ττ final state. For this scan the SM Higgs boson has not been included in the background model. Instead the expected signal for a SM Higgs boson at 125 GeV is indicated by a red diamond. |

png pdf |
Additional Figure 25:
Composition of the data in the application region (AR) used for the fake factor method, split by processes and as expected from the simulation, in the μτh final state. |

png pdf |
Additional Figure 26:
Misidentification factor, FFt¯t, as obtained from simulation as a function of the transverse momentum of the misidentified jet in the 1-prong Njet≥ 0 category, in the μτh final state. |
png pdf |
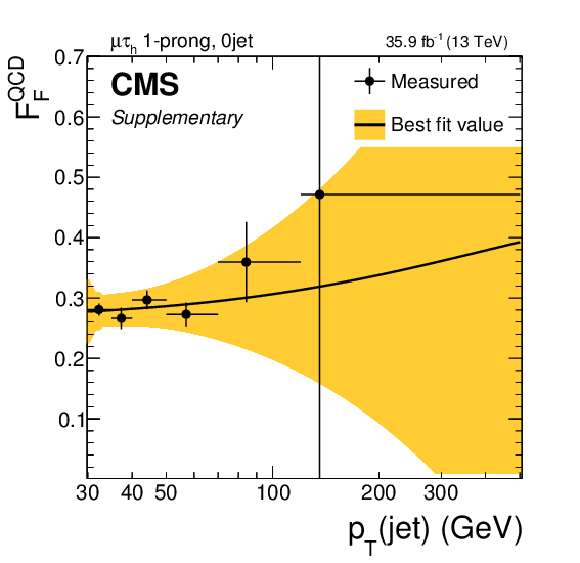
Additional Figure 27:
Misidentification factor, FFQCD, as obtained from the determination region DRQCD as a function of the transverse momentum of the misidentified jet in the 1-prong Njet= 0 category, in the μτh final state. |
png pdf |
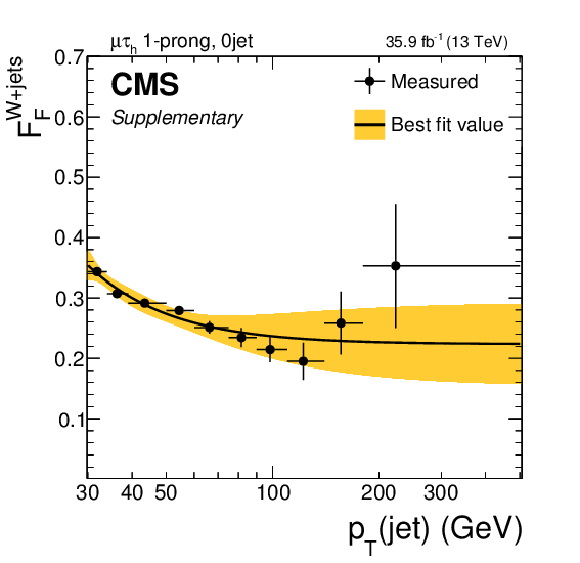
Additional Figure 28:
Misidentification factor, FFW+jets, as obtained from DRW+jets as a function of the transverse momentum of the misidentified jet in the 1-prong Njet= 0 category, in the μτh final state. |
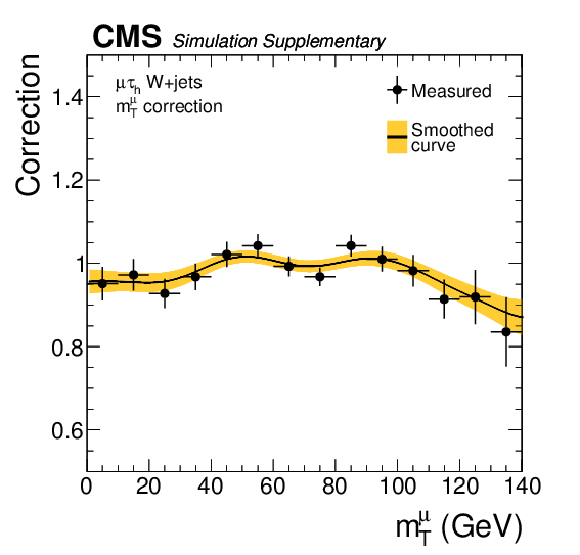
png pdf |
Additional Figure 29:
Correction to FFW+jets to account for differences between the event kinematics in the application region (AR) and signal region (SR) relative to the determination region DRW+jets, in the μτh final state. The correction has been obtained as a function of mμT from the simulation. |

png pdf |
Additional Figure 30:
Correction to FFQCD to account for differences in the event selection due to the opposite charge requirement on the selected ττ pair in the SR with respect to the same charge requirement in DRQCD, in the μτh final state. This correction has been obtained as a function of the mass of the visible decay products of the ττ system, mvis, from a sideband region in data, where the isolation requirement on the muon has been chosen to be orthogonal to the SR. |

png pdf |
Additional Figure 31:
Composition of the data in the AR used for the fake factor method, split by processes and as expected from the simulation, in the eτh final state. |
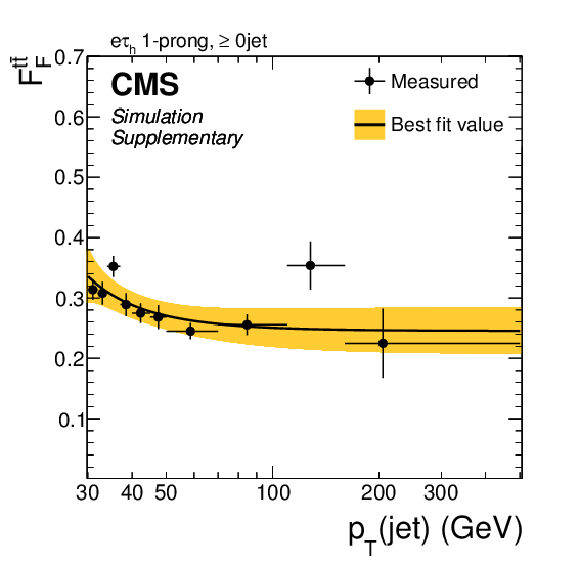
png pdf |
Additional Figure 32:
Misidentification factor, FFt¯t, as obtained from simulation as a function of the transverse momentum of the misidentified jet in the 1-prong Njet≥ 0 category, in the eτh final state. |
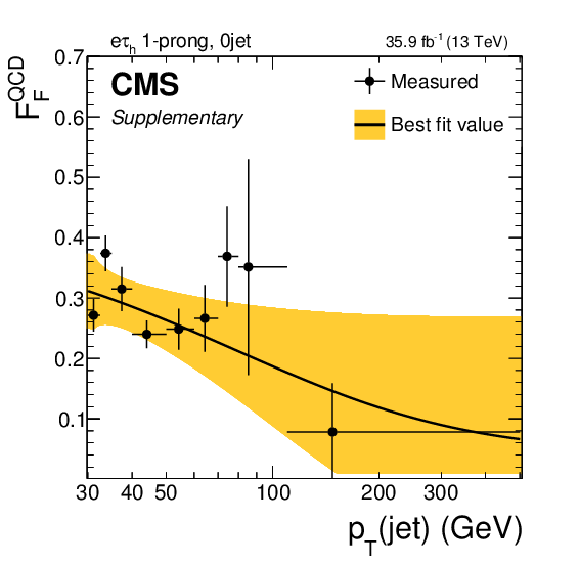
png pdf |
Additional Figure 33:
Misidentification factor, FFQCD, as obtained from DRQCD as a function of the transverse momentum of the misidentified jet in the 1-prong Njet= 0 category, in the eτh final state. |
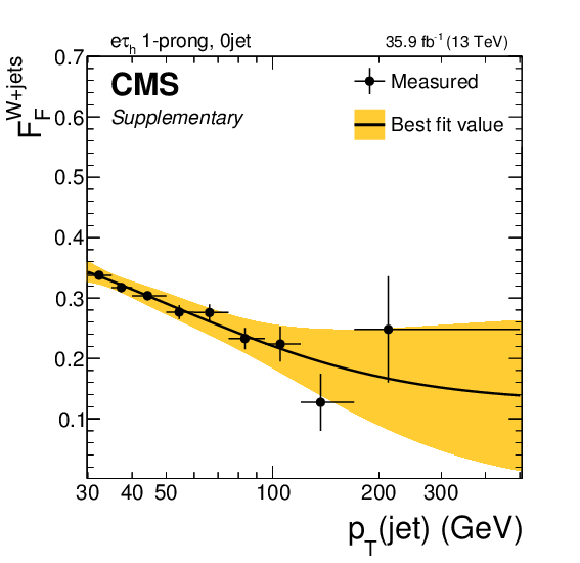
png pdf |
Additional Figure 34:
Misidentification factor, FFW+jets, as obtained from DRW+jets as a function of the transverse momentum of the misidentified jet in the 1-prong Njet= 0 category, in the eτh final state. |
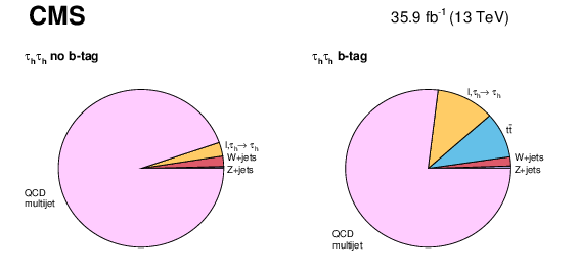
png pdf |
Additional Figure 35:
Composition of the data in the AR used for the fake factor method, split by processes and as expected from the simulation, in the τhτh final state. |
png pdf |
Additional Figure 36:
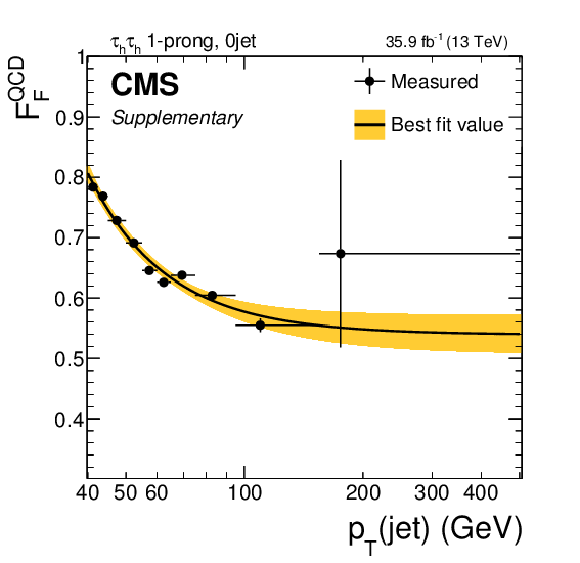
Misidentification factor, FFQCD, as obtained from DRQCD as a function of the transverse momentum of the misidentified jet in the 1-prong Njet= 0 category in the τhτh final state. |

png pdf |
Additional Figure 37:
Correction to FFQCD to account for differences in the event selection due to the opposite charge requirement on the selected ττ pair in the SR with respect to the same charge requirement in DRQCD, in the τhτh final state. This correction has been obtained as a function of the mass of the visible decay products of the ττ system, mvis, from a sideband region in data, where the isolation requirement on the other τh candidate has been chosen to be orthogonal to the SR. |
png pdf |
Additional Figure 38:
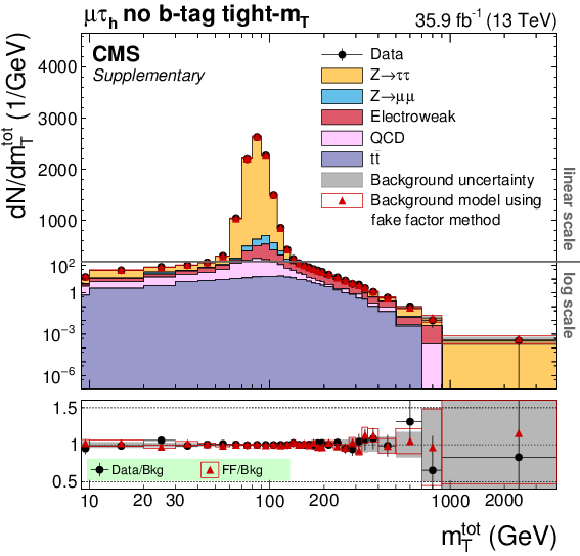
Distribution of mtotT in the no b-tag tight-mT event category in the μτh final state, using the simulation based cross check as described in the text. The triangles correspond to the background estimate from the fake factor method. The boxes enclosing the triangles correspond to the combined background uncertainty when using the fake factor method. |

png pdf |
Additional Figure 39:
Distribution of mtotT in the b-tag tight-mT event category in the μτh final state, using the simulation based cross check as described in the text. The triangles correspond to the background estimate obtained from the fake factor method. The boxes enclosing the triangles correspond to the combined background uncertainty when using the fake factor method. |
png pdf |
Additional Figure 40:
Distribution of mtotT in the no b-tag tight-mT event category in the eτh final state, using the simulation based cross check as described in the text. The triangles correspond to the background estimate obtained from the fake factor method. The boxes enclosing the triangles correspond to the combined background uncertainty when using the fake factor method. |
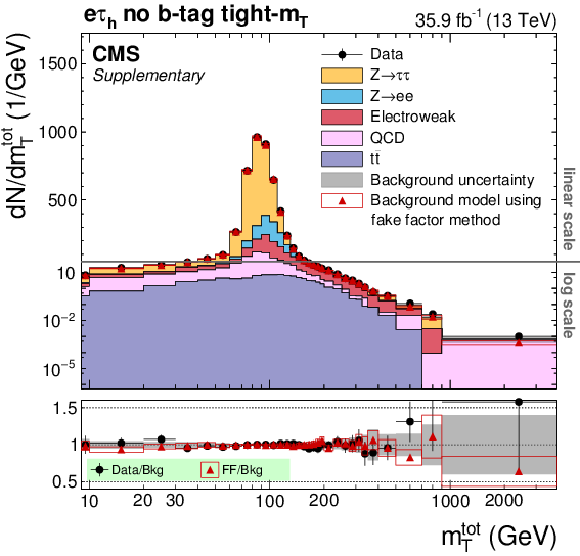
png pdf |
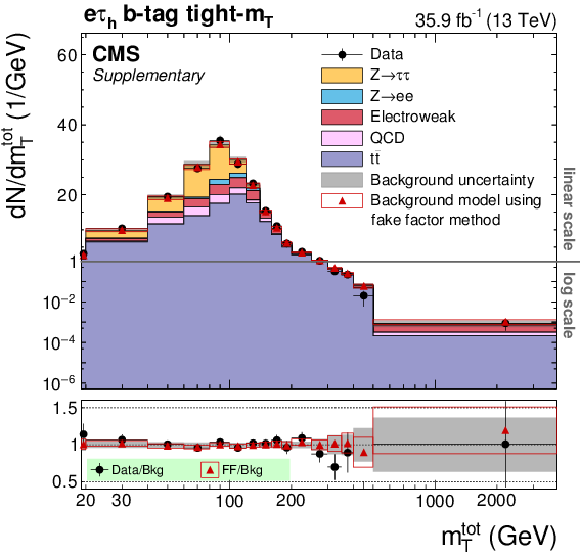
Additional Figure 41:
Distribution of mtotT in the b-tag tight-mT event category in the eτh final state, using the simulation based cross check as described in the text. The triangles correspond to the background estimate obtained from the fake factor method. The boxes enclosing the triangles correspond to the combined background uncertainty when using the fake factor method. |
png pdf |
Additional Figure 42:
Distribution of mtotT in the no b-tag tight-mT event category in the μτh final state. Shown is a comparison of the estimate from data using the μ→τ embedding technique with the estimate of the relevant processes from simulation as used for the main analysis, before performing the fit for the statistical inference of the signal. |
png pdf |
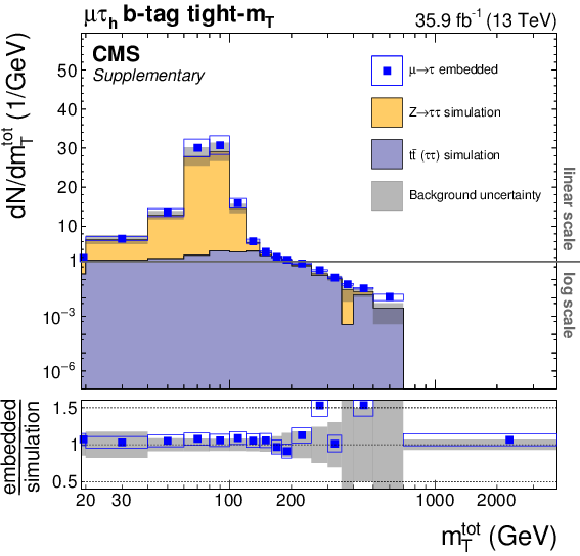
Additional Figure 43:
Distribution of mtotT in the b-tag tight-mT event category in the μτh final state. Shown is a comparison of the estimate from data using the μ→τ embedding technique with the estimate of the relevant processes from simulation as used for the main analysis, before performing the fit for the statistical inference of the signal. |
png pdf |
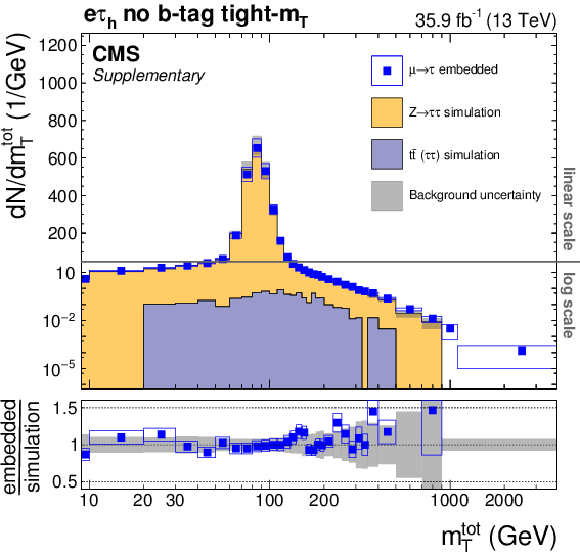
Additional Figure 44:
Distribution of mtotT in the no b-tag tight-mT event category in the eτh final state. Shown is a comparison of the estimate from data using the μ→τ embedding technique with the estimate of the relevant processes from simulation as used for the main analysis, before performing the fit for the statistical inference of the signal. |
png pdf |
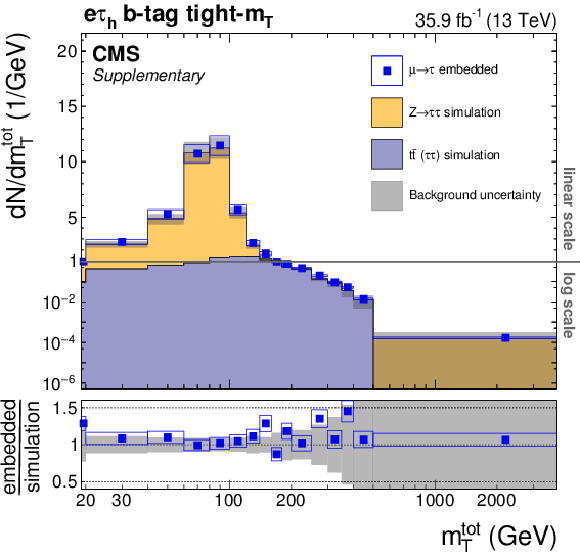
Additional Figure 45:
Distribution of mtotT in the b-tag tight-mT event category in the eτh final state. Shown is a comparison of the estimate from data using the μ→τ embedding technique with the estimate of the relevant processes from simulation as used for the main analysis, before performing the fit for the statistical inference of the signal. |
png pdf |
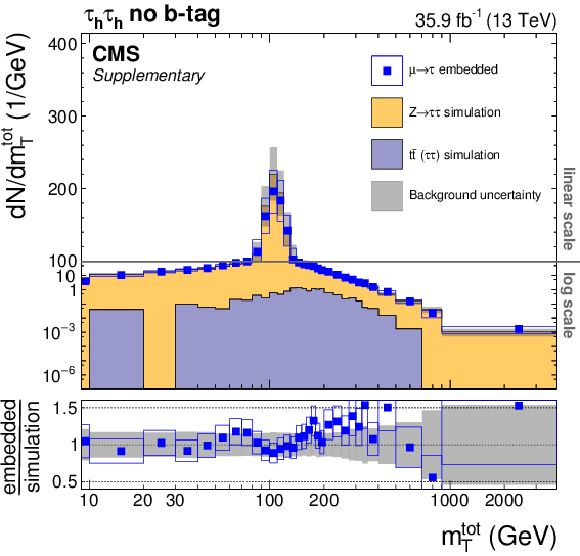
Additional Figure 46:
Distribution of mtotT in the no b-tag tight-mT event category in the τhτh final state. Shown is a comparison of the estimate from data using the μ→τ embedding technique with the estimate of the relevant processes from simulation as used for the main analysis, before performing the fit for the statistical inference of the signal. |

png pdf |
Additional Figure 47:
Distribution of mtotT in the b-tag tight-mT event category in the τhτh final state. Shown is a comparison of the estimate from data using the μ→τ embedding technique with the estimate of the relevant processes from simulation as used for the main analysis, before performing the fit for the statistical inference of the signal. |

png pdf |
Additional Figure 48:
Expected and observed 95% CL exclusion contour in the MSSM M125h scenario, as proposed in arxiv:1808.07542. The expected median is shown as a dashed black line. The dark and bright gray bands indicate the 68 and 95% confidence intervals for the variation of the expected exclusion. The observed exclusion contour is indicated by the colored blue area. For the M125h scenario, those parts of the parameter space, where mh deviates by more than ±3 GeV from the mass of the observed Higgs boson at 125 GeV are indicated by a red hatched area. |
png pdf |
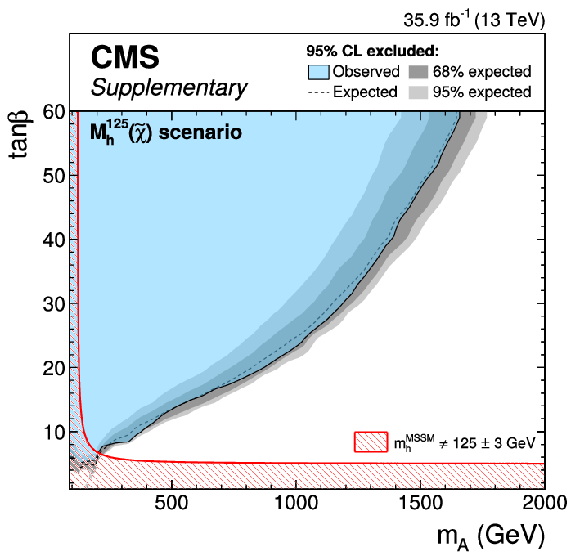
Additional Figure 49:
Expected and observed 95% CL exclusion contour in the MSSM M125h(˜χ) scenario, as proposed in arxiv:1808.07542. The expected median is shown as a dashed black line. The dark and bright gray bands indicate the 68 and 95% confidence intervals for the variation of the expected exclusion. The observed exclusion contour is indicated by the colored blue area. For the M125h(˜χ) scenario, those parts of the parameter space, where mh deviates by more than ± 3 GeV from the mass of the observed Higgs boson at 125 GeV are indicated by a red hatched area. |
png pdf |
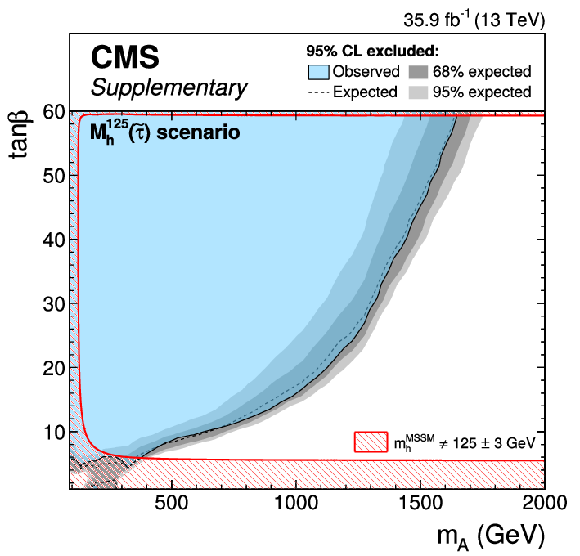
Additional Figure 50:
Expected and observed 95% CL exclusion contour in the MSSM M125h(˜τ) scenario, as proposed in arxiv:1808.07542. The expected median is shown as a dashed black line. The dark and bright gray bands indicate the 68 and 95% confidence intervals for the variation of the expected exclusion. The observed exclusion contour is indicated by the colored blue area. For the M125h(˜τ) scenario, those parts of the parameter space, where mh deviates by more than ± 3 GeV from the mass of the observed Higgs boson at 125 GeV are indicated by a red hatched area. |
| Additional Tables | |

png pdf |
Additional Table 1:
Expected and observed number of selected events in each event category in the eμ final state. The expected number of events and corresponding uncertainties are shown prior to the fit used for the signal extraction, taking into account the correlations across channels and final states. Some uncertainties, as described in the text, alter the shape of the mtotT distribution; the components of these that influence the overall normalization are taken into account. For the SM Higgs boson, the expectations from only gluon fusion and VBF are shown. The yield for the signal is given for a single narrow resonance ϕ with a cross section of 1 pb for each of the production via gluon fusion and in association with b quarks. For gluon fusion the contributions due to the b and t quarks are chosen as expected for the SM Higgs boson at the given mass. The yields are given for four different mass values. |
png pdf |
Additional Table 2:
Expected and observed number of selected events in each event category in the eτh final state. The expected number of events and corresponding uncertainties are shown prior to the fit used for the signal extraction, taking into account the correlations across channels and final states. Some uncertainties, as described in the text, alter the shape of the mtotT distribution; the components of these that influence the overall normalization are taken into account. For the SM Higgs boson, the expectations from only gluon fusion and VBF are shown. The yield for the signal is given for a single narrow resonance ϕ with a cross section of 1 pb for each of the production via gluon fusion and in association with b quarks. For gluon fusion the contributions due to the b and t quarks are chosen as expected for the SM Higgs boson at the given mass. The yields are given for four different mass values. |

png pdf |
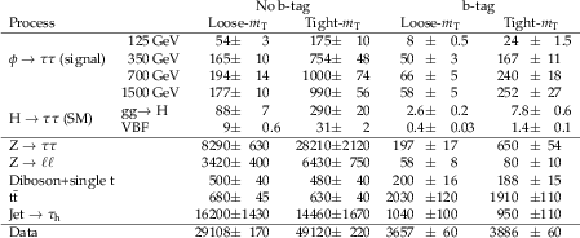
Additional Table 3:
Expected and observed number of selected events in each event category in the μτh final state. The expected number of events and corresponding uncertainties are shown prior to the fit used for the signal extraction, taking into account the correlations across channels and final states. Some uncertainties, as described in the text, alter the shape of the mtotT distribution; the components of these that influence the overall normalization are taken into account. For the SM Higgs boson, the expectations from only gluon fusion and VBF are shown. The yield for the signal is given for a single narrow resonance ϕ with a cross section of 1 pb for each of the production via gluon fusion and in association with b quarks. For gluon fusion the contributions due to the b and t quarks are chosen as expected for the SM Higgs boson at the given mass. The yields are given for four different mass values. |
png pdf |
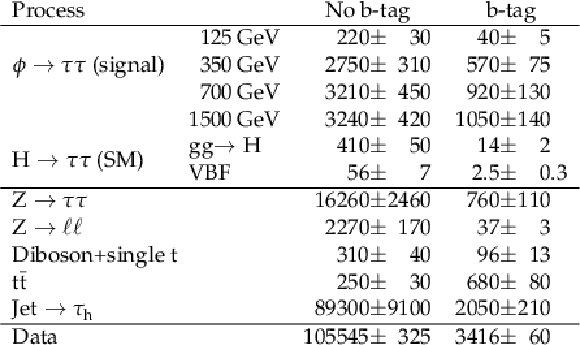
Additional Table 4:
Expected and observed number of selected events in each event category in the τhτh final state. The expected number of events and corresponding uncertainties are shown prior to the fit used for the signal extraction, taking into account the correlations across channels and final states. Some uncertainties, as described in the text, alter the shape of the mtotT distribution; the components of these that influence the overall normalization are taken into account. For the SM Higgs boson, the expectations from only gluon fusion and VBF are shown. The yield for the signal is given for a single narrow resonance ϕ with a cross section of 1 pb for each of the production via gluon fusion and in association with b quarks. For gluon fusion the contributions due to the b and t quarks are chosen as expected for the SM Higgs boson at the given mass. The yields are given for four different mass values. |
png pdf |
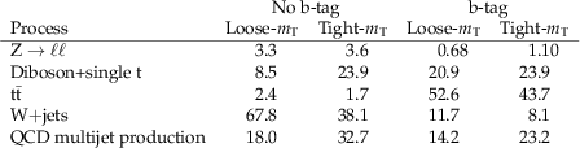
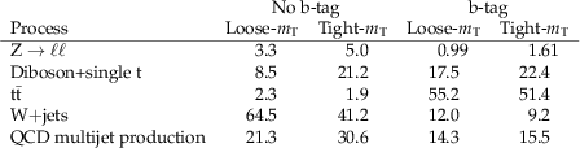
Additional Table 5:
Expected composition, from simulation, of the AR in the fake factor method in the event categories used for the analysis, split by process, in the μτh final state. The relative contribution is given in %. |
png pdf |
Additional Table 6:
Expected composition, from simulation, of the AR in the fake factor method in the event categories used for the analysis, split by process, in the eτh final state. The relative contribution is given in %. |

png pdf |
Additional Table 7:
Expected composition, from simulation, of the AR in the fake factor method in the event categories used for the analysis, split by process, in the τhτh final state. The relative contribution is given in %. |

png pdf |
Additional Table 8:
Expected composition of the AR for the estimate of QCD multijet events in the event categories used for the analysis, split by process, in the eμ final. All processes but QCD multijet production are estimated from the simulation. The relative contribution is given in %. |
| Database for the 2 dimensional likelihood scans |
| References | ||||
| 1 | ATLAS Collaboration | Observation of a new particle in the search for the standard model Higgs boson with the ATLAS detector at the LHC | PLB 716 (2012) 1 | 1207.7214 |
| 2 | CMS Collaboration | Observation of a new boson at a mass of 125 GeV with the CMS experiment at the LHC | PLB 716 (2012) 30 | CMS-HIG-12-028 1207.7235 |
| 3 | CMS Collaboration | Observation of a new boson with mass near 125 GeV in pp collisions at √s= 7 and 8 TeV | JHEP 06 (2013) 081 | CMS-HIG-12-036 1303.4571 |
| 4 | P. W. Higgs | Broken symmetries, massless particles and gauge fields | PL12 (1964) 132 | |
| 5 | P. W. Higgs | Broken symmetries and the masses of gauge bosons | PRL 13 (1964) 508 | |
| 6 | G. S. Guralnik, C. R. Hagen, and T. W. B. Kibble | Global conservation laws and massless particles | PRL 13 (1964) 585 | |
| 7 | P. W. Higgs | Spontaneous symmetry breakdown without massless bosons | PR145 (1966) 1156 | |
| 8 | T. W. B. Kibble | Symmetry breaking in non-abelian gauge theories | PR155 (1967) 1554 | |
| 9 | F. Englert and R. Brout | Broken symmetry and the mass of gauge vector mesons | PRL 13 (1964) 321 | |
| 10 | ATLAS and CMS Collaboration | Combined measurement of the Higgs boson mass in pp collisions at √s= 7 and 8 TeV with the ATLAS and CMS experiments | PRL 114 (2015) 191803 | 1503.07589 |
| 11 | ATLAS and CMS Collaboration | Measurements of the Higgs boson production and decay rates and constraints on its couplings from a combined ATLAS and CMS analysis of the LHC pp collision data at √s= 7 and 8 TeV | JHEP 08 (2016) 045 | 1606.02266 |
| 12 | Y. A. Gol'fand and E. P. Likhtman | Extension of the algebra of Poincar\'e group generators and violation of P invariance | JEPTL 13 (1971)323 | |
| 13 | J. Wess and B. Zumino | Supergauge transformations in four-dimensions | NPB 70 (1974) 39 | |
| 14 | P. Fayet | Supergauge invariant extension of the Higgs mechanism and a model for the electron and its neutrino | NPB 90 (1975) 104 | |
| 15 | P. Fayet | Spontaneously broken supersymmetric theories of weak, electromagnetic and strong interactions | PLB 69 (1977) 489 | |
| 16 | M. Carena et al. | MSSM Higgs boson searches at the LHC: Benchmark scenarios after the discovery of a Higgs-like particle | EPJC 73 (2013) 2552 | 1302.7033 |
| 17 | ATLAS Collaboration | Search for additional heavy neutral Higgs and gauge bosons in the ditau final state produced in 36fb^{-1} of pp collisions at √s= 13 TeV with the ATLAS detector | JHEP 01 (2017) 052 | 1709.07242 |
| 18 | CMS Collaboration | Search for neutral MSSM Higgs bosons decaying to a pair of tau leptons in pp collisions | JHEP 10 (2014) 160 | CMS-HIG-13-021 1408.3316 |
| 19 | DELPHI, OPAL, ALEPH, L3 and LEP Working Group for Higgs Boson Searches Collaboration | Search for neutral MSSM Higgs bosons at LEP | EPJC 47 (2006) 547 | hep-ex/0602042 |
| 20 | CDF Collaboration | Search for Higgs bosons predicted in Two-Higgs-Doublet models via decays to tau lepton pairs in 1.96 TeV pˉp collisions | PRL 103 (2009) 201801 | 0906.1014 |
| 21 | D0 Collaboration | Search for neutral Higgs bosons in the multi-b-jet topology in 5.2fb^{-1} of pˉp collisions at √s= 1.96 TeV | PLB 698 (2011) 97 | 1011.1931 |
| 22 | D0 Collaboration | Search for Higgs bosons decaying to τ+τ− pairs in pˉp collisions at √s= 1.96 TeV | PLB 707 (2012) 323 | 1106.4555 |
| 23 | CDF Collaboration | Search for Higgs bosons produced in association with b-quarks | PRD 85 (2012) 032005 | 1106.4782 |
| 24 | CMS Collaboration | Search for a Higgs boson decaying into a b-quark pair and produced in association with b quarks in proton-proton collisions at 7 TeV | PLB 722 (2013) 207 | CMS-HIG-12-033 1302.2892 |
| 25 | CMS Collaboration | Search for neutral MSSM Higgs bosons decaying into a pair of bottom quarks | JHEP 11 (2015) 071 | CMS-HIG-14-017 1506.08329 |
| 26 | ATLAS Collaboration | Search for the neutral Higgs bosons of the minimal supersymmetric standard model in pp collisions at √s= 7 TeV with the ATLAS detector | JHEP 02 (2013) 095 | 1211.6956 |
| 27 | CMS Collaboration | Search for neutral MSSM Higgs bosons decaying to μ+μ− in pp collisions at √s= 7 and 8 TeV | PLB 752 (2016) 221 | CMS-HIG-13-024 1508.01437 |
| 28 | ATLAS Collaboration | Search for neutral Higgs bosons of the minimal supersymmetric standard model in pp collisions at √s= 8 TeV with the ATLAS detector | JHEP 11 (2014) 056 | 1409.6064 |
| 29 | ATLAS Collaboration | Search for minimal supersymmetric standard model Higgs bosons H/A and for a Z′ boson in the ττ final state produced in pp collisions at √s= 13 TeV with the ATLAS detector | EPJC 76 (2016) 585 | 1608.00890 |
| 30 | CMS Collaboration | Search for neutral minimal supersymmetric standard model Higgs bosons decaying to tau pairs in pp collisions at √s= 7 TeV | PRL 106 (2011) 231801 | CMS-HIG-10-002 1104.1619 |
| 31 | CMS Collaboration | Search for neutral Higgs bosons decaying to tau pairs in pp collisions at √s= 7 TeV | PLB 713 (2012) 68 | CMS-HIG-11-029 1202.4083 |
| 32 | CMS Collaboration | Description and performance of track and primary-vertex reconstruction with the CMS tracker | JINST 9 (2014) P10009 | CMS-TRK-11-001 1405.6569 |
| 33 | CMS Collaboration | Performance of electron reconstruction and selection with the CMS detector in proton-proton collisions at √s= 8 TeV | JINST 10 (2015) P06005 | CMS-EGM-13-001 1502.02701 |
| 34 | CMS Collaboration | Performance of CMS muon reconstruction in pp collision events at √s= 7 TeV | JINST 7 (2012) P10002 | CMS-MUO-10-004 1206.4071 |
| 35 | CMS Collaboration | Performance of photon reconstruction and identification with the CMS detector in proton-proton collisions at √s= 8 TeV | JINST 10 (2015) P08010 | CMS-EGM-14-001 1502.02702 |
| 36 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 37 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | CMS-00-001 |
| 38 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 39 | K. Rose | Deterministic annealing for clustering, compression, classification, regression, and related optimization problems | Proceedings of the IEEE 86 (1998) 2210 | |
| 40 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-kT jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 41 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 42 | CMS Collaboration | Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV | Submitted to \it JINST | CMS-BTV-16-002 1712.07158 |
| 43 | CMS Collaboration | Reconstruction and identification of τlepton decays to hadrons and gnτ at CMS | JINST 11 (2016) P01019 | CMS-TAU-14-001 1510.07488 |
| 44 | CMS Collaboration | Performance of reconstruction and identification of τ leptons in their decays to hadrons and ντ in LHC Run-2 | CMS-PAS-TAU-16-002 | CMS-PAS-TAU-16-002 |
| 45 | CDF Collaboration | Search for neutral Higgs bosons of the minimal supersymmetric standard model decaying to τ pairs in pˉp collisions at √s= 1.96 TeV | PRL 96 (2006) 011802 | hep-ex/0508051 |
| 46 | J. Alwall et al. | MadGraph 5: going beyond | JHEP 06 (2011) 128 | 1106.0522 |
| 47 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 48 | P. Nason | A new method for combining NLO QCD with shower Monte Carlo algorithms | JHEP 11 (2004) 040 | hep-ph/0409146 |
| 49 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with parton shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 50 | S. Alioli, P. Nason, C. Oleari, and E. Re | NLO Higgs boson production via gluon fusion matched with shower in POWHEG | JHEP 04 (2009) 002 | 0812.0578 |
| 51 | S. Alioli, P. Nason, C. Oleari, and E. Re | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX | JHEP 06 (2010) 043 | 1002.2581 |
| 52 | S. Alioli et al. | Jet pair production in POWHEG | JHEP 04 (2011) 081 | 1012.3380 |
| 53 | E. Bagnaschi, G. Degrassi, P. Slavich, and A. Vicini | Higgs production via gluon fusion in the POWHEG approach in the SM and in the MSSM | JHEP 02 (2012) 088 | 1111.2854 |
| 54 | K. Melnikov and F. Petriello | Electroweak gauge boson production at hadron colliders through O(α2s) | PRD 74 (2006) 114017 | hep-ph/0609070 |
| 55 | M. Czakon and A. Mitov | Top++: A program for the calculation of the top-pair cross-section at hadron colliders | CPC 185 (2014) 2930 | 1112.5675 |
| 56 | N. Kidonakis | Top quark production | in Proceedings, Helmholtz International Summer School on Physics of Heavy Quarks and Hadrons (HQ 2013), p. 139 JINR, Dubna, Russia, July | 1311.0283 |
| 57 | J. M. Campbell, R. K. Ellis, and C. Williams | Vector boson pair production at the LHC | JHEP 07 (2011) 018 | 1105.0020 |
| 58 | T. Sjostrand et al. | An introduction to PYTHIA 8.2 | CPC 191 (2015) 159 | 1410.3012 |
| 59 | E. Bagnaschi et al. | Resummation ambiguities in the Higgs transverse-momentum spectrum in the standard model and beyond | JHEP 01 (2016) 090 | 1510.08850 |
| 60 | E. Bagnaschi and A. Vicini | The Higgs transverse momentum distribution in gluon fusion as a multiscale problem | JHEP 01 (2016) 056 | 1505.00735 |
| 61 | R. V. Harlander, H. Mantler, and M. Wiesemann | Transverse momentum resummation for Higgs production via gluon fusion in the MSSM | JHEP 11 (2014) 116 | 1409.0531 |
| 62 | NNPDF Collaboration | Parton distributions for the LHC run II | JHEP 04 (2015) 040 | 1410.8849 |
| 63 | CMS Collaboration | Event generator tunes obtained from underlying event and multiparton scattering measurements | EPJC 76 (2016) 155 | CMS-GEN-14-001 1512.00815 |
| 64 | GEANT4 Collaboration | GEANT4---a simulation toolkit | NIMA 506 (2003) 250 | |
| 65 | CMS Collaboration | Measurement of the Zγ∗→ττ cross section in pp collisions at √s= 13 TeV and validation of τ lepton analysis techniques | Submitted to \it EPJC | CMS-HIG-15-007 1801.03535 |
| 66 | CMS Collaboration | Measurements of inclusive W and Z cross sections in pp collisions at √s= 7 TeV | JHEP 01 (2011) 080 | CMS-EWK-10-002 1012.2466 |
| 67 | CMS Collaboration | Measurement of the differential cross section for top quark pair production in pp collisions at √s= 8 TeV | EPJC 75 (2015) 542 | CMS-TOP-12-028 1505.04480 |
| 68 | CMS Collaboration | Evidence for the 125 GeV Higgs boson decaying to a pair of τ leptons | JHEP 05 (2014) 104 | CMS-HIG-13-004 1401.5041 |
| 69 | ATLAS Collaboration | Modelling Z→ττ processes in ATLAS with τ-embedded Z→μμ data | JINST 10 (2015) P09018 | 1506.05623 |
| 70 | A. L. Read | Linear interpolation of histograms | NIMA 425 (1999) 357 | |
| 71 | CMS Collaboration | CMS luminosity measurements for the 2016 data taking period | CMS-PAS-LUM-17-001 | CMS-PAS-LUM-17-001 |
| 72 | CMS Collaboration | Cross section measurement of t-channel single top quark production in pp collisions at √s= 13 TeV | PLB 772 (2017) 752 | CMS-TOP-16-003 1610.00678 |
| 73 | CMS Collaboration | Measurement of the WZ production cross section in pp collisions at √s= 13 TeV | PLB 766 (2017) 268 | CMS-SMP-16-002 1607.06943 |
| 74 | D. de Florian et al. | Handbook of LHC Higgs cross sections: 4. deciphering the nature of the Higgs sector | CERN-2017-002-M | 1610.07922 |
| 75 | A. D. Martin, W. J. Stirling, R. S. Thorne, and G. Watt | Parton distributions for the LHC | EPJC 63 (2009) 189 | 0901.0002 |
| 76 | A. D. Martin, W. J. Stirling, R. S. Thorne, and G. Watt | Uncertainties on αs in global PDF analyses and implications for predicted hadronic cross sections | EPJC 64 (2009) 653 | 0905.3531 |
| 77 | T. Junk | Confidence level computation for combining searches with small statistics | NIMA 434 (1999) 435 | hep-ex/9902006 |
| 78 | A. L. Read | Presentation of search results: The CLs technique | JPG 28 (2002) 2693 | |
| 79 | ATLAS and CMS Collaborations | Procedure for the LHC Higgs boson search combination in summer 2011 | ATL-PHYS-PUB 2011-11, CMS NOTE 2011/005, CERN | |
| 80 | CMS Collaboration | Combined results of searches for the standard model Higgs boson in pp collisions at √s= 7 TeV | PLB 710 (2012) 26 | CMS-HIG-11-032 1202.1488 |
| 81 | G. Cowan, K. Cranmer, E. Gross, and O. Vitells | Asymptotic formulae for likelihood-based tests of new physics | EPJC 71 (2011) 1554 | 1007.1727 |
| 82 | L. Maiani, A. D. Polosa, and V. Riquer | Bounds to the Higgs sector masses in minimal supersymmetry from LHC data | PLB 724 (2013) 274 | 1305.2172 |
| 83 | A. Djouadi et al. | The post-Higgs MSSM scenario: Habemus MSSM? | EPJC 73 (2013) 2650 | 1307.5205 |
| 84 | A. Djouadi et al. | Fully covering the MSSM Higgs sector at the LHC | JHEP 06 (2015) 168 | 1502.05653 |
| 85 | G. Degrassi et al. | Towards high-precision predictions for the MSSM Higgs sector | EPJC 28 (2003) 133 | hep-ph/0212020 |
| 86 | B. C. Allanach et al. | Precise determination of the neutral Higgs boson masses in the MSSM | JHEP 09 (2004) 044 | hep-ph/0406166 |
| 87 | S. Heinemeyer et al. | Handbook of LHC Higgs cross sections: 3. Higgs properties | CERN-2013-004 | 1307.1347 |
| 88 | R. V. Harlander, S. Liebler, and H. Mantler | SusHi: A program for the calculation of Higgs production in gluon fusion and bottom-quark annihilation in the standard model and the MSSM | CPC 184 (2013) 1605 | 1212.3249 |
| 89 | M. Spira, A. Djouadi, D. Graudenz, and P. M. Zerwas | Higgs boson production at the LHC | NPB 453 (1995) 17 | hep-ph/9504378 |
| 90 | R. V. Harlander and M. Steinhauser | Supersymmetric Higgs production in gluon fusion at next-to-leading order | JHEP 09 (2004) 066 | hep-ph/0409010 |
| 91 | R. Harlander and P. Kant | Higgs production and decay: analytic results at next-to-leading order QCD | JHEP 12 (2005) 015 | hep-ph/0509189 |
| 92 | G. Degrassi and P. Slavich | NLO QCD bottom corrections to Higgs boson production in the MSSM | JHEP 11 (2010) 044 | 1007.3465 |
| 93 | G. Degrassi, S. Di Vita, and P. Slavich | NLO QCD corrections to pseudoscalar Higgs production in the MSSM | JHEP 08 (2011) 128 | 1107.0914 |
| 94 | G. Degrassi, S. Di Vita, and P. Slavich | On the NLO QCD corrections to the production of the heaviest neutral Higgs scalar in the MSSM | EPJC 72 (2012) 2032 | 1204.1016 |
| 95 | R. V. Harlander and W. B. Kilgore | Next-to-next-to-leading order Higgs production at hadron colliders | PRL 88 (2002) 201801 | hep-ph/0201206 |
| 96 | C. Anastasiou and K. Melnikov | Higgs boson production at hadron colliders in NNLO QCD | NPB 646 (2002) 220 | hep-ph/0207004 |
| 97 | V. Ravindran, J. Smith, and W. L. van Neerven | NNLO corrections to the total cross-section for Higgs boson production in hadron-hadron collisions | NPB 665 (2003) 325 | hep-ph/0302135 |
| 98 | R. V. Harlander and W. B. Kilgore | Production of a pseudo-scalar Higgs boson at hadron colliders at next-to-next-to leading order | JHEP 10 (2002) 017 | hep-ph/0208096 |
| 99 | C. Anastasiou and K. Melnikov | Pseudoscalar Higgs boson production at hadron colliders in next-to-next-to-leading order QCD | PRD 67 (2003) 037501 | hep-ph/0208115 |
| 100 | U. Aglietti, R. Bonciani, G. Degrassi, and A. Vicini | Two-loop light fermion contribution to Higgs production and decays | PLB 595 (2004) 432 | hep-ph/0404071 |
| 101 | R. Bonciani, G. Degrassi, and A. Vicini | On the generalized harmonic polylogarithms of one complex variable | CPC 182 (2011) 1253 | 1007.1891 |
| 102 | S. Dittmaier, M. Kramer, and M. Spira | Higgs radiation off bottom quarks at the Fermilab Tevatron and the CERN LHC | PRD 70 (2004) 074010 | hep-ph/0309204 |
| 103 | S. Dawson, C. B. Jackson, L. Reina, and D. Wackeroth | Exclusive Higgs boson production with bottom quarks at hadron colliders | PRD 69 (2004) 074027 | hep-ph/0311067 |
| 104 | R. V. Harlander and W. B. Kilgore | Higgs boson production in bottom quark fusion at next-to-next-to-leading order | PRD 68 (2003) 013001 | hep-ph/0304035 |
| 105 | R. Harlander, M. Kramer, and M. Schumacher | Bottom-quark associated Higgs-boson production: Reconciling the four- and five-flavour scheme approach | CERN-PH-TH-2011-134, FR-PHENO-2011-009, TTK-11-17, WUB-11-04 | 1112.3478 |
| 106 | S. Heinemeyer, W. Hollik, and G. Weiglein | FeynHiggs: A program for the calculation of the masses of the neutral CP-even Higgs bosons in the MSSM | CPC 124 (2000) 76 | hep-ph/9812320 |
| 107 | S. Heinemeyer, W. Hollik, and G. Weiglein | The masses of the neutral CP-even Higgs bosons in the MSSM: Accurate analysis at the two-loop level | EPJC 9 (1999) 343 | hep-ph/9812472 |
| 108 | M. Frank et al. | The Higgs boson masses and mixings of the complex MSSM in the Feynman-diagrammatic approach | JHEP 02 (2007) 047 | hep-ph/0611326 |
| 109 | T. Hahn et al. | High-precision predictions for the light CP-even Higgs boson mass of the minimal supersymmetric standard model | PRL 112 (2014) 141801 | 1312.4937 |
| 110 | A. Djouadi, J. Kalinowski, and M. Spira | HDECAY: A program for Higgs boson decays in the standard model and its supersymmetric extension | CPC 108 (1998) 56 | hep-ph/9704448 |

|
Compact Muon Solenoid LHC, CERN |

|

|

|

|

|

|