CMS-PAS-TOP-23-009

CMS-PAS-TOP-23-009
Probing the flavour structure of dimension-6 EFT operators in multilepton final states
CMS Collaboration
2 April 2025

Abstract: An analysis of the flavour structure of dimension-6 effective field theory (EFT) operators in multilepton final states is presented, focusing on the interactions involving Z bosons. The flavour structure of these operators is disentangled by simultaneously probing the interactions with different quark generations in final states with at least three leptons, targetting the associated production of a top quark pair and a Z boson ( $\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}$ ) as well as diboson processes (WZ and ZZ). The data were recorded by the CMS experiment in the years 2016 to 2018 in proton-proton collisions at a centre-of-mass energy of 13 TeV and correspond to an integrated luminosity of 138 fb $^{-1}$ . Consistency with the standard model of particle physics is observed and limits on the considered EFT parameters, split into couplings to light and heavy quark generations, are set.
Links: CDS record (PDF) ; Physics Briefing ; CADI line (restricted) ;

Figures	Summary	References	CMS Publications

Figures
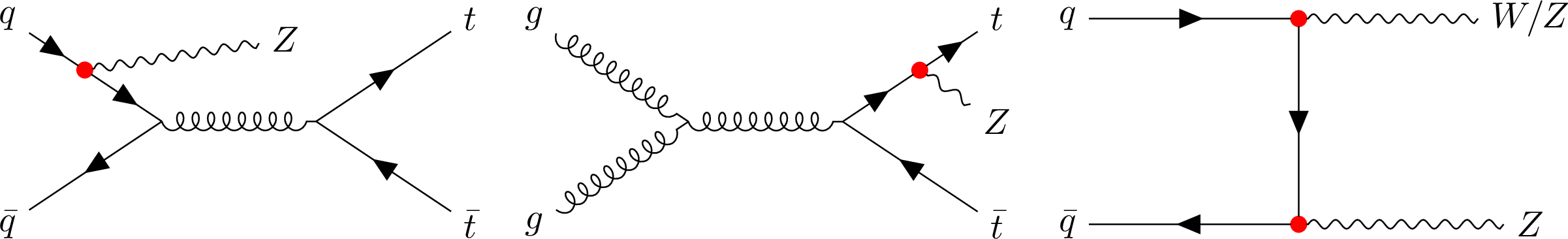
png pdf	Figure 1: Representative diagrams showing the leading-order contributions to the $\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}$ production, with the Z boson radiated from the initial-state quarks (left) and from one of the top quarks (centre). The WZ and/or ZZ production is also shown (right). The vertices affected by the EFT operators measured in this analysis are highlighted with red dots.
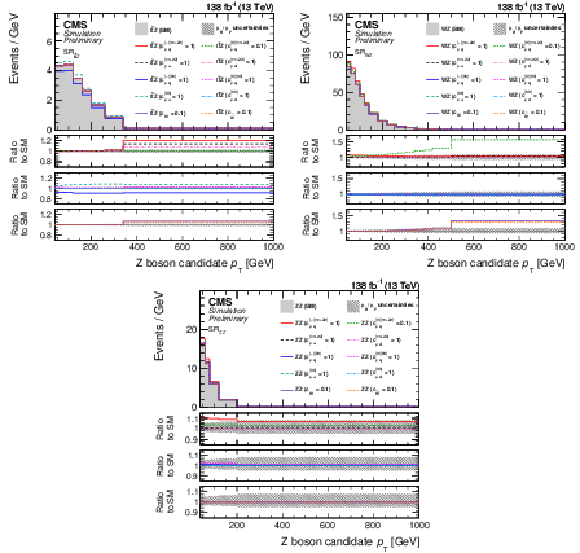
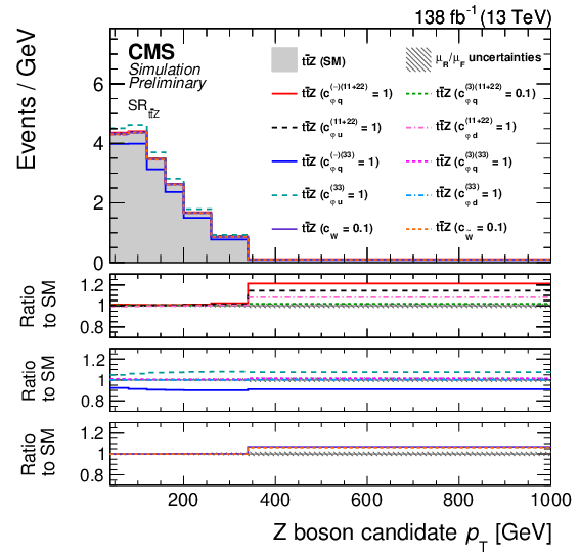
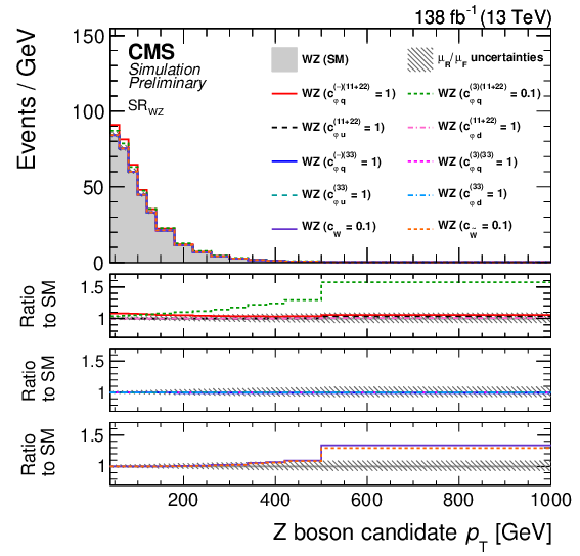
png pdf	Figure 2: Distributions in Z boson $p_{\mathrm{T}}$ in the three signal regions of this analysis. Shown are $\text{SR}_{\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}}$ (upper left), $\text{SR}_{\mathrm{W}\mathrm{Z}}$ (upper right), and $\text{SR}_{\mathrm{Z}\mathrm{Z}}$ (lower). In each region, the target process ( $\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}$ , WZ, or ZZ) is shown at the SM point (coloured areas) and various EFT hypotheses (lines). The hashed band includes only uncertainties in the renormalisation and factorisation scales. The upper, centre, and lower ratio panels show the ratio of EFT hypotheses for light quark couplings, heavy quark couplings, and electroweak boson couplings, respectively.
png pdf	Figure 2-a: Distributions in Z boson $p_{\mathrm{T}}$ in the three signal regions of this analysis. Shown are $\text{SR}_{\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}}$ (upper left), $\text{SR}_{\mathrm{W}\mathrm{Z}}$ (upper right), and $\text{SR}_{\mathrm{Z}\mathrm{Z}}$ (lower). In each region, the target process ( $\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}$ , WZ, or ZZ) is shown at the SM point (coloured areas) and various EFT hypotheses (lines). The hashed band includes only uncertainties in the renormalisation and factorisation scales. The upper, centre, and lower ratio panels show the ratio of EFT hypotheses for light quark couplings, heavy quark couplings, and electroweak boson couplings, respectively.
png pdf	Figure 2-b: Distributions in Z boson $p_{\mathrm{T}}$ in the three signal regions of this analysis. Shown are $\text{SR}_{\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}}$ (upper left), $\text{SR}_{\mathrm{W}\mathrm{Z}}$ (upper right), and $\text{SR}_{\mathrm{Z}\mathrm{Z}}$ (lower). In each region, the target process ( $\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}$ , WZ, or ZZ) is shown at the SM point (coloured areas) and various EFT hypotheses (lines). The hashed band includes only uncertainties in the renormalisation and factorisation scales. The upper, centre, and lower ratio panels show the ratio of EFT hypotheses for light quark couplings, heavy quark couplings, and electroweak boson couplings, respectively.
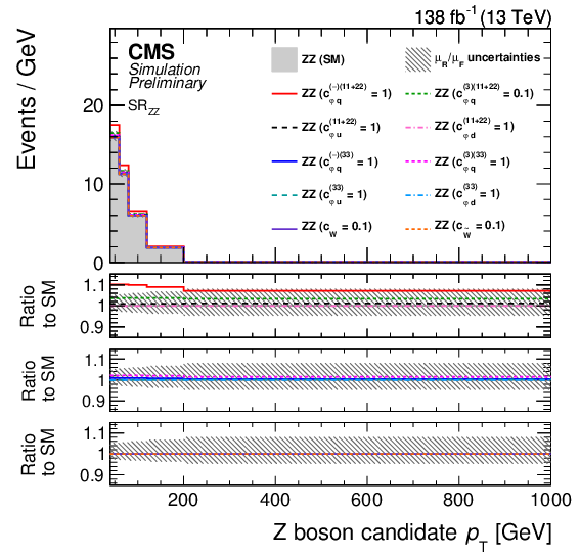
png pdf	Figure 2-c: Distributions in Z boson $p_{\mathrm{T}}$ in the three signal regions of this analysis. Shown are $\text{SR}_{\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}}$ (upper left), $\text{SR}_{\mathrm{W}\mathrm{Z}}$ (upper right), and $\text{SR}_{\mathrm{Z}\mathrm{Z}}$ (lower). In each region, the target process ( $\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}$ , WZ, or ZZ) is shown at the SM point (coloured areas) and various EFT hypotheses (lines). The hashed band includes only uncertainties in the renormalisation and factorisation scales. The upper, centre, and lower ratio panels show the ratio of EFT hypotheses for light quark couplings, heavy quark couplings, and electroweak boson couplings, respectively.
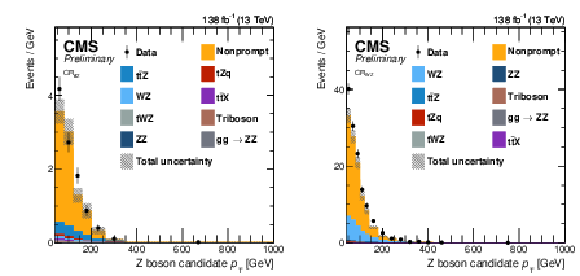
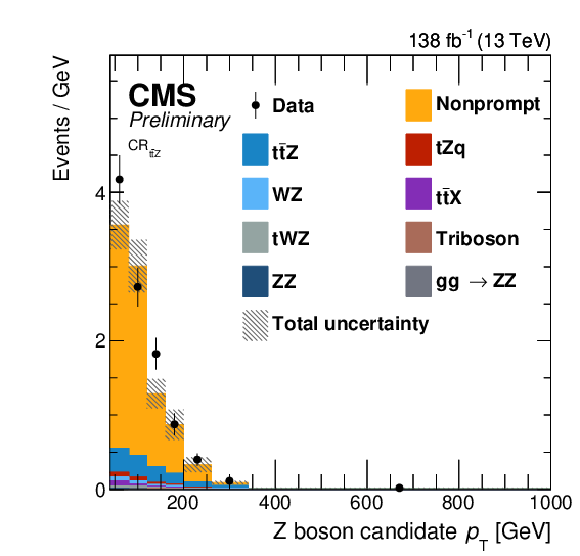
png pdf	Figure 3: Distributions in Z boson $p_{\mathrm{T}}$ in the control regions of this analysis. Shown are $\text{CR}_{\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}}$ (left) and $\text{CR}_{\mathrm{W}\mathrm{Z}}$ (right). Predictions are all obtained from simulation and are displayed as coloured areas. The hashed area shows the statistical uncertainty in the prediction. Data are displayed as markers, where the vertical bars represent the statistical uncertainty.
png pdf	Figure 3-a: Distributions in Z boson $p_{\mathrm{T}}$ in the control regions of this analysis. Shown are $\text{CR}_{\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}}$ (left) and $\text{CR}_{\mathrm{W}\mathrm{Z}}$ (right). Predictions are all obtained from simulation and are displayed as coloured areas. The hashed area shows the statistical uncertainty in the prediction. Data are displayed as markers, where the vertical bars represent the statistical uncertainty.
png pdf	Figure 3-b: Distributions in Z boson $p_{\mathrm{T}}$ in the control regions of this analysis. Shown are $\text{CR}_{\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}}$ (left) and $\text{CR}_{\mathrm{W}\mathrm{Z}}$ (right). Predictions are all obtained from simulation and are displayed as coloured areas. The hashed area shows the statistical uncertainty in the prediction. Data are displayed as markers, where the vertical bars represent the statistical uncertainty.
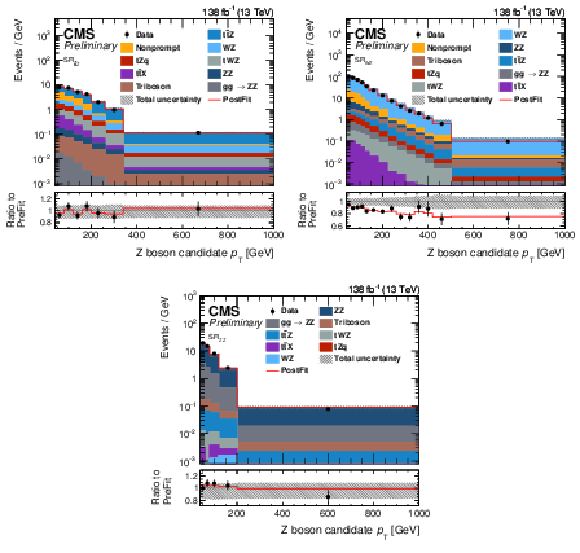
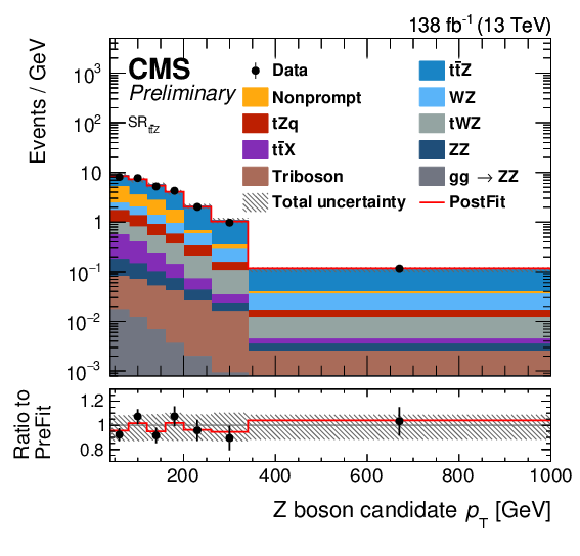
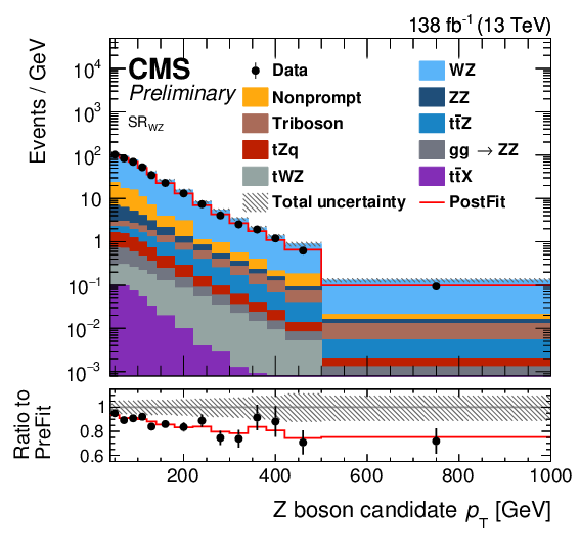
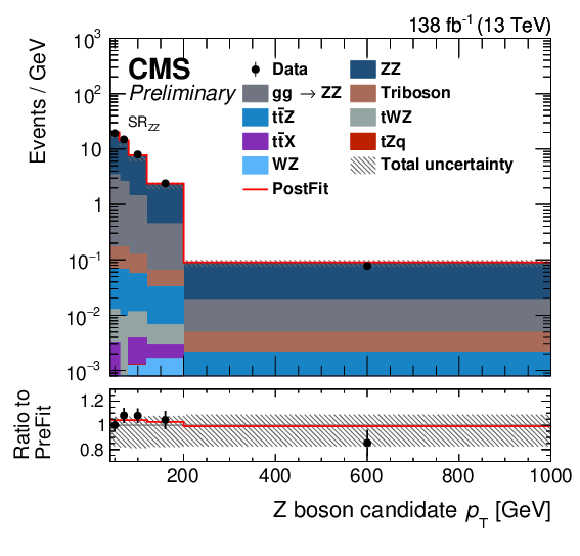
png pdf	Figure 4: Distributions in Z boson $p_{\mathrm{T}}$ in the three signal regions of this analysis. Shown are the $\text{SR}_{\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}}$ (upper left), $\text{SR}_{\mathrm{W}\mathrm{Z}}$ (upper right), and $\text{SR}_{\mathrm{Z}\mathrm{Z}}$ (lower) regions. The data (markers) are compared to the prediction from simulation and the data-driven estimate of nonprompt leptons (coloured areas). The lower panel displays the ratio of data to the prediction. The hashed area displays the total uncertainties and the red line displays the best fit result in a setup with all EFT parameters.
png pdf	Figure 4-a: Distributions in Z boson $p_{\mathrm{T}}$ in the three signal regions of this analysis. Shown are the $\text{SR}_{\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}}$ (upper left), $\text{SR}_{\mathrm{W}\mathrm{Z}}$ (upper right), and $\text{SR}_{\mathrm{Z}\mathrm{Z}}$ (lower) regions. The data (markers) are compared to the prediction from simulation and the data-driven estimate of nonprompt leptons (coloured areas). The lower panel displays the ratio of data to the prediction. The hashed area displays the total uncertainties and the red line displays the best fit result in a setup with all EFT parameters.
png pdf	Figure 4-b: Distributions in Z boson $p_{\mathrm{T}}$ in the three signal regions of this analysis. Shown are the $\text{SR}_{\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}}$ (upper left), $\text{SR}_{\mathrm{W}\mathrm{Z}}$ (upper right), and $\text{SR}_{\mathrm{Z}\mathrm{Z}}$ (lower) regions. The data (markers) are compared to the prediction from simulation and the data-driven estimate of nonprompt leptons (coloured areas). The lower panel displays the ratio of data to the prediction. The hashed area displays the total uncertainties and the red line displays the best fit result in a setup with all EFT parameters.
png pdf	Figure 4-c: Distributions in Z boson $p_{\mathrm{T}}$ in the three signal regions of this analysis. Shown are the $\text{SR}_{\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}}$ (upper left), $\text{SR}_{\mathrm{W}\mathrm{Z}}$ (upper right), and $\text{SR}_{\mathrm{Z}\mathrm{Z}}$ (lower) regions. The data (markers) are compared to the prediction from simulation and the data-driven estimate of nonprompt leptons (coloured areas). The lower panel displays the ratio of data to the prediction. The hashed area displays the total uncertainties and the red line displays the best fit result in a setup with all EFT parameters.
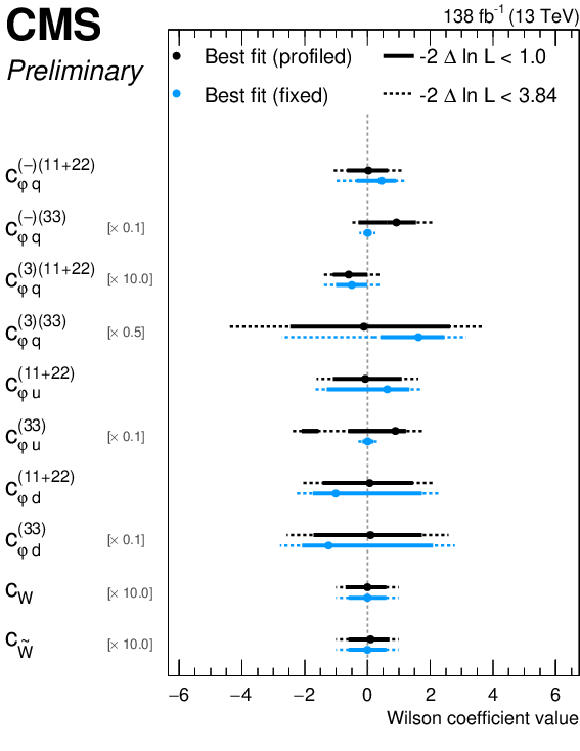
png pdf	Figure 5: Summary of the limits obtained for the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ , $c_{\varphi q}^{(-)(33)}$ , $c_{\varphi q}^{(3)(11+22)}$ , $c_{\varphi q}^{(3)(33)}$ , $c_{\varphi u}^{(11+22)}$ , $c_{\varphi u}^{(33)}$ , $c_{\varphi d}^{(11+22)}$ , $c_{\varphi d}^{(33)}$ , $c_{W}$ , and $c_{\widetilde{W}}$ . Shown are the best fit points and limits for scans where other Wilson coefficients are fixed to zero (`fixed') or are allowed to float (`profiled'). The points where the difference $-2\Delta\ln L$ with respect to the best fit increases by 1 and 3.84 are shown as horizontal error bars. For the Wilson coefficient value the EFT energy scale is assumed to be $\Lambda=$ 1 TeV.
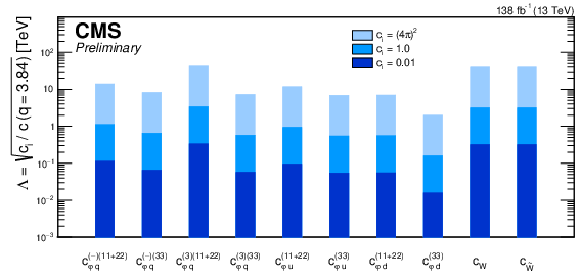
png pdf	Figure 6: Summary of the limits in the energy scale $\Lambda$ obtained from the limits on the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ , $c_{\varphi q}^{(-)(33)}$ , $c_{\varphi q}^{(3)(11+22)}$ , $c_{\varphi q}^{(3)(33)}$ , $c_{\varphi u}^{(11+22)}$ , $c_{\varphi u}^{(33)}$ , $c_{\varphi d}^{(11+22)}$ , $c_{\varphi d}^{(33)}$ , $c_{W}$ , and $c_{\widetilde{W}}$ . Shown are limits for scans where other Wilson coefficients are fixed to zero. The limit on lambda is calculated from the Wilson coefficient value at $q = -2\Delta\ln L =$ 3.84. The least stringent limit is chosen for each Wilson coefficient and limits are shown for three scenarios of $c_{i}$ .
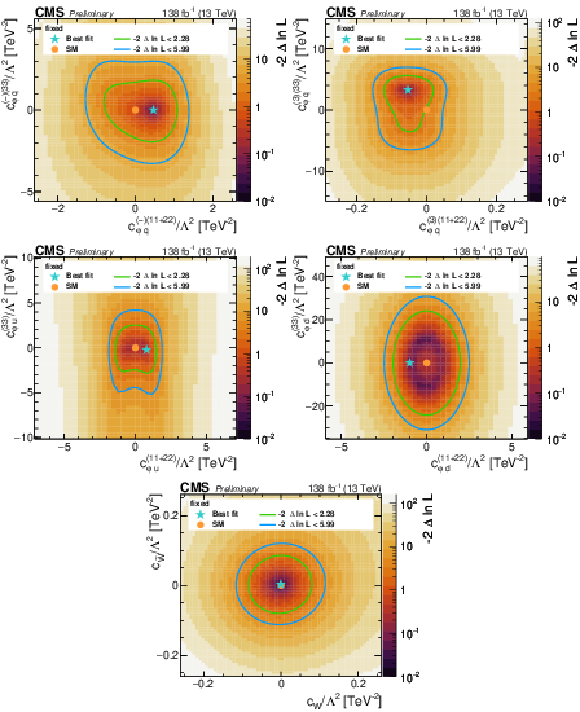
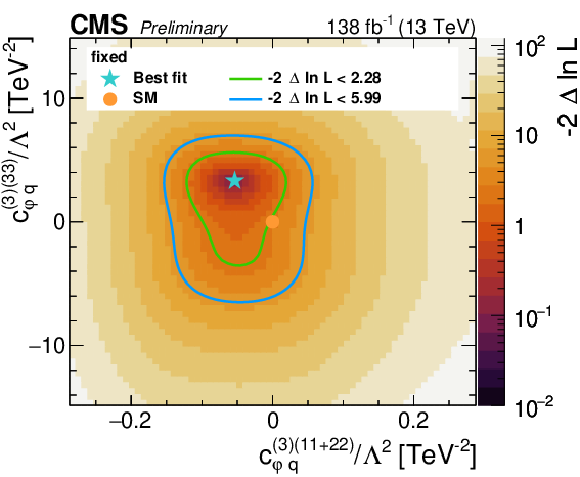
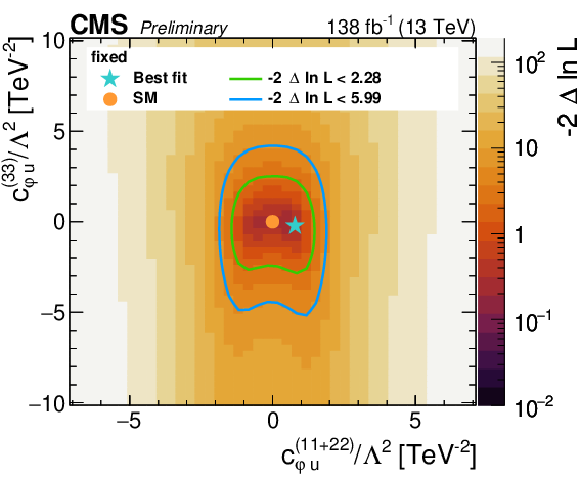
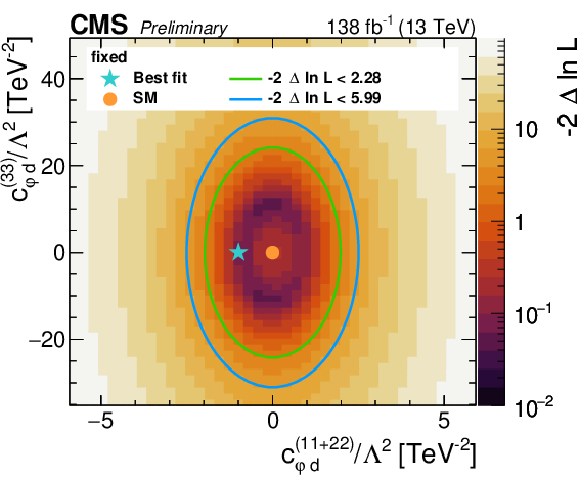
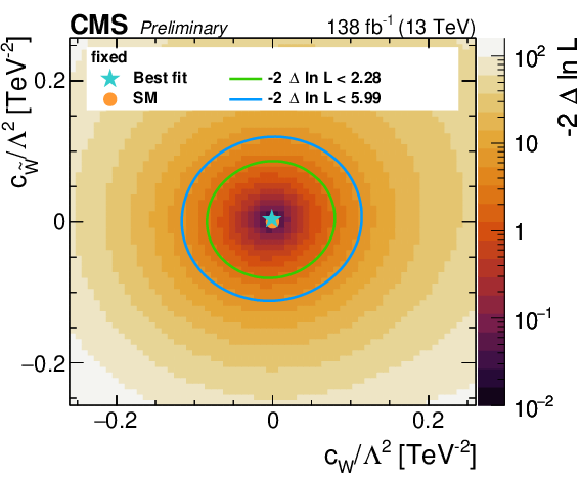
png pdf	Figure 7: Likelihood as a function of the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ and $c_{\varphi q}^{(-)(33)}$ (upper left), $c_{\varphi q}^{(3)(11+22)}$ and $c_{\varphi q}^{(3)(33)}$ (upper right), $c_{\varphi u}^{(11+22)}$ and $c_{\varphi u}^{(33)}$ (centre left), $c_{\varphi d}^{(11+22)}$ and $c_{\varphi d}^{(33)}$ (centre right), as well as $c_{W}$ and $c_{\widetilde{W}}$ (lower). All Wilson coefficients that are not scanned are fixed to zero. The best fit value is shown with a marker and the coloured lines correspond to the 68% and 95% confidence intervals.
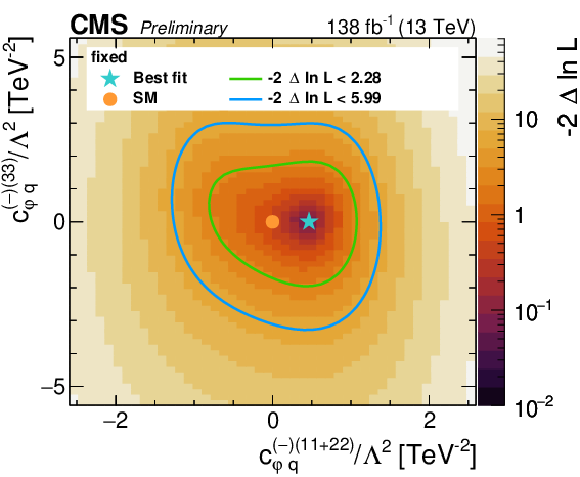
png pdf	Figure 7-a: Likelihood as a function of the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ and $c_{\varphi q}^{(-)(33)}$ (upper left), $c_{\varphi q}^{(3)(11+22)}$ and $c_{\varphi q}^{(3)(33)}$ (upper right), $c_{\varphi u}^{(11+22)}$ and $c_{\varphi u}^{(33)}$ (centre left), $c_{\varphi d}^{(11+22)}$ and $c_{\varphi d}^{(33)}$ (centre right), as well as $c_{W}$ and $c_{\widetilde{W}}$ (lower). All Wilson coefficients that are not scanned are fixed to zero. The best fit value is shown with a marker and the coloured lines correspond to the 68% and 95% confidence intervals.
png pdf	Figure 7-b: Likelihood as a function of the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ and $c_{\varphi q}^{(-)(33)}$ (upper left), $c_{\varphi q}^{(3)(11+22)}$ and $c_{\varphi q}^{(3)(33)}$ (upper right), $c_{\varphi u}^{(11+22)}$ and $c_{\varphi u}^{(33)}$ (centre left), $c_{\varphi d}^{(11+22)}$ and $c_{\varphi d}^{(33)}$ (centre right), as well as $c_{W}$ and $c_{\widetilde{W}}$ (lower). All Wilson coefficients that are not scanned are fixed to zero. The best fit value is shown with a marker and the coloured lines correspond to the 68% and 95% confidence intervals.
png pdf	Figure 7-c: Likelihood as a function of the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ and $c_{\varphi q}^{(-)(33)}$ (upper left), $c_{\varphi q}^{(3)(11+22)}$ and $c_{\varphi q}^{(3)(33)}$ (upper right), $c_{\varphi u}^{(11+22)}$ and $c_{\varphi u}^{(33)}$ (centre left), $c_{\varphi d}^{(11+22)}$ and $c_{\varphi d}^{(33)}$ (centre right), as well as $c_{W}$ and $c_{\widetilde{W}}$ (lower). All Wilson coefficients that are not scanned are fixed to zero. The best fit value is shown with a marker and the coloured lines correspond to the 68% and 95% confidence intervals.
png pdf	Figure 7-d: Likelihood as a function of the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ and $c_{\varphi q}^{(-)(33)}$ (upper left), $c_{\varphi q}^{(3)(11+22)}$ and $c_{\varphi q}^{(3)(33)}$ (upper right), $c_{\varphi u}^{(11+22)}$ and $c_{\varphi u}^{(33)}$ (centre left), $c_{\varphi d}^{(11+22)}$ and $c_{\varphi d}^{(33)}$ (centre right), as well as $c_{W}$ and $c_{\widetilde{W}}$ (lower). All Wilson coefficients that are not scanned are fixed to zero. The best fit value is shown with a marker and the coloured lines correspond to the 68% and 95% confidence intervals.
png pdf	Figure 7-e: Likelihood as a function of the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ and $c_{\varphi q}^{(-)(33)}$ (upper left), $c_{\varphi q}^{(3)(11+22)}$ and $c_{\varphi q}^{(3)(33)}$ (upper right), $c_{\varphi u}^{(11+22)}$ and $c_{\varphi u}^{(33)}$ (centre left), $c_{\varphi d}^{(11+22)}$ and $c_{\varphi d}^{(33)}$ (centre right), as well as $c_{W}$ and $c_{\widetilde{W}}$ (lower). All Wilson coefficients that are not scanned are fixed to zero. The best fit value is shown with a marker and the coloured lines correspond to the 68% and 95% confidence intervals.
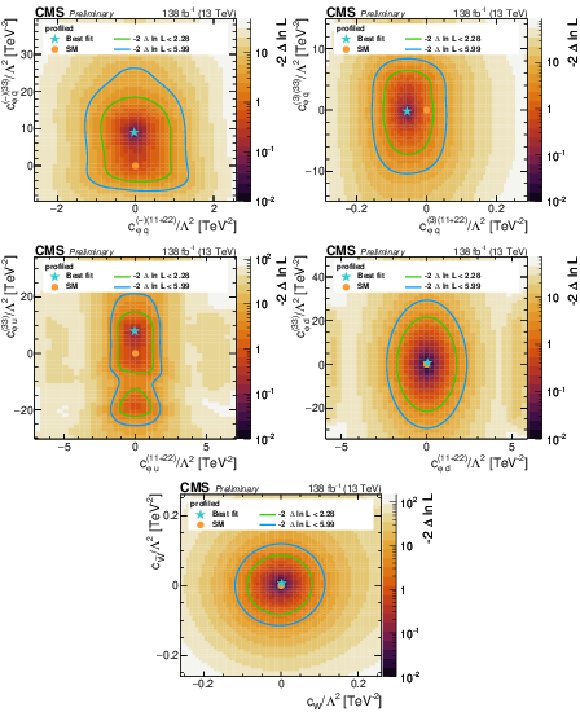
png pdf	Figure 8: Likelihood as a function of the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ and $c_{\varphi q}^{(-)(33)}$ (upper left), $c_{\varphi q}^{(3)(11+22)}$ and $c_{\varphi q}^{(3)(33)}$ (upper right), $c_{\varphi u}^{(11+22)}$ and $c_{\varphi u}^{(33)}$ (centre left), $c_{\varphi d}^{(11+22)}$ and $c_{\varphi d}^{(33)}$ (centre right), as well as $c_{W}$ and $c_{\widetilde{W}}$ (lower). Other Wilson coefficients are allowed to float in the fit. The best fit value is shown with a marker and the coloured lines correspond to the 68% and 95% confidence intervals.
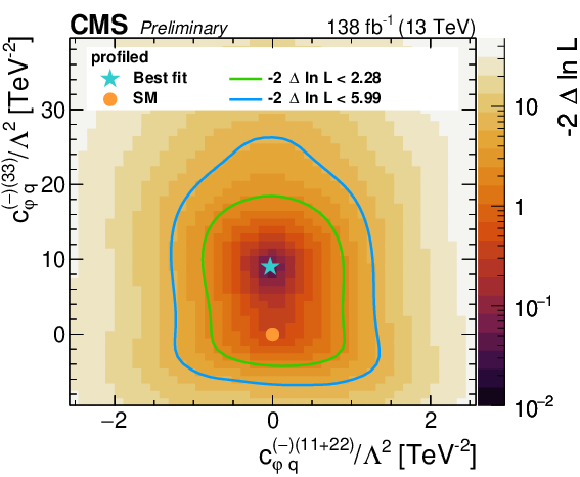
png pdf	Figure 8-a: Likelihood as a function of the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ and $c_{\varphi q}^{(-)(33)}$ (upper left), $c_{\varphi q}^{(3)(11+22)}$ and $c_{\varphi q}^{(3)(33)}$ (upper right), $c_{\varphi u}^{(11+22)}$ and $c_{\varphi u}^{(33)}$ (centre left), $c_{\varphi d}^{(11+22)}$ and $c_{\varphi d}^{(33)}$ (centre right), as well as $c_{W}$ and $c_{\widetilde{W}}$ (lower). Other Wilson coefficients are allowed to float in the fit. The best fit value is shown with a marker and the coloured lines correspond to the 68% and 95% confidence intervals.
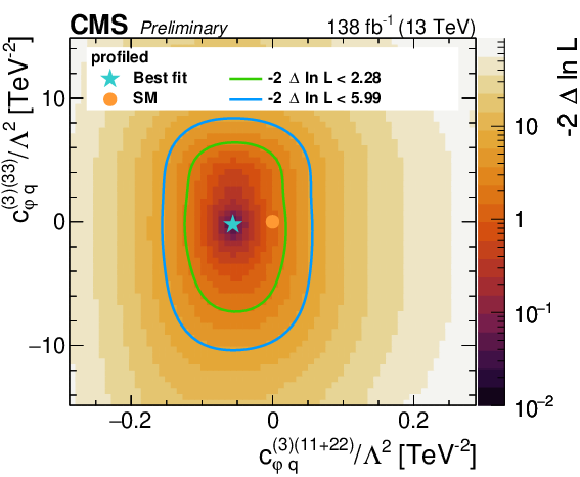
png pdf	Figure 8-b: Likelihood as a function of the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ and $c_{\varphi q}^{(-)(33)}$ (upper left), $c_{\varphi q}^{(3)(11+22)}$ and $c_{\varphi q}^{(3)(33)}$ (upper right), $c_{\varphi u}^{(11+22)}$ and $c_{\varphi u}^{(33)}$ (centre left), $c_{\varphi d}^{(11+22)}$ and $c_{\varphi d}^{(33)}$ (centre right), as well as $c_{W}$ and $c_{\widetilde{W}}$ (lower). Other Wilson coefficients are allowed to float in the fit. The best fit value is shown with a marker and the coloured lines correspond to the 68% and 95% confidence intervals.
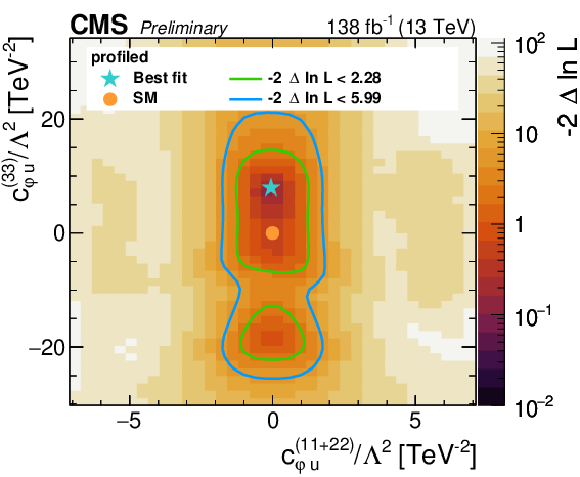
png pdf	Figure 8-c: Likelihood as a function of the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ and $c_{\varphi q}^{(-)(33)}$ (upper left), $c_{\varphi q}^{(3)(11+22)}$ and $c_{\varphi q}^{(3)(33)}$ (upper right), $c_{\varphi u}^{(11+22)}$ and $c_{\varphi u}^{(33)}$ (centre left), $c_{\varphi d}^{(11+22)}$ and $c_{\varphi d}^{(33)}$ (centre right), as well as $c_{W}$ and $c_{\widetilde{W}}$ (lower). Other Wilson coefficients are allowed to float in the fit. The best fit value is shown with a marker and the coloured lines correspond to the 68% and 95% confidence intervals.
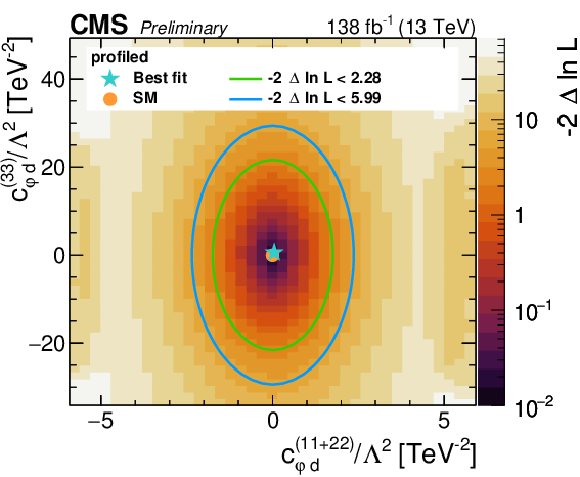
png pdf	Figure 8-d: Likelihood as a function of the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ and $c_{\varphi q}^{(-)(33)}$ (upper left), $c_{\varphi q}^{(3)(11+22)}$ and $c_{\varphi q}^{(3)(33)}$ (upper right), $c_{\varphi u}^{(11+22)}$ and $c_{\varphi u}^{(33)}$ (centre left), $c_{\varphi d}^{(11+22)}$ and $c_{\varphi d}^{(33)}$ (centre right), as well as $c_{W}$ and $c_{\widetilde{W}}$ (lower). Other Wilson coefficients are allowed to float in the fit. The best fit value is shown with a marker and the coloured lines correspond to the 68% and 95% confidence intervals.
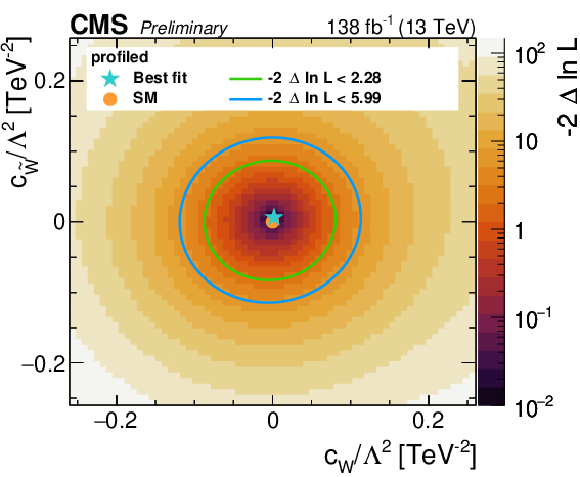
png pdf	Figure 8-e: Likelihood as a function of the Wilson coefficients $c_{\varphi q}^{(-)(11+22)}$ and $c_{\varphi q}^{(-)(33)}$ (upper left), $c_{\varphi q}^{(3)(11+22)}$ and $c_{\varphi q}^{(3)(33)}$ (upper right), $c_{\varphi u}^{(11+22)}$ and $c_{\varphi u}^{(33)}$ (centre left), $c_{\varphi d}^{(11+22)}$ and $c_{\varphi d}^{(33)}$ (centre right), as well as $c_{W}$ and $c_{\widetilde{W}}$ (lower). Other Wilson coefficients are allowed to float in the fit. The best fit value is shown with a marker and the coloured lines correspond to the 68% and 95% confidence intervals.

Summary

A measurement of the flavour structures in effective field theory (EFT) couplings has been presented, considering the electroweak coupling to quarks of different generations in the processes

$\mathrm{t}\overline{\mathrm{t}}\mathrm{Z}$ , WZ, and ZZ. By treating the couplings in a flavour aware way and simultaneously measuring the Z-quark couplings in different processes, the flavour structures of these couplings are probed. The measured Wilson coefficients are compatible with the SM hypothesis within their uncertainties and corresponding limits are placed. Extracting the EFT parameters from multiple processes simultaneously makes it possible to correctly correlate EFT effects of the three processes that are often important backgrounds of each other. Thus, these results contribute to a more comprehensive EFT interpretation that preserves correlations in combinations or global fits, rather than focusing solely on individual processes.

References
1	C. P. Burgess	Introduction to Effective Field Theory	Cambridge University Press, 12, ISBN~978-1-139-04804-0, 978-0-521-19547-8, 2020 link
2	W. Buchmuller and D. Wyler	Effective lagrangian analysis of new interactions and flavor conservation	NPB 268 (1986) 621
3	I. Brivio and M. Trott	The standard model as an effective field theory	Phys. Rept. 793 (2019) 1	1706.08945
4	G. F. Giudice, C. Grojean, A. Pomarol, and R. Rattazzi	The strongly-interacting light Higgs	JHEP 06 (2007) 045	hep-ph/0703164
5	B. Grzadkowski, M. Iskrzynski, M. Misiak, and J. Rosiek	Dimension-six terms in the standard model lagrangian	JHEP 10 (2010) 085	1008.4884
6	A. Helset and A. Kobach	Baryon number, lepton number, and operator dimension in the SMEFT with flavor symmetries	PLB 800 (2020) 135132	1909.05853
7	ATLAS Collaboration	Inclusive and differential cross-section measurements of $t\overline{t}z$ production in pp collisions at $\sqrt{s}$ = 13 TeV with the ATLAS detector, including EFT and spin-correlation interpretations	JHEP 07 (2024) 163	2312.04450
8	ATLAS Collaboration	Measurements of inclusive and differential cross-sections of $t\overline{t}\gamma$ production in pp collisions at $\sqrt{s}$ = 13 TeV with the ATLAS detector	JHEP 10 (2024) 191	2403.09452
9	CMS Collaboration	Probing effective field theory operators in the associated production of top quarks with a Z boson in multilepton final states at $\sqrt{s}$ = 13 TeV	JHEP 12 (2021) 083	CMS-TOP-21-001 2107.13896
10	CMS Collaboration	Search for new physics using effective field theory in 13 TeV pp collision events that contain a top quark pair and a boosted Z or Higgs boson	PRD 108 (2023) 032008	CMS-TOP-21-003 2208.12837
11	CMS Collaboration	Search for new physics in top quark production with additional leptons in proton-proton collisions at $\sqrt{s} =$ 13 TeV using effective field theory	JHEP 03 (2021) 095	CMS-TOP-19-001 2012.04120
12	CMS Collaboration	Search for physics beyond the standard model in top quark production with additional leptons in the context of effective field theory	JHEP 12 (2023) 068	CMS-TOP-22-006 2307.15761
13	S. Bi\ss mann, C. Grunwald, G. Hiller, and K. Kröninger	Top and beauty synergies in SMEFT-fits at present and future colliders	JHEP 06 (2021) 010	2012.10456
14	S. Bruggisser, R. Sch ä fer, D. van Dyk, and S. Westhoff	The flavor of UV physics	JHEP 05 (2021) 257	2101.07273
15	LHCb Collaboration	Differential branching fractions and isospin asymmetries of $b \to k^{(*)} \mu^+ \mu^-$ decays	JHEP 06 (2014) 133	1403.8044
16	LHCb Collaboration	Measurements of the S-wave fraction in $b^{0}\rightarrow k^{+}\pi^{-}\mu^{+}\mu^{-}$ decays and the $b^{0}\rightarrow k^{\ast}(892)^{0}\mu^{+}\mu^{-}$ differential branching fraction	JHEP 11 (2016) 047	1606.04731
17	LHCb Collaboration	Test of lepton universality with $b^{0} \rightarrow k^{*0}\ell^{+}\ell^{-}$ decays	JHEP 08 (2017) 055	1705.05802
18	LHCb Collaboration	Test of lepton universality in beauty-quark decays	Nature Phys. 18 (2022) 277	2103.11769
19	LHCb Collaboration	Test of lepton universality with ${\Lambda}_b^0\to {pK}^{-}{\mathrm{\ell}}^{+}{\mathrm{\ell}}^{-}$ decays	JHEP 05 (2020) 040	1912.08139
20	LHCb Collaboration	Tests of lepton universality using $b^0\to k^0_s \ell^+ \ell^-$ and $b^+\to k^{*+} \ell^+ \ell^-$ decays	PRL 128 (2022) 191802	2110.09501
21	LHCb Collaboration	Measurement of lepton universality parameters in $b^+\to k^+\ell^+\ell^-$ and $b^0\to k^{*0}\ell^+\ell^-$ decays	PRD 108 (2023) 032002	2212.09153
22	LHCb Collaboration	Test of lepton universality in $b \rightarrow s \ell^+ \ell^-$ decays	PRL 131 (2023) 051803	2212.09152
23	ATLAS Collaboration	Search for flavour-changing neutral current top-quark decays $t\to qz$ in proton-proton collisions at $\sqrt{s}=$ 13 TeV with the ATLAS detector	JHEP 07 (2018) 176	1803.09923
24	ATLAS Collaboration	Search for flavour-changing neutral-current interactions of a top quark and a gluon in pp collisions at $\sqrt{s}=$ 13 TeV with the ATLAS detector	EPJC 82 (2022) 334	2112.01302
25	ATLAS Collaboration	Search for flavour-changing neutral-current couplings between the top quark and the photon with the ATLAS detector at $\sqrt{s}=$ 13 TeV	[Erratum: Phys.Lett.B 847, 138286 ()], 2023 PLB 842 (2023) 137379	2205.02537
26	CMS Collaboration	Search for new physics in top quark production in dilepton final states in proton-proton collisions at $\sqrt{s}$ = 13 TeV	no.~11, 886, 2019 EPJC 79 (2019)	CMS-TOP-17-020 1903.11144
27	CMS Collaboration	Measurement of the inclusive and differential $\mathrm{t\bar{t}}\gamma$ cross sections in the dilepton channel and effective field theory interpretation in proton-proton collisions at $\sqrt{s}$ =13 TeV	JHEP 05 (2022) 091	CMS-TOP-21-004 2201.07301
28	CMS Collaboration	The CMS experiment at the CERN LHC	JINST 3 (2008) S08004
29	Tracker Group of the CMS Collaboration	The CMS Phase-1 pixel detector upgrade	JINST 16 (2021) P02027	2012.14304
30	CMS Collaboration	Performance of the CMS Level-1 trigger in proton-proton collisions at $\sqrt{s} =$ 13 TeV	JINST 15 (2020) P10017	CMS-TRG-17-001 2006.10165
31	CMS Collaboration	The CMS trigger system	JINST 12 (2017) P01020	CMS-TRG-12-001 1609.02366
32	CMS Collaboration	Performance of the CMS high-level trigger during LHC run 2	JINST 19 (2024) P11021	CMS-TRG-19-001 2410.17038
33	J. Alwall et al.	The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations	JHEP 07 (2014) 079	1405.0301
34	S. Frixione and B. R. Webber	Matching NLO QCD computations and parton shower simulations	JHEP 06 (2002) 029	hep-ph/0204244
35	O. Mattelaer	On the maximal use of Monte Carlo samples: re-weighting events at NLO accuracy	no.~12, 674, 2016 EPJC 76 (2016)	1607.00763
36	I. Brivio, Y. Jiang, and M. Trott	The SMEFTsim package, theory and tools	JHEP 12 (2017) 070	1709.06492
37	I. Brivio	SMEFTsim 3.0 \textemdash a practical guide	JHEP 04 (2021) 073	2012.11343
38	T. Melia, P. Nason, R. Rontsch, and G. Zanderighi	W $^+$ W $^-$ , WZ and ZZ production in the POWHEG BOX	JHEP 11 (2011) 078	1107.5051
39	P. Nason and G. Zanderighi	$\mathrm{W}^+ \mathrm{W}^-$ , WZ and ZZ production in the POWHEG-BOX-V2	EPJC 74 (2014) 2702	1311.1365
40	P. Nason	A new method for combining NLO QCD with shower Monte Carlo algorithms	JHEP 11 (2004) 040	hep-ph/0409146
41	S. Frixione, P. Nason, and C. Oleari	Matching NLO QCD computations with parton shower simulations: the POWHEG method	JHEP 11 (2007) 070	0709.2092
42	S. Alioli, P. Nason, C. Oleari, and E. Re	A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX	JHEP 06 (2010) 043	1002.2581
43	S. Frixione, P. Nason, and G. Ridolfi	A positive-weight next-to-leading-order Monte Carlo for heavy flavour hadroproduction	JHEP 09 (2007) 126	0707.3088
44	H. B. Hartanto, B. Jager, L. Reina, and D. Wackeroth	Higgs boson production in association with top quarks in the POWHEG BOX	PRD 91 (2015) 094003	1501.04498
45	S. Alioli, P. Nason, C. Oleari, and E. Re	NLO single-top production matched with shower in POWHEG: $s$ - and $t$ -channel contributions	JHEP 09 (2009) 111	0907.4076
46	E. Re	Single-top Wt-channel production matched with parton showers using the POWHEG method	EPJC 71 (2011) 1547	1009.2450
47	T. Sjöstrand et al.	An introduction to PYTHIA 8.2	Comput. Phys. Commun. 191 (2015) 159	1410.3012
48	CMS Collaboration	Extraction and validation of a new set of CMS PYTHIA8 tunes from underlying-event measurements	EPJC 80 (2020) 4	CMS-GEN-17-001 1903.12179
49	R. Frederix and S. Frixione	Merging meets matching in MC@NLO	JHEP 12 (2012) 061	1209.6215
50	J. Alwall et al.	Comparative study of various algorithms for the merging of parton showers and matrix elements in hadronic collisions	EPJC 53 (2008) 473	0706.2569
51	NNPDF Collaboration	Parton distributions from high-precision collider data	EPJC 77 (2017) 663	1706.00428
52	GEANT4 Collaboration	GEANT 4---A simulation toolkit	NIM A 506 (2003) 250
53	J. Allison et al.	GEANT 4 developments and applications	IEEE Trans. Nucl. Sci. 53 (2006) 270
54	CMS Collaboration	Particle-flow reconstruction and global event description with the CMS detector	JINST 12 (2017) P10003	CMS-PRF-14-001 1706.04965
55	M. Cacciari, G. P. Salam, and G. Soyez	The anti- $k_{\mathrm{T}}$ jet clustering algorithm	JHEP 04 (2008) 063	0802.1189
56	M. Cacciari, G. P. Salam, and G. Soyez	Fastjet user manual	EPJC 72 (2012) 1896	1111.6097
57	CMS Collaboration	Technical proposal for the Phase-II upgrade of the Compact Muon Solenoid	CMS Technical Proposal CERN-LHCC-2015-010, CMS-TDR-15-02, 2015 CDS
58	CMS Collaboration	Electron and photon reconstruction and identification with the CMS experiment at the CERN LHC	JINST 16 (2021) P05014	CMS-EGM-17-001 2012.06888
59	CMS Collaboration	Performance of the CMS muon detector and muon reconstruction with proton-proton collisions at $\sqrt{s}=$ 13 TeV	JINST 13 (2018) P06015	CMS-MUO-16-001 1804.04528
60	CMS Collaboration	Observation of four top quark production in proton-proton collisions at $\sqrt{s}=$ 13TeV	PLB 847 (2023) 138290	CMS-TOP-22-013 2305.13439
61	CMS Collaboration	Muon identification using multivariate techniques in the CMS experiment in proton-proton collisions at sqrt(s) = 13 TeV	JINST 19 (2024) P02031	CMS-MUO-22-001 2310.03844
62	CMS Collaboration	Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV	JINST 12 (2017) P02014	CMS-JME-13-004 1607.03663
63	CMS Collaboration	Performance of missing transverse momentum reconstruction in proton-proton collisions at $\sqrt{s} =$ 13 TeV using the CMS detector	JINST 14 (2019) P07004	CMS-JME-17-001 1903.06078
64	CMS Collaboration	Performance of b tagging algorithms in proton-proton collisions at 13 TeV with Phase 1 CMS detector	CMS Detector Performance Note CMS-DP-2018-033, 2018 CDS
65	E. Bols et al.	Jet flavour classification using DeepJet	JINST 15 (2020) P12012	2008.10519
66	Particle Data Group , S. Navas et al.	Review of particle physics	PRD 110 (2024) 030001
67	CMS Collaboration	Measurement of the higgs boson production rate in association with top quarks in final states with electrons, muons, and hadronically decaying tau leptons at $\sqrt{s} =$ 13 TeV	EPJC 81 (2021) 378	CMS-HIG-19-008 2011.03652
68	CMS Collaboration	Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV	JINST 13 (2018) P05011	CMS-BTV-16-002 1712.07158
69	CMS Collaboration	Pileup mitigation at CMS in 13 TeV data	JINST 15 (2020) P09018	CMS-JME-18-001 2003.00503
70	CMS Collaboration	Precision luminosity measurement in proton-proton collisions at $\sqrt{s} =$ 13 TeV in 2015 and 2016 at CMS	EPJC 81 (2021) 800	CMS-LUM-17-003 2104.01927
71	CMS Collaboration	CMS luminosity measurement for the 2017 data-taking period at $\sqrt{s} =$ 13 TeV	CMS Physics Analysis Summary, 2018 CMS-PAS-LUM-17-004	CMS-PAS-LUM-17-004
72	CMS Collaboration	CMS luminosity measurement for the 2018 data-taking period at $\sqrt{s} =$ 13 TeV	CMS Physics Analysis Summary, 2019 CMS-PAS-LUM-18-002	CMS-PAS-LUM-18-002
73	NNPDF Collaboration	Parton distributions for the LHC Run II	JHEP 04 (2015) 040	1410.8849
74	CMS Collaboration	Evidence for tWZ production in proton-proton collisions at $\sqrt{s}$ = 13 TeV in multilepton final states	PLB 855 (2024) 138815	CMS-TOP-22-008 2312.11668
75	CMS Collaboration	Measurement of the cross section of top quark-antiquark pair production in association with a W boson in proton-proton collisions at $\sqrt{s}$ = 13 TeV	JHEP 07 (2023) 219	CMS-TOP-21-011 2208.06485
76	A. Kulesza et al.	Associated top quark pair production with a heavy boson: differential cross sections at NLO+NNLL accuracy	EPJC 80 (2020) 428	2001.03031
77	CMS Collaboration	Inclusive and differential cross section measurements of single top quark production in association with a Z boson in proton-proton collisions at $\sqrt{s}$ = 13 TeV	JHEP 02 (2022) 107	CMS-TOP-20-010 2111.02860
78	CMS Collaboration	Measurements of production cross sections of WZ and same-sign WW boson pairs in association with two jets in proton-proton collisions at $\sqrt{s} =$ 13 TeV	PLB 809 (2020) 135710	CMS-SMP-19-012 2005.01173
79	F. Cascioli et al.	ZZ production at hadron colliders in NNLO QCD	PLB 735 (2014) 311	1405.2219
80	M. Grazzini et al.	NNLO QCD + NLO EW with Matrix+OpenLoops: precise predictions for vector-boson pair production	JHEP 02 (2020) 087	1912.00068
81	CMS Collaboration	The CMS statistical analysis and combination tool: Combine	Comput. Softw. Big Sci. 8 (2024) 19	CMS-CAT-23-001 2404.06614
82	W. Verkerke and D. Kirkby	The \textscRooFit toolkit for data modeling	in Proc. 13th International Conference on Computing in High Energy and Nuclear Physics (CHEP ): La Jolla CA, United States, 2003 [eConf C0303241 MOLT007]	physics/0306116
83	L. Moneta et al.	The \textscRooStats project	in Proc. 13th International Workshop on Advanced Computing and Analysis Techniques in Physics Research (ACAT ): Jaipur, India, 2010 [PoS (ACAT) 057]	1009.1003
84	R. J. Barlow and C. Beeston	Fitting using finite monte carlo samples	Comput. Phys. Commun. 77 (1993) 219
85	F. U. Bernlochner, D. C. Fry, S. B. Menary, and E. Persson	Cover your bases: asymptotic distributions of the profile likelihood ratio when constraining effective field theories in high-energy physics	SciPost Phys. Core 6 (2023) 013	2207.01350
86	CMS Collaboration	Constraints on standard model effective field theory effects with Higgs bosons produced in association with W or Z bosons in the H $\rightarrow$ b $\mathrm{\bar{b}}$ decay channel in proton-proton collisions at $\sqrt{s}=$ 13 TeV	technical report, CERN, Geneva, 2024 CDS
87	ATLAS Collaboration	Measurements of $W^+W^-+\ge 1$ jet production cross-sections in $pp$ collisions at $\sqrt{s}=13$ TeV with the ATLAS detector	JHEP 06 (2021) 003	2103.10319
88	CMS Collaboration	Measurement of the inclusive and differential WZ production cross sections, polarization angles, and triple gauge couplings in pp collisions at $\sqrt{s}$ = 13 TeV	JHEP 07 (2022) 032	CMS-SMP-20-014 2110.11231