Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-PAS-HIG-22-013 | ||
| Search for heavy pseudoscalar and scalar bosons decaying to top quark pairs in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | ||
| CMS Collaboration | ||
| 1 October 2024 | ||
| Abstract: A search for heavy pseudoscalar or scalar bosons decaying to a top quark pair ($ \mathrm{t\bar{t}} $) in final states with one or two charged leptons is presented, using 138 fb$ ^{-1} $ of proton-proton collisions at $ \sqrt{s}= $ 13 TeV recorded by the CMS experiment at the CERN LHC. The invariant mass of the reconstructed $ \mathrm{t\bar{t}} $ system and variables sensitive to its spin state are used to discriminate against the standard model $ \mathrm{t\bar{t}} $ background. An excess of the data above the background prediction, as modeled using perturbative quantum chromodynamics (QCD) only, is observed. The excess is located close to the $ \mathrm{t\bar{t}} $ production threshold and it significantly favors the pseudoscalar signal hypothesis over the scalar hypothesis. It is compatible with the production of a $ ^1S_0^{[1]}$ $\mathrm{t\bar{t}} $ bound state ($ \eta_{\mathrm{t}} $), as predicted by a simplified model of nonrelativistic QCD, with a cross section of 7.1 pb and an uncertainty of 11%. The excess has a significance of above five standard deviations. Including the $ \eta_{\mathrm{t}} $ contribution in the background modeling, exclusion limits at 95% confidence level are set on the coupling of further pseudoscalar or scalar bosons to top quarks in a mass range of 365-1000 GeV and relative widths of 0.5-25%. | ||
| Links: CDS record (PDF) ; CADI line (restricted) ; | ||
| Figures & Tables | Summary | Additional Figures | References | CMS Publications |
|---|
| Figures | |
png pdf |
Figure 1:
The Feynman diagram for the signal process (left) and an example diagram for the SM production of top quark pairs (right). |
png pdf |
Figure 1-a:
The Feynman diagram for the signal process (left) and an example diagram for the SM production of top quark pairs (right). |
png pdf |
Figure 1-b:
The Feynman diagram for the signal process (left) and an example diagram for the SM production of top quark pairs (right). |
png pdf |
Figure 2:
Normalized differential cross sections in the spin correlation observables $ c_\text{hel} $ (left) and $ c_\text{han} $ (right) at the parton level in the $ \ell \bar{\ell} $ channel, with no requirements on acceptance, for SM $ \mathrm{t} \overline{\mathrm{t}} $ (black), resonant $ \mathrm{A} $ (red), resonant H (blue), $ \eta_{\mathrm{t}} $ (green) production. |
png pdf |
Figure 2-a:
Normalized differential cross sections in the spin correlation observables $ c_\text{hel} $ (left) and $ c_\text{han} $ (right) at the parton level in the $ \ell \bar{\ell} $ channel, with no requirements on acceptance, for SM $ \mathrm{t} \overline{\mathrm{t}} $ (black), resonant $ \mathrm{A} $ (red), resonant H (blue), $ \eta_{\mathrm{t}} $ (green) production. |
png pdf |
Figure 2-b:
Normalized differential cross sections in the spin correlation observables $ c_\text{hel} $ (left) and $ c_\text{han} $ (right) at the parton level in the $ \ell \bar{\ell} $ channel, with no requirements on acceptance, for SM $ \mathrm{t} \overline{\mathrm{t}} $ (black), resonant $ \mathrm{A} $ (red), resonant H (blue), $ \eta_{\mathrm{t}} $ (green) production. |
png pdf |
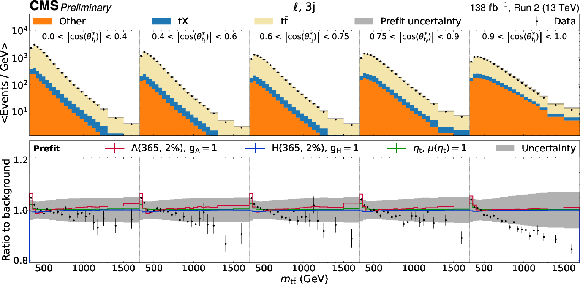
Figure 3:
Observed and expected $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ distribution in $ \lvert\cos\theta^\ast_{\mathrm{t}_{\ell}}\rvert $ bins, shown for the $ \ell,\,3{\mathrm{j}} $ channel summed over lepton flavors and analysis eras. In the first panel, the data (points with statistical error bars) and predicted pQCD-only background (colored histograms) are compared before the fit to the data, and the corresponding prefit uncertainty is shown with a gray band. In the second panel, the ratio of the data to the sum of the pQCD-only background is shown, and three signal hypotheses ($\mathrm{A(365,2\%)}$, $\mathrm{H(365,2\%)}$, and $ \eta_{\mathrm{t}} $) are overlaid for illustration. In the third and fourth panels, the ratio is shown with the best fit normalization applied for two different interpretations: either fitting only the $ {\mathrm{A}}\text{/}{\mathrm{H}} $ signal with no $ \eta_{\mathrm{t}} $ considered in the background (third panel), or fitting only $ \eta_{\mathrm{t}} $ (fourth panel). In both cases, the gray band shows the postfit uncertainty, and the respective signals are overlaid with their best fit model parameters. |
png pdf |
Figure 4:
Observed and expected $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ distribution in $ \lvert\cos\theta^\ast_{\mathrm{t}_{\ell}}\rvert $ bins, shown for the $ \ell,\,\geq4{\mathrm{j}} $ channel summed over lepton flavors and analysis eras. Notations as in Fig. 3. |
png pdf |
Figure 5:
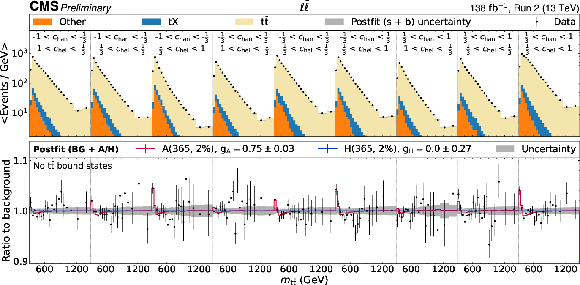
Observed and expected $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ distribution in $ c_\text{hel} $ and $ c_\text{han} $ bins, shown for the $ \ell \bar{\ell} $ channel summed over lepton flavors and analysis eras. Notations as in Fig. 3. |
png pdf |
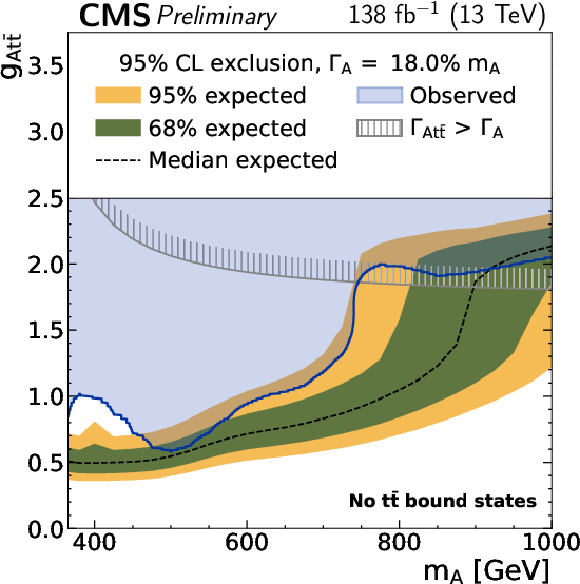
Figure 6:
Model-independent constraints on $ c $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 6-a:
Model-independent constraints on $ c $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 6-b:
Model-independent constraints on $ c $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 6-c:
Model-independent constraints on $ c $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 6-d:
Model-independent constraints on $ c $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 6-e:
Model-independent constraints on $ c $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 6-f:
Model-independent constraints on $ c $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
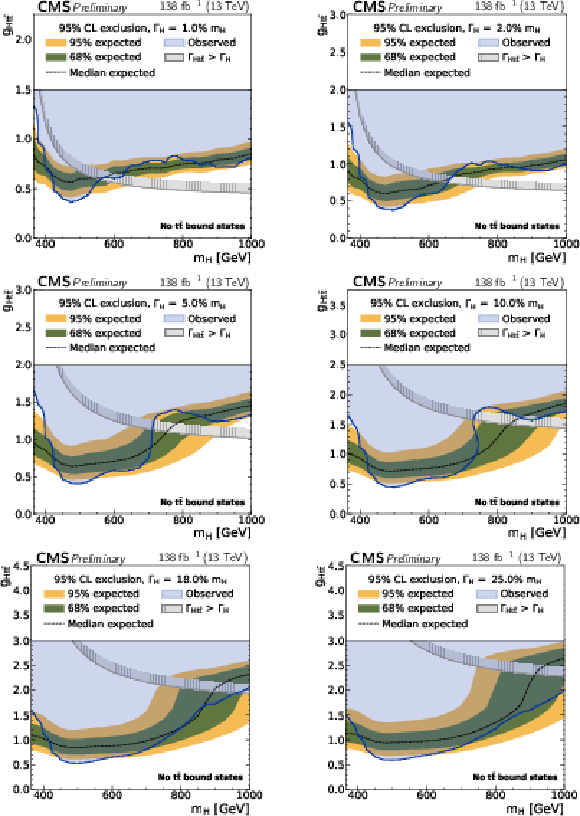
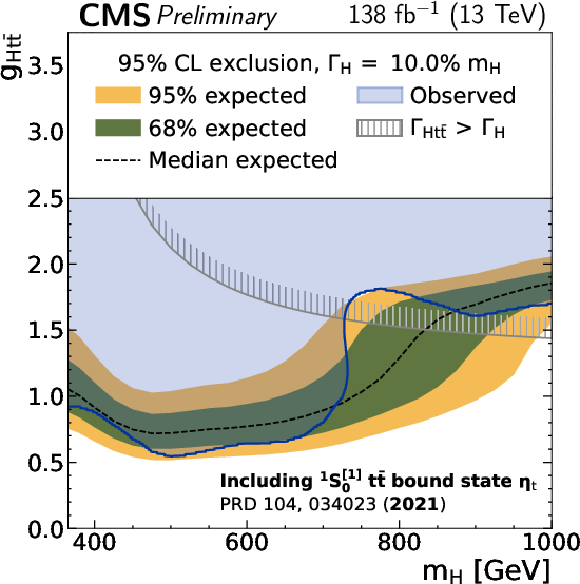
Figure 7:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 7-a:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 7-b:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 7-c:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 7-d:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 7-e:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 7-f:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 8:
Pulls and impacts of the nuisance parameters with the largest impact in the $ \eta_{\mathrm{t}} $ interpretation. A detailed description of the nuisance parameters relating to the jet $ p_{\mathrm{T}} $ scale is provided in Ref. [35]. |
png pdf |
Figure 9:
Correlation matrix between the $ \eta_{\mathrm{t}} $ signal strength and nuisance parameters with the largest impact in the $ \eta_{\mathrm{t}} $ interpretation. A detailed description of the nuisance parameters relating to the jet $ p_{\mathrm{T}} $ scale is provided in Ref. [35]. |
png pdf |
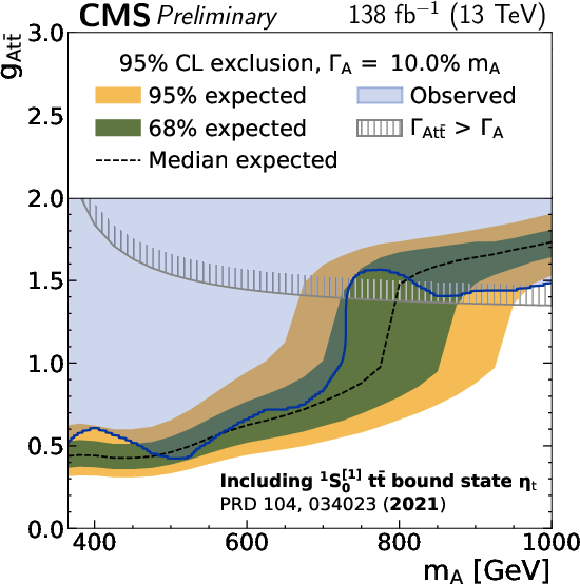
Figure 10:
Model-independent constraints on $ g_{\mathrm{A t\bar{t}}} $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 10-a:
Model-independent constraints on $ g_{\mathrm{A t\bar{t}}} $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 10-b:
Model-independent constraints on $ g_{\mathrm{A t\bar{t}}} $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 10-c:
Model-independent constraints on $ g_{\mathrm{A t\bar{t}}} $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 10-d:
Model-independent constraints on $ g_{\mathrm{A t\bar{t}}} $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 10-e:
Model-independent constraints on $ g_{\mathrm{A t\bar{t}}} $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 10-f:
Model-independent constraints on $ g_{\mathrm{A t\bar{t}}} $ as a function of the $ \mathrm{A} $ mass for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{A} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the $ \mathrm{A} $ total width is indicated by the hatched line. |
png pdf |
Figure 11:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 11-a:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 11-b:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 11-c:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 11-d:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 11-e:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
png pdf |
Figure 11-f:
Model-independent constraints on $ g_{\mathrm{H t\bar{t}}} $ as a function of the H mass, for relative widths of 1, 2, 5, 10, 18, and 25%. The observed constraints are indicated by the blue shaded area. The inner green band and the outer yellow band indicate the regions containing 68 and 95%, respectively, of the distribution of constraints expected under the background-only hypothesis. The unphysical region of phase space in which the partial width $ \Gamma_{\mathrm{H} \to {\mathrm{t}\overline{\mathrm{t}}} } $ becomes larger than the H total width is indicated by the hatched line. |
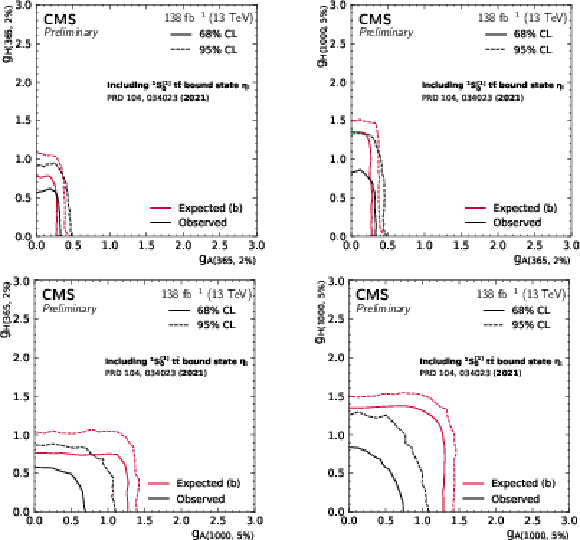
png pdf |
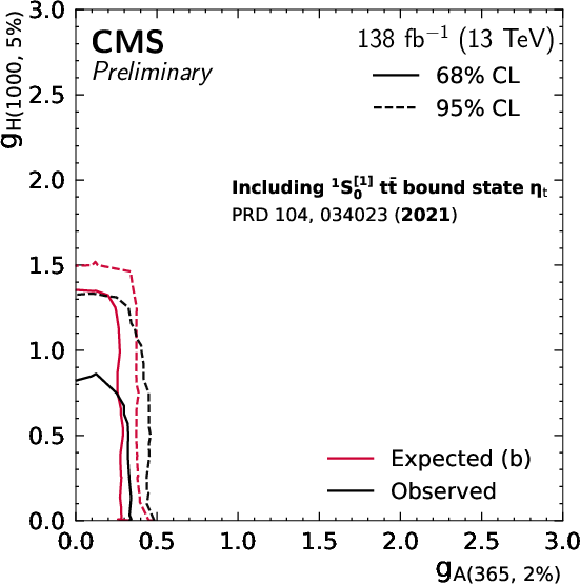
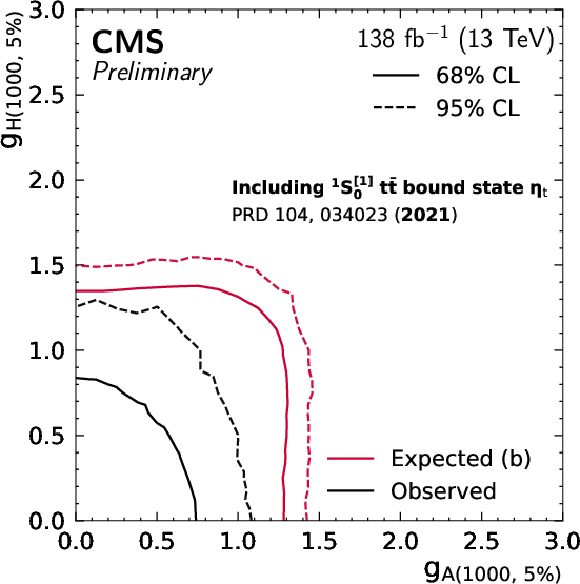
Figure 12:
Frequentist 2D exclusion contours for $ g_{\mathrm{A t\bar{t}}} $ and $ g_{\mathrm{H t\bar{t}}} $ in the $ {\mathrm{A}}\text{+}{\mathrm{H}} $ interpretation for four different signal hypotheses: $ g_{\mathrm{A(365,2\%)}} $ + $ g_{\mathrm{H(365,2\%)}} $ (upper left), $ g_{\mathrm{A(365,2\%)}} $ + $ g_{\mathrm{H(1000,5\%)}} $ (upper right), $ g_{\mathrm{A(1000,5\%)}} $ + $ g_{\mathrm{H(365,2\%)}} $ (lower left), and $ g_{\mathrm{A(1000,5\%)}} $+ $ g_{\mathrm{H(1000,5\%)}} $ (lower right). The expected and observed contours, evaluated with the Feldman--Cousins prescription [108], are shown in black and red, respectively, with the solid and dashed lines corresponding to exclusions at 68 and 95% CL, and the respective best-fit points for $ g_{\mathrm{A t\bar{t}}} $ and $ g_{\mathrm{H t\bar{t}}} $ are shown as the colored crosses. In all cases, the $ \eta_{\mathrm{t}} $ contribution is considered as part of the background. |
png pdf |
Figure 12-a:
Frequentist 2D exclusion contours for $ g_{\mathrm{A t\bar{t}}} $ and $ g_{\mathrm{H t\bar{t}}} $ in the $ {\mathrm{A}}\text{+}{\mathrm{H}} $ interpretation for four different signal hypotheses: $ g_{\mathrm{A(365,2\%)}} $ + $ g_{\mathrm{H(365,2\%)}} $ (upper left), $ g_{\mathrm{A(365,2\%)}} $ + $ g_{\mathrm{H(1000,5\%)}} $ (upper right), $ g_{\mathrm{A(1000,5\%)}} $ + $ g_{\mathrm{H(365,2\%)}} $ (lower left), and $ g_{\mathrm{A(1000,5\%)}} $+ $ g_{\mathrm{H(1000,5\%)}} $ (lower right). The expected and observed contours, evaluated with the Feldman--Cousins prescription [108], are shown in black and red, respectively, with the solid and dashed lines corresponding to exclusions at 68 and 95% CL, and the respective best-fit points for $ g_{\mathrm{A t\bar{t}}} $ and $ g_{\mathrm{H t\bar{t}}} $ are shown as the colored crosses. In all cases, the $ \eta_{\mathrm{t}} $ contribution is considered as part of the background. |
png pdf |
Figure 12-b:
Frequentist 2D exclusion contours for $ g_{\mathrm{A t\bar{t}}} $ and $ g_{\mathrm{H t\bar{t}}} $ in the $ {\mathrm{A}}\text{+}{\mathrm{H}} $ interpretation for four different signal hypotheses: $ g_{\mathrm{A(365,2\%)}} $ + $ g_{\mathrm{H(365,2\%)}} $ (upper left), $ g_{\mathrm{A(365,2\%)}} $ + $ g_{\mathrm{H(1000,5\%)}} $ (upper right), $ g_{\mathrm{A(1000,5\%)}} $ + $ g_{\mathrm{H(365,2\%)}} $ (lower left), and $ g_{\mathrm{A(1000,5\%)}} $+ $ g_{\mathrm{H(1000,5\%)}} $ (lower right). The expected and observed contours, evaluated with the Feldman--Cousins prescription [108], are shown in black and red, respectively, with the solid and dashed lines corresponding to exclusions at 68 and 95% CL, and the respective best-fit points for $ g_{\mathrm{A t\bar{t}}} $ and $ g_{\mathrm{H t\bar{t}}} $ are shown as the colored crosses. In all cases, the $ \eta_{\mathrm{t}} $ contribution is considered as part of the background. |
png pdf |
Figure 12-c:
Frequentist 2D exclusion contours for $ g_{\mathrm{A t\bar{t}}} $ and $ g_{\mathrm{H t\bar{t}}} $ in the $ {\mathrm{A}}\text{+}{\mathrm{H}} $ interpretation for four different signal hypotheses: $ g_{\mathrm{A(365,2\%)}} $ + $ g_{\mathrm{H(365,2\%)}} $ (upper left), $ g_{\mathrm{A(365,2\%)}} $ + $ g_{\mathrm{H(1000,5\%)}} $ (upper right), $ g_{\mathrm{A(1000,5\%)}} $ + $ g_{\mathrm{H(365,2\%)}} $ (lower left), and $ g_{\mathrm{A(1000,5\%)}} $+ $ g_{\mathrm{H(1000,5\%)}} $ (lower right). The expected and observed contours, evaluated with the Feldman--Cousins prescription [108], are shown in black and red, respectively, with the solid and dashed lines corresponding to exclusions at 68 and 95% CL, and the respective best-fit points for $ g_{\mathrm{A t\bar{t}}} $ and $ g_{\mathrm{H t\bar{t}}} $ are shown as the colored crosses. In all cases, the $ \eta_{\mathrm{t}} $ contribution is considered as part of the background. |
png pdf |
Figure 12-d:
Frequentist 2D exclusion contours for $ g_{\mathrm{A t\bar{t}}} $ and $ g_{\mathrm{H t\bar{t}}} $ in the $ {\mathrm{A}}\text{+}{\mathrm{H}} $ interpretation for four different signal hypotheses: $ g_{\mathrm{A(365,2\%)}} $ + $ g_{\mathrm{H(365,2\%)}} $ (upper left), $ g_{\mathrm{A(365,2\%)}} $ + $ g_{\mathrm{H(1000,5\%)}} $ (upper right), $ g_{\mathrm{A(1000,5\%)}} $ + $ g_{\mathrm{H(365,2\%)}} $ (lower left), and $ g_{\mathrm{A(1000,5\%)}} $+ $ g_{\mathrm{H(1000,5\%)}} $ (lower right). The expected and observed contours, evaluated with the Feldman--Cousins prescription [108], are shown in black and red, respectively, with the solid and dashed lines corresponding to exclusions at 68 and 95% CL, and the respective best-fit points for $ g_{\mathrm{A t\bar{t}}} $ and $ g_{\mathrm{H t\bar{t}}} $ are shown as the colored crosses. In all cases, the $ \eta_{\mathrm{t}} $ contribution is considered as part of the background. |
png pdf |
Figure A1:
Local significance contours for the pair of $\mathrm{A/H(365,2\%}$, considering only the resonant signal components. Different line styles are used to indicate the regions compatible with the data at progressive confidence levels. |
| Tables | |
png pdf |
Table 1:
Derived scale factors for the $ \mathrm{Z}\text{/}\gamma^\ast $ event yield in the different lepton flavor final states, and their statistical uncertainties. |
png pdf |
Table 2:
The systematic uncertainties considered in the analysis, indicating the number of corresponding nuisance parameters (if not one) in the statistical model, the type (affecting only normalization or also the shape of the search templates), and the affected processes and analysis channels they are applicable to. |
png pdf |
Table B1:
Results on the $ \eta_{\mathrm{t}} $ cross section, using only the $ \ell \bar{\ell} $ channels, for the $g_{\mathrm{A{365,{2\%}}}}$ background prediction and for the default setup. The quoted uncertainty for bb4$\ell$ assumes the same uncertainty as for the nominal result. |
png pdf |
Table C1:
Best-fit values of the signal strengths or coupling modifiers as well as differences in $ -2 \ln L $ between the best-fit point and the background-only hypothesis for the $ \eta_{\mathrm{t}} $, single $ \mathrm{A} $ boson, and single H boson interpretations. |
| Summary |
| A search for the production of heavy pseudoscalar or scalar bosons produced in proton-proton collisions at $ \sqrt{s}= $ 13 TeV and decaying to a top quark pair ($ \mathrm{t} \overline{\mathrm{t}} $) in the final states with one or two charged leptons is presented, using data corresponding to an integrated luminosity of 138 fb$ ^{-1} $ recorded with the CMS detector at the LHC. The invariant mass of the reconstructed $ \mathrm{t} \overline{\mathrm{t}} $ system and angular variables sensitive to its spin are used to discriminate the signal from the standard model $ \mathrm{t} \overline{\mathrm{t}} $ background. Both resonant production of the new boson and interference terms with the perturbative QCD (pQCD) $ \mathrm{t} \overline{\mathrm{t}} $ background are included in the signal model. A deviation from the background prediction, modeled only using pQCD, is observed. It is located close to the $ \mathrm{t} \overline{\mathrm{t}} $ production threshold, similar to the moderate deviation observed in a previous CMS search based on a data sample corresponding to an integrated luminosity of 35.9 fb$ ^{-1} $ [24]. This deviation significantly favors the pseudoscalar signal hypothesis over the scalar hypothesis. It is compatible with the production of a $ ^1S_0^{[1]} $ $ \mathrm{t} \overline{\mathrm{t}} $ bound state $ \eta_{\mathrm{t}} $, as predicted by a simplified model of nonrelativistic QCD. The cross section of this contribution is found to be $ \sigma (\eta_{\mathrm{t}}) = $ 7.1 pb, with an uncertainty of 11%. The excess has a significance of above five standard deviations. Further investigations by both the experimental and theoretical communities are necessary to elucidate the nature of this excess. Including $ \eta_{\mathrm{t}} $ production with an unconstrained normalization in the background prediction leads to a good description of the observed data, with no hint for further new pseudoscalar or scalar boson production. Exclusion limits at 95% confidence level are set on the coupling strength between top quarks and new bosons, covering masses of 365-1000 GeV and relative widths of 0.5-25%. Stringent constraints are found for the three cases of a new pseudoscalar boson, a new scalar boson, and the simultaneous presence of one new pseudoscalar and one new scalar boson, excluding coupling values as low as 0.4 (0.6) in the pseudoscalar (scalar) case. |
| Additional Figures | |
png pdf |
Additional Figure 1:
Normalized differential cross sections in the cosine of the top quark scattering angle $ \cos\theta^\ast_{\mathrm{t}} $ at the parton level in the $ \ell \mathrm{j} $ channel, with no requirements on acceptance, for SM $ \mathrm{t} \overline{\mathrm{t}} $ (black), resonant $ \mathrm{A} $ (red), resonant H (blue), $ \eta_{\mathrm{t}} $ (green) production. |
png pdf |
Additional Figure 2:
Observed and expected $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ distribution in $ \lvert\cos\theta^\ast_{\mathrm{t}_{\ell}}\rvert $ bins, shown for the $ \ell,\,3 \mathrm{j} $ channel summed over lepton flavors and analysis eras. In the upper panel, the data (points with statistical error bars) and predicted pQCD-only background (colored histograms) are compared before the fit to the data. In the lower panel, the ratio of the data to the sum of the pQCD-only background is shown, and the three signal hypotheses ($A(365,2\%)$, $H(365,2\%)$, and $ \eta_{\mathrm{t}} $) are overlaid for illustration. In both panels, the corresponding prefit uncertainty is shown with a gray band. The figure corresponds to the top ratio panel of Fig. 3 in the main body. |
png pdf |
Additional Figure 3:
Observed and expected $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ distribution in $ \lvert\cos\theta^\ast_{\mathrm{t}_{\ell}}\rvert $ bins, shown for the $ \ell,\,\geq4 \mathrm{j} $ channel summed over lepton flavors and analysis eras. Notations as in Additional Fig. 2. The figure corresponds to the top ratio panel of Fig. 4 in the main body. |
png pdf |
Additional Figure 4:
Observed and expected $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ distribution in $ c_\text{hel} $ and $ c_\text{han} $ bins, shown for the $ \ell \overline{\ell} $ channel summed over lepton flavors and analysis eras. Notations as in Additional Fig. 2. The figure corresponds to the top ratio panel of Fig. 5 in the main body. |
png pdf |
Additional Figure 5:
Observed and expected $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ distribution in $ \lvert\cos\theta^\ast_{\mathrm{t}_{\ell}}\rvert $ bins, shown for the $ \ell,\,3 \mathrm{j} $ channel summed over lepton flavors and analysis eras. In the upper panel, the data (points with statistical error bars) and predicted pQCD-only background (colored histograms) are compared after the fit to the data using only the $ {\mathrm{A}}\text{/}{\mathrm{H}} $ signal with no $ \eta_{\mathrm{t}} $ considered in the background. In the lower panel, the ratio of the data to the sum of the pQCD-only background is shown, and the two signal hypotheses ($A(365,2\%)$ and $H(365,2\%)$) at their best fit model parameters are overlaid for illustration. In both panels, the corresponding postfit uncertainty is shown with a gray band. The figure corresponds to the middle ratio panel of Fig. 3 in the main body. |
png pdf |
Additional Figure 6:
Observed and expected $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ distribution in $ \lvert\cos\theta^\ast_{\mathrm{t}_{\ell}}\rvert $ bins, shown for the $ \ell,\,\geq4 \mathrm{j} $ channel summed over lepton flavors and analysis eras. Notations as in Additional Fig. 5. The figure corresponds to the middle ratio panel of Fig. 4 in the main body. |
png pdf |
Additional Figure 7:
Observed and expected $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ distribution in $ c_\text{hel} $ and $ c_\text{han} $ bins, shown for the $ \ell \overline{\ell} $ channel summed over lepton flavors and analysis eras. Notations as in Additional Fig. 5. The figure corresponds to the middle ratio panel of Fig. 5 in the main body. |
png pdf |
Additional Figure 8:
Observed and expected $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ distribution in $ \lvert\cos\theta^\ast_{\mathrm{t}_{\ell}}\rvert $ bins, shown for the $ \ell,\,3 \mathrm{j} $ channel summed over lepton flavors and analysis eras. In the upper panel, the data (points with statistical error bars) and predicted pQCD-only background (colored histograms) are compared after the fit to the data using only $ \eta_{\mathrm{t}} $ as signal. In the lower panel, the ratio of the data to the sum of the pQCD-only background is shown, and the signal $ \eta_{\mathrm{t}} $ is overlaid with its best fit normalization for illustration. In both panels, the corresponding postfit uncertainty is shown with a gray band. The figure corresponds to the bottom ratio panel of Fig. 3 in the main body. |
png pdf |
Additional Figure 9:
Observed and expected $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ distribution in $ \lvert\cos\theta^\ast_{\mathrm{t}_{\ell}}\rvert $ bins, shown for the $ \ell,\,\geq4 \mathrm{j} $ channel summed over lepton flavors and analysis eras. Notations as in Additional Fig. 8. The figure corresponds to the bottom ratio panel of Fig. 4 in the main body. |
png pdf |
Additional Figure 10:
Observed and expected $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ distribution in $ c_\text{hel} $ and $ c_\text{han} $ bins, shown for the $ \ell \overline{\ell} $ channel summed over lepton flavors and analysis eras. Notations as in Additional Fig. 8. The figure corresponds to the bottom ratio panel of Fig. 5 in the main body. |
png pdf |
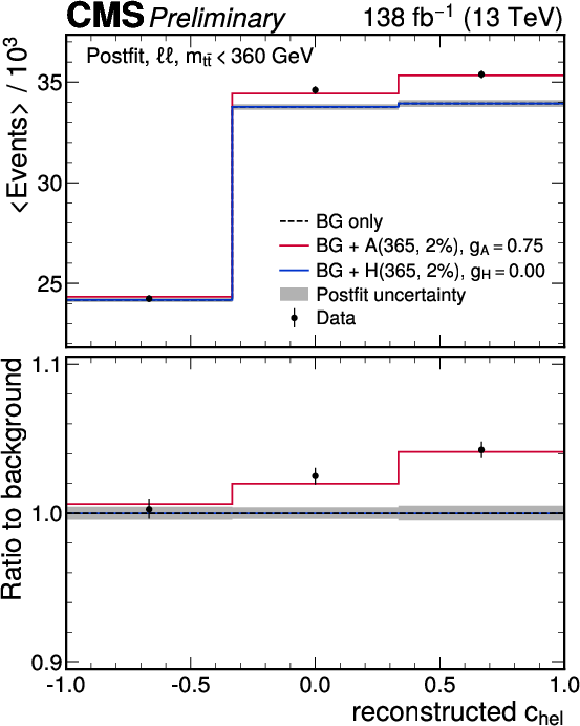
Additional Figure 11:
Observed and expected $ c_\text{hel} $ distributions in the $ \ell \overline{\ell} $ channel, summed over lepton flavors, analysis eras, $ c_\text{han} $ bins, and $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ ranges of $ m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 360 GeV (left) and 800 $ < m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 1050 GeV (right), before the fit to the data. $ \mathrm{A} $, H and $ \eta_{\mathrm{t}} $ signals are overlaid over the pQCD background prediction (black dashed line). The plots correspond to appropriate 1D projections of Fig. 5 in the main body. |
png pdf |
Additional Figure 11-a:
Observed and expected $ c_\text{hel} $ distributions in the $ \ell \overline{\ell} $ channel, summed over lepton flavors, analysis eras, $ c_\text{han} $ bins, and $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ ranges of $ m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 360 GeV (left) and 800 $ < m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 1050 GeV (right), before the fit to the data. $ \mathrm{A} $, H and $ \eta_{\mathrm{t}} $ signals are overlaid over the pQCD background prediction (black dashed line). The plots correspond to appropriate 1D projections of Fig. 5 in the main body. |
png pdf |
Additional Figure 11-b:
Observed and expected $ c_\text{hel} $ distributions in the $ \ell \overline{\ell} $ channel, summed over lepton flavors, analysis eras, $ c_\text{han} $ bins, and $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ ranges of $ m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 360 GeV (left) and 800 $ < m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 1050 GeV (right), before the fit to the data. $ \mathrm{A} $, H and $ \eta_{\mathrm{t}} $ signals are overlaid over the pQCD background prediction (black dashed line). The plots correspond to appropriate 1D projections of Fig. 5 in the main body. |
png pdf |
Additional Figure 12:
Observed and expected $ c_\text{hel} $ distributions in the $ \ell \overline{\ell} $ channel, summed over lepton flavors, analysis eras, $ c_\text{han} $ bins, and $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ ranges of $ m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 360 GeV (left) and 800 $ < m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 1050 GeV (right), after the fit to the data in the single-$ \Phi $ interpretation. $ \mathrm{A} $ and H signals are overlaid over the pQCD background prediction (black dashed line), corresponding to the best-fit values of $g_{\mathrm{A t\bar{t}}}$ and $g_{\mathrm{H t\bar{t}}}$. The plots correspond to appropriate 1D projections of Fig. 5 in the main body. |
png pdf |
Additional Figure 12-a:
Observed and expected $ c_\text{hel} $ distributions in the $ \ell \overline{\ell} $ channel, summed over lepton flavors, analysis eras, $ c_\text{han} $ bins, and $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ ranges of $ m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 360 GeV (left) and 800 $ < m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 1050 GeV (right), after the fit to the data in the single-$ \Phi $ interpretation. $ \mathrm{A} $ and H signals are overlaid over the pQCD background prediction (black dashed line), corresponding to the best-fit values of $g_{\mathrm{A t\bar{t}}}$ and $g_{\mathrm{H t\bar{t}}}$. The plots correspond to appropriate 1D projections of Fig. 5 in the main body. |
png pdf |
Additional Figure 12-b:
Observed and expected $ c_\text{hel} $ distributions in the $ \ell \overline{\ell} $ channel, summed over lepton flavors, analysis eras, $ c_\text{han} $ bins, and $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ ranges of $ m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 360 GeV (left) and 800 $ < m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 1050 GeV (right), after the fit to the data in the single-$ \Phi $ interpretation. $ \mathrm{A} $ and H signals are overlaid over the pQCD background prediction (black dashed line), corresponding to the best-fit values of $g_{\mathrm{A t\bar{t}}}$ and $g_{\mathrm{H t\bar{t}}}$. The plots correspond to appropriate 1D projections of Fig. 5 in the main body. |
png pdf |
Additional Figure 13:
Observed and expected $ c_\text{hel} $ distributions in the $ \ell \overline{\ell} $ channel, summed over lepton flavors, analysis eras, $ c_\text{han} $ bins, and a $ m_{{\mathrm{t}\overline{\mathrm{t}}} } $ range of $ m_{{\mathrm{t}\overline{\mathrm{t}}} } < $ 360 GeV, after the fit to the data in the $ \eta_{\mathrm{t}} $ interpretation. The $ \eta_{\mathrm{t}} $ signal is overlaid over the pQCD background prediction (black dashed line), corresponding to the best-fit value of $ \mu(\eta_{\mathrm{t}} ) $. The plot corresponds to an appropriate 1D projection of Fig. 5 in the main body. |
| References | ||||
| 1 | ATLAS Collaboration | Observation of a new particle in the search for the standard model Higgs boson with the ATLAS detector at the LHC | PLB 716 (2012) 1 | 1207.7214 |
| 2 | CMS Collaboration | Observation of a new boson at a mass of 125 GeV with the CMS experiment at the LHC | PLB 716 (2012) 30 | CMS-HIG-12-028 1207.7235 |
| 3 | CMS Collaboration | Observation of a new boson with mass near 125 GeV in $ {\mathrm{p}\mathrm{p}} $ collisions at $ \sqrt{s}= $ 7 and 8 TeV | JHEP 06 (2013) 081 | CMS-HIG-12-036 1303.4571 |
| 4 | G. C. Branco et al. | Theory and phenomenology of two-Higgs-doublet models | Phys. Rept. 516 (2012) 1 | 1106.0034 |
| 5 | K. Huitu et al. | Probing pseudo-Goldstone dark matter at the LHC | PRD 100 (2019) 015009 | 1812.05952 |
| 6 | M. Mühlleitner, M. O. P. Sampaio, R. Santos, and J. Wittbrodt | Phenomenological comparison of models with extended Higgs sectors | JHEP 08 (2017) 132 | 1703.07750 |
| 7 | J. Abdallah et al. | Simplified models for dark matter searches at the LHC | link | 1506.03116 |
| 8 | C. Arina et al. | A comprehensive approach to dark matter studies: exploration of simplified top-philic models | JHEP 11 (2016) 111 | 1605.09242 |
| 9 | N. Craig, J. Galloway, and S. Thomas | Searching for signs of the second Higgs doublet | 1305.2424 | |
| 10 | M. Carena and Z. Liu | Challenges and opportunities for heavy scalar searches in the $ \mathrm{t} \overline{\mathrm{t}} $ channel at the LHC | JHEP 11 (2016) 159 | 1608.07282 |
| 11 | A. Djouadi, J. Ellis, A. Popov, and J. Quevillon | Interference effects in $ \mathrm{t} \overline{\mathrm{t}} $ production at the LHC as a window on new physics | JHEP 03 (2019) 119 | 1901.03417 |
| 12 | K. J. F. Gaemers and F. Hoogeveen | Higgs production and decay into heavy flavours with the gluon fusion mechanism | PLB 146 (1984) 347 | |
| 13 | D. Dicus, A. Stange, and S. Willenbrock | Higgs decay to top quarks at hadron colliders | PLB 333 (1994) 126 | hep-ph/9404359 |
| 14 | W. Bernreuther, M. Flesch, and P. Haberl | Signatures of Higgs bosons in the top quark decay channel at hadron colliders | PRD 58 (1998) 114031 | hep-ph/9709284 |
| 15 | W. Bernreuther, A. Brandenburg, Z. G. Si, and P. Uwer | Top quark pair production and decay at hadron colliders | NPB 690 (2004) 81 | hep-ph/0403035 |
| 16 | G. Mahlon and S. J. Parke | Spin correlation effects in top quark pair production at the LHC | PRD 81 (2010) 074024 | 1001.3422 |
| 17 | A. H. Hoang et al. | Top-antitop pair production close to threshold: Synopsis of recent NNLO results | in Proc. 4th Workshop of the 2nd ECFA/DESY Study on Physics and Detectors for a Linear Electron-Positron Collider: Oxford, UK, 2000 Eur. Phys. J. direct 2 (2000) 1 |
hep-ph/0001286 |
| 18 | Y. Kiyo et al. | Top-quark pair production near threshold at LHC | EPJC 60 (2009) 375 | 0812.0919 |
| 19 | W.-L. Ju et al. | Top quark pair production near threshold: single/double distributions and mass determination | JHEP 06 (2020) 158 | 2004.03088 |
| 20 | B. Fuks, K. Hagiwara, K. Ma, and Y.-J. Zheng | Signatures of toponium formation in LHC run 2 data | PRD 104 (2021) 034023 | 2102.11281 |
| 21 | CMS Collaboration | Precision luminosity measurement in proton-proton collisions at $ \sqrt{s}= $ 13 TeV in 2015 and 2016 at CMS | EPJC 81 (2021) 800 | CMS-LUM-17-003 2104.01927 |
| 22 | CMS Collaboration | CMS luminosity measurement for the 2017 data-taking period at $ \sqrt{s}= $ 13 TeV | CMS Physics Analysis Summary, 2018 CMS-PAS-LUM-17-004 |
CMS-PAS-LUM-17-004 |
| 23 | CMS Collaboration | CMS luminosity measurement for the 2018 data-taking period at $ \sqrt{s}= $ 13 TeV | CMS Physics Analysis Summary, 2019 CMS-PAS-LUM-18-002 |
CMS-PAS-LUM-18-002 |
| 24 | CMS Collaboration | Search for heavy Higgs bosons decaying to a top quark pair in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JHEP 04 (2020) 171 | CMS-HIG-17-027 1908.01115 |
| 25 | ATLAS Collaboration | Search for heavy Higgs bosons $ \mathrm{A/H}$ decaying to a top quark pair in $ {\mathrm{p}\mathrm{p}} $ collisions at $ \sqrt{s}= $ 8 TeV with the ATLAS detector | PRL 119 (2017) 191803 | 1707.06025 |
| 26 | ATLAS Collaboration | Search for heavy neutral Higgs bosons decaying into a top quark pair in 140 fb$ ^{-1} $ of proton-proton collision data at $ \sqrt{s}= $ 13 TeV with the ATLAS detector | JHEP 08 (2024) 013 | 2404.18986 |
| 27 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | |
| 28 | CMS Collaboration | Development of the CMS detector for the CERN LHC Run 3 | JINST 19 (2024) P05064 | CMS-PRF-21-001 2309.05466 |
| 29 | CMS Collaboration | Performance of the CMS Level-1 trigger in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JINST 15 (2020) P10017 | CMS-TRG-17-001 2006.10165 |
| 30 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 31 | CMS Collaboration | Technical proposal for the Phase-II upgrade of the Compact Muon Solenoid | CMS Technical Proposal CERN-LHCC-2015-010, CMS-TDR-15-02, 2015 link |
|
| 32 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 33 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_{\mathrm{T}} $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 34 | M. Cacciari, G. P. Salam, and G. Soyez | FASTJET user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 35 | CMS Collaboration | Jet energy scale and resolution in the CMS experiment in $ {\mathrm{p}\mathrm{p}} $ collisions at 8 TeV | JINST 12 (2017) P02014 | CMS-JME-13-004 1607.03663 |
| 36 | CMS Collaboration | Identification of heavy-flavour jets with the CMS detector in $ {\mathrm{p}\mathrm{p}} $ collisions at 13 TeV | JINST 13 (2018) P05011 | CMS-BTV-16-002 1712.07158 |
| 37 | E. Bols et al. | Jet flavour classification using DeepJet | JINST 15 (2020) P12012 | 2008.10519 |
| 38 | CMS Collaboration | Performance summary of AK4 jet b tagging with data from proton-proton collisions at 13 TeV with the CMS detector | CMS Detector Performance Note CMS-DP-2023-005, 2023 CDS |
|
| 39 | CMS Collaboration | Electron and photon reconstruction and identification with the CMS experiment at the CERN LHC | JINST 16 (2021) P05014 | CMS-EGM-17-001 2012.06888 |
| 40 | CMS Collaboration | ECAL 2016 refined calibration and Run 2 summary plots | CMS Detector Performance Note CMS-DP-2020-021, 2020 CDS |
|
| 41 | CMS Collaboration | Performance of the CMS muon detector and muon reconstruction with proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JINST 13 (2018) P06015 | CMS-MUO-16-001 1804.04528 |
| 42 | CMS Collaboration | Performance of missing transverse momentum reconstruction in proton-proton collisions at $ \sqrt{s}= $ 13 TeV using the CMS detector | JINST 14 (2019) P07004 | CMS-JME-17-001 1903.06078 |
| 43 | CMS Collaboration | Simulation of the silicon strip tracker pre-amplifier in early 2016 data | CMS Detector Performance Note CMS-DP-2020-045, 2020 CDS |
|
| 44 | NNPDF Collaboration | Parton distributions for the LHC run II | JHEP 04 (2015) 040 | 1410.8849 |
| 45 | T. Sjöstrand et al. | An introduction to PYTHIA8.2 | Comput. Phys. Commun. 191 (2015) 159 | 1410.3012 |
| 46 | P. Skands, S. Carrazza, and J. Rojo | Tuning PYTHIA8.1: the Monash 2013 tune | EPJC 74 (2014) 3024 | 1404.5630 |
| 47 | CMS Collaboration | Extraction and validation of a new set of CMS PYTHIA8 tunes from underlying-event measurements | EPJC 80 (2020) 4 | CMS-GEN-17-001 1903.12179 |
| 48 | GEANT4 Collaboration | GEANT 4---a simulation toolkit | NIM A 506 (2003) 250 | |
| 49 | CMS Collaboration | Pileup mitigation at CMS in 13 TeV data | JINST 15 (2020) P09018 | CMS-JME-18-001 2003.00503 |
| 50 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 51 | M. Spira, A. Djouadi, D. Graudenz, and P. M. Zerwas | Higgs boson production at the LHC | NPB 453 (1995) 17 | hep-ph/9504378 |
| 52 | B. Hespel, F. Maltoni, and E. Vryonidou | Signal background interference effects in heavy scalar production and decay to a top-anti-top pair | JHEP 10 (2016) 016 | 1606.04149 |
| 53 | R. V. Harlander, S. Liebler, and H. Mantler | SusHi: A program for the calculation of Higgs production in gluon fusion and bottom-quark annihilation in the standard model and the MSSM | Comput. Phys. Commun. 184 (2013) 1605 | 1212.3249 |
| 54 | R. V. Harlander, S. Liebler, and H. Mantler | SusHi bento: Beyond NNLO and the heavy-top limit | Comput. Phys. Commun. 212 (2017) 239 | 1605.03190 |
| 55 | D. Eriksson, J. Rathsman, and O. St \aa l | 2hdmc---two-Higgs-doublet model calculator | Comput. Phys. Commun. 181 (2010) 189 | 0902.0851 |
| 56 | A. Banfi et al. | Higgs interference effects in top-quark pair production in the 1HSM | Submitted to JHEP, 2023 | 2309.16759 |
| 57 | Y. Sumino and H. Yokoya | Bound-state effects on kinematical distributions of top quarks at hadron colliders | JHEP 09 (2010) 034 | 1007.0075 |
| 58 | P. Nason | A new method for combining NLO QCD with shower Monte Carlo algorithms | JHEP 11 (2004) 040 | hep-ph/0409146 |
| 59 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with parton shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 60 | S. Alioli, P. Nason, C. Oleari, and E. Re | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG box | JHEP 06 (2010) 043 | 1002.2581 |
| 61 | J. M. Campbell, R. K. Ellis, P. Nason, and E. Re | Top-pair production and decay at NLO matched with parton showers | JHEP 04 (2015) 114 | 1412.1828 |
| 62 | M. Czakon and A. Mitov | top++: a program for the calculation of the top-pair cross-section at hadron colliders | Comput. Phys. Commun. 185 (2014) 2930 | 1112.5675 |
| 63 | M. Grazzini, S. Kallweit, and M. Wiesemann | Fully differential NNLO computations with matrix | EPJC 78 (2018) 537 | 1711.06631 |
| 64 | M. Aliev et al. | hathor: Hadronic top and heavy quarks cross section calculator | Comput. Phys. Commun. 182 (2011) 1034 | 1007.1327 |
| 65 | J. H. Kühn, A. Scharf, and P. Uwer | Electroweak corrections to top-quark pair production in quark-antiquark annihilation | EPJC 45 (2006) 139 | hep-ph/0508092 |
| 66 | J. H. Kühn, A. Scharf, and P. Uwer | Electroweak effects in top-quark pair production at hadron colliders | EPJC 51 (2007) 37 | hep-ph/0610335 |
| 67 | J. H. Kühn, A. Scharf, and P. Uwer | Weak interactions in top-quark pair production at hadron colliders: An update | PRD 91 (2015) 014020 | 1305.5773 |
| 68 | E. Re | Single-top $ {\mathrm{W}\mathrm{t}} $-channel production matched with parton showers using the POWHEG method | EPJC 71 (2011) 1547 | 1009.2450 |
| 69 | S. Alioli, P. Nason, C. Oleari, and E. Re | NLO single-top production matched with shower in POWHEG: $ s $- and $ t $-channel contributions | JHEP 09 (2009) 111 | 0907.4076 |
| 70 | P. Kant et al. | hathor for single top-quark production: Updated predictions and uncertainty estimates for single top-quark production in hadronic collisions | Comput. Phys. Commun. 191 (2015) 74 | 1406.4403 |
| 71 | N. Kidonakis | Top quark production | in Proc. Helmholtz International Summer School on Physics of Heavy Quarks and Hadrons, HQ 2013, Dubna, 2013 link |
1311.0283 |
| 72 | P. F. Monni et al. | MiNNLOps: a new method to match NNLO QCD to parton showers | JHEP 05 (2020) 143 | 1908.06987 |
| 73 | P. F. Monni, E. Re, and M. Wiesemann | MiNNLO\textsubscriptps: optimizing 2 $ \to $ 1 hadronic processes | EPJC 80 (2020) 1075 | 2006.04133 |
| 74 | E. Barberio and Z. W \c a s | photos---a universal Monte Carlo for QED radiative corrections: version 2.0 | Comput. Phys. Commun. 79 (1994) 291 | |
| 75 | P. Golonka and Z. Was | photos Monte Carlo: a precision tool for QED corrections in Z and W decays | EPJC 45 (2006) 97 | hep-ph/0506026 |
| 76 | J. Alwall et al. | Comparative study of various algorithms for the merging of parton showers and matrix elements in hadronic collisions | EPJC 53 (2008) 473 | 0706.2569 |
| 77 | K. Melnikov and F. Petriello | Electroweak gauge boson production at hadron colliders through $ \mathcal{O}({\alpha_\mathrm{S}^2}) $ | PRD 74 (2006) 114017 | hep-ph/0609070 |
| 78 | Y. Li and F. Petriello | Combining QCD and electroweak corrections to dilepton production in FEWZ | PRD 86 (2012) 094034 | 1208.5967 |
| 79 | T. Gehrmann et al. | $ {\mathrm{W^+}\mathrm{W^-}} $ production at hadron colliders in next to next to leading order QCD | PRL 113 (2014) 212001 | 1408.5243 |
| 80 | J. M. Campbell and R. K. Ellis | MCFM for the Tevatron and the LHC | in Proc. 10th DESY Workshop on Elementary Particle Theory: Loops and Legs in Quantum Field Theory (LL): Wörlitz, Germany, 2010 link |
1007.3492 |
| 81 | S. Frixione and B. R. Webber | Matching NLO QCD computations and parton shower simulations | JHEP 06 (2002) 029 | hep-ph/0204244 |
| 82 | B. A. Betchart, R. Demina, and A. Harel | Analytic solutions for neutrino momenta in decay of top quarks | NIM A 736 (2014) 169 | 1305.1878 |
| 83 | R. Demina, A. Harel, and D. Orbaker | Reconstructing $ \mathrm{t} \overline{\mathrm{t}} $ events with one lost jet | NIM A 788 (2015) 128 | 1310.3263 |
| 84 | CMS Collaboration | Measurement of the differential cross section for top quark pair production in $ {\mathrm{p}\mathrm{p}} $ collisions at $ \sqrt{s} $ = 8 TeV | EPJC 75 (2015) 542 | CMS-TOP-12-028 1505.04480 |
| 85 | L. Sonnenschein | Analytical solution of $ \mathrm{t} \overline{\mathrm{t}} $ dilepton equations | PRD 73 (2006) 054015 | hep-ph/0603011 |
| 86 | W. Bernreuther, D. Heisler, and Z.-G. Si | A set of top quark spin correlation and polarization observables for the LHC: Standard model predictions and new physics contributions | JHEP 12 (2015) 026 | 1508.05271 |
| 87 | J. A. Aguilar-Saavedra and J. A. Casas | Improved tests of entanglement and Bell inequalities with LHC tops | EPJC 82 (2022) 666 | 2205.00542 |
| 88 | F. Maltoni, C. Severi, S. Tentori, and E. Vryonidou | Quantum detection of new physics in top-quark pair production at the LHC | JHEP 03 (2024) 099 | 2401.08751 |
| 89 | CMS Collaboration | Measurement of the $ \mathrm{t} \overline{\mathrm{t}} $ production cross section and the top quark mass in the dilepton channel in $ {\mathrm{p}\mathrm{p}} $ collisions at $ \sqrt{s}= $ 7 TeV | JHEP 07 (2011) 049 | CMS-TOP-11-002 1105.5661 |
| 90 | CMS Collaboration | The CMS statistical analysis and combination tool: combine | Accepted by Comput. Softw. Big Sci, 2024 | CMS-CAT-23-001 2404.06614 |
| 91 | CMS Collaboration | Combined measurements of Higgs boson couplings in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | EPJC 79 (2019) 421 | CMS-HIG-17-031 1809.10733 |
| 92 | CMS Collaboration | Measurement of the top quark mass using proton-proton data at $ \sqrt{s}= $ 7 and 8 TeV | PRD 93 (2016) 072004 | CMS-TOP-14-022 1509.04044 |
| 93 | M. Botje et al. | The PDF4LHC working group interim recommendations | 1101.0538 | |
| 94 | J. R. Christiansen and P. Z. Skands | String formation beyond leading colour | JHEP 08 (2015) 003 | 1505.01681 |
| 95 | CMS Collaboration | CMS PYTHIA8 colour reconnection tunes based on underlying-event data | EPJC 83 (2023) 587 | CMS-GEN-17-002 2205.02905 |
| 96 | CMS Collaboration | Investigations of the impact of the parton shower tuning in PYTHIA8 in the modelling of $ \mathrm{t} \overline{\mathrm{t}} $ at $ \sqrt{s}= $ 8 and 13 TeV | CMS Physics Analysis Summary, 2016 CMS-PAS-TOP-16-021 |
CMS-PAS-TOP-16-021 |
| 97 | ATLAS Collaboration | Measurement of the inclusive cross-sections of single top-quark and top-antiquark $ t $-channel production in $ {\mathrm{p}\mathrm{p}} $ collisions at $ \sqrt{s}= $ 13 TeV with the ATLAS detector | JHEP 04 (2017) 086 | 1609.03920 |
| 98 | CMS Collaboration | Measurement of the single top quark and antiquark production cross sections in the $ t $ channel and their ratio in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | PLB 800 (2020) 135042 | CMS-TOP-17-011 1812.10514 |
| 99 | CMS Collaboration | Measurement of the production cross section for single top quarks in association with W bosons in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JHEP 10 (2018) 117 | CMS-TOP-17-018 1805.07399 |
| 100 | CMS Collaboration | Measurement of the cross section for top quark pair production in association with a W or Z boson in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JHEP 08 (2018) 011 | CMS-TOP-17-005 1711.02547 |
| 101 | ATLAS Collaboration | Measurement of the $ {{\mathrm{t}\overline{\mathrm{t}}} \mathrm{Z}} $ and $ {{\mathrm{t}\overline{\mathrm{t}}} \mathrm{W}} $ cross sections in proton-proton collisions at $ \sqrt{s}= $ 13 TeV with the ATLAS detector | PRD 99 (2019) 072009 | 1901.03584 |
| 102 | ATLAS Collaboration | Measurements of top-quark pair to Z-boson cross-section ratios at $ \sqrt{s}= $ 13, 8, 7 TeV with the ATLAS detector | JHEP 02 (2017) 117 | 1612.03636 |
| 103 | R. Barlow and C. Beeston | Fitting using finite Monte Carlo samples | Comput. Phys. Commun. 77 (1993) 219 | |
| 104 | G. Cowan, K. Cranmer, E. Gross, and O. Vitells | Asymptotic formulae for likelihood-based tests of new physics | EPJC 71 (2011) 1554 | 1007.1727 |
| 105 | ATLAS and CMS Collaborations, and LHC Higgs Combination Group | Procedure for the LHC Higgs boson search combination in Summer 2011 | Technical Report CMS-NOTE-2011-005, ATL-PHYS-PUB-2011-11, 2011 | |
| 106 | T. Junk | Confidence level computation for combining searches with small statistics | NIM A 434 (1999) 435 | hep-ex/9902006 |
| 107 | A. L. Read | Presentation of search results: The $ \text{CL}_\text{s} $ technique | JPG 28 (2002) 2693 | |
| 108 | G. J. Feldman and R. D. Cousins | Unified approach to the classical statistical analysis of small signals | PRD 57 (1998) 3873 | physics/9711021 |
| 109 | T. Ježo et al. | An NLO+PS generator for $ \mathrm{t} \overline{\mathrm{t}} $ and $ {\mathrm{W}\mathrm{t}} $ production and decay including non-resonant and interference effects | EPJC 76 (2016) 691 | 1607.04538 |
| 110 | T. Ježo, J. M. Lindert, and S. Pozzorini | Resonance-aware NLOPS matching for off-shell $ {\mathrm{t}\overline{\mathrm{t}}} +{\mathrm{t}\mathrm{W}} $ production with semileptonic decays | JHEP 10 (2023) 008 | 2307.15653 |
| 111 | T. Ježo and P. Nason | On the treatment of resonances in next-to-leading order calculations matched to a parton shower | JHEP 12 (2015) 065 | 1509.09071 |
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|