Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-PAS-TOP-24-001 | ||
| Measurement of the dineutrino system kinematics in dileptonic top quark pair events in pp collisions at $ \sqrt{s} = $ 13 TeV | ||
| CMS Collaboration | ||
| 31 July 2024 | ||
| Abstract: Differential top quark pair cross sections are measured in the dilepton final states $ e^{+}e^{-} $, $ \mu^{+}\mu^{-} $, and $ e^{\pm}\mu^{\mp} $, as a function of kinematics of the system of two neutrinos: the transverse momentum $ p_{T}^{\nu\nu} $ of the dineutrino system, the minimum distance in azimuthal angle between $ p_{T}^{\nu\nu} $ and leptons, and in two dimensions in bins of both observables. The measurements are performed using CERN LHC proton-proton collisions at $ \sqrt{s} = $ 13 TeV, recorded by the CMS detector between 2016 and 2018, corresponding to an integrated luminosity of 138 fb$ ^{-1} $. The measured cross sections are unfolded to the particle level using an unregularized least square method. The obtained results are found to be in agreement with the latest theory predictions and Monte Carlo simulations of the standard model. | ||
| Links: CDS record (PDF) ; Physics Briefing ; CADI line (restricted) ; | ||
| Figures | |
png pdf |
Figure 1:
In the left diagram the standard model $ \mathrm{t} \overline{\mathrm{t}} $ process is sketched, while the right diagram shows the production of a hypothetical stop pair, where both stops decay to a top and a neutralino. This analysis focuses on signatures, where both of the W bosons decay leptonically. |
png pdf |
Figure 1-a:
In the left diagram the standard model $ \mathrm{t} \overline{\mathrm{t}} $ process is sketched, while the right diagram shows the production of a hypothetical stop pair, where both stops decay to a top and a neutralino. This analysis focuses on signatures, where both of the W bosons decay leptonically. |
png pdf |
Figure 1-b:
In the left diagram the standard model $ \mathrm{t} \overline{\mathrm{t}} $ process is sketched, while the right diagram shows the production of a hypothetical stop pair, where both stops decay to a top and a neutralino. This analysis focuses on signatures, where both of the W bosons decay leptonically. |
png pdf |
Figure 2:
Observed (black markers) and expected distributions of leading lepton $ p_{\mathrm{T}} $ (top left), leading jet $ p_{\mathrm{T}} $ (top right), the number of jets (bottom left), and the number of b-tagged jets (bottom right), after event selection. The hatched (grey) areas denote the systematic (total) uncertainties on the expected yields. Events from all data-taking periods and all channels are combined. The lower panel of each plot shows the ratio between observed and expected distributions. The last bin includes all events above the plotted range. |
png pdf |
Figure 2-a:
Observed (black markers) and expected distributions of leading lepton $ p_{\mathrm{T}} $ (top left), leading jet $ p_{\mathrm{T}} $ (top right), the number of jets (bottom left), and the number of b-tagged jets (bottom right), after event selection. The hatched (grey) areas denote the systematic (total) uncertainties on the expected yields. Events from all data-taking periods and all channels are combined. The lower panel of each plot shows the ratio between observed and expected distributions. The last bin includes all events above the plotted range. |
png pdf |
Figure 2-b:
Observed (black markers) and expected distributions of leading lepton $ p_{\mathrm{T}} $ (top left), leading jet $ p_{\mathrm{T}} $ (top right), the number of jets (bottom left), and the number of b-tagged jets (bottom right), after event selection. The hatched (grey) areas denote the systematic (total) uncertainties on the expected yields. Events from all data-taking periods and all channels are combined. The lower panel of each plot shows the ratio between observed and expected distributions. The last bin includes all events above the plotted range. |
png pdf |
Figure 2-c:
Observed (black markers) and expected distributions of leading lepton $ p_{\mathrm{T}} $ (top left), leading jet $ p_{\mathrm{T}} $ (top right), the number of jets (bottom left), and the number of b-tagged jets (bottom right), after event selection. The hatched (grey) areas denote the systematic (total) uncertainties on the expected yields. Events from all data-taking periods and all channels are combined. The lower panel of each plot shows the ratio between observed and expected distributions. The last bin includes all events above the plotted range. |
png pdf |
Figure 2-d:
Observed (black markers) and expected distributions of leading lepton $ p_{\mathrm{T}} $ (top left), leading jet $ p_{\mathrm{T}} $ (top right), the number of jets (bottom left), and the number of b-tagged jets (bottom right), after event selection. The hatched (grey) areas denote the systematic (total) uncertainties on the expected yields. Events from all data-taking periods and all channels are combined. The lower panel of each plot shows the ratio between observed and expected distributions. The last bin includes all events above the plotted range. |
png pdf |
Figure 3:
Difference between $ p_{\mathrm{T,gen.}}^\text{miss} $ and $ p_{\mathrm{T,rec.}}^\text{miss} $ as a function of the $ p_{\mathrm{T,gen.}}^\text{miss} $ (left) and the number of primary vertices (right) for signal events. The mean difference between the generated and the $ p_{\mathrm{T,rec.}}^\text{miss} $ per bin is shown as solid line, while the dashed line shows the corresponding $ \sigma $, which corresponds to the resolution. The results for $ p_{\mathrm{T}}^\text{miss} $ corrected by the DNN regression (light blue), derived with the PUPPI algorithm (red), and the PF algorithm (orange) are shown. A simulated sample for the 2018 data-taking period is used. |
png pdf |
Figure 3-a:
Difference between $ p_{\mathrm{T,gen.}}^\text{miss} $ and $ p_{\mathrm{T,rec.}}^\text{miss} $ as a function of the $ p_{\mathrm{T,gen.}}^\text{miss} $ (left) and the number of primary vertices (right) for signal events. The mean difference between the generated and the $ p_{\mathrm{T,rec.}}^\text{miss} $ per bin is shown as solid line, while the dashed line shows the corresponding $ \sigma $, which corresponds to the resolution. The results for $ p_{\mathrm{T}}^\text{miss} $ corrected by the DNN regression (light blue), derived with the PUPPI algorithm (red), and the PF algorithm (orange) are shown. A simulated sample for the 2018 data-taking period is used. |
png pdf |
Figure 3-b:
Difference between $ p_{\mathrm{T,gen.}}^\text{miss} $ and $ p_{\mathrm{T,rec.}}^\text{miss} $ as a function of the $ p_{\mathrm{T,gen.}}^\text{miss} $ (left) and the number of primary vertices (right) for signal events. The mean difference between the generated and the $ p_{\mathrm{T,rec.}}^\text{miss} $ per bin is shown as solid line, while the dashed line shows the corresponding $ \sigma $, which corresponds to the resolution. The results for $ p_{\mathrm{T}}^\text{miss} $ corrected by the DNN regression (light blue), derived with the PUPPI algorithm (red), and the PF algorithm (orange) are shown. A simulated sample for the 2018 data-taking period is used. |
png pdf |
Figure 4:
Breakdown of the relative uncertainties from experimental (left) and theory (right) uncertainties on the differential cross section measurement as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center) and both variables (bottom). In the latter case, each group of 4 bins corresponds to $ p_{\text{T}}^{\nu\nu} $ bins, and $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ bin edges are indicated by vertical dashed lines. The statistical uncertainty (dark grey) takes the available statistics of both the simulated samples and the recorded data into account. |
png pdf |
Figure 4-a:
Breakdown of the relative uncertainties from experimental (left) and theory (right) uncertainties on the differential cross section measurement as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center) and both variables (bottom). In the latter case, each group of 4 bins corresponds to $ p_{\text{T}}^{\nu\nu} $ bins, and $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ bin edges are indicated by vertical dashed lines. The statistical uncertainty (dark grey) takes the available statistics of both the simulated samples and the recorded data into account. |
png pdf |
Figure 4-b:
Breakdown of the relative uncertainties from experimental (left) and theory (right) uncertainties on the differential cross section measurement as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center) and both variables (bottom). In the latter case, each group of 4 bins corresponds to $ p_{\text{T}}^{\nu\nu} $ bins, and $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ bin edges are indicated by vertical dashed lines. The statistical uncertainty (dark grey) takes the available statistics of both the simulated samples and the recorded data into account. |
png pdf |
Figure 4-c:
Breakdown of the relative uncertainties from experimental (left) and theory (right) uncertainties on the differential cross section measurement as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center) and both variables (bottom). In the latter case, each group of 4 bins corresponds to $ p_{\text{T}}^{\nu\nu} $ bins, and $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ bin edges are indicated by vertical dashed lines. The statistical uncertainty (dark grey) takes the available statistics of both the simulated samples and the recorded data into account. |
png pdf |
Figure 4-d:
Breakdown of the relative uncertainties from experimental (left) and theory (right) uncertainties on the differential cross section measurement as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center) and both variables (bottom). In the latter case, each group of 4 bins corresponds to $ p_{\text{T}}^{\nu\nu} $ bins, and $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ bin edges are indicated by vertical dashed lines. The statistical uncertainty (dark grey) takes the available statistics of both the simulated samples and the recorded data into account. |
png pdf |
Figure 4-e:
Breakdown of the relative uncertainties from experimental (left) and theory (right) uncertainties on the differential cross section measurement as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center) and both variables (bottom). In the latter case, each group of 4 bins corresponds to $ p_{\text{T}}^{\nu\nu} $ bins, and $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ bin edges are indicated by vertical dashed lines. The statistical uncertainty (dark grey) takes the available statistics of both the simulated samples and the recorded data into account. |
png pdf |
Figure 4-f:
Breakdown of the relative uncertainties from experimental (left) and theory (right) uncertainties on the differential cross section measurement as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center) and both variables (bottom). In the latter case, each group of 4 bins corresponds to $ p_{\text{T}}^{\nu\nu} $ bins, and $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ bin edges are indicated by vertical dashed lines. The statistical uncertainty (dark grey) takes the available statistics of both the simulated samples and the recorded data into account. |
png pdf |
Figure 5:
The observed (black markers) and simulated distributions of $ p_{\mathrm{T,DNN}}^\text{miss} $ (top left), $ \text{min}[\Delta\phi(p_{\mathrm{T}}^\text{miss},\ell)] $ (top right), and the two-dimensional distribution with both observables (bottom) are shown. Events from all data-taking periods and all channels are combined. The hatched (grey) areas indicate the systematic (total) uncertainty on the simulation. The bottom panel of each plot shows the ratio of observed data to the expectation from MC simulation in each bin. The last bin includes all events above the plotted range. |
png pdf |
Figure 5-a:
The observed (black markers) and simulated distributions of $ p_{\mathrm{T,DNN}}^\text{miss} $ (top left), $ \text{min}[\Delta\phi(p_{\mathrm{T}}^\text{miss},\ell)] $ (top right), and the two-dimensional distribution with both observables (bottom) are shown. Events from all data-taking periods and all channels are combined. The hatched (grey) areas indicate the systematic (total) uncertainty on the simulation. The bottom panel of each plot shows the ratio of observed data to the expectation from MC simulation in each bin. The last bin includes all events above the plotted range. |
png pdf |
Figure 5-b:
The observed (black markers) and simulated distributions of $ p_{\mathrm{T,DNN}}^\text{miss} $ (top left), $ \text{min}[\Delta\phi(p_{\mathrm{T}}^\text{miss},\ell)] $ (top right), and the two-dimensional distribution with both observables (bottom) are shown. Events from all data-taking periods and all channels are combined. The hatched (grey) areas indicate the systematic (total) uncertainty on the simulation. The bottom panel of each plot shows the ratio of observed data to the expectation from MC simulation in each bin. The last bin includes all events above the plotted range. |
png pdf |
Figure 5-c:
The observed (black markers) and simulated distributions of $ p_{\mathrm{T,DNN}}^\text{miss} $ (top left), $ \text{min}[\Delta\phi(p_{\mathrm{T}}^\text{miss},\ell)] $ (top right), and the two-dimensional distribution with both observables (bottom) are shown. Events from all data-taking periods and all channels are combined. The hatched (grey) areas indicate the systematic (total) uncertainty on the simulation. The bottom panel of each plot shows the ratio of observed data to the expectation from MC simulation in each bin. The last bin includes all events above the plotted range. |
png pdf |
Figure 6:
Result of the closure test based on simulation. The potential BSM contribution is scaled by a factor of ten. The test is performed for the $ p_{\text{T}}^{\nu\nu} $ (top) and 2D measurement (bottom) using the nominal (black), the regularized (orange), and the bin-by-bin unfolding (purple), based on all data-taking periods combined. The unfolded distributions are compared to the expected distribution (red) based on the sum of the nominal dileptonic $ \mathrm{t} \overline{\mathrm{t}} $ signal sample and BSM signal with the corresponding $ \chi^2/\text{ndf} $ values given in the legend. The nominal distribution, used for the response matrix, is shown in blue. The error bars correspond to the statistical uncertainty. The lower part shows the ratios between the unfolded and the expected distributions. |
png pdf |
Figure 6-a:
Result of the closure test based on simulation. The potential BSM contribution is scaled by a factor of ten. The test is performed for the $ p_{\text{T}}^{\nu\nu} $ (top) and 2D measurement (bottom) using the nominal (black), the regularized (orange), and the bin-by-bin unfolding (purple), based on all data-taking periods combined. The unfolded distributions are compared to the expected distribution (red) based on the sum of the nominal dileptonic $ \mathrm{t} \overline{\mathrm{t}} $ signal sample and BSM signal with the corresponding $ \chi^2/\text{ndf} $ values given in the legend. The nominal distribution, used for the response matrix, is shown in blue. The error bars correspond to the statistical uncertainty. The lower part shows the ratios between the unfolded and the expected distributions. |
png pdf |
Figure 6-b:
Result of the closure test based on simulation. The potential BSM contribution is scaled by a factor of ten. The test is performed for the $ p_{\text{T}}^{\nu\nu} $ (top) and 2D measurement (bottom) using the nominal (black), the regularized (orange), and the bin-by-bin unfolding (purple), based on all data-taking periods combined. The unfolded distributions are compared to the expected distribution (red) based on the sum of the nominal dileptonic $ \mathrm{t} \overline{\mathrm{t}} $ signal sample and BSM signal with the corresponding $ \chi^2/\text{ndf} $ values given in the legend. The nominal distribution, used for the response matrix, is shown in blue. The error bars correspond to the statistical uncertainty. The lower part shows the ratios between the unfolded and the expected distributions. |
png pdf |
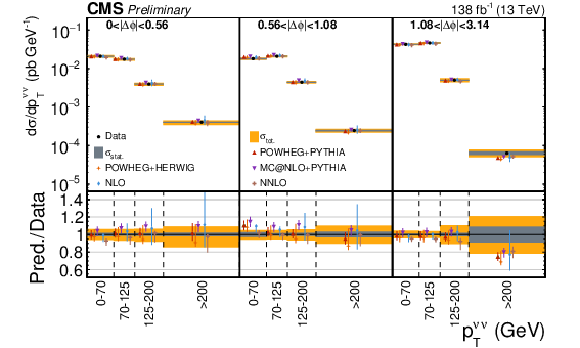
Figure 7:
The measured signal cross sections (black markers) as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center), and both observables in two dimensions (bottom) are shown. The theoretical predictions from POWHEG +PYTHIA (dark red), POWHEG + HERWIG (orange), MC@NLO+PYTHIA (purple), and the fixed-order NLO (light blue) and NNLO (brown) calculations are compared to the measurement. The total (statistical) uncertainty on the measurement is shown as an orange (dark grey) band. The bottom panel of each plot shows the ratio between theoretical predictions and the measurement. |
png pdf |
Figure 7-a:
The measured signal cross sections (black markers) as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center), and both observables in two dimensions (bottom) are shown. The theoretical predictions from POWHEG +PYTHIA (dark red), POWHEG + HERWIG (orange), MC@NLO+PYTHIA (purple), and the fixed-order NLO (light blue) and NNLO (brown) calculations are compared to the measurement. The total (statistical) uncertainty on the measurement is shown as an orange (dark grey) band. The bottom panel of each plot shows the ratio between theoretical predictions and the measurement. |
png pdf |
Figure 7-b:
The measured signal cross sections (black markers) as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center), and both observables in two dimensions (bottom) are shown. The theoretical predictions from POWHEG +PYTHIA (dark red), POWHEG + HERWIG (orange), MC@NLO+PYTHIA (purple), and the fixed-order NLO (light blue) and NNLO (brown) calculations are compared to the measurement. The total (statistical) uncertainty on the measurement is shown as an orange (dark grey) band. The bottom panel of each plot shows the ratio between theoretical predictions and the measurement. |
png pdf |
Figure 7-c:
The measured signal cross sections (black markers) as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center), and both observables in two dimensions (bottom) are shown. The theoretical predictions from POWHEG +PYTHIA (dark red), POWHEG + HERWIG (orange), MC@NLO+PYTHIA (purple), and the fixed-order NLO (light blue) and NNLO (brown) calculations are compared to the measurement. The total (statistical) uncertainty on the measurement is shown as an orange (dark grey) band. The bottom panel of each plot shows the ratio between theoretical predictions and the measurement. |
png pdf |
Figure 8:
The measured normalized signal cross sections (black markers) as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center), and both observables in two dimensions (bottom) are shown. The theoretical predictions from POWHEG +PYTHIA (dark red), POWHEG + HERWIG (orange), MC@NLO+PYTHIA (purple), and the fixed-order NLO (light blue) and NNLO (brown) calculations are compared to the measurement. The total (statistical) uncertainty on the measurement is shown as an orange (dark grey) band. The bottom panel of each plot shows the ratio between theoretical predictions and the measurement. |
png pdf |
Figure 8-a:
The measured normalized signal cross sections (black markers) as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center), and both observables in two dimensions (bottom) are shown. The theoretical predictions from POWHEG +PYTHIA (dark red), POWHEG + HERWIG (orange), MC@NLO+PYTHIA (purple), and the fixed-order NLO (light blue) and NNLO (brown) calculations are compared to the measurement. The total (statistical) uncertainty on the measurement is shown as an orange (dark grey) band. The bottom panel of each plot shows the ratio between theoretical predictions and the measurement. |
png pdf |
Figure 8-b:
The measured normalized signal cross sections (black markers) as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center), and both observables in two dimensions (bottom) are shown. The theoretical predictions from POWHEG +PYTHIA (dark red), POWHEG + HERWIG (orange), MC@NLO+PYTHIA (purple), and the fixed-order NLO (light blue) and NNLO (brown) calculations are compared to the measurement. The total (statistical) uncertainty on the measurement is shown as an orange (dark grey) band. The bottom panel of each plot shows the ratio between theoretical predictions and the measurement. |
png pdf |
Figure 8-c:
The measured normalized signal cross sections (black markers) as a function of $ p_{\text{T}}^{\nu\nu} $ (top), $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $ (center), and both observables in two dimensions (bottom) are shown. The theoretical predictions from POWHEG +PYTHIA (dark red), POWHEG + HERWIG (orange), MC@NLO+PYTHIA (purple), and the fixed-order NLO (light blue) and NNLO (brown) calculations are compared to the measurement. The total (statistical) uncertainty on the measurement is shown as an orange (dark grey) band. The bottom panel of each plot shows the ratio between theoretical predictions and the measurement. |
| Tables | |
png pdf |
Table 1:
Data and MC simulation yields after selection, combined for all data-taking periods and split by channels. The uncertainties on the simulation yield include systematic and statistical uncertainties (see also Section 6). The relative contribution in percent of each process to the total expected yield of a channel is given in parentheses. |
png pdf |
Table 2:
Definition of the fiducial phase space for the same-flavor channels and the different-flavor channel. The two leptons have to be oppositely charged. |
png pdf |
Table 3:
Results of the $ \chi^2 $ tests for the absolute and normalized differential cross section measurements for each of the predictions. The $ \chi^2 $ values including uncertainties on the predictions are given in parentheses. |
png pdf |
Table 4:
p-values of the $ \chi^2 $ tests for the absolute and normalized cross section measurements for each of the predictions. The resulting p-values including uncertainties on the predictions are given in parentheses. |
| Summary |
| Measurements of differential top pair production cross section in the dileptonic channel in pp collisions at $ \sqrt{s}= $ 13 TeV using observables based on the dineutrino kinematics have been presented. The measurements are performed based on collision data recorded by the CMS detector between 2016 and 2018 during the Run 2 operation of the LHC corresponding to an integrated luminosity of 138 fb$^{-1}$. The differential cross sections are derived as a function of the transverse momentum of the dineutrino system $ p_{\text{T}}^{\nu\nu} $ and the minimal azimuthal angle between the dineutrino system and a lepton $ \text{min}[\Delta\phi(p_{\text{T}}^{\nu\nu},\ell)] $, as well as both observables in two dimensions. To improve the resolution of the missing transverse momentum, which serves as a measure for $ p_{\text{T}}^{\nu\nu} $ in signal events, a dedicated DNN regression has been developed. The method significantly improves the resolution of both the magnitude and the azimuthal angle of the missing transverse momentum. The absolute and normalized differential cross section results are obtained based on an unregularized least square unfolding method. The differential cross sections are compared to predictions based on MC simulation as well as two fixed-order theory calculations, corresponding to NLO and NNLO accuracy in QCD. Given the so far unexplored observables and phase space regions considered in this analysis, remarkable agreement between the different theory predictions and the measured differential cross sections has been observed. For both one-dimensional measurements, the best overall description is provided by the two POWHEG predictions, while for the two-dimensional measurement the best agreement is observed for the NNLO fixed-order calculation. However, the differences between the five predictions are mostly small, such that none of the predictions is significantly disfavored by the measured differential cross sections. |
| References | ||||
| 1 | ATLAS Collaboration | Measurements of top quark pair relative differential cross-sections with ATLAS in $ pp $ collisions at $ \sqrt{s}= $ 7 TeV | EPJC 73 (2013) 2261 | 1207.5644 |
| 2 | CMS Collaboration | Measurement of Differential Top-Quark Pair Production Cross Sections in $ pp $ collisions at $ \sqrt{s}= $ 7 TeV | EPJC 73 (2013) 2339 | CMS-TOP-11-013 1211.2220 |
| 3 | ATLAS Collaboration | Measurements of normalized differential cross sections for $ t\bar{t} $ production in pp collisions at $ \sqrt{s}= $ 7 TeV using the ATLAS detector | PRD 90 (2014) 072004 | 1407.0371 |
| 4 | ATLAS Collaboration | Differential top-antitop cross-section measurements as a function of observables constructed from final-state particles using pp collisions at $ \sqrt{s}= $ 7 TeV in the ATLAS detector | JHEP 06 (2015) 100 | 1502.05923 |
| 5 | ATLAS Collaboration | Measurement of top quark pair differential cross-sections in the dilepton channel in $ pp $ collisions at $ \sqrt{s} = $ 7 and 8 TeV with ATLAS | PRD 94 (2016) 092003 | 1607.07281 |
| 6 | CMS Collaboration | Measurement of the differential cross section for top quark pair production in pp collisions at $ \sqrt{s} = $ 8 TeV | EPJC 75 (2015) 542 | CMS-TOP-12-028 1505.04480 |
| 7 | ATLAS Collaboration | Measurements of top-quark pair differential cross-sections in the lepton+jets channel in $ pp $ collisions at $ \sqrt{s}= $ 8 TeV using the ATLAS detector | EPJC 76 (2016) 538 | 1511.04716 |
| 8 | ATLAS Collaboration | Measurement of the differential cross-section of highly boosted top quarks as a function of their transverse momentum in $ \sqrt{s} = $ 8 TeV proton-proton collisions using the ATLAS detector | PRD 93 (2016) 032009 | 1510.03818 |
| 9 | CMS Collaboration | Measurement of the $ \mathrm{t}\overline{{\mathrm{t}}} $ production cross section in the all-jets final state in pp collisions at $ \sqrt{s}= $ 8 TeV | EPJC 76 (2016) 128 | CMS-TOP-14-018 1509.06076 |
| 10 | CMS Collaboration | Measurement of the integrated and differential $ t \bar t $ production cross sections for high-$ p_t $ top quarks in $ pp $ collisions at $ \sqrt s = $ 8 TeV | PRD 94 (2016) 072002 | CMS-TOP-14-012 1605.00116 |
| 11 | CMS Collaboration | Measurement of double-differential cross sections for top quark pair production in pp collisions at $ \sqrt{s} = $ 8 TeV and impact on parton distribution functions | EPJC 77 (2017) 459 | CMS-TOP-14-013 1703.01630 |
| 12 | ATLAS Collaboration | Measurement of the top-quark mass in $ t\bar{t}+ $ 1-jet events collected with the ATLAS detector in $ pp $ collisions at $ \sqrt{s}= $ 8 TeV | JHEP 11 (2019) 150 | 1905.02302 |
| 13 | CMS Collaboration | Measurement of differential cross sections for top quark pair production using the lepton+jets final state in proton-proton collisions at 13 TeV | PRD 95 (2017) 092001 | CMS-TOP-16-008 1610.04191 |
| 14 | ATLAS Collaboration | Measurement of jet activity produced in top-quark events with an electron, a muon and two $ b $-tagged jets in the final state in $ pp $ collisions at $ \sqrt{s}= $ 13 TeV with the ATLAS detector | EPJC 77 (2017) 220 | 1610.09978 |
| 15 | ATLAS Collaboration | Measurements of top-quark pair differential cross-sections in the $ e\mu $ channel in $ pp $ collisions at $ \sqrt{s} = $ 13 TeV using the ATLAS detector | EPJC 77 (2017) 292 | 1612.05220 |
| 16 | CMS Collaboration | Measurement of normalized differential $ \mathrm{t}\overline{\mathrm{t}} $ cross sections in the dilepton channel from pp collisions at $ \sqrt{s}= $ 13 TeV | JHEP 04 (2018) 060 | CMS-TOP-16-007 1708.07638 |
| 17 | CMS Collaboration | Measurement of differential cross sections for the production of top quark pairs and of additional jets in lepton+jets events from pp collisions at $ \sqrt{s} = $ 13 TeV | PRD 97 (2018) 112003 | CMS-TOP-17-002 1803.08856 |
| 18 | CMS Collaboration | Measurements of $ \mathrm{t\overline{t}} $ differential cross sections in proton-proton collisions at $ \sqrt{s}= $ 13 TeV using events containing two leptons | JHEP 02 (2019) 149 | CMS-TOP-17-014 1811.06625 |
| 19 | CMS Collaboration | Measurement of $ \mathrm{t\bar t} $ normalised multi-differential cross sections in pp collisions at $ \sqrt s= $ 13 TeV, and simultaneous determination of the strong coupling strength, top quark pole mass, and parton distribution functions | EPJC 80 (2020) 658 | CMS-TOP-18-004 1904.05237 |
| 20 | ATLAS Collaboration | Measurements of top-quark pair differential and double-differential cross-sections in the $ \ell $+jets channel with $ pp $ collisions at $ \sqrt{s}= $ 13 TeV using the ATLAS detector | EPJC 79 (2019) 1028 | 1908.07305 |
| 21 | ATLAS Collaboration | Measurement of the $ t\bar{t} $ production cross-section and lepton differential distributions in $ e\mu $ dilepton events from $ pp $ collisions at $ \sqrt{s} = $ 13 TeV with the ATLAS detector | EPJC 80 (2020) 528 | 1910.08819 |
| 22 | ATLAS Collaboration | Measurements of top-quark pair single- and double-differential cross-sections in the all-hadronic channel in $ pp $ collisions at $ \sqrt{s} = $ 13 TeV using the ATLAS detector | JHEP 01 (2021) 033 | 2006.09274 |
| 23 | CMS Collaboration | Measurement of differential $ t \bar t $ production cross sections in the full kinematic range using lepton+jets events from proton-proton collisions at $ \sqrt {s} = $ 13 TeV | PRD 104 (2021) 092013 | CMS-TOP-20-001 2108.02803 |
| 24 | CMS Collaboration | Search for top squark pair production using dilepton final states in $ {\text {p}}{\text {p}} $ collision data collected at $ \sqrt{s} = $ 13 TeV | EPJC 81 (2021) 3 | CMS-SUS-19-011 2008.05936 |
| 25 | CMS Collaboration | Combined searches for the production of supersymmetric top quark partners in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | EPJC 81 (2021) 970 | CMS-SUS-20-002 2107.10892 |
| 26 | M. Czakon, A. Mitov, and R. Poncelet | NNLO QCD corrections to leptonic observables in top-quark pair production and decay | JHEP 05 (2021) 212 | 2008.11133 |
| 27 | CMS Collaboration | Performance of the CMS Level-1 trigger in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JINST 15 (2020) P10017 | CMS-TRG-17-001 2006.10165 |
| 28 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 29 | CMS Collaboration | The CMS Experiment at the CERN LHC | JINST 3 (2008) S08004 | |
| 30 | CMS Collaboration | Development of the CMS detector for the CERN LHC Run 3 | JINST 19 (2024) P05064 | CMS-PRF-21-001 2309.05466 |
| 31 | CMS Collaboration | Precision luminosity measurement in proton-proton collisions at $ \sqrt{s} = $ 13 TeV in 2015 and 2016 at CMS | EPJC 81 (2021) 800 | CMS-LUM-17-003 2104.01927 |
| 32 | CMS Collaboration | CMS luminosity measurement for the 2017 data-taking period at $ \sqrt{s} = $ 13 TeV | CMS Physics Analysis Summary, 2018 CMS-PAS-LUM-17-004 |
CMS-PAS-LUM-17-004 |
| 33 | CMS Collaboration | CMS luminosity measurement for the 2018 data-taking period at $ \sqrt{s} = $ 13 TeV | CMS Physics Analysis Summary, 2018 CMS-PAS-LUM-18-002 |
CMS-PAS-LUM-18-002 |
| 34 | NNPDF Collaboration | Unbiased global determination of parton distributions and their uncertainties at NNLO and at LO | NPB 855 (2012) 153 | 1107.2652 |
| 35 | NNPDF Collaboration | Parton distributions from high-precision collider data | EPJC 77 (2017) 663 | 1706.00428 |
| 36 | T. Sjöstrand et al. | An introduction to PYTHIA 8.2 | Comput. Phys. Commun. 191 (2015) 159 | 1410.3012 |
| 37 | CMS Collaboration | Extraction and validation of a new set of CMS PYTHIA8 tunes from underlying-event measurements | EPJC 80 (2020) 4 | CMS-GEN-17-001 1903.12179 |
| 38 | GEANT4 Collaboration | GEANT4--a simulation toolkit | NIM A 506 (2003) 250 | |
| 39 | P. Nason | A New method for combining NLO QCD with shower Monte Carlo algorithms | JHEP 11 (2004) 040 | hep-ph/0409146 |
| 40 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with Parton Shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 41 | S. Alioli, P. Nason, C. Oleari, and E. Re | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX | JHEP 06 (2010) 043 | 1002.2581 |
| 42 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 43 | R. Frederix and S. Frixione | Merging meets matching in MC@NLO | JHEP 12 (2012) 061 | 1209.6215 |
| 44 | J. Bellm et al. | Herwig 7.0/Herwig++ 3.0 release note | EPJC 76 (2016) 196 | 1512.01178 |
| 45 | CMS Collaboration | Development and validation of HERWIG 7 tunes from CMS underlying-event measurements | EPJC 81 (2021) 312 | CMS-GEN-19-001 2011.03422 |
| 46 | J. Alwall et al. | Comparative study of various algorithms for the merging of parton showers and matrix elements in hadronic collisions | EPJC 53 (2008) 473 | 0706.2569 |
| 47 | S. Alioli, P. Nason, C. Oleari, and E. Re | NLO single-top production matched with shower in POWHEG: s- and t-channel contributions | JHEP 09 (2009) 111 | 0907.4076 |
| 48 | E. Re | Single-top Wt-channel production matched with parton showers using the POWHEG method | EPJC 71 (2011) 1547 | 1009.2450 |
| 49 | M. Czakon and A. Mitov | Top++: A Program for the Calculation of the Top-Pair Cross-Section at Hadron Colliders | Comput. Phys. Commun. 185 (2014) 2930 | 1112.5675 |
| 50 | M. Czakon, P. Fiedler, and A. Mitov | Total Top-Quark Pair-Production Cross Section at Hadron Colliders Through $ O(\alpha^4_S) $ | PRL 110 (2013) 252004 | 1303.6254 |
| 51 | M. Cacciari et al. | Top-pair production at hadron colliders with next-to-next-to-leading logarithmic soft-gluon resummation | PLB 710 (2012) 612 | 1111.5869 |
| 52 | P. B ä rnreuther, M. Czakon, and A. Mitov | Percent Level Precision Physics at the Tevatron: First Genuine NNLO QCD Corrections to $ q \bar{q} \to t \bar{t} + X $ | PRL 109 (2012) 132001 | 1204.5201 |
| 53 | M. Czakon and A. Mitov | NNLO corrections to top-pair production at hadron colliders: the all-fermionic scattering channels | JHEP 12 (2012) 054 | 1207.0236 |
| 54 | M. Czakon et al. | Top-pair production at the LHC through NNLO QCD and NLO EW | JHEP 10 (2017) 186 | 1705.04105 |
| 55 | R. Gavin, Y. Li, F. Petriello, and S. Quackenbush | FEWZ 2.0: A code for hadronic Z production at next-to-next-to-leading order | Comput. Phys. Commun. 182 (2011) 2388 | 1011.3540 |
| 56 | Y. Li and F. Petriello | Combining QCD and electroweak corrections to dilepton production in FEWZ | PRD 86 (2012) 094034 | 1208.5967 |
| 57 | N. Kidonakis | Two-loop soft anomalous dimensions for single top quark associated production with a $ W^- $ or $ H^- $ | PRD 82 (2010) 054018 | 1005.4451 |
| 58 | M. Aliev et al. | HATHOR: HAdronic Top and Heavy quarks crOss section calculatoR | Comput. Phys. Commun. 182 (2011) 1034 | 1007.1327 |
| 59 | P. Kant et al. | HatHor for single top-quark production: Updated predictions and uncertainty estimates for single top-quark production in hadronic collisions | Comput. Phys. Commun. 191 (2015) 74 | 1406.4403 |
| 60 | T. Gehrmann et al. | $ W^+W^- $ Production at Hadron Colliders in Next to Next to Leading Order QCD | PRL 113 (2014) 212001 | 1408.5243 |
| 61 | J. M. Campbell, R. K. Ellis, and C. Williams | Vector Boson Pair Production at the LHC | JHEP 07 (2011) 018 | 1105.0020 |
| 62 | D. Contardo et al. | Technical Proposal for the Phase-II Upgrade of the CMS Detector | Technical report, CERN, 2015 link |
|
| 63 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 64 | CMS Collaboration | Performance of the CMS electromagnetic calorimeter in pp collisions at $ \sqrt{s} = $ 13 TeV | Submitted to JINST, 2024 | CMS-EGM-18-002 2403.15518 |
| 65 | CMS Collaboration | Performance of CMS muon reconstruction from proton-proton to heavy ion collisions | Submitted to JINST, 2024 | CMS-MUO-21-001 2404.17377 |
| 66 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_t $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 67 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet User Manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 68 | CMS Collaboration | Pileup mitigation at CMS in 13 TeV data | JINST 15 (2020) P09018 | CMS-JME-18-001 2003.00503 |
| 69 | E. Bols et al. | Jet Flavour Classification Using DeepJet | JINST 15 (2020) P12012 | 2008.10519 |
| 70 | CMS Collaboration | Performance of missing transverse momentum reconstruction in proton-proton collisions at $ \sqrt{s} = $ 13 TeV using the CMS detector | JINST 14 (2019) P07004 | CMS-JME-17-001 1903.06078 |
| 71 | D. Bertolini, P. Harris, M. Low, and N. Tran | Pileup Per Particle Identification | JHEP 10 (2014) 059 | 1407.6013 |
| 72 | CMS Collaboration | Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV | JINST 13 (2018) P05011 | CMS-BTV-16-002 1712.07158 |
| 73 | M. Abadi et al. | TensorFlow: Large-Scale Machine Learning on Heterogeneous Distributed Systems | 1603.04467 | |
| 74 | F. Chollet et al | Keras | link | |
| 75 | R. D. Cousins | Generalization of chisquare goodness-of-fit test for binned data using saturated models, with application to histograms | link | |
| 76 | CMS Collaboration | The CMS Statistical Analysis and Combination Tool: COMBINE | Submitted to Computing and Software for Big Science, 2024 | CMS-CAT-23-001 2404.06614 |
| 77 | CMS Collaboration | Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV | JINST 12 (2017) P02014 | CMS-JME-13-004 1607.03663 |
| 78 | CMS Collaboration | Differential cross section measurements for the production of top quark pairs and of additional jets using dilepton events from pp collisions at $ \sqrt{s} = $ 13 TeV | Submitted to JHEP, 2024 | CMS-TOP-20-006 2402.08486 |
| 79 | A. Andreassen and B. Nachman | Neural Networks for Full Phase-space Reweighting and Parameter Tuning | PRD 101 (2020) 091901 | 1907.08209 |
| 80 | CMS Collaboration | Reweighting of simulated events using machine learning techniques in CMS | CMS Physics Analysis Summary, 2024 CMS-PAS-MLG-24-001 |
CMS-PAS-MLG-24-001 |
| 81 | CMS Collaboration | CMS pythia 8 colour reconnection tunes based on underlying-event data | EPJC 83 (2023) 587 | CMS-GEN-17-002 2205.02905 |
| 82 | S. Schmitt | TUnfold: an algorithm for correcting migration effects in high energy physics | JINST 7 (2012) T10003 | 1205.6201 |
| 83 | ATLAS Collaboration | Inclusive and differential cross-sections for dilepton $ t\overline{t} $ production measured in $ \sqrt{s} = $ 13 TeV pp collisions with the ATLAS detector | JHEP 07 (2023) 141 | 2303.15340 |
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|