Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-PAS-SUS-23-018 | ||
| Search for DM in association with b-quark and lepton pairs | ||
| CMS Collaboration | ||
| 20 July 2024 | ||
| Abstract: A search is performed for dark matter produced in association with bottom quark and lepton pairs in data collected by the CMS detector at the LHC, corresponding to 138 fb$ ^{-1} $ of proton-proton collisions at a center-of-mass energy of 13 TeV. The analysis explores for the first time at the LHC the associated production of a bottom quark-antiquark pair and a new neutral Higgs boson that subsequently decays into a Z boson and a pseudoscalar, where the latter acts as dark matter mediator in the context of the 2HDM+$ a $ model. The analysis probes final states with a pair of same-flavor leptons arising in decays of Z bosons, which tend to be produced in opposite directions with respect to the dark matter particles that originate from the decay of the pseudoscalar. The resulting imbalance in transverse momentum in combination with observables related to the lepton pair is exploited in this search. Furthermore, we make use of novel multivariate techniques that target the wide range of kinematic scenarios occurring for the various mass configurations. | ||
| Links: CDS record (PDF) ; Physics Briefing ; CADI line (restricted) ; | ||
| Figures | |
png pdf |
Figure 1:
Example graph for heavy pseudoscalar mediator production decaying into Dark Matter particles, in association with $ \mathrm{Z}(\to \mathrm{l}\bar{\mathrm{l}}) \mathrm{b}\overline{\mathrm{b}} $ |
png pdf |
Figure 2:
Signal and control regions definition. All requirements are on top of the baseline selection. |
png pdf |
Figure 3:
The distribution of the effective missing transverse momentum ($ p_{\mathrm{T}}^\text{miss} $) computed for each process as described in the text is shown for each CR defined. A comparison between the predicted shape in simulation and the observed distribution in data is presented for the DY (upper left), $ {\mathrm{t}\overline{\mathrm{t}}} $ (upper right), WZ (lower left), and ZZ (lower right) control regions after performing a B-only fit simultaneously across all CRs. With the exception of DY CR, where by definition a hard cut at $ p_{\mathrm{T}}^\text{miss} = $ 140 GeV exists, in all other cases the last bin includes the overflow. The lower panel shows the post-fit values and uncertainties of the ratio between the observed data and the predicted SM background. The various background processes are represented with color-filled histograms, with the color as indicated in the legend for each case. The data points are shown as black dots with vertical and horizontal error bars, in this case comprising the total collected data in Run 2. |
png pdf |
Figure 3-a:
The distribution of the effective missing transverse momentum ($ p_{\mathrm{T}}^\text{miss} $) computed for each process as described in the text is shown for each CR defined. A comparison between the predicted shape in simulation and the observed distribution in data is presented for the DY (upper left), $ {\mathrm{t}\overline{\mathrm{t}}} $ (upper right), WZ (lower left), and ZZ (lower right) control regions after performing a B-only fit simultaneously across all CRs. With the exception of DY CR, where by definition a hard cut at $ p_{\mathrm{T}}^\text{miss} = $ 140 GeV exists, in all other cases the last bin includes the overflow. The lower panel shows the post-fit values and uncertainties of the ratio between the observed data and the predicted SM background. The various background processes are represented with color-filled histograms, with the color as indicated in the legend for each case. The data points are shown as black dots with vertical and horizontal error bars, in this case comprising the total collected data in Run 2. |
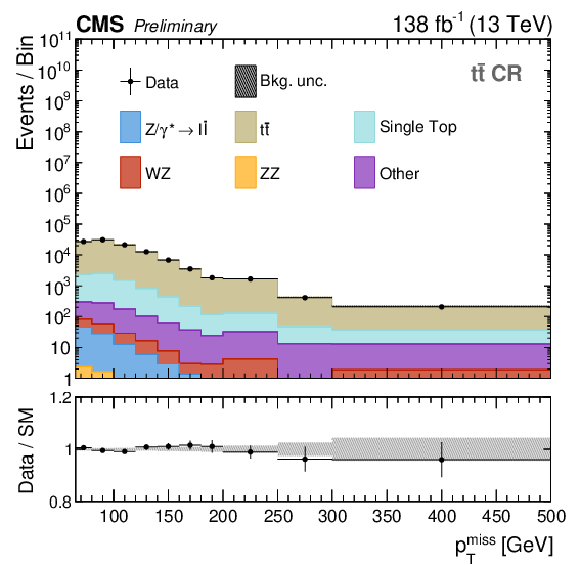
png pdf |
Figure 3-b:
The distribution of the effective missing transverse momentum ($ p_{\mathrm{T}}^\text{miss} $) computed for each process as described in the text is shown for each CR defined. A comparison between the predicted shape in simulation and the observed distribution in data is presented for the DY (upper left), $ {\mathrm{t}\overline{\mathrm{t}}} $ (upper right), WZ (lower left), and ZZ (lower right) control regions after performing a B-only fit simultaneously across all CRs. With the exception of DY CR, where by definition a hard cut at $ p_{\mathrm{T}}^\text{miss} = $ 140 GeV exists, in all other cases the last bin includes the overflow. The lower panel shows the post-fit values and uncertainties of the ratio between the observed data and the predicted SM background. The various background processes are represented with color-filled histograms, with the color as indicated in the legend for each case. The data points are shown as black dots with vertical and horizontal error bars, in this case comprising the total collected data in Run 2. |
png pdf |
Figure 3-c:
The distribution of the effective missing transverse momentum ($ p_{\mathrm{T}}^\text{miss} $) computed for each process as described in the text is shown for each CR defined. A comparison between the predicted shape in simulation and the observed distribution in data is presented for the DY (upper left), $ {\mathrm{t}\overline{\mathrm{t}}} $ (upper right), WZ (lower left), and ZZ (lower right) control regions after performing a B-only fit simultaneously across all CRs. With the exception of DY CR, where by definition a hard cut at $ p_{\mathrm{T}}^\text{miss} = $ 140 GeV exists, in all other cases the last bin includes the overflow. The lower panel shows the post-fit values and uncertainties of the ratio between the observed data and the predicted SM background. The various background processes are represented with color-filled histograms, with the color as indicated in the legend for each case. The data points are shown as black dots with vertical and horizontal error bars, in this case comprising the total collected data in Run 2. |
png pdf |
Figure 3-d:
The distribution of the effective missing transverse momentum ($ p_{\mathrm{T}}^\text{miss} $) computed for each process as described in the text is shown for each CR defined. A comparison between the predicted shape in simulation and the observed distribution in data is presented for the DY (upper left), $ {\mathrm{t}\overline{\mathrm{t}}} $ (upper right), WZ (lower left), and ZZ (lower right) control regions after performing a B-only fit simultaneously across all CRs. With the exception of DY CR, where by definition a hard cut at $ p_{\mathrm{T}}^\text{miss} = $ 140 GeV exists, in all other cases the last bin includes the overflow. The lower panel shows the post-fit values and uncertainties of the ratio between the observed data and the predicted SM background. The various background processes are represented with color-filled histograms, with the color as indicated in the legend for each case. The data points are shown as black dots with vertical and horizontal error bars, in this case comprising the total collected data in Run 2. |
png pdf |
Figure 4:
The distribution of the MLP4 discriminator is shown for each CR defined. A comparison between the simulation prediction and the observed distribution in data is presented for the DY (upper left), $ {\mathrm{t}\overline{\mathrm{t}}} $ (upper right), WZ (lower left), and ZZ (lower right) control regions after performing a B-only fit simultaneously across all CRs. The X-axis labels indicate the range of bins from the intended SR definition (Section 5.3) that have been merged into a single bin for a given CR due to statistical considerations. The lower panel shows the post-fit values and uncertainties of the ratio between the observed data and the predicted SM background. The various background processes are represented with color-filled histograms, with the color as indicated in the legend for each case. The data points are shown as black dots with vertical and horizontal error bars, in this case comprising the total collected data in Run 2. |
png pdf |
Figure 4-a:
The distribution of the MLP4 discriminator is shown for each CR defined. A comparison between the simulation prediction and the observed distribution in data is presented for the DY (upper left), $ {\mathrm{t}\overline{\mathrm{t}}} $ (upper right), WZ (lower left), and ZZ (lower right) control regions after performing a B-only fit simultaneously across all CRs. The X-axis labels indicate the range of bins from the intended SR definition (Section 5.3) that have been merged into a single bin for a given CR due to statistical considerations. The lower panel shows the post-fit values and uncertainties of the ratio between the observed data and the predicted SM background. The various background processes are represented with color-filled histograms, with the color as indicated in the legend for each case. The data points are shown as black dots with vertical and horizontal error bars, in this case comprising the total collected data in Run 2. |
png pdf |
Figure 4-b:
The distribution of the MLP4 discriminator is shown for each CR defined. A comparison between the simulation prediction and the observed distribution in data is presented for the DY (upper left), $ {\mathrm{t}\overline{\mathrm{t}}} $ (upper right), WZ (lower left), and ZZ (lower right) control regions after performing a B-only fit simultaneously across all CRs. The X-axis labels indicate the range of bins from the intended SR definition (Section 5.3) that have been merged into a single bin for a given CR due to statistical considerations. The lower panel shows the post-fit values and uncertainties of the ratio between the observed data and the predicted SM background. The various background processes are represented with color-filled histograms, with the color as indicated in the legend for each case. The data points are shown as black dots with vertical and horizontal error bars, in this case comprising the total collected data in Run 2. |
png pdf |
Figure 4-c:
The distribution of the MLP4 discriminator is shown for each CR defined. A comparison between the simulation prediction and the observed distribution in data is presented for the DY (upper left), $ {\mathrm{t}\overline{\mathrm{t}}} $ (upper right), WZ (lower left), and ZZ (lower right) control regions after performing a B-only fit simultaneously across all CRs. The X-axis labels indicate the range of bins from the intended SR definition (Section 5.3) that have been merged into a single bin for a given CR due to statistical considerations. The lower panel shows the post-fit values and uncertainties of the ratio between the observed data and the predicted SM background. The various background processes are represented with color-filled histograms, with the color as indicated in the legend for each case. The data points are shown as black dots with vertical and horizontal error bars, in this case comprising the total collected data in Run 2. |
png pdf |
Figure 4-d:
The distribution of the MLP4 discriminator is shown for each CR defined. A comparison between the simulation prediction and the observed distribution in data is presented for the DY (upper left), $ {\mathrm{t}\overline{\mathrm{t}}} $ (upper right), WZ (lower left), and ZZ (lower right) control regions after performing a B-only fit simultaneously across all CRs. The X-axis labels indicate the range of bins from the intended SR definition (Section 5.3) that have been merged into a single bin for a given CR due to statistical considerations. The lower panel shows the post-fit values and uncertainties of the ratio between the observed data and the predicted SM background. The various background processes are represented with color-filled histograms, with the color as indicated in the legend for each case. The data points are shown as black dots with vertical and horizontal error bars, in this case comprising the total collected data in Run 2. |
png pdf |
Figure 5:
Main statistical discriminant of the analysis used to extract the signal. The left side of the upper panel (divided by a vertical greyish dotted line) shows the four CRs defined to estimate the normalization of the main background processes entering the signal region, whereas the right side of the upper panel shows the full MLP spectrum (seventeen bins) in the SR used to ultimately discriminate between signal and background. The lower panel shows the post-fit values and uncertainties of the ratio between the observed data and the predicted SM background. The various background processes are represented with color-filled histograms, with the color as indicated in the legend for each case. The data points are shown as black dots with vertical and horizontal error bars, while the signal scenarios under consideration are represented with a dashed-dotted line. The benchmark signal cross section is set to 0.05 pb for proper visualization purposes. The figure comprises the full combination of all search channels and categories for the total collected data in Run 2. |
png pdf |
Figure 6:
Expected upper limits at 95% CL on the product of the cross section and branching fraction ($ \sigma \times B $) as specified in the text on the signal process here studied. The dependence of the limits on the pair $ (m_{\mathrm{H}},m_{\mathrm{a}}) $ has been accommodated into various 1D projections for a fixed value of $ m_{\mathrm{H}} $, where the corresponding limits have been scaled by an appropriate factor to easy the visualization of those. The y-axis contains the obtained cross section upper limit for the various combinations, whereas the x-axis exhibits the dependence on the mass of the pseudoscalar. The dashed line corresponds to the central expected limits, while the green and yellow bands indicate the regions that contain 68% and 95% of the distribution of the expected limits. |
png pdf |
Figure 7:
Excluded regions on the parameter phase space for the 2HDM+$ a $ model. The solid blue line encompasses the central expected excluded region for all the various projections, while the red-dotted lines represent the 95% CL coverage of the central contour. Projections are presented for the $ m_{\mathrm{H}} $-vs-$ m_{\mathrm{a}} $ plane (upper left), $ m_{\mathrm{H}} $-vs-$ \tan\beta $ plane (upper right), $ m_{\mathrm{a}} $-vs-$ \sin\theta $ plane (lower left), and $ \tan\beta $-vs-$ \sin\theta $ plane (lower right), for fixed values of the corresponding non-varied parameters as in Eq. 1. The olive green band represents the allowed phase-space values for each projection as estimated by assuming $ \langle \sigma v \rangle = $ (2-4) $\times$ 10$^{-26}$ cm$^{3}$/$s$, which covers a range around the central value required by the observed DM relic. The cases where this curve is not visible in the figures correspond to the scenario where the preferable values of $ \tan\beta $ for this range of $ \langle \sigma v \rangle $ fall beyond the threshold ($ \tan\beta > $ 25) depicted in the projections. |
png pdf |
Figure 7-a:
Excluded regions on the parameter phase space for the 2HDM+$ a $ model. The solid blue line encompasses the central expected excluded region for all the various projections, while the red-dotted lines represent the 95% CL coverage of the central contour. Projections are presented for the $ m_{\mathrm{H}} $-vs-$ m_{\mathrm{a}} $ plane (upper left), $ m_{\mathrm{H}} $-vs-$ \tan\beta $ plane (upper right), $ m_{\mathrm{a}} $-vs-$ \sin\theta $ plane (lower left), and $ \tan\beta $-vs-$ \sin\theta $ plane (lower right), for fixed values of the corresponding non-varied parameters as in Eq. 1. The olive green band represents the allowed phase-space values for each projection as estimated by assuming $ \langle \sigma v \rangle = $ (2-4) $\times$ 10$^{-26}$ cm$^{3}$/$s$, which covers a range around the central value required by the observed DM relic. The cases where this curve is not visible in the figures correspond to the scenario where the preferable values of $ \tan\beta $ for this range of $ \langle \sigma v \rangle $ fall beyond the threshold ($ \tan\beta > $ 25) depicted in the projections. |
png pdf |
Figure 7-b:
Excluded regions on the parameter phase space for the 2HDM+$ a $ model. The solid blue line encompasses the central expected excluded region for all the various projections, while the red-dotted lines represent the 95% CL coverage of the central contour. Projections are presented for the $ m_{\mathrm{H}} $-vs-$ m_{\mathrm{a}} $ plane (upper left), $ m_{\mathrm{H}} $-vs-$ \tan\beta $ plane (upper right), $ m_{\mathrm{a}} $-vs-$ \sin\theta $ plane (lower left), and $ \tan\beta $-vs-$ \sin\theta $ plane (lower right), for fixed values of the corresponding non-varied parameters as in Eq. 1. The olive green band represents the allowed phase-space values for each projection as estimated by assuming $ \langle \sigma v \rangle = $ (2-4) $\times$ 10$^{-26}$ cm$^{3}$/$s$, which covers a range around the central value required by the observed DM relic. The cases where this curve is not visible in the figures correspond to the scenario where the preferable values of $ \tan\beta $ for this range of $ \langle \sigma v \rangle $ fall beyond the threshold ($ \tan\beta > $ 25) depicted in the projections. |
png pdf |
Figure 7-c:
Excluded regions on the parameter phase space for the 2HDM+$ a $ model. The solid blue line encompasses the central expected excluded region for all the various projections, while the red-dotted lines represent the 95% CL coverage of the central contour. Projections are presented for the $ m_{\mathrm{H}} $-vs-$ m_{\mathrm{a}} $ plane (upper left), $ m_{\mathrm{H}} $-vs-$ \tan\beta $ plane (upper right), $ m_{\mathrm{a}} $-vs-$ \sin\theta $ plane (lower left), and $ \tan\beta $-vs-$ \sin\theta $ plane (lower right), for fixed values of the corresponding non-varied parameters as in Eq. 1. The olive green band represents the allowed phase-space values for each projection as estimated by assuming $ \langle \sigma v \rangle = $ (2-4) $\times$ 10$^{-26}$ cm$^{3}$/$s$, which covers a range around the central value required by the observed DM relic. The cases where this curve is not visible in the figures correspond to the scenario where the preferable values of $ \tan\beta $ for this range of $ \langle \sigma v \rangle $ fall beyond the threshold ($ \tan\beta > $ 25) depicted in the projections. |
png pdf |
Figure 7-d:
Excluded regions on the parameter phase space for the 2HDM+$ a $ model. The solid blue line encompasses the central expected excluded region for all the various projections, while the red-dotted lines represent the 95% CL coverage of the central contour. Projections are presented for the $ m_{\mathrm{H}} $-vs-$ m_{\mathrm{a}} $ plane (upper left), $ m_{\mathrm{H}} $-vs-$ \tan\beta $ plane (upper right), $ m_{\mathrm{a}} $-vs-$ \sin\theta $ plane (lower left), and $ \tan\beta $-vs-$ \sin\theta $ plane (lower right), for fixed values of the corresponding non-varied parameters as in Eq. 1. The olive green band represents the allowed phase-space values for each projection as estimated by assuming $ \langle \sigma v \rangle = $ (2-4) $\times$ 10$^{-26}$ cm$^{3}$/$s$, which covers a range around the central value required by the observed DM relic. The cases where this curve is not visible in the figures correspond to the scenario where the preferable values of $ \tan\beta $ for this range of $ \langle \sigma v \rangle $ fall beyond the threshold ($ \tan\beta > $ 25) depicted in the projections. |
| Summary |
| The first dedicated DM search performed in the $ \mathrm{b}\overline{\mathrm{b}}\mathrm{H} \rightarrow \mathrm{Z}\mathrm{a} $ channel is presented. A data set of proton-proton collisions at a center-of-mass energy of 13 TeV, corresponding to an integrated luminosity of 138 fb$ ^{-1} $ is analyzed. The analysis is relevant in the context of non-minimal DM models such as the 2HDM+$ a $, where the mediator can couple to other scalar states in the sector and can be produced in decays of those. The presence of b jets makes this search suitable to probe those scenarios in which the production of scalar in association with b-quarks is enhanced. In the context of the 2HDM+$ a $, those scenarios are favored by cosmological observations, which could indicate a large amount of DM particles being annihilated through an s-channel process producing a substantial number of b-quarks. The analysis exploits the Z boson decay into a pair of muons or electrons, thus benefiting from a clean signature and high efficiency in the reconstruction of the main physics object and of the event triggering. A powerful statistical discriminator between the varied signal topologies and the predominant backgrounds is deployed by making use of sophisticated machine learning techniques. The multivariate classifier is trained to reach a high level of discrimination across a broad range of kinematic variations that arise from the different configurations in which the Z boson and the DM mediator are produced. In order to better estimate the normalization of the main SM processes entering the selection and thus contributing to the background events, a set of dedicated CRs has been devised. These CRs are fitted simultaneously with the SR, which allows, on top of correcting the normalization of the key backgrounds, to properly correlate and constrain the dominant systematic uncertainties. Aside from estimating the normalization using a data-driven approach, also the shape of the largest background is corrected using a subsidiary measurement to improve the modeling of one of the key variables. Moreover, state-of-the-art theoretical calculations are also included in the shape modeling of the second-largest background by making use of event-by-event reweighing techniques. The results are presented in terms of model-independent limits on the signal process cross section, assuming the validity of the narrow width approximation, and as constraints on the parameter phase space of the specific 2HDM+$ a $ case. Exclusion regions in the 2D planes formed by four relevant 2HDM+$ a $ parameters are shown, while at the same time, the results are compared with expectations for this model in the context of cosmological predictions. |
| References | ||||
| 1 | L. Roszkowski, E. M. Sessolo, and S. Trojanowski | WIMP dark matter candidates and searches\textemdashcurrent status and future prospects | Rept. Prog. Phys. 81 (2018) 066201 | 1707.06277 |
| 2 | P. Tunney, J. M. No, and M. Fairbairn | Probing the pseudoscalar portal to dark matter via $ \bar bbZ(\to\ell\ell)+ \not{E}_T $: From the LHC to the Galactic Center excess | PRD 96 (2017) 095020 | 1705.09670 |
| 3 | G. Bertone, D. Hooper, and J. Silk | Particle dark matter: Evidence, candidates and constraints | Phys. Rept. 405 (2005) 279 | hep-ph/0404175 |
| 4 | D. E. Morrissey, T. Plehn, and T. M. P. Tait | Physics searches at the LHC | Phys. Rept. 515 (2012) 1 | 0912.3259 |
| 5 | Fermi-LAT Collaboration | Fermi-LAT Observations of High-Energy $ \gamma $-Ray Emission Toward the Galactic Center | Astrophys. J. 819 (2016) 44 | 1511.02938 |
| 6 | L. Goodenough and D. Hooper | Possible Evidence For Dark Matter Annihilation In The Inner Milky Way From The Fermi Gamma Ray Space Telescope | 0910.2998 | |
| 7 | D. Hooper and L. Goodenough | Dark Matter Annihilation in The Galactic Center As Seen by the Fermi Gamma Ray Space Telescope | PLB 697 (2011) 412 | 1010.2752 |
| 8 | D. Hooper and T. Linden | On The Origin Of The Gamma Rays From The Galactic Center | PRD 84 (2011) 123005 | 1110.0006 |
| 9 | K. N. Abazajian and M. Kaplinghat | Detection of a Gamma-Ray Source in the Galactic Center Consistent with Extended Emission from Dark Matter Annihilation and Concentrated Astrophysical Emission | PRD 86 (2012) 083511 | 1207.6047 |
| 10 | C. Karwin et al. | Dark Matter Interpretation of the Fermi-LAT Observation Toward the Galactic Center | PRD 95 (2017) 103005 | 1612.05687 |
| 11 | MAGIC, Fermi-LAT Collaboration | Limits to Dark Matter Annihilation Cross-Section from a Combined Analysis of MAGIC and Fermi-LAT Observations of Dwarf Satellite Galaxies | JCAP 02 (2016) 039 | 1601.06590 |
| 12 | C. Boehm et al. | Extended gamma-ray emission from Coy Dark Matter | JCAP 05 (2014) 009 | 1401.6458 |
| 13 | E. Izaguirre, G. Krnjaic, and B. Shuve | The Galactic Center Excess from the Bottom Up | PRD 90 (2014) 055002 | 1404.2018 |
| 14 | S. Ipek, D. McKeen, and A. E. Nelson | A Renormalizable Model for the Galactic Center Gamma Ray Excess from Dark Matter Annihilation | PRD 90 (2014) 055021 | 1404.3716 |
| 15 | ATLAS Collaboration | Search for new phenomena in events with an energetic jet and missing transverse momentum in $ pp $ collisions at $ \sqrt {s} $ =13 TeV with the ATLAS detector | PRD 103 (2021) 112006 | 2102.10874 |
| 16 | CMS Collaboration | Search for new particles in events with energetic jets and large missing transverse momentum in proton-proton collisions at $ \sqrt{s} $ = 13 TeV | JHEP 11 (2021) 153 | CMS-EXO-20-004 2107.13021 |
| 17 | ATLAS Collaboration | Search for dark matter in association with an energetic photon in $ pp $ collisions at $ \sqrt{s} $ = 13 TeV with the ATLAS detector | JHEP 02 (2021) 226 | 2011.05259 |
| 18 | CMS Collaboration | Search for new physics in final states with a single photon and missing transverse momentum in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JHEP 02 (2019) 074 | CMS-EXO-16-053 1810.00196 |
| 19 | ATLAS Collaboration | Search for associated production of a $ Z $ boson with an invisibly decaying Higgs boson or dark matter candidates at $ \sqrt s $ =13 TeV with the ATLAS detector | PLB 829 (2022) 137066 | 2111.08372 |
| 20 | CMS Collaboration | Search for dark matter produced in association with a leptonically decaying Z boson in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | EPJC 81 (2021) 13 | CMS-EXO-19-003 2008.04735 |
| 21 | ATLAS Collaboration | Search for dark matter produced in association with a Standard Model Higgs boson decaying into b-quarks using the full Run 2 dataset from the ATLAS detector | JHEP 11 (2021) 209 | 2108.13391 |
| 22 | ATLAS Collaboration | Search for dark matter in events with missing transverse momentum and a Higgs boson decaying into two photons in pp collisions at $ \sqrt{s} $ = 13 TeV with the ATLAS detector | JHEP 10 (2021) 013 | 2104.13240 |
| 23 | CMS Collaboration | Search for dark matter particles produced in association with a Higgs boson in proton-proton collisions at $ \sqrt{\mathrm{s}} $ = 13 TeV | JHEP 03 (2020) 025 | CMS-EXO-18-011 1908.01713 |
| 24 | CMS Collaboration | Search for dark matter produced in association with a Higgs boson decaying to a pair of bottom quarks in proton-proton collisions at $ \sqrt{s}=13\,\text {Te}\text {V} $ | EPJC 79 (2019) 280 | CMS-EXO-16-050 1811.06562 |
| 25 | CMS Collaboration | Search for dark matter produced in association with a Higgs boson decaying to $ \gamma\gamma $ or $ \tau^+\tau^- $ at $ \sqrt{s} = $ 13 TeV | JHEP 09 (2018) 046 | CMS-EXO-16-055 1806.04771 |
| 26 | ATLAS Collaboration | Search for dark matter produced in association with a dark Higgs boson decaying into $ W^{+}W^{-} $ in the one-lepton final state at $ \sqrt{s} $=13 TeV using 139 fb$ ^{-1} $ of $ pp $ collisions recorded with the ATLAS detector | JHEP 07 (2023) 116 | 2211.07175 |
| 27 | ATLAS Collaboration | Search for Dark Matter Produced in Association with a Dark Higgs Boson Decaying into $ W^\pm W^\mp $ or $ ZZ $ in Fully Hadronic Final States from $ \sqrt{s}= $ 13 TeV pp Collisions Recorded with the ATLAS Detector | PRL 126 (2021) 121802 | 2010.06548 |
| 28 | ATLAS Collaboration | Search for dark matter produced in association with a single top quark and an energetic $ W $ boson in $ \sqrt{s}= $ 13 TeV $ pp $ collisions with the ATLAS detector | EPJC 83 (2023) 603 | 2211.13138 |
| 29 | ATLAS Collaboration | Search for dark matter produced in association with a single top quark in $ \sqrt{s}= $ 13 TeV $ pp $ collisions with the ATLAS detector | EPJC 81 (2021) 860 | 2011.09308 |
| 30 | CMS Collaboration | Search for dark matter particles produced in association with a top quark pair at $ \sqrt{s} = $ 13 TeV | PRL 122 (2019) 011803 | CMS-EXO-16-049 1807.06522 |
| 31 | CMS Collaboration | Search for dark matter produced in association with a single top quark or a top quark pair in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JHEP 03 (2019) 141 | CMS-EXO-18-010 1901.01553 |
| 32 | CMS Collaboration | Measurement of the $ B^0_s \to \mu^+ \mu^- $ Branching Fraction and Search for $ B^0 \to \mu^+ \mu^- $ with the CMS Experiment | PRL 111 (2013) 101804 | CMS-BPH-13-004 1307.5025 |
| 33 | CMS Collaboration | Searches for additional Higgs bosons and for vector leptoquarks in $ \tau\tau $ final states in proton-proton collisions at $ \sqrt{s} $ = 13 TeV | JHEP 07 (2023) 073 | CMS-HIG-21-001 2208.02717 |
| 34 | Y. Nomura and J. Thaler | Dark Matter through the Axion Portal | PRD 79 (2009) 075008 | 0810.5397 |
| 35 | D. Goncalves, P. A. N. Machado, and J. M. No | Simplified Models for Dark Matter Face their Consistent Completions | PRD 95 (2017) 055027 | 1611.04593 |
| 36 | J. M. No | Looking through the pseudoscalar portal into dark matter: Novel mono-Higgs and mono-Z signatures at the LHC | PRD 93 (2016) 031701 | 1509.01110 |
| 37 | M. Bauer, U. Haisch, and F. Kahlhoefer | Simplified dark matter models with two Higgs doublets: I. Pseudoscalar mediators | JHEP 05 (2017) 138 | 1701.07427 |
| 38 | G. C. Branco et al. | Theory and phenomenology of two-Higgs-doublet models | Phys. Rept. 516 (2012) 1 | 1106.0034 |
| 39 | LHC Dark Matter Working Group Collaboration | LHC Dark Matter Working Group: Next-generation spin-0 dark matter models | Phys. Dark Univ. 27 (2020) 100351 | 1810.09420 |
| 40 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | |
| 41 | CMS Collaboration | Performance of the CMS Level-1 trigger in proton-proton collisions at $ \sqrt{s} = $ 13\,TeV | JINST 15 (2020) P10017 | CMS-TRG-17-001 2006.10165 |
| 42 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 43 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 44 | R. Frederix and S. Frixione | Merging meets matching in MC@NLO | JHEP 12 (2012) 061 | 1209.6215 |
| 45 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with Parton Shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 46 | S. Alioli, P. Nason, C. Oleari, and E. Re | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX | JHEP 06 (2010) 043 | 1002.2581 |
| 47 | M. Czakon et al. | Top-pair production at the LHC through NNLO QCD and NLO EW | JHEP 10 (2017) 186 | 1705.04105 |
| 48 | M. Grazzini, S. Kallweit, and M. Wiesemann | Fully differential NNLO computations with MATRIX | EPJC 78 (2018) 537 | 1711.06631 |
| 49 | CMS Collaboration | Measurement of the inclusive and differential WZ production cross sections, polarization angles, and triple gauge couplings in pp collisions at $ \sqrt{s} $ = 13 TeV | JHEP 07 (2022) 032 | CMS-SMP-20-014 2110.11231 |
| 50 | NNPDF Collaboration | Parton distributions from high-precision collider data | EPJC 77 (2017) 663 | 1706.00428 |
| 51 | T. Sjostrand, S. Mrenna, and P. Z. Skands | A Brief Introduction to PYTHIA 8.1 | Comput. Phys. Commun. 178 (2008) 852 | 0710.3820 |
| 52 | CMS Collaboration | Event generator tunes obtained from underlying event and multiparton scattering measurements | EPJC 76 (2016) 155 | CMS-GEN-14-001 1512.00815 |
| 53 | GEANT4 Collaboration | GEANT4--a simulation toolkit | NIM A 506 (2003) 250 | |
| 54 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 55 | D. Contardo et al. | Technical Proposal for the Phase-II Upgrade of the CMS Detector | technical report, 2015 link |
|
| 56 | W. Adam, R. Fr \" u hwirth, A. Strandlie, and T. Todor | Reconstruction of Electrons with the Gaussian-Sum Filter in the CMS Tracker at the LHC | technical report, CERN, 2005 | |
| 57 | CMS Collaboration | Performance of CMS Muon Reconstruction in $ pp $ Collision Events at $ \sqrt{s}= $ 7 TeV | JINST 7 (2012) P10002 | CMS-MUO-10-004 1206.4071 |
| 58 | CMS Collaboration | Performance of the CMS muon detector and muon reconstruction with proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JINST 13 (2018) P06015 | CMS-MUO-16-001 1804.04528 |
| 59 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_t $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 60 | L. Sonnenschein | Analytical solution of ttbar dilepton equations | PRD 73 (2006) 054015 | hep-ph/0603011 |
| 61 | L. Sonnenschein | Analytical solution of ttbar dilepton equations | PRD 73 (2006) 054015 | hep-ph/0603011 |
| 62 | Particle Data Group Collaboration | Review of Particle Physics | PTEP 2022 (2022) 083C01 | |
| 63 | A. Paszke et al. | Pytorch: An imperative style, high-performance deep learning library | in Advances in Neural Information Processing Systems 32 link |
|
| 64 | C. G. Lester and D. J. Summers | Measuring masses of semiinvisibly decaying particles pair produced at hadron colliders | PLB 463 (1999) 99 | hep-ph/9906349 |
| 65 | C. G. Lester and B. Nachman | Bisection-based asymmetric M$ _{T2} $ computation: a higher precision calculator than existing symmetric methods | JHEP 03 (2015) 100 | 1411.4312 |
| 66 | D.-A. Clevert, T. Unterthiner, and S. Hochreiter | Fast and accurate deep network learning by exponential linear units (elus) | ||
| 67 | D. P. Kingma and J. Ba | Adam: A method for stochastic optimization | ||
| 68 | K. H. Zou, A. J. O'Malley, and L. Mauri | Receiver-operating characteristic analysis for evaluating diagnostic tests and predictive models | CIRCULATIONAHA link |
|
| 69 | J. Butterworth et al. | PDF4LHC recommendations for LHC Run II | JPG 43 (2016) 023001 | 1510.03865 |
| 70 | A. Accardi et al. | A Critical Appraisal and Evaluation of Modern PDFs | EPJC 76 (2016) 471 | 1603.08906 |
| 71 | R. Barlow and C. Beeston | Fitting using finite monte carlo samples | Computer Physics Communications 77 (1993) 219 | |
| 72 | CMS Collaboration | Precision luminosity measurement in proton-proton collisions at $ \sqrt{s} = $ 13 TeV in 2015 and 2016 at CMS | EPJC 81 (2021) 800 | CMS-LUM-17-003 2104.01927 |
| 73 | CMS Collaboration | CMS luminosity measurement for the 2017 data-taking period at $ \sqrt{s} = $ 13 TeV | technical report, CERN, 2018 CDS |
|
| 74 | CMS Collaboration | CMS luminosity measurement for the 2018 data-taking period at $ \sqrt{s} = $ 13 TeV | technical report, CERN, Geneva, 2019 CDS |
|
| 75 | CMS Collaboration | Electron and photon reconstruction and identification with the CMS experiment at the CERN LHC | JINST 16 (2021) P05014 | CMS-EGM-17-001 2012.06888 |
| 76 | CMS Collaboration | Measurement of the $ \mathrm{t}\overline{\mathrm{t}} $ production cross section, the top quark mass, and the strong coupling constant using dilepton events in pp collisions at $ \sqrt{s} = $ 13 TeV | EPJC 79 (2019) 368 | CMS-TOP-17-001 1812.10505 |
| 77 | CMS Collaboration | Measurement of the inelastic proton-proton cross section at $ \sqrt{s}= $ 13 TeV | JHEP 07 (2018) 161 | CMS-FSQ-15-005 1802.02613 |
| 78 | CMS Collaboration | Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV | JINST 13 (2018) P05011 | CMS-BTV-16-002 1712.07158 |
| 79 | CMS Collaboration | The CMS Statistical Analysis and Combination Tool: COMBINE | CMS-CAT-23-001 2404.06614 |
|
| 80 | J. Alwall et al. | Computing decay rates for new physics theories with FeynRules and MadGraph 5\_aMC@NLO | Comput. Phys. Commun. 197 (2015) 312 | 1402.1178 |
| 81 | M. Backovic, K. Kong, and M. McCaskey | MadDM v.1.0: Computation of Dark Matter Relic Abundance Using MadGraph5 | Physics of the Dark Universe, 2014 link |
1308.4955 |
| 82 | Planck Collaboration | Planck 2015 results. XIII. Cosmological parameters | Astron. Astrophys. 594 (2016) A13 | 1502.01589 |
| 83 | G. Steigman, B. Dasgupta, and J. F. Beacom | Precise Relic WIMP Abundance and its Impact on Searches for Dark Matter Annihilation | PRD 86 (2012) 023506 | 1204.3622 |
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|