Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-PAS-HIN-21-007 | ||
| Observation of the $\Upsilon$(3S) meson and sequential suppression of $\Upsilon$ states in PbPb collisions at $\sqrt{\smash[b]{s_{_{\mathrm{NN}}}}}= $ 5.02 TeV | ||
| CMS Collaboration | ||
| April 2022 | ||
| Abstract: The production of $\Upsilon$(2S) and $\Upsilon$(3S) mesons in lead-lead (PbPb) and proton-proton (pp) collisions is studied using the CMS detector at the LHC. Their nuclear modification factors, $R_{\textrm{AA}}$, derived from the ratio of yields measured in PbPb to pp collisions, are reported as functions of transverse momentum and PbPb collision centrality. The $\Upsilon$(3S) meson is observed for the first time in PbPb collisions. The suppression is found to be stronger for $\Upsilon$(3S) mesons compared to $\Upsilon$(2S) mesons, extending the pattern of sequential suppression of bottomonium states in nuclear collisions previously seen for the $\Upsilon$(1S) and $\Upsilon$(2S) states. The results provide new constraints on models describing the dynamics of quarkonium states in heavy ion collisions. | ||
|
Links:
CDS record (PDF) ;
CADI line (restricted) ;
These preliminary results are superseded in this paper, PRL 133 (2024) 022302. The superseded preliminary plots can be found here. |
||
| Figures & Tables | Summary | Additional Figures | References | CMS Publications |
|---|
| Figures | |
png pdf |
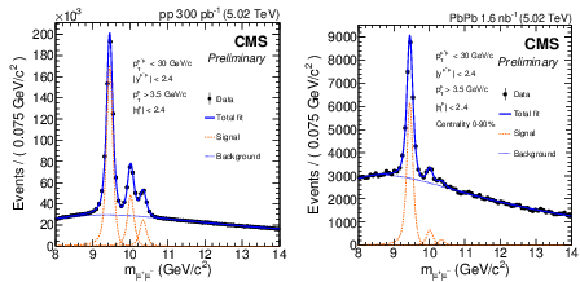
Figure 1:
Invariant mass distributions of selected dimuons in pp (left) and PbPb (right) collisions, for the kinematic range $ {p_{\mathrm {T}}} ^{\mu^{+} \mu^{-}} < $ 30 GeV/$c$ and $ {| y^{\mu^{+} \mu^{-}} |} < $ 2.4. The solid lines in both figures show the results of the fits to data, while the dashed and dotted lines represent the separate yields for each $\Upsilon$ state and background dimuons, respectively. |
png pdf |
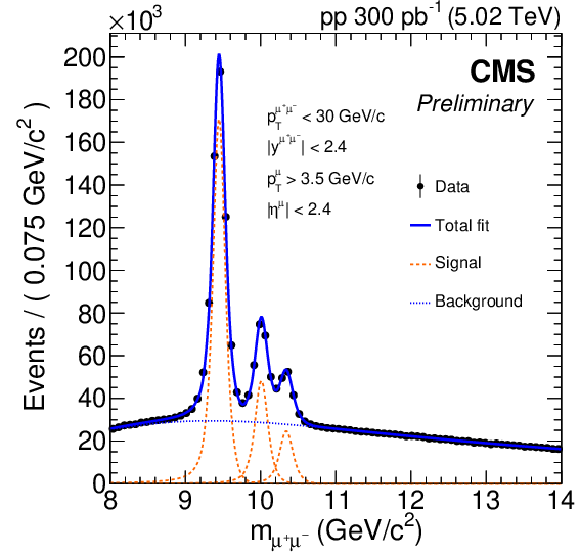
Figure 1-a:
Invariant mass distributions of selected dimuons in pp (left) and PbPb (right) collisions, for the kinematic range $ {p_{\mathrm {T}}} ^{\mu^{+} \mu^{-}} < $ 30 GeV/$c$ and $ {| y^{\mu^{+} \mu^{-}} |} < $ 2.4. The solid lines in both figures show the results of the fits to data, while the dashed and dotted lines represent the separate yields for each $\Upsilon$ state and background dimuons, respectively. |
png pdf |
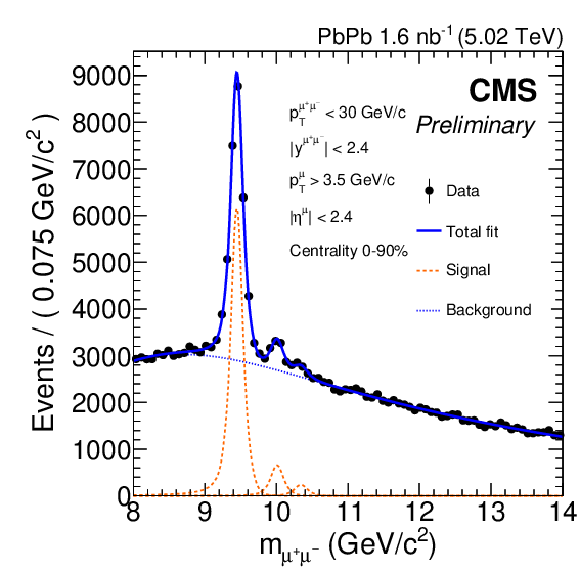
Figure 1-b:
Invariant mass distributions of selected dimuons in pp (left) and PbPb (right) collisions, for the kinematic range $ {p_{\mathrm {T}}} ^{\mu^{+} \mu^{-}} < $ 30 GeV/$c$ and $ {| y^{\mu^{+} \mu^{-}} |} < $ 2.4. The solid lines in both figures show the results of the fits to data, while the dashed and dotted lines represent the separate yields for each $\Upsilon$ state and background dimuons, respectively. |
png pdf |
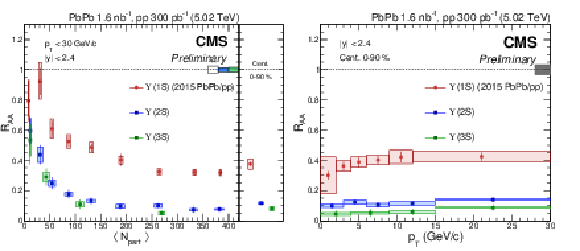
Figure 2:
Measured ${R_ {\text {AA}}}$ for $\Upsilon$ states as functions of $\langle {N_{\text {part}}} \rangle $ (left) including the centrality integrated bin and ${p_{\mathrm {T}}}$ (right). The vertical lines correspond to statistical uncertainties, while the boxes represent the systematic uncertainties. In the left figure, the left-most box at unity combines the uncertainties of pp luminosity and PbPb ${N_{\mathrm {MB}}}$, while the second (third) box corresponds to the uncertainty of pp yields for the $\Upsilon$(2S) ($\Upsilon$(3S)) state. The box at unity in the right plot combines the uncertainties of ${T_{{\text {AA}}}}$, pp luminosity, and PbPb ${N_{\mathrm {MB}}}$. The results for the $\Upsilon$(1S) meson are taken from Ref. [18] and are not affected by the boxes at unity. |
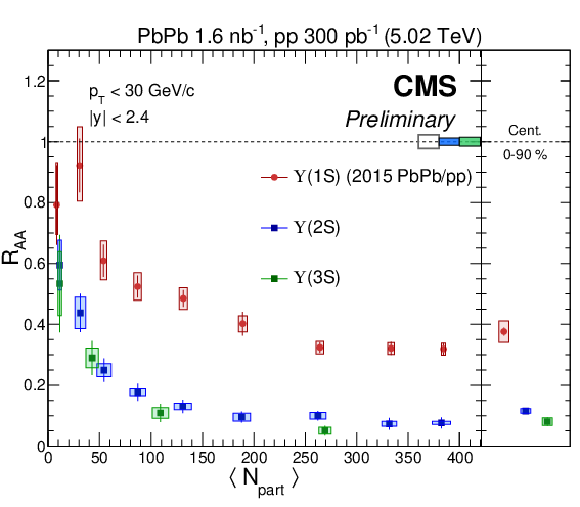
png pdf |
Figure 2-a:
Measured ${R_ {\text {AA}}}$ for $\Upsilon$ states as functions of $\langle {N_{\text {part}}} \rangle $ (left) including the centrality integrated bin and ${p_{\mathrm {T}}}$ (right). The vertical lines correspond to statistical uncertainties, while the boxes represent the systematic uncertainties. In the left figure, the left-most box at unity combines the uncertainties of pp luminosity and PbPb ${N_{\mathrm {MB}}}$, while the second (third) box corresponds to the uncertainty of pp yields for the $\Upsilon$(2S) ($\Upsilon$(3S)) state. The box at unity in the right plot combines the uncertainties of ${T_{{\text {AA}}}}$, pp luminosity, and PbPb ${N_{\mathrm {MB}}}$. The results for the $\Upsilon$(1S) meson are taken from Ref. [18] and are not affected by the boxes at unity. |
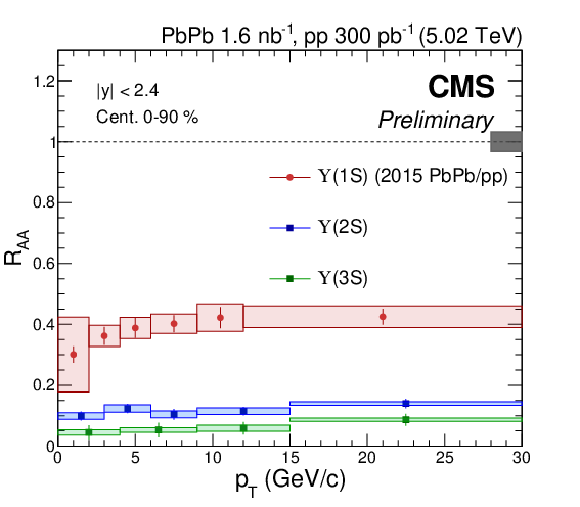
png pdf |
Figure 2-b:
Measured ${R_ {\text {AA}}}$ for $\Upsilon$ states as functions of $\langle {N_{\text {part}}} \rangle $ (left) including the centrality integrated bin and ${p_{\mathrm {T}}}$ (right). The vertical lines correspond to statistical uncertainties, while the boxes represent the systematic uncertainties. In the left figure, the left-most box at unity combines the uncertainties of pp luminosity and PbPb ${N_{\mathrm {MB}}}$, while the second (third) box corresponds to the uncertainty of pp yields for the $\Upsilon$(2S) ($\Upsilon$(3S)) state. The box at unity in the right plot combines the uncertainties of ${T_{{\text {AA}}}}$, pp luminosity, and PbPb ${N_{\mathrm {MB}}}$. The results for the $\Upsilon$(1S) meson are taken from Ref. [18] and are not affected by the boxes at unity. |
png pdf |
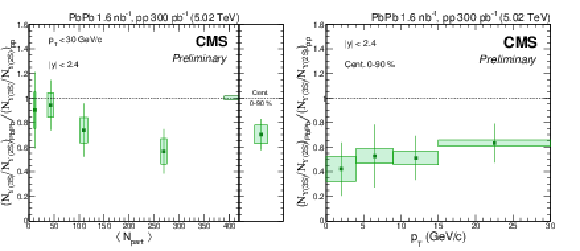
Figure 3:
The double ratio of $\Upsilon$(3S)/$\Upsilon$(2S) as functions of $\langle {N_{\text {part}}} \rangle $ (left) and ${p_{\mathrm {T}}}$ (right). The vertical lines correspond to statistical uncertainties, while the boxes are the systematic uncertainties. The box at unity on the left figure shows the combined systematic and statistical uncertainties from pp data, which is common to all of the points. |
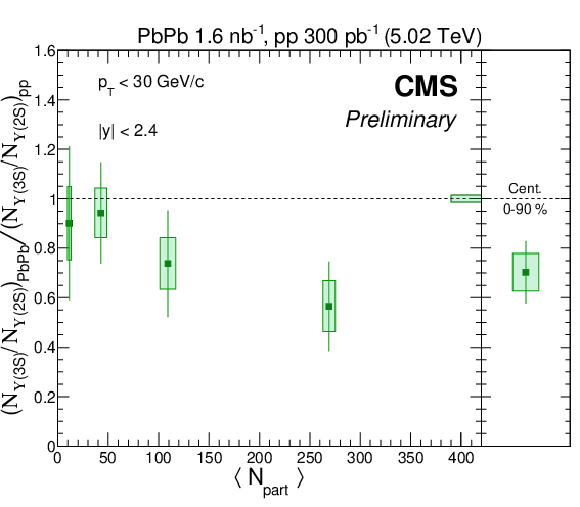
png pdf |
Figure 3-a:
The double ratio of $\Upsilon$(3S)/$\Upsilon$(2S) as functions of $\langle {N_{\text {part}}} \rangle $ (left) and ${p_{\mathrm {T}}}$ (right). The vertical lines correspond to statistical uncertainties, while the boxes are the systematic uncertainties. The box at unity on the left figure shows the combined systematic and statistical uncertainties from pp data, which is common to all of the points. |
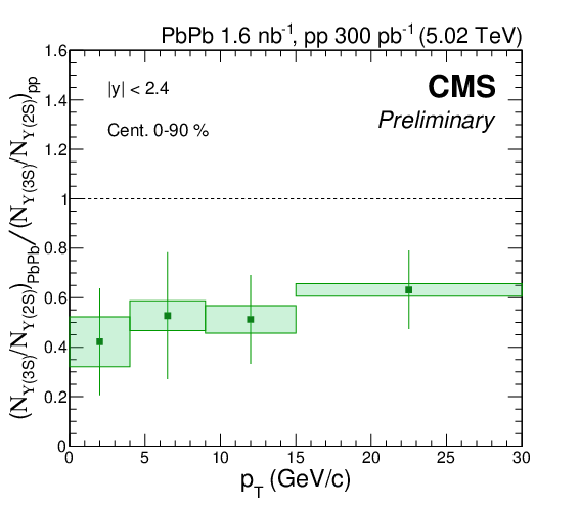
png pdf |
Figure 3-b:
The double ratio of $\Upsilon$(3S)/$\Upsilon$(2S) as functions of $\langle {N_{\text {part}}} \rangle $ (left) and ${p_{\mathrm {T}}}$ (right). The vertical lines correspond to statistical uncertainties, while the boxes are the systematic uncertainties. The box at unity on the left figure shows the combined systematic and statistical uncertainties from pp data, which is common to all of the points. |
| Tables | |
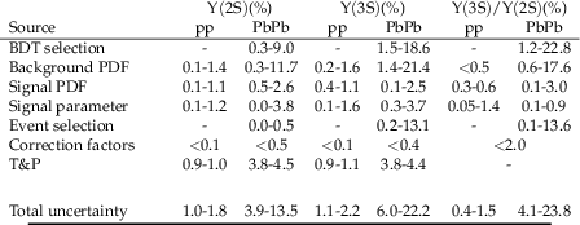
png pdf |
Table 1:
Systematic uncertainties from various sources in pp and PbPb collisions listed in percentage. The global uncertainties are not included in the total uncertainties. |
| Summary |
| In summary, data from lead-lead (PbPb) and proton-proton (pp) collisions at a nucleon-nucleon center-of-mass energy of $\sqrt{\smash[b]{s_{_{\mathrm{NN}}}}} = $ 5.02 TeV collected with the CMS detector were analyzed to measure the nuclear modification factors ($R_{\textrm{AA}}$) of $\Upsilon$(2S) and $\Upsilon$(3S) mesons, and double ratios $\Upsilon$(3S)/$\Upsilon$(2S). The values of the observables are given as functions of $\Upsilon$ transverse momentum ($p_{\mathrm{T}}$) and PbPb collision centrality. The $\Upsilon$(3S) meson is observed for the first time in PbPb collisions, and the amount of suppression is found to be stronger than for the $\Upsilon$(2S) meson. The values of $R_{\textrm{AA}}$ for both $\Upsilon$(2S) and $\Upsilon$(3S) are observed to decrease gradually for more central collisions. On the other hand, the $R_{\textrm{AA}}$ values suggest at most a small increase with increasing $p_{\mathrm{T}}$ for the $\Upsilon$(3S) meson. The double ratios are found to be smaller than unity, showing a stronger suppression for the $\Upsilon$(3S) meson compared to the $\Upsilon$(2S) meson. No significant $p_{\mathrm{T}}$ dependence is observed for the double ratios $\Upsilon$(3S)/$\Upsilon$(2S), indicating the relative modification between the two states to be similar in the studied $p_{\mathrm{T}}$ region. Combined with previous measurements, the results in this note reveal the sequential suppression of $\Upsilon$(1S), $\Upsilon$(2S), and $\Upsilon$(3S) mesons, which provides new constraints on the understanding of quarkonium suppression in heavy ion collisions. |
| Additional Figures | |
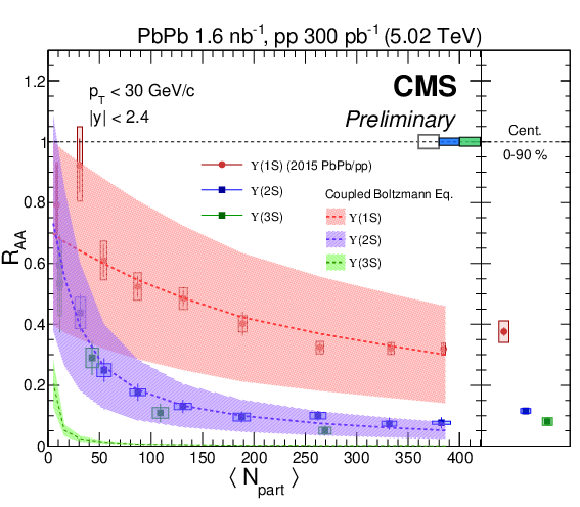
png pdf |
Additional Figure 1:
Nuclear modification factors for the $\Upsilon$(1S), $\Upsilon$(2S), and $\Upsilon$(3S) mesons as a function of $\langle {N_{\text {part}}} \rangle $, including the centrality integrated bin. The vertical lines correspond to statistical uncertainties, while the boxes represent the systematic uncertainties. The left-most box at unity combines the uncertainties of pp luminosity and PbPb ${N_{\mathrm {MB}}}$, while the second (third) box corresponds to the uncertainty of pp yields for the $\Upsilon$(2S) ($\Upsilon$(3S)) state. Results for the $\Upsilon$(1S) meson are taken from Ref. [18]. The bands represent calculations from Ref. [7]. |
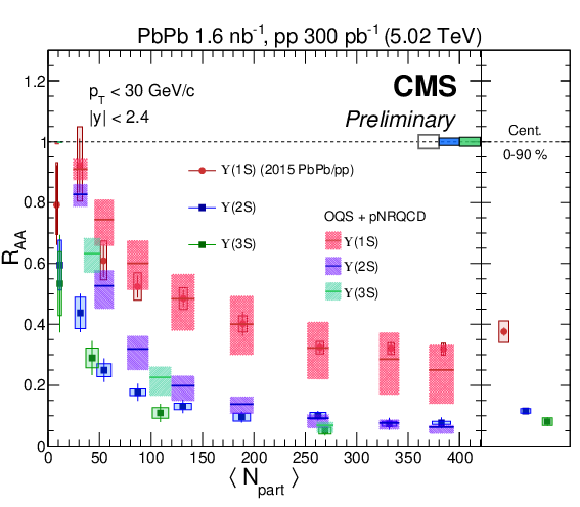
png pdf |
Additional Figure 2:
Nuclear modification factors for the $\Upsilon$(1S), $\Upsilon$(2S), and $\Upsilon$(3S) mesons as a function of $\langle {N_{\text {part}}} \rangle $, including the centrality integrated bin. The vertical lines correspond to statistical uncertainties, while the boxes represent the systematic uncertainties. The left-most box at unity combines the uncertainties of pp luminosity and PbPb ${N_{\mathrm {MB}}}$, while the second (third) box corresponds to the uncertainty of pp yields for the $\Upsilon$(2S) ($\Upsilon$(3S)) state. Results for the $\Upsilon$(1S) meson are taken from Ref. [18]. The bands represent calculations from Ref. [6]. |
png pdf |
Additional Figure 3:
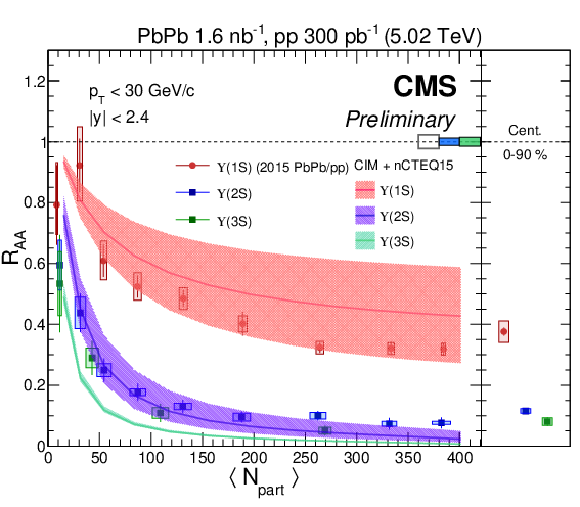
Nuclear modification factors for the $\Upsilon$(1S), $\Upsilon$(2S), and $\Upsilon$(3S) mesons as a function of $\langle {N_{\text {part}}} \rangle $, including the centrality integrated bin. The vertical lines correspond to statistical uncertainties, while the boxes represent the systematic uncertainties. The left-most box at unity combines the uncertainties of pp luminosity and PbPb ${N_{\mathrm {MB}}}$, while the second (third) box corresponds to the uncertainty of pp yields for the $\Upsilon$(2S) ($\Upsilon$(3S)) state. Results for the $\Upsilon$(1S) meson are taken from Ref. [18]. The bands represent calculations from Ref. [50]. |
png pdf |
Additional Figure 4:
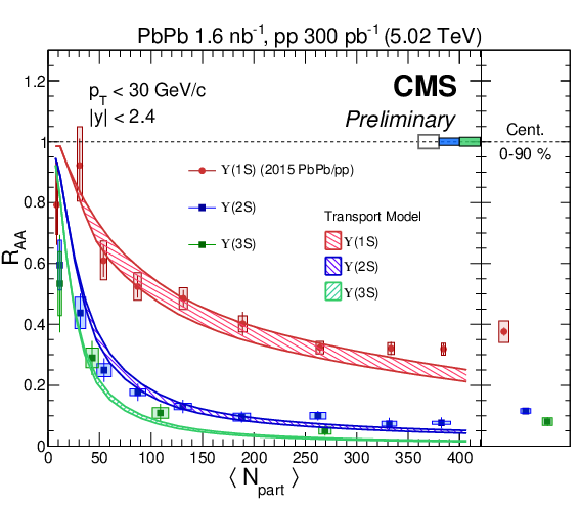
Nuclear modification factors for the $\Upsilon$(1S), $\Upsilon$(2S), and $\Upsilon$(3S) mesons as a function of $\langle {N_{\text {part}}} \rangle $, including the centrality integrated bin. The vertical lines correspond to statistical uncertainties, while the boxes represent the systematic uncertainties. The left-most box at unity combines the uncertainties of pp luminosity and PbPb ${N_{\mathrm {MB}}}$, while the second (third) box corresponds to the uncertainty of pp yields for the $\Upsilon$(2S) ($\Upsilon$(3S) state. Results for the $\Upsilon$(1S) meson are taken from Ref. [18]. The bands represent calculations from Ref. [10]. |
png pdf |
Additional Figure 5:
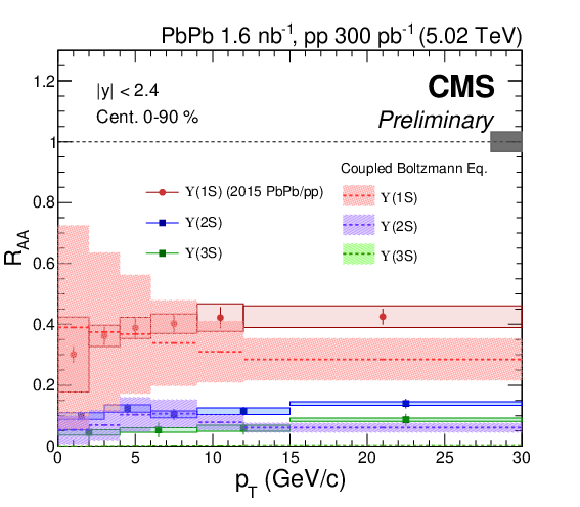
Nuclear modification factors for the $\Upsilon$(1S), $\Upsilon$(2S), and $\Upsilon$(3S) mesons as a function of ${p_{\mathrm {T}}}$. The vertical lines correspond to statistical uncertainties, while the boxes represent the systematic uncertainties. The box at unity represents the global uncertainty, which combines uncertainties from ${T_{{\text {AA}}}}$, pp luminosity, and PbPb ${N_{\mathrm {MB}}}$. Results for the $\Upsilon$(1S) meson are taken from Ref. [18]. The bands represent calculations from Ref. [7]. |
png pdf |
Additional Figure 6:
Nuclear modification factors for the $\Upsilon$(1S), $\Upsilon$(2S), and $\Upsilon$(3S) mesons as a function of ${p_{\mathrm {T}}}$. The vertical lines correspond to statistical uncertainties, while the boxes represent the systematic uncertainties. The box at unity represents the global uncertainty, which combines uncertainties from ${T_{{\text {AA}}}}$, pp luminosity, and PbPb ${N_{\mathrm {MB}}}$. Results for the $\Upsilon$(1S) meson are taken from Ref. [18]. The bands represent calculations from Ref. [6]. |
png pdf |
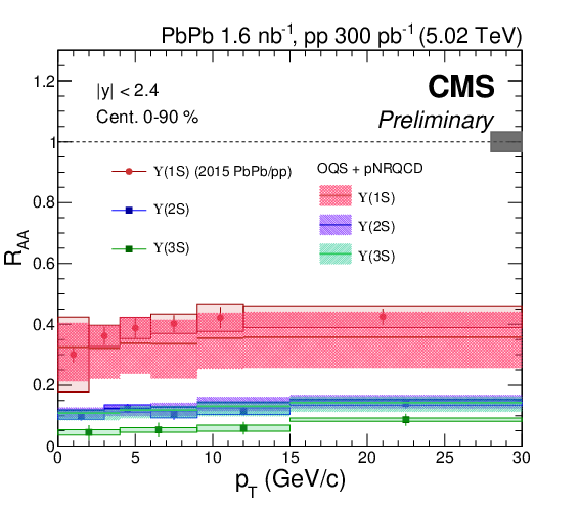
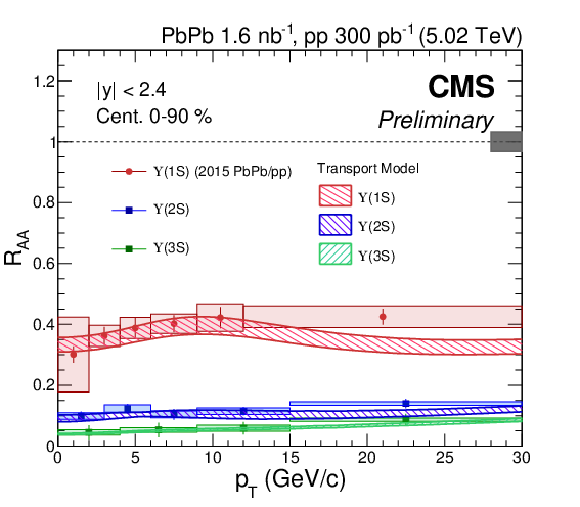
Additional Figure 7:
Nuclear modification factors for the $\Upsilon$(1S), $\Upsilon$(2S), and $\Upsilon$(3S) mesons as a function of ${p_{\mathrm {T}}}$. The vertical lines correspond to statistical uncertainties, while the boxes represent the systematic uncertainties. The box at unity represents the global uncertainty, which combines uncertainties from ${T_{{\text {AA}}}}$, pp luminosity, and PbPb ${N_{\mathrm {MB}}}$. Results for the $\Upsilon$(1S) meson are taken from Ref. [18]. The bands represent calculations from Ref. [10]. |
png pdf |
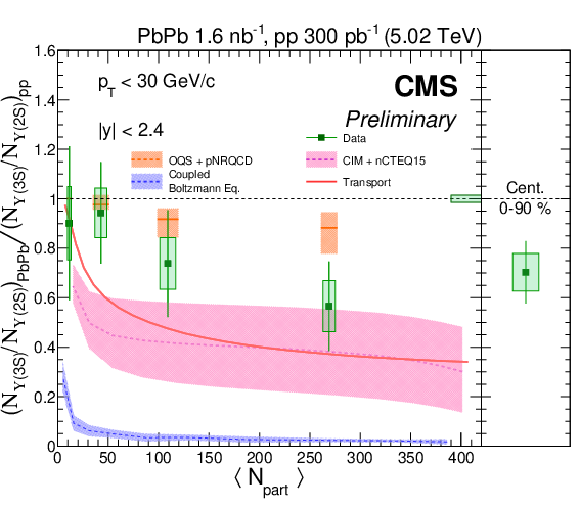
Additional Figure 8:
The double ratio of $\Upsilon$(3S) /$\Upsilon$(2S) as functions of $\langle {N_{\text {part}}} \rangle $. The vertical lines correspond to statistical uncertainties, while the boxes are the systematic uncertainties. The box at unity shows the combined systematic and statistical uncertainties from pp data. The three different types of bands represent calculations from Ref. [7,6,50,10]. |
png pdf |
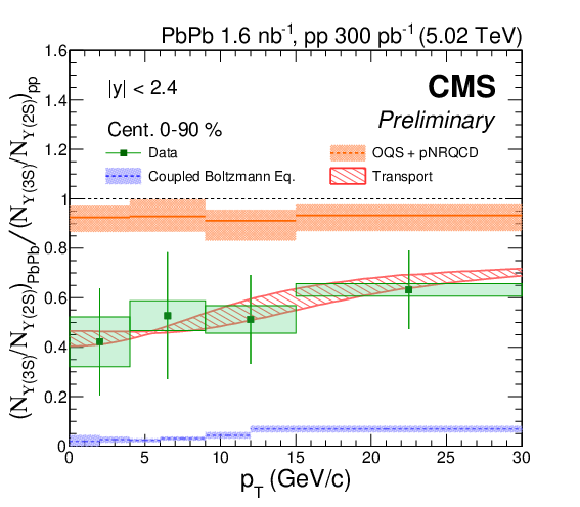
Additional Figure 9:
The double ratio of $\Upsilon$(3S) /$\Upsilon$(2S) as functions of ${p_{\mathrm {T}}}$. The vertical lines correspond to statistical uncertainties, while the boxes are the systematic uncertainties. The two bands represent calculations from Ref. [7,6,10]. |
png pdf |
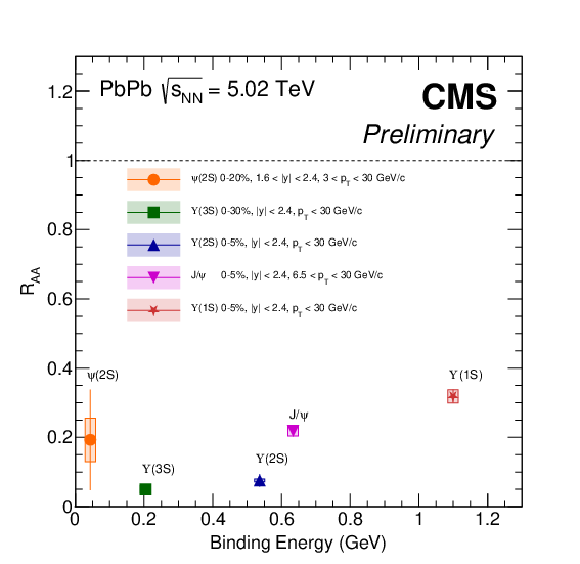
Additional Figure 10:
The nuclear modification factors for various quarkonium mesons as a function of quarkonium binding energy at $\sqrt{\smash[b]{s_{_{\mathrm{NN}}}}}=$ 5.02 TeV. The values for the binding energy of each quarkonium state are taken from Ref. [5]. The error bars and boxes represent the statistical and systematic uncertainties, respectively. The results for the $\Upsilon$(1S) meson and charmonium states (J/$\psi$ and $\psi$(2S)) are taken from Ref. [18] and Ref. [51], respectively. |
png pdf |
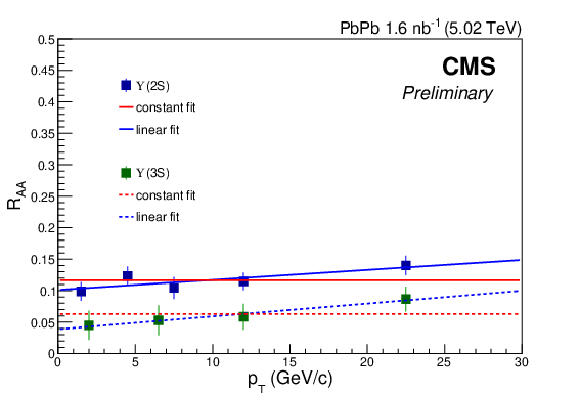
Additional Figure 11:
The nuclear modification factors for the $\Upsilon$(2S) and $\Upsilon$(3S) mesons as a function of ${p_{\mathrm {T}}}$. The vertical lines correspond to statistical uncertainties, while the boxes represent the systematic uncertainties. The solid (dashed) lines are the results of the fit to the ${R_ {\text {AA}}}$ values for the $\Upsilon$(2S) ($\Upsilon$(3S)) state using a constant and a linear function. A F-test [52] is performed to examine the significance of the dependence of the two functions. The significance for the preference of the linear fit is found to be more than 3 standard deviations for the $\Upsilon$(3S) meson and less than 2 standard deviations for the $\Upsilon$(2S) meson, thus verifying an increasing trend of the ${R_ {\text {AA}}}$ of $\Upsilon$(3S) with increasing ${p_{\mathrm {T}}}$. |
| References | ||||
| 1 | T. Matsui and H. Satz | J/$\psi$ suppression by quark gluon plasma formation | PLB 178 (1986) 416 | |
| 2 | M. Laine, O. Philipsen, P. Romatschke, and M. Tassler | Real-time static potential in hot QCD | JHEP 03 (2007) 054 | hep-ph/0611300 |
| 3 | N. Brambilla, J. Ghiglieri, A. Vairo, and P. Petreczky | Static quark antiquark pairs at finite temperature | PRD 78 (2008) 014017 | 0804.0993 |
| 4 | N. Brambilla et al. | Heavy quarkonium in a weakly coupled quark-gluon plasma below the melting temperature | JHEP 09 (2010) 038 | 1007.4156 |
| 5 | J.-P. Blaizot, D. De Boni, P. Faccioli, and G. Garberoglio | Heavy quark bound states in a quark-gluon plasma: Dissociation and recombination | NP A 946 (2016) 49 | 1503.03857 |
| 6 | N. Brambilla et al. | Bottomonium production in heavy-ion collisions using quantum trajectories: Differential observables and momentum anisotropy | PRD 104 (2021) 094049 | 2107.06222 |
| 7 | X. Yao et al. | Coupled Boltzmann transport equations of heavy quarks and quarkonia in quark-gluon plasma | JHEP 01 (2021) 046 | 2004.06746 |
| 8 | N. Brambilla, M. A. Escobedo, J. Soto, and A. Vairo | Quarkonium suppression in heavy ion collisions: an open quantum system approach | PRD 96 (2017) 034021 | 1612.07248 |
| 9 | A. Emerick, X. Zhao, and R. Rapp | Bottomonia in the quark gluon plasma and their production at RHIC and LHC | EPJA 48 (2012) 72 | 1111.6537 |
| 10 | X. Du, R. Rapp, and M. He | Color screening and regeneration of bottomonia in high-energy heavy-ion collisions | PRC 96 (2017) 054901 | 1706.08670 |
| 11 | STAR Collaboration | Suppression of $ \Upsilon $ production in dAu and AuAu collisions at $ \sqrt{\smash[b]{s_{_{\mathrm{NN}}}}} = $ 200 GeV | PLB 735 (2014) 127 | 1312.3675 |
| 12 | A. Andronic et al. | Heavy flavour and quarkonium production in the LHC era: from proton-proton to heavy ion collisions | EPJC 76 (2016) 107 | 1506.03981 |
| 13 | ALICE Collaboration | Studies of J/$\psi$ production at forward rapidity in PbPb collisions at $ \sqrt{\smash[b]{s_{_{\mathrm{NN}}}}} = $ 5.02 TeV | JHEP 02 (2020) 041 | 1909.03158 |
| 14 | R. R. Xiaojian Du | Sequential Regeneration of Charmonia in Heavy-Ion Collisions | Nuclear Physics A 943 (2015) 147 | 1504.00670 |
| 15 | Anton Andronic, Peter Braun-Munzinger, Markus K. Kohler, Krzysztof Redlich, Johanna Stachel | Transverse momentum distributions of charmonium states with the statistical hadronization model | PLB 797 (2019) 134836 | 1901.09200 |
| 16 | ALICE Collaboration | $ \Upsilon $ suppression at forward rapidity in PbPb collisions at $ \sqrt{\smash[b]{s_{_{\mathrm{NN}}}}} = $ 5.02 TeV | PLB 790 (2019) 89 | 1805.04387 |
| 17 | ALICE Collaboration | $ \Upsilon $ production and nuclear modification at forward rapidity in Pb-Pb collisions at $ \sqrt{\smash[b]{s_{_{\mathrm{NN}}}}} = $ 5.02 TeV | PLB 822 (2021) 136579 | 2011.05758 |
| 18 | CMS Collaboration | Measurement of nuclear modification factors of $\Upsilon$(1S), $\Upsilon$(2S), and $\Upsilon$(3S) mesons in PbPb collisions at $ \sqrt{\smash[b]{s_{_{\mathrm{NN}}}}} = $ 5.02 TeV | PLB 790 (2019) 270 | CMS-HIN-16-023 1805.09215 |
| 19 | M. L. Miller, K. Reygers, S. J. Sanders, and P. Steinberg | Glauber modeling in high energy nuclear collisions | Ann. Rev. Nucl. Part. Sci. 57 (2007) 205 | nucl-ex/0701025 |
| 20 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | CMS-00-001 |
| 21 | CMS Collaboration | Performance of the CMS Level-1 trigger in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JINST 15 (2020) P10017 | CMS-TRG-17-001 2006.10165 |
| 22 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 23 | CMS Collaboration | Performance of electron reconstruction and selection with the CMS detector in proton-proton collisions at $ \sqrt{s} = $ 8 TeV | JINST 10 (2015) P06005 | CMS-EGM-13-001 1502.02701 |
| 24 | CMS Collaboration | Performance of the CMS muon detector and muon reconstruction with proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JINST 13 (2018) P06015 | CMS-MUO-16-001 1804.04528 |
| 25 | CMS Collaboration | Performance of photon reconstruction and identification with the CMS detector in proton-proton collisions at sqrt(s) = 8 TeV | JINST 10 (2015) P08010 | CMS-EGM-14-001 1502.02702 |
| 26 | CMS Collaboration | Description and performance of track and primary-vertex reconstruction with the CMS tracker | JINST 9 (2014) P10009 | CMS-TRK-11-001 1405.6569 |
| 27 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 28 | CMS Collaboration | Performance of reconstruction and identification of $ \tau $ leptons decaying to hadrons and $ \nu_\tau $ in pp collisions at $ \sqrt{s}= $ 13 TeV | JINST 13 (2018), no. 10, P10005 | CMS-TAU-16-003 1809.02816 |
| 29 | CMS Collaboration | Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV | JINST 12 (2017) P02014 | CMS-JME-13-004 1607.03663 |
| 30 | CMS Collaboration | Performance of missing transverse momentum reconstruction in proton-proton collisions at $ \sqrt{s} = $ 13 TeV using the CMS detector | JINST 14 (2019) P07004 | CMS-JME-17-001 1903.06078 |
| 31 | CMS Collaboration | Meaurement of the azimuthal anisotropy of $\Upsilon$(1S) and $\Upsilon$(2S) mesons in PbPb collisions at $ \sqrt{\smash[b]{s_{_{\mathrm{NN}}}}} = $ 5.02 TeV | PLB 819 (2021) 136385 | CMS-HIN-19-002 2006.07707 |
| 32 | CMS Collaboration | Charged-particle nuclear modification factors in PbPb and pPb collisions at $ \sqrt{\smash[b]{s_{_{\mathrm{NN}}}}} = $ 5.02 TeV | JHEP 04 (2017) 039 | CMS-HIN-15-015 1611.01664 |
| 33 | CMS Collaboration | Transverse momentum and pseudorapidity distributions of charged hadrons in pp collisions at $ \sqrt{s} = $ 0.9 and 2.36 TeV | JHEP 02 (2010) 041 | CMS-QCD-09-010 1002.0621 |
| 34 | T. Sjostrand et al. | An introduction to PYTHIA 8.2 | CPC 191 (2015) 159 | 1410.3012 |
| 35 | CMS Collaboration | Extraction and validation of a new set of CMS PYTHIA-8 tunes from underlying event measurements | EPJC 80 (2020) 4 | CMS-GEN-17-001 1903.12179 |
| 36 | LHCb Collaboration | Measurement of the $ \Upsilon $ polarizations in pp collisions at $ \sqrt{s} = $ 7 and 8TeV | JHEP 12 (2017) 110 | 1709.01301 |
| 37 | CMS Collaboration | Measurement of the $\Upsilon$(1S), $\Upsilon$(2S), and $\Upsilon$(3S) polarizations in pp collisions at $ \sqrt{s} = $ 7 TeV | PRL 110 (2013) 081802 | CMS-BPH-11-023 1209.2922 |
| 38 | ALICE Collaboration | First measurement of quarkonium polarization in nuclear collisions at the LHC | PLB 815 (2021) 136146 | 2005.11128 |
| 39 | I. P. Lokhtin et al. | Heavy ion event generator HYDJET++ (hydrodynamics plus jets) | CPC 180 (2009) 779 | 0809.2708 |
| 40 | GEANT4 Collaboration | GEANT4--a simulation toolkit | NIMA 506 (2003) 250 | |
| 41 | H. Voss, A. Hocker, J. Stelzer, and F. Tegenfeldt | TMVA, the toolkit for multivariate data analysis with ROOT | in XIth International Workshop on Advanced Computing and Analysis Techniques in Physics Research (ACAT), p. 40 2007 [PoS(ACAT)040] | physics/0703039 |
| 42 | M. J. Oreglia | A study of the reactions $\psi' \to \gamma\gamma \psi$ | PhD thesis, Stanford University, 1980 SLAC Report SLAC-R-236, see Appendix D | |
| 43 | Particle Data Group Collaboration | Review of Particle Physics | PTEP 2020 (2020), no. 8, 083C01 | |
| 44 | H. Akaike | A new look at the statistical model identification | IEEE Transactions on Automatic Control 19 (1974) 716 | |
| 45 | M. S. Barlett | Tests of significance in factor analysis | British Journal of Statistical Psychology 3 (1950) 77 | |
| 46 | CMS Collaboration | Performance of the CMS muon trigger system in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JINST 16 (2021) P07001 | CMS-MUO-19-001 2102.04790 |
| 47 | CMS Collaboration | Luminosity measurement in proton-proton collisions at 5.02 TeV in 2017 at CMS | CMS-PAS-LUM-19-001 | CMS-PAS-LUM-19-001 |
| 48 | CMS Collaboration | Fragmentation of jets containing a prompt J/$\psi$ meson in PbPb and pp collisions at $ \sqrt{\smash[b]{s_{_{\mathrm{NN}}}}} = $ 5.02 TeV | PLB 825 (2022) 136842 | CMS-HIN-19-007 2106.13235 |
| 49 | C. Loizides, J. Kamin, and D. d'Enterria | Improved Monte Carlo Glauber predictions at present and future nuclear colliders | PRC 97 (2018) 054910 | 1710.07098 |
| 50 | E. Ferreiro, J.-P. Lansberg | Is bottomonium suppression in proton-nucleus and nucleus-nucleus collisions at LHC energies due to the same | JHEP 10 (2018) 094 | 1804.04474 |
| 51 | CMS Collaboration | Measurement of prompt and nonprompt charmonium suppression in PbPb collisions at 5.02 TeV | EPJC 87 (2018) 509 | 1712.08959 |
| 52 | A. Bacchi, V. S. Lamzin and K. S. Wilson | A self-validation technique for protein structure refinement: the extended Hamilton test | Acta Cryst. D52 (1996) 641 | |
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|