Compact Muon Solenoid
LHC, CERN

Visit us: CMS Public Website, CMS Physics ; Contact us: CMS Publications Committee

CMS-SUS-16-009 ; CERN-EP-2016-293

Search for supersymmetry in the all-hadronic final state using top quark tagging in pp collisions at $ \sqrt{s} = $ 13 TeV

CMS Collaboration

8 January 2017

Phys. Rev. D 96 (2017) 012004

Abstract: A search is presented for supersymmetry in all-hadronic events with missing transverse momentum based on tagging of top quarks. The data sample corresponds to an integrated luminosity of 2.3 fb$^{-1}$ of proton-proton collisions at a center-of-mass energy of 13 TeV, collected with the CMS detector at the LHC. Search regions are defined using the properties of reconstructed jets, the presence of bottom and top quark candidates, and an imbalance in transverse momentum. With no statistically significant excess of events observed beyond the expected contributions from the standard model, we set exclusion limits at 95% confidence level on the masses of new particles in the context of simplified models of direct and gluino-mediated top squark production. For direct top squark production with decays to a top quark and a neutralino, top squark masses up to 740 GeV and neutralino masses up to 240 GeV are excluded. Gluino masses up to 1550 GeV and neutralino masses up to 900 GeV are excluded for models of gluino pair production where each gluino decays to a top-antitop quark pair and a neutralino.

Links: e-print arXiv:1701.01954 [hep-ex] (PDF) ; CDS record ; inSPIRE record ; CADI line (restricted) ;

Additional technical material for CMS speakers can be found here.

Figures
png pdf	Figure 1: Diagrams representing the two simplified models of direct top squark pair production and decay considered in this study: the T2tt model with top squark decay via a top quark (left), and the T2tb model with the top squark decaying either via a top quark or via an intermediate chargino (right).
png pdf	Figure 1-a: Diagram representing one of the two simplified models of direct top squark pair production and decay considered in this study: the T2tt model with top squark decay via a top quark.
png pdf	Figure 1-b: Diagram representing one of the two simplified models of direct top squark pair production and decay considered in this study: the T2tb model with the top squark decaying either via a top quark or via an intermediate chargino.
png pdf	Figure 2: Diagrams representing the simplified models of gluino-mediated top squark production considered in this study: the T1tttt model (left) where the gluino decays to top quarks and the LSP via an off-shell top squark, and the T5ttcc model (right) where the gluino decays to an on-shell top squark, which decays to a charm quark and the LSP.
png pdf	Figure 2-a: Diagram representing a simplified model of gluino-mediated top squark production considered in this study: the T1tttt model, where the gluino decays to top quarks and the LSP via an off-shell top squark.
png pdf	Figure 2-b: Diagram representing a simplified model of gluino-mediated top squark production considered in this study: the T5ttcc model, where the gluino decays to an on-shell top squark, which decays to a charm quark and the LSP.
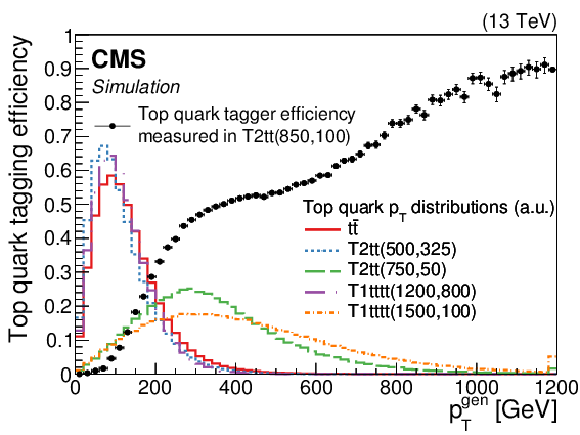
png pdf	Figure 3: The tagging efficiency of the top quark tagger as a function of the generator-level hadronically decaying top quark $ {p_{\mathrm {T}}} $ (black points). The efficiency was computed using the T2tt signal model with $m_{\tilde{ \mathrm{ t } } } = $ 850 GeV and $m_{\tilde{\chi}^0_1 } = $ 100 GeV, and it is similar for ${\mathrm{ t } \mathrm{ \bar{t} } } $ events. The vertical bars depict the statistical uncertainty. The colored lines show the expected hadronically decaying top quark $ {p_{\mathrm {T}}} $ distribution from ${\mathrm{ t } \mathrm{ \bar{t} } } $ (red solid line), the T2tt signal model with $m_{\tilde{ \mathrm{ t } } } = $ 500 GeV and $m_{\tilde{\chi}^0_1 } = $ 325 GeV (blue short-dashed line), the T2tt signal model with $m_{\tilde{ \mathrm{ t } } } = $ 750 GeV and $m_{\tilde{\chi}^0_1 } = $ 50 GeV (green long-dashed line), the T1tttt signal model with $m_{\tilde{ \mathrm{g} } } = $ 1200 GeV and $m_{\tilde{\chi}^0_1 } = $ 800 GeV (purple long-dash-dotted line), and the T1tttt signal model with $m_{\tilde{ \mathrm{g} } } = $ 1500 GeV and $m_{\tilde{\chi}^0_1 } = $ 100 GeV (orange short-dash-dotted line). The last bin contains the overflow entries and the top quark $ {p_{\mathrm {T}}} $ distributions are normalized to unit area.
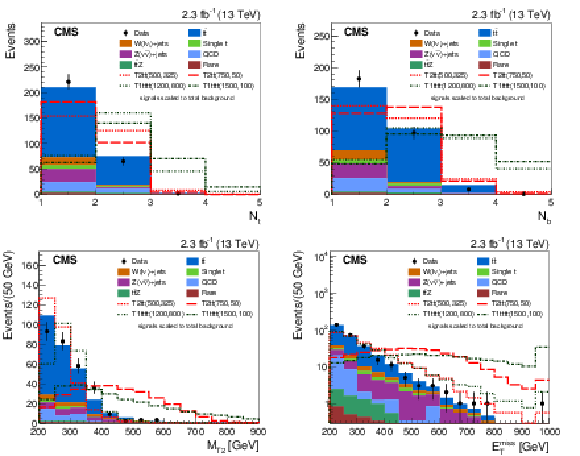
png pdf	Figure 4: Comparison of the distributions in data (black points), simulated SM backgrounds (filled stacked histograms) and several signal models in ${N_{\mathrm{ t } }}$ (top left), ${N_{\mathrm{ b } }}$ (top right), ${M_{\mathrm {T2}}}$ (bottom left), and ${E_{\mathrm {T}}^{\text {miss}}}$ (bottom right), after the preselection requirements have been applied. The T2tt signal model with $m_{\tilde{ \mathrm{ t } } } = $ 500 (750) GeV and $m_{\tilde{\chi}^0_1 } = $ 325 (50) GeV is shown with a red short-dashed (long-dashed) line, and the T1tttt signal model with $m_{\tilde{ \mathrm{g} } } = $ 1200 (1500) GeV and $m_{\tilde{\chi}^0_1 } = $ 800 (100) GeV with a dark green short-dash-dotted (long-dash-dotted) line. The distributions for the signal events have been normalized to the same area as the total background distribution, and the last bin contains the overflow events.
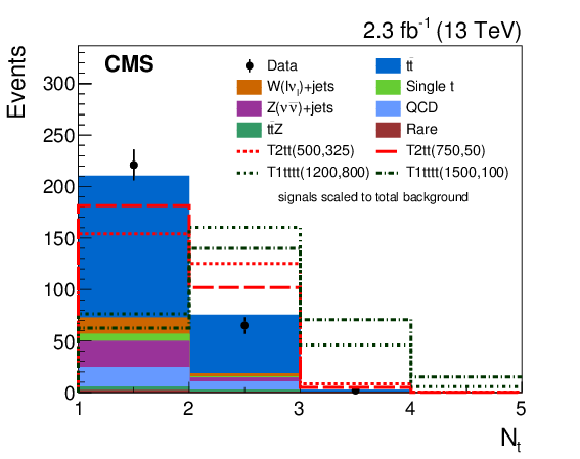
png pdf	Figure 4-a: Comparison of the distributions in data (black points), simulated SM backgrounds (filled stacked histograms) and several signal models in ${N_{\mathrm{ t } }}$, after the preselection requirements have been applied. The T2tt signal model with $m_{\tilde{ \mathrm{ t } } } = $ 500 (750) GeV and $m_{\tilde{\chi}^0_1 } = $ 325 (50) GeV is shown with a red short-dashed (long-dashed) line, and the T1tttt signal model with $m_{\tilde{ \mathrm{g} } } = $ 1200 (1500) GeV and $m_{\tilde{\chi}^0_1 } = $ 800 (100) GeV with a dark green short-dash-dotted (long-dash-dotted) line. The distribution for the signal events has been normalized to the same area as the total background distribution, and the last bin contains the overflow events.
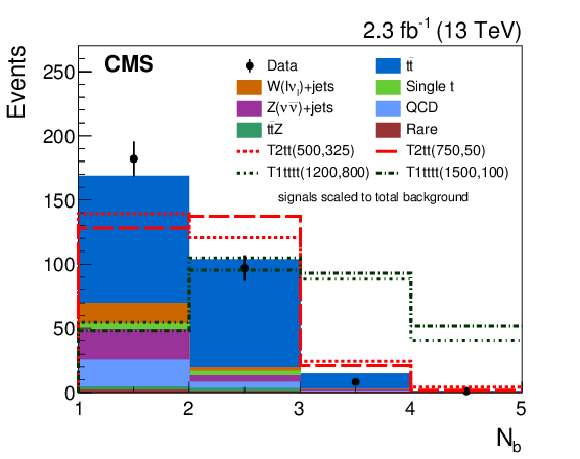
png pdf	Figure 4-b: Comparison of the distributions in data (black points), simulated SM backgrounds (filled stacked histograms) and several signal models in ${N_{\mathrm{ b } }}$, after the preselection requirements have been applied. The T2tt signal model with $m_{\tilde{ \mathrm{ t } } } = $ 500 (750) GeV and $m_{\tilde{\chi}^0_1 } = $ 325 (50) GeV is shown with a red short-dashed (long-dashed) line, and the T1tttt signal model with $m_{\tilde{ \mathrm{g} } } = $ 1200 (1500) GeV and $m_{\tilde{\chi}^0_1 } = $ 800 (100) GeV with a dark green short-dash-dotted (long-dash-dotted) line. The distribution for the signal events has been normalized to the same area as the total background distribution, and the last bin contains the overflow events.
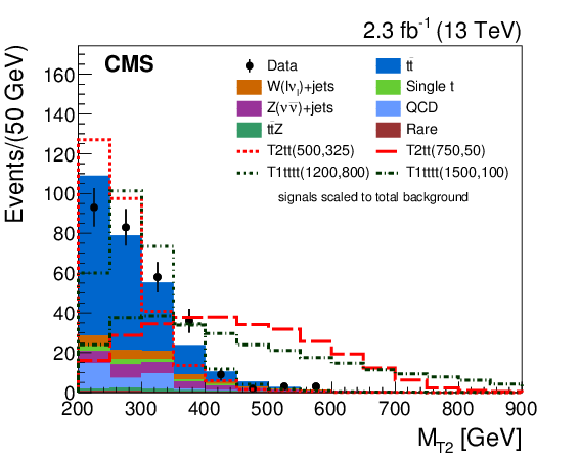
png pdf	Figure 4-c: Comparison of the distributions in data (black points), simulated SM backgrounds (filled stacked histograms) and several signal models in ${M_{\mathrm {T2}}}$, after the preselection requirements have been applied. The T2tt signal model with $m_{\tilde{ \mathrm{ t } } } = $ 500 (750) GeV and $m_{\tilde{\chi}^0_1 } = $ 325 (50) GeV is shown with a red short-dashed (long-dashed) line, and the T1tttt signal model with $m_{\tilde{ \mathrm{g} } } = $ 1200 (1500) GeV and $m_{\tilde{\chi}^0_1 } = $ 800 (100) GeV with a dark green short-dash-dotted (long-dash-dotted) line. The distribution for the signal events has been normalized to the same area as the total background distribution, and the last bin contains the overflow events.
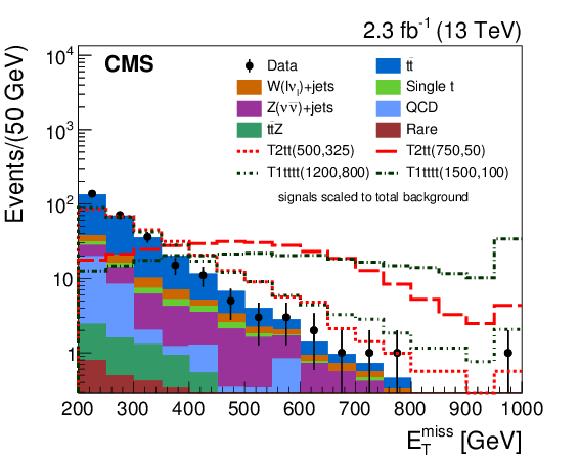
png pdf	Figure 4-d: Comparison of the distributions in data (black points), simulated SM backgrounds (filled stacked histograms) and several signal models in ${E_{\mathrm {T}}^{\text {miss}}}$, after the preselection requirements have been applied. The T2tt signal model with $m_{\tilde{ \mathrm{ t } } } = $ 500 (750) GeV and $m_{\tilde{\chi}^0_1 } = $ 325 (50) GeV is shown with a red short-dashed (long-dashed) line, and the T1tttt signal model with $m_{\tilde{ \mathrm{g} } } = $ 1200 (1500) GeV and $m_{\tilde{\chi}^0_1 } = $ 800 (100) GeV with a dark green short-dash-dotted (long-dash-dotted) line. The distribution for the signal events has been normalized to the same area as the total background distribution, and the last bin contains the overflow events.
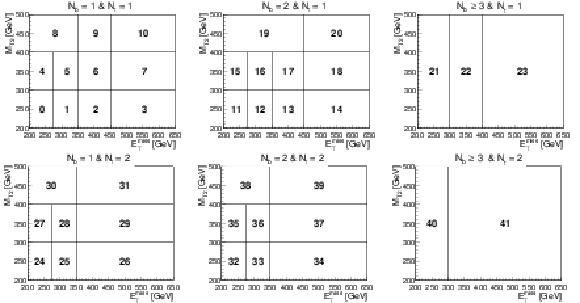
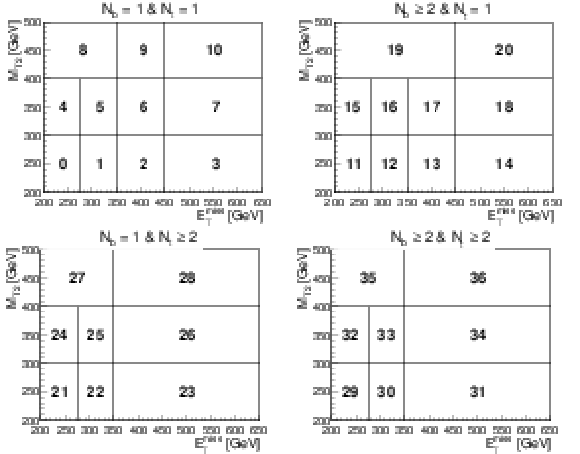
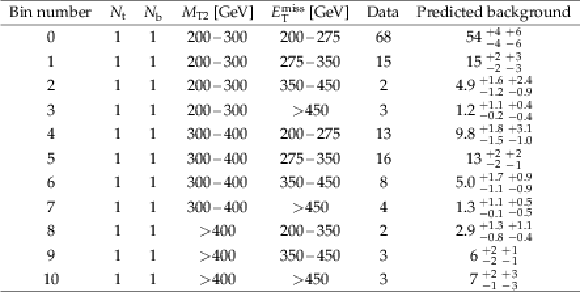
png pdf	Figure 5: Search region definitions for bin numbers 0-41 of the gluino-mediated production optimization, as defined in the text. The highest ${E_{\mathrm {T}}^{\text {miss}}}$ and ${M_{\mathrm {T2}}}$ bins are open-ended, e.g., bin 10 requires $ {E_{\mathrm {T}}^{\text {miss}}} > $ 450 GeV and $ {M_{\mathrm {T2}}} > $ 400 GeV. In addition to the search bins shown in this figure, there are three bins (42-44) with $ {N_{\mathrm{ t } }} \ge $ 3, one for each $ {N_{\mathrm{ b } }} $ bin, that contain no further binning in ${E_{\mathrm {T}}^{\text {miss}}}$ or ${M_{\mathrm {T2}}}$ beyond baseline selection requirements.
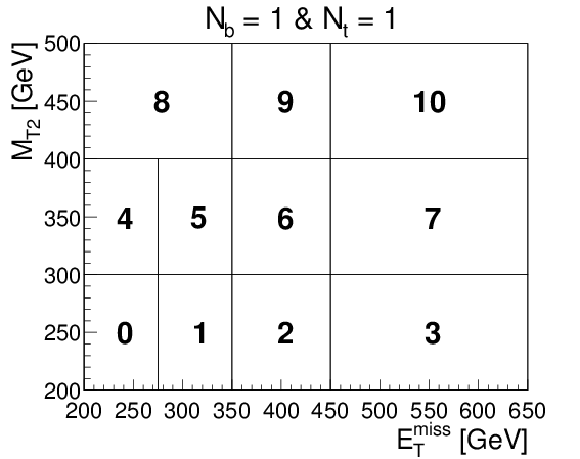
png pdf	Figure 5-a: Search region definitions for bin numbers 0-8 of the gluino-mediated production optimization, as defined in the text. The highest ${E_{\mathrm {T}}^{\text {miss}}}$ and ${M_{\mathrm {T2}}}$ bins are open-ended, e.g., bin 10 requires $ {E_{\mathrm {T}}^{\text {miss}}} > $ 450 GeV and $ {M_{\mathrm {T2}}} > $ 400 GeV.
png pdf	Figure 5-b: Search region definitions for bin numbers 11-20 of the gluino-mediated production optimization, as defined in the text. The highest ${E_{\mathrm {T}}^{\text {miss}}}$ and ${M_{\mathrm {T2}}}$ bins are open-ended, e.g., bin 20 requires $ {E_{\mathrm {T}}^{\text {miss}}} > $ 450 GeV and $ {M_{\mathrm {T2}}} > $ 400 GeV.
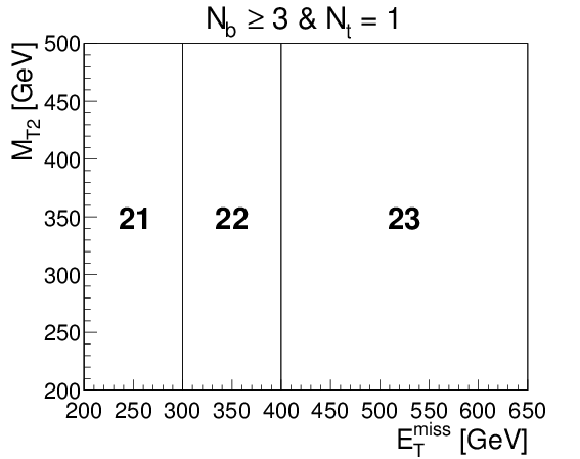
png pdf	Figure 5-c: Search region definitions for bin numbers 21-23 of the gluino-mediated production optimization, as defined in the text. The highest ${E_{\mathrm {T}}^{\text {miss}}}$ and ${M_{\mathrm {T2}}}$ bins are open-ended, e.g., bin 23 requires $ {E_{\mathrm {T}}^{\text {miss}}} > $ 450 GeV and $ {M_{\mathrm {T2}}} > $ 200 GeV.
png pdf	Figure 5-d: Search region definitions for bin numbers 25-31 of the gluino-mediated production optimization, as defined in the text. The highest ${E_{\mathrm {T}}^{\text {miss}}}$ and ${M_{\mathrm {T2}}}$ bins are open-ended, e.g., bin 31 requires $ {E_{\mathrm {T}}^{\text {miss}}} > $ 350 GeV and $ {M_{\mathrm {T2}}} > $ 200 GeV.
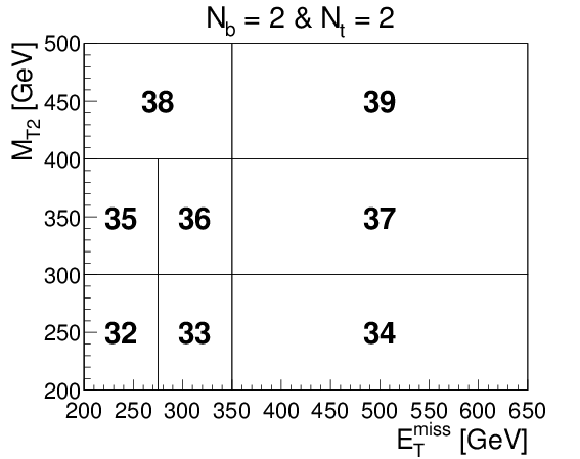
png pdf	Figure 5-e: Search region definitions for bin numbers 32-39 of the gluino-mediated production optimization, as defined in the text. The highest ${E_{\mathrm {T}}^{\text {miss}}}$ and ${M_{\mathrm {T2}}}$ bins are open-ended, e.g., bin 39 requires $ {E_{\mathrm {T}}^{\text {miss}}} > $ 350 GeV and $ {M_{\mathrm {T2}}} > $ 400 GeV.
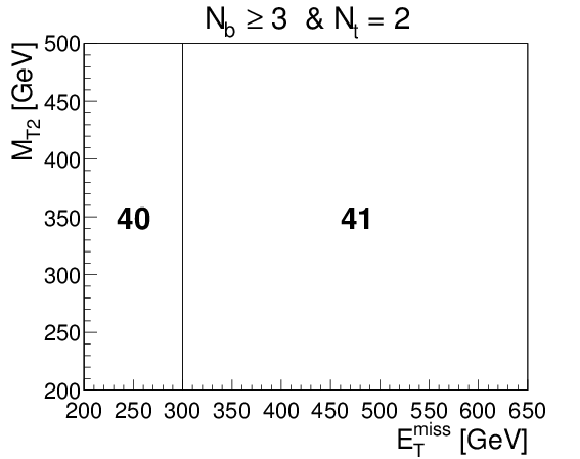
png pdf	Figure 5-f: Search region definitions for bin numbers 40-41 of the gluino-mediated production optimization, as defined in the text. The highest ${E_{\mathrm {T}}^{\text {miss}}}$ and ${M_{\mathrm {T2}}}$ bins are open-ended, e.g., bin 41 requires $ {E_{\mathrm {T}}^{\text {miss}}} > $ 300 GeV and $ {M_{\mathrm {T2}}} > $ 200 GeV.
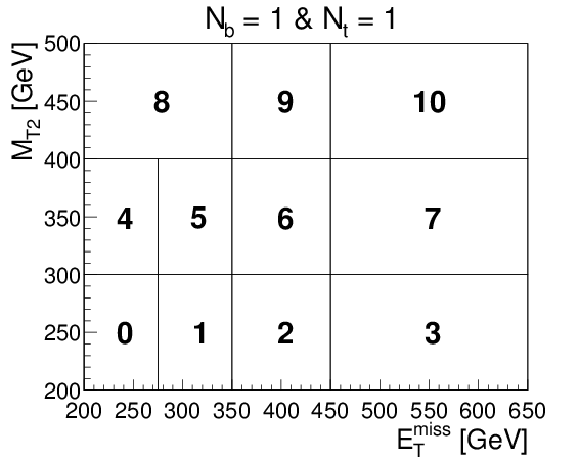
png pdf	Figure 6: Search region definitions for bin numbers 0-36 for the direct top squark production optimization, as defined in the text. The highest ${E_{\mathrm {T}}^{\text {miss}}}$ and ${M_{\mathrm {T2}}}$ bins are open-ended, e.g., bin 10 requires $ {E_{\mathrm {T}}^{\text {miss}}} > $ 450 GeV and $ {M_{\mathrm {T2}}} > $ 400 GeV.
png pdf	Figure 6-a: Search region definitions for bin numbers 0-10 for the direct top squark production optimization, as defined in the text. The highest ${E_{\mathrm {T}}^{\text {miss}}}$ and ${M_{\mathrm {T2}}}$ bins are open-ended, e.g., bin 10 requires $ {E_{\mathrm {T}}^{\text {miss}}} > $ 450 GeV and $ {M_{\mathrm {T2}}} > $ 400 GeV.
png pdf	Figure 6-b: Search region definitions for bin numbers 11-20 for the direct top squark production optimization, as defined in the text. The highest ${E_{\mathrm {T}}^{\text {miss}}}$ and ${M_{\mathrm {T2}}}$ bins are open-ended, e.g., bin 20 requires $ {E_{\mathrm {T}}^{\text {miss}}} > $ 450 GeV and $ {M_{\mathrm {T2}}} > $ 400 GeV.
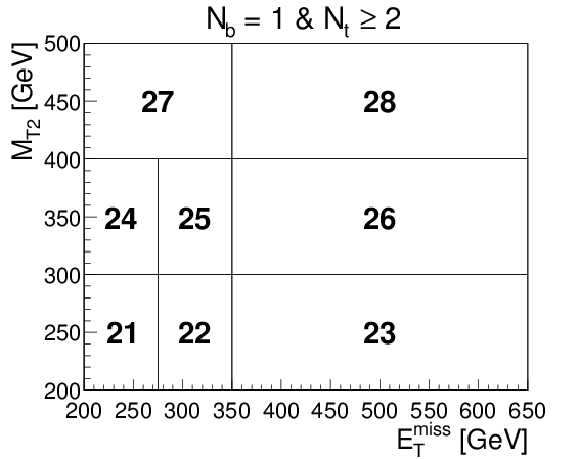
png pdf	Figure 6-c: Search region definitions for bin numbers 21-28 for the direct top squark production optimization, as defined in the text. The highest ${E_{\mathrm {T}}^{\text {miss}}}$ and ${M_{\mathrm {T2}}}$ bins are open-ended, e.g., bin 28 requires $ {E_{\mathrm {T}}^{\text {miss}}} > $ 350 GeV and $ {M_{\mathrm {T2}}} > $ 400 GeV.
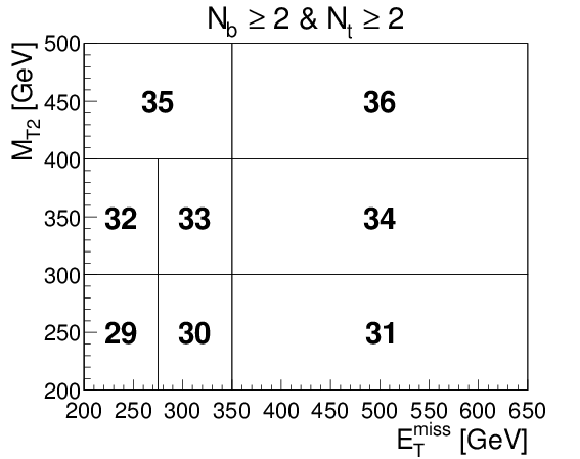
png pdf	Figure 6-d: Search region definitions for bin numbers 29-36 for the direct top squark production optimization, as defined in the text. The highest ${E_{\mathrm {T}}^{\text {miss}}}$ and ${M_{\mathrm {T2}}}$ bins are open-ended, e.g., bin 36 requires $ {E_{\mathrm {T}}^{\text {miss}}} > $ 350 GeV and $ {M_{\mathrm {T2}}} > $ 400 GeV.
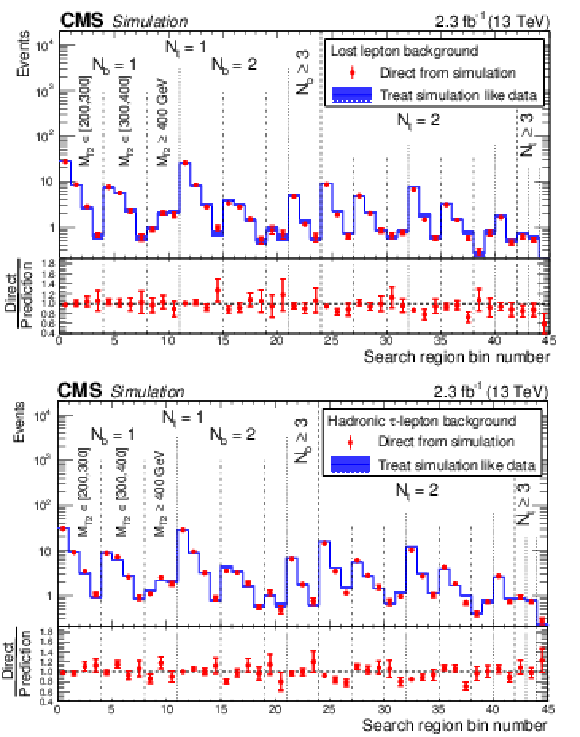
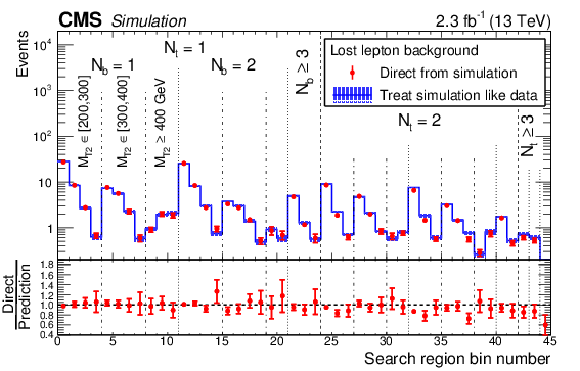
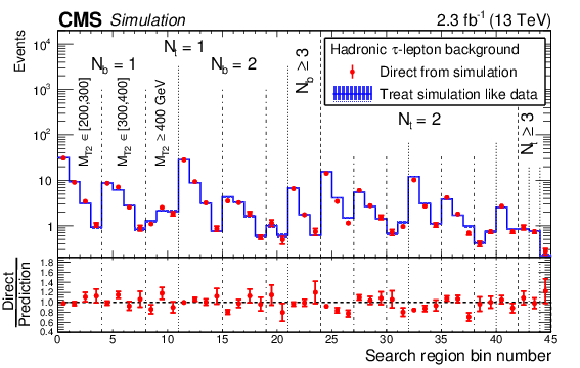
png pdf	Figure 7: (Top) The lost-lepton background in the 45 search regions optimized for gluino-mediated production as determined directly from ${\mathrm{ t } \mathrm{ \bar{t} } } $, single top quark, and W+jets simulation (points) and as predicted by applying the lost-lepton background determination procedure to the simulated muon control sample (histograms). The lower panel shows the same results after dividing by the predicted value. (Bottom) The corresponding simulated results for the background from hadronically decaying $\tau $ leptons. For both plots, vertical lines indicate search regions with different ${N_{\mathrm{ t } }}$, ${N_{\mathrm{ b } }}$, and ${M_{\mathrm {T2}}}$ values. Within each ($ {N_{\mathrm{ t } }}$, ${N_{\mathrm{ b } }}$, ${M_{\mathrm {T2}}}$) region, the bins indicate the different ${E_{\mathrm {T}}^{\text {miss}}}$ selections, as defined in Fig. 5. Only statistical uncertainties are shown.
png pdf	Figure 7-a: The lost-lepton background in the 45 search regions optimized for gluino-mediated production as determined directly from ${\mathrm{ t } \mathrm{ \bar{t} } } $, single top quark, and W+jets simulation (points) and as predicted by applying the lost-lepton background determination procedure to the simulated muon control sample (histograms). The lower panel shows the same results after dividing by the predicted value.
png pdf	Figure 7-b: The simulated results corresponding to Fig.007-a for the background from hadronically decaying $\tau $ leptons. For both plots, vertical lines indicate search regions with different ${N_{\mathrm{ t } }}$, ${N_{\mathrm{ b } }}$, and ${M_{\mathrm {T2}}}$ values. Within each ($ {N_{\mathrm{ t } }}$, ${N_{\mathrm{ b } }}$, ${M_{\mathrm {T2}}}$) region, the bins indicate the different ${E_{\mathrm {T}}^{\text {miss}}}$ selections, as defined in Fig. 5. Only statistical uncertainties are shown.
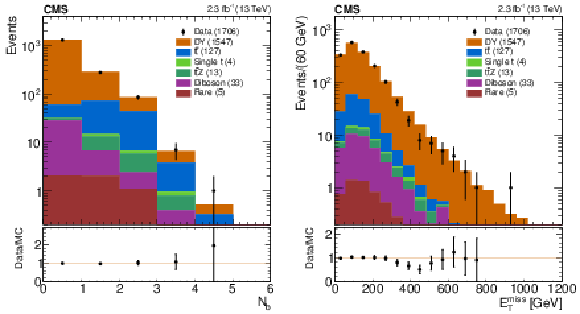
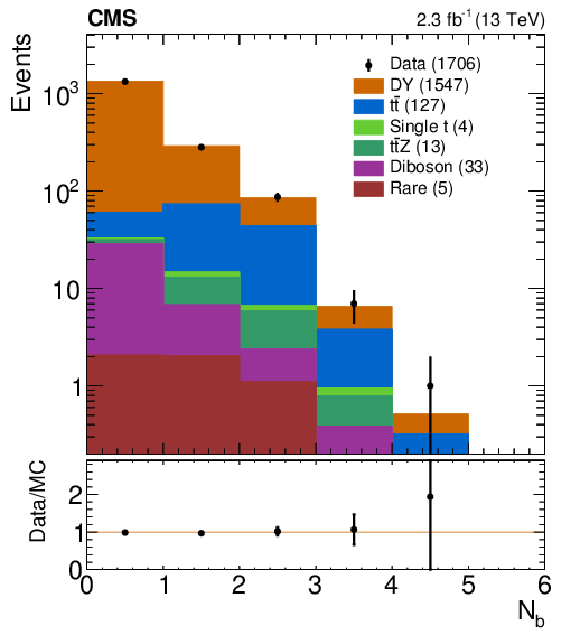
png pdf	Figure 8: Determination of the $ {\mathrm{ Z } \to \nu \bar{\nu} } $ background: The ${N_{\mathrm{ b } }}$ (left) and ${E_{\mathrm {T}}^{\text {miss}}}$ (right) distributions in data and simulation in the loose $\mathrm{ Z } \to \mu \mu $ control region, after applying the $S_\mathrm {DY}(N_\mathrm {j})$ scale factor to the simulation. The lower panels show the ratio between data and simulation. Only statistical uncertainties are shown. The values in parentheses in the legend indicate the integrated yield for each given process.
png pdf	Figure 8-a: Determination of the $ {\mathrm{ Z } \to \nu \bar{\nu} } $ background: The ${N_{\mathrm{ b } }}$ distributions in data and simulation in the loose $\mathrm{ Z } \to \mu \mu $ control region, after applying the $S_\mathrm {DY}(N_\mathrm {j})$ scale factor to the simulation. The lower panel shows the ratio between data and simulation. Only statistical uncertainties are shown. The values in parentheses in the legend indicate the integrated yield for each given process.
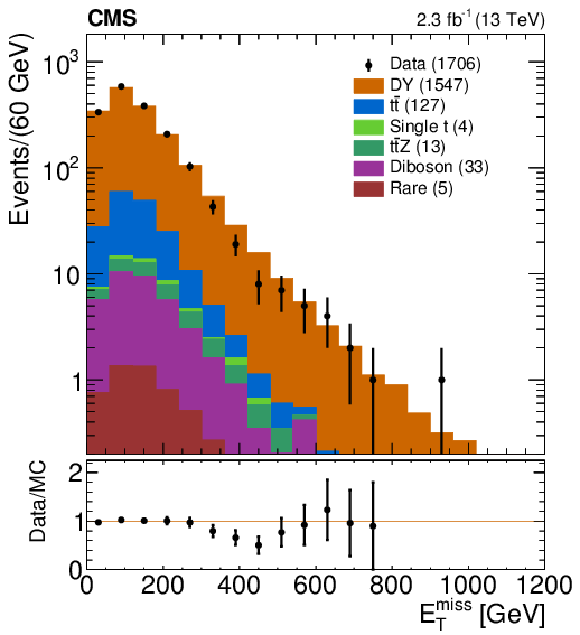
png pdf	Figure 8-b: Determination of the $ {\mathrm{ Z } \to \nu \bar{\nu} } $ background: The ${E_{\mathrm {T}}^{\text {miss}}}$ distributions in data and simulation in the loose $\mathrm{ Z } \to \mu \mu $ control region, after applying the $S_\mathrm {DY}(N_\mathrm {j})$ scale factor to the simulation. The lower panel shows the ratio between data and simulation. Only statistical uncertainties are shown. The values in parentheses in the legend indicate the integrated yield for each given process.
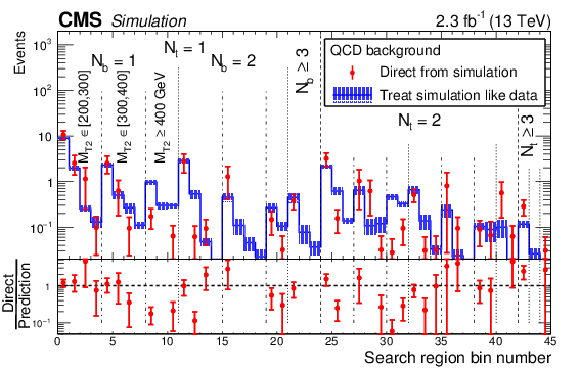
png pdf	Figure 9: The QCD multijet background in the 45 search regions optimized for gluino-mediated production as determined directly from simulation (points) and as predicted by applying the QCD multijet background determination procedure to simulated event samples in the inverted-$\Delta \phi $ control region (histograms). The lower panel shows the same results after dividing by the predicted value. Only statistical uncertainties are shown. The labeling of the search regions is the same as in Fig. 7.
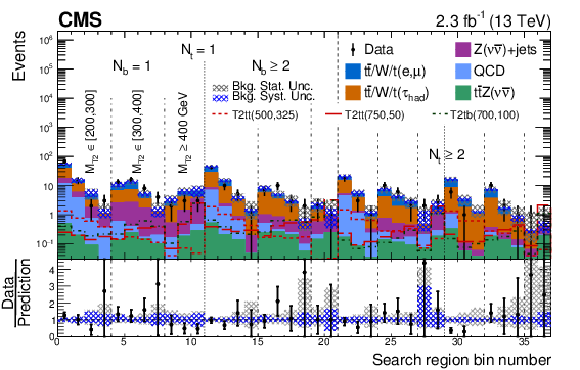
png pdf	Figure 10: Observed event yields in data (black points) and predicted SM background (filled solid area) for the 37 search bins optimized for direct top squark production. The red and dark green lines indicate various signal models: the T2tt model with $m_{\tilde{ \mathrm{ t } } } = $ 500 GeV and $m_{\tilde{\chi}^0_1 } = $ 325 GeV (red short-dashed line), the T2tt model with $m_{\tilde{ \mathrm{ t } } } = $ 750 GeV and $m_{\tilde{\chi}^0_1 } = $ 50 GeV (red long-dashed line), and the T2tb model with $m_{\tilde{ \mathrm{ t } } } = $ 700 GeV and $m_{\tilde{\chi}^0_1 } = $ 100 GeV (dark green dashed-dotted line). The lower panel shows the ratio of data over total background prediction in each search bin. For both panels, the error bars show the statistical uncertainty associated with the observed data counts, and the grey (blue) hatched bands indicate the statistical (systematic) uncertainties in the total predicted background.
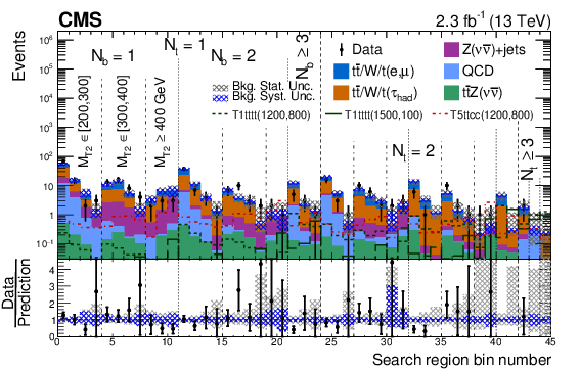
png pdf	Figure 11: Observed event yields in data (black points) and predicted SM background (filled solid area) for the 45 search bins optimized for gluino models. The red and dark green lines indicate various signal models: the T1tttt model with $m_{\tilde{ \mathrm{g} } } = $ 1200 GeV and $m_{\tilde{\chi}^0_1 } = $ 800 GeV (dark green short-dashed line), the T1tttt model with $m_{\tilde{ \mathrm{g} } } = $ 1500 GeV and $m_{\tilde{\chi}^0_1 } = $ 100 GeV (dark green long-dashed line), and the T5ttcc model with $m_{\tilde{ \mathrm{g} } } = $ 1200 GeV and $m_{\tilde{\chi}^0_1 } = $ 800 GeV (red dashed-dotted line). The lower panel shows the ratio of data over total background prediction in each search bin. For both panels, the error bars show the statistical uncertainty associated with the observed data counts, and the grey (blue) hatched bands indicate the statistical (systematic) uncertainties in the total predicted background.
png pdf	Figure 12: Exclusion limits at 95% CL for simplified models of top squark pair production in the T2tt (left) and T2tb (right) scenario, assuming a 50% branching fraction for each of the $\tilde{ \mathrm{ t } } \to \mathrm{ t } \tilde{\chi}^0_1 /\tilde{ \mathrm{ t } } \to \mathrm{b} \tilde{\chi}^{pm}_1 $ modes and a 5 GeV mass difference between the $\tilde{\chi}^{pm}_1 $ and $\tilde{\chi}^0_1 $ (right). The solid black curves represent the observed exclusion contour with respect to NLO+NLL cross section calculations [89] and the corresponding $\pm $1 standard deviation uncertainties. The dashed red curves indicate the expected exclusion contour and the $\pm $1 standard deviation uncertainties including experimental uncertainties. No interpretation is provided for signal models for which $ {| m_{\tilde{ \mathrm{ t } } } - m_{\tilde{\chi}^0_1 } - m_{\mathrm{ t } } | } \le $ 25 GeV and $m_{\tilde{ \mathrm{ t } } } \leq $ 275 GeV because of significant differences between the fast simulation and the Geant4-based simulation for these low-$ {E_{\mathrm {T}}^{\text {miss}}}$ scenarios.
png pdf root	Figure 12-a: Exclusion limits at 95% CL for simplified models of top squark pair production in the T2tt scenario, assuming a 50% branching fraction for each of the $\tilde{ \mathrm{ t } } \to \mathrm{ t } \tilde{\chi}^0_1 /\tilde{ \mathrm{ t } } \to \mathrm{b} \tilde{\chi}^{pm}_1 $ modes and a 5 GeV mass difference between the $\tilde{\chi}^{pm}_1 $ and $\tilde{\chi}^0_1 $ (right). The solid black curves represent the observed exclusion contour with respect to NLO+NLL cross section calculations [89] and the corresponding $\pm $1 standard deviation uncertainties. The dashed red curves indicate the expected exclusion contour and the $\pm $1 standard deviation uncertainties including experimental uncertainties. No interpretation is provided for signal models for which $ {| m_{\tilde{ \mathrm{ t } } } - m_{\tilde{\chi}^0_1 } - m_{\mathrm{ t } } | } \le $ 25 GeV and $m_{\tilde{ \mathrm{ t } } } \leq $ 275 GeV because of significant differences between the fast simulation and the Geant4-based simulation for these low-$ {E_{\mathrm {T}}^{\text {miss}}}$ scenarios.
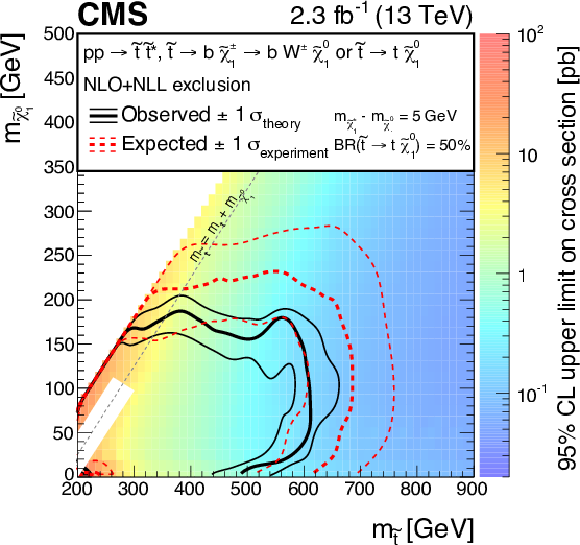
png pdf root	Figure 12-b: Exclusion limits at 95% CL for simplified models of top squark pair production in the T2tb scenario, assuming a 50% branching fraction for each of the $\tilde{ \mathrm{ t } } \to \mathrm{ t } \tilde{\chi}^0_1 /\tilde{ \mathrm{ t } } \to \mathrm{b} \tilde{\chi}^{pm}_1 $ modes and a 5 GeV mass difference between the $\tilde{\chi}^{pm}_1 $ and $\tilde{\chi}^0_1 $ (right). The solid black curves represent the observed exclusion contour with respect to NLO+NLL cross section calculations [89] and the corresponding $\pm $1 standard deviation uncertainties. The dashed red curves indicate the expected exclusion contour and the $\pm $1 standard deviation uncertainties including experimental uncertainties. No interpretation is provided for signal models for which $ {| m_{\tilde{ \mathrm{ t } } } - m_{\tilde{\chi}^0_1 } - m_{\mathrm{ t } } | } \le $ 25 GeV and $m_{\tilde{ \mathrm{ t } } } \leq $ 275 GeV because of significant differences between the fast simulation and the Geant4-based simulation for these low-$ {E_{\mathrm {T}}^{\text {miss}}}$ scenarios.
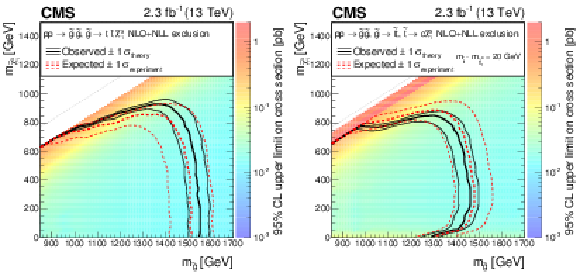
png pdf	Figure 13: Exclusion limits at 95% CL for simplified models of top squarks produced via decays of gluino pairs in the T1tttt (left) and T5ttcc (right) scenarios. The solid black curves represent the observed exclusion contour with respect to NLO+NLL cross section calculations [89] and the corresponding $\pm $1 standard deviation uncertainties. The dashed red curves indicate the expected exclusion contour and the $\pm $1 standard deviation uncertainties including experimental uncertainties.
png pdf root	Figure 13-a: Exclusion limits at 95% CL for simplified models of top squarks produced via decays of gluino pairs in the T1tttt scenario. The solid black curves represent the observed exclusion contour with respect to NLO+NLL cross section calculations [89] and the corresponding $\pm $1 standard deviation uncertainties. The dashed red curves indicate the expected exclusion contour and the $\pm $1 standard deviation uncertainties including experimental uncertainties.
png pdf root	Figure 13-b: Exclusion limits at 95% CL for simplified models of top squarks produced via decays of gluino pairs in the T5ttcc scenario. The solid black curves represent the observed exclusion contour with respect to NLO+NLL cross section calculations [89] and the corresponding $\pm $1 standard deviation uncertainties. The dashed red curves indicate the expected exclusion contour and the $\pm $1 standard deviation uncertainties including experimental uncertainties.

Tables
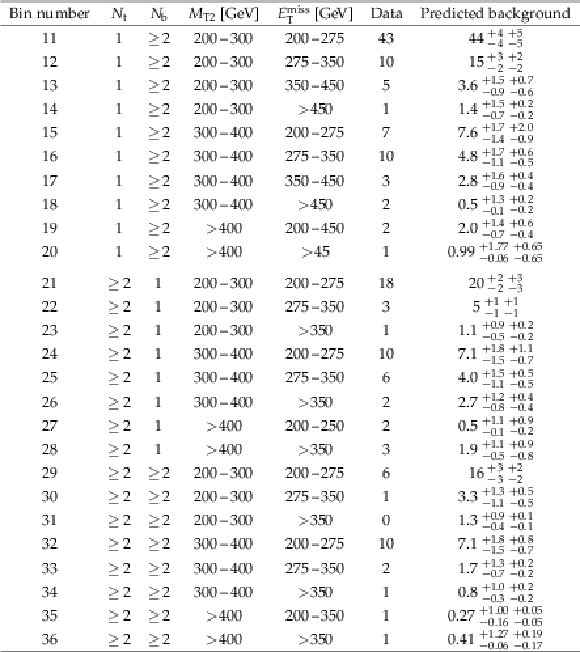
png pdf	Table 1: Observed yields from the data compared to the total background predictions for the search bins that are common between the direct top squark and gluino-mediated production optimizations. The quoted uncertainties on the predicted background yields are statistical and systematic, respectively.
png pdf	Table 2: Observed yields from the data compared to the total background predictions for the search bins that are specific to the direct top squark production optimization. The quoted uncertainties on the predicted background yields are statistical and systematic, respectively.
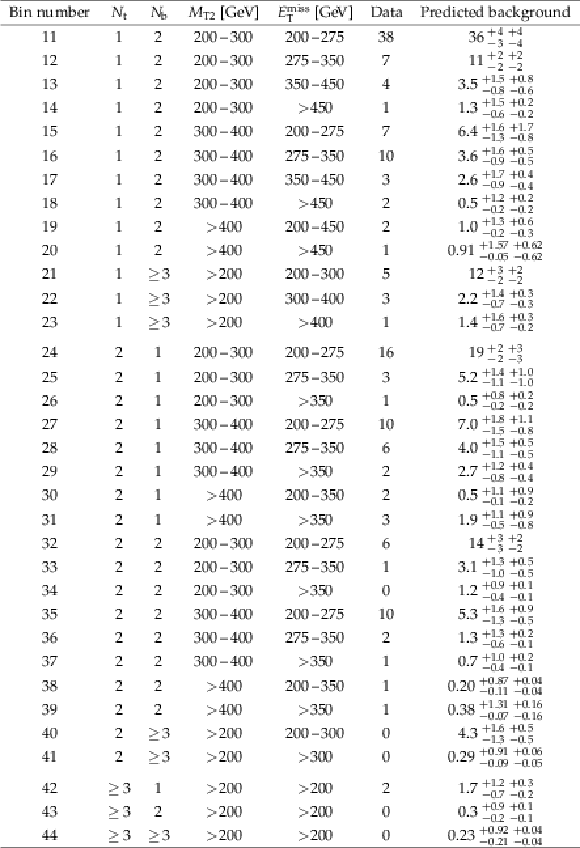
png pdf	Table 3: Observed yields from the data compared to the total background predictions for the search bins that are specific to the gluino-mediated production optimization. The quoted uncertainties on the predicted background yields are statistical and systematic, respectively.

Summary

Results have been presented from a search for direct and gluino-mediated top squark production in final states that include tagged top quark decays. The search uses all-hadronic events with at least four jets and a large imbalance in transverse momentum ($E_{\mathrm{T}}^{\text{miss}}$), selected from data corresponding to an integrated luminosity of 2.3 fb$^{-1}$ collected in proton-proton collisions at a center-of-mass energy of 13 TeV with the CMS detector. A set of search regions is defined based on $E_{\mathrm{T}}^{\text{miss}}$, ${M_{\mathrm{T2}}} $, the number of top quark tagged objects, and the number of b-tagged jets. No statistically significant excess of events is observed above the expected standard model background. Exclusion limits are set at 95% confidence level for simplified models of direct top squark pair production and of gluino pair production, where the gluinos decay to final states that include top quarks. For simplified models of pair production of top squarks, which decay to a top quark and a neutralino (T2tt), top squark masses of up to 740 GeV and neutralino masses up to 240 GeV are excluded at 95% confidence level. For models that assume 50% branching fractions for top squark decays to a top quark and a neutralino, or to a bottom quark and a chargino that is nearly degenerate in mass with the neutralino (T2tb), top squark masses of up to 610 GeV and neutralino masses up to 190 GeV are also excluded. For simplified models of gluino pair production where each gluino decays to a top-antitop quark pair and a neutralino (T1tttt), gluino masses of up to 1550 GeV, and neutralino masses up to 900 GeV are excluded. Gluino masses of up to 1450 GeV, and neutralino masses up to 820 GeV are excluded for models in which the gluino decays to an on-shell top squark and a top quark, and the top squarks decays to a charm quark and a neutralino (T5ttcc). These are among the most restrictive currently available limits.

Additional Figures
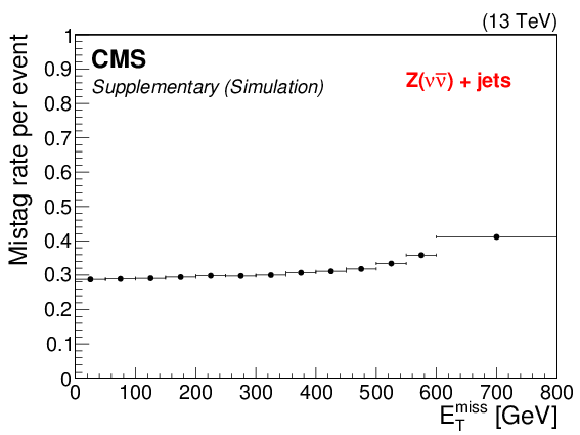
png pdf	Additional Figure 1: The event mistag rate of the top quark tagger as a function of $E_\text {T}^\text {miss}$ in the $\mathrm{ Z } \rightarrow \nu \nu $ simulated sample, with the following event selection requirements applied: $N_{\text {j}} \ge $ 4 for $ {p_{\mathrm {T}}} > $ 30 GeV, $|\eta | < $ 2.4 and $N_{\text {j}} \ge $ 2 for $ {p_{\mathrm {T}}} > $ 50 GeV, $|\eta | < $ 2.4; no electrons, muons, or isolated tracks; $\Delta \phi (E_\text {T}^\text {miss}, \text {jets})$ matching preselection requirements; and $N_{\text {b}} \ge $ 1.
png pdf	Additional Figure 2: The purity of the top quark tagger as a function of the reconstructed top quark $p_{\text {T}}$. The purity is defined as the fraction of reconstructed top quarks that are matched to a generator-level hadronically decaying top quark within a cone of $\Delta R=$ 0.4, and was measured in a sample of simulated one-lepton $\mathrm{ t \bar{t} } $ events. The following event selection requirements were applied: $N_{\text {j}} \ge $ 4 for $ {p_{\mathrm {T}}} > $ 30 GeV, $|\eta | < $ 2.4 and $N_{\text {j}} \ge $ 2 for $ {p_{\mathrm {T}}} > $ 50 GeV, $|\eta | < $ 2.4; $N_{\text {b}} \ge $ 1; and $E_\text {T}^\text {miss}> $ 200 GeV.
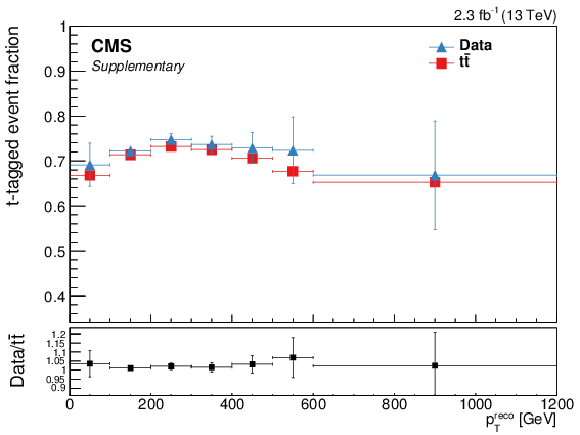
png pdf	Additional Figure 3: The t-tagged event fraction measured in data and $\mathrm{ t \bar{t} } $ simulated samples, as a function of the reconstructed top quark candidate ${p_{\mathrm {T}}} $. The data are selected from a single muon dataset with the following selection applied: events pass noise filters; $N_{\text {j}} \ge $ 4 for $ {p_{\mathrm {T}}} > $ 30 GeV, $|\eta | < $ 2.4 and $N_{\text {j}} \ge $ 2 for $ {p_{\mathrm {T}}} > $ 50 GeV, $|\eta | < $ 2.4; at least one muon with $ {p_{\mathrm {T}}} > $ 45 GeV, $|\eta | < $ 2.1; no electrons; muon $ {p_{\mathrm {T}}} + E_\text {T}^\text {miss} > $ 150 GeV; $\Delta \phi (E_\text {T}^\text {miss}, \text {jets})$ matching preselection requirements; $N_{\text {b}} \ge $ 1; and $E_\text {T}^\text {miss} > $ 20 GeV. We also require the presence of at least one candidate from the t-tagger in the event. This candidate can be either: (i) a trijet candidate, composed of three jets with $p_\text {T}> $ 30 GeV that are within $\Delta R= $ 1.5 of the candidate four-momentum, (ii) a dijet candidate, composed of two jets that are within $\Delta R= $ 1.5 of the candidate four-momentum, one of which should have a mass between 70 and 110 GeV, or (iii) a monojet candidate, which is simply a single jet with a mass between 110 and 220 GeV. The candidate used to compute the t-tagged event fraction is the candidate whose mass is closest to the top quark mass. The t-tagged event fraction then is defined as the fraction of events for which this top quark candidate satisfies all requirements of the top quark tagging algorithm. The error bar depicts the statistical uncertainty. The ratio of the t-tagged event fraction in data and simulated $\mathrm{ t \bar{t} } $ is shown in the lower plot, indicating good agreement.

Additional Tables
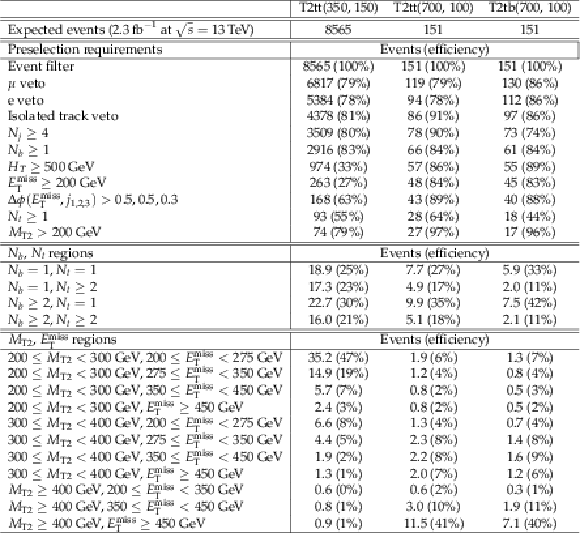
png pdf	Additional Table 1: The cut flow for a few benchmark signal models of direct top squark production. For entries in the block labeled "Preselection requirements'', each efficiency is computed with respect to the previous one. For the other two blocks, all efficiencies are computed with respect to the last line of the "Preselection requirements" block.
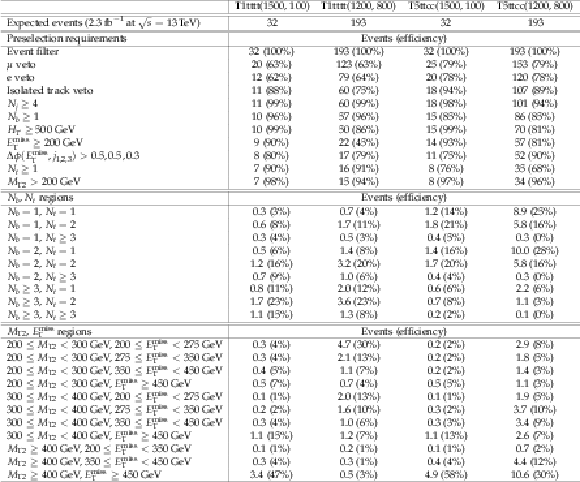
png pdf	Additional Table 2: The cut flow for a few benchmark signal models of gluino mediated top squark production. For entries in the block labeled "Preselection requirements'', each efficiency is computed with respect to the previous one. For the other two blocks, all efficiencies are computed with respect to the last line of the "Preselection requirements" block.

ROOT files with efficiency maps for each simplified model and each search region bin are provided in the following files: T2tt: acc_maps_T2tt.root T2tb: acc_maps_T2tb.root T1tttt: acc_maps_T1tttt.root T5ttcc: acc_maps_T5ttcc.root

References
1	ATLAS Collaboration	Observation of a new particle in the search for the Standard Model Higgs boson with the ATLAS detector at the LHC	PLB 716 (2012) 1	1207.7214
2	CMS Collaboration	Observation of a new boson at a mass of 125 GeV with the CMS experiment at the LHC	PLB 716 (2012) 30	CMS-HIG-12-028 1207.7235
3	CMS Collaboration	Observation of a new boson with mass near 125 GeV in pp collisions at $ \sqrt{s} = $ 7 and 8 TeV	JHEP 06 (2013) 081	CMS-HIG-12-036 1303.4571
4	L. Evans and P. Bryant	LHC Machine	JINST 3 (2008) S08001
5	ATLAS and CMS Collaborations	Combined Measurement of the Higgs Boson Mass in $ pp $ Collisions at $ \sqrt{s}= $ 7 and 8 TeV with the ATLAS and CMS Experiments	PRL 114 (2015) 191803	1503.07589
6	S. Dimopoulos and S. Raby	Supercolor	Nucl. Phys. B 192 (1981) 353
7	E. Witten	Dynamical Breaking of Supersymmetry	Nucl. Phys. B 188 (1981) 513
8	M. Dine, W. Fischler, and M. Srednicki	Supersymmetric Technicolor	Nucl. Phys. B 189 (1981) 575
9	S. Dimopoulos and H. Georgi	Softly Broken Supersymmetry and SU(5)	Nucl. Phys. B 193 (1981) 150
10	R. K. Kaul and P. Majumdar	Cancellation of quadratically divergent mass corrections in globally supersymmetric spontaneously broken gauge theories	Nucl. Phys. B 199 (1982) 36
11	R. Barbieri, S. Ferrara, and C. A. Savoy	Gauge models with spontaneously broken local supersymmetry	PLB 119 (1982) 343
12	R. Barbieri and G. F. Giudice	Upper bounds on supersymmetric particle masses	Nucl. Phys. B 306 (1988) 63
13	J. Wess and B. Zumino	Supergauge transformations in four-dimensions	Nucl. Phys. B 70 (1974) 39
14	Y. A. Gol'fand and E. P. Likhtman	Extension of the algebra of Poincar$ \'e $ group generators and violation of P invariance	JEPTL 13 (1971)323
15	D. V. Volkov and V. P. Akulov	Possible universal neutrino interaction	JEPTL 16 (1972) 438
16	A. H. Chamseddine, R. L. Arnowitt, and P. Nath	Locally Supersymmetric Grand Unification	PRL 49 (1982) 970
17	G. L. Kane, C. F. Kolda, L. Roszkowski, and J. D. Wells	Study of constrained minimal supersymmetry	PRD 49 (1994) 6173	hep-ph/9312272
18	P. Fayet	Supergauge invariant extension of the Higgs mechanism and a model for the electron and its neutrino	Nucl. Phys. B 90 (1975) 104
19	L. J. Hall, J. D. Lykken, and S. Weinberg	Supergravity as the messenger of supersymmetry breaking	PRD 27 (1983) 2359
20	P. Ramond	Dual theory for free fermions	PRD 3 (1971) 2415
21	B. de Carlos and J. A. Casas	One-loop analysis of the electroweak breaking in supersymmetric models and the fine-tuning problem	PLB 309 (1993) 320	hep-ph/9303291
22	S. Dimopoulos and G. F. Giudice	Naturalness constraints in supersymmetric theories with non-universal soft terms	PLB 357 (1995) 573	hep-ph/9507282
23	N. Sakai	Naturalness in supersymmetric GUTS	Z. Phys. C 11 (1981) 153
24	M. Papucci, J. T. Ruderman, and A. Weiler	Natural SUSY Endures	JHEP 09 (2012) 035	1110.6926
25	C. Brust, A. Katz, S. Lawrence, and R. Sundrum	SUSY, the Third Generation and the LHC	JHEP 03 (2012) 103	1110.6670
26	J. L. Feng	Naturalness and the Status of Supersymmetry	Ann. Rev. Nucl. Part. Sci. 63 (2013) 351	1302.6587
27	A. Delgado et al.	The light stop window	EPJC 73 (2013) 2370	1212.6847
28	G. R. Farrar and P. Fayet	Phenomenology of the production, decay, and detection of new hadronic states associated with supersymmetry	PLB 76 (1978) 575
29	J. L. Feng	Dark Matter Candidates from Particle Physics and Methods of Detection	Ann. Rev. Astron. Astrophys. 48 (2010) 495	1003.0904
30	J. Alwall, P. Schuster, and N. Toro	Simplified Models for a First Characterization of New Physics at the LHC	PRD 79 (2009) 075020	0810.3921
31	J. Alwall, M.-P. Le, M. Lisanti, and J. G. Wacker	Model-Independent Jets plus Missing Energy Searches	PRD 79 (2009) 015005	0809.3264
32	LHC New Physics Working Group Collaboration	Simplified Models for LHC New Physics Searches	JPG 39 (2012) 105005	1105.2838
33	D. Alves, E. Izaguirre, and J. G. Wacker	Where the sidewalk ends: jets and missing energy search strategies for the 7$ TeV $ LHC	JHEP 10 (2011) 012	1102.5338
34	CMS Collaboration	Interpretation of searches for supersymmetry with simplified models	PRD 88 (2013) 052017	CMS-SUS-11-016 1301.2175
35	ATLAS Collaboration	ATLAS Run 1 searches for direct pair production of third-generation squarks at the Large Hadron Collider	EPJC 75 (2015) 510	1506.08616
36	ATLAS Collaboration	Search for pair-produced third-generation squarks decaying via charm quarks or in compressed supersymmetric scenarios in $ pp $ collisions at $ \sqrt{s}= 8 $ TeV with the ATLAS detector	PRD 90 (2014) 052008	1407.0608
37	ATLAS Collaboration	Search for direct pair production of the top squark in all-hadronic final states in proton-proton collisions at $ \sqrt{s} = $ 8 TeV with the ATLAS detector	JHEP 09 (2014) 015	1406.1122
38	ATLAS Collaboration	Search for direct third-generation squark pair production in final states with missing transverse momentum and two $ b $-jets in $ \sqrt{s} = $ 8 TeV $ pp $ collisions with the ATLAS detector	JHEP 10 (2013) 189	1308.2631
39	ATLAS Collaboration	Search for direct top-squark pair production in final states with two leptons in pp collisions at $ \sqrt{s}= $ 8 TeV with the ATLAS detector	JHEP 06 (2014) 124	1403.4853
40	ATLAS Collaboration	Search for supersymmetry at $ \sqrt{s}= $ 8 TeV in final states with jets and two same-sign leptons or three leptons with the ATLAS detector	JHEP 06 (2014) 035	1404.2500
41	CMS Collaboration	Searches for third-generation squark production in fully hadronic final states in proton-proton collisions at $ \sqrt{s} = $ 8 TeV	JHEP 06 (2015) 116	CMS-SUS-14-001 1503.08037
42	CMS Collaboration	Search for direct pair production of supersymmetric top quarks decaying to all-hadronic final states in pp collisions at $ \sqrt{s} = 8\;\text {TeV} $	EPJC 76 (2016) 460	CMS-SUS-13-023 1603.00765
43	CMS Collaboration	Search for direct pair production of scalar top quarks in the single- and dilepton channels in proton-proton collisions at $ \sqrt{s} = $ 8 TeV	JHEP 07 (2016) 027	CMS-SUS-14-015 1602.03169
44	CMS Collaboration	Search for top-squark pair production in the single-lepton final state in pp collisions at $ \sqrt{s} = $ 8 TeV	EPJC 73 (2013) 2677	CMS-SUS-13-011 1308.1586
45	CMS Collaboration	Search for supersymmetry in events with soft leptons, low jet multiplicity, and missing transverse energy in proton-proton collisions at $ \sqrt{s} = $ 8 TeV	PLB 759 (2016) 9	CMS-SUS-14-021 1512.08002
46	ATLAS Collaboration	Summary of the searches for squarks and gluinos using $ \sqrt{s} = $ 8 TeV $ pp $ collisions with the ATLAS experiment at the LHC	JHEP 10 (2015) 054	1507.05525
47	ATLAS Collaboration	Search for squarks and gluinos with the ATLAS detector in final states with jets and missing transverse momentum using $ \sqrt{s} = $ 8 TeV proton-proton collision data	JHEP 09 (2014) 176	1405.7875
48	ATLAS Collaboration	Search for new phenomena in final states with large jet multiplicities and missing transverse momentum at $ \sqrt{s}= $ 8 TeV proton-proton collisions using the ATLAS experiment	JHEP 10 (2013) 130	1308.1841
49	ATLAS Collaboration	Search for new phenomena in final states with an energetic jet and large missing transverse momentum in $ pp $ collisions at $ \sqrt{s}= $ 8 TeV with the ATLAS detector	EPJC 75 (2015) 299	1502.01518
50	ATLAS Collaboration	Search for squarks and gluinos in events with isolated leptons, jets and missing transverse momentum at $ \sqrt{s} = $ 8 TeV with the ATLAS detector	JHEP 04 (2015) 116	1501.03555
51	ATLAS Collaboration	Search for strong production of supersymmetric particles in final states with missing transverse momentum and at least three $ b $-jets at $ \sqrt{s}= $ 8 TeV proton-proton collisions with the ATLAS detector	JHEP 10 (2014) 024	1407.0600
52	CMS Collaboration	Search for supersymmetry in pp collisions at $ \sqrt{s} = $ 8 TeV in events with a single lepton, large jet multiplicity, and multiple b jets	PLB 733 (2014) 328	CMS-SUS-13-007 1311.4937
53	CMS Collaboration	Searches for supersymmetry based on events with b jets and four W bosons in pp collisions at 8 TeV	PLB 745 (2015) 5	CMS-SUS-14-010 1412.4109
54	CMS Collaboration	Search for supersymmetry in pp collisions at $ \sqrt{s} = $ 8 TeV in final states with boosted W bosons and b jets using razor variables	PRD 93 (2016) 092009	CMS-SUS-14-007 1602.02917
55	CMS Collaboration	Searches for supersymmetry using the M$ _\text{T2} $ variable in hadronic events produced in pp collisions at 8 TeV	JHEP 05 (2015) 078	CMS-SUS-13-019 1502.04358
56	CMS Collaboration	Search for supersymmetry using razor variables in events with b-tagged jets in pp collisions at $ \sqrt{s} = $ 8 TeV	PRD 91 (2015) 052018	CMS-SUS-13-004 1502.00300
57	CMS Collaboration	Search for new physics in the multijet and missing transverse momentum final state in proton-proton collisions at $ \sqrt{s} = $ 8 TeV	JHEP 06 (2014) 055	CMS-SUS-13-012 1402.4770
58	ATLAS Collaboration	Search for top squarks in final states with one isolated lepton, jets, and missing transverse momentum in $ \sqrt{s} = $ 13 TeV pp collisions with the ATLAS detector	PRD 94 (2016) 052009	1606.03903
59	CMS Collaboration	Search for new physics with the M$ _{T2} $ variable in all-jets final states produced in pp collisions at $ \sqrt{s} = $ 13 TeV	JHEP 10 (2016) 006	CMS-SUS-15-003 1603.04053
60	CMS Collaboration	Searches for pair production of third-generation squarks in $ \sqrt{s} = $ 13 TeV pp collisions	Submitted to EPJC	CMS-SUS-16-008 1612.03877
61	ATLAS Collaboration	Search for pair production of gluinos decaying via stop and sbottom in events with b-jets and large missing transverse momentum in $ pp $ collisions at $ \sqrt{s} = $ 13 TeV with the ATLAS detector	PRD 94 (2016) 032003	1605.09318
62	ATLAS Collaboration	Search for supersymmetry at $ \sqrt{s} = $ 13 TeV in final states with jets and two same-sign leptons or three leptons with the ATLAS detector	EPJC 76 (2016) 259	1602.09058
63	CMS Collaboration	Search for supersymmetry in the multijet and missing transverse momentum final state in pp collisions at 13 TeV	PLB 758 (2016) 152	CMS-SUS-15-002 1602.06581
64	CMS Collaboration	Search for new physics in same-sign dilepton events in proton--proton collisions at $ \sqrt{s} = $ 13 TeV	EPJC 76 (2016) 439	CMS-SUS-15-008 1605.03171
65	CMS Collaboration	Search for supersymmetry in pp collisions at $ \sqrt{s} = $ 13 TeV in the single-lepton final state using the sum of masses of large-radius jets	JHEP 08 (2016) 122	CMS-SUS-15-007 1605.04608
66	C. G. Lester and D. J. Summers	Measuring masses of semi-invisibly decaying particles pair produced at hadron colliders	PLB 463 (1999) 99	hep-ph/9906349
67	A. Barr, C. Lester, and P. Stephens	A variable for measuring masses at hadron colliders when missing energy is expected; $ m_{T2} $: the truth behind the glamour	JPG 29 (2003) 2343	hep-ph/0304226
68	CMS Collaboration	The CMS experiment at the CERN LHC	JINST 3 (2008) S08004	CMS-00-001
69	CMS Collaboration	Commissioning of the Particle-flow Event Reconstruction with the first LHC collisions recorded in the CMS detector	CDS
70	CMS Collaboration	Particle-Flow Event Reconstruction in CMS and Performance for Jets, Taus, and $ E_{\mathrm{T}}^{\text{miss}} $	CDS
71	M. Cacciari, G. P. Salam, and G. Soyez	The anti-$ k_t $ jet clustering algorithm	JHEP 04 (2008) 063	0802.1189
72	M. Cacciari and G. P. Salam	Pileup subtraction using jet areas	PLB 659 (2007) 119	0707.1378
73	M. Cacciari, G. P. Salam, and G. Soyez	FastJet user manual	EPJC 72 (2012) 1896	1111.6097
74	CMS Collaboration	Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV	Submitted to JINST (2016)	CMS-JME-13-004 1607.03663
75	CMS Collaboration	Identification of b quark jets at the CMS Experiment in the LHC Run 2	CMS-PAS-BTV-15-001	CMS-PAS-BTV-15-001
76	CMS Collaboration	Identification of b-quark jets with the CMS experiment	JINST 8 (2013) P04013	CMS-BTV-12-001 1211.4462
77	CMS Collaboration	Performance of CMS muon reconstruction in pp collision events at $ \sqrt{s} = $ 7 TeV	JINST 7 (2012) P10002	CMS-MUO-10-004 1206.4071
78	CMS Collaboration	Performance of electron reconstruction and selection with the CMS detector in proton-proton collisions at $ \sqrt{s} = $ 8 TeV	JINST 10 (2015) P06005	CMS-EGM-13-001 1502.02701
79	J. Alwall et al.	The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations	JHEP 07 (2014) 079	1405.0301
80	NNPDF Collaboration	Parton distributions for the LHC Run II	JHEP 04 (2015) 040	1410.8849
81	P. Nason	A new method for combining NLO QCD with shower Monte Carlo algorithms	JHEP 11 (2004) 040	hep-ph/0409146
82	S. Frixione, P. Nason, and C. Oleari	Matching NLO QCD computations with Parton Shower simulations: the POWHEG method	JHEP 11 (2007) 070	0709.2092
83	S. Alioli, P. Nason, C. Oleari, and E. Re	A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX	JHEP 06 (2010) 043	1002.2581
84	E. Re	Single-top $ Wt $-channel production matched with parton showers using the POWHEG method	EPJC 71 (2011) 1547	1009.2450
85	T. Sjostrand, S. Mrenna, and P. Z. Skands	A brief introduction to PYTHIA 8.1	CPC 178 (2008) 852	0710.3820
86	CMS Collaboration	Event generator tunes obtained from underlying event and multiparton scattering measurements	EPJC 76 (2016) 155	CMS-GEN-14-001 1512.00815
87	GEANT4 Collaboration	GEANT4---a simulation toolkit	NIMA 506 (2003) 250
88	S. Abdullin et al.	The fast simulation of the CMS detector at LHC	J. Phys. Conf. Ser. 331 (2011) 032049
89	C. Borschensky et al.	Squark and gluino production cross sections in pp collisions at $ \sqrt{s} =$ 13, 14, 33 and 100 TeV	EPJC 74 (2014) 3174	1407.5066
90	M. Czakon and A. Mitov	Top++: A Program for the Calculation of the Top-Pair Cross-Section at Hadron Colliders	CPC 185 (2014) 2930	1112.5675
91	P. Kant et al.	HATHOR for single top-quark production: Updated predictions and uncertainty estimates for single top-quark production in hadronic collisions	CPC 191 (2015) 74	1406.4403
92	M. Aliev et al.	HATHOR: HAdronic Top and Heavy quarks crOss section calculatoR	CPC 182 (2011) 1034	1007.1327
93	T. Gehrmann et al.	W$ ^+ $W$ ^- $ Production at Hadron Colliders in Next to Next to Leading Order QCD	PRL 113 (2014) 212001	1408.5243
94	J. M. Campbell and R. K. Ellis	An update on vector boson pair production at hadron colliders	PRD 60 (1999) 113006	hep-ph/9905386
95	J. M. Campbell, R. K. Ellis, and C. Williams	Vector boson pair production at the LHC	JHEP 07 (2011) 018	1105.0020
96	Y. Li and F. Petriello	Combining QCD and electroweak corrections to dilepton production in FEWZ	PRD 86 (2012) 094034	1208.5967
97	D. E. Kaplan, K. Rehermann, M. D. Schwartz, and B. Tweedie	Top Tagging: A Method for Identifying Boosted Hadronically Decaying Top Quarks	PRL 101 (2008) 142001	0806.0848
98	T. Plehn, M. Spannowsky, M. Takeuchi, and D. Zerwas	Stop reconstruction with tagged tops	JHEP 10 (2010) 078	1006.2833
99	D. E. Kaplan, K. Rehermann, and D. Stolarski	Searching for Direct Stop Production in Hadronic Top Data at the LHC	JHEP 07 (2012) 119	1205.5816
100	Particle Data Group, K. A. Olive et al.	Review of particle physics	CPC 38 (2014) 090001
101	CMS Collaboration	Jet Performance in pp Collisions at 7 TeV	CDS
102	ATLAS and CMS Collaborations	Procedure for the LHC Higgs boson search combination in summer 2011	Technical Report ATL-PHYS-PUB-2011-011, CMS NOTE-2011/005
103	A. L. Read	Presentation of search results: the $ CL_s $ technique	JPG 28 (2002) 2693
104	T. Junk	Confidence level computation for combining searches with small statistics	NIMA 434 (1999) 435	hep-ex/9902006
105	G. Cowan, K. Cranmer, E. Gross, and O. Vitells	Asymptotic formulae for likelihood-based tests of new physics	EPJC 71 (2011) 1554	1007.1727

Compact Muon Solenoid LHC, CERN

© Copyright CERN 2015 for the benefit of the CMS Collaboration