Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-SUS-15-007 ; CERN-EP-2016-111 | ||
| Search for supersymmetry in pp collisions at $ \sqrt{s} = $ 13 TeV in the single-lepton final state using the sum of masses of large-radius jets | ||
| CMS Collaboration | ||
| 15 May 2016 | ||
| J. High Energy Phys. 08 (2016) 122 | ||
| Abstract: Results are reported from a search for supersymmetric particles in proton-proton collisions in the final state with a single, high transverse momentum lepton; multiple jets, including at least one b-tagged jet; and large missing transverse momentum. The data sample corresponds to an integrated luminosity of 2.3 fb$^{-1}$ at $ \sqrt{s} = $ 13 TeV, recorded by the CMS experiment at the LHC. The search focuses on processes leading to high jet multiplicities, such as gluino pair production with ${\mathrm{ \tilde g}}\to{\mathrm{ t \bar{t} }}\,{\tilde{\chi}^0_1}$. The quantity $M_J$, defined as the sum of the masses of the large-radius jets in the event, is used in conjunction with other kinematic variables to provide discrimination between signal and background and as a key part of the background estimation method. The observed event yields in the signal regions in data are consistent with those expected for standard model backgrounds, estimated from control regions in data. Exclusion limits are obtained for a simplified model corresponding to gluino pair production with three-body decays into top quarks and neutralinos. Gluinos with a mass below 1600 GeV are excluded at a 95% confidence level for scenarios with low $\tilde{\chi}^0_1$ mass, and neutralinos with a mass below 800 GeV are excluded for a gluino mass of about 1300 GeV. For models with two-body gluino decays producing on-shell top squarks, the excluded region is only weakly sensitive to the top squark mass. | ||
| Links: e-print arXiv:1605.04608 [hep-ex] (PDF) ; CDS record ; inSPIRE record ; HepData record ; CADI line (restricted) ; | ||
| Figures & Tables | Summary | Additional Figures | References | CMS Publications |
|---|
| Figures | |
png pdf |
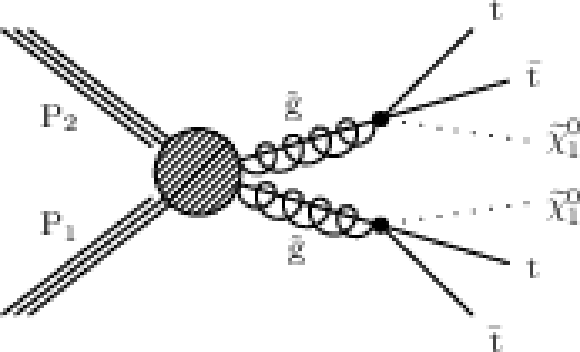
Figure 1:
Gluino pair production and decay for the simplified models T1tttt (left) and T5tttt (right). In T1tttt, the gluino undergoes three-body decay $\mathrm{ \tilde{g} } \to {\mathrm{ t \bar{t} } } {\tilde{\chi}^0_1} $ via a virtual intermediate top squark. In T5tttt, the gluino decays via the sequential two-body process $\mathrm{ \tilde{g} } \to \tilde{ \mathrm{ t } } _1\mathrm{ \bar{t} } $, $\tilde{ \mathrm{ t } } _1\to \mathrm{ t } {\tilde{\chi}^0_1} $. Because gluinos are Majorana particles, each one can decay to $\tilde{ \mathrm{ t } } _1\mathrm{ \bar{t} } $ and to the charge conjugate final state ${\tilde{ \mathrm{ \bar{t} } } }_1\mathrm{ t } $. |
png pdf |
Figure 1-a:
Gluino pair production and decay for the simplified model T1tttt. The gluino undergoes three-body decay $\mathrm{ \tilde{g} } \to {\mathrm{ t \bar{t} } } {\tilde{\chi}^0_1} $ via a virtual intermediate top squark. Because gluinos are Majorana particles, each one can decay to $\tilde{ \mathrm{ t } } _1\mathrm{ \bar{t} } $ and to the charge conjugate final state ${\tilde{ \mathrm{ \bar{t} } } }_1\mathrm{ t } $. |
png pdf |
Figure 1-b:
Gluino pair production and decay for the simplified model T5tttt. In T5tttt, the gluino decays via the sequential two-body process $\mathrm{ \tilde{g} } \to \tilde{ \mathrm{ t } } _1\mathrm{ \bar{t} } $, $\tilde{ \mathrm{ t } } _1\to \mathrm{ t } {\tilde{\chi}^0_1} $. Because gluinos are Majorana particles, each one can decay to $\tilde{ \mathrm{ t } } _1\mathrm{ \bar{t} } $ and to the charge conjugate final state ${\tilde{ \mathrm{ \bar{t} } } }_1\mathrm{ t } $. |
png pdf |
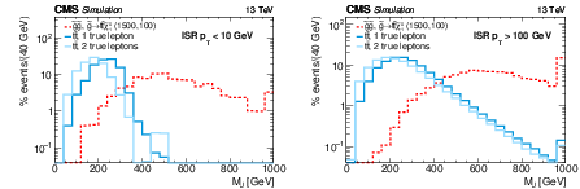
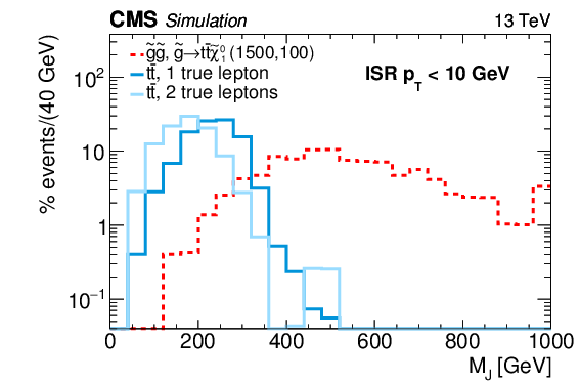
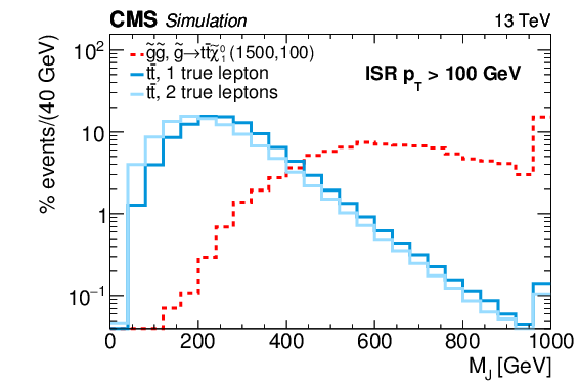
Figure 2:
Distributions of ${M_J} $, normalized to the same area, from simulated event samples with a small ISR contribution (left) and a significant ISR contribution (right). These components are defined according to whether the $ {p_{\mathrm {T}}} $ of the $ {\mathrm{ t \bar{t} } } $ system (or, in the case of signal events, that of the $\mathrm{ \tilde{g} } \mathrm{ \tilde{g} } $ system) is $< $ 10 GeV or $> $ 100 GeV, respectively. The T1tttt(NC) signal model (dashed red line), is described in Section 3; the first model parameter in parentheses corresponds to ${m_{\mathrm{ \tilde{g} } }}$ and the second to ${m_{ {\tilde{\chi}^0_1} }} $, both in units of GeV. The events satisfy the requirements $ {E_{\mathrm {T}}^{\text {miss}}} > $ 200 GeV and $ {H_{\mathrm {T}}} > $ 500 GeV and have at least one reconstructed lepton. |
png pdf |
Figure 2-a:
Distributions of ${M_J} $, normalized to the same area, from simulated event samples with a small ISR contribution. These components are defined according to whether the $ {p_{\mathrm {T}}} $ of the $ {\mathrm{ t \bar{t} } } $ system (or, in the case of signal events, that of the $\mathrm{ \tilde{g} } \mathrm{ \tilde{g} } $ system) is $< $ 10 GeV or $> $ 100 GeV, respectively. The T1tttt(NC) signal model (dashed red line), is described in Section 3; the first model parameter in parentheses corresponds to ${m_{\mathrm{ \tilde{g} } }}$ and the second to ${m_{ {\tilde{\chi}^0_1} }} $, both in units of GeV. The events satisfy the requirements $ {E_{\mathrm {T}}^{\text {miss}}} > $ 200 GeV and $ {H_{\mathrm {T}}} > $ 500 GeV and have at least one reconstructed lepton. |
png pdf |
Figure 2-b:
Distributions of ${M_J} $, normalized to the same area, from simulated event samples with a significant ISR contribution. These components are defined according to whether the $ {p_{\mathrm {T}}} $ of the $ {\mathrm{ t \bar{t} } } $ system (or, in the case of signal events, that of the $\mathrm{ \tilde{g} } \mathrm{ \tilde{g} } $ system) is $< $ 10 GeV or $> $ 100 GeV, respectively. The T1tttt(NC) signal model (dashed red line), is described in Section 3; the first model parameter in parentheses corresponds to ${m_{\mathrm{ \tilde{g} } }}$ and the second to ${m_{ {\tilde{\chi}^0_1} }} $, both in units of GeV. The events satisfy the requirements $ {E_{\mathrm {T}}^{\text {miss}}} > $ 200 GeV and $ {H_{\mathrm {T}}} > $ 500 GeV and have at least one reconstructed lepton. |
png pdf |
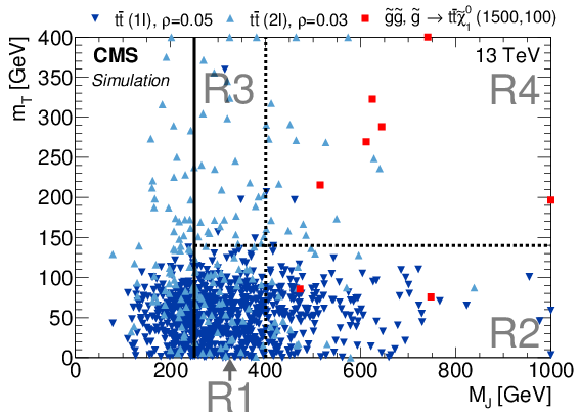
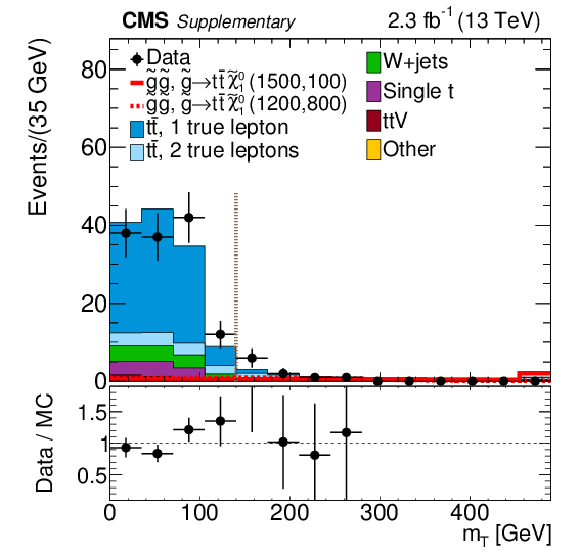
Figure 3:
Distribution of ${m_{\mathrm {T}}}$ in data and simulated event samples after the baseline selection is applied. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {m_{\mathrm {T}}} > $ 140 GeV threshold that separates the signal regions from the control samples. |
png pdf |
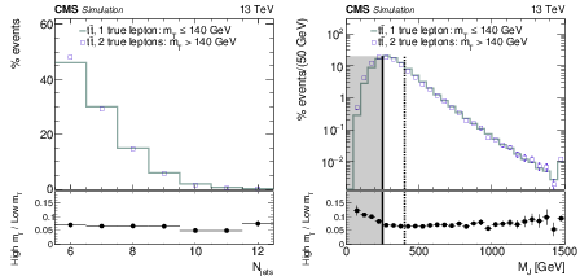
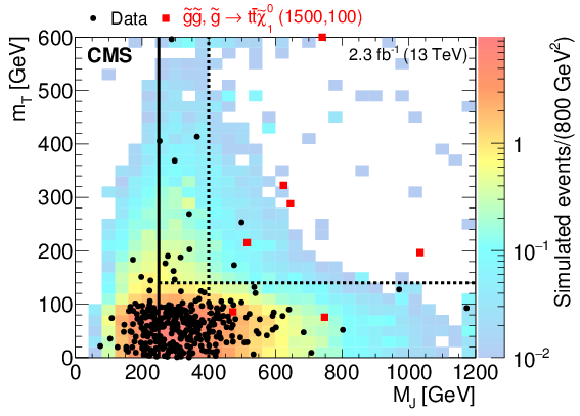
Figure 4:
Distribution of simulated single-lepton ${\mathrm{ t \bar{t} } }$ events (dark-blue triangles), dilepton ${\mathrm{ t \bar{t} } }$ events (light-blue inverted triangles), and T1tttt(1500,100) events (red squares) in the ${M_J} - {m_{\mathrm {T}}}$ plane after the baseline selection. Each marker represents one expected event at 2.3 fb$^{-1}$. Overflow events are placed on the edge of the plot. The values of the correlation coefficients $\rho $ for each background process are given in the legend. Region R4 is the nominal signal region, while R1, R2, and R3 serve as control regions. The small signal contributions in the control regions are taken into account in one of the global fits, as discussed in the text. |
png pdf |
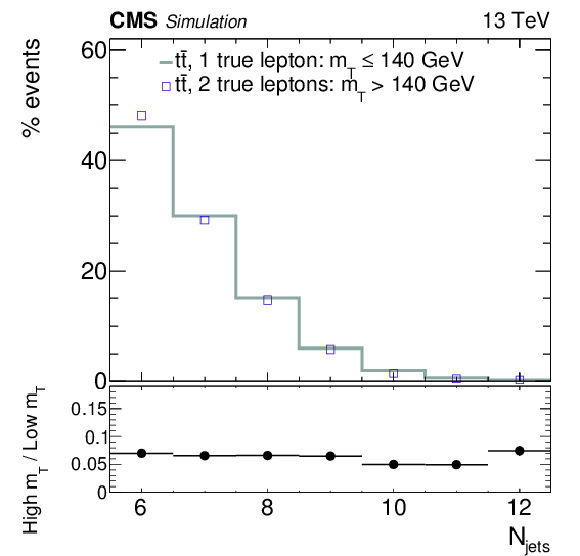
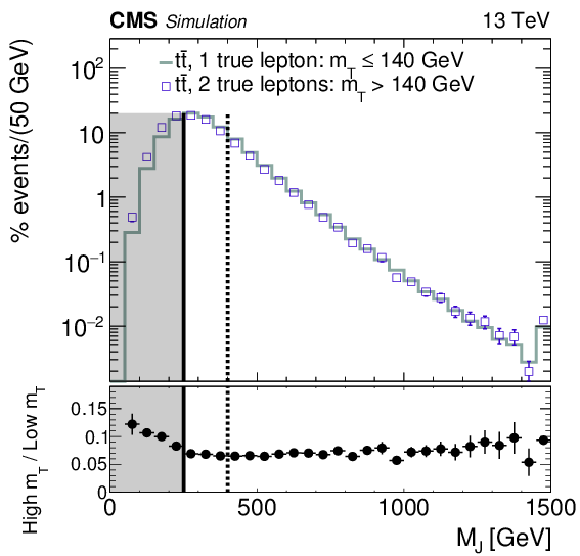
Figure 5:
Comparison of ${N_{\text {jets}}}$ and ${M_J}$ distributions, normalized to the same area, in simulated ${\mathrm{ t \bar{t} } } $ events with two true leptons at high ${m_{\mathrm {T}}}$ and one true lepton at low ${m_{\mathrm {T}}} $, after the baseline selection is applied. The shapes of these distributions are similar. These two contributions are the dominant backgrounds in their respective ${m_{\mathrm {T}}}$ regions. The dashed vertical line on the (b) plot indicates the $ {M_J} > $ 400 GeV threshold that separates the signal regions from the control samples. The shaded region corresponding to $ {M_J} < $ 250 GeV is not used in the background estimation. |
png pdf |
Figure 5-a:
Comparison of the ${N_{\text {jets}}}$ distribution, normalized to the same area, in simulated ${\mathrm{ t \bar{t} } } $ events with two true leptons at high ${m_{\mathrm {T}}}$ and one true lepton at low ${m_{\mathrm {T}}} $, after the baseline selection is applied. The shapes of these distributions are similar. These two contributions are the dominant backgrounds in their respective ${m_{\mathrm {T}}}$ regions. |
png pdf |
Figure 5-b:
Comparison of the ${M_J}$ distribution, normalized to the same area, in simulated ${\mathrm{ t \bar{t} } } $ events with two true leptons at high ${m_{\mathrm {T}}}$ and one true lepton at low ${m_{\mathrm {T}}} $, after the baseline selection is applied. The shapes of these distributions are similar. These two contributions are the dominant backgrounds in their respective ${m_{\mathrm {T}}}$ regions. The dashed vertical line on the plot indicates the $ {M_J} > $ 400 GeV threshold that separates the signal regions from the control samples. The shaded region corresponding to $ {M_J} < $ 250 GeV is not used in the background estimation. |
png pdf |
Figure 6:
The ratio $R( {m_{\mathrm {T}}} )$ of high-$ {m_{\mathrm {T}}}$ (R3+R4) to low-$ {m_{\mathrm {T}}}$ (R1+R2) event yields for the simulated SM background, as a function of ${N_{\text {jets}}}$ and ${N_{\text {b}}} $. The baseline selection requires $ {N_{\text {jets}}} \geq$ 6. The uncertainties shown are statistical only. |
png pdf |
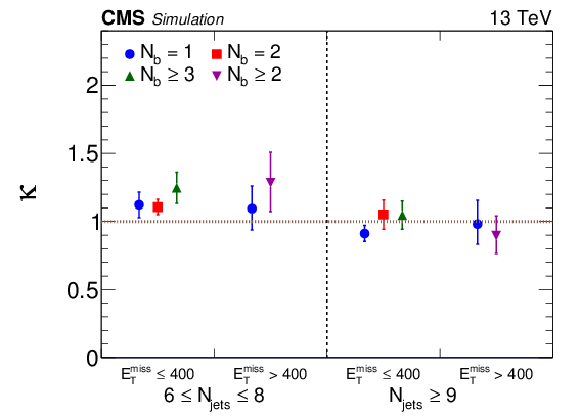
Figure 7:
Values of the double-ratio $\kappa $ in each of the 10 signal bins, calculated using the simulated SM background. The $\kappa $ factors are close to unity, indicating the small correlation between ${M_J} $ and ${m_{\mathrm {T}}} $. The uncertainties shown are statistical only. |
png pdf |
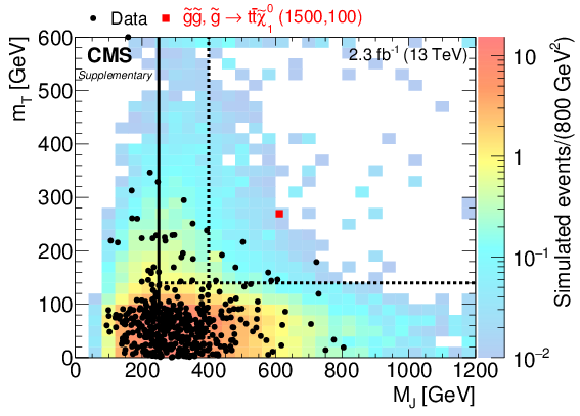
Figure 8:
Two-dimensional distributions for data and simulated event samples in the variables ${m_{\mathrm {T}}}$ and ${M_J}$ in the $ {N_{\text {b}}} \ge$ 2 region after the baseline selection. The distributions integrate over the ${N_{\text {jets}}}$ and ${E_{\mathrm {T}}^{\text {miss}}}$ bins. The black dots are the data; the colored histogram is the total simulated background, normalized to the data; and the red dots are a particular signal sample drawn from the expected distribution for gluino pair production in the T1tttt model with $ {m_{\mathrm{ \tilde{g} } }} =$ 1500 GeV and $m_{ {\tilde{\chi}^0_1} } = $ 100 GeV for 2.3 fb$^{-1}$. Overflow events are shown on the edges of the plot. The definitions of the signal and control regions are the same as those shown in Fig. 4. |
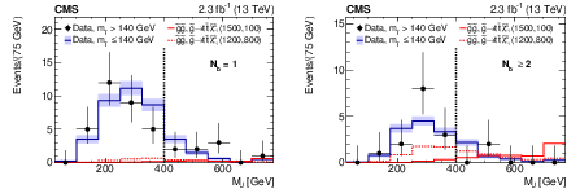
png pdf |
Figure 9:
Comparison of the ${M_J}$ distributions for low- and high-$ {m_{\mathrm {T}}}$ in data with $ {N_{\text {b}}} = $ 1 (left) and $ {N_{\text {b}}} \ge$ 2 (right) after the baseline selection. The expected ${M_J}$ distributions of the two benchmark T1tttt scenarios for $ {m_{\mathrm {T}}} > $ 140 GeV are overlaid. The distributions integrate over the ${N_{\text {jets}}}$ and ${E_{\mathrm {T}}^{\text {miss}}}$ bins. The low-$ {m_{\mathrm {T}}}$ distribution is normalized to the number of events in the high-$ {m_{\mathrm {T}}}$ region. The dashed vertical lines indicate the $ {M_J} > $ 400 GeV threshold that separates the signal regions from the control samples. |
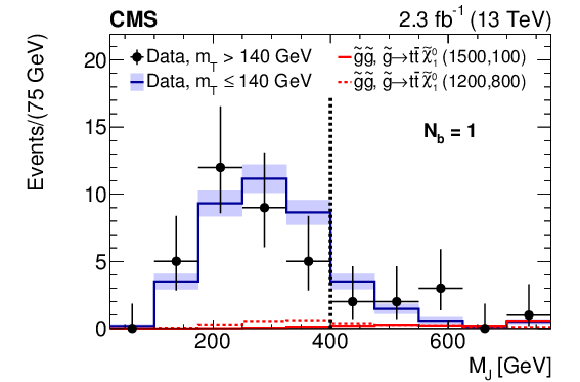
png pdf |
Figure 9-a:
Comparison of the ${M_J}$ distributions for low- and high-$ {m_{\mathrm {T}}}$ in data with $ {N_{\text {b}}} = $ 1 after the baseline selection. The expected ${M_J}$ distributions of the two benchmark T1tttt scenarios for $ {m_{\mathrm {T}}} > $ 140 GeV are overlaid. The distributions integrate over the ${N_{\text {jets}}}$ and ${E_{\mathrm {T}}^{\text {miss}}}$ bins. The low-$ {m_{\mathrm {T}}}$ distribution is normalized to the number of events in the high-$ {m_{\mathrm {T}}}$ region. The dashed vertical lines indicate the $ {M_J} > $ 400 GeV threshold that separates the signal regions from the control samples. |
png pdf |
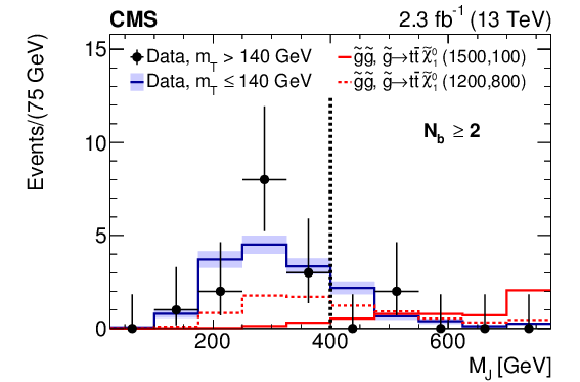
Figure 9-b:
Comparison of the ${M_J}$ distributions for low- and high-$ {m_{\mathrm {T}}}$ in data with $ {N_{\text {b}}} \ge$ 2 after the baseline selection. The expected ${M_J}$ distributions of the two benchmark T1tttt scenarios for $ {m_{\mathrm {T}}} > $ 140 GeV are overlaid. The distributions integrate over the ${N_{\text {jets}}}$ and ${E_{\mathrm {T}}^{\text {miss}}}$ bins. The low-$ {m_{\mathrm {T}}}$ distribution is normalized to the number of events in the high-$ {m_{\mathrm {T}}}$ region. The dashed vertical lines indicate the $ {M_J} > $ 400 GeV threshold that separates the signal regions from the control samples. |
png pdf |
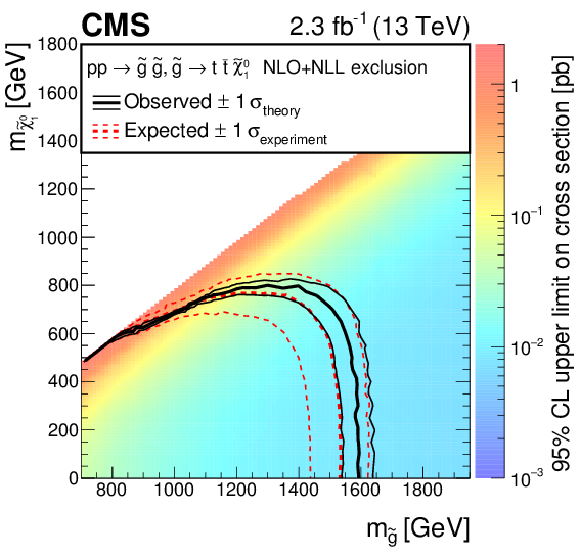
Figure 10:
Interpretation of results in the T1tttt model. The colored regions show the upper limits (95% CL) on the production cross section for $\mathrm{ p } \mathrm{ p } \rightarrow \mathrm{ \tilde{g} } \mathrm{ \tilde{g} } ,\mathrm{ \tilde{g} } \rightarrow {\mathrm{ t \bar{t} } } {\tilde{\chi}^0_1} $ in the $ {m_{\mathrm{ \tilde{g} } }} $-$ {m_{ {\tilde{\chi}^0_1} }} $ plane. The curves show the expected and observed limits on the corresponding SUSY particle masses obtained by comparing the excluded cross section with theoretical cross sections. |
png pdf |
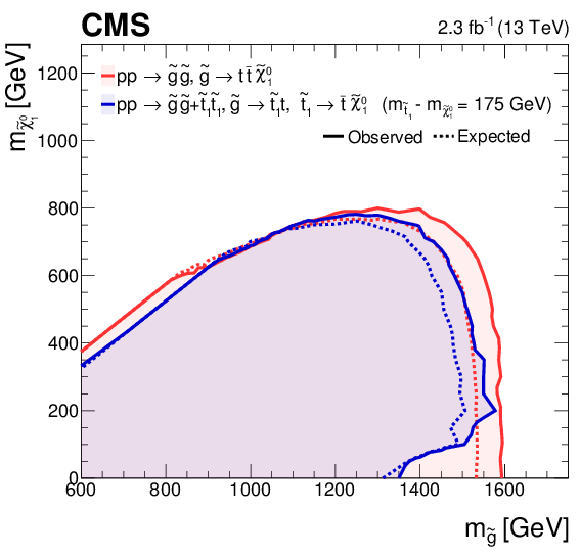
Figure 11:
Excluded region (95% CL), shown in blue, in the $ {m_{\mathrm{ \tilde{g} } }} $-$ {m_{ {\tilde{\chi}^0_1} }} $ plane for a model combining T5tttt, gluino pair production, followed by gluino decay to an on-shell top squark, together with a model for direct top squark pair production. The top squarks decay via the two-body process $\tilde{ mathrm{ t } } \to \mathrm{ t } {\tilde{\chi}^0_1} $. The neutralino and top squark masses are related by the constraint $ {m_{\tilde{ mathrm{ t } } _1}} = {m_{ {\tilde{\chi}^0_1} }}$ + 175 GeV. For comparison, the excluded region (95% CL) from Fig. 10 for the T1tttt model, which has three-body gluino decay, is shown in red. The small difference between the two boundary curves shows that the limits for the scenarios with two-body gluino decay have only a weak dependence on the top squark mass. |
| Tables | |
png pdf |
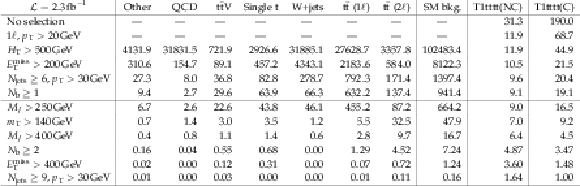
Table 1:
Event yields obtained from simulated event samples, as the event selection criteria are applied. The category Other includes Drell-Yan, $ {\mathrm{ t \bar{t} } } \mathrm{ H } (\rightarrow {\mathrm{ b \bar{b} } } )$, $ {\mathrm{ t \bar{t} } } {\mathrm{ t \bar{t} } } $, $\mathrm{ W } \mathrm{ Z } $, and $\mathrm{ W } \mathrm{ W } $. The yields for ${\mathrm{ t \bar{t} } }$ events in fully hadronic final states are included in the QCD multijet category. The category $ {\mathrm{ t \bar{t} } } {\rm V}$ includes $ {\mathrm{ t \bar{t} } } \mathrm{ W } $, $ {\mathrm{ t \bar{t} } } \mathrm{ Z } $, and $ {\mathrm{ t \bar{t} } } \gamma $. The benchmark signal models, T1tttt(NC) and T1tttt(C), are described in Section 3. The event selection requirements listed above the horizontal line in the middle of the table are defined as the baseline selection. The background estimates before the $ {H_{\mathrm {T}}} $ requirement are not specified because some of the simulated event samples do not extend to the low $ {H_{\mathrm {T}}} $ region. Given the size of the MC samples described in Section 3, rows with zero yield have statistical uncertainties of at most 0.16 events, and below 0.05 events in most cases. |
png pdf |
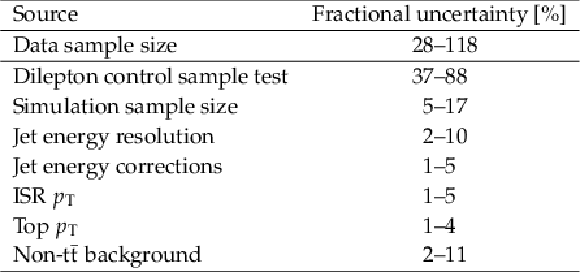
Table 2:
Summary of uncertainties in the background predictions. All entries in the table except for data sample size correspond to a relative uncertainty on $\kappa $. The ranges indicate the spread of each uncertainty across the signal bins. Uncertainties from a particular source are treated as fully correlated across bins, while uncertainties from different sources are treated as uncorrelated. |
png pdf |
Table 3:
Observed and predicted event yields for the signal regions (R4) and background regions (R1-R3) in data (2.3 fb$^{-1}$). Expected yields for the two SUSY T1tttt benchmark scenarios are also given. The results from two types of fits are reported: the predictive fit (PF) and the version of the global fit (GF) performed under the assumption of the null hypothesis ($r= $ 0 ). The predictive fit uses the observed yields in regions R1, R2, and R3 only and is effectively just a propagation of uncertainties. The global fit uses all four regions. The values of $\kappa $ obtained from the simulation fit are also listed. The first uncertainty in $\kappa $ is statistical, while the second corresponds to the total systematic uncertainty. The benchmark signal models, T1tttt(NC) and T1tttt(C), are described in Section 3. |
png pdf |
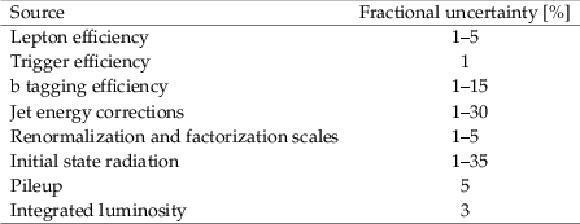
Table 4:
Typical values of the signal-related systematic uncertainties. Uncertainties due to a particular source are treated as fully correlated between bins, while uncertainties due to different sources are treated as uncorrelated. |
| Summary |
|
Using a sample of proton-proton collisions at $\sqrt s = $ 13 GeV with an integrated luminosity of 2.3 fb$^{-1}$, a search for supersymmetry is performed in the final state with a single lepton, b-tagged jets, and large missing transverse momentum. The search focuses on final states resulting from the pair production of gluinos, which subsequently decay via $\mathrm{ \tilde{g} }\to\mathrm{ t \bar{t} }{\tilde{\chi}^0_1} $, leading to high jet multiplicities. A key feature of the analysis is the use of the variable ${M_J} $, the sum of the masses of large-$R$ jets, which are formed by clustering anti-$k_{\mathrm{T}}$ $R= $ 0.4 jets and leptons. Used in conjunction with the variable ${m_{\mathrm{T}}} $, the transverse mass of the system consisting of the lepton and the missing transverse momentum vector, ${M_J} $ provides a powerful background estimation method that is well suited to this high jet multiplicity search. After the baseline selection is applied, signal (R4) and control regions (R1, R2, and R3) are defined in the ${M_J} $-${m_{\mathrm{T}}} $ plane, which are further divided into bins of $E_{\mathrm{T}}^{\text{miss}}$, ${N_{\text{jets}}} $, and ${N_{\text{b}}} $ to provide additional sensitivity. In regions R3 and R4, the requirement ${m_{\mathrm{T}}} > $ 140 GeV provides strong suppression of the single-lepton $\mathrm{ t \bar{t} }$ background, so that dilepton $\mathrm{ t \bar{t} }$ events dominate over all other background sources. For these dilepton events to enter a signal region, however, they must contain a substantial amount of initial-state radiation (ISR). For this extreme range of ISR jet momentum and multiplicity, the single-lepton and dilepton $\mathrm{ t \bar{t} }$ events have very similar kinematic properties. The variables ${M_J} $ and ${m_{\mathrm{T}}} $ are nearly uncorrelated, even though different processes dominate the low- and high-${m_{\mathrm{T}}} $ regions. As a consequence, the low-${m_{\mathrm{T}}} $ regions (R1 and R2) can be used to measure the background shape for the ${M_J} $ distribution at high ${m_{\mathrm{T}}} $. A correction factor, near unity, is taken from simulation and is used to account for a possible correlation between ${M_J} $ and ${m_{\mathrm{T}}} $. The observed event yields in the signal regions are consistent with the predictions for the SM background contributions, and exclusion limits are set on the gluino pair production cross sections in the ${m_{\mathrm{ \tilde{g} }}} $-${m_{{\tilde{\chi}^0_1} }} $ plane, as described by the simplified models T1tttt and T5tttt, where the latter is augmented with a model of direct top squark pair production for consistency. In the T1tttt model, gluinos decay via the three-body process $\mathrm{ \tilde{g} }\to\mathrm{ t \bar{t} }{\tilde{\chi}^0_1} $, which proceeds via a virtual top squark in the intermediate state. Under the assumption of a 100% branching fraction to this final state, the cross section limit for each model point is compared with the theoretical cross section to determine the excluded particle masses. Gluinos with a mass below 1600 GeV are excluded at a 95% CL for scenarios with low $\tilde\chi_1^0$ mass, and neutralinos with a mass below 800 GeV are excluded for a gluino mass of about 1300 GeV. In the T5tttt model, the top squark is lighter than the gluino, which therefore decays via a two-body process. The boundary of the excluded region in the ${m_{\mathrm{ \tilde{g} }}} $-${m_{{\tilde{\chi}^0_1} }} $ plane for T5tttt is found to be only weakly sensitive to the top squark mass. These results significantly extend the sensitivity of single-lepton searches based on data at $\sqrt s = $ 8 GeV. |
| Additional Figures | |
png pdf |
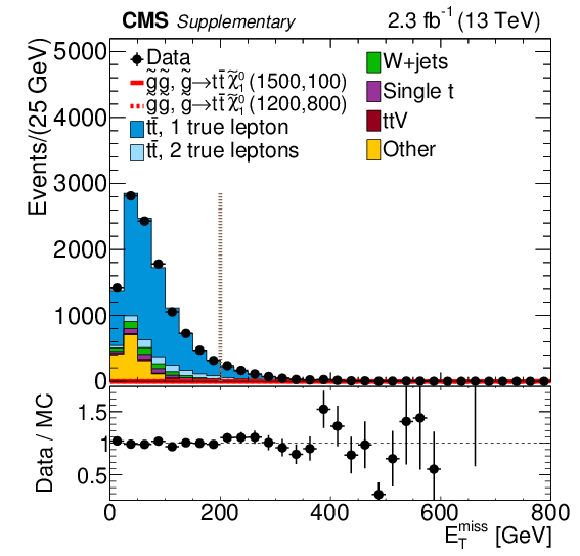
Additional Figure 1-a:
Distribution of ${E_{\mathrm {T}}^{\text {miss}}}$ in data and simulated event samples after the baseline selection is applied (except for the ${E_{\mathrm {T}}^{\text {miss}}}$ requirement) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {E_{\mathrm {T}}^{\text {miss}}} > $ 200 GeV baseline requirement. |
png pdf |
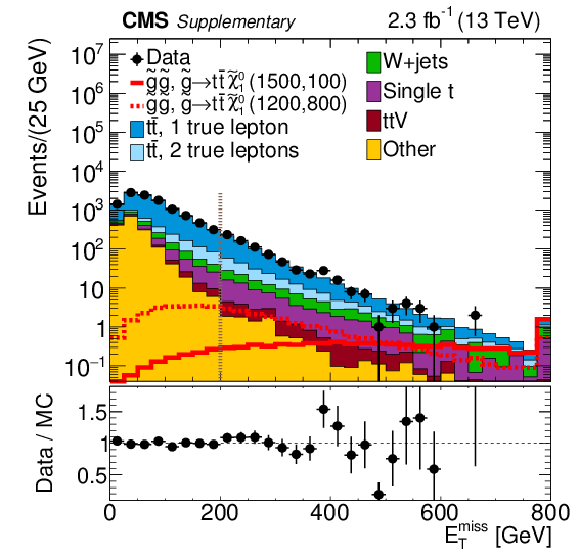
Additional Figure 1-b:
Distribution of ${E_{\mathrm {T}}^{\text {miss}}}$ in data and simulated event samples after the baseline selection is applied (except for the ${E_{\mathrm {T}}^{\text {miss}}}$ requirement) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {E_{\mathrm {T}}^{\text {miss}}} > $ 200 GeV baseline requirement. |
png pdf |
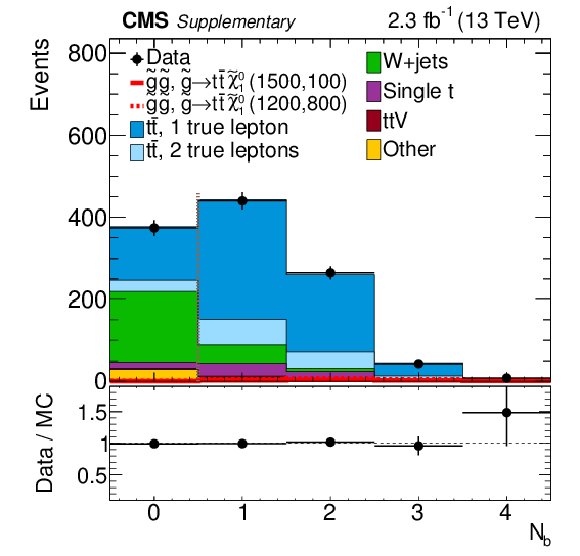
Additional Figure 2-a:
Distribution of $ {N_{\text {b}}}$ in data and simulated event samples after the baseline selection is applied (except for the $ {N_{\text {b}}}$ requirement) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {N_{\text {b}}} \geq 1$ baseline requirement. |
png pdf |
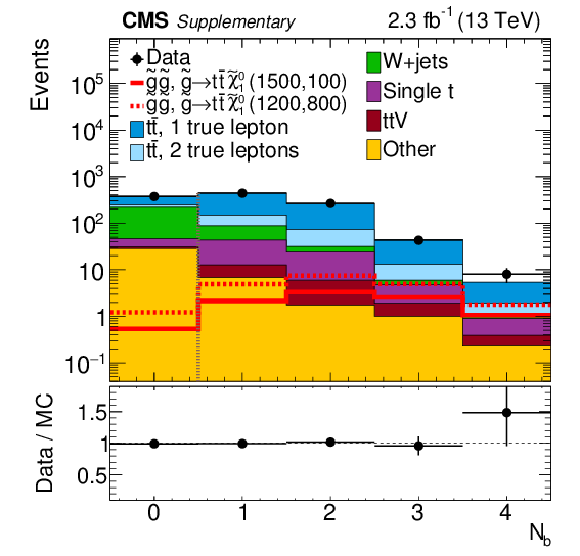
Additional Figure 2-b:
Distribution of $ {N_{\text {b}}}$ in data and simulated event samples after the baseline selection is applied (except for the $ {N_{\text {b}}}$ requirement) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {N_{\text {b}}} \geq 1$ baseline requirement. |
png pdf |
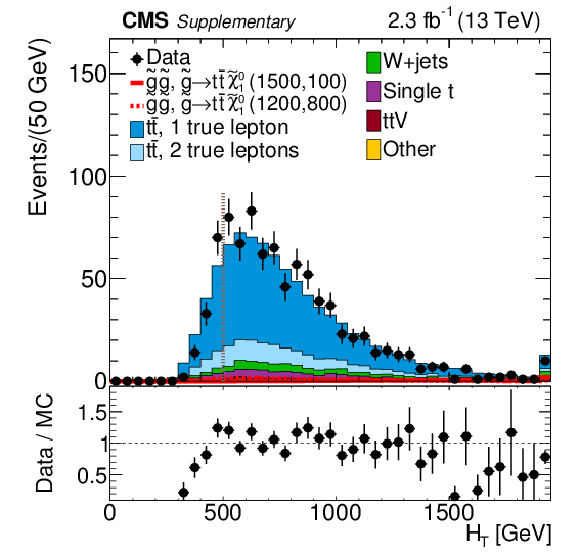
Additional Figure 3-a:
Distribution of ${H_{\mathrm {T}}}$ in data and simulated event samples after the baseline selection is applied (except for the ${H_{\mathrm {T}}}$ requirement) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. For low ${H_{\mathrm {T}}}$, the data points go below the simulation due to trigger inefficiency. The dashed vertical line indicates the $ {H_{\mathrm {T}}} > $ 500 GeV baseline requirement that ensures trigger plateau efficiency. |
png pdf |
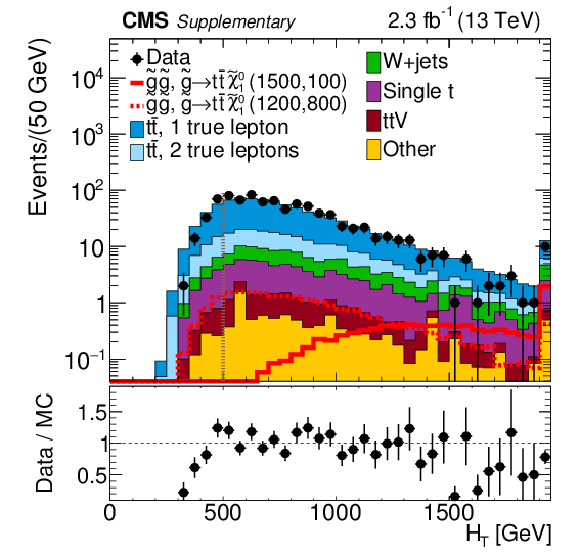
Additional Figure 3-b:
Distribution of ${H_{\mathrm {T}}}$ in data and simulated event samples after the baseline selection is applied (except for the ${H_{\mathrm {T}}}$ requirement) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. For low ${H_{\mathrm {T}}}$, the data points go below the simulation due to trigger inefficiency. The dashed vertical line indicates the $ {H_{\mathrm {T}}} > $ 500 GeV baseline requirement that ensures trigger plateau efficiency. |
png pdf |
Additional Figure 4-a:
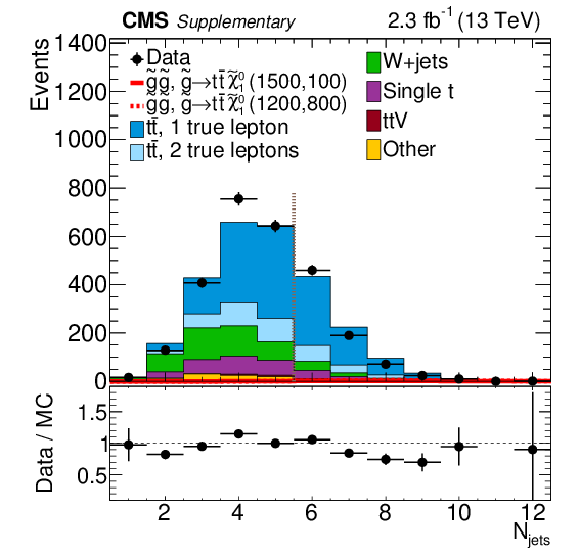
Distribution of ${N_{\text {jets}}}$ in data and simulated event samples after the baseline selection is applied (except for the ${N_{\text {jets}}}$ requirement) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {N_{\text {jets}}} \geq 6$ baseline requirement. |
png pdf |
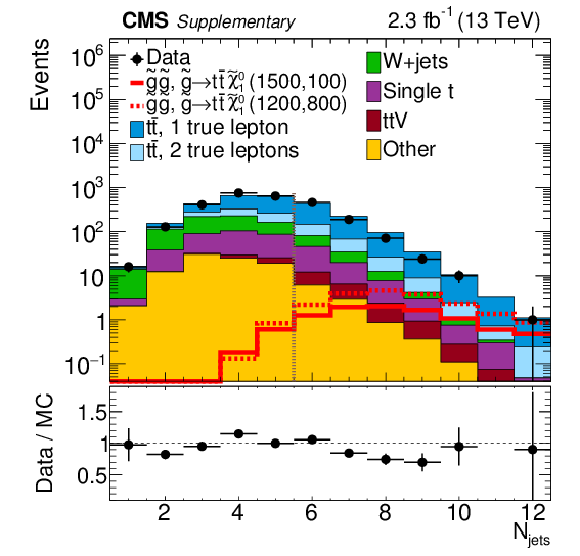
Additional Figure 4-b:
Distribution of ${N_{\text {jets}}}$ in data and simulated event samples after the baseline selection is applied (except for the ${N_{\text {jets}}}$ requirement) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {N_{\text {jets}}} \geq 6$ baseline requirement. |
png pdf |
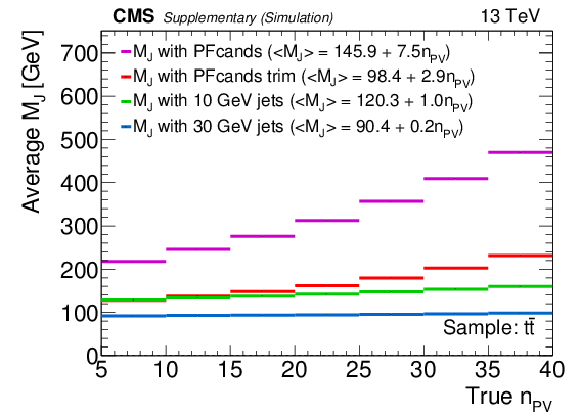
Additional Figure 5-a:
Average ${M_J}$ as a function of the number of primary vertices for ${\mathrm{ t } \mathrm{ \bar{t} } }$ and a signal model. ${M_J}$ is shown as calculated from all CHS PF candidates (magenta), all CHS PF candidates plus trimming (red), AK4 PF jets with $p_T> $ 10 GeV (green), or AK4 PF jets with $p_T> $ 30 GeV (blue). The legend includes the results of linear fits to each set of points, showing that for the choice of 30 GeV jets, the PU dependence is very small. |
png pdf |
Additional Figure 5-b:
Average ${M_J}$ as a function of the number of primary vertices for ${\mathrm{ t } \mathrm{ \bar{t} } }$ and a signal model. ${M_J}$ is shown as calculated from all CHS PF candidates (magenta), all CHS PF candidates plus trimming (red), AK4 PF jets with $p_T> $ 10 GeV (green), or AK4 PF jets with $p_T> $ 30 GeV (blue). The legend includes the results of linear fits to each set of points, showing that for the choice of 30 GeV jets, the PU dependence is very small. |
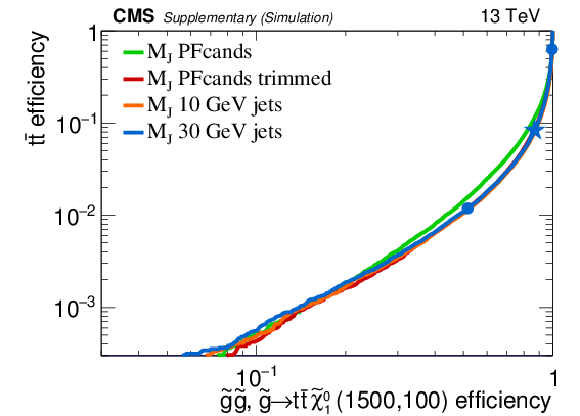
png pdf |
Additional Figure 6-a:
Comparison of performance (signal and background efficiencies) for ${M_J}$ made with all PF candidates (with and without trimming) and jets (with minimum ${p_{\mathrm {T}}}$ 10 and 30 GeV) after $ {H_{\mathrm {T}}} > $ 500 GeV and $ {E_{\mathrm {T}}^{\text {miss}}} > $ 200 GeV requirements. The performance is similar for all options, so we choose to cluster calibrated 30 GeV jets which have the smallest PU-dependence. The blue star corresponds to $ {M_J} > $ 400 GeV and the blue circles to $ {M_J} > [200,600]$ GeV. |
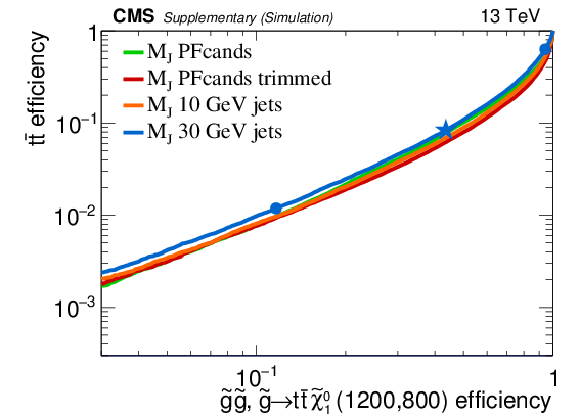
png pdf |
Additional Figure 6-b:
Comparison of performance (signal and background efficiencies) for ${M_J}$ made with all PF candidates (with and without trimming) and jets (with minimum ${p_{\mathrm {T}}}$ 10 and 30 GeV) after $ {H_{\mathrm {T}}} > $ 500 GeV and $ {E_{\mathrm {T}}^{\text {miss}}} > $ 200 GeV requirements. The performance is similar for all options, so we choose to cluster calibrated 30 GeV jets which have the smallest PU-dependence. The blue star corresponds to $ {M_J} > $ 400 GeV and the blue circles to $ {M_J} > [200,600]$ GeV. |
png pdf |
Additional Figure 7-a:
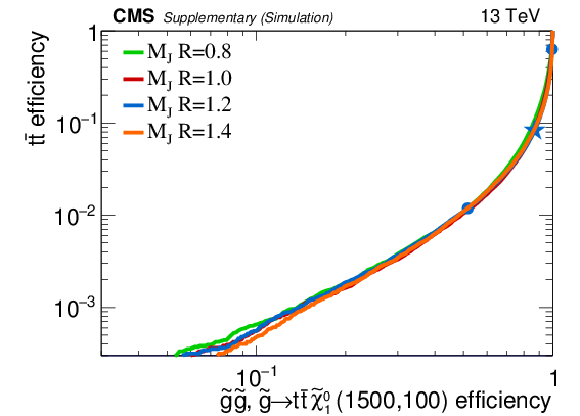
Comparison of performance (signal and background efficiencies) for ${M_J}$ made of large-$R$ jets for various values of $R$ after $ {H_{\mathrm {T}}} > $ 500 GeV and $ {E_{\mathrm {T}}^{\text {miss}}} > $ 200 GeV requirements. The blue star corresponds to $ {M_J} > $ 400 GeV and the blue circles to $ {M_J} > [200,600]$ GeV. For the compressed point, larger $R$ gives better performance, but it saturates at about $R= $ 1.2. |
png pdf |
Additional Figure 7-b:
Comparison of performance (signal and background efficiencies) for ${M_J}$ made of large-$R$ jets for various values of $R$ after $ {H_{\mathrm {T}}} > $ 500 GeV and $ {E_{\mathrm {T}}^{\text {miss}}} > $ 200 GeV requirements. The blue star corresponds to $ {M_J} > $ 400 GeV and the blue circles to $ {M_J} > [200,600]$ GeV. For the compressed point, larger $R$ gives better performance, but it saturates at about $R= $ 1.2. |
png pdf |
Additional Figure 8-a:
Comparison of performance (signal and background efficiencies) for ${H_{\mathrm {T}}}$, ${N_{\text {jets}}}$, ${M_J}$, and combination of these variables using boosted decision trees (BDTs) after $ {H_{\mathrm {T}}} > $ 500 GeV and $ {E_{\mathrm {T}}^{\text {miss}}} > $ 200 GeV requirements. The red star corresponds to $ {H_{\mathrm {T}}} > $ 1000 GeV and the red circles to $ {H_{\mathrm {T}}} > [1500,2000]$ GeV. The orange star corresponds to $ {N_{\text {jets}}} \geq 9$ and the orange circles to $ {N_{\text {jets}}} > [6,11]$. The blue star corresponds to $ {M_J} > $ 400 GeV and the blue circles to $ {M_J} > [200,600,800]$ GeV. The BDT curves do not extend all the way to the bottom-left corner due to limited MC statistics for the BDT training. The performance of ${M_J}$ alone is superior to that of ${H_{\mathrm {T}}}$, but the combination of ${M_J}$ and ${N_{\text {jets}}}$ has similar performance as the combination of ${H_{\mathrm {T}}}$ and ${N_{\text {jets}}}$. |
png pdf |
Additional Figure 8-b:
Comparison of performance (signal and background efficiencies) for ${H_{\mathrm {T}}}$, ${N_{\text {jets}}}$, ${M_J}$, and combination of these variables using boosted decision trees (BDTs) after $ {H_{\mathrm {T}}} > $ 500 GeV and $ {E_{\mathrm {T}}^{\text {miss}}} > $ 200 GeV requirements. The red star corresponds to $ {H_{\mathrm {T}}} > $ 1000 GeV and the red circles to $ {H_{\mathrm {T}}} > [1500,2000]$ GeV. The orange star corresponds to $ {N_{\text {jets}}} \geq 9$ and the orange circles to $ {N_{\text {jets}}} > [6,11]$. The blue star corresponds to $ {M_J} > $ 400 GeV and the blue circles to $ {M_J} > [200,600,800]$ GeV. The BDT curves do not extend all the way to the bottom-left corner due to limited MC statistics for the BDT training. The performance of ${M_J}$ alone is superior to that of ${H_{\mathrm {T}}}$, but the combination of ${M_J}$ and ${N_{\text {jets}}}$ has similar performance as the combination of ${H_{\mathrm {T}}}$ and ${N_{\text {jets}}}$. |
png pdf |
Additional Figure 9-a:
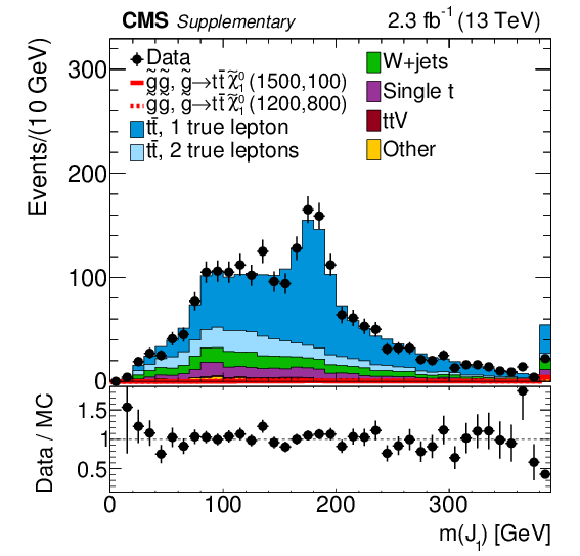
Distribution of $m(J_{1})$ in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
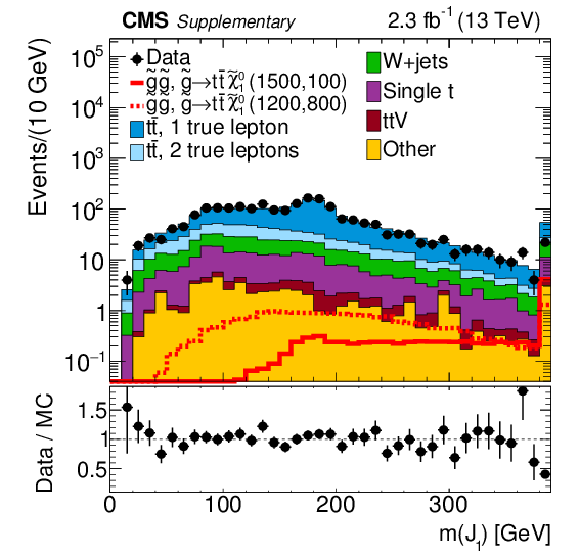
png pdf |
Additional Figure 9-b:
Distribution of $m(J_{1})$ in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
png pdf |
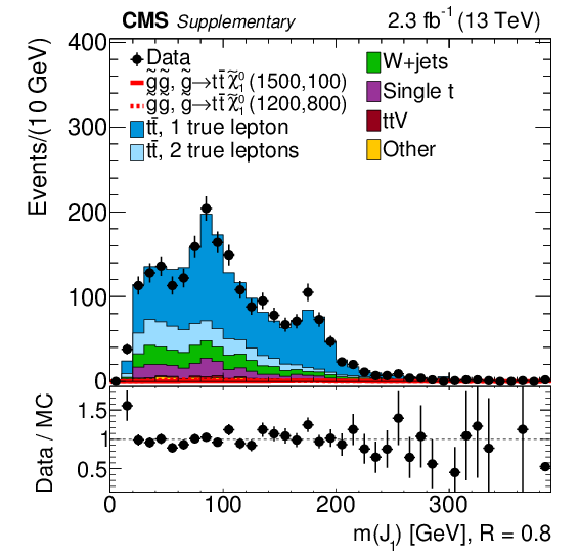
Additional Figure 10-a:
Distribution of $m(J_{1})$ clustered with radius R = 0.8, in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
png pdf |
Additional Figure 10-b:
Distribution of $m(J_{1})$ clustered with radius R = 0.8, in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
png pdf |
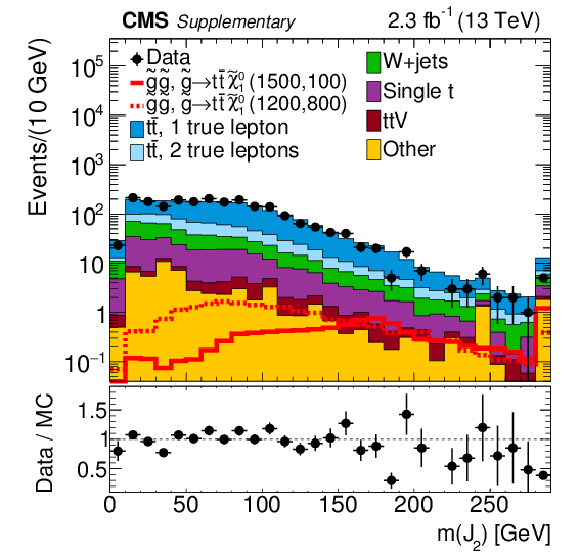
Additional Figure 11-a:
Distribution of $m(J_{2})$ in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
png pdf |
Additional Figure 11-b:
Distribution of $m(J_{2})$ in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
png pdf |
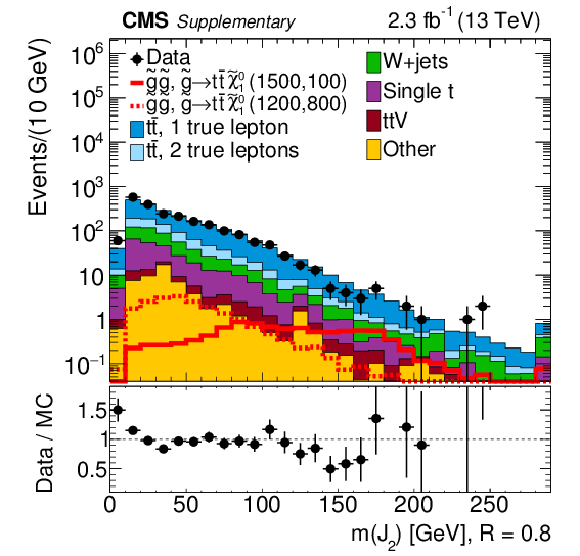
Additional Figure 12-a:
Distribution of $m(J_{2})$ clustered with radius R = 0.8, in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
png pdf |
Additional Figure 12-b:
Distribution of $m(J_{2})$ clustered with radius R = 0.8, in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
png pdf |
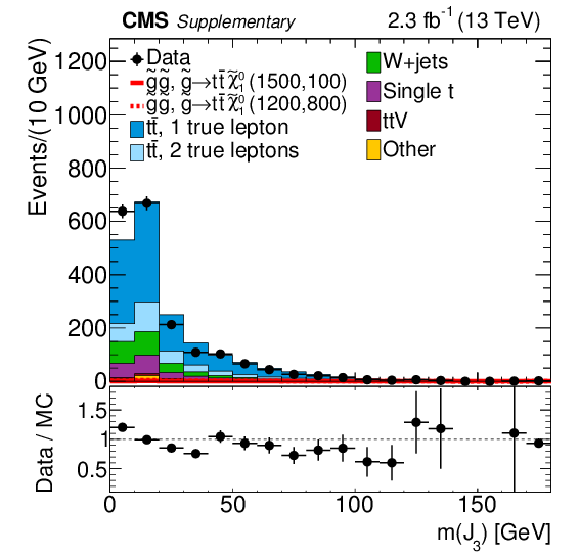
Additional Figure 13-a:
Distribution of $m(J_{3})$ in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
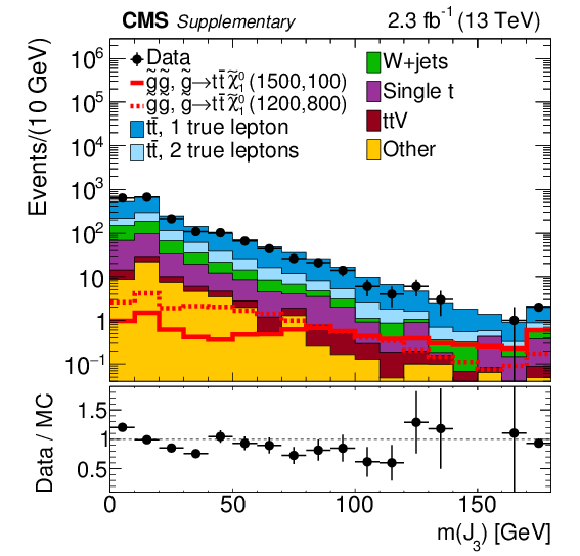
png pdf |
Additional Figure 13-b:
Distribution of $m(J_{3})$ in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
png pdf |
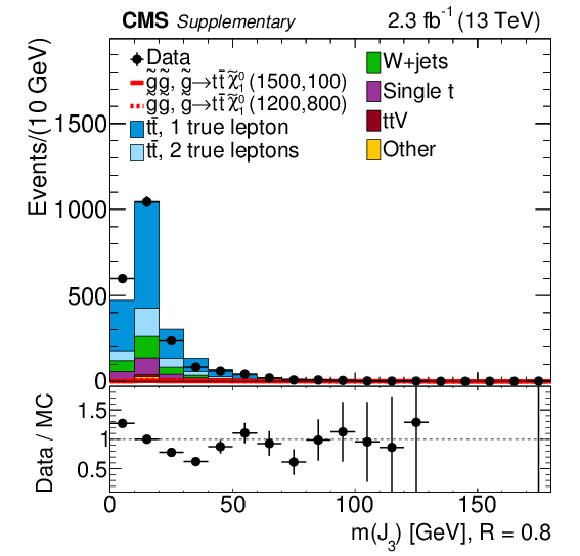
Additional Figure 14-a:
Distribution of $m(J_{3})$ clustered with radius $R =$ 0.8, in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
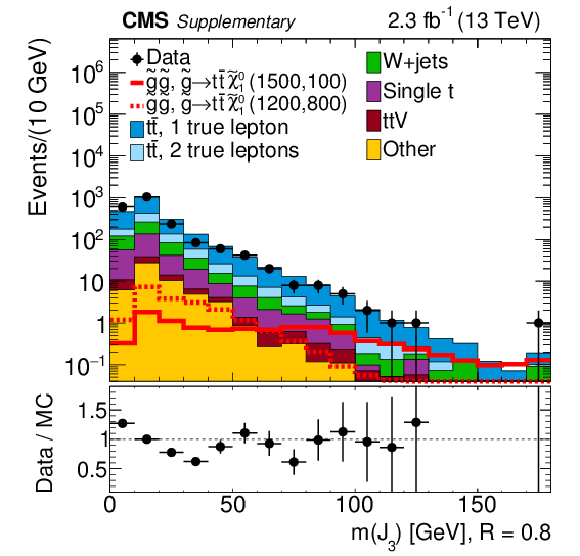
png pdf |
Additional Figure 14-b:
Distribution of $m(J_{3})$ clustered with radius $R =$ 0.8, in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
png pdf |
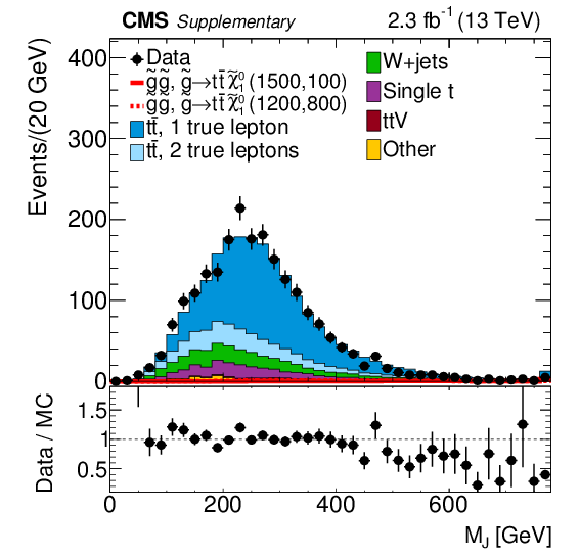
Additional Figure 15-a:
Distribution of ${M_J}$ in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
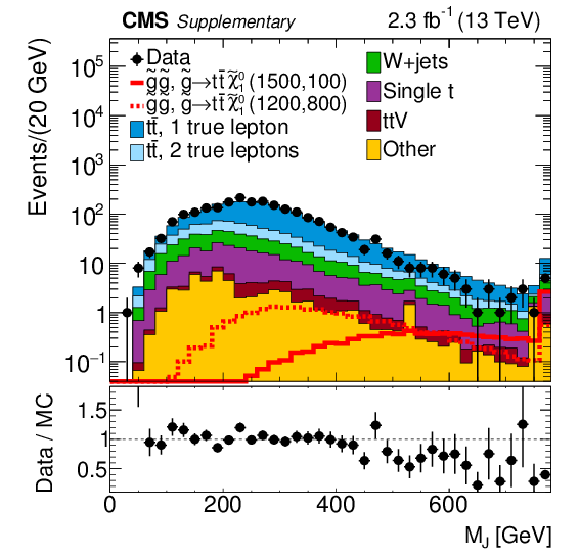
png pdf |
Additional Figure 15-b:
Distribution of ${M_J}$ in data and simulated event samples after the baseline selection (with the ${N_{\text {jets}}}$ requirement relaxed to $ {N_{\text {jets}}} \geq 4$) is applied in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
png pdf |
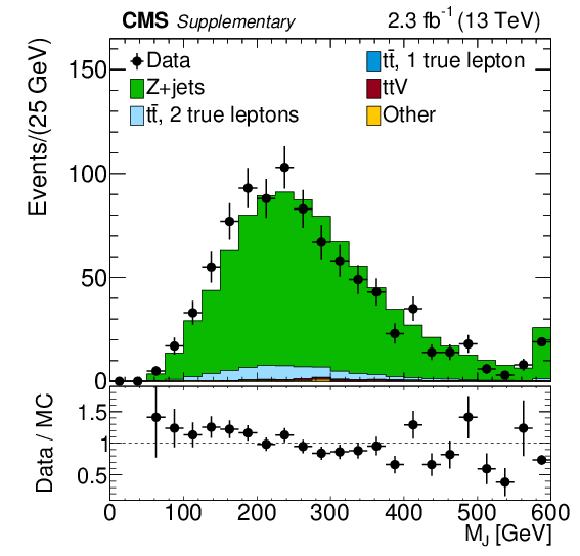
Additional Figure 16-a:
Distribution of ${M_J}$ in data and simulated event samples after a selection targetting dilepton Z+jets events is applied in linear (a) and logarithmic (b) scales. The selection requires a pair of opposite sign, same flavor leptons with invariant mass between 80 and 100 GeV, as well as $ {H_{\mathrm {T}}} > $ 500 and $ {N_{\text {jets}}} \geq 4$. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
png pdf |
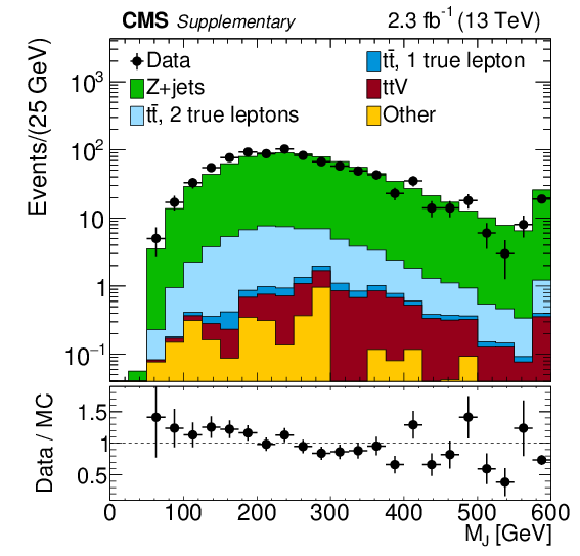
Additional Figure 16-b:
Distribution of ${M_J}$ in data and simulated event samples after a selection targetting dilepton Z+jets events is applied in linear (a) and logarithmic (b) scales. The selection requires a pair of opposite sign, same flavor leptons with invariant mass between 80 and 100 GeV, as well as $ {H_{\mathrm {T}}} > $ 500 and $ {N_{\text {jets}}} \geq 4$. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. |
png pdf |
Additional Figure 17-a:
Distribution of $m(J_{1})$ in data and simulated event samples after a selection targetting dilepton Z+jets events is applied in linear (a) and logarithmic (b) scales. The selection requires a pair of opposite sign, same flavor leptons with invariant mass between 80 and 100 GeV, as well as $ {H_{\mathrm {T}}} > $ 500 and $ {N_{\text {jets}}} \geq 4$. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The peak at around 100 GeV corresponds to the case of the two jets associated with the leptons coming from the Z boson being clustered in the same large-$R$ jet. |
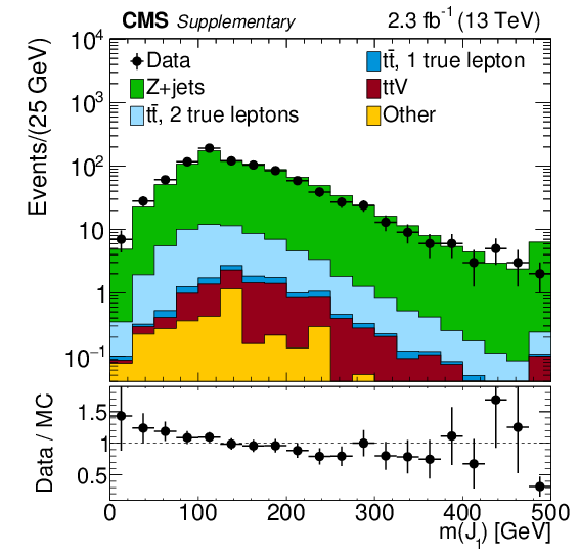
png pdf |
Additional Figure 17-b:
Distribution of $m(J_{1})$ in data and simulated event samples after a selection targetting dilepton Z+jets events is applied in linear (a) and logarithmic (b) scales. The selection requires a pair of opposite sign, same flavor leptons with invariant mass between 80 and 100 GeV, as well as $ {H_{\mathrm {T}}} > $ 500 and $ {N_{\text {jets}}} \geq 4$. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The peak at around 100 GeV corresponds to the case of the two jets associated with the leptons coming from the Z boson being clustered in the same large-$R$ jet. |
png pdf |
Additional Figure 18-a:
Distribution of ${m_{\mathrm {T}}}$ in signal (red) and simulated ${\mathrm{ t } \mathrm{ \bar{t} } }$ samples (blue) after the baseline selection is applied in linear (a) and logarithmic (b) scales. |
png pdf |
Additional Figure 18-b:
Distribution of ${m_{\mathrm {T}}}$ in signal (red) and simulated ${\mathrm{ t } \mathrm{ \bar{t} } }$ samples (blue) after the baseline selection is applied in linear (a) and logarithmic (b) scales. |
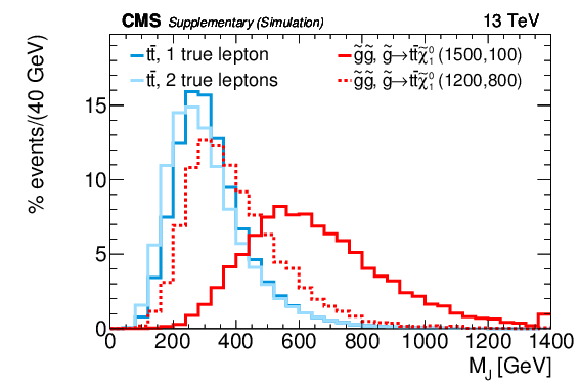
png pdf |
Additional Figure 19-a:
Distribution of ${M_J}$ in signal (red) and simulated ${\mathrm{ t } \mathrm{ \bar{t} } }$ samples (blue) after the baseline selection is applied in linear (a) and logarithmic (b) scales. |
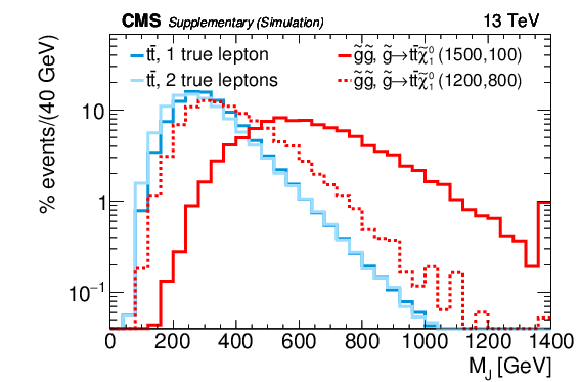
png pdf |
Additional Figure 19-b:
Distribution of ${M_J}$ in signal (red) and simulated ${\mathrm{ t } \mathrm{ \bar{t} } }$ samples (blue) after the baseline selection is applied in linear (a) and logarithmic (b) scales. |
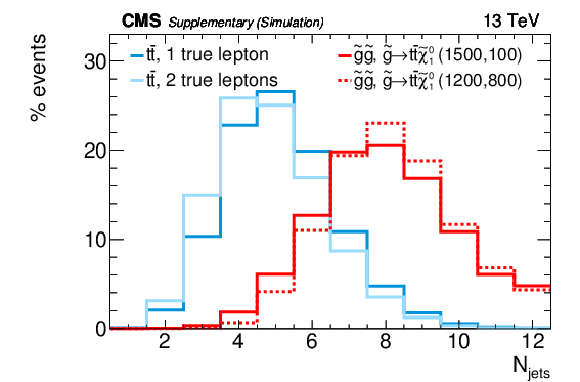
png pdf |
Additional Figure 20-a:
Distribution of ${N_{\text {jets}}}$ in signal (red) and simulated ${\mathrm{ t } \mathrm{ \bar{t} } }$ samples (blue) after the baseline selection is applied in linear (a) and logarithmic (b) scales. |
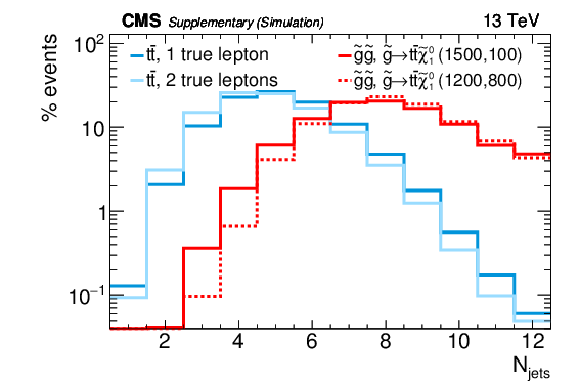
png pdf |
Additional Figure 20-b:
Distribution of ${N_{\text {jets}}}$ in signal (red) and simulated ${\mathrm{ t } \mathrm{ \bar{t} } }$ samples (blue) after the baseline selection is applied in linear (a) and logarithmic (b) scales. |
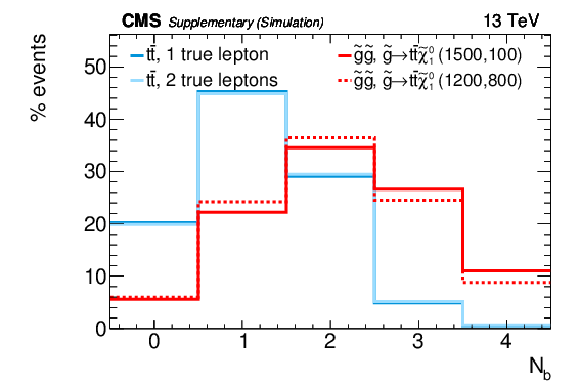
png pdf |
Additional Figure 21-a:
Distribution of $ {N_{\text {b}}}$ in signal (red) and simulated ${\mathrm{ t } \mathrm{ \bar{t} } }$ samples (blue) after the baseline selection is applied in linear (a) and logarithmic (b) scales. |
png pdf |
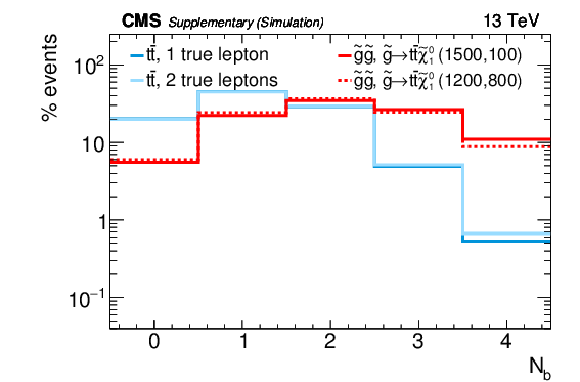
Additional Figure 21-b:
Distribution of $ {N_{\text {b}}}$ in signal (red) and simulated ${\mathrm{ t } \mathrm{ \bar{t} } }$ samples (blue) after the baseline selection is applied in linear (a) and logarithmic (b) scales. |
png pdf |
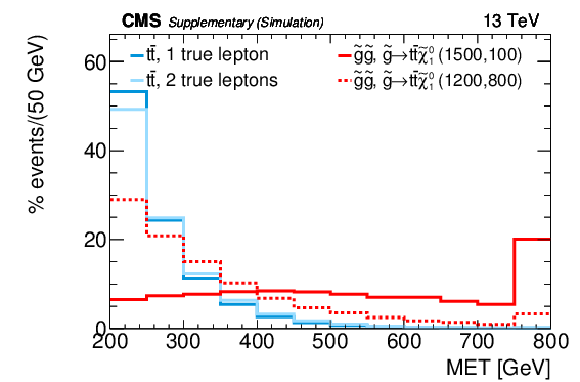
Additional Figure 22-a:
Distribution of ${E_{\mathrm {T}}^{\text {miss}}}$ in signal (red) and simulated ${\mathrm{ t } \mathrm{ \bar{t} } }$ samples (blue) after the baseline selection is applied in linear (a) and logarithmic (b) scales. |
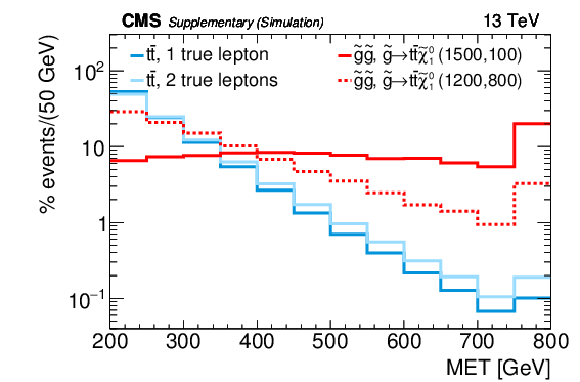
png pdf |
Additional Figure 22-b:
Distribution of ${E_{\mathrm {T}}^{\text {miss}}}$ in signal (red) and simulated ${\mathrm{ t } \mathrm{ \bar{t} } }$ samples (blue) after the baseline selection is applied in linear (a) and logarithmic (b) scales. |
png pdf |
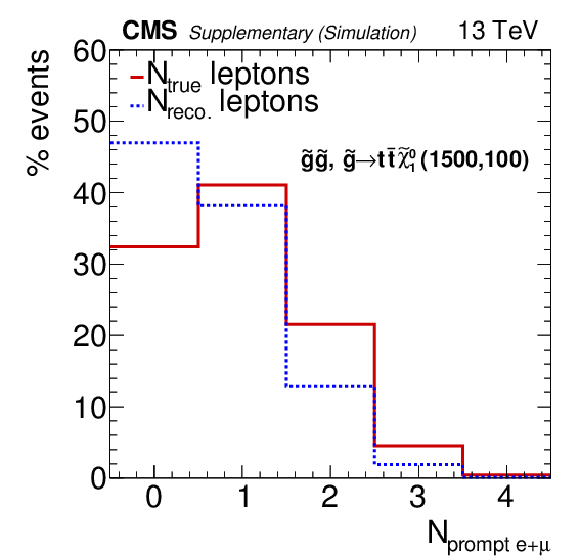
Additional Figure 23:
Distribution of the number of true e, $\mu $, and $\tau \rightarrow \mathrm{e}/\mu $ coming from W bosons (red) and reconstructed leptons (dashed blue) in gluino pair production events, with $\tilde{\mathrm{g}} \to {\mathrm{ t } \mathrm{ \bar{t} } } \tilde{\chi}^0_1 $, for $ {m_{\tilde{\mathrm{g}} }} = $ 1500 GeV and $ {m_{ \tilde{\chi}^0_1 }} = $ 100 GeV. |
png pdf |
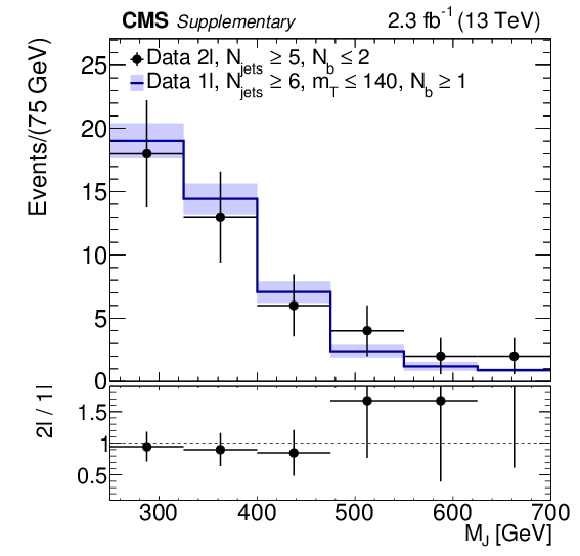
Additional Figure 24:
Distribution of ${M_J}$ for a single-lepton selection with $ {m_{\mathrm {T}}} < $ 140 GeV (histogram) and a dilepton selection (points). To increase statistics, dilepton events with $ {N_{\text {b}}} = $ 0 are included. To avoid signal contamination, dilepton events with $ {N_{\text {b}}} \geq 3$ are excluded and a cut $ {E_{\mathrm {T}}^{\text {miss}}} < $ 400 is applied to all events. The overall yield from the single-lepton sample is normalized to that for the dilepton sample to compare shapes. |
png pdf |
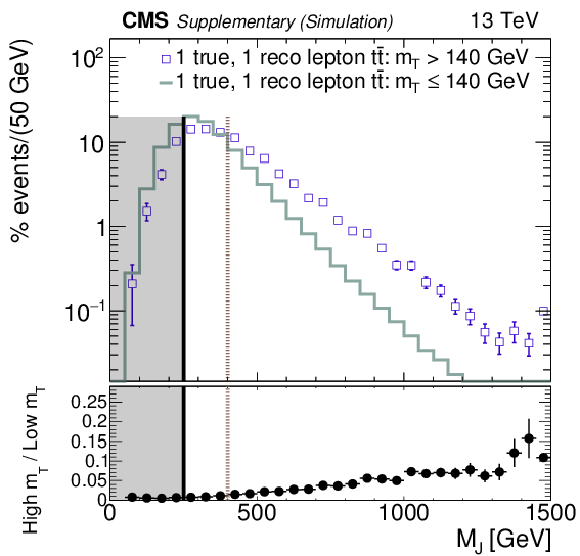
Additional Figure 25:
Comparison of the ${M_J}$ shape after the baseline selection in ${\mathrm{ t } \mathrm{ \bar{t} } }$ events with 1 true lepton at low and high ${m_{\mathrm {T}}}$. The different ${M_J}$ shape at high ${m_{\mathrm {T}}}$ is produced predominantly from the mismeasurement of jets. While this difference can introduce a correlation between ${M_J}$ and ${m_{\mathrm {T}}}$, single-lepton events at high ${m_{\mathrm {T}}}$ are only a small component (${\sim}$ 15%) of the full high ${m_{\mathrm {T}}}$ background. |
png pdf |
Additional Figure 26:
The ISR ${p_{\mathrm {T}}}$ distribution in a dilepton ${\mathrm{ t } \mathrm{ \bar{t} } }$ sample. This sample is selected by requiring exactly two leptons and two b-tagged jets. The momenta of the remaining jets are summed vectorially to form the ${p_{\mathrm {T}}}$ of the ISR for the event. |
png pdf |
Additional Figure 27-a:
Distribution of ${m_{\mathrm {T}}}$ in data and simulated event samples in the R2 and R4 region in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {m_{\mathrm {T}}} > $ 140 GeV requirement for the signal region R4. |
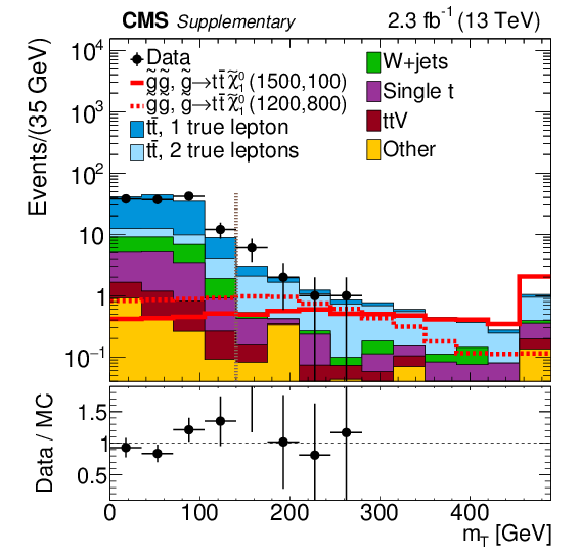
png pdf |
Additional Figure 27-b:
Distribution of ${m_{\mathrm {T}}}$ in data and simulated event samples in the R2 and R4 region in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {m_{\mathrm {T}}} > $ 140 GeV requirement for the signal region R4. |
png pdf |
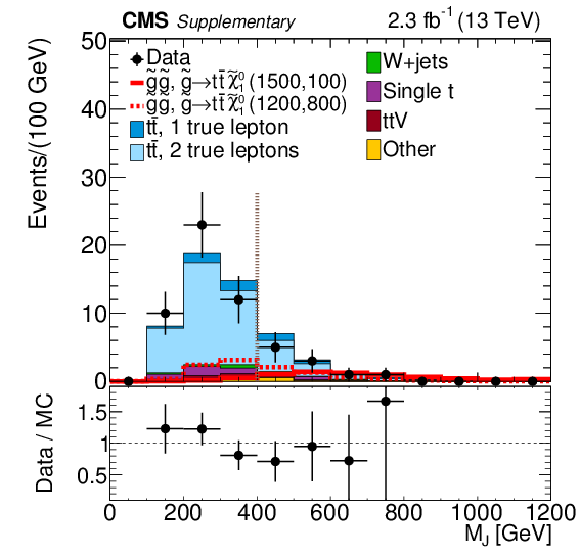
Additional Figure 28-a:
Distribution of ${M_J}$ in data and simulated event samples in the R3 and R4 regions in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {M_J} > $ 400 GeV requirement for the signal region R4. |
png pdf |
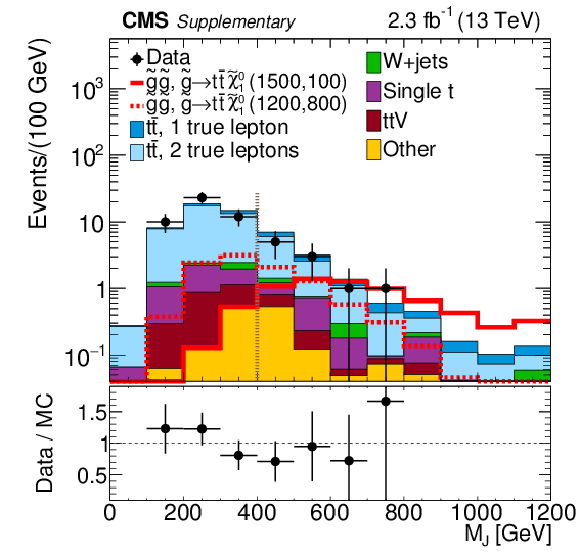
Additional Figure 28-b:
Distribution of ${M_J}$ in data and simulated event samples in the R3 and R4 regions in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {M_J} > $ 400 GeV requirement for the signal region R4. |
png pdf |
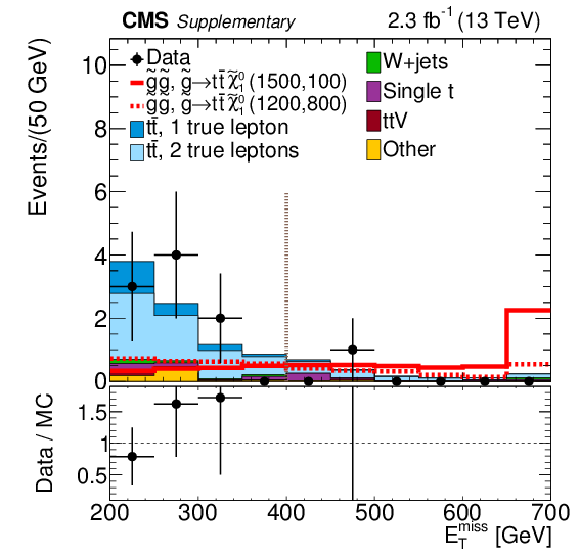
Additional Figure 29-a:
Distribution of ${E_{\mathrm {T}}^{\text {miss}}}$ in data and simulated event samples in the signal region R4 (baseline selection plus $ {m_{\mathrm {T}}} > $ 140 GeV and $ {M_J} > $ 400 GeV) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {E_{\mathrm {T}}^{\text {miss}}} > $ 400 GeV requirement for the tightest ${E_{\mathrm {T}}^{\text {miss}}}$ bin. |
png pdf |
Additional Figure 29-b:
Distribution of ${E_{\mathrm {T}}^{\text {miss}}}$ in data and simulated event samples in the signal region R4 (baseline selection plus $ {m_{\mathrm {T}}} > $ 140 GeV and $ {M_J} > $ 400 GeV) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {E_{\mathrm {T}}^{\text {miss}}} > $ 400 GeV requirement for the tightest ${E_{\mathrm {T}}^{\text {miss}}}$ bin. |
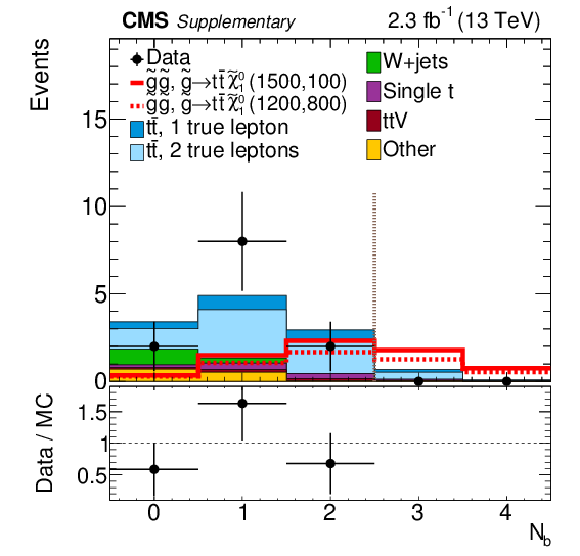
png pdf |
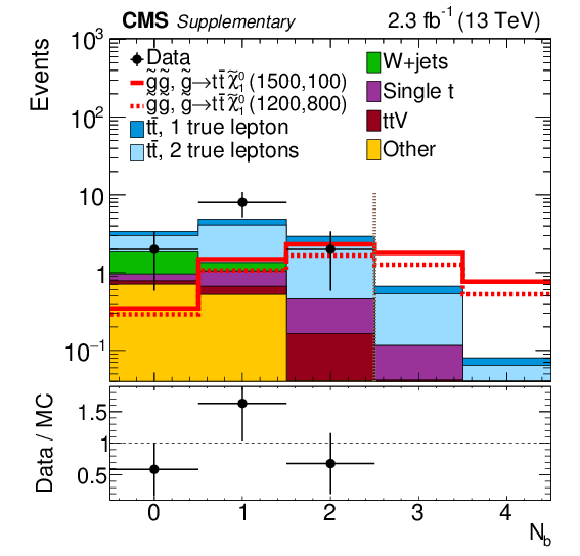
Additional Figure 30-a:
Distribution of $ {N_{\text {b}}}$ in data and simulated event samples in the signal region R4 (baseline selection except for the $ {N_{\text {b}}}$ requirement plus $ {m_{\mathrm {T}}} > $ 140 GeV and $ {M_J} > $ 400 GeV) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {N_{\text {b}}} \geq 3$ requirement for the tightest $ {N_{\text {b}}}$ bin. |
png pdf |
Additional Figure 30-b:
Distribution of $ {N_{\text {b}}}$ in data and simulated event samples in the signal region R4 (baseline selection except for the $ {N_{\text {b}}}$ requirement plus $ {m_{\mathrm {T}}} > $ 140 GeV and $ {M_J} > $ 400 GeV) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {N_{\text {b}}} \geq 3$ requirement for the tightest $ {N_{\text {b}}}$ bin. |
png pdf |
Additional Figure 31-a:
Distribution of ${N_{\text {jets}}}$ in data and simulated event samples in the signal region R4 (baseline selection except for the ${N_{\text {jets}}}$ requirement plus $ {m_{\mathrm {T}}} > $ 140 GeV and $ {M_J} > $ 400 GeV) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {N_{\text {jets}}} \geq 9$ requirement for the tightest ${N_{\text {jets}}}$ bin. |
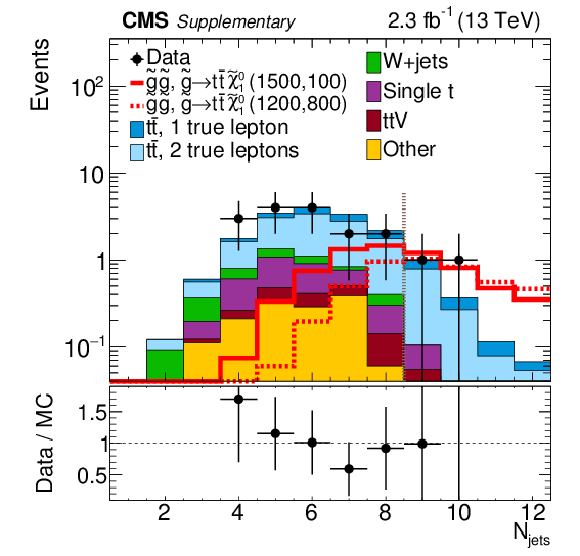
png pdf |
Additional Figure 31-b:
Distribution of ${N_{\text {jets}}}$ in data and simulated event samples in the signal region R4 (baseline selection except for the ${N_{\text {jets}}}$ requirement plus $ {m_{\mathrm {T}}} > $ 140 GeV and $ {M_J} > $ 400 GeV) in linear (a) and logarithmic (b) scales. The background contributions shown here are from simulation, and their total yield is normalized to the number of events observed in data. The signal distributions are normalized to the expected cross sections. The dashed vertical line indicates the $ {N_{\text {jets}}} \geq 9$ requirement for the tightest ${N_{\text {jets}}}$ bin. |
png pdf |
Additional Figure 32:
Two-dimensional distributions for data and simulated event samples in the variables ${m_{\mathrm {T}}}$ and ${M_J}$ in the $ {N_{\text {b}}} = $ 1 region after the baseline selection. The ${N_{\text {jets}}}$ and the ${E_{\mathrm {T}}^{\text {miss}}}$ bins are integrated over. The black dots are the data; the colored histogram is the total simulated background, normalized to the data; and the red dots are a particular signal sample drawn from the expected distribution for gluino pair production with $\tilde{\mathrm{g}} \to {\mathrm{ t } \mathrm{ \bar{t} } } \tilde{\chi}^0_1 $ $( {m_{\tilde{\mathrm{g}} }} =$1500 GeV and ${m_{ \tilde{\chi}^0_1 }} =$ 100 GeV) for 2.3 fb$^{-1}$. Overflow events are shown on the edges of the plot. The definitions of the signal and control regions are the same as those shown in Fig. 4. |
png |
Additional Figure 33:
Event display showing the highest-${M_J}$ event (256843:282:408328426) in 3D Tower view mode. This event has $ {M_J} = $ 1173 GeV, $ {m_{\mathrm {T}}} = $ 91 GeV, $ {E_{\mathrm {T}}^{\text {miss}}} = $ 347 GeV, $ {N_{\text {jets}}} = $ 9, $ {N_{\text {b}}} = $ 2 with a 172 GeV electron. |
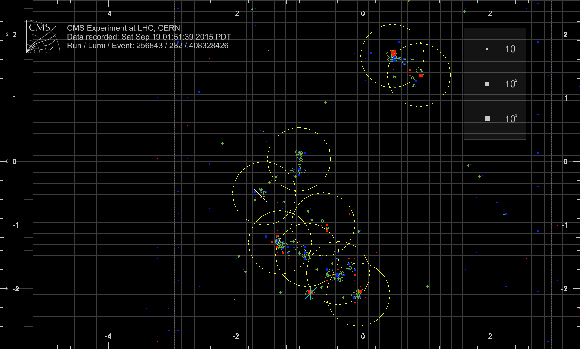
png |
Additional Figure 34:
Event display showing the highest-${M_J}$ event (256843:282:408328426) in the $z$-$\phi $ plane (lego plot). This event has $ {M_J} = $ 1173 GeV, $ {m_{\mathrm {T}}} = $ 91 GeV, $ {E_{\mathrm {T}}^{\text {miss}}} = $ 347 GeV, $ {N_{\text {jets}}} = $ 9, $ {N_{\text {b}}} = $ 2 with a 172 GeV electron. |
png |
Additional Figure 35:
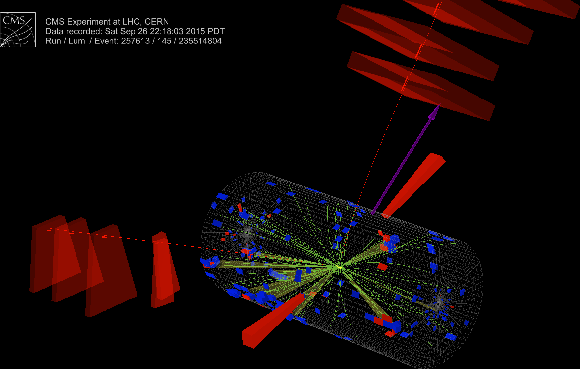
Event display showing a high ${m_{\mathrm {T}}}$ event (257613:145:235514804) in 3D Tower view mode compatible with a $2\ell $ ${\mathrm{ t } \mathrm{ \bar{t} } }$ event. This event has $ {M_J} = $ 365 GeV, $ {m_{\mathrm {T}}} = $ 414 GeV, $ {E_{\mathrm {T}}^{\text {miss}}} = $ 266 GeV, $ {N_{\text {jets}}} = $ 8, $ {N_{\text {b}}} = $ 2 with a 181 GeV reconstructed electron and muon with 16 GeV that does not satisfy the minimum ${p_{\mathrm {T}}}$ requirement of 20 GeV (there is an additional 10 GeV non-isolated muon). |
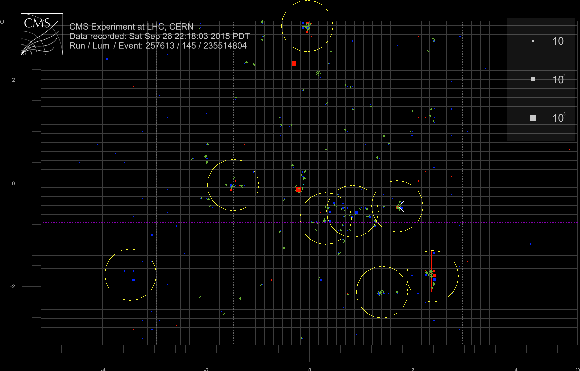
png |
Additional Figure 36:
Event display showing a high ${m_{\mathrm {T}}}$ event (257613:145:235514804) in the $z$-$\phi $ plane (lego plot) compatible with a $2\ell $ ${\mathrm{ t } \mathrm{ \bar{t} } }$ event. This event has $ {M_J} = $ 365 GeV, $ {m_{\mathrm {T}}} = $ 414 GeV, $ {E_{\mathrm {T}}^{\text {miss}}} = $ 266 GeV, $ {N_{\text {jets}}} = $ 8, $ {N_{\text {b}}} = $ 2 with a 181 GeV reconstructed electron and another electron with 16 GeV that does not satisfy the minimum ${p_{\mathrm {T}}}$ requirement of 20 GeV. |
png pdf |
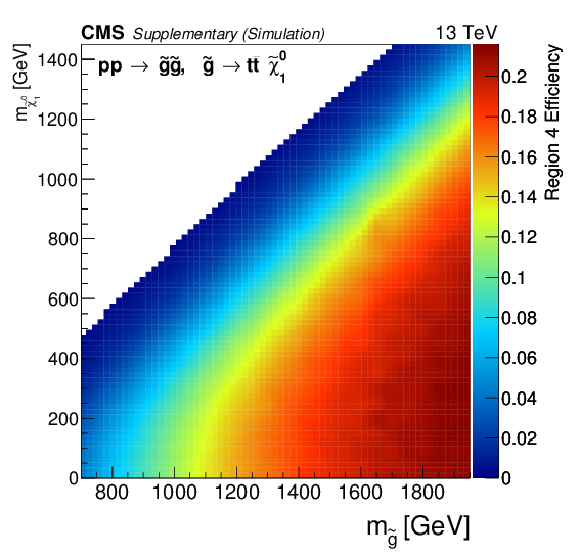
Additional Figure 37-a:
Signal efficiency (a) and expected yields for 2.3 fb$^{-1}$ (b) in region 4 for the process $\mathrm{ p } \mathrm{ p } \rightarrow \tilde{\mathrm{g}} \tilde{\mathrm{g}},\tilde{\mathrm{g}} \rightarrow {\mathrm{ t } \mathrm{ \bar{t} } } \tilde{\chi}^0_1 $ in the $ {m_{\tilde{\mathrm{g}} }} $-$ {m_{ \tilde{\chi}^0_1 }} $ plane. The efficiency is taken with respect to the total $\mathrm{ p } \mathrm{ p } \rightarrow \tilde{\mathrm{g}} \tilde{\mathrm{g}},\tilde{\mathrm{g}} \rightarrow {\mathrm{ t } \mathrm{ \bar{t} } } \tilde{\chi}^0_1 $ cross section, and therefore includes losses to final states with a different number of leptons. |
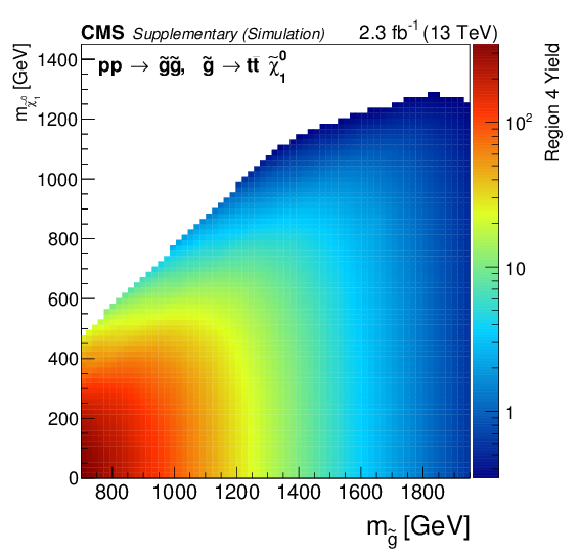
png pdf |
Additional Figure 37-b:
Signal efficiency (a) and expected yields for 2.3 fb$^{-1}$ (b) in region 4 for the process $\mathrm{ p } \mathrm{ p } \rightarrow \tilde{\mathrm{g}} \tilde{\mathrm{g}},\tilde{\mathrm{g}} \rightarrow {\mathrm{ t } \mathrm{ \bar{t} } } \tilde{\chi}^0_1 $ in the $ {m_{\tilde{\mathrm{g}} }} $-$ {m_{ \tilde{\chi}^0_1 }} $ plane. The efficiency is taken with respect to the total $\mathrm{ p } \mathrm{ p } \rightarrow \tilde{\mathrm{g}} \tilde{\mathrm{g}},\tilde{\mathrm{g}} \rightarrow {\mathrm{ t } \mathrm{ \bar{t} } } \tilde{\chi}^0_1 $ cross section, and therefore includes losses to final states with a different number of leptons. |
png pdf |
Additional Figure 38-a:
Signal efficiency (a) and expected yields for 2.3 fb$^{-1}$ (b) in region 4 for the process $\mathrm{ p } \mathrm{ p } \rightarrow \tilde{\mathrm{g}} \tilde{\mathrm{g}},\tilde{\mathrm{g}} \rightarrow \tilde{\mathrm{t}} \tilde{\mathrm{\bar{t}}},\tilde{\mathrm{t}} \rightarrow t \tilde{\chi}^0_1 $ in the $ {m_{\tilde{\mathrm{g}} }} $-$ {m_{ \tilde{\chi}^0_1 }} $ plane. The mass of the top squark is fixed to $ {m_{\tilde{ \mathrm{ t } } _1}} = {m_{ \tilde{\chi}^0_1 }} $ +175 GeV. The efficiency is taken with respect to the total $\mathrm{ p } \mathrm{ p } \rightarrow \tilde{\mathrm{g}} \tilde{\mathrm{g}},\tilde{\mathrm{g}} \rightarrow \tilde{\mathrm{t}} \tilde{\mathrm{\bar{t}}},\tilde{\mathrm{t}} \rightarrow \mathrm{t} \tilde{\chi}^0_1 $ cross section, and therefore includes losses to final states with a different number of leptons. |
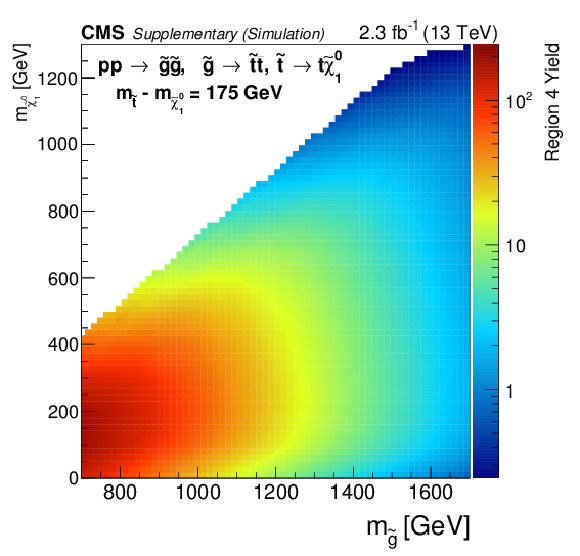
png pdf |
Additional Figure 38-b:
Signal efficiency (a) and expected yields for 2.3 fb$^{-1}$ (b) in region 4 for the process $\mathrm{ p } \mathrm{ p } \rightarrow \tilde{\mathrm{g}} \tilde{\mathrm{g}},\tilde{\mathrm{g}} \rightarrow \tilde{\mathrm{t}} \tilde{\mathrm{\bar{t}}},\tilde{\mathrm{t}} \rightarrow t \tilde{\chi}^0_1 $ in the $ {m_{\tilde{\mathrm{g}} }} $-$ {m_{ \tilde{\chi}^0_1 }} $ plane. The mass of the top squark is fixed to $ {m_{\tilde{ \mathrm{ t } } _1}} = {m_{ \tilde{\chi}^0_1 }} $ +175 GeV. The efficiency is taken with respect to the total $\mathrm{ p } \mathrm{ p } \rightarrow \tilde{\mathrm{g}} \tilde{\mathrm{g}},\tilde{\mathrm{g}} \rightarrow \tilde{\mathrm{t}} \tilde{\mathrm{\bar{t}}},\tilde{\mathrm{t}} \rightarrow \mathrm{t} \tilde{\chi}^0_1 $ cross section, and therefore includes losses to final states with a different number of leptons. |
| References | ||||
| 1 | P. Ramond | Dual theory for free fermions | PRD 3 (1971) 2415 | |
| 2 | Y. A. Gol'fand and E. P. Likhtman | Extension of the algebra of Poincar$ \'e $ group generators and violation of P invariance | JEPTL 13 (1971)323 | |
| 3 | A. Neveu and J. H. Schwarz | Factorizable dual model of pions | Nucl. Phys. B 31 (1971) 86 | |
| 4 | D. V. Volkov and V. P. Akulov | Possible universal neutrino interaction | JEPTL 16 (1972) 438 | |
| 5 | J. Wess and B. Zumino | A Lagrangian model invariant under supergauge transformations | PLB 49 (1974) 52 | |
| 6 | J. Wess and B. Zumino | Supergauge transformations in four dimensions | Nucl. Phys. B 70 (1974) 39 | |
| 7 | P. Fayet | Supergauge invariant extension of the Higgs mechanism and a model for the electron and its neutrino | Nucl. Phys. B 90 (1975) 104 | |
| 8 | H. P. Nilles | Supersymmetry, supergravity and particle physics | Phys. Rep. 110 (1984) 1 | |
| 9 | G. 't Hooft | Naturalness, chiral symmetry, and spontaneous chiral symmetry breaking | in Recent Developments in Gauge Theories, G. 't Hooft et al., eds., p. 135 Springer, 1980NATO Advanced Study Institutes Series B | |
| 10 | E. Witten | Dynamical breaking of supersymmetry | Nucl. Phys. B 188 (1981) 513 | |
| 11 | M. Dine, W. Fischler, and M. Srednicki | Supersymmetric technicolor | Nucl. Phys. B 189 (1981) 575 | |
| 12 | S. Dimopoulos and S. Raby | Supercolor | Nucl. Phys. B 192 (1981) 353 | |
| 13 | S. Dimopoulos and H. Georgi | Softly broken supersymmetry and SU(5) | Nucl. Phys. B 193 (1981) 150 | |
| 14 | R. K. Kaul and P. Majumdar | Cancellation of quadratically divergent mass corrections in globally supersymmetric spontaneously broken gauge theories | Nucl. Phys. B 199 (1982) 36 | |
| 15 | F. Zwicky | Die Rotverschiebung von Extragalaktischen Nebeln | Helv. Phys. Acta 6 (1933)110 | |
| 16 | V. C. Rubin and W. K. Ford Jr | Rotation of the Andromeda nebula from a spectroscopic survey of emission regions | Astrophys. J. 159 (1970) 379 | |
| 17 | Particle Data Group, K. A. Olive et al. | Review of Particle Physics | CPC 38 (2014) 090001 | |
| 18 | S. Dimopoulos, S. Raby, and F. Wilczek | Supersymmetry and the scale of unification | PRD 24 (1981) 1681 | |
| 19 | N. Sakai | Naturalness in supersymmetric GUTS | Z. Phys. C 11 (1981) 153 | |
| 20 | L. E. Ibanez and G. G. Ross | Low-energy predictions in supersymmetric grand unified theories | PLB 105 (1981) 439 | |
| 21 | M. B. Einhorn and D. R. T. Jones | The weak mixing angle and unification mass in supersymmetric SU(5) | Nucl. Phys. B 196 (1982) 475 | |
| 22 | W. J. Marciano and G. Senjanovic | Predictions of supersymmetric grand unified theories | PRD 25 (Jun, 1982) 3092 | |
| 23 | G. R. Farrar and P. Fayet | Phenomenology of the production, decay, and detection of new hadronic states associated with supersymmetry | PLB 76 (1978) 575 | |
| 24 | S. P. Martin | A Supersymmetry Primer | Adv. Ser. Direct. High Energy Phys., vol. 21 | hep-ph/9709356 |
| 25 | ATLAS Collaboration | Observation of a new particle in the search for the Standard Model Higgs boson with the ATLAS detector at the LHC | PLB 716 (2012) 1 | 1207.7214 |
| 26 | CMS Collaboration | Observation of a new boson at a mass of 125 GeV with the CMS experiment at the LHC | PLB 716 (2012) 30 | CMS-HIG-12-028 1207.7235 |
| 27 | CMS Collaboration | Observation of a new boson with mass near 125 GeV in pp collisions at $ \sqrt{s} $ = 7 and 8 TeV | JHEP 06 (2013) 081 | CMS-HIG-12-036 1303.4571 |
| 28 | CMS Collaboration | Precise determination of the mass of the Higgs boson and tests of compatibility of its couplings with the standard model predictions using proton collisions at 7 and 8 TeV | EPJC 75 (2015) 212 | CMS-HIG-14-009 1412.8662 |
| 29 | ATLAS Collaboration | Measurement of the Higgs boson mass from the $ \textrm{H}\rightarrow \gamma\gamma $ and $ \textrm{H} \rightarrow \textrm{ZZ}^{*} \rightarrow 4\ell $ channels with the ATLAS detector using 25 fb$ ^{-1} $ of pp collision data | PRD 90 (2014) 052004 | 1406.3827 |
| 30 | ATLAS and CMS Collaborations | Combined measurement of the Higgs boson mass in pp collisions at $ \sqrt{s} = 7 $ and 8 TeV with the ATLAS and CMS experiments | PRL 114 (2015) 191803 | 1503.07589 |
| 31 | S. Dimopoulos and G. F. Giudice | Naturalness constraints in supersymmetric theories with nonuniversal soft terms | PLB 357 (1995) 573 | hep-ph/9507282 |
| 32 | R. Barbieri and D. Pappadopulo | S-particles at their naturalness limits | JHEP 10 (2009) 061 | 0906.4546 |
| 33 | M. Papucci, J. T. Ruderman, and A. Weiler | Natural SUSY endures | JHEP 09 (2012) 035 | 1110.6926 |
| 34 | J. L. Feng | Naturalness and the status of supersymmetry | Ann. Rev. Nucl. Part. Sci. 63 (2013) 351 | 1302.6587 |
| 35 | N. Craig | The state of supersymmetry after Run I of the LHC | in Beyond the Standard Model after the first run of the LHC, Arcetri, Florence, Italy, May 20-July 12, 2013 2013 | 1309.0528 |
| 36 | ATLAS Collaboration | Search for strong production of supersymmetric particles in final states with missing transverse momentum and at least three $ b $-jets at $ \sqrt{s} $= 8 TeV proton-proton collisions with the ATLAS detector | JHEP 10 (2014) 024 | 1407.0600 |
| 37 | ATLAS Collaboration | Search for squarks and gluinos in events with isolated leptons, jets and missing transverse momentum at $ \sqrt{s} = $ 8 TeV with the ATLAS detector | JHEP 04 (2015) 116 | 1501.03555 |
| 38 | CMS Collaboration | Search for supersymmetry in pp collisions at $ \sqrt{s} = $ 8 TeV in events with a single lepton, large jet multiplicity, and multiple b jets | PLB 733 (2014) 328 | CMS-SUS-13-007 1311.4937 |
| 39 | C. Borschensky et al. | Squark and gluino production cross sections in pp collisions at $ \sqrt{s} = 13 $, 14, 33 and 100 TeV | EPJC 74 (2014) 3174 | 1407.5066 |
| 40 | M. Czakon, P. Fiedler, and A. Mitov | The total top quark pair production cross-section at $ \mathcal{O}(\alpha^4_S) $ | PRL 110 (2013) 252004 | 1303.6254 |
| 41 | CMS Collaboration | Interpretation of searches for supersymmetry with simplified models | PRD 88 (2013) 052017 | CMS-SUS-11-016 1301.2175 |
| 42 | J. Alwall, P. Schuster, and N. Toro | Simplified models for a first characterization of new physics at the LHC | PRD 79 (2009) 075020 | 0810.3921 |
| 43 | J. Alwall, M.-P. Le, M. Lisanti, and J. G. Wacker | Model-independent jets plus missing energy searches | PRD 79 (2009) 015005 | 0809.3264 |
| 44 | D. Alves et al. | Simplified models for LHC new physics searches | JPG 39 (2012) 105005 | 1105.2838 |
| 45 | A. Hook, E. Izaguirre, M. Lisanti, and J. G. Wacker | High multiplicity searches at the LHC using jet masses | PRD 85 (2012) 055029 | 1202.0558 |
| 46 | T. Cohen, E. Izaguirre, M. Lisanti, and H. K. Lou | Jet substructure by accident | JHEP 03 (2013) 161 | 1212.1456 |
| 47 | S. El Hedri, A. Hook, M. Jankowiak, and J. G. Wacker | Learning how to count: a high multiplicity search for the LHC | JHEP 08 (2013) 136 | 1302.1870 |
| 48 | ATLAS Collaboration | Search for massive supersymmetric particles decaying to many jets using the ATLAS detector in pp collisions at $ \sqrt{s} = $ 8 TeV | PRD 91 (2015) 112016 | 1502.05686 |
| 49 | ATLAS Collaboration | Search for new phenomena in final states with large jet multiplicities and missing transverse momentum at $ \sqrt{s} = $ 8 TeV proton-proton collisions using the ATLAS experiment | JHEP 10 (2013) 130, , [Erratum: \DOI10.1007/JHEP01(2014)109] | 1308.1841 |
| 50 | CMS Collaboration | Commissioning the performance of key observables used in SUSY searches with the first 13 TeV data | CDS | |
| 51 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | CMS-00-001 |
| 52 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 53 | S. Alioli, P. Nason, C. Oleari, and E. Re | NLO single-top production matched with shower in POWHEG: $ s $- and $ t $-channel contributions | JHEP 09 (2009) 111, , [Erratum: \DOI10.1007/JHEP02(2010)011] | 0907.4076 |
| 54 | E. Re | Single-top $ \mathrm{ W }\mathrm{ t } $-channel production matched with parton showers using the POWHEG method | EPJC 71 (2011) 1547 | 1009.2450 |
| 55 | NNPDF Collaboration | Parton distributions for the LHC Run II | JHEP 04 (2015) 040 | 1410.8849 |
| 56 | T. Sjostrand et al. | An Introduction to PYTHIA 8.2 | CPC 191 (2015) 159 | 1410.3012 |
| 57 | CMS Collaboration | Event generator tunes obtained from underlying event and multiparton scattering measurements | EPJC 76 (2016), no. 3, 155 | CMS-GEN-14-001 1512.00815 |
| 58 | GEANT4 Collaboration | GEANT4---a simulation toolkit | NIMA 506 (2003) 250 | |
| 59 | CMS Collaboration | Measurement of the differential cross section for top quark pair production in pp collisions at $ \sqrt{s} = $ 8 TeV | EPJC 75 (2015) 542 | CMS-TOP-12-028 1505.04480 |
| 60 | CMS Collaboration | The fast simulation of the CMS detector at LHC | J. Phys. Conf. Ser. 331 (2011) 032049 | |
| 61 | W. Beenakker, R. Hopker, M. Spira, and P. M. Zerwas | Squark and gluino production at hadron colliders | Nucl. Phys. B 492 (1997) 51 | hep-ph/9610490 |
| 62 | A. Kulesza and L. Motyka | Threshold resummation for squark-antisquark and gluino-pair production at the LHC | PRL 102 (2009) 111802 | 0807.2405 |
| 63 | A. Kulesza and L. Motyka | Soft gluon resummation for the production of gluino-gluino and squark-antisquark pairs at the LHC | PRD 80 (2009) 095004 | 0905.4749 |
| 64 | W. Beenakker et al. | Soft-gluon resummation for squark and gluino hadroproduction | JHEP 12 (2009) 041 | 0909.4418 |
| 65 | CMS Collaboration | Particle flow event reconstruction in CMS and performance for jets, taus and $ E_{\mathrm{T}}^{\text{miss}} $ | CDS | |
| 66 | CMS Collaboration | Commissioning of the particle-flow event reconstruction with the first LHC collisions recorded in the CMS detector | CDS | |
| 67 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_{\rm t} $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 68 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 69 | M. Cacciari and G. P. Salam | Pileup subtraction using jet areas | PLB 659 (2008) 119 | 0707.1378 |
| 70 | CMS Collaboration | Determination of jet energy calibration and transverse momentum resolution in CMS | JINST 6 (2011) P11002 | CMS-JME-10-011 1107.4277 |
| 71 | CMS Collaboration | Identification of $ \mathrm{ b } $-quark jets with the CMS experiment | JINST 8 (2013) P04013 | CMS-BTV-12-001 1211.4462 |
| 72 | CMS Collaboration | Identification of $ \mathrm{ b } $ quark jets at the CMS Experiment in the LHC Run 2 | CMS-PAS-BTV-15-001 | CMS-PAS-BTV-15-001 |
| 73 | CMS Collaboration | Performance of electron reconstruction and selection with the CMS detector in proton-proton collisions at $ \sqrt{s} = $ 8 TeV | JINST 10 (2015) P06005 | CMS-EGM-13-001 1502.02701 |
| 74 | CMS Collaboration | Performance of CMS muon reconstruction in pp collision events at $ \sqrt{s} = $ 7 TeV | JINST 7 (2012) P10002 | CMS-MUO-10-004 1206.4071 |
| 75 | K. Rehermann and B. Tweedie | Efficient identification of boosted semileptonic top quarks at the LHC | JHEP 03 (2011) 059 | 1007.2221 |
| 76 | ATLAS Collaboration | Search for direct pair production of the top squark in all-hadronic final states in proton-proton collisions at $ \sqrt{s} = $ 8 TeV with the ATLAS detector | JHEP 09 (2014) 015 | 1406.1122 |
| 77 | CMS Collaboration | Search for supersymmetry in the multijet and missing transverse momentum final state in pp collisions at 13 TeV | Submitted to PLB | CMS-SUS-15-002 1602.06581 |
| 78 | CMS Collaboration | CMS luminosity measurement for the 2015 data taking period | CMS-PAS-LUM-15-001 | CMS-PAS-LUM-15-001 |
| 79 | T. Junk | Confidence level computation for combining searches with small statistics | NIMA 434 (1999) 435 | hep-ex/9902006 |
| 80 | A. L. Read | Presentation of search results: the $ \rm CL_s $ technique | JPG 28 (2002) 2693 | |
| 81 | ATLAS Collaboration, CMS Collaboration, LHC Higgs Combination Group | Procedure for the LHC Higgs boson search combination in Summer 2011 | CMS-NOTE-2011-005 | |
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|