Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-PAS-SUS-18-005 | ||
| Combined search for gauge-mediated supersymmetry with photons in 13 TeV collisions at the CMS experiment | ||
| CMS Collaboration | ||
| March 2019 | ||
| Abstract: A combination of searches for signatures with at least one photon motivated by generalized models of gauge-mediated supersymmetry breaking is presented. All searches make use of proton-proton collision data at $\sqrt{s}= $ 13 TeV recorded by the CMS detector at the LHC. The results of four analyses targeting separate experimental signatures incorporating an isolated photon and significant missing transverse energy are combined. These signatures include events with two isolated photons, events with single leptons that accompany the photon, and events with additional jets. Based on the 35.9 fb$^{-1}$ of integrated luminosity collected in 2016, this combination probes the allowed parameter space of General Gauge Mediation and exceeds the sensitivity of the individual searches to supersymmetric particles by up to 100 GeV in mass. | ||
|
Links:
CDS record (PDF) ;
CADI line (restricted) ;
These preliminary results are superseded in this paper, PLB 801 (2020) 135183. The superseded preliminary plots can be found here. |
||
| Figures | |
png pdf |
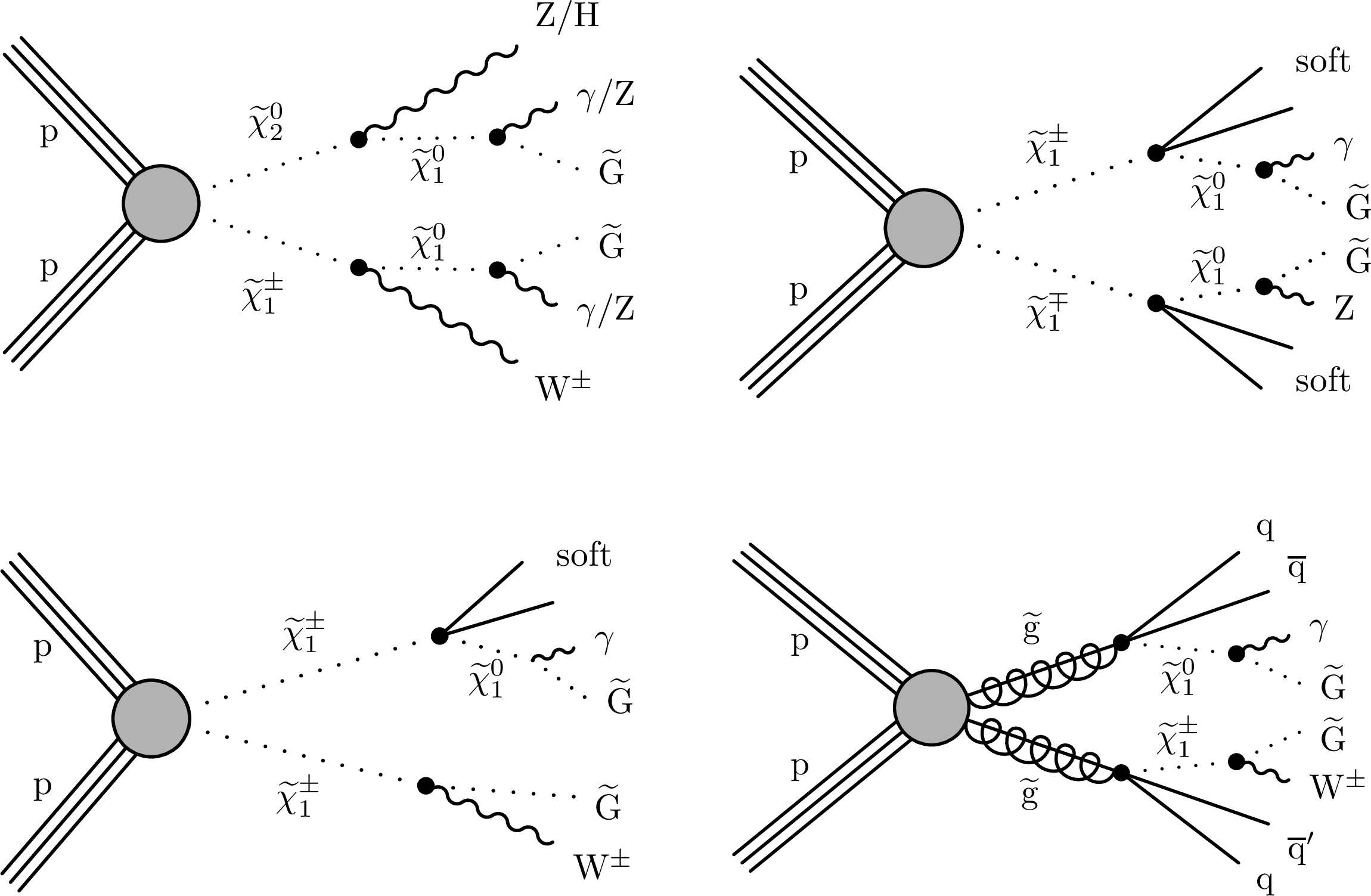
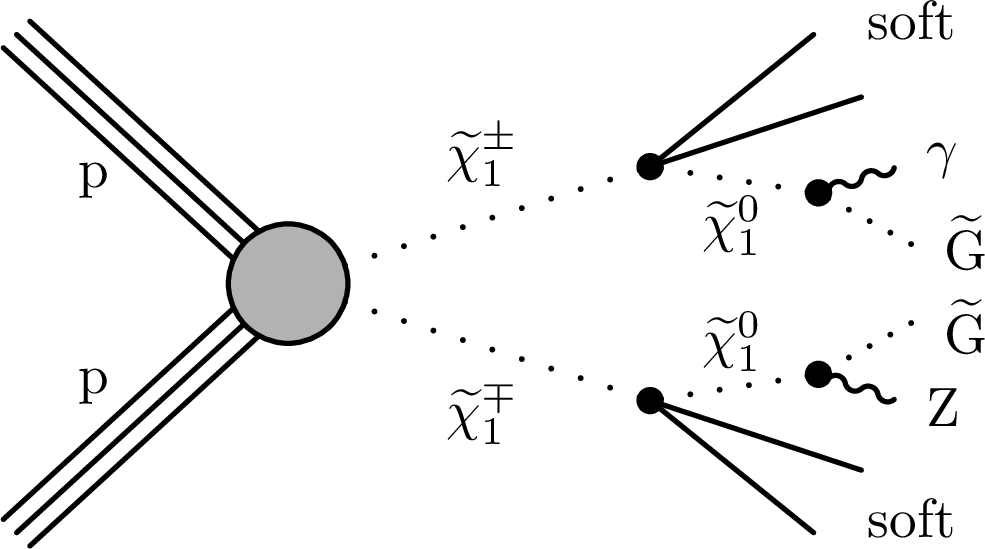
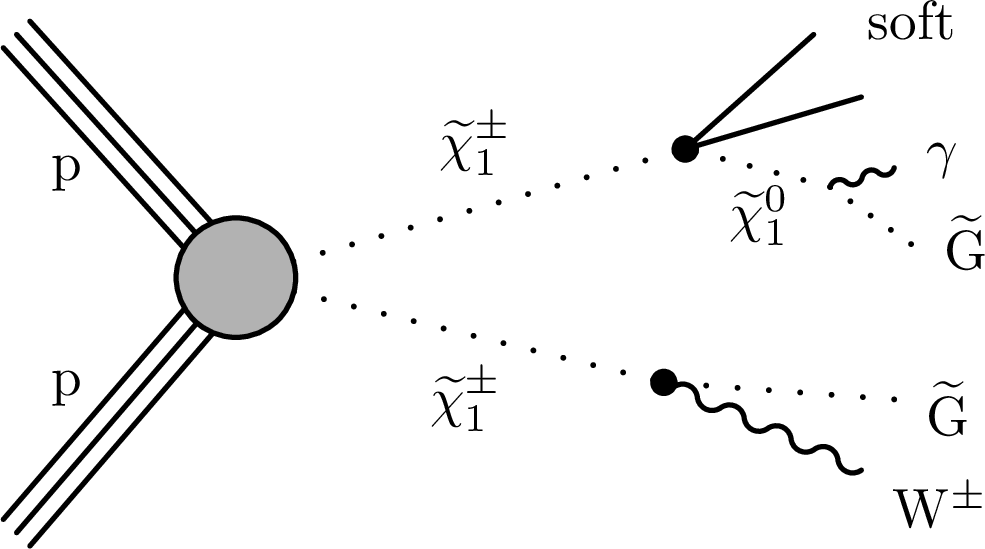
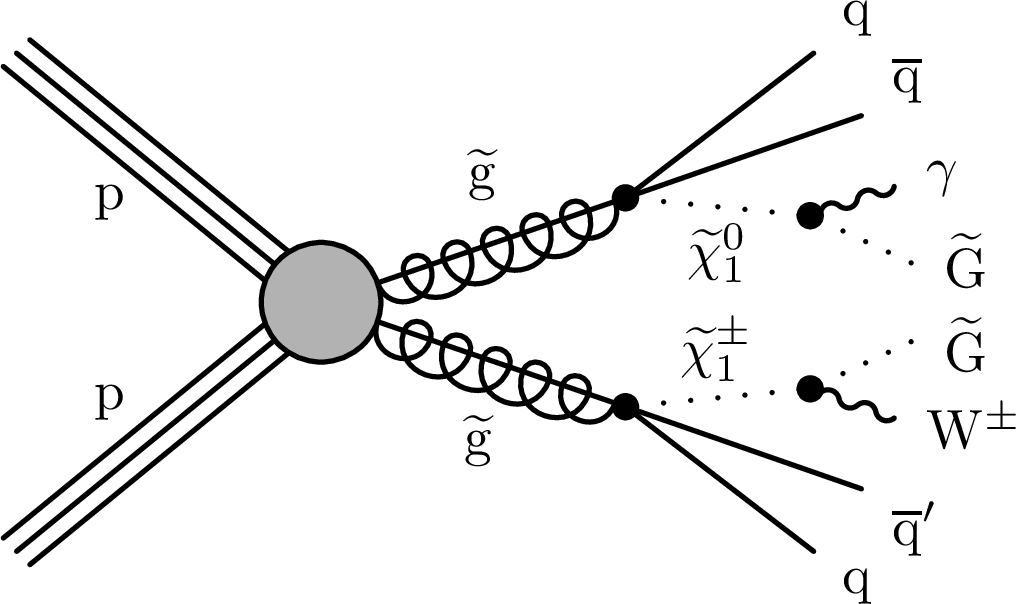
Figure 1:
In the GGM scenarios several production and decay channels are possible. The diagram of one possible process based on $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{2}} $ production is shown (upper left), where the gaugino decays depend on the corresponding composition of the gauge eigenstates. In the Neutralino BF scenario $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ (upper right) and $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{1}} $ production are probed. The Chargino BF scenario (lower left) probes $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ production. In the lower right diagram gluino pair production is shown including the charged and uncharged gluino decay. |
png pdf |
Figure 1-a:
In the GGM scenarios several production and decay channels are possible. The diagram of one possible process based on $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{2}} $ production is shown (upper left), where the gaugino decays depend on the corresponding composition of the gauge eigenstates. In the Neutralino BF scenario $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ (upper right) and $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{1}} $ production are probed. The Chargino BF scenario (lower left) probes $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ production. In the lower right diagram gluino pair production is shown including the charged and uncharged gluino decay. |
png pdf |
Figure 1-b:
In the GGM scenarios several production and decay channels are possible. The diagram of one possible process based on $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{2}} $ production is shown (upper left), where the gaugino decays depend on the corresponding composition of the gauge eigenstates. In the Neutralino BF scenario $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ (upper right) and $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{1}} $ production are probed. The Chargino BF scenario (lower left) probes $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ production. In the lower right diagram gluino pair production is shown including the charged and uncharged gluino decay. |
png pdf |
Figure 1-c:
In the GGM scenarios several production and decay channels are possible. The diagram of one possible process based on $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{2}} $ production is shown (upper left), where the gaugino decays depend on the corresponding composition of the gauge eigenstates. In the Neutralino BF scenario $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ (upper right) and $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{1}} $ production are probed. The Chargino BF scenario (lower left) probes $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ production. In the lower right diagram gluino pair production is shown including the charged and uncharged gluino decay. |
png pdf |
Figure 1-d:
In the GGM scenarios several production and decay channels are possible. The diagram of one possible process based on $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{2}} $ production is shown (upper left), where the gaugino decays depend on the corresponding composition of the gauge eigenstates. In the Neutralino BF scenario $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ (upper right) and $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{1}} $ production are probed. The Chargino BF scenario (lower left) probes $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ production. In the lower right diagram gluino pair production is shown including the charged and uncharged gluino decay. |
png pdf |
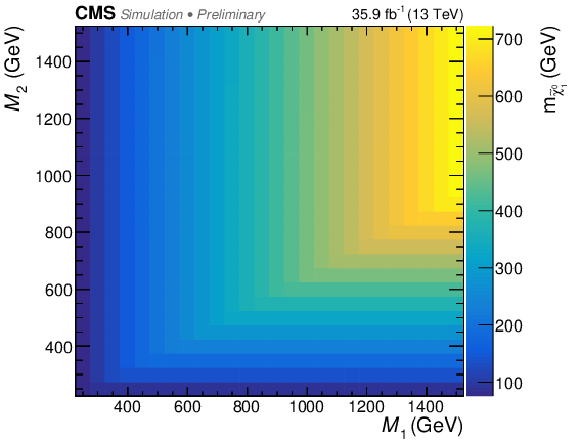
Figure 2:
Branching fractions for the NLSP decay to a photon and a gravitino for the M1M2 scenario (left). The phase space is spanned by the bino and wino mass parameters and a change of the NLSP composition is visible. The change of the NLSP composition also influences the dependence of the physical mass of the neutralino on the gauge mass parameters (right). |
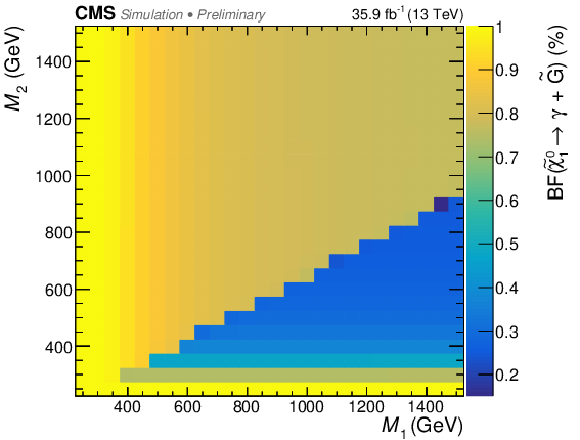
png pdf |
Figure 2-a:
Branching fractions for the NLSP decay to a photon and a gravitino for the M1M2 scenario (left). The phase space is spanned by the bino and wino mass parameters and a change of the NLSP composition is visible. The change of the NLSP composition also influences the dependence of the physical mass of the neutralino on the gauge mass parameters (right). |
png pdf |
Figure 2-b:
Branching fractions for the NLSP decay to a photon and a gravitino for the M1M2 scenario (left). The phase space is spanned by the bino and wino mass parameters and a change of the NLSP composition is visible. The change of the NLSP composition also influences the dependence of the physical mass of the neutralino on the gauge mass parameters (right). |
png pdf |
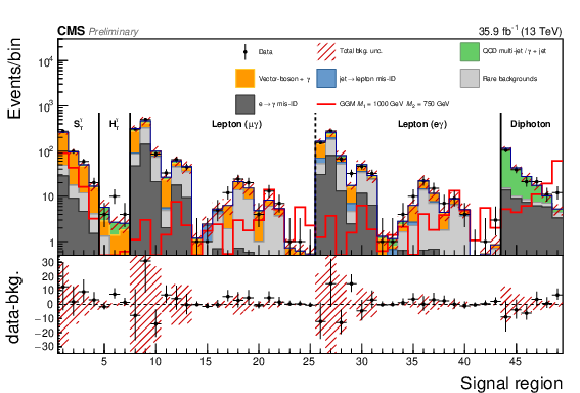
Figure 3:
Comparison between the observed yield and the background prediction for all search bins used in the combination. The yields for the Photon+Lepton{} and Diphoton{} categories correspond to the published results, while the yields of the Photon+${S^{\gamma}_{T}}$ and Photon+$ {H^{\gamma}_{T}}$ categories are based on the modified event selections ensuring exclusive signal regions. |
png pdf |
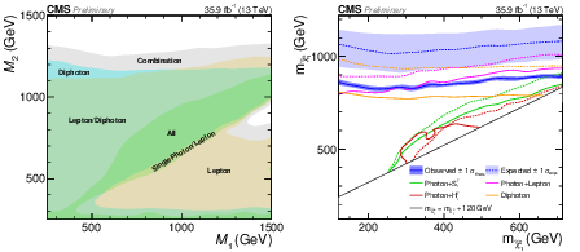
Figure 4:
Combined exclusion limits for the M1M2 scenario in terms of the GGM model parameters (left) and the physical neutralino and chargino masses (right). The left panel shows the expected exclusion, while the right panel shows both the observed and the expected exclusion limits. In the physical mass plane only signal points with a mass splitting above $120 GeV $ are shown to enable a precise assignment of the physical masses and the GGM model parameters. The uncertainty bands around the expected and observed limit of the combination in the right panel correspond to the experimental ($\sigma _{\text {exp.}}$) and theory uncertainty ($\sigma _{\text {theo.}}$), respectively. |
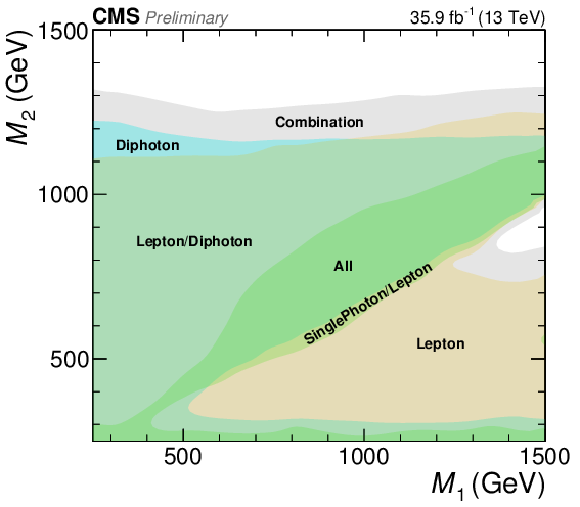
png pdf |
Figure 4-a:
Combined exclusion limits for the M1M2 scenario in terms of the GGM model parameters (left) and the physical neutralino and chargino masses (right). The left panel shows the expected exclusion, while the right panel shows both the observed and the expected exclusion limits. In the physical mass plane only signal points with a mass splitting above $120 GeV $ are shown to enable a precise assignment of the physical masses and the GGM model parameters. The uncertainty bands around the expected and observed limit of the combination in the right panel correspond to the experimental ($\sigma _{\text {exp.}}$) and theory uncertainty ($\sigma _{\text {theo.}}$), respectively. |
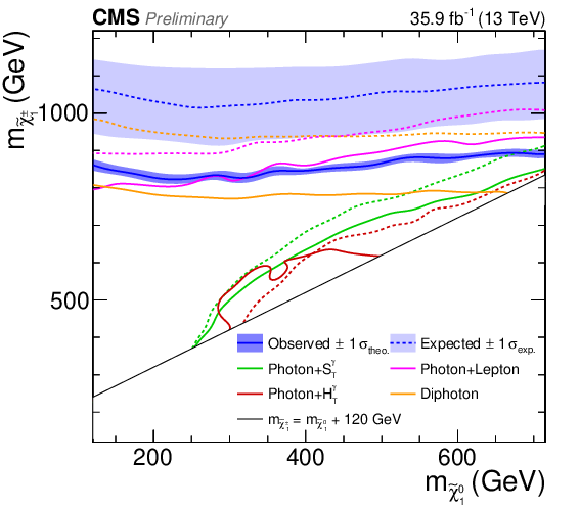
png pdf |
Figure 4-b:
Combined exclusion limits for the M1M2 scenario in terms of the GGM model parameters (left) and the physical neutralino and chargino masses (right). The left panel shows the expected exclusion, while the right panel shows both the observed and the expected exclusion limits. In the physical mass plane only signal points with a mass splitting above $120 GeV $ are shown to enable a precise assignment of the physical masses and the GGM model parameters. The uncertainty bands around the expected and observed limit of the combination in the right panel correspond to the experimental ($\sigma _{\text {exp.}}$) and theory uncertainty ($\sigma _{\text {theo.}}$), respectively. |
png pdf |
Figure 5:
Combined exclusion for the Neutralino BF scenario (left), which probes $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{1}} $ and $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ production combined with the NLSP BF scanned between decays to $Z\tilde{G}$ and $\gamma \tilde{G}$. Combined exclusion for the Chargino BF scenario (right), which probes $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ production combined with the Chargino BF scanned between decays to $W\tilde{G}$ and $\tilde{\chi}^0_1+\mathrm {soft}$, where the $\tilde{\chi}^0_1$ decays into a photon and a gravitino. The uncertainty bands around the expected and observed limit of the combination correspond to the experimental ($\sigma _{\text {exp.}}$) and theory uncertainty ($\sigma _{\text {theo.}}$), respectively. |
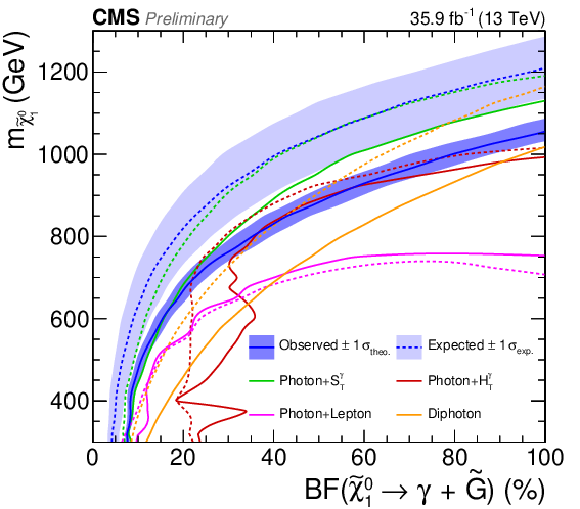
png pdf |
Figure 5-a:
Combined exclusion for the Neutralino BF scenario (left), which probes $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{1}} $ and $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ production combined with the NLSP BF scanned between decays to $Z\tilde{G}$ and $\gamma \tilde{G}$. Combined exclusion for the Chargino BF scenario (right), which probes $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ production combined with the Chargino BF scanned between decays to $W\tilde{G}$ and $\tilde{\chi}^0_1+\mathrm {soft}$, where the $\tilde{\chi}^0_1$ decays into a photon and a gravitino. The uncertainty bands around the expected and observed limit of the combination correspond to the experimental ($\sigma _{\text {exp.}}$) and theory uncertainty ($\sigma _{\text {theo.}}$), respectively. |
png pdf |
Figure 5-b:
Combined exclusion for the Neutralino BF scenario (left), which probes $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}^{0}_{1}} $ and $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ production combined with the NLSP BF scanned between decays to $Z\tilde{G}$ and $\gamma \tilde{G}$. Combined exclusion for the Chargino BF scenario (right), which probes $ {\tilde{\chi}^\pm _{1}} {\tilde{\chi}_{1}^{\mp}}$ production combined with the Chargino BF scanned between decays to $W\tilde{G}$ and $\tilde{\chi}^0_1+\mathrm {soft}$, where the $\tilde{\chi}^0_1$ decays into a photon and a gravitino. The uncertainty bands around the expected and observed limit of the combination correspond to the experimental ($\sigma _{\text {exp.}}$) and theory uncertainty ($\sigma _{\text {theo.}}$), respectively. |
png pdf |
Figure 6:
Combined exclusion for the nominal Gluino scenario (left) assuming equal probabilities for the charged and uncharged gluino decay. For the Gluino BF scenario (right) the ratio of the probabilities for both decays are scanned and the gluino mass is fixed to $1950 GeV $. For both interpretations the Diphoton{} category is not used. The uncertainty bands around the expected and observed limit of the combination correspond to the experimental ($\sigma _{\text {exp.}}$) and theory uncertainty ($\sigma _{\text {theo.}}$), respectively. |
png pdf |
Figure 6-a:
Combined exclusion for the nominal Gluino scenario (left) assuming equal probabilities for the charged and uncharged gluino decay. For the Gluino BF scenario (right) the ratio of the probabilities for both decays are scanned and the gluino mass is fixed to $1950 GeV $. For both interpretations the Diphoton{} category is not used. The uncertainty bands around the expected and observed limit of the combination correspond to the experimental ($\sigma _{\text {exp.}}$) and theory uncertainty ($\sigma _{\text {theo.}}$), respectively. |
png pdf |
Figure 6-b:
Combined exclusion for the nominal Gluino scenario (left) assuming equal probabilities for the charged and uncharged gluino decay. For the Gluino BF scenario (right) the ratio of the probabilities for both decays are scanned and the gluino mass is fixed to $1950 GeV $. For both interpretations the Diphoton{} category is not used. The uncertainty bands around the expected and observed limit of the combination correspond to the experimental ($\sigma _{\text {exp.}}$) and theory uncertainty ($\sigma _{\text {theo.}}$), respectively. |
| Tables | |
png pdf |
Table 1:
Exclusive definitions of the four categories. The kinematic cuts and the search bins are based on the four initial searches. The additional vetoes ensure exclusive event categories. Diphoton and Lepton veto match the event selection described in [19] and [18]. |
png pdf |
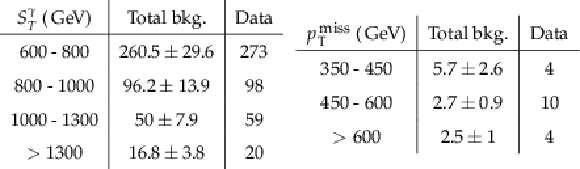
Table 2:
Predicted background yields and observed data for the Photon+${S^{\gamma}_{T}}$ category (left) and the Photon+$ {H^{\gamma}_{T}}$ category (right). The excess in the second bin of the Photon+$ {H^{\gamma}_{T}}$ category was also found in the initial search [16]. |
| Summary |
|
A combination of four different searches for gauge-mediated supersymmetry in final states with photons and a large transverse momentum imbalance has been performed. Based on the event selection of the individual searches four event categories were defined. Overlaps between the categories were removed by additional vetoes designed to maximize the sensitivity of the combination. Using 36 fb$^{-1}$ of data recorded by the CMS detector at the LHC at a center-of-mass energy of 13 TeV the combination maximizes the exclusion power of the searches described in [17,16,18,19]. The results are interpreted in the context of General Gauge Mediation (GGM) and in model-independent mass scans using simplified topologies. The sensitivity of the combination is also interpreted across a range of branching fractions allowing for generalization to a wide range of SUSY scenarios. The GGM scan is performed in an ${{M_\mathrm{1}}} $ and ${{M_\mathrm{2}}} $ parameter space where most of the signal points below $M2 < $ 1100 (1300) GeV are excluded based on the observed (expected) limit. The results of the GGM scenario is also shown in physical mass parameters, which show competitive constraints on the chargino and neutralino masses. Here, chargino masses up to 890 (1080) GeV are excluded by the the observed (expected) limit across the tested neutralino mass spectrum. For a strong production scenario based on gluino pair production the highest observed (expected) excluded gluino masses are at 1975 (2050) GeV. In electroweak production models observed (expected) limits for neutralino masses are set up to 1050 (1200) GeV for combined ${\tilde{\chi}_{1}^{\mp}}{\tilde{\chi}_{1}^{0}}$ and ${\tilde{\chi}_{1}^{\mp}} {\tilde{\chi}_{1}^{\mp}}$ production, while for pure ${\tilde{\chi}_{1}^{\mp}} {\tilde{\chi}_{1}^{\mp}}$ production these limits are reduced to 850 (1000) GeV. The combination improves on the expected limits on neutralino and chargino masses by 100 GeV while the expected limit on the gluino mass is increased by 50 GeV compared to the individual searches. |
| References | ||||
| 1 | M. Dine and W. Fischler | A phenomenological model of particle physics based on supersymmetry | PLB 110 (1982) 227 | |
| 2 | L. Alvarez-Gaume, M. Claudson, and M. B. Wise | Low-energy supersymmetry | NPB 207 (1982) 96 | |
| 3 | C. R. Nappi and B. A. Ovrut | Supersymmetric extension of the SU(3)xSU(2)xU(1) model | PLB 113 (1982) 175 | |
| 4 | M. Dine and A. E. Nelson | Dynamical supersymmetry breaking at low energies | PRD 48 (1993) 1277 | hep-ph/9303230 |
| 5 | M. Dine, A. E. Nelson, and Y. Shirman | Low energy dynamical supersymmetry breaking simplified | PRD 51 (1995) 1362 | hep-ph/9408384 |
| 6 | M. Dine, A. E. Nelson, Y. Nir, and Y. Shirman | New tools for low-energy dynamical supersymmetry breaking | PRD 53 (1996) 2658 | hep-ph/9507378 |
| 7 | G. R. Farrar and P. Fayet | Phenomenology of the production, decay, and detection of new hadronic states associated with supersymmetry | PLB 76 (1978) 575 | |
| 8 | S. Dimopoulos, G. F. Giudice, and A. Pomarol | Dark matter in theories of gauge mediated supersymmetry breaking | PLB 389 (1996) 37 | hep-ph/9607225 |
| 9 | S. P. Martin | Generalized messengers of supersymmetry breaking and the sparticle mass spectrum | PRD 55 (1997) 3177 | hep-ph/9608224 |
| 10 | E. Poppitz and S. P. Trivedi | Some remarks on gauge mediated supersymmetry breaking | PLB 401 (1997) 38 | hep-ph/9703246 |
| 11 | P. Meade, N. Seiberg, and D. Shih | General Gauge Mediation | Prog. Theor. Phys. Suppl. 177 (2009) 143--158 | 0801.3278 |
| 12 | M. Buican, P. Meade, N. Seiberg, and D. Shih | Exploring general gauge mediation | JHEP 03 (2009) 016 | 0812.3668 |
| 13 | S. Abel, M. J. Dolan, J. Jaeckel, and V. V. Khoze | Phenomenology of pure general gauge mediation | JHEP 12 (2009) 001 | 0910.2674 |
| 14 | L. M. Carpenter, M. Dine, G. Festuccia, and J. D. Mason | Implementing general gauge mediation | PRD 79 (2009) 035002 | 0805.2944 |
| 15 | T. T. Dumitrescu, Z. Komargodski, N. Seiberg, and D. Shih | General Messenger Gauge Mediation | JHEP 05 (2010) 096 | 1003.2661 |
| 16 | CMS Collaboration | Search for supersymmetry in events with at least one photon, missing transverse momentum, and large transverse event activity in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JHEP 12 (2017) 142 | CMS-SUS-16-047 1707.06193 |
| 17 | CMS Collaboration | Search for gauge-mediated supersymmetry in events with at least one photon and missing transverse momentum in pp collisions at $ \sqrt{s} = $ 13 TeV | PLB780 (2018) 118--143 | CMS-SUS-16-046 1711.08008 |
| 18 | CMS Collaboration | Search for supersymmetry in events with a photon, a lepton, and missing transverse momentum in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JHEP 01 (2019) 154 | CMS-SUS-17-012 1812.04066 |
| 19 | CMS Collaboration | Search for supersymmetry in final states with photons and missing transverse momentum in proton-proton collisions at 13 TeV | Submitted to JHEP | CMS-SUS-17-011 1903.07070 |
| 20 | CMS Collaboration | Interpretation of searches for supersymmetry with simplified models | PRD 88 (2013) 052017 | CMS-SUS-11-016 1301.2175 |
| 21 | P. Grajek, A. Mariotti, and D. Redigolo | Phenomenology of General Gauge Mediation in light of a 125 GeV Higgs | JHEP 07 (2013) 109 | 1303.0870 |
| 22 | S. Knapen, D. Redigolo, and D. Shih | General Gauge Mediation at the Weak Scale | JHEP 03 (2016) 046 | 1507.04364 |
| 23 | S. Knapen and D. Redigolo | Gauge mediation at the LHC: status and prospects | JHEP 01 (2017) 135 | 1606.07501 |
| 24 | W. Beenakker, R. Hopker, and M. Spira | PROSPINO: A Program for the production of supersymmetric particles in next-to-leading order QCD | hep-ph/9611232 | |
| 25 | A. Buckley et al. | LHAPDF6: parton density access in the LHC precision era | EPJC75 (2015) 132 | 1412.7420 |
| 26 | J. Butterworth et al. | PDF4LHC recommendations for LHC Run II | JPG43 (2016) 023001 | 1510.03865 |
| 27 | NNPDF Collaboration | Parton distributions for the LHC Run II | JHEP 04 (2015) 040 | 1410.8849 |
| 28 | The NNPDF collaboration et al. | Parton distributions for the lhc run ii | Journal of High Energy Physics 2015 (Apr, 2015) 40 | |
| 29 | CMS Collaboration | Event generator tunes obtained from underlying event and multiparton scattering measurements | EPJC76 (2016), no. 3, 155 | CMS-GEN-14-001 1512.00815 |
| 30 | W. Beenakker et al. | Production of Charginos, Neutralinos, and Sleptons at Hadron Colliders | PRL 83 (1999) 3780 | hep-ph/9906298 |
| 31 | B. Fuks, M. Klasen, D. R. Lamprea, and M. Rothering | Gaugino production in proton-proton collisions at a center-of-mass energy of 8 TeV | JHEP 10 (2012) 081 | 1207.2159 |
| 32 | B. Fuks, M. Klasen, D. R. Lamprea, and M. Rothering | Precision predictions for electroweak superpartner production at hadron colliders with $ \sc $ resummino | EPJC 73 (2013) 2480 | 1304.0790 |
| 33 | W. Beenakker, R. Hopker, M. Spira, and P. M. Zerwas | Squark and gluino production at hadron colliders | NPB 492 (1997) 51 | hep-ph/9610490 |
| 34 | A. Kulesza and L. Motyka | Threshold resummation for squark-antisquark and gluino-pair production at the LHC | PRL 102 (2009) 111802 | 0807.2405 |
| 35 | A. Kulesza and L. Motyka | Soft gluon resummation for the production of gluino-gluino and squark-antisquark pairs at the LHC | PRD 80 (2009) 095004 | 0905.4749 |
| 36 | W. Beenakker et al. | Soft-gluon resummation for squark and gluino hadroproduction | JHEP 12 (2009) 041 | 0909.4418 |
| 37 | W. Beenakker et al. | Squark and Gluino Hadroproduction | Int. J. Mod. Phys. A 26 (2011) 2637 | 1105.1110 |
| 38 | C. Borschensky et al. | Squark and gluino production cross sections in pp collisions at $ \sqrt{s} = $ 13, 14, 33 and 100 TeV | EPJC 74 (2014) 3174 | 1407.5066 |
| 39 | S. Abdullin et al. | The fast simulation of the CMS detector at LHC | J. Phys.: Conf. Ser. 331 (2011) 032049 | |
| 40 | CMS Collaboration | Performance of photon reconstruction and identification with the CMS detector in proton-proton collisions at $ \sqrt{s} = $ 8 TeV | JINST 10 (2015) P08010 | CMS-EGM-14-001 1502.02702 |
| 41 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | CMS-00-001 |
| 42 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017), no. 10, P10003 | CMS-PRF-14-001 1706.04965 |
| 43 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_t $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 44 | CMS Collaboration | Performance of missing transverse momentum in pp collisions at sqrt(s)=13 TeV using the CMS detector | CMS-PAS-JME-17-001 | CMS-PAS-JME-17-001 |
| 45 | K. Rehermann and B. Tweedie | Efficient Identification of Boosted Semileptonic Top Quarks at the LHC | JHEP 03 (2011) 059 | 1007.2221 |
| 46 | T. Junk | Confidence level computation for combining searches with small statistics | NIMA 434 (1999) 435 | hep-ex/9902006 |
| 47 | A. L. Read | Presentation of search results: the CLs technique | JPG 28 (2002) 2693 | |
| 48 | ATLAS, CMS, and LHC Higgs Combination Group collaborations | Procedure for the LHC Higgs boson search combination in Summer 2011 | CMS-NOTE-2011-005 | |
| 49 | G. Cowan, K. Cranmer, E. Gross, and O. Vitells | Asymptotic formulae for likelihood-based tests of new physics | EPJC 71 (2011) 1554 | 1007.1727 |
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|