CMS-EXO-23-014

CMS-EXO-23-014 ; CERN-EP-2024-025
Search for long-lived particles decaying to final states with a pair of muons in proton-proton collisions at $ \sqrt{s} = $ 13.6 TeV
CMS Collaboration
22 February 2024
JHEP 05 (2024) 047
Abstract: An inclusive search for long-lived exotic particles (LLPs) decaying to final states with a pair of muons is presented. The search uses data corresponding to an integrated luminosity of 36.6 fb$ ^{-1} $ collected by the CMS experiment from the proton-proton collisions at $ \sqrt{s} = $ 13.6 TeV in 2022, the first year of Run 3 of the CERN LHC. The experimental signature is a pair of oppositely charged muons originating from a common vertex spatially separated from the proton-proton interaction point by distances ranging from several hundred $ \mu $m to several meters. The sensitivity of the search benefits from new triggers for displaced dimuons developed for Run 3. The results are interpreted in the framework of the hidden Abelian Higgs model, in which the Higgs boson decays to a pair of long-lived dark photons, and of an $ R $-parity violating supersymmetry model, in which long-lived neutralinos decay to a pair of muons and a neutrino. The limits set on these models are the most stringent to date in wide regions of lifetimes for LLPs with masses larger than 10 GeV.
Links: e-print arXiv:2402.14491 [hep-ex] (PDF) ; CDS record ; inSPIRE record ; HepData record ; Physics Briefing ; CADI line (restricted) ;

Figures	Summary	Additional Figures & Material	References	CMS Publications

Instructions for reinterpretation can be found here

Figures
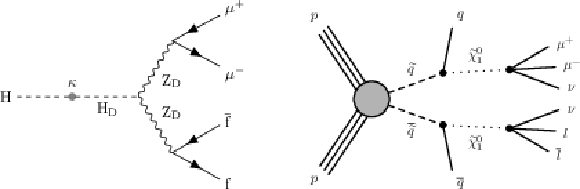
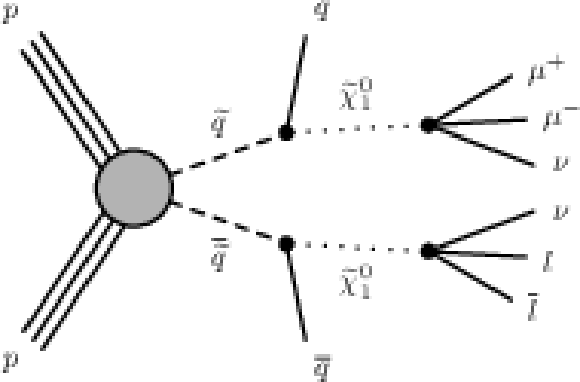
png pdf	Figure 1: Feynman diagrams for (left) the HAHM model, showing the production of long-lived dark photons $ \mathrm{Z}_\text{D} $ via the Higgs portal, through $ \mathrm{H} $-$ \mathrm{H}_\text{D} $ mixing with the parameter $ \kappa $, with subsequent decays to pairs of muons or other fermions via the vector portal; and (right) pair production of squarks followed by $ \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} $ decays, where the RPV neutralino is assumed to be a long-lived particle that decays into a neutrino and two charged leptons.
png pdf	Figure 1-a: Feynman diagrams for (left) the HAHM model, showing the production of long-lived dark photons $ \mathrm{Z}_\text{D} $ via the Higgs portal, through $ \mathrm{H} $-$ \mathrm{H}_\text{D} $ mixing with the parameter $ \kappa $, with subsequent decays to pairs of muons or other fermions via the vector portal; and (right) pair production of squarks followed by $ \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} $ decays, where the RPV neutralino is assumed to be a long-lived particle that decays into a neutrino and two charged leptons.
png pdf	Figure 1-b: Feynman diagrams for (left) the HAHM model, showing the production of long-lived dark photons $ \mathrm{Z}_\text{D} $ via the Higgs portal, through $ \mathrm{H} $-$ \mathrm{H}_\text{D} $ mixing with the parameter $ \kappa $, with subsequent decays to pairs of muons or other fermions via the vector portal; and (right) pair production of squarks followed by $ \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} $ decays, where the RPV neutralino is assumed to be a long-lived particle that decays into a neutrino and two charged leptons.
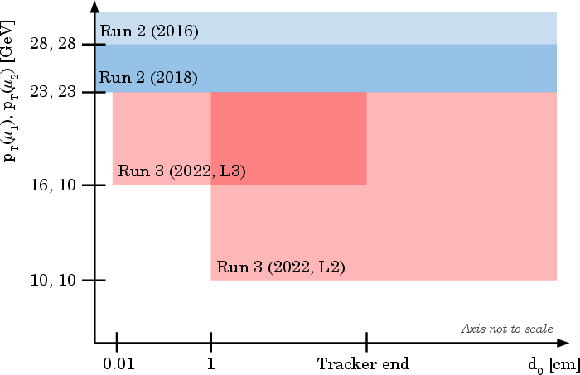
png pdf	Figure 2: The $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ coverage of the 2016 Run 2 triggers (light blue), 2018 Run 2 triggers (blue), and newly designed 2022 Run 3 triggers described in the text (red). The two values of the $ p_{\mathrm{T}} $ refer to the trigger thresholds for the muons.
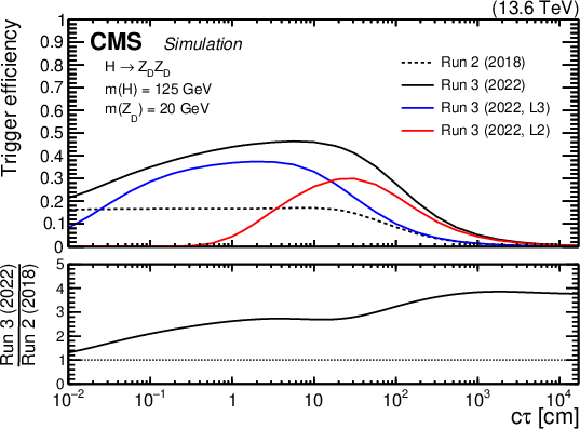
png pdf	Figure 3: Efficiencies of the Run 2 and Run 3 displaced dimuon triggers as a function of $ c\tau $ for the HAHM signal events with $ m(\mathrm{Z}_\text{D}) = $ 20 GeV. The efficiency is defined as the fraction of simulated events that satisfy the detector acceptance and the requirements of the following sets of trigger paths: the Run 2 (2018) triggers (dashed black); the Run 3 (2022, L3) triggers (blue); the Run 3 (2022, L2) triggers (red); and the OR of all these triggers (Run 3 (2022), black). The lower panel shows the ratio of the overall Run 3 (2022) efficiency to the Run 2 (2018) efficiency.
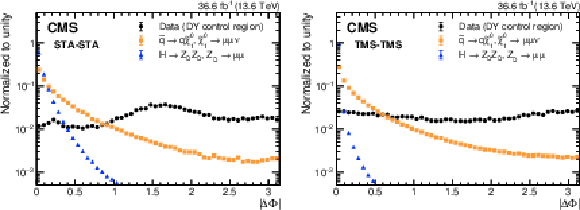
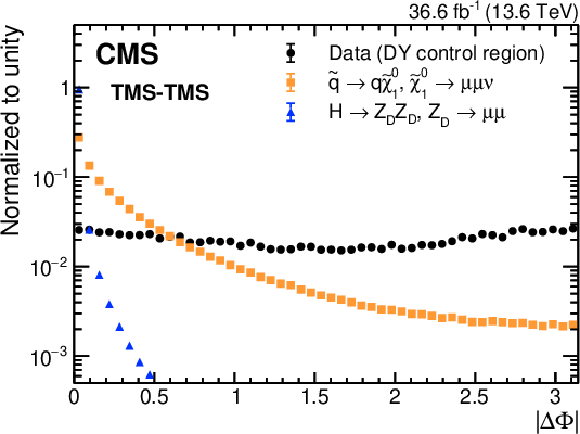
png pdf	Figure 4: Distributions of $ \|\Delta\Phi\| $ for (left) STA-STA and (right) TMS-TMS dimuons in data samples obtained by inverting some of the selection criteria and enriched in DY events (black circles) and for events passing all selection criteria except for a requirement on $ \|\Delta\Phi\| $ in all HAHM (blue triangles) and RPV SUSY (orange squares) generated signal samples combined. All distributions are normalized to unit area.
png pdf	Figure 4-a: Distributions of $ \|\Delta\Phi\| $ for (left) STA-STA and (right) TMS-TMS dimuons in data samples obtained by inverting some of the selection criteria and enriched in DY events (black circles) and for events passing all selection criteria except for a requirement on $ \|\Delta\Phi\| $ in all HAHM (blue triangles) and RPV SUSY (orange squares) generated signal samples combined. All distributions are normalized to unit area.
png pdf	Figure 4-b: Distributions of $ \|\Delta\Phi\| $ for (left) STA-STA and (right) TMS-TMS dimuons in data samples obtained by inverting some of the selection criteria and enriched in DY events (black circles) and for events passing all selection criteria except for a requirement on $ \|\Delta\Phi\| $ in all HAHM (blue triangles) and RPV SUSY (orange squares) generated signal samples combined. All distributions are normalized to unit area.
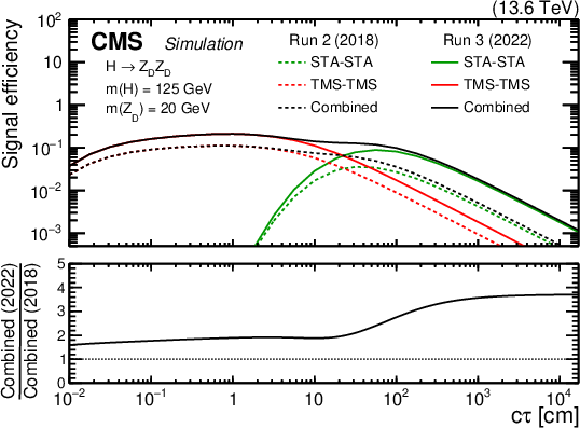
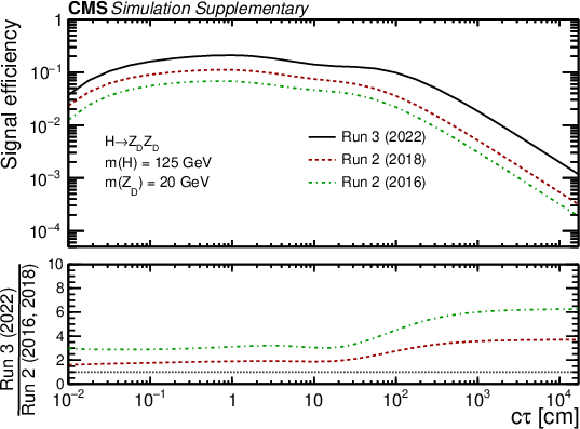
png pdf	Figure 5: Overall efficiencies in the STA-STA (green) and TMS-TMS (red) dimuon categories, as well as their combination (black) as a function of $ c\tau $ for the HAHM signal events with $ m(\mathrm{Z}_\text{D})= $ 20 GeV. The solid curves show efficiencies achieved with the 2022 Run 3 triggers, whereas dashed curves show efficiencies for the subset of events selected by the triggers used in the 2018 Run 2 analysis. The efficiency is defined as the fraction of signal events that satisfy the criteria of the indicated trigger as well as the full set of offline selection criteria. The lower panel shows the relative improvement of the overall signal efficiency brought in by improvements in the trigger.
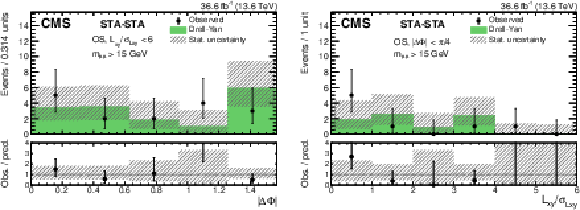
png pdf	Figure 6: Example of background prediction checks in the STA-STA category: distributions of (left) $ \|\Delta\Phi\| $ and (right) $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} $ for events with $ m_{\mu\mu} > $ 15 GeV in the $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} < $ 6 validation region in data (black circles) compared to the background predictions (histograms). The lower panels show the ratio of the observed to predicted number of events. Hatched histograms show the statistical uncertainty in the background prediction.
png pdf	Figure 6-a: Example of background prediction checks in the STA-STA category: distributions of (left) $ \|\Delta\Phi\| $ and (right) $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} $ for events with $ m_{\mu\mu} > $ 15 GeV in the $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} < $ 6 validation region in data (black circles) compared to the background predictions (histograms). The lower panels show the ratio of the observed to predicted number of events. Hatched histograms show the statistical uncertainty in the background prediction.
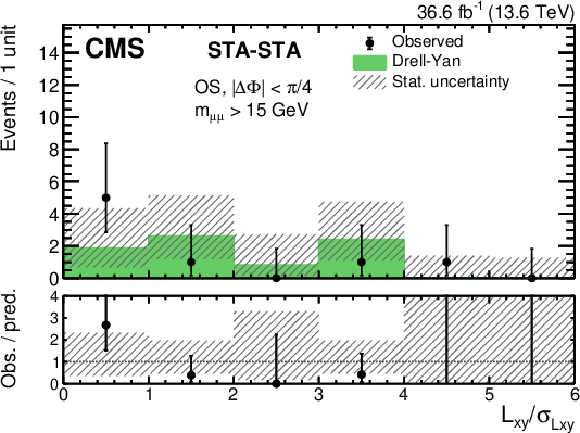
png pdf	Figure 6-b: Example of background prediction checks in the STA-STA category: distributions of (left) $ \|\Delta\Phi\| $ and (right) $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} $ for events with $ m_{\mu\mu} > $ 15 GeV in the $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} < $ 6 validation region in data (black circles) compared to the background predictions (histograms). The lower panels show the ratio of the observed to predicted number of events. Hatched histograms show the statistical uncertainty in the background prediction.
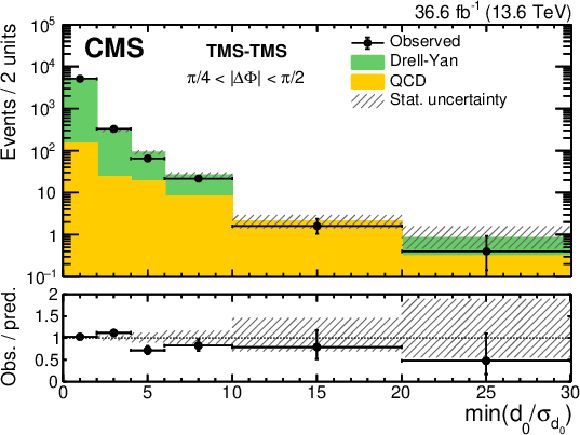
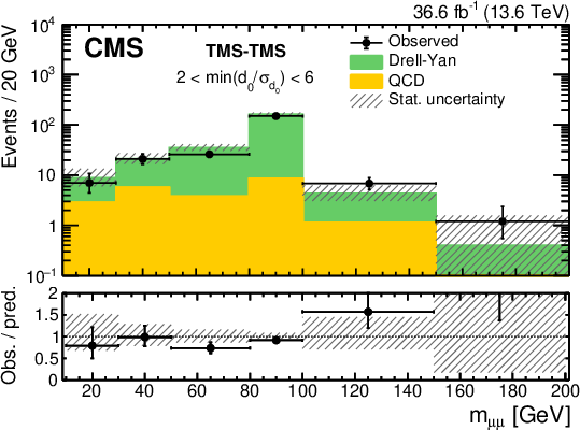
png pdf	Figure 7: Example of background prediction checks in the TMS-TMS category: $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} $ distributions for events with (left) $ \|\Delta\Phi\| < \pi/ $4 and (right) $ \|\Delta\Phi\| < \pi/ $ 30 in the 2 $ < \text{min}(d_{\text{0}}/\sigma_{d_{\text{0}}}) < $ 6 validation regions compared to the background predictions. The number of observed events (black circles) is overlaid with stacked histograms showing the expected numbers of QCD (yellow) and DY (green) background events. The last bin includes events in the histogram overflow. The lower panels show the ratio of the observed to predicted number of events. Hatched histograms show the statistical uncertainty in the background prediction.
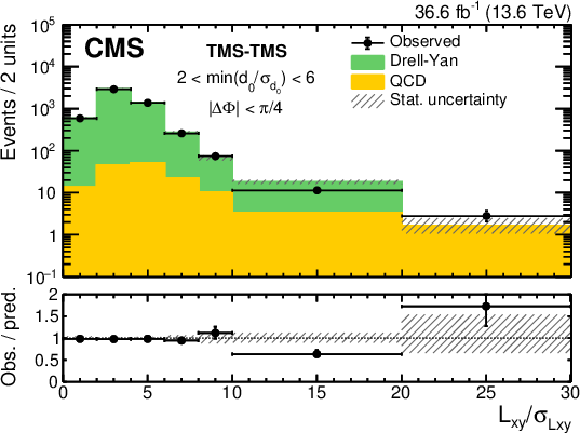
png pdf	Figure 7-a: Example of background prediction checks in the TMS-TMS category: $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} $ distributions for events with (left) $ \|\Delta\Phi\| < \pi/ $4 and (right) $ \|\Delta\Phi\| < \pi/ $ 30 in the 2 $ < \text{min}(d_{\text{0}}/\sigma_{d_{\text{0}}}) < $ 6 validation regions compared to the background predictions. The number of observed events (black circles) is overlaid with stacked histograms showing the expected numbers of QCD (yellow) and DY (green) background events. The last bin includes events in the histogram overflow. The lower panels show the ratio of the observed to predicted number of events. Hatched histograms show the statistical uncertainty in the background prediction.
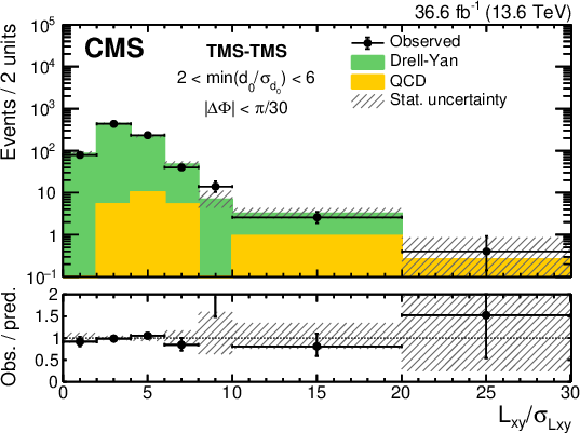
png pdf	Figure 7-b: Example of background prediction checks in the TMS-TMS category: $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} $ distributions for events with (left) $ \|\Delta\Phi\| < \pi/ $4 and (right) $ \|\Delta\Phi\| < \pi/ $ 30 in the 2 $ < \text{min}(d_{\text{0}}/\sigma_{d_{\text{0}}}) < $ 6 validation regions compared to the background predictions. The number of observed events (black circles) is overlaid with stacked histograms showing the expected numbers of QCD (yellow) and DY (green) background events. The last bin includes events in the histogram overflow. The lower panels show the ratio of the observed to predicted number of events. Hatched histograms show the statistical uncertainty in the background prediction.
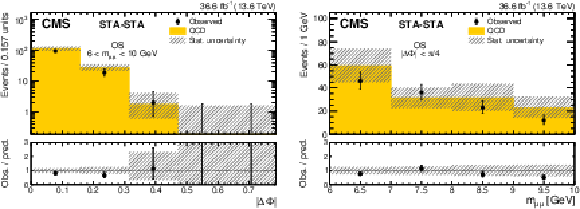
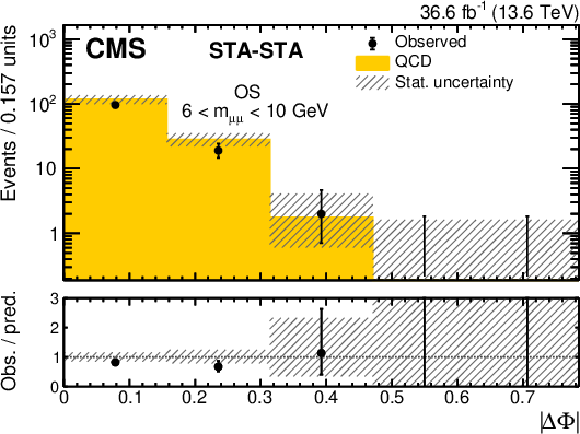
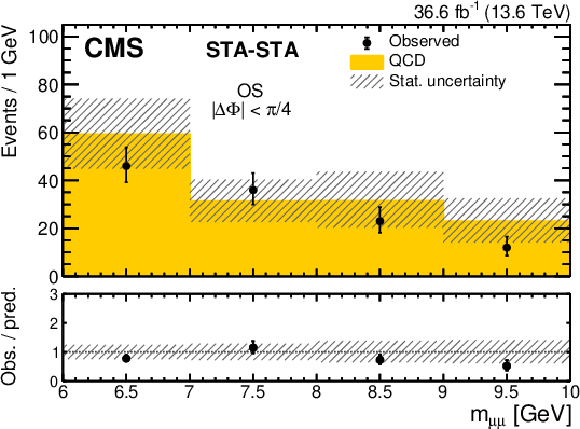
png pdf	Figure 8: Example of background prediction checks in the STA-STA category: distributions of (left) $ \|\Delta\Phi\| $ and (right) $ m_{\mu\mu} $ for dimuons in the low-mass (6 $ < m_{\mu\mu} < $ 10 GeV) validation region in data (black circles) compared to the background predictions (histograms). The lower panels show the ratio of the observed to predicted number of events. Hatched histograms show the statistical uncertainty in the background prediction.
png pdf	Figure 8-a: Example of background prediction checks in the STA-STA category: distributions of (left) $ \|\Delta\Phi\| $ and (right) $ m_{\mu\mu} $ for dimuons in the low-mass (6 $ < m_{\mu\mu} < $ 10 GeV) validation region in data (black circles) compared to the background predictions (histograms). The lower panels show the ratio of the observed to predicted number of events. Hatched histograms show the statistical uncertainty in the background prediction.
png pdf	Figure 8-b: Example of background prediction checks in the STA-STA category: distributions of (left) $ \|\Delta\Phi\| $ and (right) $ m_{\mu\mu} $ for dimuons in the low-mass (6 $ < m_{\mu\mu} < $ 10 GeV) validation region in data (black circles) compared to the background predictions (histograms). The lower panels show the ratio of the observed to predicted number of events. Hatched histograms show the statistical uncertainty in the background prediction.
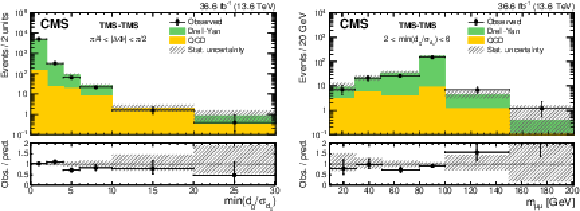
png pdf	Figure 9: Example of background prediction checks in the TMS-TMS category: (left) distribution of min($ d_{\text{0}}/\sigma_{d_{\text{0}}} $) for events in the $ \pi/4 < \|\Delta\Phi\| < \pi/ $2 validation region; (right) distribution of $ m_{\mu\mu} $ for events in the 2 $ < \text{min}(d_{\text{0}}/\sigma_{d_{\text{0}}}) < $ 6 validation region. The number of observed events (black circles) is overlaid with stacked histograms showing the expected numbers of QCD (yellow) and DY (green) background events. The last bin includes events in the histogram overflow. The lower panels show the ratios of the observed to predicted number of events. Hatched histograms show the statistical uncertainty in the background prediction.
png pdf	Figure 9-a: Example of background prediction checks in the TMS-TMS category: (left) distribution of min($ d_{\text{0}}/\sigma_{d_{\text{0}}} $) for events in the $ \pi/4 < \|\Delta\Phi\| < \pi/ $2 validation region; (right) distribution of $ m_{\mu\mu} $ for events in the 2 $ < \text{min}(d_{\text{0}}/\sigma_{d_{\text{0}}}) < $ 6 validation region. The number of observed events (black circles) is overlaid with stacked histograms showing the expected numbers of QCD (yellow) and DY (green) background events. The last bin includes events in the histogram overflow. The lower panels show the ratios of the observed to predicted number of events. Hatched histograms show the statistical uncertainty in the background prediction.
png pdf	Figure 9-b: Example of background prediction checks in the TMS-TMS category: (left) distribution of min($ d_{\text{0}}/\sigma_{d_{\text{0}}} $) for events in the $ \pi/4 < \|\Delta\Phi\| < \pi/ $2 validation region; (right) distribution of $ m_{\mu\mu} $ for events in the 2 $ < \text{min}(d_{\text{0}}/\sigma_{d_{\text{0}}}) < $ 6 validation region. The number of observed events (black circles) is overlaid with stacked histograms showing the expected numbers of QCD (yellow) and DY (green) background events. The last bin includes events in the histogram overflow. The lower panels show the ratios of the observed to predicted number of events. Hatched histograms show the statistical uncertainty in the background prediction.
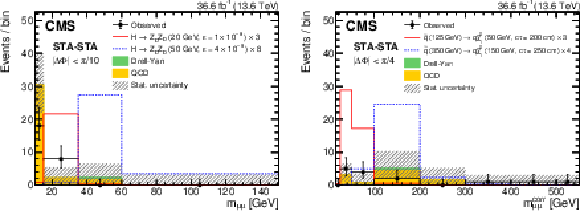
png pdf	Figure 10: Comparison of the observed (black points) and expected (histograms) numbers of events in nonoverlapping (left) $ m_{\mu\mu} $ and (right) $ m^\text{corr}_{\mu\mu} $ intervals in the STA-STA dimuon category, in the signal regions optimized for the (left) HAHM and (right) RPV SUSY model. Yellow and green stacked filled histograms represent mean expected background contributions from QCD and DY, respectively, while statistical uncertainties in the total expected background are shown as hatched histograms. Signal contributions expected from simulated signals indicated in the legends are shown in red and blue. Their yields are set to the corresponding median expected 95% CL exclusion limits obtained from the ensemble of both dimuon categories, scaled up as indicated in the legend to improve visibility. The last bin includes events in the histogram overflow.
png pdf	Figure 10-a: Comparison of the observed (black points) and expected (histograms) numbers of events in nonoverlapping (left) $ m_{\mu\mu} $ and (right) $ m^\text{corr}_{\mu\mu} $ intervals in the STA-STA dimuon category, in the signal regions optimized for the (left) HAHM and (right) RPV SUSY model. Yellow and green stacked filled histograms represent mean expected background contributions from QCD and DY, respectively, while statistical uncertainties in the total expected background are shown as hatched histograms. Signal contributions expected from simulated signals indicated in the legends are shown in red and blue. Their yields are set to the corresponding median expected 95% CL exclusion limits obtained from the ensemble of both dimuon categories, scaled up as indicated in the legend to improve visibility. The last bin includes events in the histogram overflow.
png pdf	Figure 10-b: Comparison of the observed (black points) and expected (histograms) numbers of events in nonoverlapping (left) $ m_{\mu\mu} $ and (right) $ m^\text{corr}_{\mu\mu} $ intervals in the STA-STA dimuon category, in the signal regions optimized for the (left) HAHM and (right) RPV SUSY model. Yellow and green stacked filled histograms represent mean expected background contributions from QCD and DY, respectively, while statistical uncertainties in the total expected background are shown as hatched histograms. Signal contributions expected from simulated signals indicated in the legends are shown in red and blue. Their yields are set to the corresponding median expected 95% CL exclusion limits obtained from the ensemble of both dimuon categories, scaled up as indicated in the legend to improve visibility. The last bin includes events in the histogram overflow.
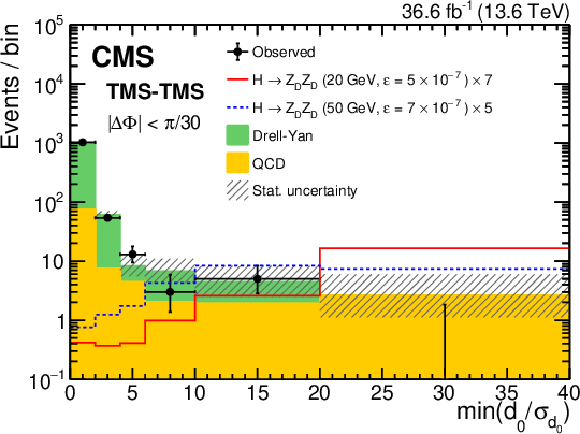
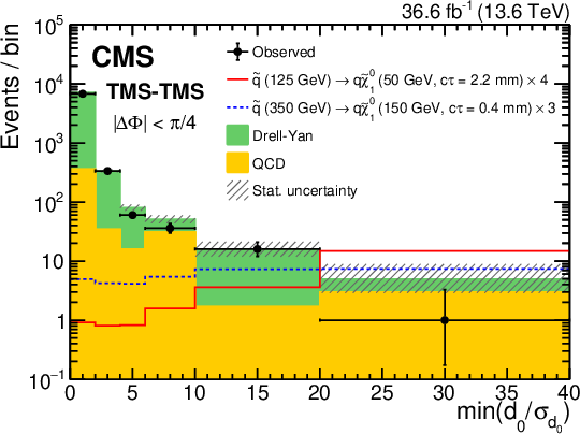
png pdf	Figure 11: Distributions of $ \text{min}(d_{\text{0}}/\sigma_{d_{\text{0}}}) $ for TMS-TMS dimuons with (left) $ \|\Delta\Phi\| < \pi/ $ 30 and (right) $ \|\Delta\Phi\| < \pi/ $ 4, for events in all mass intervals combined. The number of observed events (black circles) is overlaid with the stacked histograms showing the expected numbers of QCD (yellow) and DY (green) background events. Statistical uncertainties in the total expected background are shown as hatched histograms. Signal contributions expected from simulated signals indicated in the legends are shown in red and blue. Their yields are set to the corresponding median expected 95% CL exclusion limits obtained from the ensemble of both dimuon categories, scaled up as indicated in the legend to improve visibility. Events are required to satisfy all nominal selection criteria with the exception of the $ d_{\text{0}}/\sigma_{d_{\text{0}}} $ requirement. The last bin includes events in the histogram overflow.
png pdf	Figure 11-a: Distributions of $ \text{min}(d_{\text{0}}/\sigma_{d_{\text{0}}}) $ for TMS-TMS dimuons with (left) $ \|\Delta\Phi\| < \pi/ $ 30 and (right) $ \|\Delta\Phi\| < \pi/ $ 4, for events in all mass intervals combined. The number of observed events (black circles) is overlaid with the stacked histograms showing the expected numbers of QCD (yellow) and DY (green) background events. Statistical uncertainties in the total expected background are shown as hatched histograms. Signal contributions expected from simulated signals indicated in the legends are shown in red and blue. Their yields are set to the corresponding median expected 95% CL exclusion limits obtained from the ensemble of both dimuon categories, scaled up as indicated in the legend to improve visibility. Events are required to satisfy all nominal selection criteria with the exception of the $ d_{\text{0}}/\sigma_{d_{\text{0}}} $ requirement. The last bin includes events in the histogram overflow.
png pdf	Figure 11-b: Distributions of $ \text{min}(d_{\text{0}}/\sigma_{d_{\text{0}}}) $ for TMS-TMS dimuons with (left) $ \|\Delta\Phi\| < \pi/ $ 30 and (right) $ \|\Delta\Phi\| < \pi/ $ 4, for events in all mass intervals combined. The number of observed events (black circles) is overlaid with the stacked histograms showing the expected numbers of QCD (yellow) and DY (green) background events. Statistical uncertainties in the total expected background are shown as hatched histograms. Signal contributions expected from simulated signals indicated in the legends are shown in red and blue. Their yields are set to the corresponding median expected 95% CL exclusion limits obtained from the ensemble of both dimuon categories, scaled up as indicated in the legend to improve visibility. Events are required to satisfy all nominal selection criteria with the exception of the $ d_{\text{0}}/\sigma_{d_{\text{0}}} $ requirement. The last bin includes events in the histogram overflow.
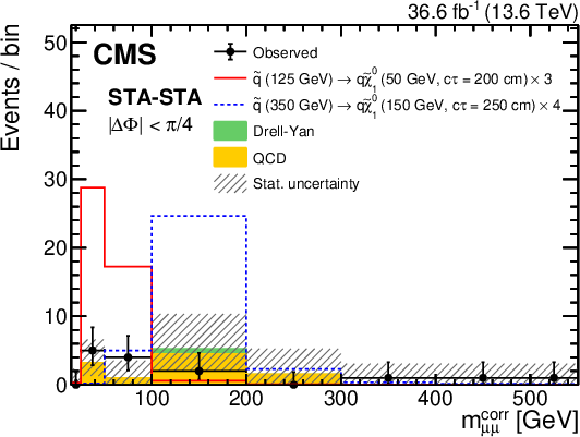
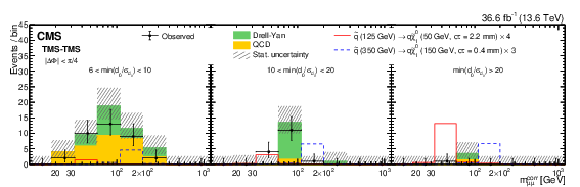
png pdf	Figure 12: Comparison of observed and expected numbers of events in the TMS-TMS dimuon category, in the RPV SUSY study that requires $ \|\Delta\Phi\| < \pi/ $ 4, in bins of $ m^\text{corr}_{\mu\mu} $. The number of observed events (black circles) is overlaid with the stacked filled histograms showing the expected numbers of QCD (yellow) and DY (green) background events in bins of $ m^\text{corr}_{\mu\mu} $ in three min($ d_{\text{0}}/\sigma_{d_{\text{0}}} $) bins: (left) 6--10, (center) 10--20, and (right) $ {>} $ 20. Hatched histograms show statistical uncertainties in the total expected background. Contributions expected from signal events predicted by the RPV SUSY model with the parameters indicated in the legends are shown as red and blue histograms. Their yields are set to the corresponding median expected 95% CL exclusion limits obtained from the ensemble of both dimuon categories, scaled up as indicated in the legend to improve visibility. The last bin includes events in the histogram overflow.
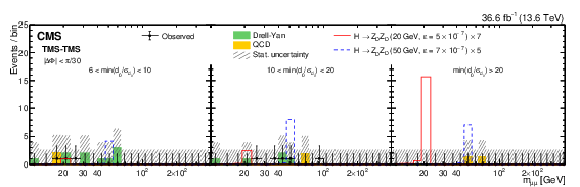
png pdf	Figure 13: Comparison of observed and expected numbers of events in the TMS-TMS dimuon category, in the HAHM study that requires $ \|\Delta\Phi\| < \pi/ $30, in bins of $ m_{\mu\mu} $. The number of observed events (black circles) is overlaid with the stacked histograms showing the expected numbers of QCD (yellow) and DY (green) background events in bins of $ m_{\mu\mu} $ in three min($ d_{\text{0}}/\sigma_{d_{\text{0}}} $) bins: (left) 6--10, (center) 10--20, and (right) $ {>} $ 20. Hatched histograms show statistical uncertainties in the total expected background. Signal contributions expected from simulated $ \mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D} $ events with the parameters indicated in the legends are shown as red and blue histograms. Their yields are set to the corresponding median expected 95% CL exclusion limits obtained from the ensemble of both dimuon categories, scaled up as indicated in the legend to improve visibility. The last bin includes events in the histogram overflow.
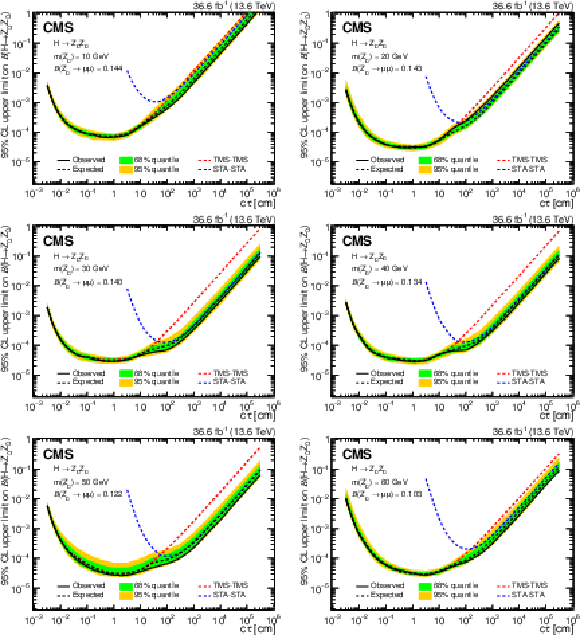
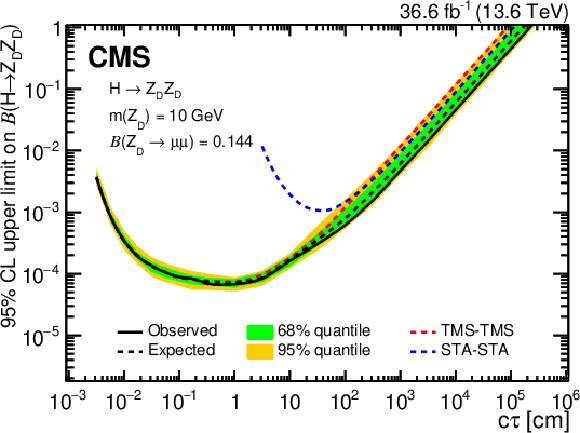
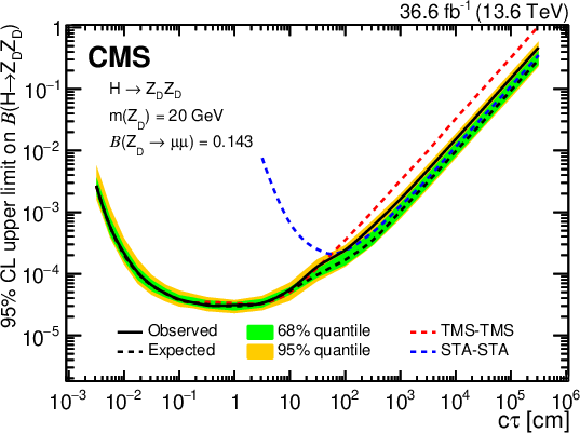
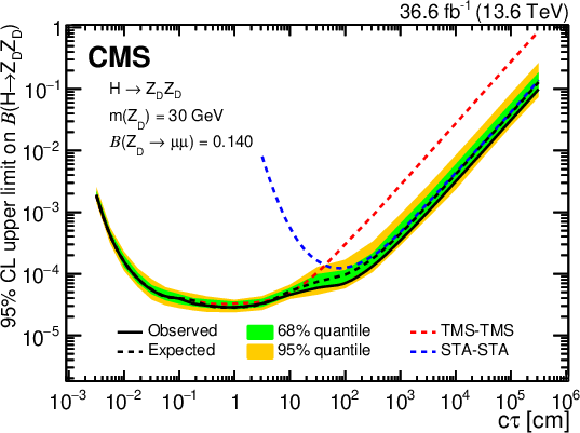
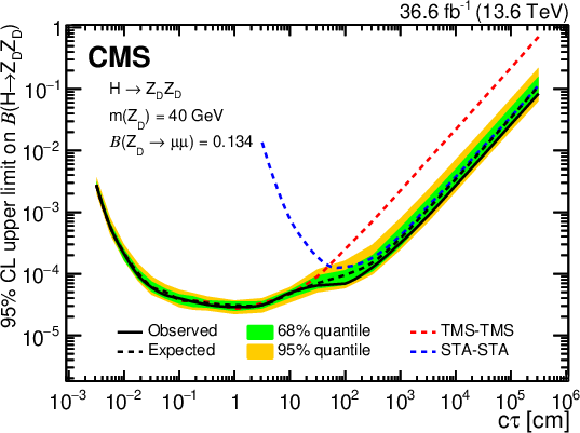
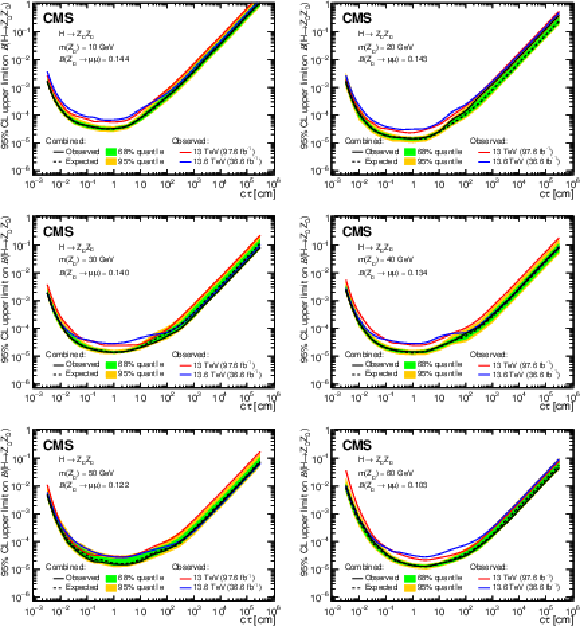
png pdf	Figure 14: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, in the STA-STA and TMS-TMS dimuon categories in 2022 data and their combination. The median expected limits obtained from the STA-STA and TMS-TMS dimuon categories are shown as dashed blue and red curves, respectively; the combined median expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
png pdf	Figure 14-a: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, in the STA-STA and TMS-TMS dimuon categories in 2022 data and their combination. The median expected limits obtained from the STA-STA and TMS-TMS dimuon categories are shown as dashed blue and red curves, respectively; the combined median expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
png pdf	Figure 14-b: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, in the STA-STA and TMS-TMS dimuon categories in 2022 data and their combination. The median expected limits obtained from the STA-STA and TMS-TMS dimuon categories are shown as dashed blue and red curves, respectively; the combined median expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
png pdf	Figure 14-c: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, in the STA-STA and TMS-TMS dimuon categories in 2022 data and their combination. The median expected limits obtained from the STA-STA and TMS-TMS dimuon categories are shown as dashed blue and red curves, respectively; the combined median expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
png pdf	Figure 14-d: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, in the STA-STA and TMS-TMS dimuon categories in 2022 data and their combination. The median expected limits obtained from the STA-STA and TMS-TMS dimuon categories are shown as dashed blue and red curves, respectively; the combined median expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
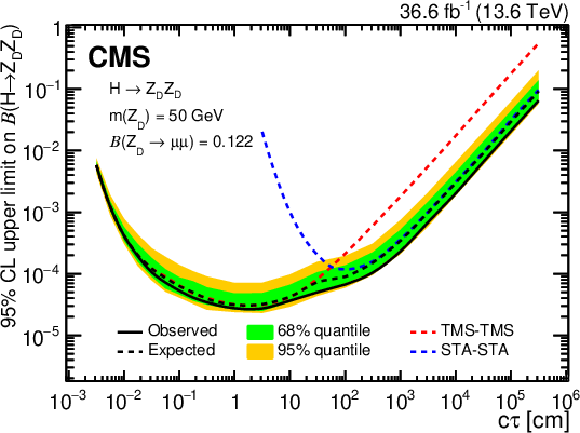
png pdf	Figure 14-e: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, in the STA-STA and TMS-TMS dimuon categories in 2022 data and their combination. The median expected limits obtained from the STA-STA and TMS-TMS dimuon categories are shown as dashed blue and red curves, respectively; the combined median expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
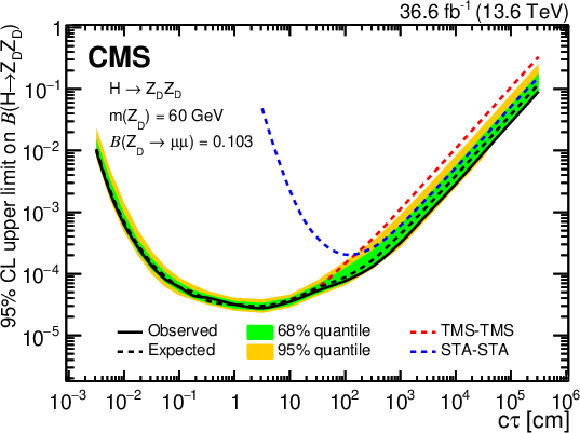
png pdf	Figure 14-f: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, in the STA-STA and TMS-TMS dimuon categories in 2022 data and their combination. The median expected limits obtained from the STA-STA and TMS-TMS dimuon categories are shown as dashed blue and red curves, respectively; the combined median expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
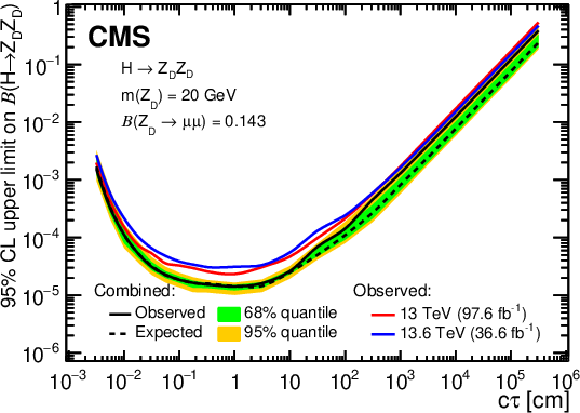
png pdf	Figure 15: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, obtained in this analysis, the Run 2 analysis [5], and their combination. The observed limits in this analysis and in the Run 2 analysis [5] are shown as blue and red curves, respectively; the median combined expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
png pdf	Figure 15-a: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, obtained in this analysis, the Run 2 analysis [5], and their combination. The observed limits in this analysis and in the Run 2 analysis [5] are shown as blue and red curves, respectively; the median combined expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
png pdf	Figure 15-b: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, obtained in this analysis, the Run 2 analysis [5], and their combination. The observed limits in this analysis and in the Run 2 analysis [5] are shown as blue and red curves, respectively; the median combined expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
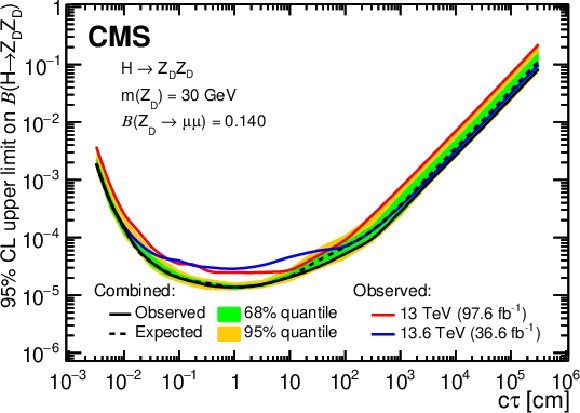
png pdf	Figure 15-c: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, obtained in this analysis, the Run 2 analysis [5], and their combination. The observed limits in this analysis and in the Run 2 analysis [5] are shown as blue and red curves, respectively; the median combined expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
png pdf	Figure 15-d: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, obtained in this analysis, the Run 2 analysis [5], and their combination. The observed limits in this analysis and in the Run 2 analysis [5] are shown as blue and red curves, respectively; the median combined expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
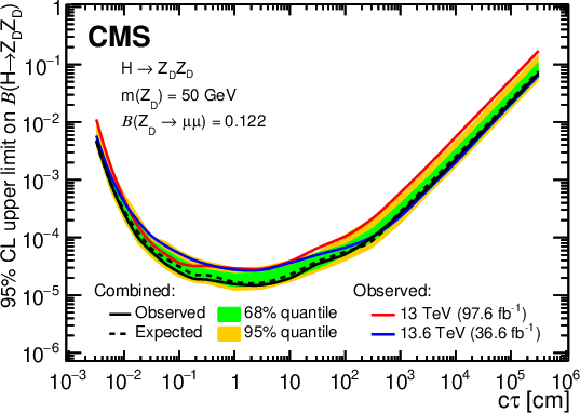
png pdf	Figure 15-e: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, obtained in this analysis, the Run 2 analysis [5], and their combination. The observed limits in this analysis and in the Run 2 analysis [5] are shown as blue and red curves, respectively; the median combined expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
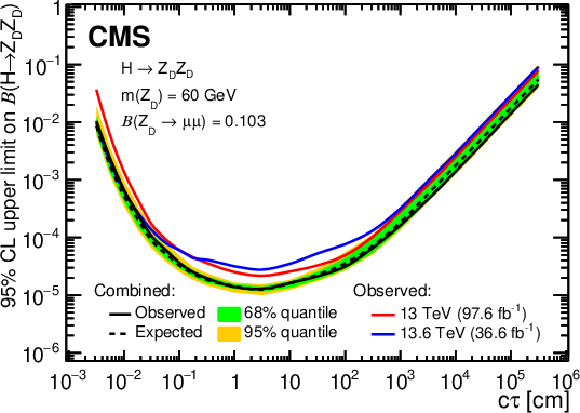
png pdf	Figure 15-f: The 95% CL upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ in the HAHM model, for $ m(\mathrm{Z}_\text{D}) $ ranging from (upper left) 10 GeV to (lower right) 60 GeV, obtained in this analysis, the Run 2 analysis [5], and their combination. The observed limits in this analysis and in the Run 2 analysis [5] are shown as blue and red curves, respectively; the median combined expected limits are shown as dashed black curves; and the combined observed limits are shown as solid black curves. The green and yellow bands correspond, respectively, to the 68 and 95% quantiles for the combined expected limits.
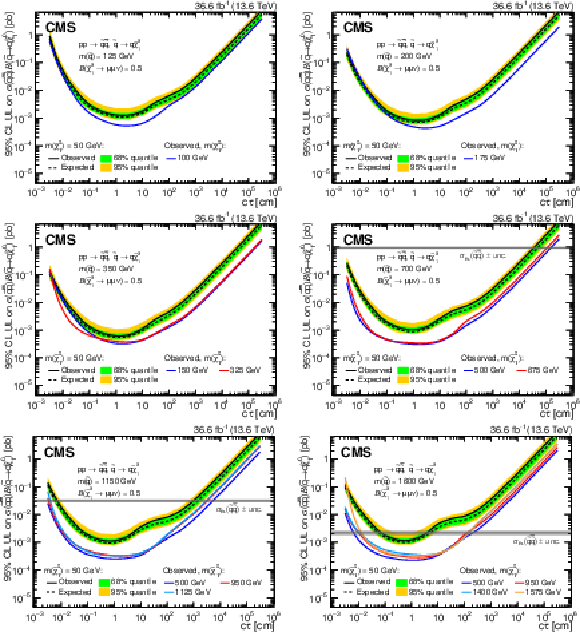
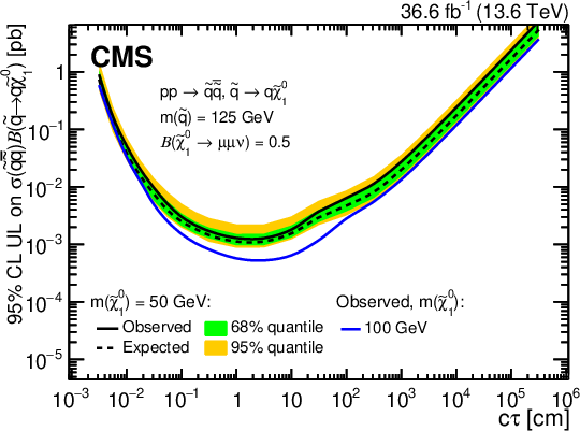
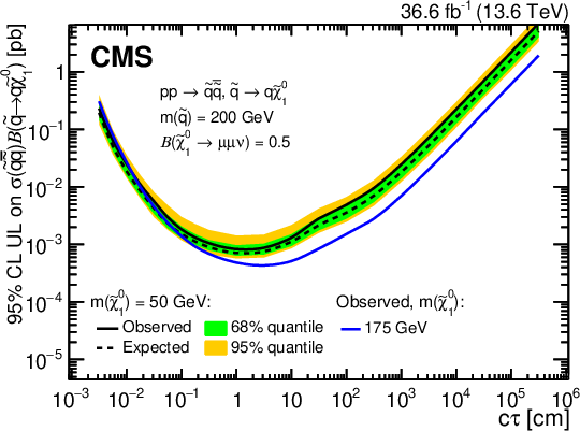
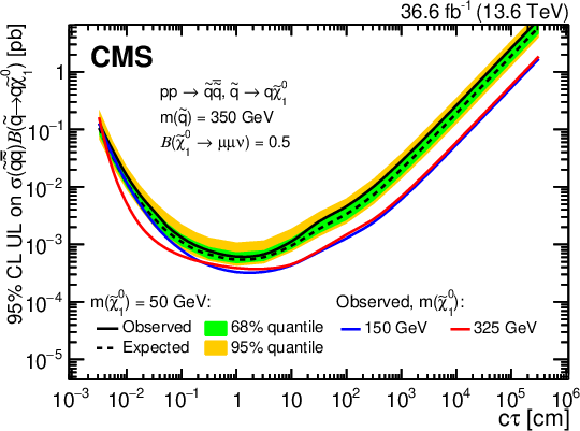
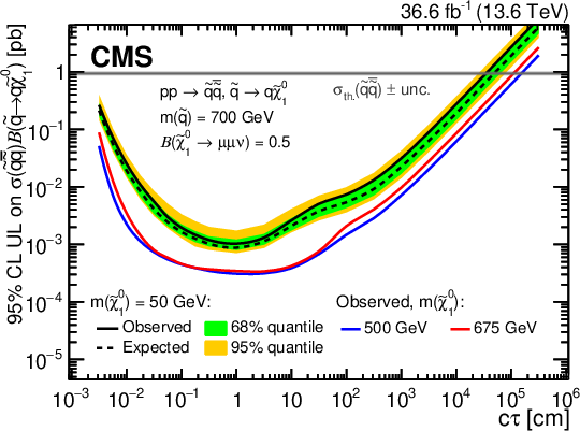
png pdf	Figure 16: The 95% CL upper limits on $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}})\mathcal{B}( \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} ) $ as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ in the RPV SUSY model, for $ \mathcal{B}(\tilde{\chi}_{1}^{0}\to\mu^{+}\mu^{-}\nu) = $ 0.5 and $ m({\tilde{\mathrm{q}}} ) $ ranging from (upper left) 125 GeV to (lower right) 1.6 TeV. The observed limits for various combinations of $ m({\tilde{\mathrm{q}}} ) $ and $ m(\tilde{\chi}_{1}^{0}) $ indicated in the legends are shown as solid curves. The median expected limits and their 68 and 95% quantiles are shown, respectively, as dashed black curves and green and yellow bands for the case of $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV and omitted for other neutralino masses for clarity. The gray horizontal lines indicate the theoretical values of the squark-antisquark production cross sections with the uncertainties shown as gray shaded bands. The predicted cross sections for $ m({\tilde{\mathrm{q}}} ) = $ 125, 200, and 350 GeV are, respectively, 7200, 840, and 50 pb, and fall outside the $ y $-axis range.
png pdf	Figure 16-a: The 95% CL upper limits on $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}})\mathcal{B}( \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} ) $ as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ in the RPV SUSY model, for $ \mathcal{B}(\tilde{\chi}_{1}^{0}\to\mu^{+}\mu^{-}\nu) = $ 0.5 and $ m({\tilde{\mathrm{q}}} ) $ ranging from (upper left) 125 GeV to (lower right) 1.6 TeV. The observed limits for various combinations of $ m({\tilde{\mathrm{q}}} ) $ and $ m(\tilde{\chi}_{1}^{0}) $ indicated in the legends are shown as solid curves. The median expected limits and their 68 and 95% quantiles are shown, respectively, as dashed black curves and green and yellow bands for the case of $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV and omitted for other neutralino masses for clarity. The gray horizontal lines indicate the theoretical values of the squark-antisquark production cross sections with the uncertainties shown as gray shaded bands. The predicted cross sections for $ m({\tilde{\mathrm{q}}} ) = $ 125, 200, and 350 GeV are, respectively, 7200, 840, and 50 pb, and fall outside the $ y $-axis range.
png pdf	Figure 16-b: The 95% CL upper limits on $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}})\mathcal{B}( \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} ) $ as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ in the RPV SUSY model, for $ \mathcal{B}(\tilde{\chi}_{1}^{0}\to\mu^{+}\mu^{-}\nu) = $ 0.5 and $ m({\tilde{\mathrm{q}}} ) $ ranging from (upper left) 125 GeV to (lower right) 1.6 TeV. The observed limits for various combinations of $ m({\tilde{\mathrm{q}}} ) $ and $ m(\tilde{\chi}_{1}^{0}) $ indicated in the legends are shown as solid curves. The median expected limits and their 68 and 95% quantiles are shown, respectively, as dashed black curves and green and yellow bands for the case of $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV and omitted for other neutralino masses for clarity. The gray horizontal lines indicate the theoretical values of the squark-antisquark production cross sections with the uncertainties shown as gray shaded bands. The predicted cross sections for $ m({\tilde{\mathrm{q}}} ) = $ 125, 200, and 350 GeV are, respectively, 7200, 840, and 50 pb, and fall outside the $ y $-axis range.
png pdf	Figure 16-c: The 95% CL upper limits on $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}})\mathcal{B}( \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} ) $ as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ in the RPV SUSY model, for $ \mathcal{B}(\tilde{\chi}_{1}^{0}\to\mu^{+}\mu^{-}\nu) = $ 0.5 and $ m({\tilde{\mathrm{q}}} ) $ ranging from (upper left) 125 GeV to (lower right) 1.6 TeV. The observed limits for various combinations of $ m({\tilde{\mathrm{q}}} ) $ and $ m(\tilde{\chi}_{1}^{0}) $ indicated in the legends are shown as solid curves. The median expected limits and their 68 and 95% quantiles are shown, respectively, as dashed black curves and green and yellow bands for the case of $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV and omitted for other neutralino masses for clarity. The gray horizontal lines indicate the theoretical values of the squark-antisquark production cross sections with the uncertainties shown as gray shaded bands. The predicted cross sections for $ m({\tilde{\mathrm{q}}} ) = $ 125, 200, and 350 GeV are, respectively, 7200, 840, and 50 pb, and fall outside the $ y $-axis range.
png pdf	Figure 16-d: The 95% CL upper limits on $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}})\mathcal{B}( \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} ) $ as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ in the RPV SUSY model, for $ \mathcal{B}(\tilde{\chi}_{1}^{0}\to\mu^{+}\mu^{-}\nu) = $ 0.5 and $ m({\tilde{\mathrm{q}}} ) $ ranging from (upper left) 125 GeV to (lower right) 1.6 TeV. The observed limits for various combinations of $ m({\tilde{\mathrm{q}}} ) $ and $ m(\tilde{\chi}_{1}^{0}) $ indicated in the legends are shown as solid curves. The median expected limits and their 68 and 95% quantiles are shown, respectively, as dashed black curves and green and yellow bands for the case of $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV and omitted for other neutralino masses for clarity. The gray horizontal lines indicate the theoretical values of the squark-antisquark production cross sections with the uncertainties shown as gray shaded bands. The predicted cross sections for $ m({\tilde{\mathrm{q}}} ) = $ 125, 200, and 350 GeV are, respectively, 7200, 840, and 50 pb, and fall outside the $ y $-axis range.
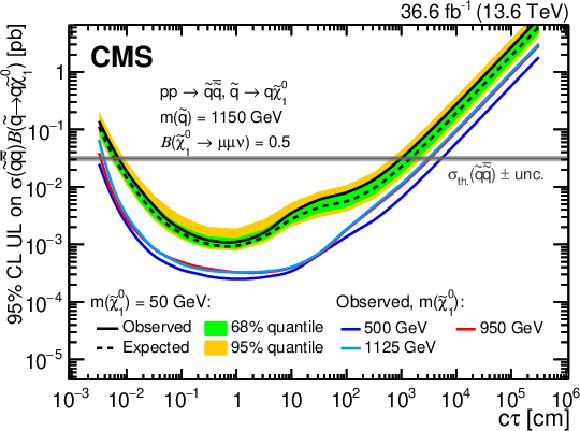
png pdf	Figure 16-e: The 95% CL upper limits on $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}})\mathcal{B}( \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} ) $ as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ in the RPV SUSY model, for $ \mathcal{B}(\tilde{\chi}_{1}^{0}\to\mu^{+}\mu^{-}\nu) = $ 0.5 and $ m({\tilde{\mathrm{q}}} ) $ ranging from (upper left) 125 GeV to (lower right) 1.6 TeV. The observed limits for various combinations of $ m({\tilde{\mathrm{q}}} ) $ and $ m(\tilde{\chi}_{1}^{0}) $ indicated in the legends are shown as solid curves. The median expected limits and their 68 and 95% quantiles are shown, respectively, as dashed black curves and green and yellow bands for the case of $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV and omitted for other neutralino masses for clarity. The gray horizontal lines indicate the theoretical values of the squark-antisquark production cross sections with the uncertainties shown as gray shaded bands. The predicted cross sections for $ m({\tilde{\mathrm{q}}} ) = $ 125, 200, and 350 GeV are, respectively, 7200, 840, and 50 pb, and fall outside the $ y $-axis range.
png pdf	Figure 16-f: The 95% CL upper limits on $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}})\mathcal{B}( \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} ) $ as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ in the RPV SUSY model, for $ \mathcal{B}(\tilde{\chi}_{1}^{0}\to\mu^{+}\mu^{-}\nu) = $ 0.5 and $ m({\tilde{\mathrm{q}}} ) $ ranging from (upper left) 125 GeV to (lower right) 1.6 TeV. The observed limits for various combinations of $ m({\tilde{\mathrm{q}}} ) $ and $ m(\tilde{\chi}_{1}^{0}) $ indicated in the legends are shown as solid curves. The median expected limits and their 68 and 95% quantiles are shown, respectively, as dashed black curves and green and yellow bands for the case of $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV and omitted for other neutralino masses for clarity. The gray horizontal lines indicate the theoretical values of the squark-antisquark production cross sections with the uncertainties shown as gray shaded bands. The predicted cross sections for $ m({\tilde{\mathrm{q}}} ) = $ 125, 200, and 350 GeV are, respectively, 7200, 840, and 50 pb, and fall outside the $ y $-axis range.

Summary

Data collected by the CMS experiment in proton-proton collisions at $ \sqrt{s} = $ 13.6 TeV in 2022 and corresponding to an integrated luminosity of 36.6 fb$ ^{-1} $ have been used to conduct an inclusive search for long-lived exotic neutral particles decaying to final states with a pair of oppositely charged muons. The search strategy is largely model independent and is sensitive to a broad range of lifetimes and masses. No significant excess of events above the standard model background is observed. The results are interpreted as limits on the parameters of the hidden Abelian Higgs model, in which the Higgs boson H decays to a pair of long-lived dark photons $ \mathrm{Z}_\text{D} $, and of an $ R $-parity violating supersymmetry model, in which long-lived neutralinos decay to a pair of muons and a neutrino. Even though the size of the data sample used by this analysis is about a factor of 2.5 smaller than that used in the previous search for displaced dimuons by the CMS experiment in pp collisions at $ \sqrt{s} = $ 13 TeV, the constraints on the parameters of the hidden Abelian Higgs model are comparable or tighter in a significant fraction of the parameter space, thanks mainly to improvements in the trigger algorithms. The combination of the results of this analysis with the results obtained at $ \sqrt{s} = $ 13 TeV improves the constraints on the branching fraction of the Higgs boson to dark photons, $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $, by approximately a factor of 2. In the range 10--60 GeV of the $ \mathrm{Z}_\text{D} $ mass $ m(\mathrm{Z}_\text{D}) $, $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) = $ 1% is excluded at 95% confidence level in the range of proper decay length $ c\tau(\mathrm{Z}_\text{D}) $ from a few tens of $ \mu $m to 30 m (700 m) for $ m(\mathrm{Z}_\text{D}) $ = 10 GeV (60 GeV). For $ m(\mathrm{Z}_\text{D}) $ greater than 20 GeV and less than $ m(\mathrm{H})/ $ 2, the combined limits provide the most stringent constraints to date on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D} \mathrm{Z}_\text{D}) $ for $ c\tau(\mathrm{Z}_\text{D}) $ between 30 $ \mu $m and $ {\approx}$ 0.1 cm, and above $ {\approx}$ 10 cm. When interpreted in the framework of the $ R $-parity violating supersymmetry model at a squark mass of 1.6 TeV, the results exclude mean proper neutralino decay lengths between 0.07 and 4 cm for a 50 GeV neutralino and between 70 $ \mu $m and 2 m for a 500 GeV neutralino.

Additional Figures
png pdf	Additional Figure 1: Illustration showing two long-lived particles with different lifetimes decaying into a pair of muons, depicting how the signals of the muons can be traced back to the long-lived particle decay point using data from the tracker and muon detectors.
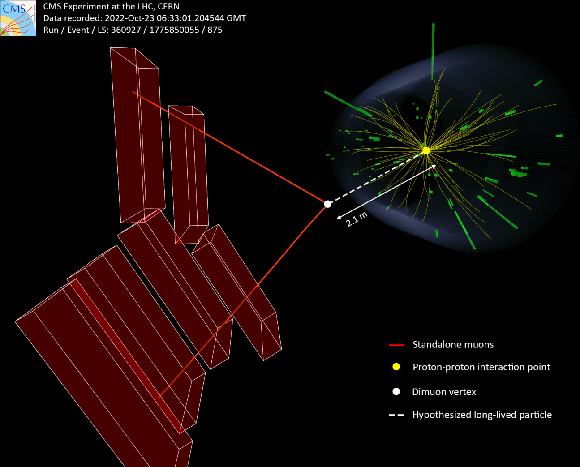
png pdf	Additional Figure 2: Run 3 event containing a candidate for a long-lived particle that decays into a pair of muons away from the interaction point, reconstructed in the CMS detector. The red lines correspond to the two standalone muons, which are detected only in the muon system. The muon tracks are used to calculate a dimuon vertex, indicated by the white circle, where the long-lived particle is hypothesized to have decayed.
png pdf root	Additional Figure 3: Fractions of signal events with zero (green), one (blue), and two (red) STA muons matched to TMS muons by the STA to TMS association procedure, as a function of generated $ L_{\text{xy}} $, in all HAHM signal samples combined.
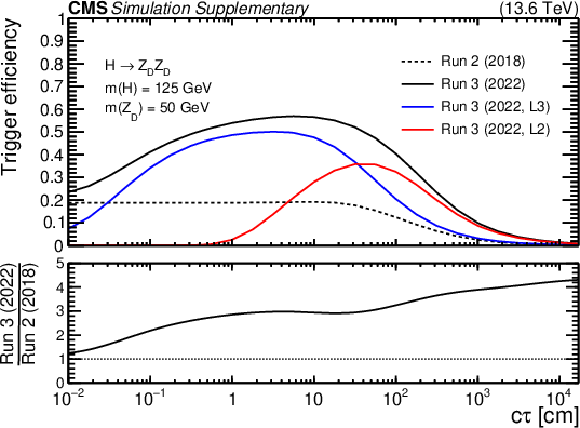
png pdf root	Additional Figure 4: Efficiencies of the Run 2 and Run 3 displaced dimuon triggers as a function of $ c\tau $ for the HAHM signal events with $ m(\mathrm{Z}_\text{D}) = $ 50 GeV. The efficiency is defined as the fraction of simulated events that satisfy the detector acceptance and the requirements of the following sets of trigger paths: the Run 2 (2018) triggers (dashed black); the Run 3 (2022, L3) triggers (blue); the Run 3 (2022, L2) triggers (red); and the OR of all these triggers (Run 3 (2022), black). The lower panel shows the ratio of the overall Run 3 (2022) efficiency to the Run 2 (2018) efficiency.
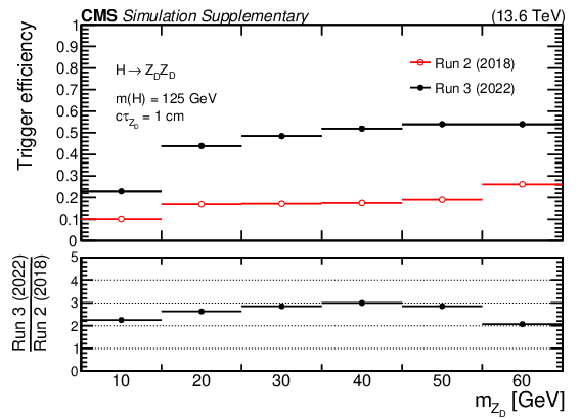
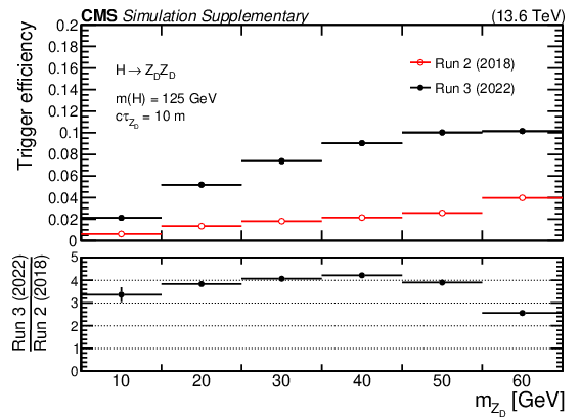
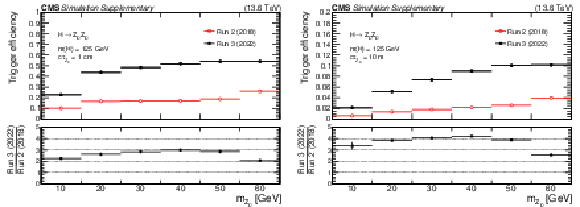
png pdf	Additional Figure 5: Efficiencies of the Run 2 (2018) (red) and Run 3 (2022) (black) sets of displaced dimuon triggers as a function of $ m(\mathrm{Z}_\text{D}) $ for the HAHM signal events with $ c\tau = $ 1 cm (left) and 10 m (right). The efficiency is defined as the fraction of simulated events that satisfy the detector acceptance and the requirements of the indicated set of trigger paths. The lower panel shows the ratio of the Run 3 (2022) efficiency to the Run 2 (2018) efficiency.
png pdf root	Additional Figure 5-a: Efficiencies of the Run 2 (2018) (red) and Run 3 (2022) (black) sets of displaced dimuon triggers as a function of $ m(\mathrm{Z}_\text{D}) $ for the HAHM signal events with $ c\tau = $ 1 cm (left) and 10 m (right). The efficiency is defined as the fraction of simulated events that satisfy the detector acceptance and the requirements of the indicated set of trigger paths. The lower panel shows the ratio of the Run 3 (2022) efficiency to the Run 2 (2018) efficiency.
png pdf root	Additional Figure 5-b: Efficiencies of the Run 2 (2018) (red) and Run 3 (2022) (black) sets of displaced dimuon triggers as a function of $ m(\mathrm{Z}_\text{D}) $ for the HAHM signal events with $ c\tau = $ 1 cm (left) and 10 m (right). The efficiency is defined as the fraction of simulated events that satisfy the detector acceptance and the requirements of the indicated set of trigger paths. The lower panel shows the ratio of the Run 3 (2022) efficiency to the Run 2 (2018) efficiency.
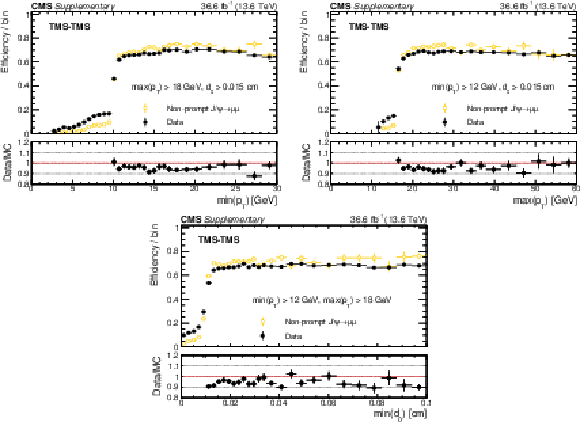
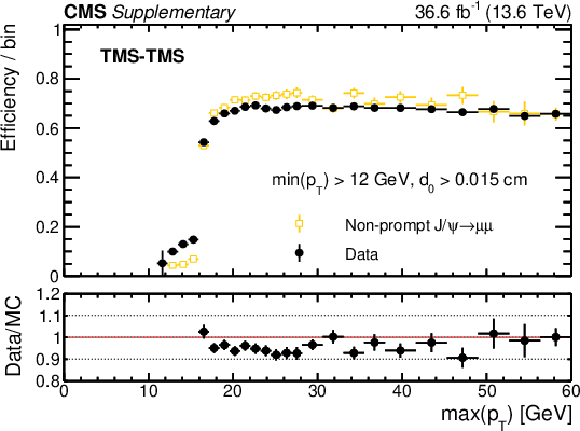
png pdf	Additional Figure 6: Efficiency of the Run 3 (2022, L3) triggers in data (black) and in simulation (yellow) as a function of min($ p_{\mathrm{T}} $) (upper left), max($ p_{\mathrm{T}} $) (upper right), and min($ d_{\text{0}} $) (lower) of the two muons forming a dimuon in events enriched in $ {\mathrm{J}/\psi} \to\mu\mu $. Efficiency in data is the fraction of $ {\mathrm{J}/\psi} \to\mu\mu $ events recorded by the triggers based on the information from jets and missing transverse energy that also satisfy the requirements of the Run 3 (2022, L3) triggers. It is compared to the efficiency of the Run 3 (2022, L3) triggers in a combination of simulated samples of $ {\mathrm{J}/\psi} \to\mu\mu $ events produced in various B hadron decays.
png pdf root	Additional Figure 6-a: Efficiency of the Run 3 (2022, L3) triggers in data (black) and in simulation (yellow) as a function of min($ p_{\mathrm{T}} $) (upper left), max($ p_{\mathrm{T}} $) (upper right), and min($ d_{\text{0}} $) (lower) of the two muons forming a dimuon in events enriched in $ {\mathrm{J}/\psi} \to\mu\mu $. Efficiency in data is the fraction of $ {\mathrm{J}/\psi} \to\mu\mu $ events recorded by the triggers based on the information from jets and missing transverse energy that also satisfy the requirements of the Run 3 (2022, L3) triggers. It is compared to the efficiency of the Run 3 (2022, L3) triggers in a combination of simulated samples of $ {\mathrm{J}/\psi} \to\mu\mu $ events produced in various B hadron decays.
png pdf root	Additional Figure 6-b: Efficiency of the Run 3 (2022, L3) triggers in data (black) and in simulation (yellow) as a function of min($ p_{\mathrm{T}} $) (upper left), max($ p_{\mathrm{T}} $) (upper right), and min($ d_{\text{0}} $) (lower) of the two muons forming a dimuon in events enriched in $ {\mathrm{J}/\psi} \to\mu\mu $. Efficiency in data is the fraction of $ {\mathrm{J}/\psi} \to\mu\mu $ events recorded by the triggers based on the information from jets and missing transverse energy that also satisfy the requirements of the Run 3 (2022, L3) triggers. It is compared to the efficiency of the Run 3 (2022, L3) triggers in a combination of simulated samples of $ {\mathrm{J}/\psi} \to\mu\mu $ events produced in various B hadron decays.
png pdf root	Additional Figure 6-c: Efficiency of the Run 3 (2022, L3) triggers in data (black) and in simulation (yellow) as a function of min($ p_{\mathrm{T}} $) (upper left), max($ p_{\mathrm{T}} $) (upper right), and min($ d_{\text{0}} $) (lower) of the two muons forming a dimuon in events enriched in $ {\mathrm{J}/\psi} \to\mu\mu $. Efficiency in data is the fraction of $ {\mathrm{J}/\psi} \to\mu\mu $ events recorded by the triggers based on the information from jets and missing transverse energy that also satisfy the requirements of the Run 3 (2022, L3) triggers. It is compared to the efficiency of the Run 3 (2022, L3) triggers in a combination of simulated samples of $ {\mathrm{J}/\psi} \to\mu\mu $ events produced in various B hadron decays.
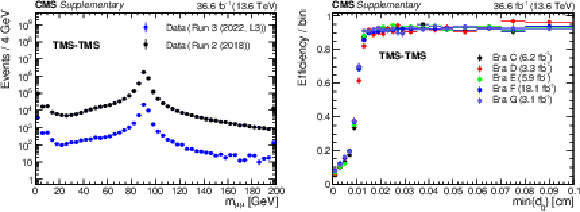
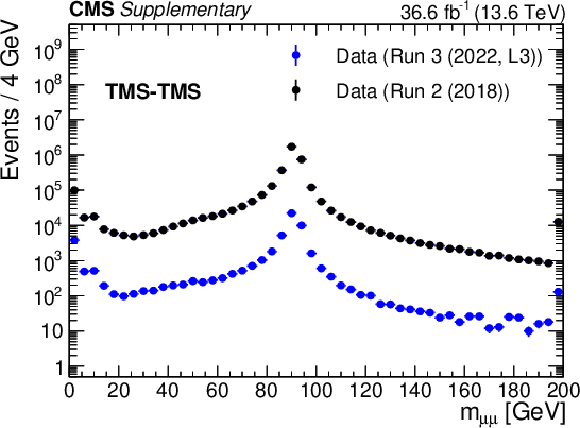
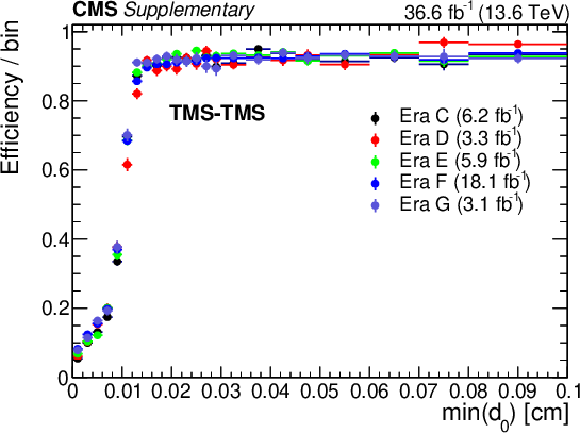
png pdf	Additional Figure 7: Left: Invariant mass distribution for TMS-TMS dimuons with $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} < $ 1 in events recorded by the Run 2 (2018) triggers in 2022 data (black), and in the subset of events also selected by the Run 3 (2022, L3) triggers (blue), illustrating the background rejection of the Run 3 (2022, L3) triggers. Right: Trigger efficiency, defined as the fraction of events recorded by the Run 2 (2018) triggers that also satisfied the requirements of the Run 3 (2022, L3) triggers, as a function of offline-reconstructed min($ d_{\text{0}} $) of the two muons forming TMS-TMS dimuons in events enriched in $ {\mathrm{J}/\psi} \to\mu\mu $. Different symbols show efficiencies in different periods of the 2022 data taking corresponding to the integrated luminosities indicated in the legend. For dimuons with offline $ \text{min}(d_{\text{0}}) > $ 0.012 cm, the combined efficiency of the L3 muon reconstruction and the online min($ d_{\text{0}} $) requirement is larger than 90% in all data taking periods.
png pdf root	Additional Figure 7-a: Left: Invariant mass distribution for TMS-TMS dimuons with $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} < $ 1 in events recorded by the Run 2 (2018) triggers in 2022 data (black), and in the subset of events also selected by the Run 3 (2022, L3) triggers (blue), illustrating the background rejection of the Run 3 (2022, L3) triggers. Right: Trigger efficiency, defined as the fraction of events recorded by the Run 2 (2018) triggers that also satisfied the requirements of the Run 3 (2022, L3) triggers, as a function of offline-reconstructed min($ d_{\text{0}} $) of the two muons forming TMS-TMS dimuons in events enriched in $ {\mathrm{J}/\psi} \to\mu\mu $. Different symbols show efficiencies in different periods of the 2022 data taking corresponding to the integrated luminosities indicated in the legend. For dimuons with offline $ \text{min}(d_{\text{0}}) > $ 0.012 cm, the combined efficiency of the L3 muon reconstruction and the online min($ d_{\text{0}} $) requirement is larger than 90% in all data taking periods.
png pdf root	Additional Figure 7-b: Left: Invariant mass distribution for TMS-TMS dimuons with $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} < $ 1 in events recorded by the Run 2 (2018) triggers in 2022 data (black), and in the subset of events also selected by the Run 3 (2022, L3) triggers (blue), illustrating the background rejection of the Run 3 (2022, L3) triggers. Right: Trigger efficiency, defined as the fraction of events recorded by the Run 2 (2018) triggers that also satisfied the requirements of the Run 3 (2022, L3) triggers, as a function of offline-reconstructed min($ d_{\text{0}} $) of the two muons forming TMS-TMS dimuons in events enriched in $ {\mathrm{J}/\psi} \to\mu\mu $. Different symbols show efficiencies in different periods of the 2022 data taking corresponding to the integrated luminosities indicated in the legend. For dimuons with offline $ \text{min}(d_{\text{0}}) > $ 0.012 cm, the combined efficiency of the L3 muon reconstruction and the online min($ d_{\text{0}} $) requirement is larger than 90% in all data taking periods.
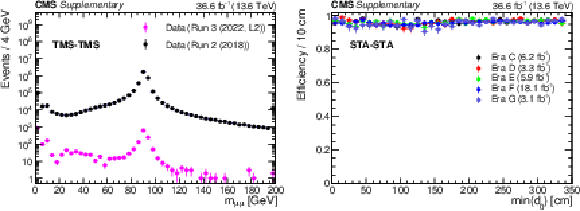
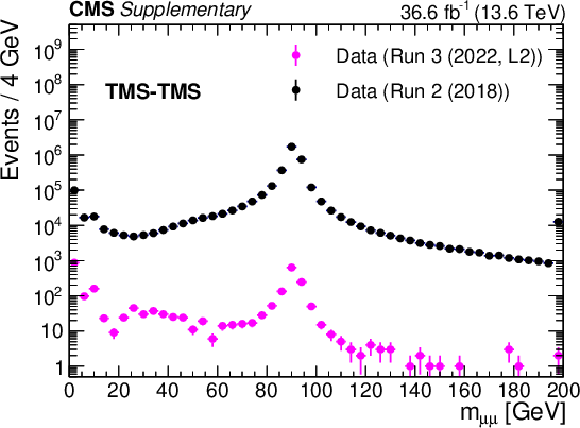
png pdf	Additional Figure 8: Left: Invariant mass distribution for TMS-TMS dimuons with $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} < $ 1 in events recorded by the Run 2 (2018) triggers in 2022 data (black), and in the subset of events also selected by the Run 3 (2022, L2) triggers (pink), illustrating the background rejection of the Run 3 (2022, L2) triggers. Right: Trigger efficiency, defined as the fraction of events recorded by the Run 2 (2018) triggers that also satisfied the requirements of the Run 3 (2022, L2) triggers, as a function of offline-reconstructed min($ d_{\text{0}} $) of the two muons forming STA-STA dimuons in events enriched in cosmic ray muons. Different symbols show efficiencies in different periods of the 2022 data taking corresponding to the integrated luminosities indicated in the legend. For displaced muons, the efficiency of the online min($ d_{\text{0}} $) requirement is larger than 95% in all data taking periods.
png pdf root	Additional Figure 8-a: Left: Invariant mass distribution for TMS-TMS dimuons with $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} < $ 1 in events recorded by the Run 2 (2018) triggers in 2022 data (black), and in the subset of events also selected by the Run 3 (2022, L2) triggers (pink), illustrating the background rejection of the Run 3 (2022, L2) triggers. Right: Trigger efficiency, defined as the fraction of events recorded by the Run 2 (2018) triggers that also satisfied the requirements of the Run 3 (2022, L2) triggers, as a function of offline-reconstructed min($ d_{\text{0}} $) of the two muons forming STA-STA dimuons in events enriched in cosmic ray muons. Different symbols show efficiencies in different periods of the 2022 data taking corresponding to the integrated luminosities indicated in the legend. For displaced muons, the efficiency of the online min($ d_{\text{0}} $) requirement is larger than 95% in all data taking periods.
png pdf root	Additional Figure 8-b: Left: Invariant mass distribution for TMS-TMS dimuons with $ L_{\text{xy}}/\sigma_{L_{\text{xy}}} < $ 1 in events recorded by the Run 2 (2018) triggers in 2022 data (black), and in the subset of events also selected by the Run 3 (2022, L2) triggers (pink), illustrating the background rejection of the Run 3 (2022, L2) triggers. Right: Trigger efficiency, defined as the fraction of events recorded by the Run 2 (2018) triggers that also satisfied the requirements of the Run 3 (2022, L2) triggers, as a function of offline-reconstructed min($ d_{\text{0}} $) of the two muons forming STA-STA dimuons in events enriched in cosmic ray muons. Different symbols show efficiencies in different periods of the 2022 data taking corresponding to the integrated luminosities indicated in the legend. For displaced muons, the efficiency of the online min($ d_{\text{0}} $) requirement is larger than 95% in all data taking periods.
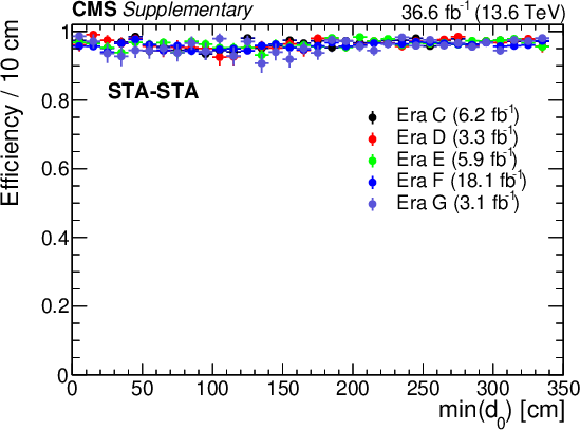
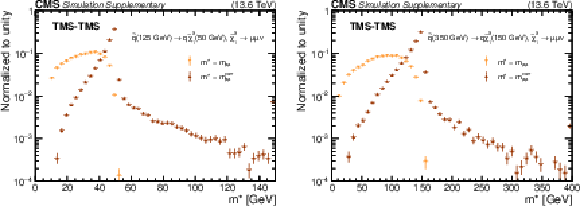
png pdf	Additional Figure 9: Distributions of $ m_{\mu\mu} $ (red) and $ m^\text{corr}_{\mu\mu} $ (orange) in the TMS-TMS dimuon category, for simulated signal events with $ m({\tilde{\mathrm{q}}} ) = $ 125 GeV and $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV (left), and $ m({\tilde{\mathrm{q}}} ) = $ 350 GeV and $ m(\tilde{\chi}_{1}^{0}) = $ 150 GeV (right) in the RPV SUSY model. The last bin includes events in the histogram overflow.
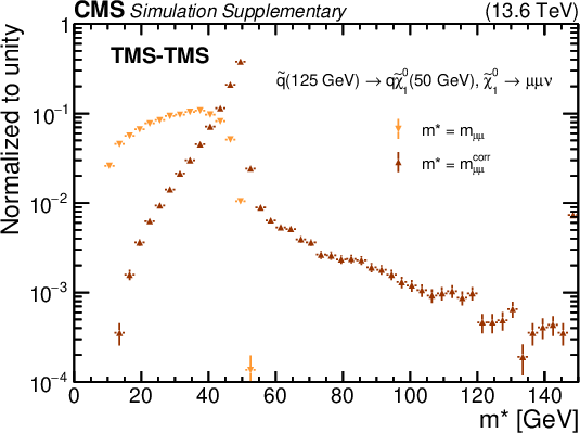
png pdf root	Additional Figure 9-a: Distributions of $ m_{\mu\mu} $ (red) and $ m^\text{corr}_{\mu\mu} $ (orange) in the TMS-TMS dimuon category, for simulated signal events with $ m({\tilde{\mathrm{q}}} ) = $ 125 GeV and $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV (left), and $ m({\tilde{\mathrm{q}}} ) = $ 350 GeV and $ m(\tilde{\chi}_{1}^{0}) = $ 150 GeV (right) in the RPV SUSY model. The last bin includes events in the histogram overflow.
png pdf root	Additional Figure 9-b: Distributions of $ m_{\mu\mu} $ (red) and $ m^\text{corr}_{\mu\mu} $ (orange) in the TMS-TMS dimuon category, for simulated signal events with $ m({\tilde{\mathrm{q}}} ) = $ 125 GeV and $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV (left), and $ m({\tilde{\mathrm{q}}} ) = $ 350 GeV and $ m(\tilde{\chi}_{1}^{0}) = $ 150 GeV (right) in the RPV SUSY model. The last bin includes events in the histogram overflow.
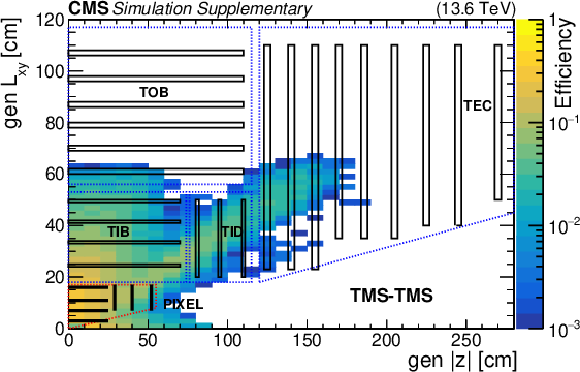
png pdf root	Additional Figure 10: Offline selection efficiency of TMS-TMS dimuons as a function of simulated $ L_{\text{xy}} $ and $ \|z\| $ decay positions of $ \mathrm{Z}_\text{D} $, in all generated HAHM signal samples combined. Approximate locations of silicon pixel and strip tracker modules -- tracker inner barrel (TIB) and disks (TID), tracker outer barrel (TOB), and tracker endcap (TEC) -- are drawn superimposed as black boxes.
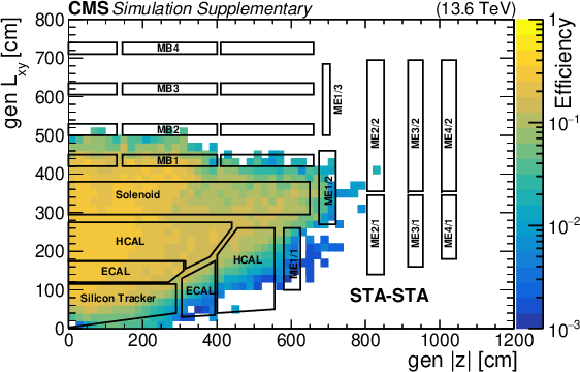
png pdf root	Additional Figure 11: Offline selection efficiency of STA-STA dimuons as a function of simulated $ L_{\text{xy}} $ and $ \|z\| $ decay positions of $ \mathrm{Z}_\text{D} $, in all generated HAHM signal samples combined. Approximate locations of various CMS subdetectors are drawn superimposed as black boxes. MB1-MB4 denote four DT stations in the barrel, while ME followed by two indices denote CSC stations (the first index) and rings (the second index) in the endcap.
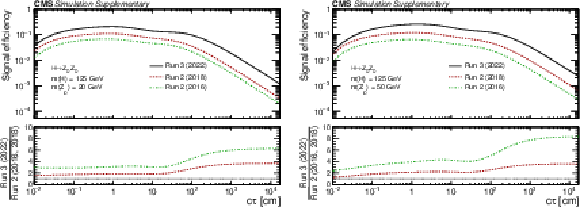
png pdf	Additional Figure 12: Overall selection efficiencies as a function of $ c\tau(\mathrm{Z}_\text{D}) $ for the HAHM signal with $ m(\mathrm{Z}_\text{D}) = $ 20 GeV (left) and 50 GeV (right) in different years of data taking. Efficiencies are computed as the ratios of the number of simulated signal events in which at least one dimuon candidate passes all 2016 (dashed green), 2018 (dashed red), and 2022 (solid black) trigger and offline selection criteria to the total number of simulated signal events. The lower panel shows the ratio of the 2022 efficiency to the 2018 efficiency (dashed red) and to the 2016 efficiency (dashed green).
png pdf root	Additional Figure 12-a: Overall selection efficiencies as a function of $ c\tau(\mathrm{Z}_\text{D}) $ for the HAHM signal with $ m(\mathrm{Z}_\text{D}) = $ 20 GeV (left) and 50 GeV (right) in different years of data taking. Efficiencies are computed as the ratios of the number of simulated signal events in which at least one dimuon candidate passes all 2016 (dashed green), 2018 (dashed red), and 2022 (solid black) trigger and offline selection criteria to the total number of simulated signal events. The lower panel shows the ratio of the 2022 efficiency to the 2018 efficiency (dashed red) and to the 2016 efficiency (dashed green).
png pdf root	Additional Figure 12-b: Overall selection efficiencies as a function of $ c\tau(\mathrm{Z}_\text{D}) $ for the HAHM signal with $ m(\mathrm{Z}_\text{D}) = $ 20 GeV (left) and 50 GeV (right) in different years of data taking. Efficiencies are computed as the ratios of the number of simulated signal events in which at least one dimuon candidate passes all 2016 (dashed green), 2018 (dashed red), and 2022 (solid black) trigger and offline selection criteria to the total number of simulated signal events. The lower panel shows the ratio of the 2022 efficiency to the 2018 efficiency (dashed red) and to the 2016 efficiency (dashed green).
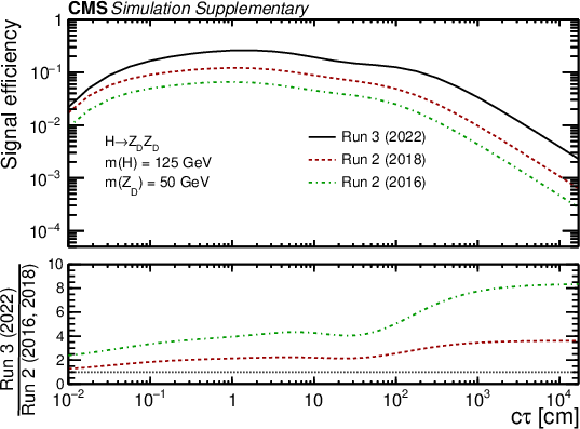
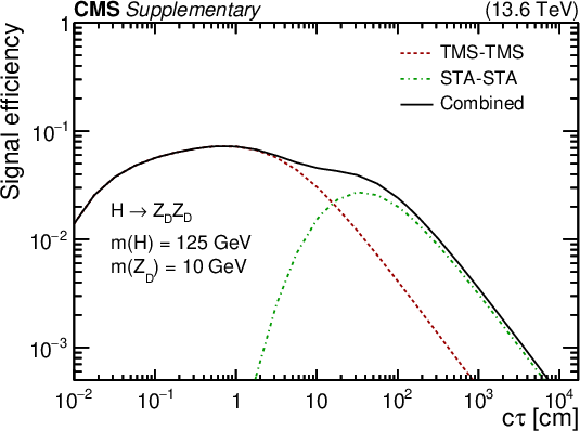
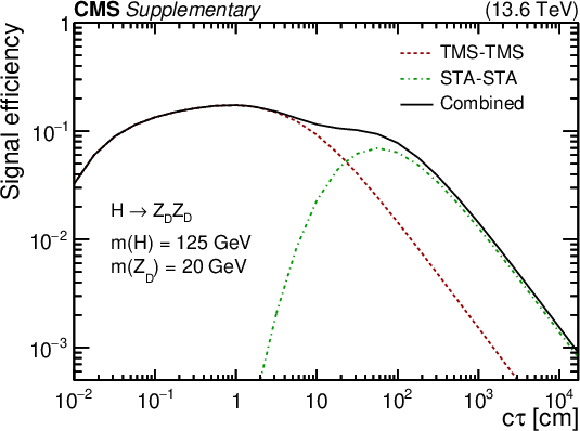
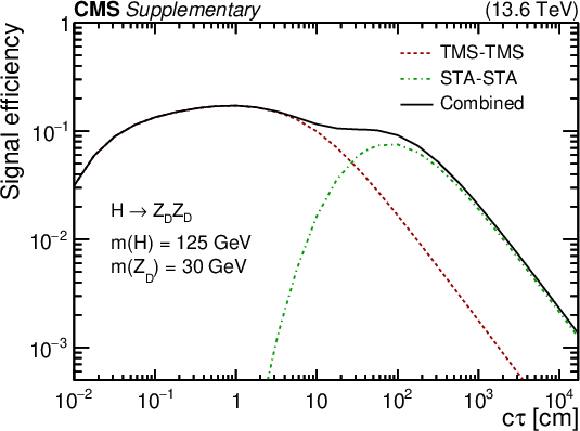
png pdf	Additional Figure 13: Overall selection efficiencies as a function of $ c\tau(\mathrm{Z}_\text{D}) $ for the HAHM model with $ m(\mathrm{Z}_\text{D}) $ ranging from 10 GeV (upper left) to 60 GeV (lower right). Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
png pdf root	Additional Figure 13-a: Overall selection efficiencies as a function of $ c\tau(\mathrm{Z}_\text{D}) $ for the HAHM model with $ m(\mathrm{Z}_\text{D}) $ ranging from 10 GeV (upper left) to 60 GeV (lower right). Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
png pdf root	Additional Figure 13-b: Overall selection efficiencies as a function of $ c\tau(\mathrm{Z}_\text{D}) $ for the HAHM model with $ m(\mathrm{Z}_\text{D}) $ ranging from 10 GeV (upper left) to 60 GeV (lower right). Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
png pdf root	Additional Figure 13-c: Overall selection efficiencies as a function of $ c\tau(\mathrm{Z}_\text{D}) $ for the HAHM model with $ m(\mathrm{Z}_\text{D}) $ ranging from 10 GeV (upper left) to 60 GeV (lower right). Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
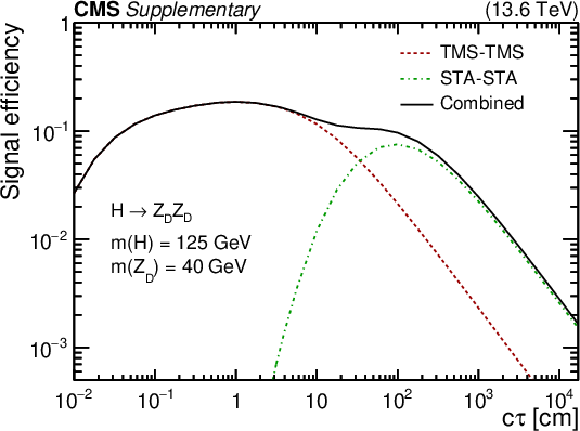
png pdf root	Additional Figure 13-d: Overall selection efficiencies as a function of $ c\tau(\mathrm{Z}_\text{D}) $ for the HAHM model with $ m(\mathrm{Z}_\text{D}) $ ranging from 10 GeV (upper left) to 60 GeV (lower right). Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
png pdf root	Additional Figure 13-e: Overall selection efficiencies as a function of $ c\tau(\mathrm{Z}_\text{D}) $ for the HAHM model with $ m(\mathrm{Z}_\text{D}) $ ranging from 10 GeV (upper left) to 60 GeV (lower right). Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
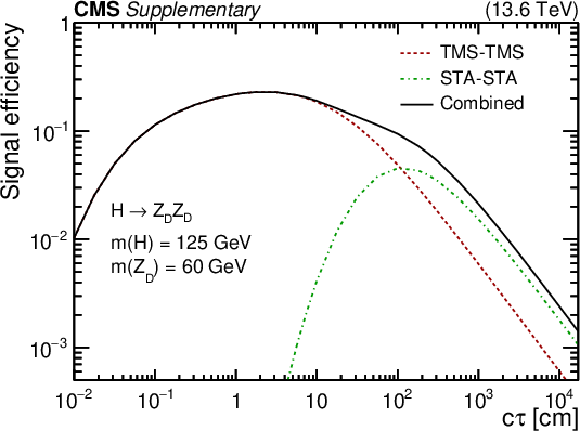
png pdf root	Additional Figure 13-f: Overall selection efficiencies as a function of $ c\tau(\mathrm{Z}_\text{D}) $ for the HAHM model with $ m(\mathrm{Z}_\text{D}) $ ranging from 10 GeV (upper left) to 60 GeV (lower right). Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
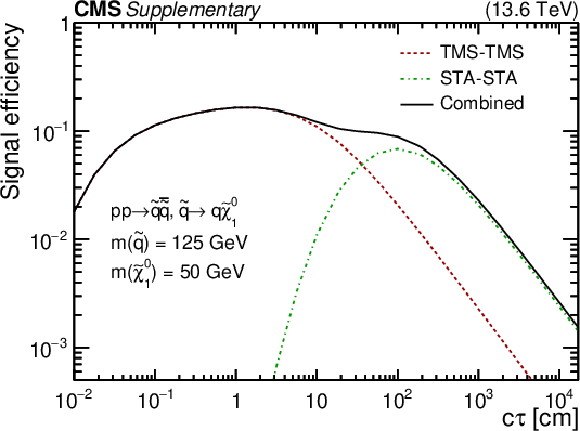
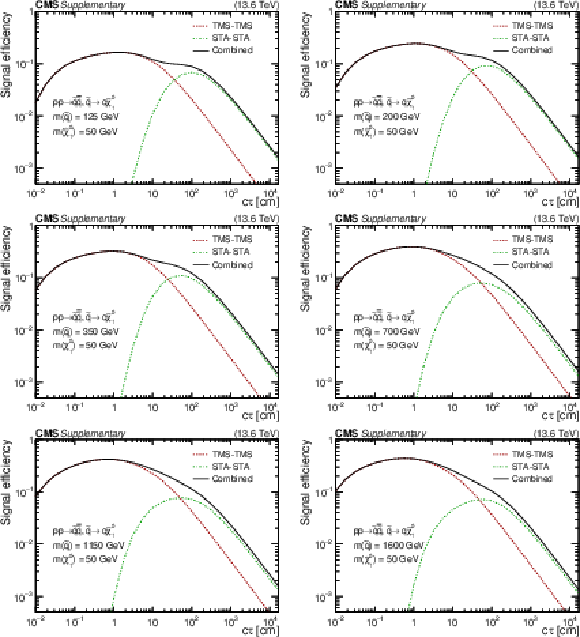
png pdf	Additional Figure 14: Overall selection efficiencies as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ for the RPV SUSY model, for events with $ m({\tilde{\mathrm{q}}} ) $ ranging from 125 GeV (upper left) to 1600 GeV (lower right) and $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV. Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
png pdf root	Additional Figure 14-a: Overall selection efficiencies as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ for the RPV SUSY model, for events with $ m({\tilde{\mathrm{q}}} ) $ ranging from 125 GeV (upper left) to 1600 GeV (lower right) and $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV. Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
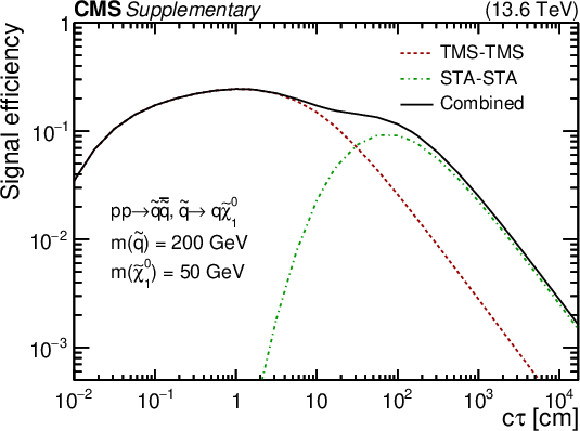
png pdf root	Additional Figure 14-b: Overall selection efficiencies as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ for the RPV SUSY model, for events with $ m({\tilde{\mathrm{q}}} ) $ ranging from 125 GeV (upper left) to 1600 GeV (lower right) and $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV. Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
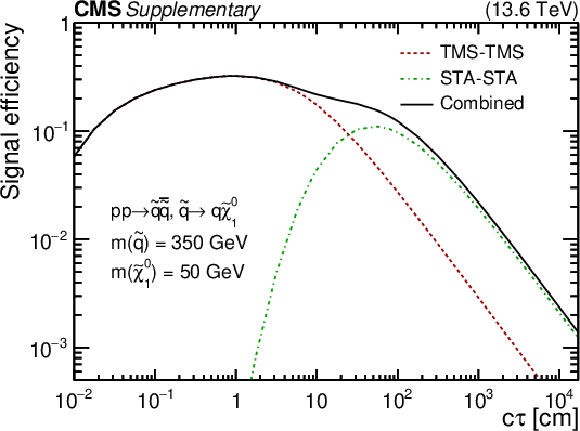
png pdf root	Additional Figure 14-c: Overall selection efficiencies as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ for the RPV SUSY model, for events with $ m({\tilde{\mathrm{q}}} ) $ ranging from 125 GeV (upper left) to 1600 GeV (lower right) and $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV. Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
png pdf root	Additional Figure 14-d: Overall selection efficiencies as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ for the RPV SUSY model, for events with $ m({\tilde{\mathrm{q}}} ) $ ranging from 125 GeV (upper left) to 1600 GeV (lower right) and $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV. Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
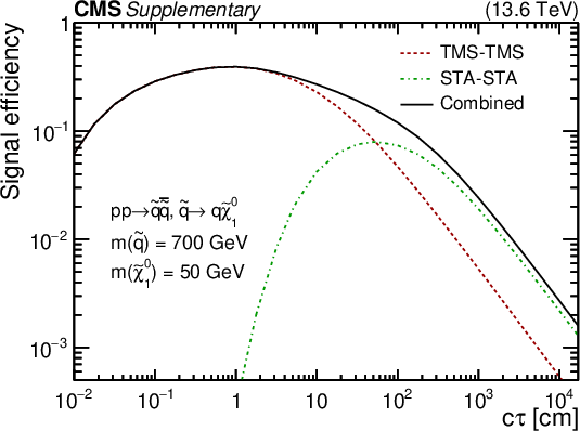
png pdf root	Additional Figure 14-e: Overall selection efficiencies as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ for the RPV SUSY model, for events with $ m({\tilde{\mathrm{q}}} ) $ ranging from 125 GeV (upper left) to 1600 GeV (lower right) and $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV. Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
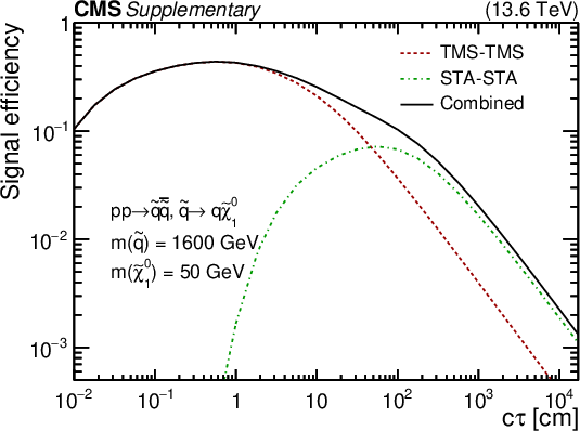
png pdf root	Additional Figure 14-f: Overall selection efficiencies as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ for the RPV SUSY model, for events with $ m({\tilde{\mathrm{q}}} ) $ ranging from 125 GeV (upper left) to 1600 GeV (lower right) and $ m(\tilde{\chi}_{1}^{0}) = $ 50 GeV. Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
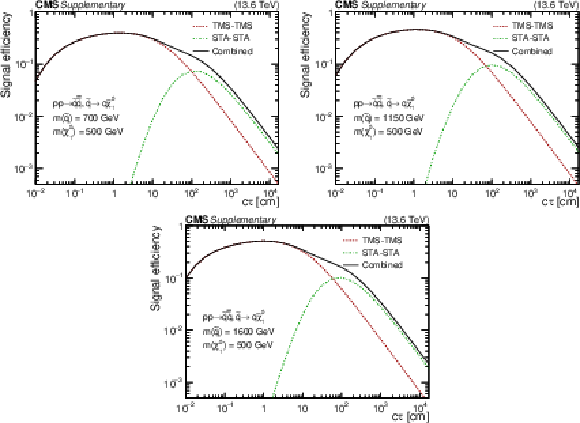
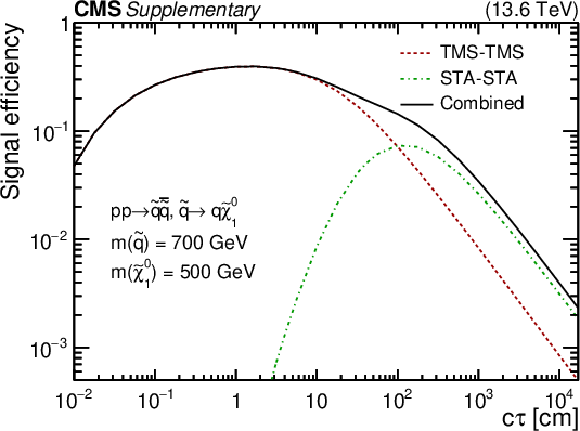
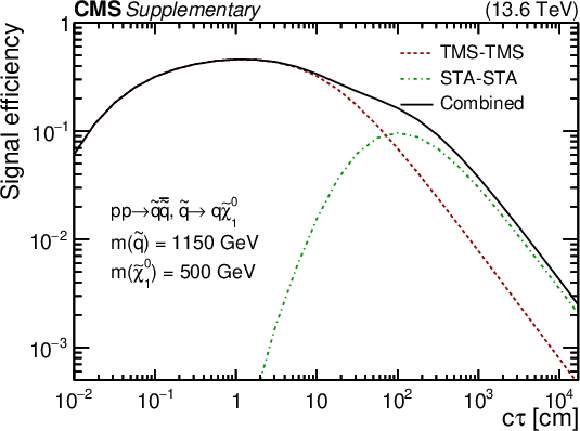
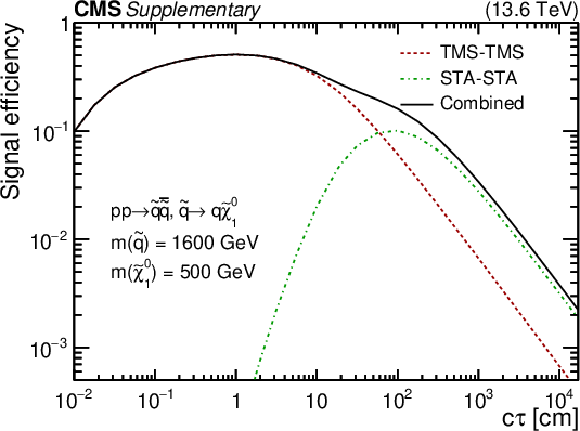
png pdf	Additional Figure 15: Overall selection efficiencies as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ for the RPV SUSY model, for events with $ m({\tilde{\mathrm{q}}} ) $ ranging from 700 GeV (upper left) to 1600 GeV (lower) and $ m(\tilde{\chi}_{1}^{0}) = $ 500 GeV. Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
png pdf root	Additional Figure 15-a: Overall selection efficiencies as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ for the RPV SUSY model, for events with $ m({\tilde{\mathrm{q}}} ) $ ranging from 700 GeV (upper left) to 1600 GeV (lower) and $ m(\tilde{\chi}_{1}^{0}) = $ 500 GeV. Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
png pdf root	Additional Figure 15-b: Overall selection efficiencies as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ for the RPV SUSY model, for events with $ m({\tilde{\mathrm{q}}} ) $ ranging from 700 GeV (upper left) to 1600 GeV (lower) and $ m(\tilde{\chi}_{1}^{0}) = $ 500 GeV. Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
png pdf root	Additional Figure 15-c: Overall selection efficiencies as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ for the RPV SUSY model, for events with $ m({\tilde{\mathrm{q}}} ) $ ranging from 700 GeV (upper left) to 1600 GeV (lower) and $ m(\tilde{\chi}_{1}^{0}) = $ 500 GeV. Each plot shows efficiencies of the two dimuon categories, TMS-TMS (dashed red) and STA-STA (dashed green), as well as their combination (solid black). Each efficiency is computed as the ratio of the number of simulated signal events in which at least one dimuon candidate of a given type (or any type for the combined efficiency) passes all selection criteria (including the trigger) to the total number of simulated signal events. All efficiencies are corrected by the data-to-simulation scale factors described in the paper.
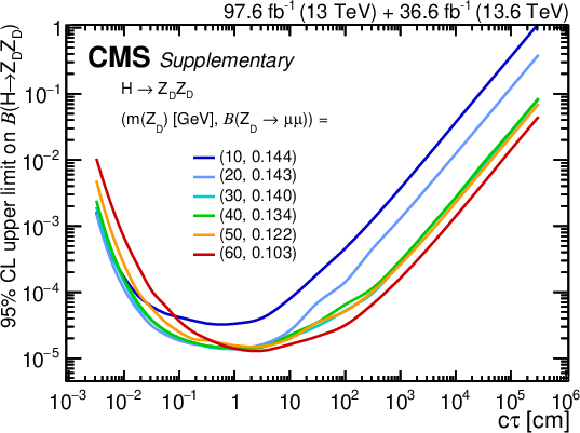
png pdf root	Additional Figure 16: The 95% CL observed upper limits on $ \mathcal{B}(\mathrm{H} \to \mathrm{Z}_\text{D}\mathrm{Z}_\text{D}) $ as a function of $ c\tau(\mathrm{Z}_\text{D}) $ for the HAHM model with $ m(\mathrm{Z}_\text{D}) $ ranging from 10 to 60 GeV, obtained by combining the results of this analysis with those of the Run 2 analysis (JHEP 05 (2023) 228).
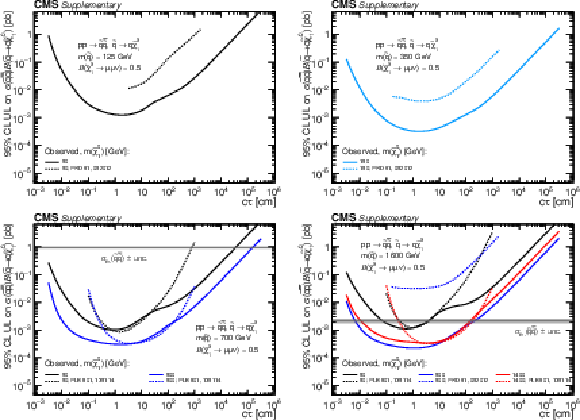
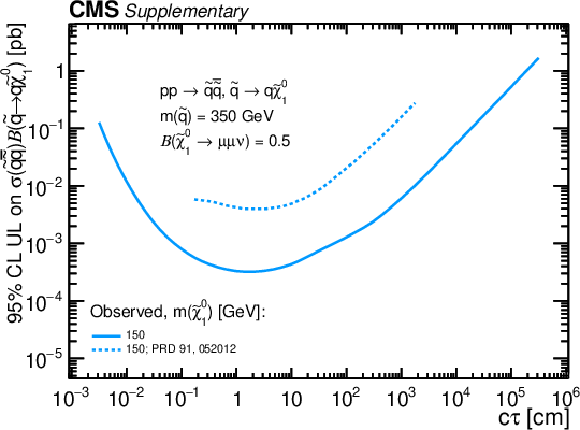
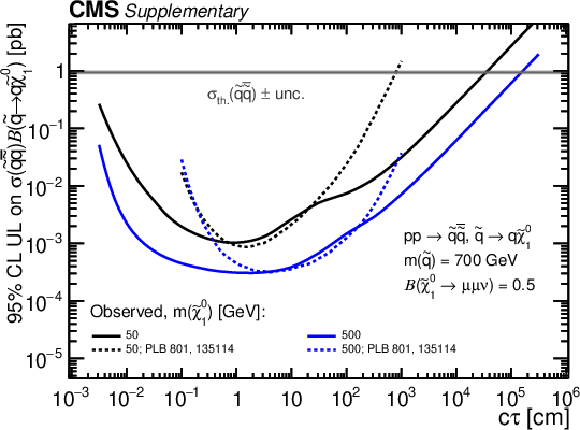
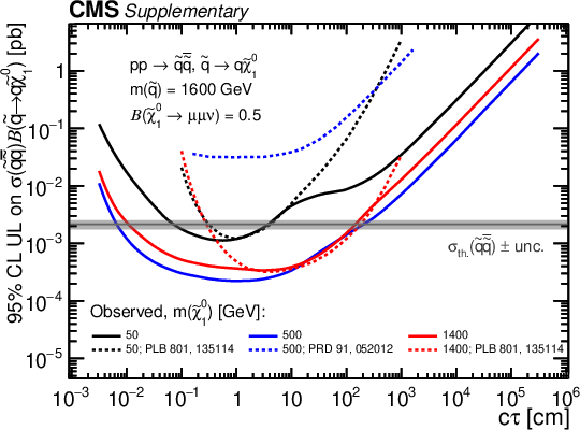
png pdf	Additional Figure 17: Observed 95% CL upper limits on $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}})\mathcal{B}( \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} ) $ as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ in the RPV SUSY model, for $ \mathcal{B}(\tilde{\chi}_{1}^{0}\to\mu^{+}\mu^{-}\nu) = $ 0.5 and various combinations of $ m({\tilde{\mathrm{q}}} ) $ and $ m(\tilde{\chi}_{1}^{0}) $ indicated in the legends. The limits obtained in this analysis (solid) are compared with the corresponding limits (dashed) from the analysis of CMS Run 1 data (PRD 91, 052012) rescaled by the ratio of $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}}) $ at 13.6 TeV and 8 TeV, and from the ATLAS search for displaced vertices of oppositely charged leptons in the 2016 data set (PLB 801, 135114) rescaled by the ratio of $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}}) $ at 13.6 TeV and 13 TeV. The gray horizontal lines indicate the theoretical values of the squark-antisquark production cross sections with the uncertainties shown as gray shaded bands. The predicted cross sections for $ m({\tilde{\mathrm{q}}} ) $ = 125 and 350 GeV are, respectively, 7200 and 50 pb, and fall outside the $ y $-axis range.
png pdf root	Additional Figure 17-a: Observed 95% CL upper limits on $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}})\mathcal{B}( \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} ) $ as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ in the RPV SUSY model, for $ \mathcal{B}(\tilde{\chi}_{1}^{0}\to\mu^{+}\mu^{-}\nu) = $ 0.5 and various combinations of $ m({\tilde{\mathrm{q}}} ) $ and $ m(\tilde{\chi}_{1}^{0}) $ indicated in the legends. The limits obtained in this analysis (solid) are compared with the corresponding limits (dashed) from the analysis of CMS Run 1 data (PRD 91, 052012) rescaled by the ratio of $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}}) $ at 13.6 TeV and 8 TeV, and from the ATLAS search for displaced vertices of oppositely charged leptons in the 2016 data set (PLB 801, 135114) rescaled by the ratio of $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}}) $ at 13.6 TeV and 13 TeV. The gray horizontal lines indicate the theoretical values of the squark-antisquark production cross sections with the uncertainties shown as gray shaded bands. The predicted cross sections for $ m({\tilde{\mathrm{q}}} ) $ = 125 and 350 GeV are, respectively, 7200 and 50 pb, and fall outside the $ y $-axis range.
png pdf root	Additional Figure 17-b: Observed 95% CL upper limits on $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}})\mathcal{B}( \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} ) $ as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ in the RPV SUSY model, for $ \mathcal{B}(\tilde{\chi}_{1}^{0}\to\mu^{+}\mu^{-}\nu) = $ 0.5 and various combinations of $ m({\tilde{\mathrm{q}}} ) $ and $ m(\tilde{\chi}_{1}^{0}) $ indicated in the legends. The limits obtained in this analysis (solid) are compared with the corresponding limits (dashed) from the analysis of CMS Run 1 data (PRD 91, 052012) rescaled by the ratio of $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}}) $ at 13.6 TeV and 8 TeV, and from the ATLAS search for displaced vertices of oppositely charged leptons in the 2016 data set (PLB 801, 135114) rescaled by the ratio of $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}}) $ at 13.6 TeV and 13 TeV. The gray horizontal lines indicate the theoretical values of the squark-antisquark production cross sections with the uncertainties shown as gray shaded bands. The predicted cross sections for $ m({\tilde{\mathrm{q}}} ) $ = 125 and 350 GeV are, respectively, 7200 and 50 pb, and fall outside the $ y $-axis range.
png pdf root	Additional Figure 17-c: Observed 95% CL upper limits on $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}})\mathcal{B}( \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} ) $ as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ in the RPV SUSY model, for $ \mathcal{B}(\tilde{\chi}_{1}^{0}\to\mu^{+}\mu^{-}\nu) = $ 0.5 and various combinations of $ m({\tilde{\mathrm{q}}} ) $ and $ m(\tilde{\chi}_{1}^{0}) $ indicated in the legends. The limits obtained in this analysis (solid) are compared with the corresponding limits (dashed) from the analysis of CMS Run 1 data (PRD 91, 052012) rescaled by the ratio of $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}}) $ at 13.6 TeV and 8 TeV, and from the ATLAS search for displaced vertices of oppositely charged leptons in the 2016 data set (PLB 801, 135114) rescaled by the ratio of $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}}) $ at 13.6 TeV and 13 TeV. The gray horizontal lines indicate the theoretical values of the squark-antisquark production cross sections with the uncertainties shown as gray shaded bands. The predicted cross sections for $ m({\tilde{\mathrm{q}}} ) $ = 125 and 350 GeV are, respectively, 7200 and 50 pb, and fall outside the $ y $-axis range.
png pdf root	Additional Figure 17-d: Observed 95% CL upper limits on $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}})\mathcal{B}( \tilde{\mathrm{q}} \to \mathrm{q} \tilde{\chi}_{1}^{0} ) $ as a function of $ c\tau(\tilde{\chi}_{1}^{0}) $ in the RPV SUSY model, for $ \mathcal{B}(\tilde{\chi}_{1}^{0}\to\mu^{+}\mu^{-}\nu) = $ 0.5 and various combinations of $ m({\tilde{\mathrm{q}}} ) $ and $ m(\tilde{\chi}_{1}^{0}) $ indicated in the legends. The limits obtained in this analysis (solid) are compared with the corresponding limits (dashed) from the analysis of CMS Run 1 data (PRD 91, 052012) rescaled by the ratio of $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}}) $ at 13.6 TeV and 8 TeV, and from the ATLAS search for displaced vertices of oppositely charged leptons in the 2016 data set (PLB 801, 135114) rescaled by the ratio of $ \sigma(\mathrm{p}\mathrm{p}\to{\tilde{\mathrm{q}}} \overline{\tilde{\mathrm{q}}}) $ at 13.6 TeV and 13 TeV. The gray horizontal lines indicate the theoretical values of the squark-antisquark production cross sections with the uncertainties shown as gray shaded bands. The predicted cross sections for $ m({\tilde{\mathrm{q}}} ) $ = 125 and 350 GeV are, respectively, 7200 and 50 pb, and fall outside the $ y $-axis range.
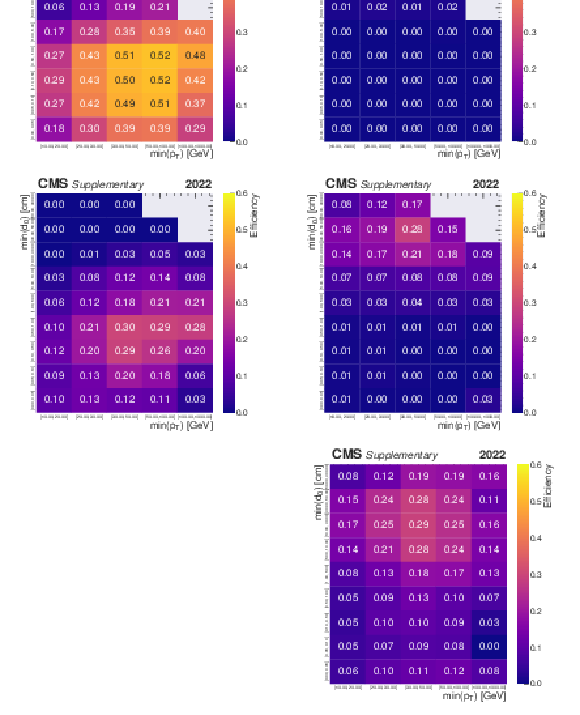
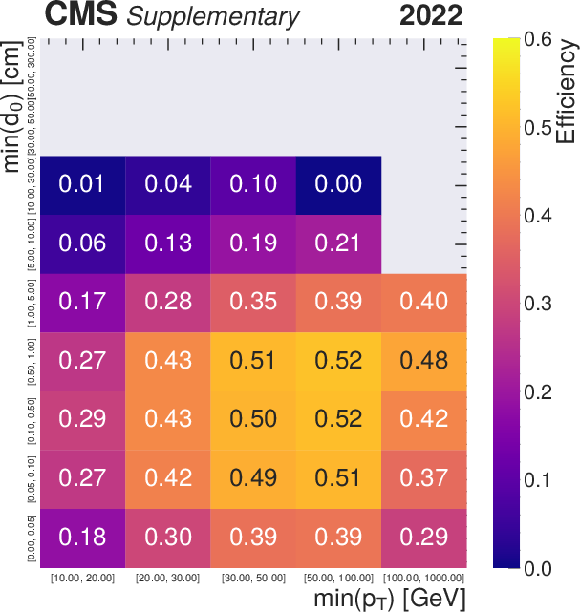
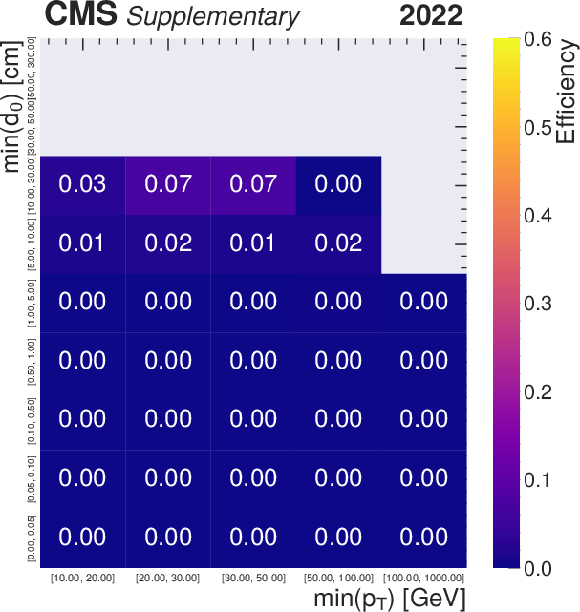
png pdf	Additional Figure 18: Signal efficiencies in the TMS-TMS (left) and STA-STA (right) dimuon category as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ in dimuons with $ L_{\text{xy}}^\mathrm{true} $ smaller than 20 cm (upper), 20--70 cm (middle), and 70-500 cm (lower) in the HAHM signal model. The efficiency in each bin is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The geometric acceptance is defined as the generated longitudinal decay length $ L_{\text{z}}^\mathrm{true} $ smaller than 8 m and $ \|\eta\| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are corrected by the data-to-simulation scale factors described in the paper. Efficiency in the TMS-TMS dimuon category for dimuons with $ L_{\text{xy}}^\mathrm{true} > $ 70 cm is zero and is not shown.
png pdf	Additional Figure 18-a: Signal efficiencies in the TMS-TMS (left) and STA-STA (right) dimuon category as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ in dimuons with $ L_{\text{xy}}^\mathrm{true} $ smaller than 20 cm (upper), 20--70 cm (middle), and 70-500 cm (lower) in the HAHM signal model. The efficiency in each bin is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The geometric acceptance is defined as the generated longitudinal decay length $ L_{\text{z}}^\mathrm{true} $ smaller than 8 m and $ \|\eta\| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are corrected by the data-to-simulation scale factors described in the paper. Efficiency in the TMS-TMS dimuon category for dimuons with $ L_{\text{xy}}^\mathrm{true} > $ 70 cm is zero and is not shown.
png pdf	Additional Figure 18-b: Signal efficiencies in the TMS-TMS (left) and STA-STA (right) dimuon category as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ in dimuons with $ L_{\text{xy}}^\mathrm{true} $ smaller than 20 cm (upper), 20--70 cm (middle), and 70-500 cm (lower) in the HAHM signal model. The efficiency in each bin is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The geometric acceptance is defined as the generated longitudinal decay length $ L_{\text{z}}^\mathrm{true} $ smaller than 8 m and $ \|\eta\| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are corrected by the data-to-simulation scale factors described in the paper. Efficiency in the TMS-TMS dimuon category for dimuons with $ L_{\text{xy}}^\mathrm{true} > $ 70 cm is zero and is not shown.
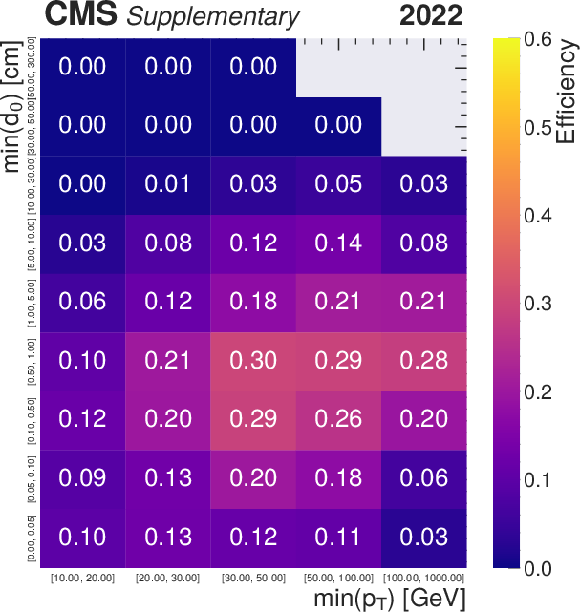
png pdf	Additional Figure 18-c: Signal efficiencies in the TMS-TMS (left) and STA-STA (right) dimuon category as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ in dimuons with $ L_{\text{xy}}^\mathrm{true} $ smaller than 20 cm (upper), 20--70 cm (middle), and 70-500 cm (lower) in the HAHM signal model. The efficiency in each bin is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The geometric acceptance is defined as the generated longitudinal decay length $ L_{\text{z}}^\mathrm{true} $ smaller than 8 m and $ \|\eta\| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are corrected by the data-to-simulation scale factors described in the paper. Efficiency in the TMS-TMS dimuon category for dimuons with $ L_{\text{xy}}^\mathrm{true} > $ 70 cm is zero and is not shown.
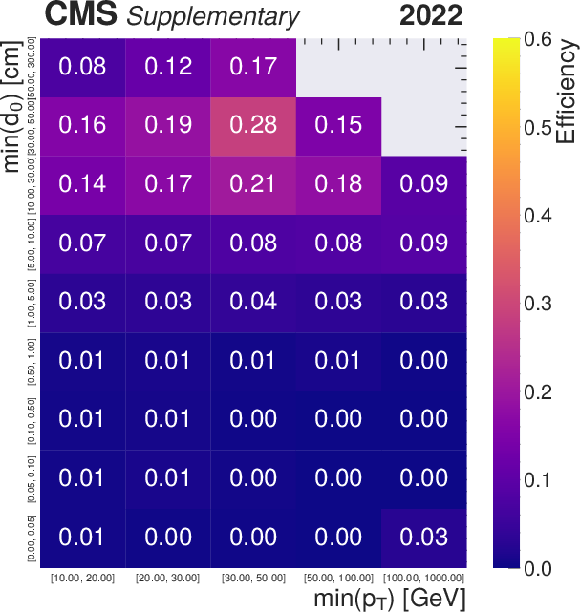
png pdf	Additional Figure 18-d: Signal efficiencies in the TMS-TMS (left) and STA-STA (right) dimuon category as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ in dimuons with $ L_{\text{xy}}^\mathrm{true} $ smaller than 20 cm (upper), 20--70 cm (middle), and 70-500 cm (lower) in the HAHM signal model. The efficiency in each bin is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The geometric acceptance is defined as the generated longitudinal decay length $ L_{\text{z}}^\mathrm{true} $ smaller than 8 m and $ \|\eta\| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are corrected by the data-to-simulation scale factors described in the paper. Efficiency in the TMS-TMS dimuon category for dimuons with $ L_{\text{xy}}^\mathrm{true} > $ 70 cm is zero and is not shown.
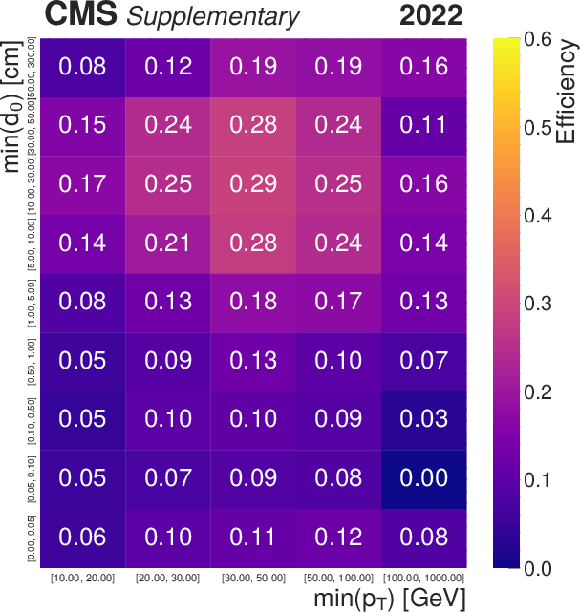
png pdf	Additional Figure 18-e: Signal efficiencies in the TMS-TMS (left) and STA-STA (right) dimuon category as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ in dimuons with $ L_{\text{xy}}^\mathrm{true} $ smaller than 20 cm (upper), 20--70 cm (middle), and 70-500 cm (lower) in the HAHM signal model. The efficiency in each bin is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The geometric acceptance is defined as the generated longitudinal decay length $ L_{\text{z}}^\mathrm{true} $ smaller than 8 m and $ \|\eta\| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are corrected by the data-to-simulation scale factors described in the paper. Efficiency in the TMS-TMS dimuon category for dimuons with $ L_{\text{xy}}^\mathrm{true} > $ 70 cm is zero and is not shown.
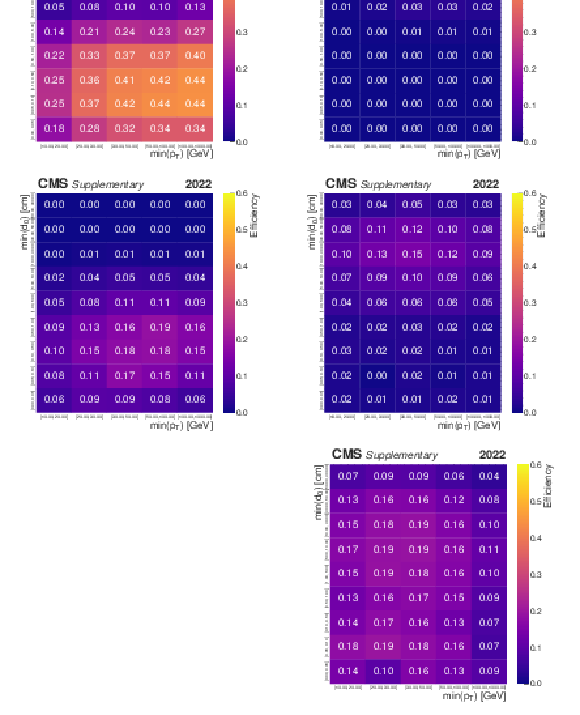
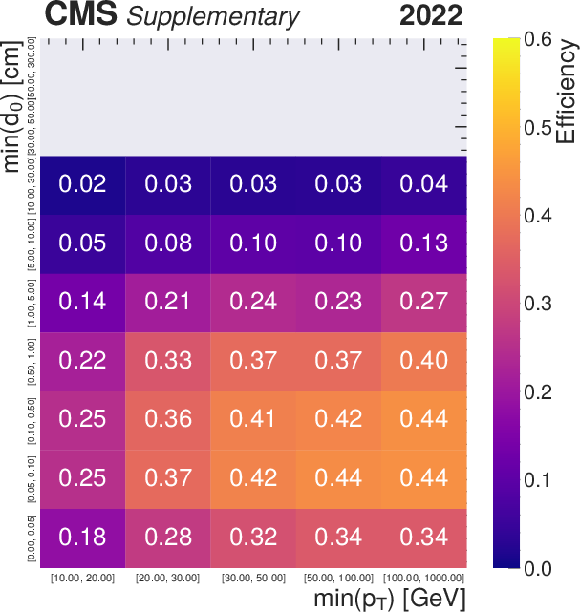
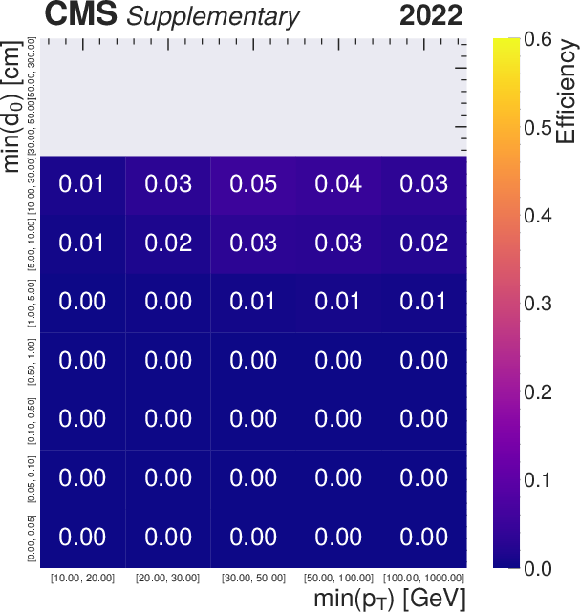
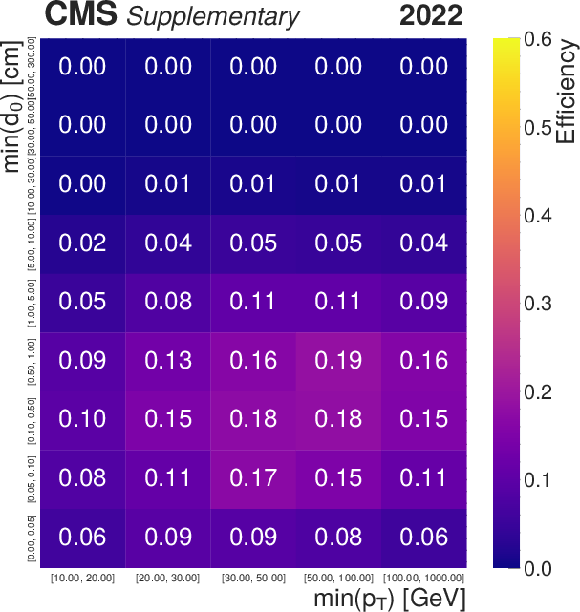
png pdf	Additional Figure 19: Signal efficiencies in the TMS-TMS (left) and STA-STA (right) dimuon category as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ in dimuons with $ L_{\text{xy}}^\mathrm{true} $ smaller than 20 cm (upper), 20--70 cm (middle), and 70-500 cm (lower) in the RPV SUSY signal model. The efficiency in each bin is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The geometric acceptance is defined as the generated longitudinal decay length $ L_{\text{z}}^\mathrm{true} $ smaller than 8 m and $ \|\eta\| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are corrected by the data-to-simulation scale factors described in the paper. Efficiency in the TMS-TMS dimuon category for dimuons with $ L_{\text{xy}}^\mathrm{true} > $ 70 cm is zero and is not shown.
png pdf	Additional Figure 19-a: Signal efficiencies in the TMS-TMS (left) and STA-STA (right) dimuon category as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ in dimuons with $ L_{\text{xy}}^\mathrm{true} $ smaller than 20 cm (upper), 20--70 cm (middle), and 70-500 cm (lower) in the RPV SUSY signal model. The efficiency in each bin is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The geometric acceptance is defined as the generated longitudinal decay length $ L_{\text{z}}^\mathrm{true} $ smaller than 8 m and $ \|\eta\| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are corrected by the data-to-simulation scale factors described in the paper. Efficiency in the TMS-TMS dimuon category for dimuons with $ L_{\text{xy}}^\mathrm{true} > $ 70 cm is zero and is not shown.
png pdf	Additional Figure 19-b: Signal efficiencies in the TMS-TMS (left) and STA-STA (right) dimuon category as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ in dimuons with $ L_{\text{xy}}^\mathrm{true} $ smaller than 20 cm (upper), 20--70 cm (middle), and 70-500 cm (lower) in the RPV SUSY signal model. The efficiency in each bin is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The geometric acceptance is defined as the generated longitudinal decay length $ L_{\text{z}}^\mathrm{true} $ smaller than 8 m and $ \|\eta\| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are corrected by the data-to-simulation scale factors described in the paper. Efficiency in the TMS-TMS dimuon category for dimuons with $ L_{\text{xy}}^\mathrm{true} > $ 70 cm is zero and is not shown.
png pdf	Additional Figure 19-c: Signal efficiencies in the TMS-TMS (left) and STA-STA (right) dimuon category as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ in dimuons with $ L_{\text{xy}}^\mathrm{true} $ smaller than 20 cm (upper), 20--70 cm (middle), and 70-500 cm (lower) in the RPV SUSY signal model. The efficiency in each bin is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The geometric acceptance is defined as the generated longitudinal decay length $ L_{\text{z}}^\mathrm{true} $ smaller than 8 m and $ \|\eta\| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are corrected by the data-to-simulation scale factors described in the paper. Efficiency in the TMS-TMS dimuon category for dimuons with $ L_{\text{xy}}^\mathrm{true} > $ 70 cm is zero and is not shown.
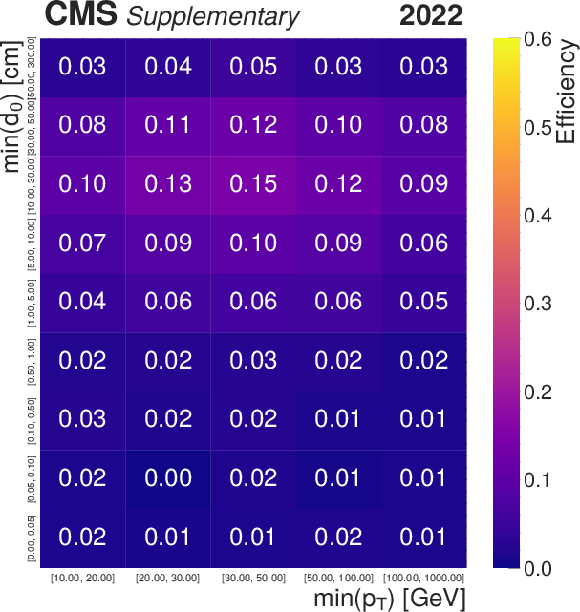
png pdf	Additional Figure 19-d: Signal efficiencies in the TMS-TMS (left) and STA-STA (right) dimuon category as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ in dimuons with $ L_{\text{xy}}^\mathrm{true} $ smaller than 20 cm (upper), 20--70 cm (middle), and 70-500 cm (lower) in the RPV SUSY signal model. The efficiency in each bin is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The geometric acceptance is defined as the generated longitudinal decay length $ L_{\text{z}}^\mathrm{true} $ smaller than 8 m and $ \|\eta\| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are corrected by the data-to-simulation scale factors described in the paper. Efficiency in the TMS-TMS dimuon category for dimuons with $ L_{\text{xy}}^\mathrm{true} > $ 70 cm is zero and is not shown.
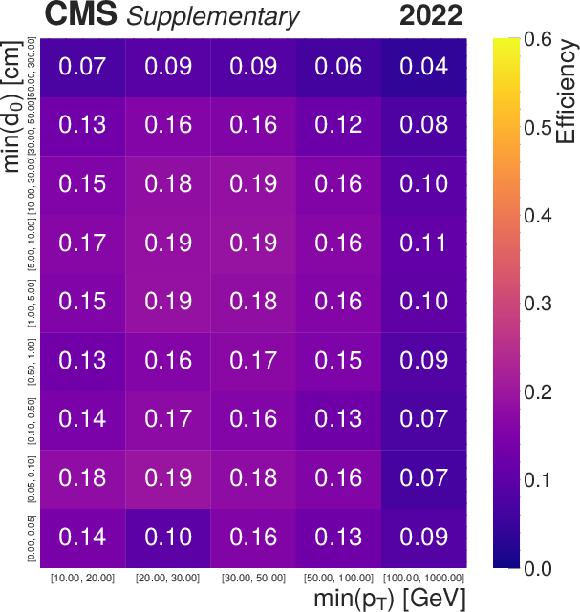
png pdf	Additional Figure 19-e: Signal efficiencies in the TMS-TMS (left) and STA-STA (right) dimuon category as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $ and $ d_{\text{0}} $ in dimuons with $ L_{\text{xy}}^\mathrm{true} $ smaller than 20 cm (upper), 20--70 cm (middle), and 70-500 cm (lower) in the RPV SUSY signal model. The efficiency in each bin is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The geometric acceptance is defined as the generated longitudinal decay length $ L_{\text{z}}^\mathrm{true} $ smaller than 8 m and $ \|\eta\| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are corrected by the data-to-simulation scale factors described in the paper. Efficiency in the TMS-TMS dimuon category for dimuons with $ L_{\text{xy}}^\mathrm{true} > $ 70 cm is zero and is not shown.

HEPData Instructions for Reinterpretation

While the search presented specifically addresses two benchmark models described in the paper, its results may be applied to other models predicting long-lived particles that decay to final states with a pair of oppositely charged muons. In Figs. A18-A19, we provide a set of generator-level efficiency maps that approximate the reconstruction-level efficiencies of this analysis and allow for reinterpretation of the results in the framework of other models.

Signal efficiencies are provided as a function of the smaller of the two values of generated muon $ p_{\mathrm{T}} $, $ \mathrm{min}( p_{\mathrm{T}} ) $, and of generated muon $ d_{\text{0}} $, $ \mathrm{min}( d_{0} ) $, in three intervals of the generated transverse decay length $ L_{\text{xy}} $ of the signal dimuon, $ L_{\text{xy}} < $ 20 cm, 20 $ < L_{\text{xy}} < $ 70 cm, and 70 $ < L_{\text{xy}} < $ 500 cm. The efficiencies are given separately for the HAHM and RPV SUSY signal models used in the paper. Efficiency maps obtained from HAHM samples are recommended for models featuring $ \mu\mu $ decay vertices and collinear signatures, while maps obtained from RPV SUSY samples are recommended for models featuring $ \mu\mu\nu $ decay vertices and non-collinear signatures. The 3D efficiency maps $ \epsilon \left( \mathrm{min}( p_{\mathrm{T}} ); \mathrm{min}( d_{0} ); L_{\text{xy}} \right) $ are provided separately for the two dimuon categories, TMS-TMS and STA-STA, and are shown in Fig. A18 for the HAHM signal model and Fig. A19 for the RPV SUSY signal model. They are valid to approximate the efficiencies in the analysis of the 2022 data set.

The efficiency in each ($ \mathrm{min}( p_{\mathrm{T}} ) $; $ \mathrm{min}( d_{0} ) $; $ L_{\text{xy}} $) bin of the 3D efficiency map is computed as the ratio of the number of simulated signal dimuons in that bin that pass the trigger requirements and selection criteria applied for a given dimuon category to the total number of simulated signal dimuons in that bin and within the geometric acceptance. The computation is performed separately using the ensemble of all generated HAHM and RPV SUSY signal samples listed in the paper. The geometric acceptance is defined as $ \mathrm{min}( d_{0} ) < $ 300 cm, $ L_{\text{xy}} < $ 500 cm, generated longitudinal decay length $ L_{\text{z}} $ smaller than 800 cm, and $ |\eta| $ of both generated muons forming the dimuon smaller than 2.0. The efficiencies obtained from simulation are further corrected by the data-to-simulation scale factors described in the paper.

When applied to the previously untested models, the signal efficiency in each dimuon category $ j $, $ \epsilon_j $, can be obtained from the 3D efficiency maps using the $ p_{\mathrm{T}} $, $ d_{\text{0}} $, and $ L_{\text{xy}} $ at generator level:

$\epsilon_{j} = \frac{1}{N} \sum^{N^\mathrm{acc}}_{n=1} \left[ 1 - \left[ 1 - \epsilon^{n}_{j} \left( \mathrm{min} ( p_{\mathrm{T}} ) ; \mathrm{min} ( d_{0} ) ; L_{\mathrm{xy}} \right) \right]^{k_n} \right] $,
where $ k_{n} $ is the number of dimuons in the $ n $-th event, the sum is over the number of generated signal events $ N^\mathrm{acc} $ in the geometric acceptance defined above and with the true mass larger than 10 GeV, and $ N $ is the total number of generated signal events. In cases where more than one dimuon is present in the event, the one with the larger of the two $ \mathrm{min}( d_{0} ) $ values should be taken as a reference to apply the efficiency map.

In the TMS-TMS category, where the signal region is divided into three bins in the minimum of the two $ d_{\text{0}}/\sigma_{d_{\text{0}}} $ values, $ \epsilon_j $ should be subdivided into three efficiencies depending on $ \mathrm{min}( d_{0} ) $, namely 90-150 $ \mu $m, 150-300 $ \mu $m, and $ > $ 300 $ \mu $m approximately corresponding to the three chosen min($ d_{\text{0}}/\sigma_{d_{\text{0}}} $) bins. The combined signal efficiency, $ \epsilon_\mathrm{tot} $, can be computed as the sum of signal efficiencies in the two dimuon categories:

$ \epsilon_{ \mathrm{tot} } = \sum_j \epsilon_j $,
where $ j $ runs over the TMS-TMS and STA-STA categories.

We have checked that the generator-level efficiency $ \epsilon_\mathrm{tot} $ obtained from the provided 3D efficiency maps approximates the reconstruction-level efficiencies for the HAHM and RPV SUSY signal, shown in Fig. A13 and Figs. A14-A15, respectively, with an accuracy of 20\% or better when the ratio of the LLP mass m(LLP) to the mediator mass m(M) is between 0.05 and 0.35. The method tends to overestimate the efficiency of signal events with a large Lorentz boost (m(LLP)/m(M) $ < $ 0.05), and that of non-relativistic LLPs (0.35 $ < $ m(LLP)/m(M) $ < $ 0.5) with $ c\tau > $ 250 cm. The method is valid for m(LLP) $ > $ 20 GeV.

To obtain the exclusions limits, one should use the signal efficiencies $ \epsilon_j $ in each dimuon category together with the expected number of background events from the paper.

(this text in pdf)
In case of questions, contact Muhammad Ansar Iqbal and Alberto Escalante del Valle.

References
1	J. L. Hewett, B. Lillie, M. Masip, and T. G. Rizzo	Signatures of long-lived gluinos in split supersymmetry	JHEP 09 (2004) 070	hep-ph/0408248
2	R. Barbier et al.	$ R $-parity-violating supersymmetry	Phys. Rept. 420 (2005) 1	hep-ph/0406039
3	M. J. Strassler and K. M. Zurek	Echoes of a hidden valley at hadron colliders	PLB 651 (2007) 374	hep-ph/0604261
4	T. Han, Z. Si, K. M. Zurek, and M. J. Strassler	Phenomenology of hidden valleys at hadron colliders	JHEP 07 (2008) 008	0712.2041
5	CMS Collaboration	Search for long-lived particles decaying to a pair of muons in proton-proton collisions at $ \sqrt{s} = $ 13 TeV	JHEP 05 (2023) 228	CMS-EXO-21-006 2205.08582
6	D. Curtin, R. Essig, S. Gori, and J. Shelton	Illuminating dark photons with high-energy colliders	JHEP 02 (2015) 157	1412.0018
7	CMS Collaboration	Search for long-lived particles decaying into muon pairs in proton-proton collisions at $ \sqrt{s} = $ 13 TeV collected with a dedicated high-rate data stream	JHEP 04 (2022) 062	CMS-EXO-20-014 2112.13769
8	CMS Collaboration	Search for long-lived particles that decay into final states containing two electrons or two muons in proton-proton collisions at $ \sqrt{s} = $ 8 TeV	PRD 91 (2015) 052012	CMS-EXO-12-037 1411.6977
9	CMS Collaboration	Search for long-lived particles that decay into final states containing two muons, reconstructed using only the CMS muon chambers	CMS Physics Analysis Summary, 2015 CMS-PAS-EXO-14-012	CMS-PAS-EXO-14-012
10	ATLAS Collaboration	Search for long-lived particles in final states with displaced dimuon vertices in pp collisions at $ \sqrt{s} = $ 13 TeV with the ATLAS detector	PRD 99 (2019) 012001	1808.03057
11	ATLAS Collaboration	Search for displaced vertices of oppositely charged leptons from decays of long-lived particles in pp collisions at $ \sqrt{s} = $ 13 TeV with the ATLAS detector	PLB 801 (2020) 135114	1907.10037
12	CMS Collaboration	HEPData record for this analysis	link
13	CMS Collaboration	Performance of the CMS muon detector and muon reconstruction with proton-proton collisions at $ \sqrt{s} = $ 13 TeV	JINST 13 (2018) P06015	CMS-MUO-16-001 1804.04528
14	CMS Collaboration	The CMS experiment at the CERN LHC	JINST 3 (2008) S08004
15	CMS Collaboration	Development of the CMS detector for the CERN LHC Run 3	Accepted by JINST, 2023	CMS-PRF-21-001 2309.05466
16	CMS Collaboration	Performance of the CMS Level-1 trigger in proton-proton collisions at $ \sqrt{s} = $ 13 TeV	JINST 15 (2020) P10017	CMS-TRG-17-001 2006.10165
17	CMS Collaboration	The CMS trigger system	JINST 12 (2017) P01020	CMS-TRG-12-001 1609.02366
18	J. D. Wells	How to find a hidden world at the Large Hadron Collider	in Perspectives on LHC Physics, G. Kane and A. Pierce, eds., World Scientific, Singapore, 2008 link	0803.1243
19	J. Alwall et al.	The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations	JHEP 07 (2014) 079	1405.0301
20	M. Cepeda et al.	Report from working group 2: Higgs physics at the HL-LHC and HE-LHC	in Proceedings of the HL/HE-LHC Workshop, A. Dainese et al., eds., vol 7, CERN Yellow Rep. Monogr., 2019 link	1902.00134
21	LHC Higgs Cross Section Working Group	Ad interim 13.6 TeV cross sections	link
22	T. Sjöstrand et al.	An introduction to PYTHIA 8.2	Comp. Phys. Com. 191 (2015) 159	1410.3012
23	H. K. Dreiner	An introduction to explicit $ R $-parity violation	Adv. Ser. Direct. HEP 21 (2010) 565	hep-ph/9707435
24	D. Dercks et al.	$ R $-parity violation at the LHC	EPJC 77 (2017) 856	1706.09418
25	W. Beenakker et al.	NNLL-fast: predictions for coloured supersymmetric particle production at the LHC with threshold and Coulomb resummation	JHEP 12 (2016) 133	1607.07741
26	PDF4LHC Working Group , R. D. Ball et al.	The PDF4LHC21 combination of global PDF fits for the LHC Run III	JPG 49 (2022) 080501	2203.05506
27	NNPDF Collaboration	Parton distributions from high-precision collider data	EPJC 77 (2017) 663	1706.00428
28	CMS Collaboration	Extraction and validation of a new set of CMS PYTHIA8 tunes from underlying-event measurements	EPJC 80 (2020) 4	CMS-GEN-17-001 1903.12179
29	\GEANTfour Collaboration	GEANT 4---a simulation toolkit	NIM A 506 (2003) 250
30	W. A. Nash	Search for long-lived particles that decay to muon pairs at 13 TeV and an algorithm to improve resolution in the muon system of the Compact Muon Solenoid	PhD thesis, UCLA, 2022 link
31	CMS Collaboration	Technical proposal for the Phase-II upgrade of the Compact Muon Solenoid	CMS Technical Proposal CERN-LHCC-2015-010, CMS-TDR-15-02, 2015 CDS
32	CMS Collaboration	Performance of the CMS muon trigger system in proton-proton collisions at $ \sqrt{s} = $ 13 TeV	JINST 16 (2021) P07001	CMS-MUO-19-001 2102.04790
33	CMS Collaboration	Performance of CMS muon reconstruction in pp collision events at $ \sqrt{s} = $ 7 TeV	JINST 7 (2012) P10002	CMS-MUO-10-004 1206.4071
34	LHCb Collaboration	Identification of beauty and charm quark jets at LHCb	JINST 10 (2015) P06013	1504.07670
35	CMS Collaboration	Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV	JINST 13 (2018) P05011	CMS-BTV-16-002 1712.07158
36	CMS Collaboration	First measurement of the top quark pair production cross section in proton-proton collisions at $ \sqrt{s} = $ 13.6 TeV	JHEP 08 (2023) 204	CMS-TOP-22-012 2303.10680
37	A. L. Read	Presentation of search results: The CL$ _{\text{s}} $ technique	JPG 28 (2002) 2693
38	T. Junk	Confidence level computation for combining searches with small statistics	NIM A 434 (1999) 435	hep-ex/9902006
39	CMS Collaboration	The CMS statistical analysis and combination tool: Combine	Submitted to Comput. Softw. Big Sci, 2024	CMS-CAT-23-001 2404.06614
40	CMS Collaboration	A search for decays of the Higgs boson to invisible particles in events with a top-antitop quark pair or a vector boson in proton-proton collisions at $ \sqrt{s}= $ 13 TeV	EPJC 83 (2023) 933	CMS-HIG-21-007 2303.01214
41	CMS Collaboration	Search for invisible decays of a Higgs boson produced through vector boson fusion in proton--proton collisions at $ \sqrt{s} = $ 13 TeV	PRD 105 (2022) 092007	CMS-HIG-20-003 2201.11585
42	CMS Collaboration	Combined measurements of Higgs boson couplings in proton-proton collisions at $ \sqrt{s}= $ 13 TeV	EPJC 79 (2019) 421	CMS-HIG-17-031 1809.10733