Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-PAS-SUS-23-012 | ||
| Search for dark matter produced in association with a Higgs boson decaying to a $ \tau $ lepton pair at $ \sqrt{s}= $ 13 TeV | ||
| CMS Collaboration | ||
| 29 July 2024 | ||
| Abstract: A search for dark matter particles produced in association with a Higgs boson decaying into a pair of $ \tau $ leptons is performed using data collected in proton-proton collisions with the CMS detector at a center-of-mass energy of 13 TeV. The analysis is based on a data set corresponding to an integrated luminosity of 101 fb$ ^{-1} $ collected in 2017--2018 with the CMS detector. No significant excess over the expected standard model background is observed. This result is interpreted within the framework of two benchmark simplified models: the baryonic-Z' and 2HDM+a models. Upper limits at the 95% confidence level are set on the product of the production cross section and branching fraction in the two simplified models. For the baryonic-Z' model, a statistical combination is made with an earlier search based on a data set of 36 fb$ ^{-1} $ collected in 2016. In this model, Z' masses up to 1050 GeV are excluded for a dark matter particle mass of 1 GeV. In the 2HDM+a model, heavy pseudoscalar masses between 400 and 700 GeV are excluded for a light pseudoscalar mass of 100 GeV. | ||
| Links: CDS record (PDF) ; CADI line (restricted) ; | ||
| Figures | |
png pdf |
Figure 1:
Representative Feynman diagrams for leading order (LO) DM associated production with a Higgs boson in the 2HDM+a (left) and baryonic-Z' (right) models. |
png |
Figure 1-a:
Representative Feynman diagrams for leading order (LO) DM associated production with a Higgs boson in the 2HDM+a (left) and baryonic-Z' (right) models. |
png pdf |
Figure 1-b:
Representative Feynman diagrams for leading order (LO) DM associated production with a Higgs boson in the 2HDM+a (left) and baryonic-Z' (right) models. |
png pdf |
Figure 2:
Distributions of the total transverse mass $ M_\mathrm{T}^{\text{tot}} $ in the SR, comparing observed data with the SM prediction in the $ \mathrm{e}\tau_{\mathrm{h}} $ (upper), $ \mu\tau_{\mathrm{h}} $ (center), and $ \tau_{\mathrm{h}}\!\tau_{\mathrm{h}} $ (lower) final states in 2017 (left) and 2018 (right) after the simultaneous maximum likelihood fit. Representative signal distributions are shown for the 2HDM+a (dashed red curve) and baryonic-Z' (dashed black curve) models. The data points are shown with their statistical uncertainties, and the last bin includes overflow. The ``Other MC'' background contribution includes events from ggh, ggZ, VBF, Wh, Zh, and electroweak vector boson production. The uncertainty band accounts for all systematic and statistical sources of uncertainty, after the fit to the data. |
png pdf |
Figure 2-a:
Distributions of the total transverse mass $ M_\mathrm{T}^{\text{tot}} $ in the SR, comparing observed data with the SM prediction in the $ \mathrm{e}\tau_{\mathrm{h}} $ (upper), $ \mu\tau_{\mathrm{h}} $ (center), and $ \tau_{\mathrm{h}}\!\tau_{\mathrm{h}} $ (lower) final states in 2017 (left) and 2018 (right) after the simultaneous maximum likelihood fit. Representative signal distributions are shown for the 2HDM+a (dashed red curve) and baryonic-Z' (dashed black curve) models. The data points are shown with their statistical uncertainties, and the last bin includes overflow. The ``Other MC'' background contribution includes events from ggh, ggZ, VBF, Wh, Zh, and electroweak vector boson production. The uncertainty band accounts for all systematic and statistical sources of uncertainty, after the fit to the data. |
png pdf |
Figure 2-b:
Distributions of the total transverse mass $ M_\mathrm{T}^{\text{tot}} $ in the SR, comparing observed data with the SM prediction in the $ \mathrm{e}\tau_{\mathrm{h}} $ (upper), $ \mu\tau_{\mathrm{h}} $ (center), and $ \tau_{\mathrm{h}}\!\tau_{\mathrm{h}} $ (lower) final states in 2017 (left) and 2018 (right) after the simultaneous maximum likelihood fit. Representative signal distributions are shown for the 2HDM+a (dashed red curve) and baryonic-Z' (dashed black curve) models. The data points are shown with their statistical uncertainties, and the last bin includes overflow. The ``Other MC'' background contribution includes events from ggh, ggZ, VBF, Wh, Zh, and electroweak vector boson production. The uncertainty band accounts for all systematic and statistical sources of uncertainty, after the fit to the data. |
png pdf |
Figure 2-c:
Distributions of the total transverse mass $ M_\mathrm{T}^{\text{tot}} $ in the SR, comparing observed data with the SM prediction in the $ \mathrm{e}\tau_{\mathrm{h}} $ (upper), $ \mu\tau_{\mathrm{h}} $ (center), and $ \tau_{\mathrm{h}}\!\tau_{\mathrm{h}} $ (lower) final states in 2017 (left) and 2018 (right) after the simultaneous maximum likelihood fit. Representative signal distributions are shown for the 2HDM+a (dashed red curve) and baryonic-Z' (dashed black curve) models. The data points are shown with their statistical uncertainties, and the last bin includes overflow. The ``Other MC'' background contribution includes events from ggh, ggZ, VBF, Wh, Zh, and electroweak vector boson production. The uncertainty band accounts for all systematic and statistical sources of uncertainty, after the fit to the data. |
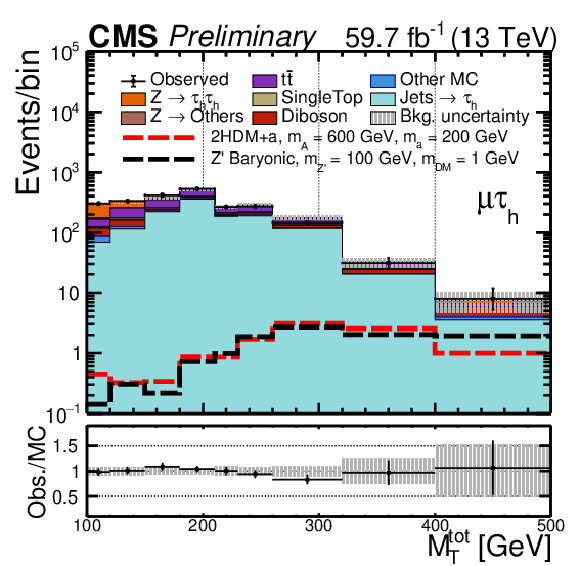
png pdf |
Figure 2-d:
Distributions of the total transverse mass $ M_\mathrm{T}^{\text{tot}} $ in the SR, comparing observed data with the SM prediction in the $ \mathrm{e}\tau_{\mathrm{h}} $ (upper), $ \mu\tau_{\mathrm{h}} $ (center), and $ \tau_{\mathrm{h}}\!\tau_{\mathrm{h}} $ (lower) final states in 2017 (left) and 2018 (right) after the simultaneous maximum likelihood fit. Representative signal distributions are shown for the 2HDM+a (dashed red curve) and baryonic-Z' (dashed black curve) models. The data points are shown with their statistical uncertainties, and the last bin includes overflow. The ``Other MC'' background contribution includes events from ggh, ggZ, VBF, Wh, Zh, and electroweak vector boson production. The uncertainty band accounts for all systematic and statistical sources of uncertainty, after the fit to the data. |
png pdf |
Figure 2-e:
Distributions of the total transverse mass $ M_\mathrm{T}^{\text{tot}} $ in the SR, comparing observed data with the SM prediction in the $ \mathrm{e}\tau_{\mathrm{h}} $ (upper), $ \mu\tau_{\mathrm{h}} $ (center), and $ \tau_{\mathrm{h}}\!\tau_{\mathrm{h}} $ (lower) final states in 2017 (left) and 2018 (right) after the simultaneous maximum likelihood fit. Representative signal distributions are shown for the 2HDM+a (dashed red curve) and baryonic-Z' (dashed black curve) models. The data points are shown with their statistical uncertainties, and the last bin includes overflow. The ``Other MC'' background contribution includes events from ggh, ggZ, VBF, Wh, Zh, and electroweak vector boson production. The uncertainty band accounts for all systematic and statistical sources of uncertainty, after the fit to the data. |
png pdf |
Figure 2-f:
Distributions of the total transverse mass $ M_\mathrm{T}^{\text{tot}} $ in the SR, comparing observed data with the SM prediction in the $ \mathrm{e}\tau_{\mathrm{h}} $ (upper), $ \mu\tau_{\mathrm{h}} $ (center), and $ \tau_{\mathrm{h}}\!\tau_{\mathrm{h}} $ (lower) final states in 2017 (left) and 2018 (right) after the simultaneous maximum likelihood fit. Representative signal distributions are shown for the 2HDM+a (dashed red curve) and baryonic-Z' (dashed black curve) models. The data points are shown with their statistical uncertainties, and the last bin includes overflow. The ``Other MC'' background contribution includes events from ggh, ggZ, VBF, Wh, Zh, and electroweak vector boson production. The uncertainty band accounts for all systematic and statistical sources of uncertainty, after the fit to the data. |
png pdf |
Figure 3:
Upper left: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ m_{\mathrm{a}} $ scan using $ m_{\mathrm{A}} $ = 600 GeV, $ \tan\beta $ = 1, and $ m_{\chi} $ = 10 GeV. Upper right: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ m_{\mathrm{A}} $ using $ m_{\mathrm{a}} $ = 150 GeV, $ \sin\theta $ = 0.35, and $ \tan\beta $ = 1. Lower left: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ \sin\theta $ using $ m_{\mathrm{a}} $ = 200 GeV, $ m_{\mathrm{A}} $ = 600 GeV, $ \tan\beta $ = 1, and $ m_{\chi} $ = 10 GeV. Lower right: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ \tan\beta $ using $ m_{\mathrm{a}} $ = 150 GeV, $ m_{\mathrm{A}} $ = 600 GeV, $ \sin\theta $ = 0.35, and $ m_{\chi} $ = 10 GeV. The interpolation between the points in the 1-d scan is linear. |
png pdf |
Figure 3-a:
Upper left: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ m_{\mathrm{a}} $ scan using $ m_{\mathrm{A}} $ = 600 GeV, $ \tan\beta $ = 1, and $ m_{\chi} $ = 10 GeV. Upper right: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ m_{\mathrm{A}} $ using $ m_{\mathrm{a}} $ = 150 GeV, $ \sin\theta $ = 0.35, and $ \tan\beta $ = 1. Lower left: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ \sin\theta $ using $ m_{\mathrm{a}} $ = 200 GeV, $ m_{\mathrm{A}} $ = 600 GeV, $ \tan\beta $ = 1, and $ m_{\chi} $ = 10 GeV. Lower right: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ \tan\beta $ using $ m_{\mathrm{a}} $ = 150 GeV, $ m_{\mathrm{A}} $ = 600 GeV, $ \sin\theta $ = 0.35, and $ m_{\chi} $ = 10 GeV. The interpolation between the points in the 1-d scan is linear. |
png pdf |
Figure 3-b:
Upper left: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ m_{\mathrm{a}} $ scan using $ m_{\mathrm{A}} $ = 600 GeV, $ \tan\beta $ = 1, and $ m_{\chi} $ = 10 GeV. Upper right: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ m_{\mathrm{A}} $ using $ m_{\mathrm{a}} $ = 150 GeV, $ \sin\theta $ = 0.35, and $ \tan\beta $ = 1. Lower left: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ \sin\theta $ using $ m_{\mathrm{a}} $ = 200 GeV, $ m_{\mathrm{A}} $ = 600 GeV, $ \tan\beta $ = 1, and $ m_{\chi} $ = 10 GeV. Lower right: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ \tan\beta $ using $ m_{\mathrm{a}} $ = 150 GeV, $ m_{\mathrm{A}} $ = 600 GeV, $ \sin\theta $ = 0.35, and $ m_{\chi} $ = 10 GeV. The interpolation between the points in the 1-d scan is linear. |
png pdf |
Figure 3-c:
Upper left: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ m_{\mathrm{a}} $ scan using $ m_{\mathrm{A}} $ = 600 GeV, $ \tan\beta $ = 1, and $ m_{\chi} $ = 10 GeV. Upper right: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ m_{\mathrm{A}} $ using $ m_{\mathrm{a}} $ = 150 GeV, $ \sin\theta $ = 0.35, and $ \tan\beta $ = 1. Lower left: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ \sin\theta $ using $ m_{\mathrm{a}} $ = 200 GeV, $ m_{\mathrm{A}} $ = 600 GeV, $ \tan\beta $ = 1, and $ m_{\chi} $ = 10 GeV. Lower right: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ \tan\beta $ using $ m_{\mathrm{a}} $ = 150 GeV, $ m_{\mathrm{A}} $ = 600 GeV, $ \sin\theta $ = 0.35, and $ m_{\chi} $ = 10 GeV. The interpolation between the points in the 1-d scan is linear. |
png pdf |
Figure 3-d:
Upper left: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ m_{\mathrm{a}} $ scan using $ m_{\mathrm{A}} $ = 600 GeV, $ \tan\beta $ = 1, and $ m_{\chi} $ = 10 GeV. Upper right: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ m_{\mathrm{A}} $ using $ m_{\mathrm{a}} $ = 150 GeV, $ \sin\theta $ = 0.35, and $ \tan\beta $ = 1. Lower left: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ \sin\theta $ using $ m_{\mathrm{a}} $ = 200 GeV, $ m_{\mathrm{A}} $ = 600 GeV, $ \tan\beta $ = 1, and $ m_{\chi} $ = 10 GeV. Lower right: 95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ \tan\beta $ using $ m_{\mathrm{a}} $ = 150 GeV, $ m_{\mathrm{A}} $ = 600 GeV, $ \sin\theta $ = 0.35, and $ m_{\chi} $ = 10 GeV. The interpolation between the points in the 1-d scan is linear. |
png pdf |
Figure 4:
95% CL upper limit on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ in the $ m_{\mathrm{a}} $--$ m_{\mathrm{A}} $ plane. The regions inside the red and black curves correspond to the observed and expected exclusions at 95% CL, respectively. |
png pdf |
Figure 5:
Observed and expected 95% CL upper limits on the signal strength modifier $ \mu=\sigma/\sigma_{theory} $ as a function of $ m_{\mathrm{Z}^{'}} $, using $ m_{\chi} $ = 1 GeV. |
| Tables | |
png pdf |
Table 1:
Target $ \tau_\mathrm{h} $ identification efficiencies for the different working points defined for the three different discriminators [30] that are used in the analysis. These identification efficiencies are evaluated for the genuine $ \tau_\mathrm{h} $ with the $ \mathrm{H}\to\tau\tau $ event sample for $ \tau_\mathrm{h} $ with $ p_{\mathrm{T}} \in $[30, 70] GeV. |
png pdf |
Table 2:
Offline selection requirement applied to e, $ \mu $ and $ \tau_\mathrm{h} $ candidates used for the selection of $ \tau $ pairs. The expressions first and second lepton refer to the label of the final state in the first column for both years. The $ p_{\mathrm{T}} $ requirements are given in GeV. |
png pdf |
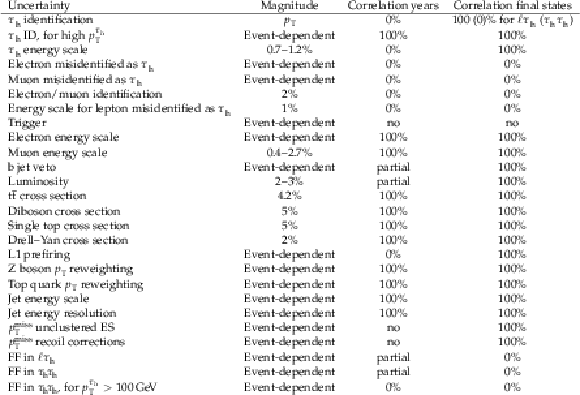
Table 3:
Sources of systematic uncertainties. |
| Summary |
| A search for dark matter produced in association with a Higgs boson decaying to a pair of $ \tau $ leptons has been performed. A data set of proton-proton collisions at a center-of-mass energy of 13 TeV, corresponding to an integrated luminosity of 101 fb$ ^{-1} $ is analyzed, and for the baryonic-Z' model, the analysis results are combined with those of an earlier search using an independent data set collected at the same center-of-mass energy, corresponding to an integrated luminosity of 36 fb$ ^{-1} $ [5]. The data are found to be in good agreement with the fit results, with no evidence for a significant signal contribution. This result is interpreted within the framework of two benchmark simplified models: the baryonic-Z' model, where a high mass resonance (Z') decays into a dark matter particle $ \chi $ and a standard model Higgs boson h, and the 2HDM+a model, where a heavy pseudoscalar couples to a Higgs boson and a ligher pseudoscalar decaying to dark matter particles. Upper limits at the 95% confidence level are set on the product of the production cross section and branching fraction for the baryonic-Z' and 2HDM+a models. For the baryonic-Z' model,Z' masses up to 1050 GeV are excluded for a dark matter particle mass of 1 GeV using 137 fb$ ^{-1} $ of proton-proton data. In the 2HDM+a model, heavy pseudoscalar masses between 400 and 700 GeV are excluded for 101 fb$ ^{-1} $ of proton-proton data for a light pseudoscalar mass around 100 GeV. |
| References | ||||
| 1 | J. L. Feng | Dark matter candidates from particle physics and methods of detection | Ann. Rev. Astron. Astrophys. 48 (2010) 495 | 1003.0904 |
| 2 | T. A. Porter, R. P. Johnson, and P. W. Graham | Dark matter searches with astroparticle data | Ann. Rev. Astron. Astrophys. 49 (2011) 155 | 1104.2836 |
| 3 | D. Clowe, A. Gonzalez, and M. Markevitch | Weak lensing mass reconstruction of the interacting cluster 1e0657-558: Direct evidence for the existence of dark matter | Astrophys. J. 604 (2004) 596 | astro-ph/0312273 |
| 4 | Planck Collaboration | Planck 2015 results. xiii. cosmological parameters | Astron. Astrophys. 594 (2016) A13 | 1502.01589 |
| 5 | CMS Collaboration | Search for dark matter produced in association with a Higgs boson decaying to $ \gamma\gamma $ or $ \tau^+\tau^- $ at $ \sqrt{s} = $ 13 TeV | JHEP 09 (2018) 046 | CMS-EXO-16-055 1806.04771 |
| 6 | G. Bertone, D. Hooper, and J. Silk | Particle dark matter: Evidence, candidates and constraints | Phys. Rept. 405 (2005) 279 | hep-ph/0404175 |
| 7 | C. P. Burgess, M. Pospelov, and T. ter Veldhuis | The minimal model of nonbaryonic dark matter: A singlet scalar | NPB 619 (2001) 709 | hep-ph/0011335 |
| 8 | J. March-Russell, S. M. West, D. Cumberbatch, and D. Hooper | Heavy dark matter through the Higgs portal | JHEP 07 (2008) 058 | 0801.3440 |
| 9 | L. Carpenter et al. | Mono-Higgs-boson: A new collider probe of dark matter | PRD 89 (2014) 075017 | 1312.2592 |
| 10 | A. Berlin, T. Lin, and L.-T. Wang | Mono-Higgs detection of dark matter at the LHC | JHEP 06 (2014) 078 | 1402.7074 |
| 11 | A. A. Petrov and W. Shepherd | Searching for dark matter at LHC with mono-Higgs production | PLB 730 (2014) 178 | 1311.1511 |
| 12 | D. Abercrombie et al. | Dark matter benchmark models for early LHC run-2 searches: Report of the ATLAS/CMS dark matter forum | Phys. Dark Univ. 27 (2020) 100371 | 1507.00966 |
| 13 | LHC Dark Matter Working Group Collaboration | LHC dark matter working group: Next-generation spin-0 dark matter models | Phys. Dark Univ. 27 (2020) 100351 | 1810.09420 |
| 14 | M. Bauer, U. Haisch, and F. Kahlhoefer | Simplified dark matter models with two Higgs doublets: I. Pseudoscalar mediators | JHEP 05 (2017) 138 | 1701.07427 |
| 15 | A. Boveia et al. | Recommendations on presenting LHC searches for missing transverse energy signals using simplified $ s $-channel models of dark matter | Phys. Dark Univ. 27 (2020) 100365 | 1603.04156 |
| 16 | ATLAS Collaboration | Search for dark matter produced in association with a Higgs boson decaying to tau leptons at $ \sqrt{s} = $ 13 TeV with the ATLAS detector | JHEP 09 (2023) 189 | 2305.12938 |
| 17 | CMS Collaboration | Performance of the CMS level-1 trigger in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | JINST 15 (2020) P10017 | CMS-TRG-17-001 2006.10165 |
| 18 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 19 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | |
| 20 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 21 | CMS Collaboration | Electron and photon reconstruction and identification with the CMS experiment at the CERN LHC | JINST 16 (2021) P05014 | CMS-EGM-17-001 2012.06888 |
| 22 | CMS Collaboration | Performance of the CMS muon detector and muon reconstruction with proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JINST 13 (2018) P06015 | CMS-MUO-16-001 1804.04528 |
| 23 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_{\mathrm{T}} $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 24 | M. Cacciari, G. P. Salam, and G. Soyez | Fastjet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 25 | CMS Collaboration | Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV | JINST 12 (2017) P02014 | CMS-JME-13-004 1607.03663 |
| 26 | CMS Collaboration | Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV | JINST 13 (2018) P05011 | CMS-BTV-16-002 1712.07158 |
| 27 | E. Bols et al. | Jet flavour classification using DeepJet | JINST 15 (2020) P12012 | 2008.10519 |
| 28 | CMS Collaboration | Jet algorithms performance in 13 TeV data | CMS Physics Analysis Summary, 2017 CMS-PAS-JME-16-003 |
CMS-PAS-JME-16-003 |
| 29 | CMS Collaboration | Performance of reconstruction and identification of $ \tau $ leptons decaying to hadrons and $ \nu_\tau $ in pp collisions at $ \sqrt{s}= $ 13 TeV | JINST 13 (2018) P10005 | CMS-TAU-16-003 1809.02816 |
| 30 | CMS Collaboration | Identification of hadronic tau lepton decays using a deep neural network | JINST 17 (2022) P07023 | CMS-TAU-20-001 2201.08458 |
| 31 | CMS Collaboration | Performance of missing transverse momentum reconstruction in proton-proton collisions at $ \sqrt{s} = $ 13 TeV using the CMS detector | JINST 14 (2019) P07004 | CMS-JME-17-001 1903.06078 |
| 32 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 33 | T. Sjöstrand et al. | An introduction to PYTHIA 8.2 | Comput. Phys. Commun. 191 (2015) 159 | 1410.3012 |
| 34 | CMS Collaboration | Extraction and validation of a new set of CMS PYTHIA8 tunes from underlying-event measurements | EPJC 80 (2020) 4 | CMS-GEN-17-001 1903.12179 |
| 35 | J. Alwall et al. | Comparative study of various algorithms for the merging of parton showers and matrix elements in hadronic collisions | EPJC 53 (2008) 473 | 0706.2569 |
| 36 | K. Melnikov and F. Petriello | Electroweak gauge boson production at hadron colliders through $ \mathcal{O}(\alpha_s^2) $ | PRD 74 (2006) 114017 | hep-ph/0609070 |
| 37 | CMS Collaboration | Observation of the Higgs boson decay to a pair of $ \tau $ leptons with the CMS detector | PLB 779 (2018) 283 | CMS-HIG-16-043 1708.00373 |
| 38 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with parton shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 39 | S. Alioli, P. Nason, C. Oleari, and E. Re | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX | JHEP 06 (2010) 043 | 1002.2581 |
| 40 | S. Alioli et al. | Jet pair production in POWHEG | JHEP 04 (2011) 081 | 1012.3380 |
| 41 | S. Alioli, P. Nason, C. Oleari, and E. Re | NLO Higgs boson production via gluon fusion matched with shower in POWHEG | JHEP 04 (2009) 002 | 0812.0578 |
| 42 | R. Frederix and S. Frixione | Merging meets matching in MC@NLO | JHEP 12 (2012) 061 | 1209.6215 |
| 43 | NNPDF Collaboration | Parton distributions for the LHC Run II | JHEP 04 (2015) 040 | 1410.8849 |
| 44 | P. Skands, S. Carrazza, and J. Rojo | Tuning PYTHIA 8.1: the Monash 2013 tune | EPJC 74 (2014) 3024 | 1404.5630 |
| 45 | GEANT4 Collaboration | GEANT 4---a simulation toolkit | NIM A 506 (2003) 250 | |
| 46 | CMS Collaboration | Measurement of differential cross sections for the production of top quark pairs and of additional jets in lepton+jets events from pp collisions at $ \sqrt{s} = $ 13 TeV | PRD 97 (2018) 112003 | CMS-TOP-17-002 1803.08856 |
| 47 | CMS Collaboration | Measurements of Higgs boson production in the decay channel with a pair of $ \tau $ leptons in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | EPJC 83 (2023) 562 | CMS-HIG-19-010 2204.12957 |
| 48 | CMS Collaboration | Performance of CMS muon reconstruction in pp collision events at $ \sqrt{s}= $ 7 TeV | JINST 7 (2012) P10002 | CMS-MUO-10-004 1206.4071 |
| 49 | CMS Collaboration | Measurement of the production cross section of a Higgs boson with large transverse momentum in its decays to a pair of $ \tau $ leptons in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | Submitted to Phys. Lett. B, 2024 | CMS-HIG-21-017 2403.20201 |
| 50 | CMS Collaboration | The CMS statistical analysis and combination tool: Combine | Submitted to Comput. Softw. Big Sci, 2024 | CMS-CAT-23-001 2404.06614 |
| 51 | T. Junk | Confidence level computation for combining searches with small statistics | NIM A 434 (1999) 435 | hep-ex/9902006 |
| 52 | A. L. Read | Presentation of search results: The CL$ _s $ technique | JPG 28 (2002) 2693 | |
| 53 | G. Cowan, K. Cranmer, E. Gross, and O. Vitells | Asymptotic formulae for likelihood-based tests of new physics | EPJC 71 (2011) 1554 | 1007.1727 |
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|