

Compact Muon Solenoid
LHC, CERN
| CMS-PAS-SUS-17-003 | ||
| Search for pair production of tau sleptons in √s= 13 TeV pp collisions in the all-hadronic final state | ||
| CMS Collaboration | ||
| July 2017 | ||
| Abstract: A search for direct tau slepton pair production in pp collisions at a center-of-mass energy of 13 TeV is presented. The data correspond to an integrated luminosity of 35.9 fb−1 collected with the CMS detector at the Run-2 of the CERN LHC in 2016. The search is performed using events with two hadronically decaying tau leptons and a large imbalance in the measured transverse momentum of the event. The results are interpreted as upper limits on the cross section for tau slepton pair production in different helicity scenarios. | ||
|
Links:
CDS record (PDF) ;
inSPIRE record ;
CADI line (restricted) ;
These preliminary results are superseded in this paper, JHEP 11 (2018) 151. The superseded preliminary plots can be found here. |
||
| Figures & Tables | Summary | Additional Figures & Tables | References | CMS Publications |
|---|
|
Additional information on efficiencies needed for reinterpretation of these results are available here. Additional technical material for CMS speakers can be found here. |
| Figures | |

png pdf |
Figure 1:
Simplified model for direct stau pair production followed by each stau decaying to a τ lepton and an LSP. |

png pdf |
Figure 2:
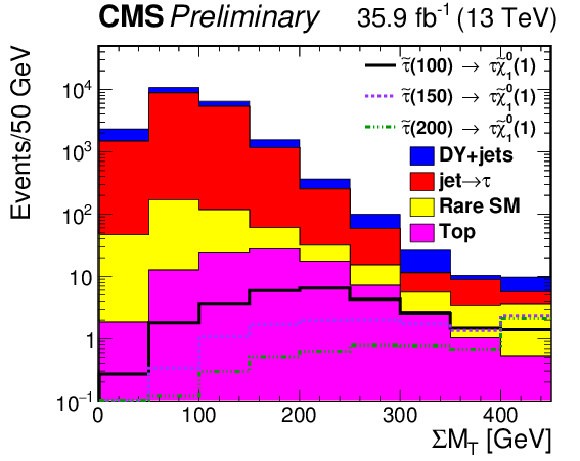
The ΣMT (left) and MT2 (right) distributions after the baseline selection. The signatures of stau pair production with different stau masses are shown. A requirement of large MT2, while efficient at reducing the SM background, greatly reduces the signal acceptance for low stau masses. We therefore define additional search regions with moderate MT2 and use ΣMT as a discriminating variable to target smaller stau masses. Three signal hypotheses in the maximally-mixed scenario are overlaid, with the first number indicating the stau mass and the second the LSP mass. |
png pdf |
Figure 2-a:
The ΣMT distribution after the baseline selection. The signatures of stau pair production with different stau masses are shown. A requirement of large MT2, while efficient at reducing the SM background, greatly reduces the signal acceptance for low stau masses. We therefore define additional search regions with moderate MT2 and use ΣMT as a discriminating variable to target smaller stau masses. Three signal hypotheses in the maximally-mixed scenario are overlaid, with the first number indicating the stau mass and the second the LSP mass. |

png pdf |
Figure 2-b:
The MT2 distribution after the baseline selection. The signatures of stau pair production with different stau masses are shown. A requirement of large MT2, while efficient at reducing the SM background, greatly reduces the signal acceptance for low stau masses. We therefore define additional search regions with moderate MT2 and use ΣMT as a discriminating variable to target smaller stau masses. Three signal hypotheses in the maximally-mixed scenario are overlaid, with the first number indicating the stau mass and the second the LSP mass. |
png pdf |
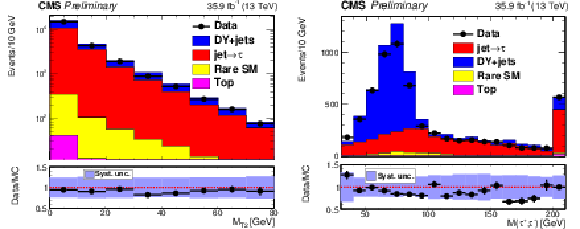
Figure 3:
(Left) Closure test for the fake rate method in a data control region where the MT2 or ΣMT requirements are inverted. The predicted and observed yields show good agreement. (Right) The visible mass spectrum is used to validate our modeling of Drell-Yan backgrounds. A minimum di-τh pT of 50 GeV is required to reduce the QCD multijet background. Data and simulation agree within the experimental uncertainties. |
png pdf |
Figure 3-a:
Closure test for the fake rate method in a data control region where the MT2 or ΣMT requirements are inverted. The predicted and observed yields show good agreement. |

png pdf |
Figure 3-b:
The visible mass spectrum is used to validate our modeling of Drell-Yan backgrounds. A minimum di-τh pT of 50 GeV is required to reduce the QCD multijet background. Data and simulation agree within the experimental uncertainties. |

png pdf |
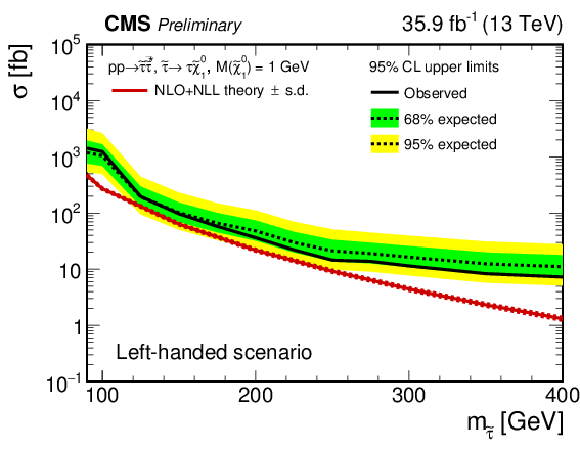
Figure 4:
The excluded stau pair production cross section as a function of the stau mass for the three different helicities: left-handed (left), maximally-mixed (middle), right-handed (right). The plots in the top row assume a fixed LSP mass of 1 GeV, the ones on the middle row 20 GeV, and the ones on the bottom row 50 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. |
png pdf root |
Figure 4-a:
The excluded stau pair production cross section as a function of the stau mass for left-handed helicity. The plot assumes a fixed LSP mass of 1 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. |
png pdf root |
Figure 4-b:
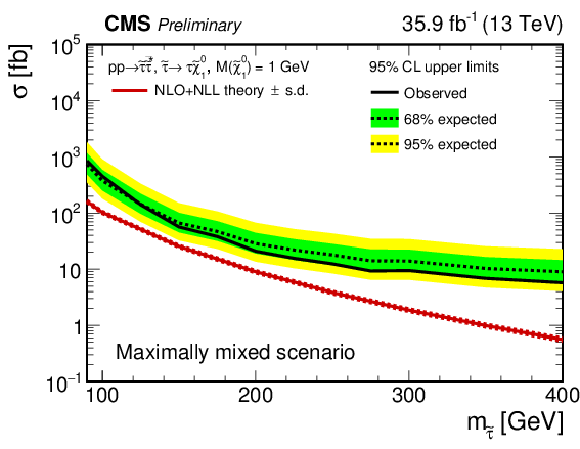
The excluded stau pair production cross section as a function of the stau mass for maximally-mixed helicity. The plot assumes a fixed LSP mass of 1 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. |
png pdf root |
Figure 4-c:
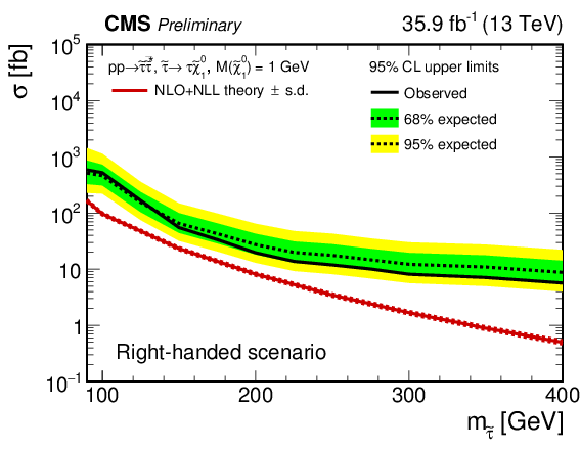
The excluded stau pair production cross section as a function of the stau mass for right-handed helicity. The plot assumes a fixed LSP mass of 1 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. |
png pdf root |
Figure 4-d:
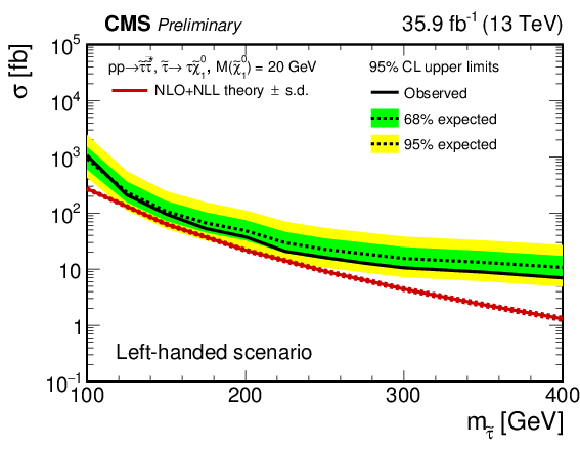
The excluded stau pair production cross section as a function of the stau mass for left-handed helicity. The plot assumes a fixed LSP mass of 20 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. |
png pdf root |
Figure 4-e:
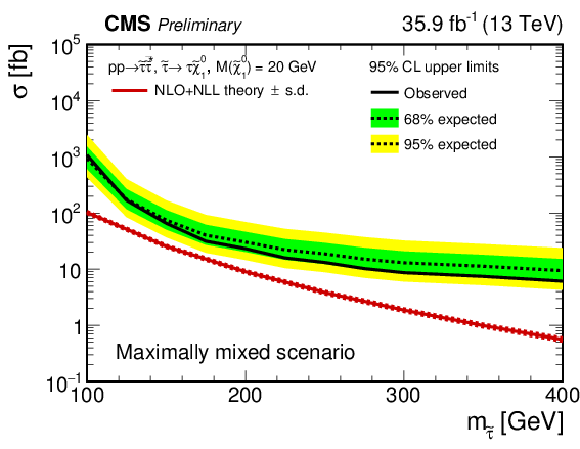
The excluded stau pair production cross section as a function of the stau mass for maximally-mixed helicity. The plot assumes a fixed LSP mass of 20 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. |
png pdf root |
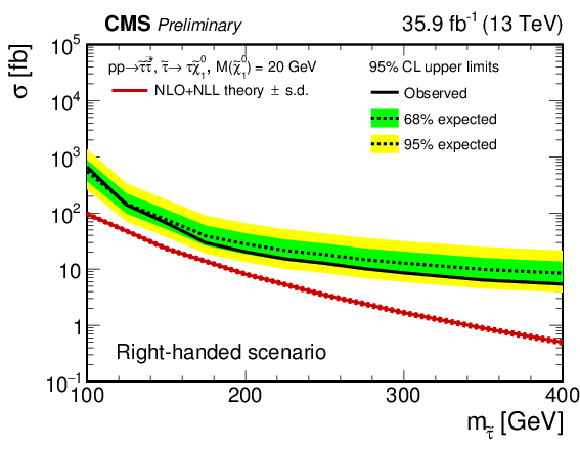
Figure 4-f:
The excluded stau pair production cross section as a function of the stau mass for right-handed helicity. The plot assumes a fixed LSP mass of 20 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. |
png pdf root |
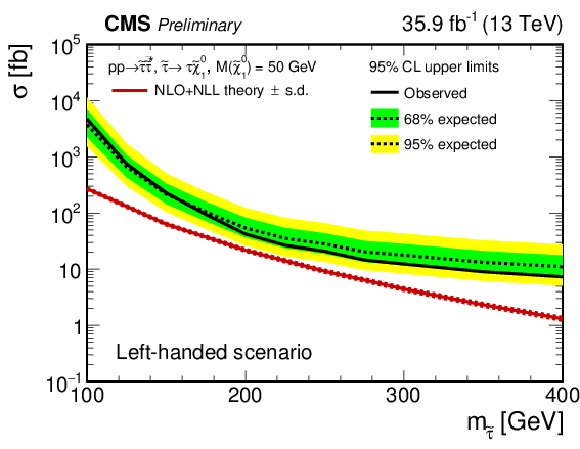
Figure 4-g:
The excluded stau pair production cross section as a function of the stau mass for left-handed helicity. The plot assumes a fixed LSP mass of 50 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. |
png pdf root |
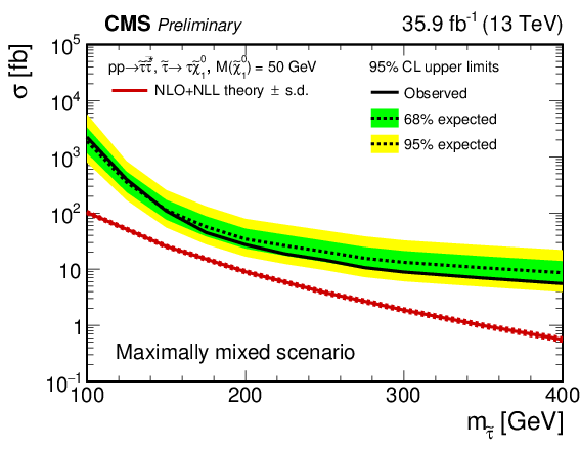
Figure 4-h:
The excluded stau pair production cross section as a function of the stau mass for maximally-mixed helicity. The plot assumes a fixed LSP mass of 50 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. |
png pdf root |
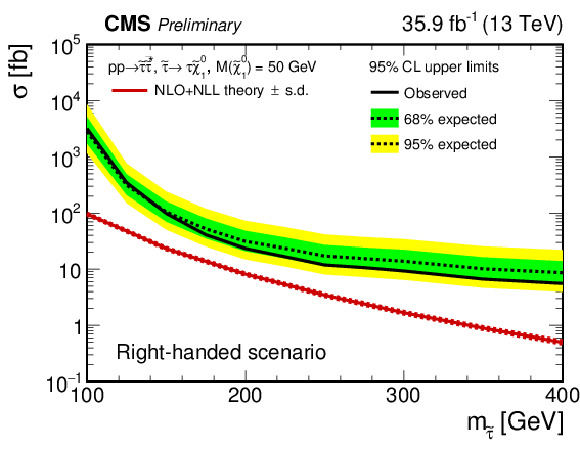
Figure 4-i:
The excluded stau pair production cross section as a function of the stau mass for right-handed helicity. The plot assumes a fixed LSP mass of 50 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. |
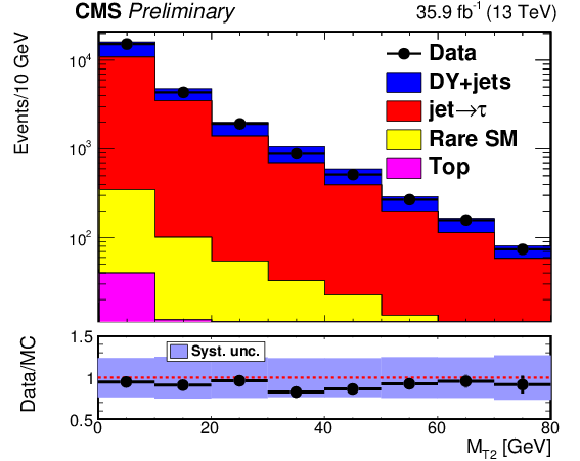
| Tables | |
png pdf |
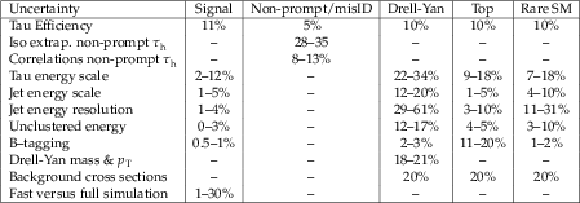
Table 1:
The largest systematic uncertainties in the analysis for the signal models and the different SM background predictions. For the signal models the uncertainties are re-evaluated for the different mass hypotheses. |

png pdf |
Table 2:
Final predicted and observed event yields in all SRs with all statistical and systematic uncertainties combined. For the background estimates with no events in the sideband or the simulated sample, the 68% statistical upper limit is presented. For the total background estimate the central value and the uncertainties are extracted from the full pre-fit likelihood. |
| Summary |
| A search for tau sleptons in the all-hadronic final state was performed in pp collisions at a center-of-mass energy of 13 TeV using three complementary search regions. The data sample corresponds to an integrated luminosity of 35.9 fb−1. No excess was observed in any of the search regions. Upper limits on the cross section of direct tau slepton (stau) pair production are derived, for each stau decaying to a tau lepton and an LSP. The analysis is most sensitive to left-handed staus. For a left-handed stau of 125 GeV decaying to a massless LSP the observed limit is 1.5 times the expected production cross section in the simplified model. |
| Additional Figures | |
png pdf |
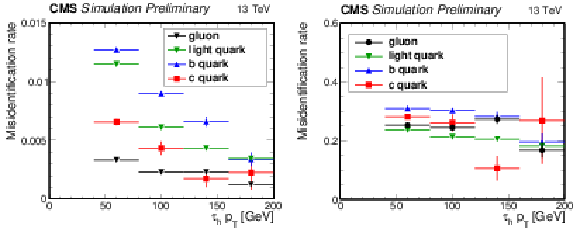
Additional Figure 1:
Misidentification rate as a function of τ pT for various parton types without any cut on the isolation (left) or after applying a loose isolation requirement as done in the analysis (right). The variation across parton types is greatly reduced by applying such a requirement. After applying the loose isolation requirement, a systematic uncertainty of 30% is assigned to the misidentification rate to cover the jet parton dependence as this analysis does not determine the jet parton type. |

png pdf |
Additional Figure 1-a:
Misidentification rate as a function of τ pT for various parton types without any cut on the isolation. The variation across parton types is greatly reduced by applying such a requirement. |

png pdf |
Additional Figure 1-b:
Misidentification rate as a function of τ pT for various parton types after applying a loose isolation requirement as done in the analysis. The variation across parton types is greatly reduced by applying such a requirement. After applying the loose isolation requirement, a systematic uncertainty of 30% is assigned to the misidentification rate to cover the jet parton dependence as this analysis does not determine the jet parton type. |
png pdf |
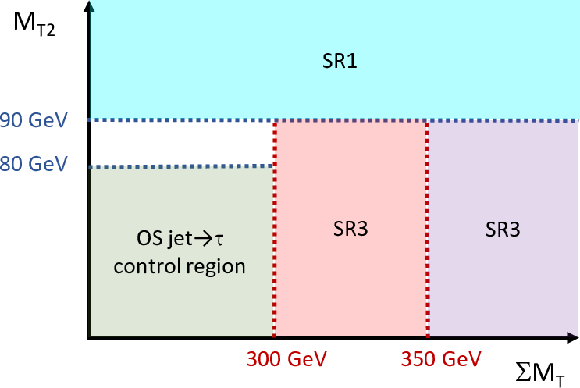
Additional Figure 2:
Illustration showing the complementarity of the different search and control regions. |

png pdf |
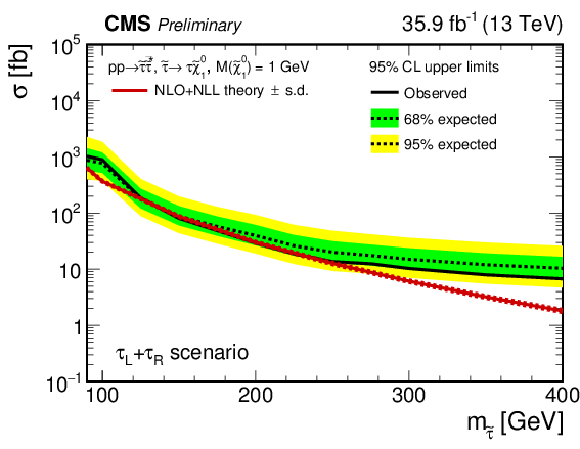
Additional Figure 3:
The excluded cross sections for stau pair production as a function of the stau mass for a model where the left-handed and right-handed staus are considered to be mass degenerate. The plots are shown for a fixed LSP mass of 1 GeV (left), 20 GeV (middle), and 50 GeV (right). The inner (green) band and the outer (yellow) band indicate the regions containing 68% (1 s.d.) and 95% (2 s.d.), respectively, of the distribution of limits expected under the background-only hypothesis. Several mass hypotheses are just excluded in this model. |
png pdf |
Additional Figure 3-a:
The excluded cross sections for stau pair production as a function of the stau mass for a model where the left-handed and right-handed staus are considered to be mass degenerate. The plots are shown for a fixed LSP mass of 1 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68% (1 s.d.) and 95% (2 s.d.), respectively, of the distribution of limits expected under the background-only hypothesis. Several mass hypotheses are just excluded in this model. |

png pdf |
Additional Figure 3-b:
The excluded cross sections for stau pair production as a function of the stau mass for a model where the left-handed and right-handed staus are considered to be mass degenerate. The plots are shown for a fixed LSP mass of 20 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68% (1 s.d.) and 95% (2 s.d.), respectively, of the distribution of limits expected under the background-only hypothesis. Several mass hypotheses are just excluded in this model. |

png pdf |
Additional Figure 3-c:
The excluded cross sections for stau pair production as a function of the stau mass for a model where the left-handed and right-handed staus are considered to be mass degenerate. The plots are shown for a fixed LSP mass of 50 GeV. The inner (green) band and the outer (yellow) band indicate the regions containing 68% (1 s.d.) and 95% (2 s.d.), respectively, of the distribution of limits expected under the background-only hypothesis. Several mass hypotheses are just excluded in this model. |
| Additional Tables | |

png pdf |
Additional Table 1:
Cut-flow for different mass points of the left-handed stau sample for all three search regions corresponding to 35.9 fb−1 of integrated luminosity for various signal model points, given as the mass pair (˜τ,˜χ01). The yields are normalized to the theoretical cross sections. The baseline selection requires exactly two hadronic tau candidates passing the kinematic and trigger requirements and no additional electrons or muons. |
| References | ||||
| 1 | G. 't Hooft | Naturalness, chiral symmetry, and spontaneous chiral symmetry breaking | NATO Sci. Ser. B 59 (1980)135 | |
| 2 | E. Witten | Dynamical breaking of supersymmetry | Nucl. Phys. B 188 (1981) 513 | |
| 3 | M. Dine, W. Fischler, and M. Srednicki | Supersymmetric technicolor | Nucl. Phys. B 189 (1981) 575 | |
| 4 | S. Dimopoulos and S. Raby | Supercolor | Nucl. Phys. B 192 (1981) 353 | |
| 5 | S. Dimopoulos and H. Georgi | Softly broken supersymmetry and SU(5) | Nucl. Phys. B 193 (1981) 150 | |
| 6 | R. K. Kaul and P. Majumdar | Cancellation of quadratically divergent mass corrections in globally supersymmetric spontaneously broken gauge theories | Nucl. Phys. B 199 (1982) 36 | |
| 7 | J. Wess and B. Zumino | Supergauge transformations in four-dimensions | Nucl. Phys. B 70 (1974) 39 | |
| 8 | G. R. Farrar and P. Fayet | Phenomenology of the Production, Decay, and Detection of New Hadronic States Associated with Supersymmetry | PLB 76 (1978) 575 | |
| 9 | C. Boehm, A. Djouadi, and M. Drees | Light scalar top quarks and supersymmetric dark matter | PRD 62 (2000) 035012 | hep-ph/9911496 |
| 10 | C. Bal\'azs, M. Carena, and C. E. M. Wagner | Dark matter, light stops and electroweak baryogenesis | PRD 70 (2004) 015007 | hep-ph/403224 |
| 11 | G. Jungman, M. Kamionkowski, and K. Griest | Supersymmetric dark matter | PR 267 (1996) 195 | hep-ph/9506380 |
| 12 | G. Hinshaw and et al. | Nine-Year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Cosmological Parameter Results | The Astrophysical Journal Supplement Series 208 (2013) 19 | 1212.5226 |
| 13 | K. Griest and D. Seckel | Three exceptions in the calculation of relic abundances | PRD 43 (1991) 3191 | |
| 14 | D. A. Vasquez, G. Belanger, and C. Boehm | Revisiting light neutralino scenarios in the MSSM | PRD 84 (2011) 095015 | 1108.1338 |
| 15 | S. King, J. Roberts, and D. Roy | Natural Dark Matter in SUSY GUTs with Non-universal Gaugino Masses | JHEP 10 (2007) 106 | 0705.4219 |
| 16 | J. Ellis, T. Fak, K. A. Olive, and M. Srednicki | Natural Dark Matter in SUSY GUTs with Non-universal Gaugino Masses | Astropart. Phys. 13 (2000) 181 | 9905481 |
| 17 | LEP SUSY Working Group | Notes LEPSUSYWG/04-01.1 | ||
| 18 | ATLAS Collaboration | Search for the direct production of charginos, neutralinos and staus in final states with at least two hadronically decaying taus and missing transverse momentum in pp collisions at √s= 8 TeV with the ATLAS detector | JHEP 10 (2014) 96 | 1407.0350 |
| 19 | ATLAS Collaboration | Search for the electroweak production of supersymmetric particles in √s= 8 TeV pp collisions with the ATLAS detector | PRD 93 (2016) 052002 | 1509.07152 |
| 20 | CMS Collaboration | Search for electroweak production of charginos in final states with two tau leptons in pp collisions at √s= 8 TeV | JHEP 04 (2017) 018 | CMS-SUS-14-022 1610.04870 |
| 21 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | Submitted to JINST | CMS-PRF-14-001 1706.04965 |
| 22 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-kt jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 23 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 24 | CMS Collaboration | Study of pileup removal algorithms for jets | CMS-PAS-JME-14-001 | CMS-PAS-JME-14-001 |
| 25 | CMS Collaboration | Identification of b-quark jets with the CMS experiment | JINST 8 (2013) P04013 | CMS-BTV-12-001 1211.4462 |
| 26 | CMS Collaboration | Identification of b quark jets at the CMS experiment in the LHC Run 2 | CMS-PAS-BTV-15-001 | CMS-PAS-BTV-15-001 |
| 27 | CMS Collaboration | Reconstruction and identification of τ lepton decays to hadrons and ντ at CMS | JINST 11 (2016) P01019 | CMS-TAU-14-001 1510.07488 |
| 28 | CMS Collaboration | Performance of reconstruction and identification of tau leptons in their decays to hadrons and tau neutrino in LHC Run-2 | CMS-PAS-TAU-16-002 | CMS-PAS-TAU-16-002 |
| 29 | CMS Collaboration | Performance of electron reconstruction and selection with the CMS detector in proton-proton collisions at √s= 8 TeV | JINST 10 (2015) P06005 | CMS-EGM-13-001 1502.02701 |
| 30 | CMS Collaboration | Performance of CMS muon reconstruction in pp collision events at √s= 7 TeV | JINST 7 (2012) P10002 | CMS-MUO-10-004 1206.4071 |
| 31 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 32 | NNPDF Collaboration | Parton distributions for the LHC Run II | JHEP 04 (2015) 040 | 1410.8849 |
| 33 | P. Nason | A new method for combining NLO QCD with shower Monte Carlo algorithms | JHEP 11 (2004) 040 | hep-ph/0409146 |
| 34 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with Parton Shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 35 | S. Alioli, P. Nason, C. Oleari, and E. Re | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX | JHEP 06 (2010) 043 | 1002.2581 |
| 36 | E. Re | Single-top Wt-channel production matched with parton showers using the POWHEG method | EPJC 71 (2011) 1547 | 1009.2450 |
| 37 | T. Sjostrand et al. | An introduction to PYTHIA 8.2 | CPC 191 (2015) 159 | 1410.3012 |
| 38 | GEANT4 Collaboration | GEANT4 --- a simulation toolkit | NIMA 506 (2003) 250 | |
| 39 | S. Abdullin et al. | The fast simulation of the CMS detector at LHC | J. Phys. Conf. Ser. 331 (2011) 032049 | |
| 40 | B. Fuks, M. Klasen, D. R. Lamprea, and M. Rothering | Revisiting slepton pair production at the Large Hadron Collider | JHEP 01 (2014) 168 | 1310.2621 |
| 41 | C. G. Lester and D. J. Summers | Measuring masses of semiinvisibly decaying particles pair produced at hadron colliders | PLB 463 (1999) 99 | hep-ph/9906349 |
| 42 | A. Barr, C. Lester, and P. Stephens | m(T2): The truth behind the glamour | JPG 29 (2003) 2343 | hep-ph/0304226 |
| 43 | CMS Collaboration | Interpretation of searches for supersymmetry with simplified models | PRD 88 (2013) 052017 | CMS-SUS-11-016 1301.2175 |
| 44 | J. Alwall, P. Schuster, and N. Toro | Simplified models for a first characterization of new physics at the lhc | PRD 79 (2009) 075020 | |
| 45 | J. Alwall, M.-P. Le, M. Lisanti, and J. Wacker | Model-independent jets plus missing energy searches | PRD 79 (2009) 015005 | |
| 46 | LHC New Physics Working Group, D. Alves et al. | Simplified models for LHC new physics searches | JPG 39 (2012) 105005 | 1105.2838 |
| 47 | T. Junk | Confidence level computation for combining searches with small statistics | NIMA 434 (1999) 435 | hep-ex/9902006 |
| 48 | A. L. Read | Presentation of search results: the CLS technique | JPG 28 (2002) 2693 | |
| 49 | ATLAS and CMS Collaborations, LHC Higgs Combination Group | Procedure for the LHC Higgs boson search combination in Summer 2011 | ATL-PHYS-PUB 2011-11, CMS NOTE 2011/005 | |
| 50 | G. Cowan, K. Cranmer, E. Gross, and O. Vitells | Asymptotic formulae for likelihood-based tests of new physics | EPJC 71 (2011) 1554 | 1007.1727 |

|
Compact Muon Solenoid LHC, CERN |

|

|

|

|

|

|