

Compact Muon Solenoid
LHC, CERN
| CMS-PAS-HIG-17-002 | ||
| Search for pair production of Higgs bosons in the two tau leptons and two bottom quarks final state using proton-proton collisions at √s= 13 TeV | ||
| CMS Collaboration | ||
| March 2017 | ||
| Abstract: A search for the production of Higgs boson pairs in proton-proton collisions at a centre of mass energy of 13 TeV is presented, making use of a data integrated luminosity of 35.9 fb−1 collected with the CMS detector at the LHC. Final states with one Higgs boson decaying into two tau leptons and the other one decaying into two bottom quarks are explored to investigate both the resonant and non-resonant production mechanisms. The observed data are well described by standard model processes and no evidence for a signal contribution is found. For resonant production, upper limits at 95% confidence level are set on the production cross section of Higgs boson pairs as a function of the resonance mass, and are interpreted in the context of the minimal supersymmetric standard model. For non-resonant production, upper limits on the production cross section constrain the parameter space for anomalous Higgs boson couplings. The observed (expected) limit corresponds to about 28 (25) times the prediction of the standard model. | ||
|
Links:
CDS record (PDF) ;
inSPIRE record ;
CADI line (restricted) ;
These preliminary results are superseded in this paper, PLB 778 (2018) 101. The superseded preliminary plots can be found here. |
||
| Figures | |
png pdf |
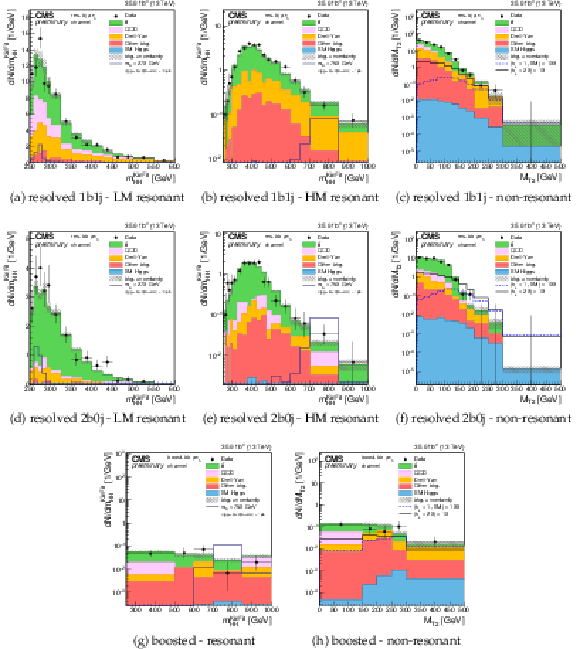
Figure 1:
Distributions of the events observed in the signal regions of the τμτh final state. The first, second, and third row show the resolved 1b1j, 2b0j, and boosted regions respectively. Figures (a),(b),(d),(e),(g) show the distribution of the mKinFitHH variable and Figures (c),(f),(h) show the distribution of the MT2 variable. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
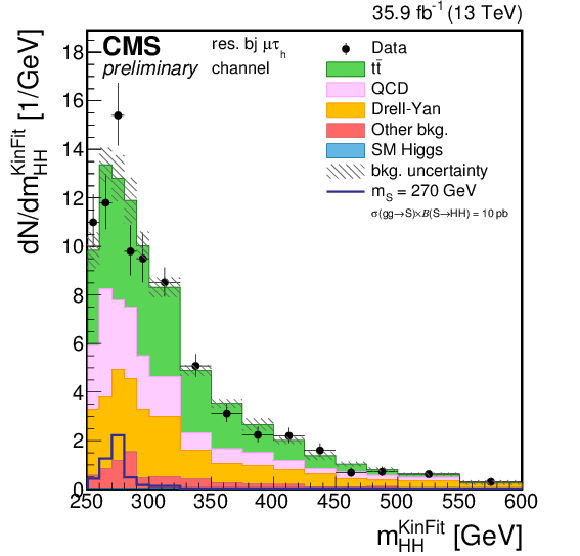
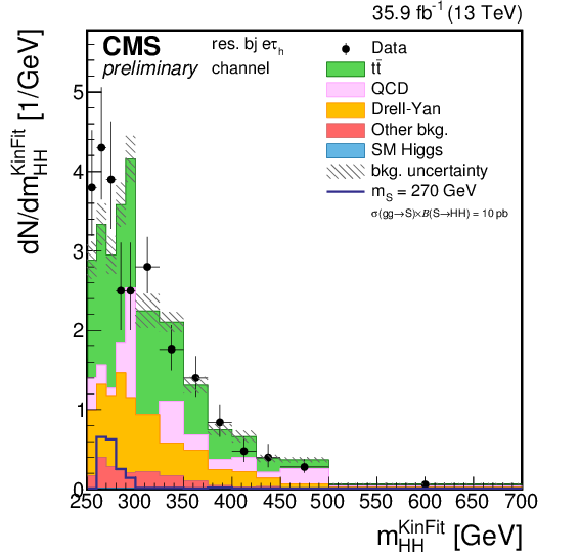
Figure 1-a:
Distribution of the mKinFitHH variable in the resolved 1b1j - LM resonant signal region of the τμτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
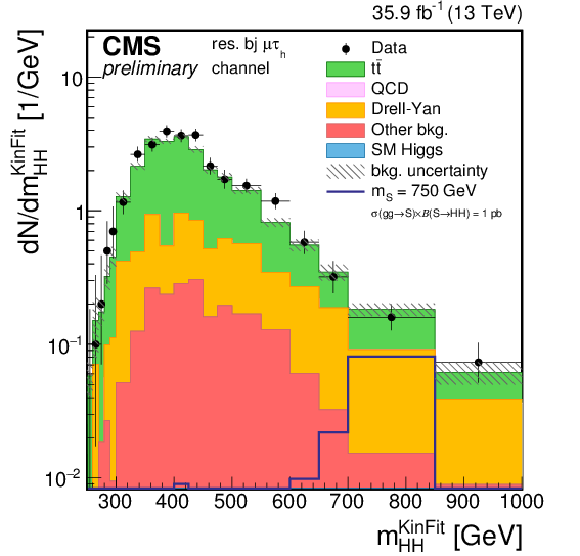
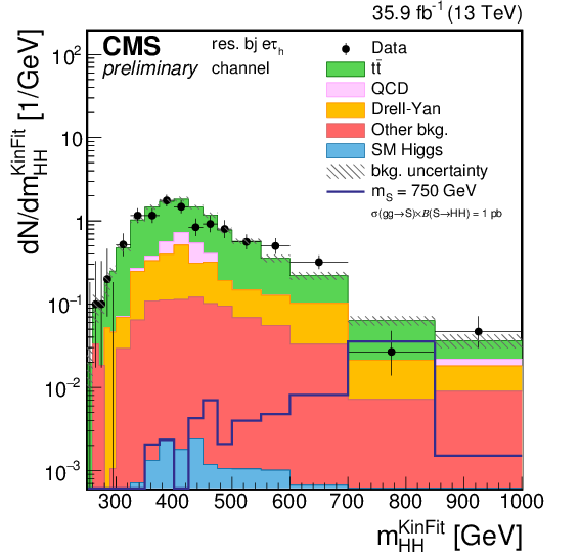
Figure 1-b:
Distribution of the mKinFitHH variable in the resolved 1b1j - HM resonant signal region of the τμτh final state.Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
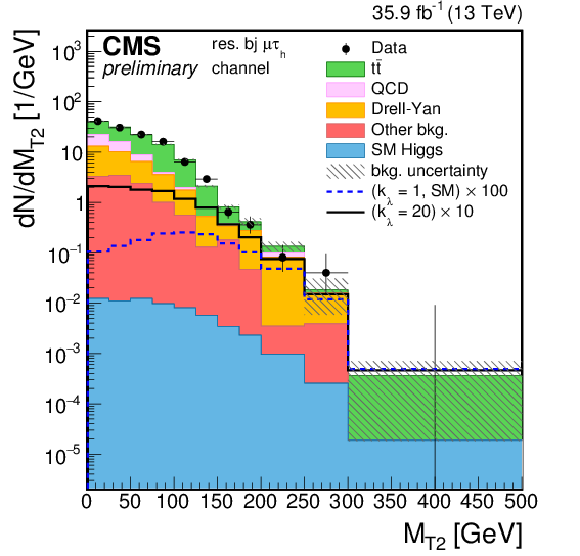
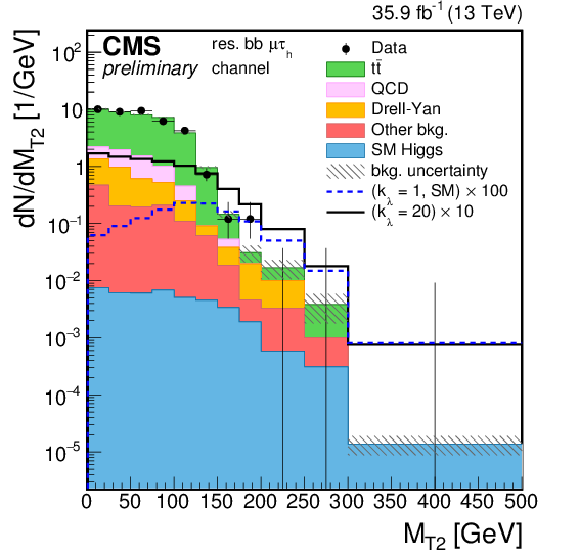
Figure 1-c:
Distribution of the MT2 variable in the resolved 1b1j - non-resonant signal region of the τμτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
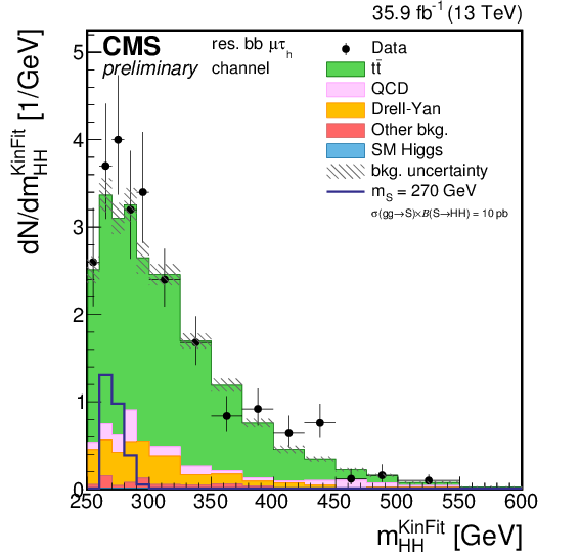
Figure 1-d:
Distribution of the mKinFitHH variable in the resolved 2b0j - LM resonant signal region of the τμτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
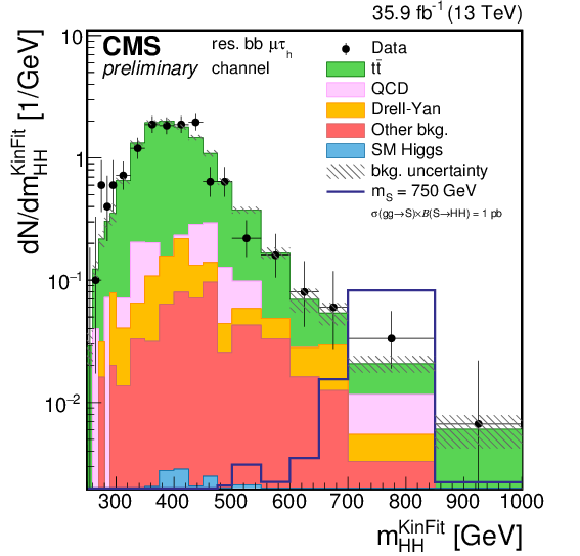
Figure 1-e:
Distribution of the mKinFitHH variable in the resolved 2b0j - HM resonant signal region of the τμτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
Figure 1-f:
Distribution of the MT2 variable in the resolved 2b0j - non-resonant signal region of the τμτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
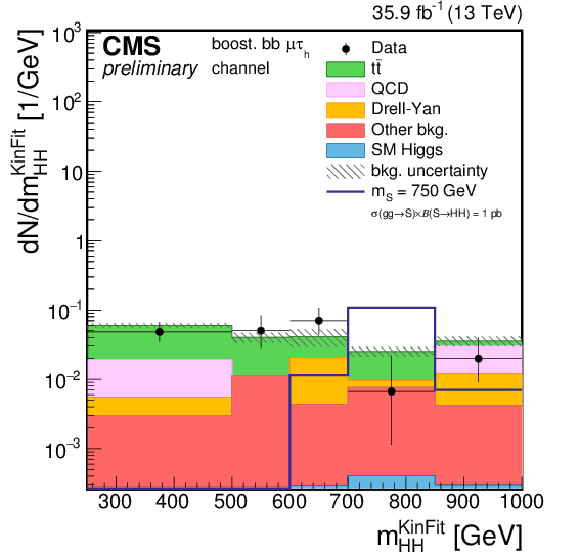
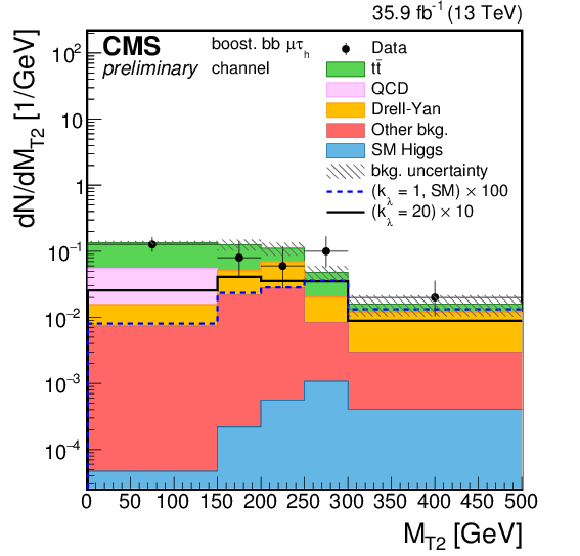
Figure 1-g:
Distribution of the mKinFitHH variable in the boosted - resonant signal region of the τμτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
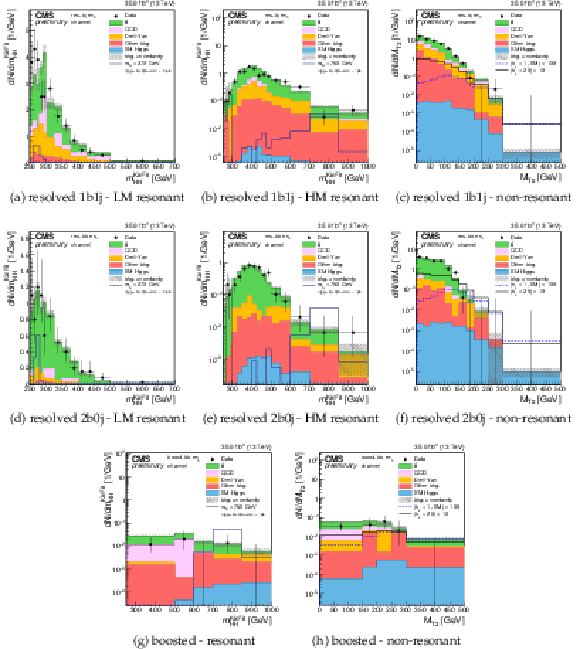
Figure 1-h:
Distribution of the MT2 variable in the boosted - non-resonant signal region of the τμτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
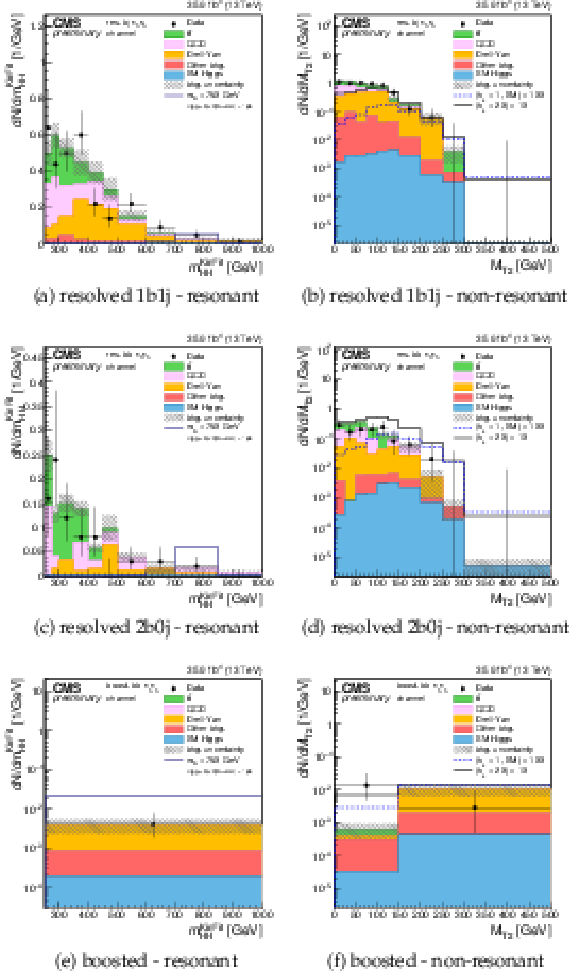
Figure 2:
Distributions of the events observed in the signal regions of the τeτh final state. The first, second, and third row show the resolved 1b1j, 2b0j, and boosted regions respectively. Figures (a),(b),(d),(e),(g) show the distribution of the mKinFitHH variable and Figures (c),(f),(h) show the distribution of the MT2 variable. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
Figure 2-a:
Distribution of the mKinFitHH variable in the resolved 1b1j - LM resonant signal region of the τeτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
Figure 2-b:
Distribution of the mKinFitHH variable in the resolved 1b1j - HM resonant signal region of the τeτh final state.Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
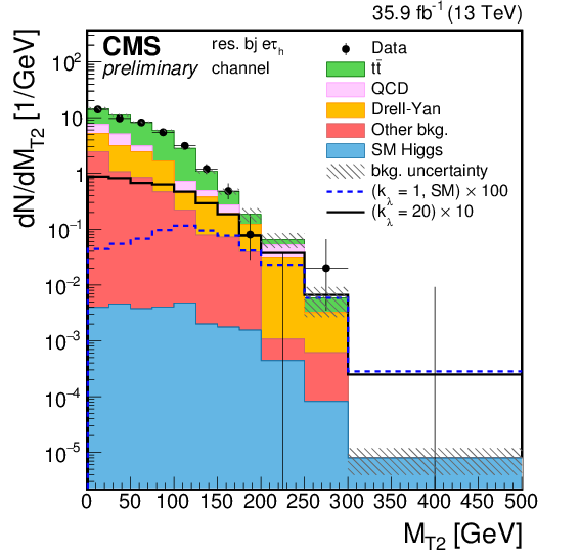
Figure 2-c:
Distribution of the MT2 variable in the resolved 1b1j - non-resonant signal region of the τeτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
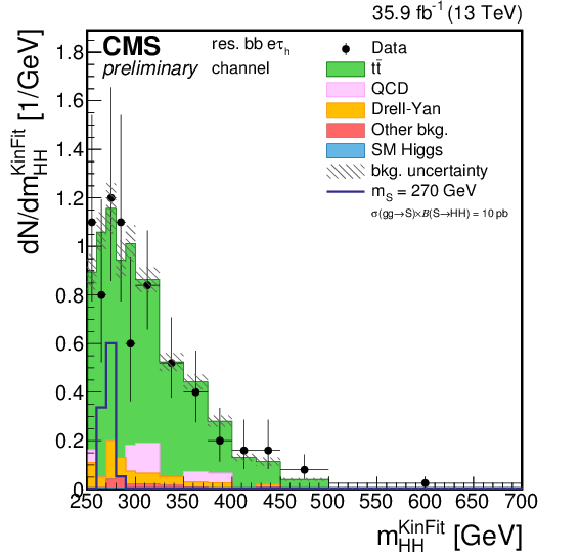
Figure 2-d:
Distribution of the mKinFitHH variable in the resolved 2b0j - LM resonant signal region of the τeτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
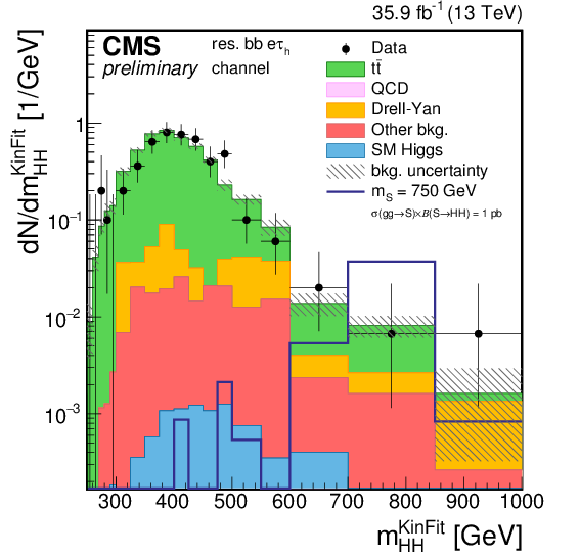
Figure 2-e:
Distribution of the mKinFitHH variable in the resolved 2b0j - HM resonant signal region of the τeτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
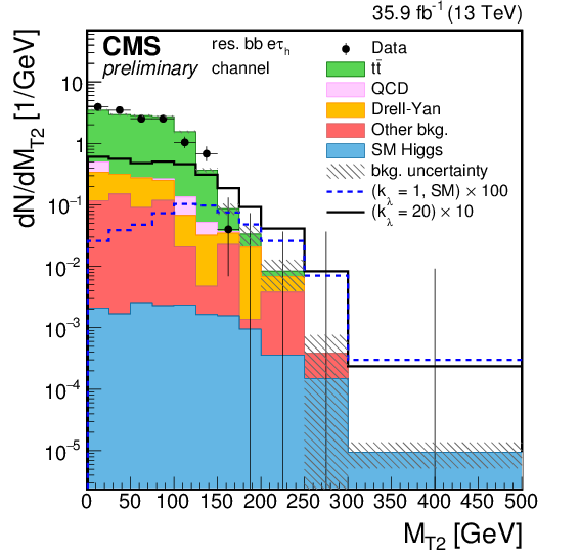
Figure 2-f:
Distribution of the MT2 variable in the resolved 2b0j - non-resonant signal region of the τeτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
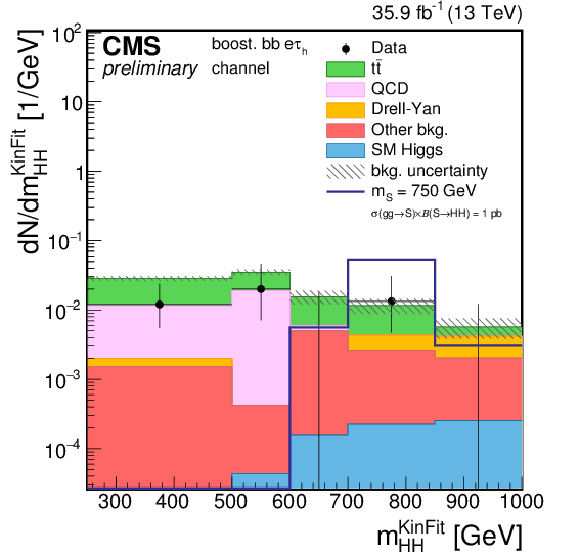
Figure 2-g:
Distribution of the mKinFitHH variable in the boosted - resonant signal region of the τeτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
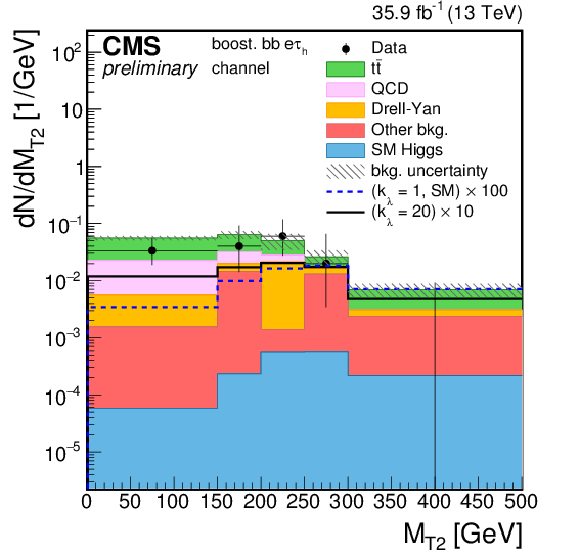
Figure 2-h:
Distribution of the MT2 variable in the boosted - non-resonant signal region of the τeτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
Figure 3:
Distributions of the events observed in the signal regions of the τhτh final state. The first, second, and third row show the resolved 1b1j, 2b0j, and boosted regions respectively. Figures (a),(c),(e) show the distribution of the mKinFitHH variable and Figures (b),(d),(f) show the distribution of the MT2 variable. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
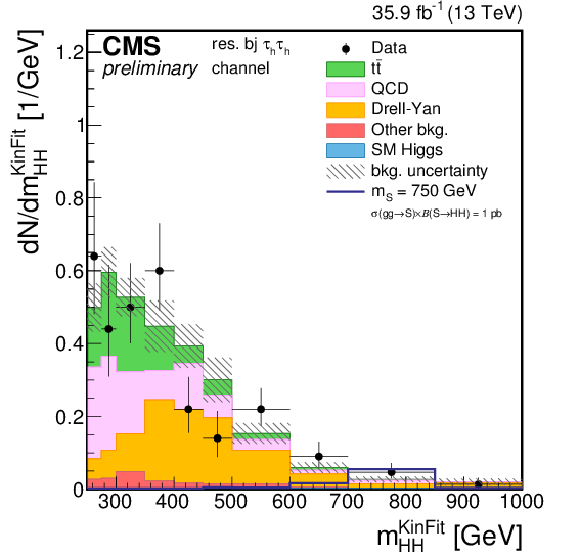
png pdf |
Figure 3-a:
Distribution of the mKinFitHH variable in the resolved 1b1j - resonant signal region of the τhτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
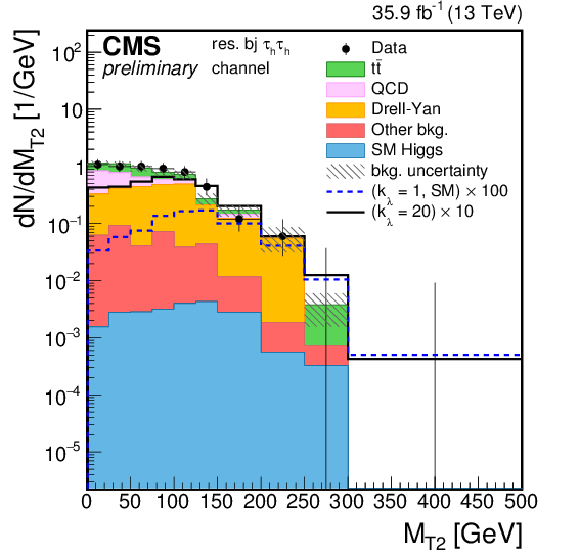
png pdf |
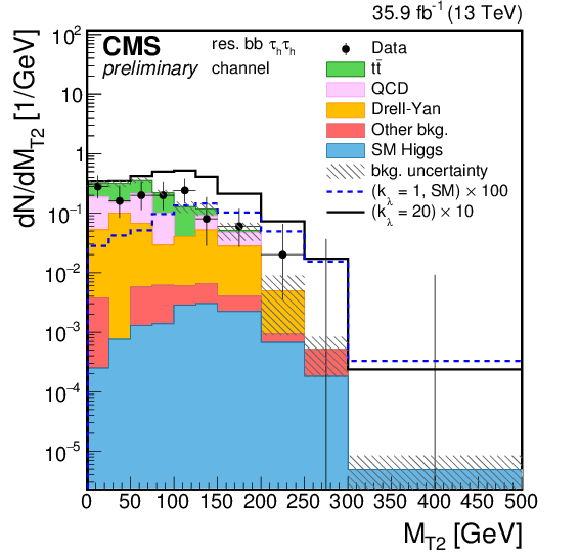
Figure 3-b:
Distribution of the MT2 variable in the resolved 1b1j - non-resonant signal region of the τhτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
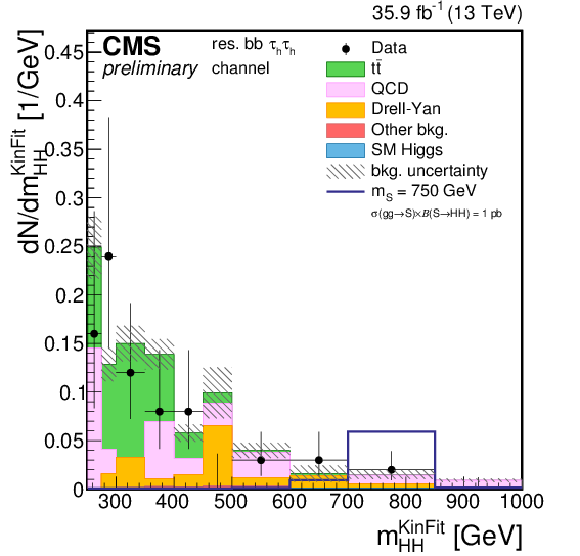
Figure 3-c:
Distribution of the mKinFitHH variable in the resolved 2b0j - resonant signal region of the τhτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
Figure 3-d:
Distribution of the MT2 variable in the resolved 2b0j - non-resonant signal region of the τhτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
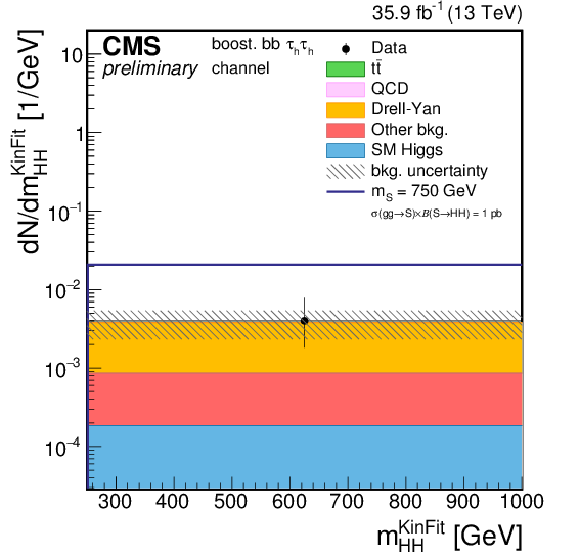
png pdf |
Figure 3-e:
Distribution of the mKinFitHH variable in the boosted - resonant signal region of the τhτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
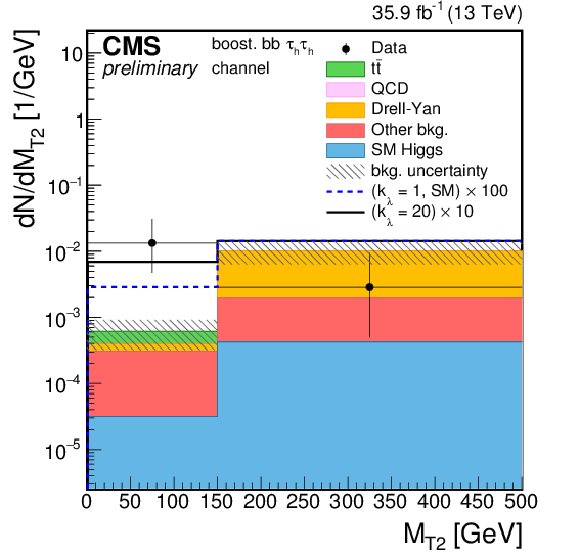
Figure 3-f:
Distribution of the MT2 variable in the boosted - non-resonant signal region of the τhτh final state. Points with error bars represent the data and shaded histograms represent the backgrounds, while the solid lines represent the expected signal yields and are not stacked to the background histograms. The dashed areas correspond to the systematic uncertainty band of the background estimates. Distributions and nuisances are shown after the fit to the observed data. |
png pdf |
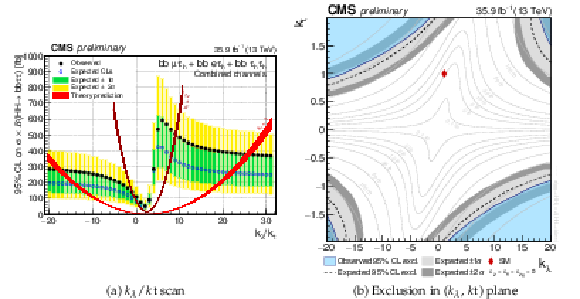
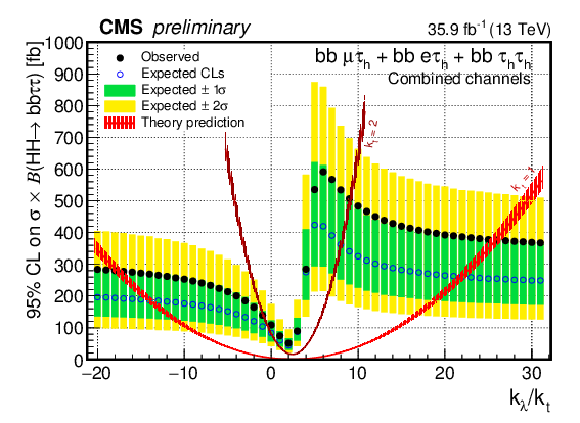
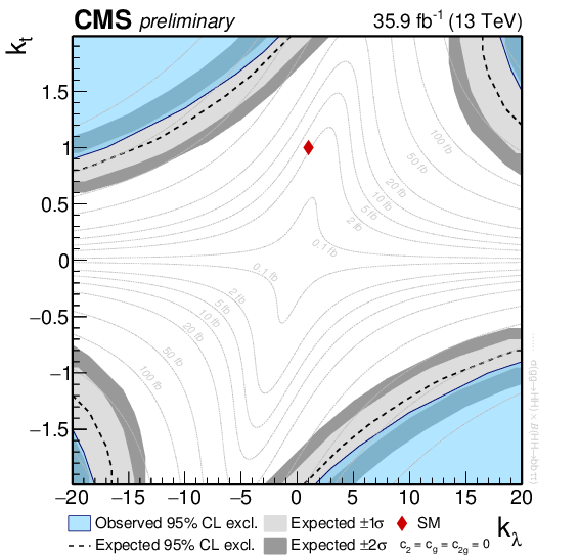
Figure 4:
(a): observed and expected 95% CL upper limits on cross section times branching fraction as a function of kλ/kt. The two red bands show the theoretical cross section expectations and the corresponding uncertainties for kt= 1 and kt= 2. (b): test of kλ and kt anomalous couplings. The blue region denotes the parameters excluded at 95% CL with the observed data, while the dashed black line and the gray regions denote the expected exclusions and the 1σ and 2σ bands. The dotted gray lines indicate trajectories in the plane with equal values of cross section times branching fraction that are displayed in the associated labels. The red marker denotes the couplings predicted by the SM. In both figures, the couplings that are not explicitly tested are assumed to correspond to the SM prediction. |
png pdf |
Figure 4-a:
Observed and expected 95% CL upper limits on cross section times branching fraction as a function of kλ/kt. The two red bands show the theoretical cross section expectations and the corresponding uncertainties for kt= 1 and kt= 2. The couplings that are not explicitly tested are assumed to correspond to the SM prediction. |
png pdf |
Figure 4-b:
Test of kλ and kt anomalous couplings. The blue region denotes the parameters excluded at 95% CL with the observed data, while the dashed black line and the gray regions denote the expected exclusions and the 1σ and 2σ bands. The dotted gray lines indicate trajectories in the plane with equal values of cross section times branching fraction that are displayed in the associated labels. The red marker denotes the couplings predicted by the SM. The couplings that are not explicitly tested are assumed to correspond to the SM prediction. |
png pdf |
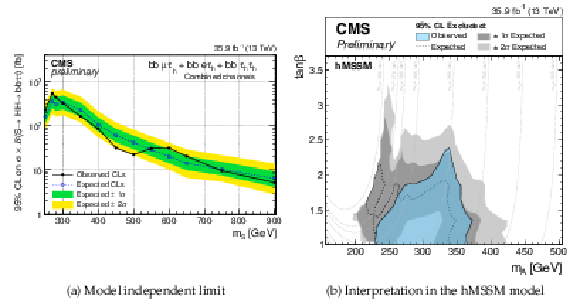
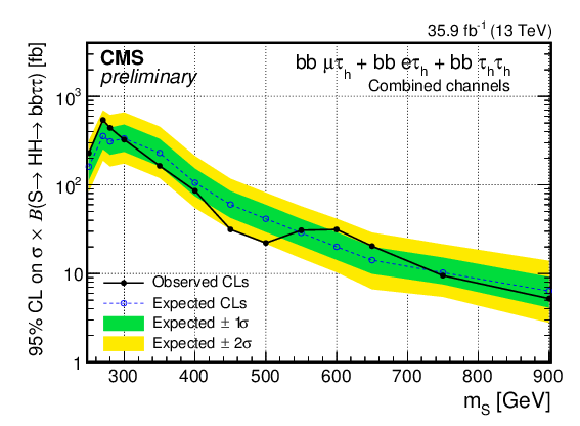
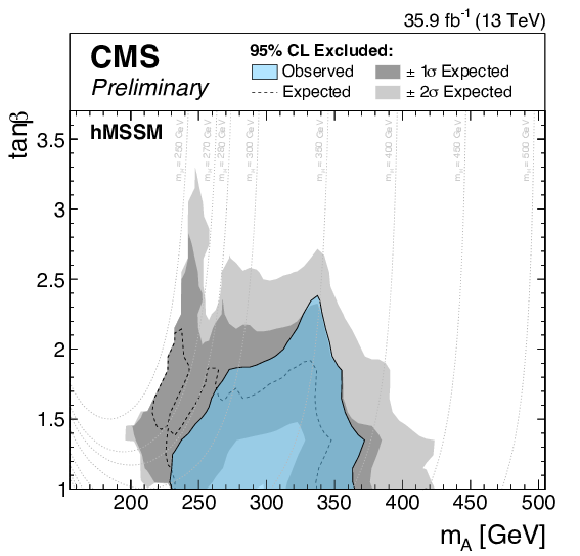
Figure 5:
(a) : observed and expected 95% CL upper limits on cross section times branching fraction as a function of the mass of the resonance mS under the hypothesis that its intrinsic width is negligible with respect to the experimental resolution. (b) : interpretation of the exclusion limit in the context of the hMSSM model, parametrized as a function of the tanβ and mA parameters. In this model, the CP-even lighter scalar is assumed to be the observed 125 GeV Higgs boson and is denoted as h, while the CP-even heavier scalar is denoted as H. The gray dotted lines indicate trajectories in the plane corresponding to the same values of mH. |
png pdf |
Figure 5-a:
Observed and expected 95% CL upper limits on cross section times branching fraction as a function of the mass of the resonance mS under the hypothesis that its intrinsic width is negligible with respect to the experimental resolution. |
png pdf |
Figure 5-b:
Interpretation of the exclusion limit in the context of the hMSSM model, parametrized as a function of the tanβ and mA parameters. In this model, the CP-even lighter scalar is assumed to be the observed 125 GeV Higgs boson and is denoted as h, while the CP-even heavier scalar is denoted as H. The gray dotted lines indicate trajectories in the plane corresponding to the same values of mH. |
| Tables | |
png pdf |
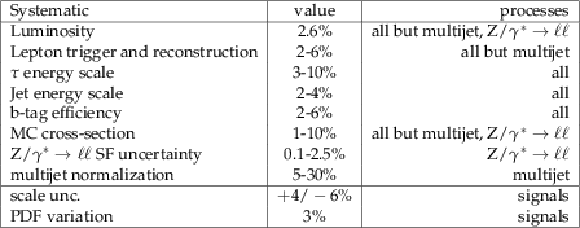
Table 1:
Systematic uncertainties affecting the normalization of the different processes. |
| Summary |
|
The search for resonant and non-resonant Higgs boson (HH) production in the bbττ final state is presented. The search is performed using 35.9 fb−1 of data collected in proton-proton collisions at √s= 13 TeV and uses the three most sensitive decay channels of the τ lepton pair, namely τμτh, τeτh, and τhτh. The results are found to be compatible within the uncertainties to the expected SM background contribution, and upper confidence limits on the HH production cross sections are set. For the non-resonant production mechanism, the theoretical framework of the effective Lagrangian is used to parametrize the cross section as a function of anomalous couplings of the Higgs boson. Upper confidence limits on the HH cross section are derived as a function of kλ=λHHH/λSMHHH and kt=yt/ySMt. The 95% CL observed upper limit corresponds to approximately 28 times the theoretical prediction for the SM cross section, and the expected one is about 25 times the SM prediction. For the resonant production mechanism, upper exclusion limits at 95% CL are derived for the production of a narrow resonance of mass mX ranging from 250 to 900 GeV. These model independent limits are interpreted in the context of MSSM scenarios where the HH production cross section is parametrized as a function of the mA and tanβ parameters of the model. |
| References | ||||
| 1 | ATLAS Collaboration | Observation of a new particle in the search for the Standard Model Higgs boson with the ATLAS detector at the LHC | Physics Letters B 716 (2012), no. 1, 1 -- 29 | |
| 2 | CMS Collaboration | Observation of a new boson at a mass of 125 GeV with the CMS experiment at the LHC | Physics Letters B 716 (2012), no. 1, 30 -- 61 | |
| 3 | ATLAS, CMS Collaboration | Combined Measurement of the Higgs Boson Mass in pp Collisions at √s= 7 and 8 TeV with the ATLAS and CMS Experiments | PRL 114 (2015) 191803 | 1503.07589 |
| 4 | ATLAS, CMS Collaboration | Measurements of the Higgs boson production and decay rates and constraints on its couplings from a combined ATLAS and CMS analysis of the LHC pp collision data at √s= 7 and 8 TeV | JHEP 08 (2016) 045 | 1606.02266 |
| 5 | LHC Higgs Cross Section Working Group Collaboration | Handbook of LHC Higgs Cross Sections: 4. Deciphering the Nature of the Higgs Sector | 1610.07922 | |
| 6 | T. Binoth and J. J. van der Bij | Influence of strongly coupled, hidden scalars on Higgs signals | Z. Phys. C75 (1997) 17--25 | hep-ph/9608245 |
| 7 | R. Schabinger and J. D. Wells | Minimal spontaneously broken hidden sector and its impact on Higgs boson physics at the CERN Large Hadron Collider | PRD 72 (Nov, 2005) 093007 | |
| 8 | B. Patt and F. Wilczek | Higgs-field Portal into Hidden Sectors | hep-ph/0605188 | |
| 9 | G. C. Branco et al. | Theory and phenomenology of two-Higgs-doublet models | PR 516 (2012) 1--102 | 1106.0034 |
| 10 | P. Fayet | Supergauge invariant extension of the Higgs mechanism and a model for the electron and its neutrino | Nuclear Physics B 90 (1975) 104 -- 124 | |
| 11 | P. Fayet | Spontaneously broken supersymmetric theories of weak, electromagnetic and strong interactions | Physics Letters B 69 (1977), no. 4, 489 -- 494 | |
| 12 | K. Agashe, H. Davoudiasl, G. Perez, and A. Soni | Warped gravitons at the CERN LHC and beyond | PRD 76 (Aug, 2007) 036006 | |
| 13 | A. L. Fitzpatrick, J. Kaplan, L. Randall, and L.-T. Wang | Searching for the Kaluza-Klein graviton in bulk RS models | JHEP 09 (2007) 013 | hep-ph/0701150 |
| 14 | F. Goertz, A. Papaefstathiou, L. L. Yang, and J. Zurita | Higgs boson pair production in the D=6 extension of the SM | JHEP 04 (2015) 167 | 1410.3471 |
| 15 | ATLAS Collaboration | Searches for Higgs boson pair production in the hh→bbττ, γγWW∗, γγbb, bbbb channels with the ATLAS detector | PRD 92 (Nov, 2015) 092004 | |
| 16 | ATLAS Collaboration | Search for pair production of Higgs bosons in the bˉbbˉb final state using proton−proton collisions at √s= 13 TeV with the ATLAS detector | Technical Report ATLAS-CONF-2016-049, CERN, Geneva, Aug | |
| 17 | ATLAS Collaboration | Search for Higgs boson pair production in the final state of γγWW∗(→lνjj) using 13.3 fb−1 of pp collision data recorded at √s= 13 TeV with the ATLAS detector | Technical Report ATLAS-CONF-2016-071, CERN, Geneva, Aug | |
| 18 | ATLAS Collaboration | Search for Higgs boson pair production in the bˉbγγ final state using pp collision data at √s= 13 TeV with the ATLAS detector | Technical Report ATLAS-CONF-2016-004, CERN, Geneva, Mar | |
| 19 | CMS Collaboration | Search for two Higgs bosons in final states containing two photons and two bottom quarks in proton-proton collisions at 8 TeV | PRD 94 (Sep, 2016) 052012 | |
| 20 | CMS Collaboration | Search for resonant pair production of Higgs bosons decaying to two bottom quark-antiquark pairs in proton-proton collisions at 8 TeV | PLB749 (2015) 560--582 | CMS-HIG-14-013 1503.04114 |
| 21 | CMS Collaboration | Search for non-resonant pair production of Higgs bosons in the bˉbbˉb final state with 13 TeV CMS data | CMS-PAS-HIG-16-026 | CMS-PAS-HIG-16-026 |
| 22 | CMS Collaboration | Search for H(bb)H(gammagamma) decays at 13TeV | CMS-PAS-HIG-16-032 | CMS-PAS-HIG-16-032 |
| 23 | CMS Collaboration | Search for Higgs boson pair production in the b¯blνlν final state at √s= 13 TeV | CMS-PAS-HIG-16-024 | CMS-PAS-HIG-16-024 |
| 24 | CMS Collaboration | Search for non-resonant Higgs boson pair production in the b¯bτ+τ− final state | ||
| 25 | CMS Collaboration | Search for resonant Higgs boson pair production in the b¯bτ+τ− final state | ||
| 26 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 27 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | CMS-00-001 |
| 28 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | Journal of High Energy Physics 2014 (2014), no. 7, 79 | |
| 29 | A. Carvalho et al. | Higgs Pair Production: Choosing Benchmarks With Cluster Analysis | JHEP 04 (2016) 126 | 1507.02245 |
| 30 | A. Carvalho et al. | Analytical parametrization and shape classification of anomalous HH production in the EFT approach | 1608.06578 | |
| 31 | E. Re | Single-top Wt-channel production matched with parton showers using the POWHEG method | EPJC71 (2011) 1547 | 1009.2450 |
| 32 | J. M. Campbell, R. K. Ellis, P. Nason, and E. Re | Top-pair production and decay at NLO matched with parton showers | Journal of High Energy Physics 2015 (2015), no. 4, 114 | |
| 33 | NNPDF Collaboration | Parton distributions for the LHC Run II | JHEP 04 (2015) 040 | 1410.8849 |
| 34 | CMS Collaboration | Particle-Flow Event Reconstruction in CMS and Performance for Jets, Taus, and MET | CDS | |
| 35 | CMS Collaboration | Commissioning of the particle-flow event reconstruction with the first LHC collisions recorded in the CMS detector | CDS | |
| 36 | CMS Collaboration | Determination of jet energy calibration and transverse momentum resolution in CMS | JINST 6 (2011) P11002 | CMS-JME-10-011 1107.4277 |
| 37 | CMS Collaboration | Performance of electron reconstruction and selection with the CMS detector in proton-proton collisions at √s= 8 TeV | JINST 10 (2015), no. 06, P06005 | CMS-EGM-13-001 1502.02701 |
| 38 | CMS Collaboration | Performance of CMS muon reconstruction in pp collision events at √s= 7 TeV | JINST 7 (2012) P10002 | CMS-MUO-10-004 1206.4071 |
| 39 | CMS Collaboration | Reconstruction and identification of τ lepton decays to hadrons and ντ at CMS | JINST 11 (2016), no. 01, P01019 | CMS-TAU-14-001 1510.07488 |
| 40 | J. M. Butterworth, A. R. Davison, M. Rubin, and G. P. Salam | Jet substructure as a new Higgs search channel at the LHC | PRL 100 (2008) 242001 | 0802.2470 |
| 41 | CMS Collaboration | Identification of b-quark jets with the CMS experiment | JINST 8 (2013) P04013 | CMS-BTV-12-001 1211.4462 |
| 42 | L. Bianchini, J. Conway, E. K. Friis, and C. Veelken | Reconstruction of the Higgs mass in H→ττ Events by Dynamical Likelihood techniques | J. Phys. Conf. Ser. 513 (2014) 022035 | |
| 43 | A. J. Larkoski, S. Marzani, G. Soyez, and J. Thaler | Soft drop | Journal of High Energy Physics 2014 (2014), no. 5, 146 | |
| 44 | A. Hocker et al. | TMVA - Toolkit for Multivariate Data Analysis | PoS ACAT (2007) 040 | physics/0703039 |
| 45 | J. H. Friedman | Greedy function approximation: A gradient boosting machine. | Ann. Statist. 29 (10, 2001) 1189--1232 | |
| 46 | CMS Collaboration | Searches for a heavy scalar boson H decaying to a pair of 125 GeV Higgs bosons hh or for a heavy pseudoscalar boson A decaying to Zh, in the final states with h→ττ | PLB755 (2016) 217--244 | CMS-HIG-14-034 1510.01181 |
| 47 | C. G. Lester and D. J. Summers | Measuring masses of semiinvisibly decaying particles pair produced at hadron colliders | PLB463 (1999) 99--103 | hep-ph/9906349 |
| 48 | A. Barr, C. Lester, and P. Stephens | m(T2): The Truth behind the glamour | JPG29 (2003) 2343--2363 | hep-ph/0304226 |
| 49 | A. J. Barr, M. J. Dolan, C. Englert, and M. Spannowsky | Di-Higgs final states augMT2ed -- selecting hh events at the high luminosity LHC | PLB728 (2014) 308--313 | 1309.6318 |
| 50 | C. G. Lester and B. Nachman | Bisection-based asymmetric MT2 computation: a higher precision calculator than existing symmetric methods | Journal of High Energy Physics 2015 (2015), no. 3, 100 | |
| 51 | A. Djouadi et al. | The post-Higgs MSSM scenario: Habemus MSSM? | EPJC73 (2013) 2650 | 1307.5205 |
| 52 | A. Djouadi et al. | Fully covering the MSSM Higgs sector at the LHC | JHEP 06 (2015) 168 | 1502.05653 |

|
Compact Muon Solenoid LHC, CERN |

|

|

|

|

|

|