Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-PAS-SMP-19-003 | ||
| Measurement of the dependence of inclusive jet production cross sections on the anti-$k_{\mathrm{T}}$ distance parameter in proton-proton collisions at $\sqrt{\text{s}}$ = 13 TeV | ||
| CMS Collaboration | ||
| July 2019 | ||
| Abstract: The dependence of inclusive jet production cross sections on the anti-$k_{\mathrm{T}}$ distance parameter in proton-proton collisions with a center-of-mass energy of 13 TeV is studied using 35.9 fb$^{-1}$ data collected by the CMS experiment. The ratio of the inclusive cross sections as a function of transverse momentum $p_{\mathrm{T}}$ and rapidity $y$ for distance parameters ranging from 0.1 to 1.2 to that using a distance parameter of 0.4 is presented in the region 84 $ < p_{\mathrm{T}} < $ 1588 GeV and $|y| < $ 2.0. The results are compared to calculations at leading order and next-to-leading order in the strong coupling constant using different parton shower models. The shape of the variation of ratio of cross sections with distance parameter is described well by calculations including a parton shower model, but not by a pure leading order QCD calculation including only nonperturbative effects. The descriptions of the ratios of cross sections are significantly improved when both next-to-leading order QCD calculations and nonperturbative effects are included. | ||
|
Links:
CDS record (PDF) ;
CADI line (restricted) ;
These preliminary results are superseded in this paper, JHEP 12 (2020) 082. The superseded preliminary plots can be found here. |
||
| Figures | |
png pdf |
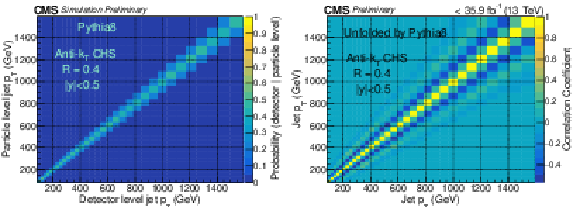
Figure 1:
Response matrix for AK4 jets constructed using detector simulation based on a PYTHIA8 Monte Carlo sample (left). Correlation matrix after data is unfolded by the D'Agostini technique using PYTHIA8 simulation for AK4 jets (right) in the rapidity bin $|y| < $ 0.5. |
png pdf |
Figure 1-a:
Response matrix for AK4 jets constructed using detector simulation based on a PYTHIA8 Monte Carlo sample. |
png pdf |
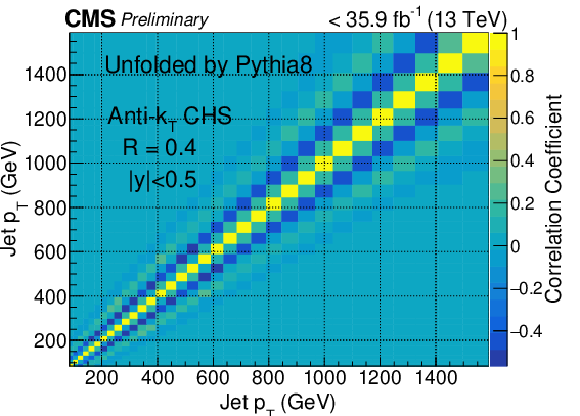
Figure 1-b:
Correlation matrix after data is unfolded by the D'Agostini technique using PYTHIA8 simulation for AK4 jets in the rapidity bin $|y| < $ 0.5. |
png pdf |
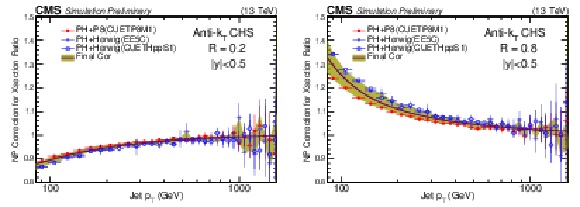
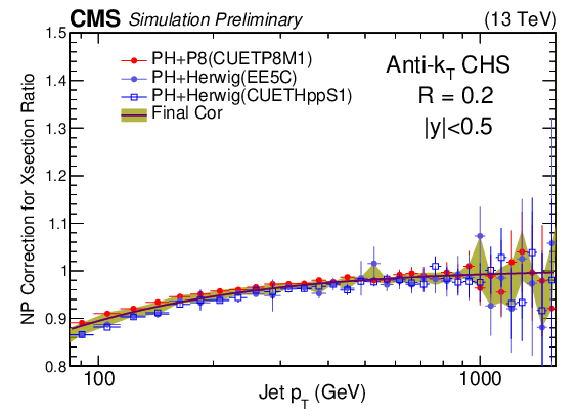
Figure 2:
Nonperturbative correction for the cross section ratio of inclusive AK2 (left) and AK8 jets (right) with respect to AK4 jets in the rapidity bin $|y| < $ 0.5. |
png pdf |
Figure 2-a:
Nonperturbative correction for the cross section ratio of inclusive AK2 jets with respect to AK4 jets in the rapidity bin $|y| < $ 0.5. |
png pdf |
Figure 2-b:
Nonperturbative correction for the cross section ratio of inclusive AK8 jets with respect to AK4 jets in the rapidity bin $|y| < $ 0.5. |
png pdf |
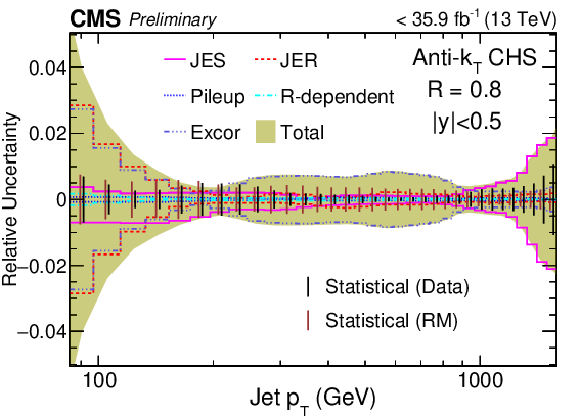
Figure 3:
Total uncertainty (relative) from experimental systematics for ratio of cross section of inclusive jets of size 0.2 (left), 0.8 (right) with respect to that of AK4 jet in the rapidity bin $|y| < $ 0.5. Statistical errors are also overlaid as black lines for data and as dark red lines for response matrices (RM) in simulation. |
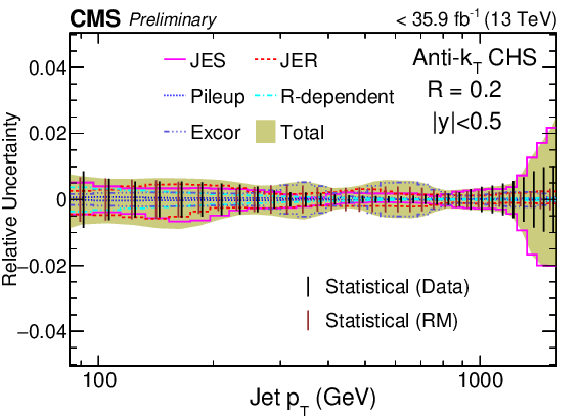
png pdf |
Figure 3-a:
Total uncertainty (relative) from experimental systematics for ratio of cross section of inclusive jets of size 0.2 with respect to that of AK4 jet in the rapidity bin $|y| < $ 0.5. Statistical errors are also overlaid as black lines for data and as dark red lines for response matrices (RM) in simulation. |
png pdf |
Figure 3-b:
Total uncertainty (relative) from experimental systematics for ratio of cross section of inclusive jets of size 0.8 with respect to that of AK4 jet in the rapidity bin $|y| < $ 0.5. Statistical errors are also overlaid as black lines for data and as dark red lines for response matrices (RM) in simulation. |
png pdf |
Figure 4:
Comparison of ratio of differential cross section of jets of different sizes with respect to that of AK4 jets from data and from NLO predictions using POWHEG + PYTHIA8 (CUETP8M1 tune) in the region $|\text {y}| < $ 0.5. Colored symbols indicate data and colored lines represent prediction from MC. Numbers written in the parentheses have been added to the corresponding data points to separate the results for different jet sizes. |
png pdf |
Figure 5:
Comparison of ratio of differential cross section of AK2 (top) and AK8 (bottom) jets with respect to that of AK4 jets from data and pQCD predictions using NLOJET++ in the region $|\text {y}| < $ 0.5. Black symbols indicate data and colored lines represent pQCD predictions. Statistical error bars are shown for data and NLO prediction with nonperturbative (NP) correction; yellowish olive region around data represents experimental systematic uncertainty where as region shaded in light blue color around NLO$\otimes $NP prediction shows the theory uncertainty in the prediction. |
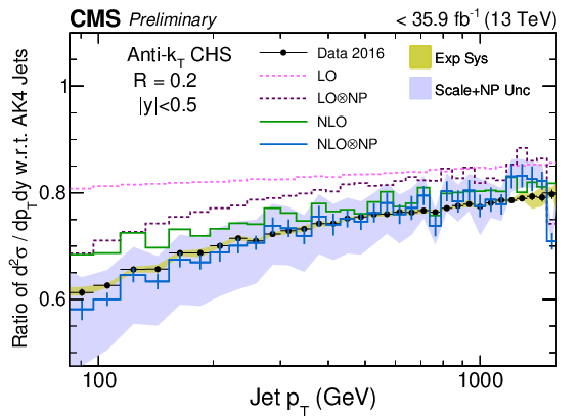
png pdf |
Figure 5-a:
Comparison of ratio of differential cross section of AK2 jets with respect to that of AK4 jets from data and pQCD predictions using NLOJET++ in the region $|\text {y}| < $ 0.5. Black symbols indicate data and colored lines represent pQCD predictions. Statistical error bars are shown for data and NLO prediction with nonperturbative (NP) correction; yellowish olive region around data represents experimental systematic uncertainty where as region shaded in light blue color around NLO$\otimes $NP prediction shows the theory uncertainty in the prediction. |
png pdf |
Figure 5-b:
Comparison of ratio of differential cross section of AK8 jets with respect to that of AK4 jets from data and pQCD predictions using NLOJET++ in the region $|\text {y}| < $ 0.5. Black symbols indicate data and colored lines represent pQCD predictions. Statistical error bars are shown for data and NLO prediction with nonperturbative (NP) correction; yellowish olive region around data represents experimental systematic uncertainty where as region shaded in light blue color around NLO$\otimes $NP prediction shows the theory uncertainty in the prediction. |
png pdf |
Figure 6:
Comparison of the ratio of cross sections of inclusive jets of various sizes with respect to AK4 jets as a function of jet size in different regions of jet ${p_{\mathrm {T}}}$ from data and from several theory predictions in rapidity bin $|y| < $ 0.5 (upper row) and 1.5 $ < |y| < $ 2.0 (lower row) at particle level. When the cross section ratio is taken between fixed NLO predictions for two jet sizes, the ratio becomes LO at ${\alpha _S}$, this is quoted as LO$\otimes $NP in the figure. Experimental uncertainties on the ratio of cross sections are shown in bands around data points, theory uncertainties are shown in bands around fixed order predictions respectively. |
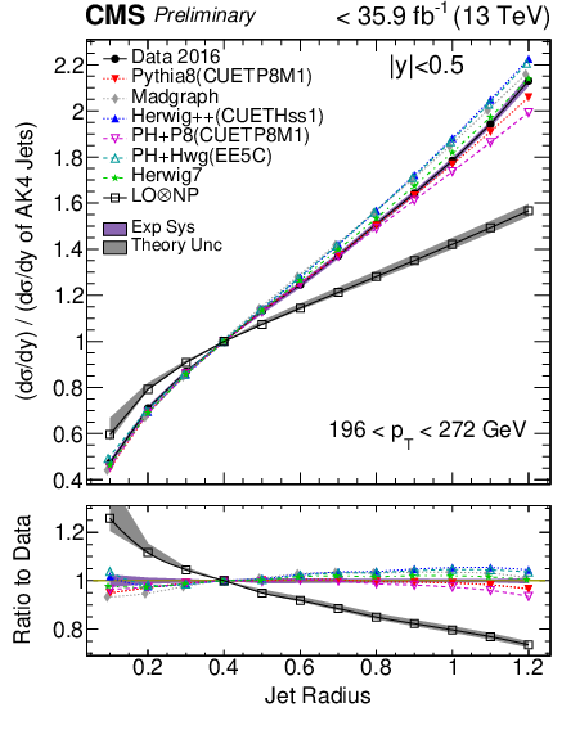
png pdf |
Figure 6-a:
Comparison of the ratio of cross sections of inclusive jets of various sizes with respect to AK4 jets as a function of jet size for jet 196 $< {p_{\mathrm {T}}} <$ 272 GeV from data and from several theory predictions in rapidity bin $|y| < $ 0.5 at particle level. When the cross section ratio is taken between fixed NLO predictions for two jet sizes, the ratio becomes LO at ${\alpha _S}$, this is quoted as LO$\otimes $NP in the figure. Experimental uncertainties on the ratio of cross sections are shown in bands around data points, theory uncertainties are shown in bands around fixed order predictions respectively. |
png pdf |
Figure 6-b:
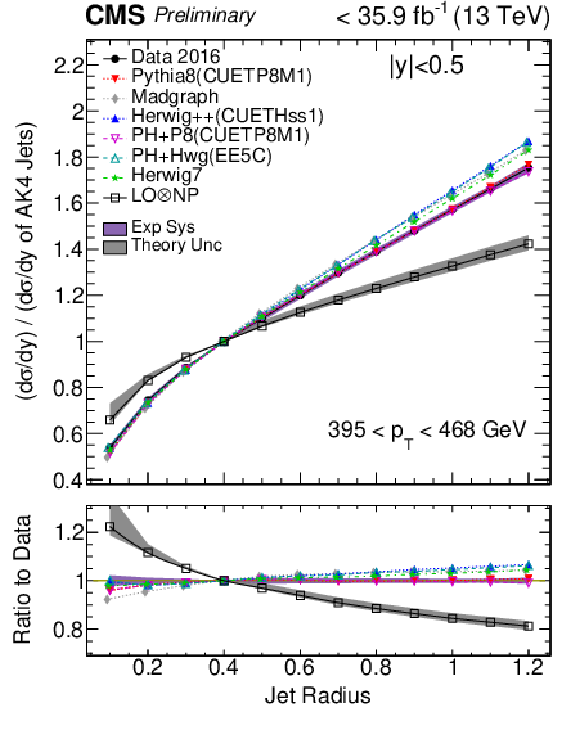
Comparison of the ratio of cross sections of inclusive jets of various sizes with respect to AK4 jets as a function of jet size for jet 395 $< {p_{\mathrm {T}}} <$ 468 GeV from data and from several theory predictions in rapidity bin $|y| < $ 0.5 at particle level. When the cross section ratio is taken between fixed NLO predictions for two jet sizes, the ratio becomes LO at ${\alpha _S}$, this is quoted as LO$\otimes $NP in the figure. Experimental uncertainties on the ratio of cross sections are shown in bands around data points, theory uncertainties are shown in bands around fixed order predictions respectively. |
png pdf |
Figure 6-c:
Comparison of the ratio of cross sections of inclusive jets of various sizes with respect to AK4 jets as a function of jet size for jet 548 $< {p_{\mathrm {T}}} <$ 638 GeV from data and from several theory predictions in rapidity bin $|y| < $ 0.5 at particle level. When the cross section ratio is taken between fixed NLO predictions for two jet sizes, the ratio becomes LO at ${\alpha _S}$, this is quoted as LO$\otimes $NP in the figure. Experimental uncertainties on the ratio of cross sections are shown in bands around data points, theory uncertainties are shown in bands around fixed order predictions respectively. |
png pdf |
Figure 6-d:
Comparison of the ratio of cross sections of inclusive jets of various sizes with respect to AK4 jets as a function of jet size for jet 196 $< {p_{\mathrm {T}}} <$ 272 GeV from data and from several theory predictions in rapidity bin 1.5 $ < |y| < $ 2.0 at particle level. When the cross section ratio is taken between fixed NLO predictions for two jet sizes, the ratio becomes LO at ${\alpha _S}$, this is quoted as LO$\otimes $NP in the figure. Experimental uncertainties on the ratio of cross sections are shown in bands around data points, theory uncertainties are shown in bands around fixed order predictions respectively. |
png pdf |
Figure 6-e:
Comparison of the ratio of cross sections of inclusive jets of various sizes with respect to AK4 jets as a function of jet size for jet 395 $< {p_{\mathrm {T}}} <$ 468 GeV from data and from several theory predictions in rapidity bin 1.5 $ < |y| < $ 2.0 at particle level. When the cross section ratio is taken between fixed NLO predictions for two jet sizes, the ratio becomes LO at ${\alpha _S}$, this is quoted as LO$\otimes $NP in the figure. Experimental uncertainties on the ratio of cross sections are shown in bands around data points, theory uncertainties are shown in bands around fixed order predictions respectively. |
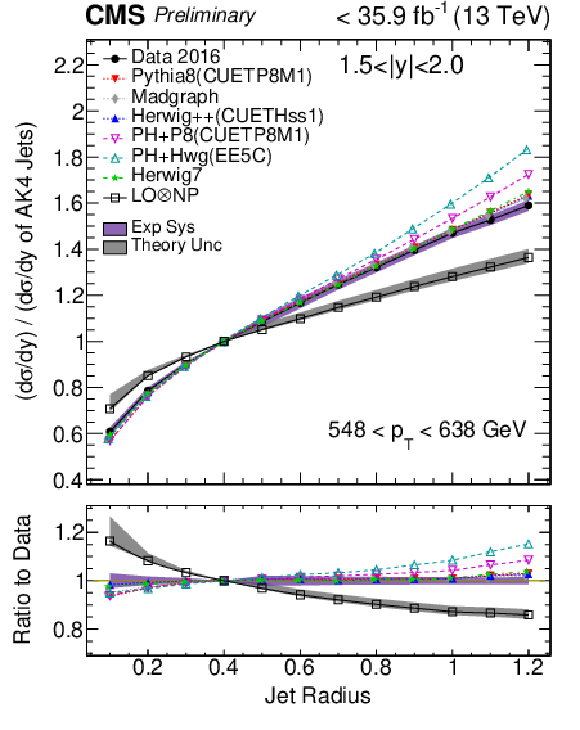
png pdf |
Figure 6-f:
Comparison of the ratio of cross sections of inclusive jets of various sizes with respect to AK4 jets as a function of jet size for jet 548 $< {p_{\mathrm {T}}} <$ 638 GeV from data and from several theory predictions in rapidity bin 1.5 $ < |y| < $ 2.0 at particle level. When the cross section ratio is taken between fixed NLO predictions for two jet sizes, the ratio becomes LO at ${\alpha _S}$, this is quoted as LO$\otimes $NP in the figure. Experimental uncertainties on the ratio of cross sections are shown in bands around data points, theory uncertainties are shown in bands around fixed order predictions respectively. |
| Tables | |
png pdf |
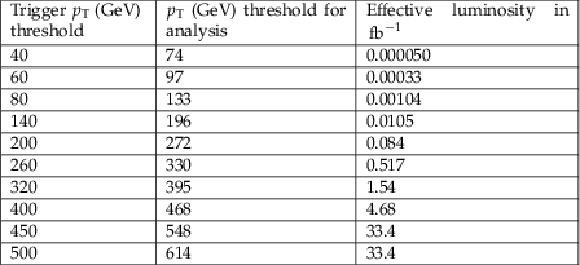
Table 1:
Trigger ${p_{\mathrm {T}}}$ thresholds and effective luminosity of the HLT triggers for AK8 jets. |
| Summary |
|
This paper reports a measurement of the ratio of cross section of inclusive jets of multiple sizes w.r.t. AK4 jets for the first time in the CMS experiment. Due to cancellation of many experimental and theoretical systematic uncertainties for the ratio, it is more sensitive to perturbative and nonperturbative effects than the absolute cross section measurement; the experimental systematic uncertainty for cross section ratio is of similar size as the statistical uncertainty, and theory uncertainty is dominated by the choice of renormalization and factorization scales. From the ratio measurement, it is observed that the NP correction is important to describe the data at low ${p_{\mathrm{T}}}$. So the modelling of nonperturbative effects, like hadronization and underlying events, and also of perturbative radiation have significant impact to describe the data in different regions of phase-space. Finally, the variation of the ratio of cross sections with $R$ emphasizes the importance of parton showers to capture the effects of higher order terms in the perturbation series by resummation approach, which is absent in the case of fixed order computation. Therefore this study shows the importance of final-state radiation modelled in Monte Carlo simulation to describe the data, and also points that differences between different parton shower and hadronization models are significant. |
| References | ||||
| 1 | G. Sterman and S. Weinberg | Jets from quantum chromodynamics | PRL 39 (1977) 1436 | |
| 2 | T. Sjostrand | A model for initial state parton showers | PLB 157 (1985) 321 | |
| 3 | T. D. Gottschalk | Backwards evolved initial state parton showers | NPB 277 (1986) 700 | |
| 4 | G. Marchesini and B. R. Webber | Simulation of QCD jets including soft gluon interference | NPB 238 (1984) 1 | |
| 5 | Y. L. Dokshitzer and B. R. Webber | Calculation of power corrections to hadronic event shapes | PLB 352 (1995) 451 | hep-ph/9504219 |
| 6 | CDF Collaboration | Charged jet evolution and the underlying event in proton-antiproton collisions at 1.8 TeV | PRD 65 (2002) 092002 | |
| 7 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_T $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 8 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | CMS-00-001 |
| 9 | CMS Collaboration | Measurement of the inclusive jet cross section in $ pp $ collisions at $ \sqrt{s}= $ 7 TeV | PRL 107 (2011) 132001 | CMS-QCD-10-011 1106.0208 |
| 10 | CMS Collaboration | Measurement and QCD analysis of double-differential inclusive jet cross sections in pp collisions at $ \sqrt{s}= $ 8 TeV and cross section ratios to 2.76 and 7 TeV | JHEP 03 (2017) 156 | CMS-SMP-14-001 1609.05331 |
| 11 | CMS Collaboration | Measurement of the double-differential inclusive jet cross section in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | EPJC 76 (2016) 451 | CMS-SMP-15-007 1605.04436 |
| 12 | Z. Nagy | Next-to-leading order calculation of three jet observables in hadron hadron collision | PRD 68 (2003) 094002 | hep-ph/0307268 |
| 13 | T. Kluge, K. Rabbertz, and M. Wobisch | FastNLO: Fast pQCD calculations for PDF fits | in Deep inelastic scattering. Proceedings, 14th International Workshop, DIS 2006, Tsukuba, Japan, April 20-24, 2006, p. 483 2006 | hep-ph/0609285 |
| 14 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with parton shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 15 | ATLAS Collaboration | Measurement of the inclusive jet cross sections in proton-proton collisions at $ \sqrt{s}= $ 8 TeV with the ATLAS detector | JHEP 09 (2017) 020 | 1706.03192 |
| 16 | ATLAS Collaboration | Measurement of inclusive jet and dijet cross sections in proton-proton collisions at $ \sqrt{s}= $ 13 TeV with the ATLAS detector | JHEP 05 (2018) 195 | 1711.02692 |
| 17 | ALICE Collaboration | Measurement of the inclusive differential jet cross section in $ pp $ collisions at $ \sqrt{s} = $ 2.76 TeV | PLB 722 (2013) 262 | 1301.3475 |
| 18 | CMS Collaboration | Measurement of the ratio of inclusive jet cross sections using the anti-$ k_T $ algorithm with radius parameters R=0.5 and 0.7 in pp collisions at $ \sqrt{s}= $ 7 TeV | PRD 90 (2014) 072006 | CMS-SMP-13-002 1406.0324 |
| 19 | F. Aversa, P. Chiappetta, M. Greco, and J. P. Guillet | QCD corrections to parton-parton scattering processes | NPB 327 (1989) 105 | |
| 20 | D. de Florian and W. Vogelsang | Resummed cross section for jet production at hadron colliders | PRD 76 (2007) 074031 | 0704.1677 |
| 21 | M. Dasgupta, L. Magnea, and G. P. Salam | Nonperturbative QCD effects in jets at hadron colliders | JHEP 02 (2008) 055 | 0712.3014 |
| 22 | M. Beneke | Renormalons | PR 317 (1999) 1 | hep-ph/9807443 |
| 23 | M. Cacciari, G. P. Salam, and G. Soyez | The catchment area of jets | JHEP 04 (2008) 005 | 0802.1188 |
| 24 | Z. Nagy | Three jet cross sections in hadron hadron collisions at next-to-leading order | PRL 88 (2002) 122003 | hep-ph/0110315 |
| 25 | G. Soyez | A Simple description of jet cross section ratios | PLB 698 (2011) 59 | 1101.2665 |
| 26 | CMS Collaboration | Performance of photon reconstruction and identification with the CMS detector in proton-proton collisions at $ \sqrt{s} = $ 8 TeV | JINST 10 (2015) P08010 | CMS-EGM-14-001 1502.02702 |
| 27 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 28 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 29 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 30 | CMS Collaboration | Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV | JINST 12 (2017) P02014 | CMS-JME-13-004 1607.03663 |
| 31 | T. Sjöstrand et al. | An introduction to PYTHIA 8.2 | CPC 191 (2015) 159 | 1410.3012 |
| 32 | NNPDF Collaboration | A determination of parton distributions with faithful uncertainty estimation | NPB 809 (2009) 1 | 0808.1231 |
| 33 | CMS Collaboration | Event generator tunes obtained from underlying event and multiparton scattering measurements | EPJC 76 (2016) 155 | CMS-GEN-14-001 1512.00815 |
| 34 | P. Skands, S. Carrazza, and J. Rojo | Tuning PYTHIA 8.1: the Monash 2013 tune | EPJC 74 (2014) 3024 | 1404.5630 |
| 35 | M. Bahr et al. | Herwig++ physics and manual | EPJC 58 (2008) 639 | 0803.0883 |
| 36 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 37 | J. Alwall et al. | Comparative study of various algorithms for the merging of parton showers and matrix elements in hadronic collisions | EPJC 53 (2008) 473 | 0706.2569 |
| 38 | J. Bellm et al. | Herwig 7.0/Herwig++ 3.0 release note | EPJC 76 (2016) 196 | 1512.01178 |
| 39 | R. Frederix and S. Frixione | Merging meets matching in MC@NLO | JHEP 12 (2012) 061 | 1209.6215 |
| 40 | T. Adye | Unfolding algorithms and tests using RooUnfold | in Proceedings, PHYSTAT 2011 Workshop on Statistical Issues Related to Discovery Claims in Search Experiments and Unfolding, CERN 2011 | 1105.1160 |
| 41 | G. D'Agostini | A Multidimensional unfolding method based on Bayes' theorem | NIMA 362 (1995) 487 | |
| 42 | A. Hocker and V. Kartvelishvili | SVD approach to data unfolding | NIMA 372 (1996) 469 | hep-ph/9509307 |
| 43 | S. Schmitt | Data unfolding methods in high energy physics | EPJ Web Conf. 137 (2017) 11008 | 1611.01927 |
| 44 | S. Schmitt | TUnfold: an algorithm for correcting migration effects in high energy physics | JINST 7 (2012) T10003 | 1205.6201 |
| 45 | M. H. Seymour and A. Siodmok | Constraining MPI models using $ \sigma_{eff} $ and recent Tevatron and LHC Underlying Event data | JHEP 10 (2013) 113 | 1307.5015 |
| 46 | B. Efron and R. Tibshirani | An Introduction to the Bootstrap | Monographs on statistics and applied probabilities. Chapman \& Hall/CRC, 1993 ISBN 9780412042317 | |
| 47 | CMS Collaboration | Jet energy scale and resolution performance with 13 TeV data collected by CMS in 2016 | CDS | |
| 48 | CMS Collaboration | Measurement of the inelastic proton-proton cross section at $ \sqrt{s}= $ 13 TeV | JHEP 07 (2018) 161 | CMS-FSQ-15-005 1802.02613 |
| 49 | S. Dulat et al. | New parton distribution functions from a global analysis of quantum chromodynamics | PRD 93 (2016) 033006 | 1506.07443 |
| 50 | L. A. Harland-Lang, A. D. Martin, P. Motylinski, and R. S. Thorne | Parton distributions in the LHC era: MMHT 2014 PDFs | EPJC 75 (2015) 204 | 1412.3989 |
| 51 | J. Butterworth et al. | PDF4LHC recommendations for LHC Run II | JPG 43 (2016) 023001 | 1510.03865 |
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|