

Compact Muon Solenoid
LHC, CERN
| CMS-PAS-EXO-19-013 | ||
| Search for long-lived particles decaying to jets with displaced vertices | ||
| CMS Collaboration | ||
| September 2020 | ||
| Abstract: We report the results of a search for long-lived particles produced in pairs in proton-proton collisions at the LHC operating at a center-of-mass energy of $\sqrt{s}= $ 13 TeV. The data were collected by the CMS detector during the full Run 2 data taking period from 2015 through 2018, corresponding to a total integrated luminosity of 140 fb$^{-1}$. This search targets long-lived particles with a mean proper decay length between 0.1 and 100 mm that each decay into at least two quarks. The signature is a pair of displaced vertices each formed from many tracks. This search extends a previous CMS search using the 2015 and 2016 dataset, with improvements in background rejection, background estimation techniques, as well as uncertainty estimation. By requiring two reconstructed vertices inside the beam pipe, this search has particularly high sensitivity to decay lengths below 20 mm, which complements similar long-lived search strategies that are less sensitive to such short decay lengths. Results are compared with $R$-parity violating supersymmetry models that predict pair-produced long-lived particles, each decaying into multijet or dijet final states. No events are observed with two reconstructed high-track-multiplicity vertices. For models of long-lived pair-produced neutralinos, gluinos, and top squarks, pair-production cross sections larger than 0.08 fb are excluded at 95% confidence level for masses between 800 and 3000 GeV and mean proper decay lengths between 1 and 25 mm. | ||
|
Links:
CDS record (PDF) ;
CADI line (restricted) ;
These preliminary results are superseded in this paper, Submitted to PRD. The superseded preliminary plots can be found here. |
||
| Figures | |

png pdf |
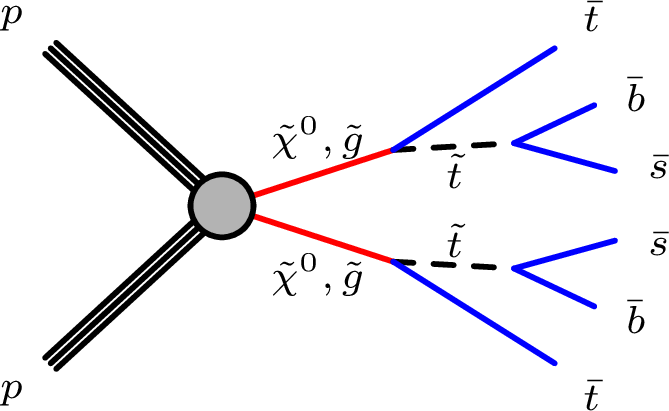
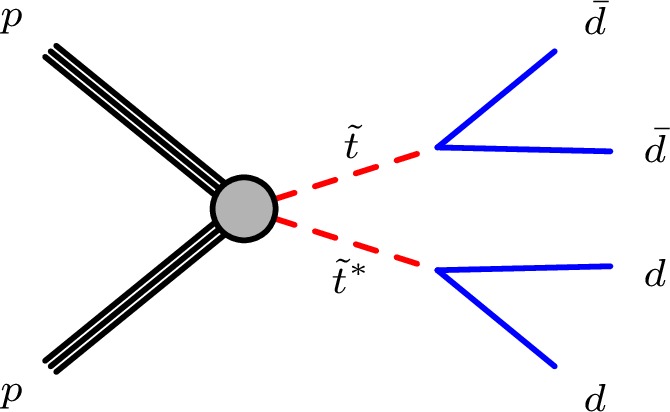
Figure 1:
Diagrams of the multijet signal model (left) showing a long-lived neutralino ($\tilde{\chi}^{0}$) or gluino (${\mathrm{\widetilde{g}}}$) decaying into top, bottom, and strange antiquarks via a virtual top squark ($\tilde{\mathrm{t}}$), and the dijet signal model (right) showing a long-lived top squark decaying into two down-type quarks. In both cases, the long-lived particle is the LSP in their respective models. |
png pdf |
Figure 1-a:
Diagram of the multijet signal model showing a long-lived neutralino ($\tilde{\chi}^{0}$) or gluino (${\mathrm{\widetilde{g}}}$) decaying into top, bottom, and strange antiquarks via a virtual top squark ($\tilde{\mathrm{t}}$). The long-lived particle is the LSP in this model. |
png pdf |
Figure 1-b:
Diagram of the dijet signal model showing a long-lived top squark decaying into two down-type quarks. The long-lived particle is the LSP in this model. |
png pdf |
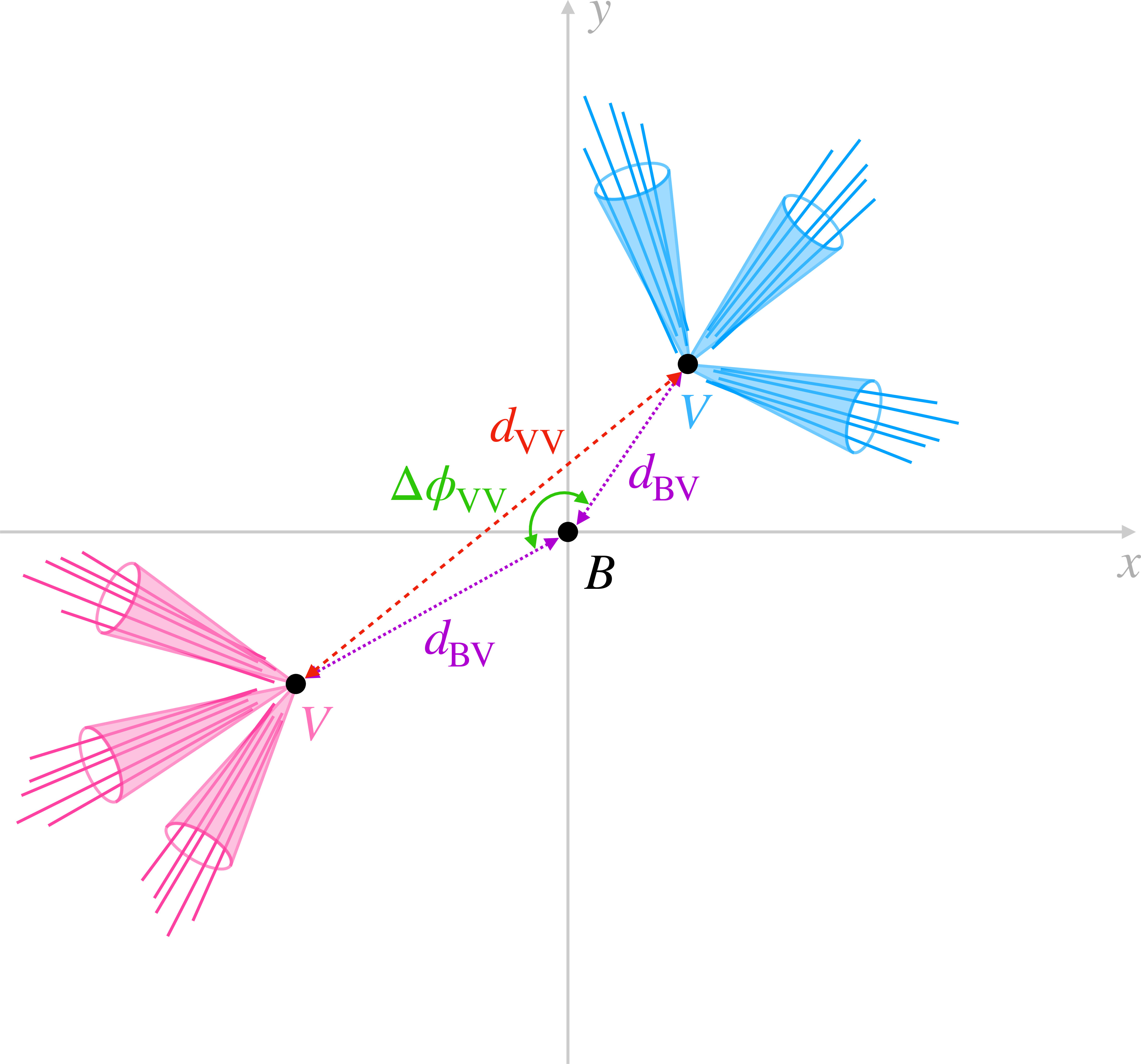
Figure 2:
Cartoon diagram of an event with two signal vertices with the beamspot $B$ at the origin. The beam direction is perpendicular to the $x$-$y$ plane shown. The distance between the vertices is defined as ${d_{\mathrm {VV}}}$. The distance from the beamspot to the vertices is defined as ${d_{\mathrm {BV}}}$ and the angle between the vertices with respect to the beamspot is defined as ${\Delta \phi _{\mathrm {VV}}}$. |
png pdf |
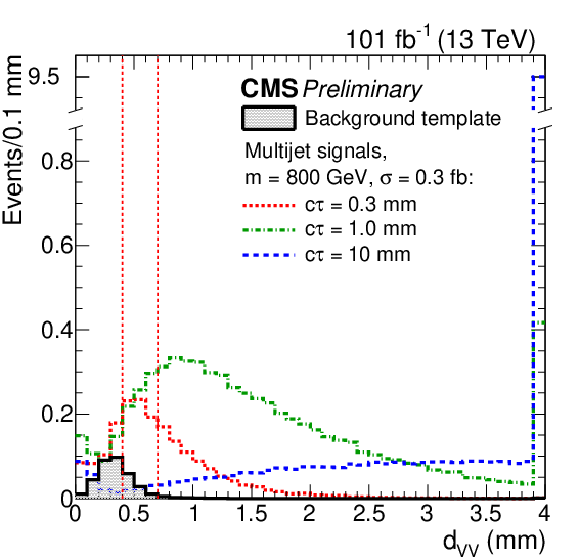
Figure 3:
The distribution of distances between vertices in the $x$-$y$ plane, ${d_{\mathrm {VV}}}$, for simulated multijet signals with mass of 800 GeV, production cross section of 0.3 fb, and $c\tau = $ 0.3, 1.0, and 10 mm with the background template distribution overlaid. The last bin includes the overflow events. The two vertical red dashed lines delineate the boundaries of the bins used in the fit. |

png pdf |
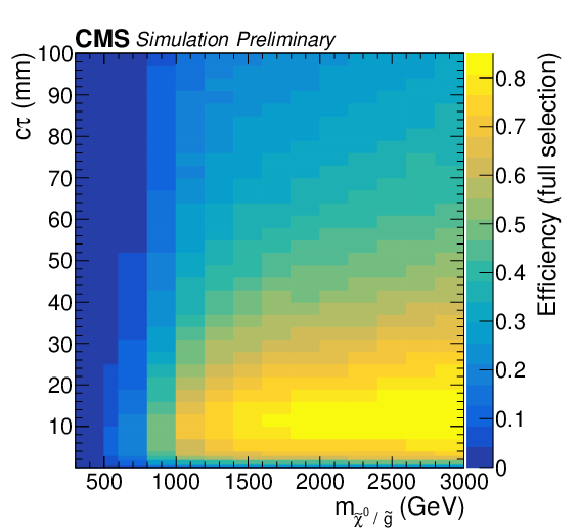
Figure 4:
Multijet (left) and dijet (right) signal efficiencies as a function of the signal mass and lifetime for events satisfying all event and vertex requirements with corrections based on systematic differences in the vertex reconstruction efficiency between data and simulation. |
png pdf |
Figure 4-a:
Multijet signal efficiency as a function of the signal mass and lifetime for events satisfying all event and vertex requirements with corrections based on systematic differences in the vertex reconstruction efficiency between data and simulation. |

png pdf |
Figure 4-b:
Dijet signal efficiency as a function of the signal mass and lifetime for events satisfying all event and vertex requirements with corrections based on systematic differences in the vertex reconstruction efficiency between data and simulation. |
png pdf |
Figure 5:
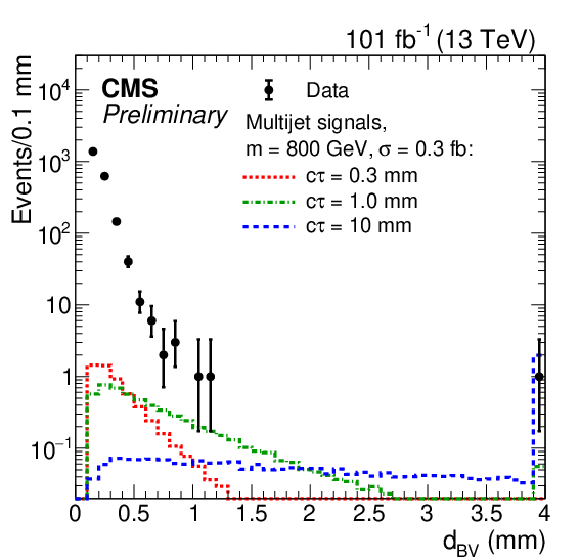
The distribution of ${d_{\mathrm {BV}}}$ for $\geq $5-track one-vertex events in data and simulated multijet signal samples with mass of 800 GeV, production cross section of 0.3 fb, and $c\tau = $ 0.3, 1.0, and 10 mm. The last bin includes the overflow events. |
png pdf |
Figure 6:
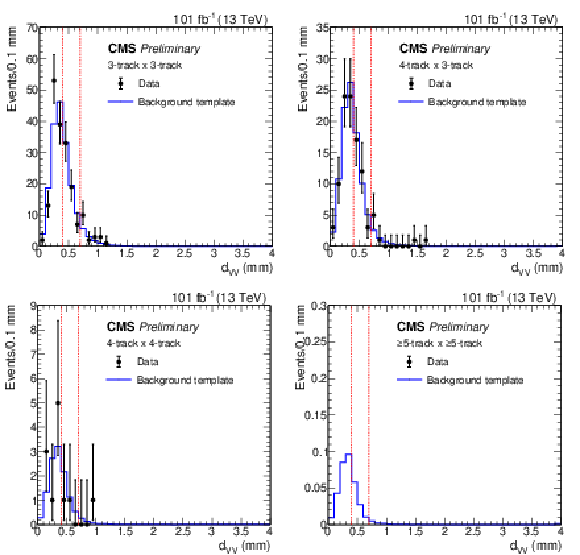
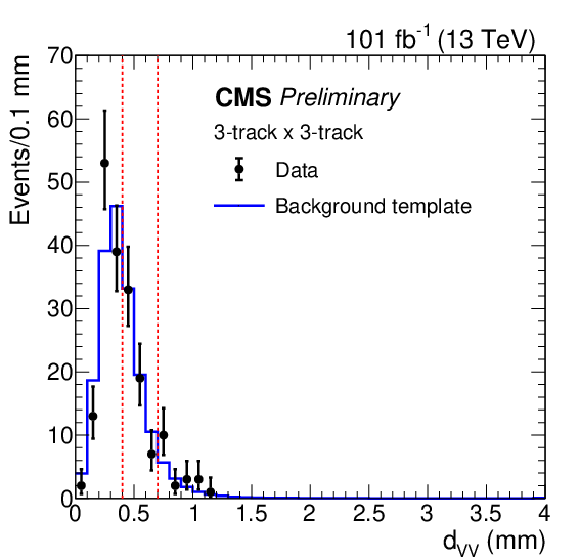
Distribution of the $x$-$y$ distances between vertices, ${d_{\mathrm {VV}}}$, for 2017 and 2018 data, overlaid on the background template ${d_{\mathrm {VV}}^{\,\mathrm {C}}}$ constructed from one-vertex events in data normalized to the two-vertex data for events with 3-track vertices (top left), events with exactly one 4-track vertex and one 3-track vertex (top right), and events with 4-track vertices (bottom left). The background template ${d_{\mathrm {VV}}^{\,\mathrm {C}}}$ for $\geq $5-track two-vertex events (bottom right) is normalized using one-vertex event information as described in the text. The two vertical red dashed lines delineate the three ${d_{\mathrm {VV}}}$ bins. |
png pdf |
Figure 6-a:
Distribution of the $x$-$y$ distances between vertices, ${d_{\mathrm {VV}}}$, for 2017 and 2018 data, overlaid on the background template ${d_{\mathrm {VV}}^{\,\mathrm {C}}}$ constructed from one-vertex events in data normalized to the two-vertex data for events with 3-track vertices. The two vertical red dashed lines delineate the three ${d_{\mathrm {VV}}}$ bins. |

png pdf |
Figure 6-b:
Distribution of the $x$-$y$ distances between vertices, ${d_{\mathrm {VV}}}$, for 2017 and 2018 data, overlaid on the background template ${d_{\mathrm {VV}}^{\,\mathrm {C}}}$ constructed from one-vertex events in data normalized to the two-vertex data for events with exactly one 4-track vertex and one 3-track vertex. The two vertical red dashed lines delineate the three ${d_{\mathrm {VV}}}$ bins. |
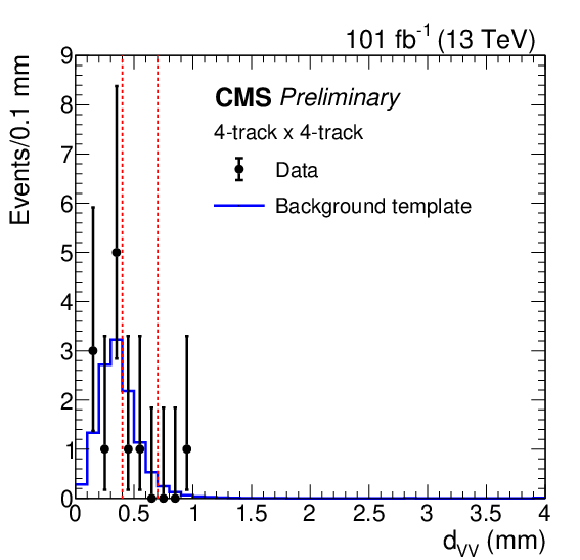
png pdf |
Figure 6-c:
Distribution of the $x$-$y$ distances between vertices, ${d_{\mathrm {VV}}}$, for 2017 and 2018 data, overlaid on the background template ${d_{\mathrm {VV}}^{\,\mathrm {C}}}$ constructed from one-vertex events in data normalized to the two-vertex data for events with 4-track vertices. The two vertical red dashed lines delineate the three ${d_{\mathrm {VV}}}$ bins. |
png pdf |
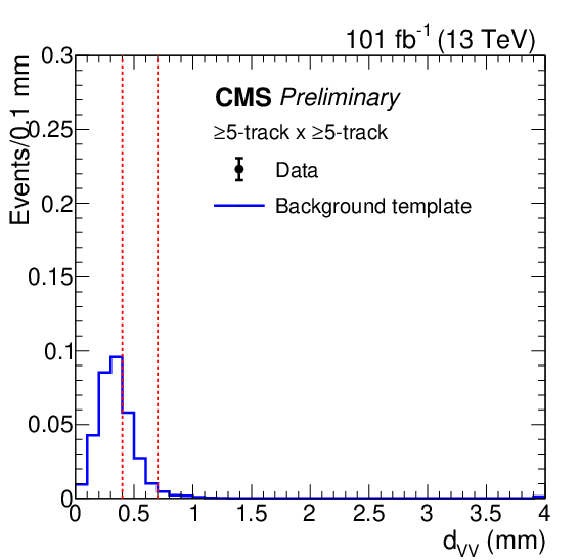
Figure 6-d:
The background template ${d_{\mathrm {VV}}^{\,\mathrm {C}}}$ for $\geq $5-track two-vertex events is normalized using one-vertex event information as described in the text. The two vertical red dashed lines delineate the three ${d_{\mathrm {VV}}}$ bins. |
png pdf |
Figure 7:
Observed 95% CL upper limits on cross section times branching fraction squared for the multijet (left) and dijet (right) signals as a function of mass and $c\tau $. The overlaid mass-lifetime exclusion curves assume pair-production cross sections for the neutralino (red) and gluino (pink) in multijet signals and top squark cross sections for the dijet signals with 100% branching fraction to each model's respective decay mode specified. The solid black (dashed colored) lines represent the observed (median expected) limits at 95% CL. The thin black lines represent the variation of the observed limit within theoretical uncertainties of the signal cross section. The thin dashed colored lines represent the region containing 68% of the expected limit distribution under the background-only hypothesis. The observed limits from the CMS displaced jets search [29] are also shown in green for comparison. |

png pdf |
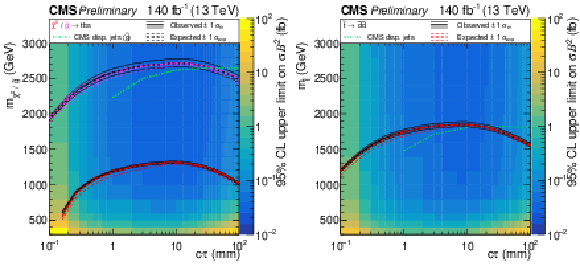
Figure 7-a:
Observed 95% CL upper limits on cross section times branching fraction squared for the multijet signal as a function of mass and $c\tau $. The overlaid mass-lifetime exclusion curves assume pair-production cross sections for the neutralino (red) and gluino (pink) in multijet signals and top squark cross sections for the dijet signals with 100% branching fraction to each model's respective decay mode specified. The solid black (dashed colored) lines represent the observed (median expected) limits at 95% CL. The thin black lines represent the variation of the observed limit within theoretical uncertainties of the signal cross section. The thin dashed colored lines represent the region containing 68% of the expected limit distribution under the background-only hypothesis. The observed limits from the CMS displaced jets search [29] are also shown in green for comparison. |
png pdf |
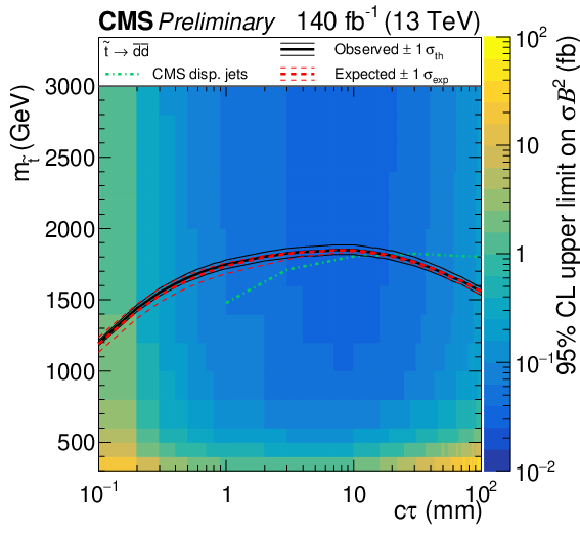
Figure 7-b:
Observed 95% CL upper limits on cross section times branching fraction squared for the dijet signal as a function of mass and $c\tau $. The overlaid mass-lifetime exclusion curves assume pair-production cross sections for the neutralino (red) and gluino (pink) in multijet signals and top squark cross sections for the dijet signals with 100% branching fraction to each model's respective decay mode specified. The solid black (dashed colored) lines represent the observed (median expected) limits at 95% CL. The thin black lines represent the variation of the observed limit within theoretical uncertainties of the signal cross section. The thin dashed colored lines represent the region containing 68% of the expected limit distribution under the background-only hypothesis. The observed limits from the CMS displaced jets search [29] are also shown in green for comparison. |

png pdf |
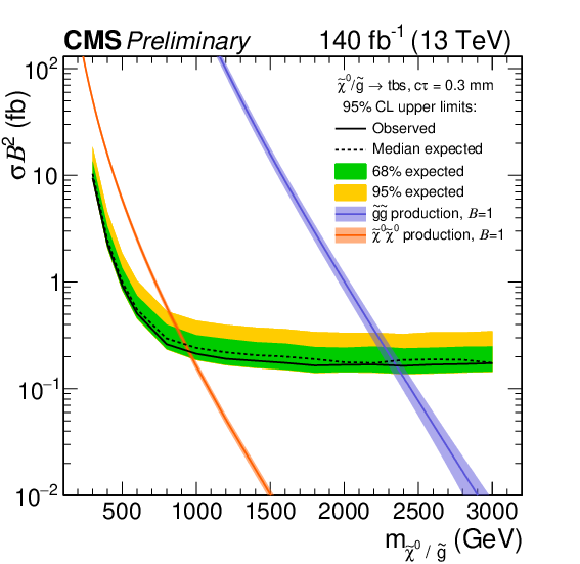
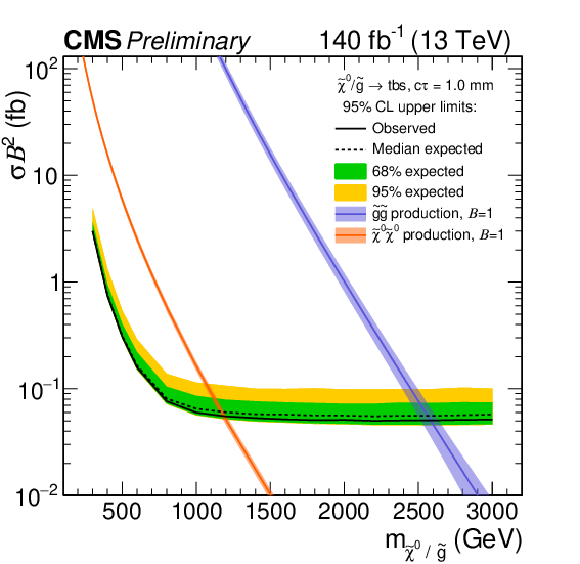
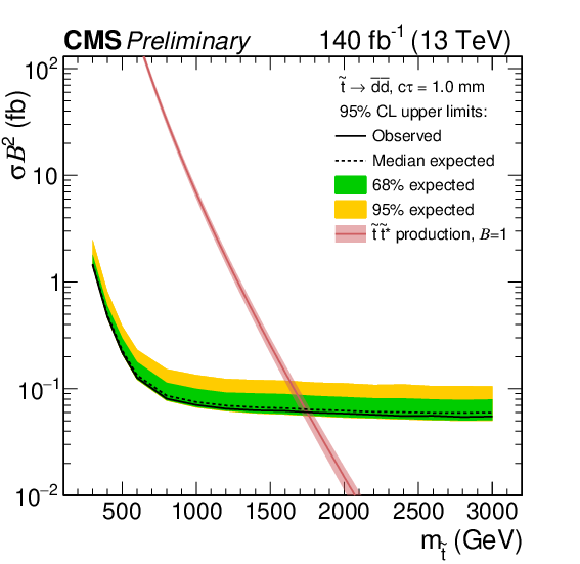
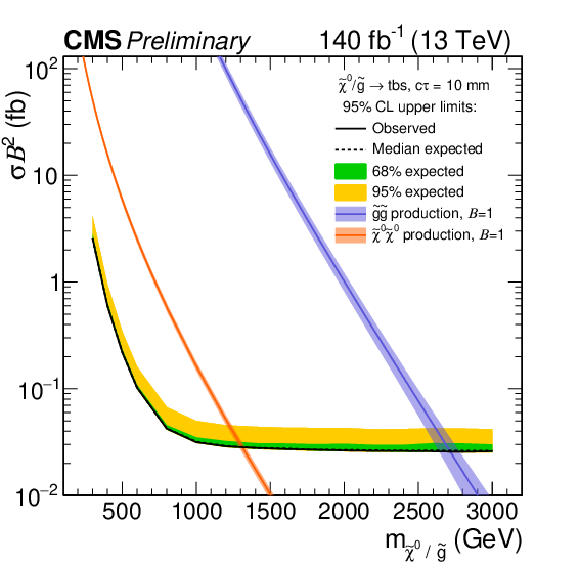
Figure 8:
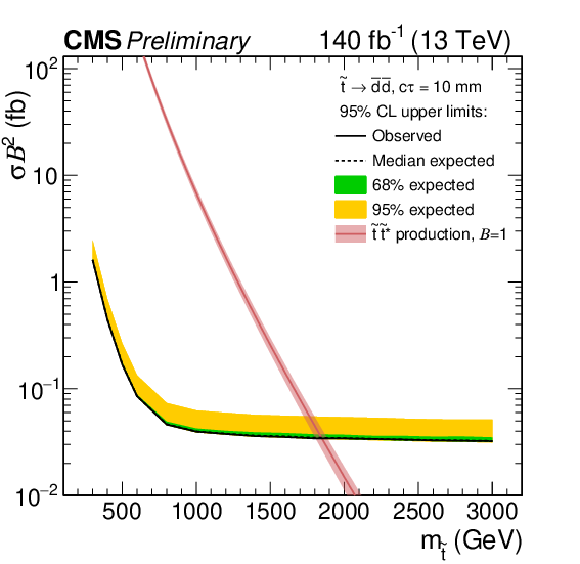
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of mass for multijet signals (left) and dijet signals (right), for a fixed $c\tau $ of 0.3 mm (top), 1 mm (middle), and 10 mm (bottom) in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |
png pdf |
Figure 8-a:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of mass for the multijet signal, for a fixed $c\tau $ of 0.3 mm in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |

png pdf |
Figure 8-b:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of mass for the dijet signal, for a fixed $c\tau $ of 0.3 mm in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |
png pdf |
Figure 8-c:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of mass for the multijet signal, for a fixed $c\tau $ of 1 mm in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |
png pdf |
Figure 8-d:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of mass for the dijet signal, for a fixed $c\tau $ of 1 mm in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |
png pdf |
Figure 8-e:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of mass for the multijet signal, for a fixed $c\tau $ of 10 mm in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |
png pdf |
Figure 8-f:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of mass for the dijet signal, for a fixed $c\tau $ of 10 mm in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |
png pdf |
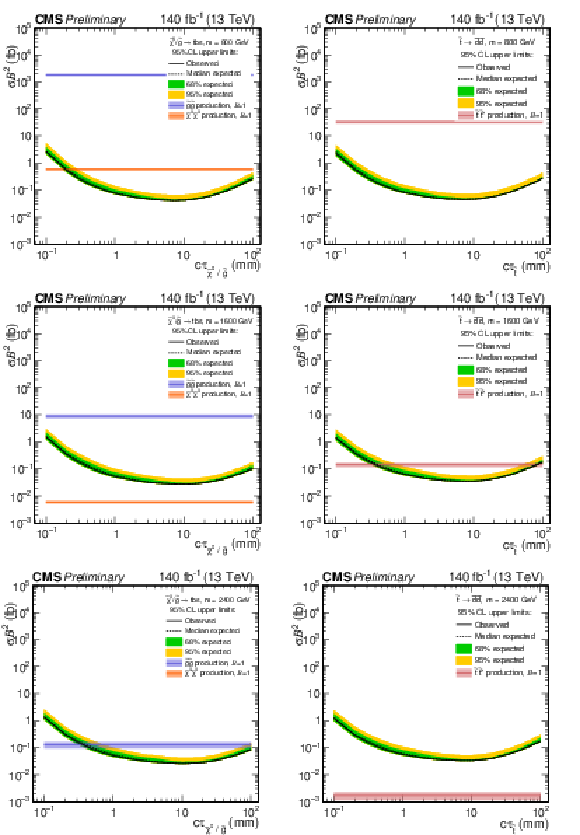
Figure 9:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of $c\tau $ for multijet signals (left) and dijet signals (right), for a fixed mass of 800 GeV (top), 1600 GeV (middle), and 2400 GeV (bottom) in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |
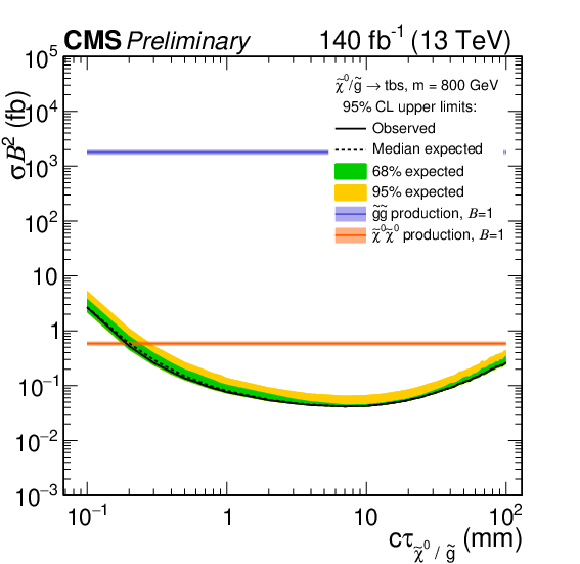
png pdf |
Figure 9-a:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of $c\tau $ for multijet dijet signal, for a fixed mass of 800 GeV in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |
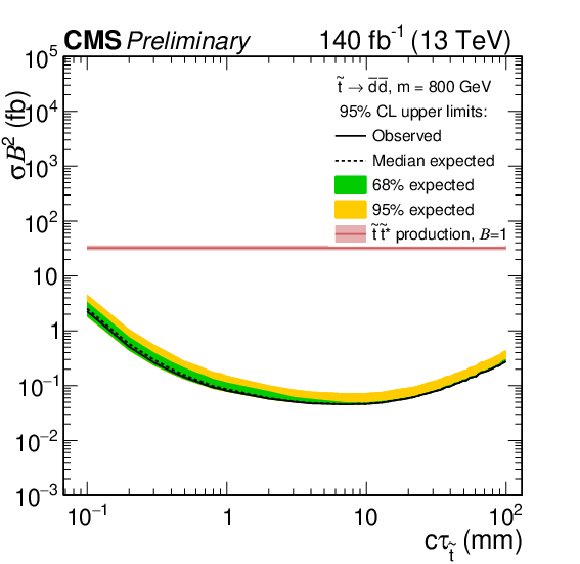
png pdf |
Figure 9-b:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of $c\tau $ for multijet dijet signal, for a fixed mass of 800 GeV in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |

png pdf |
Figure 9-c:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of $c\tau $ for multijet dijet signal, for a fixed mass of 1600 GeV in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |

png pdf |
Figure 9-d:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of $c\tau $ for multijet dijet signal, for a fixed mass of 1600 GeV in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |
png pdf |
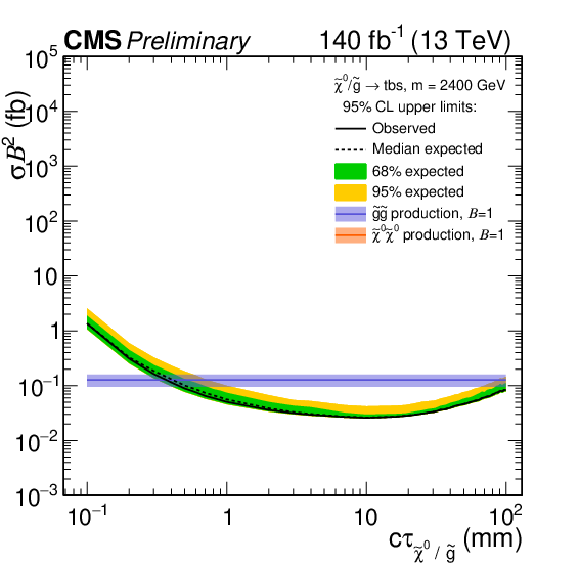
Figure 9-e:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of $c\tau $ for multijet dijet signal, for a fixed mass of 2400 GeV in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |
png pdf |
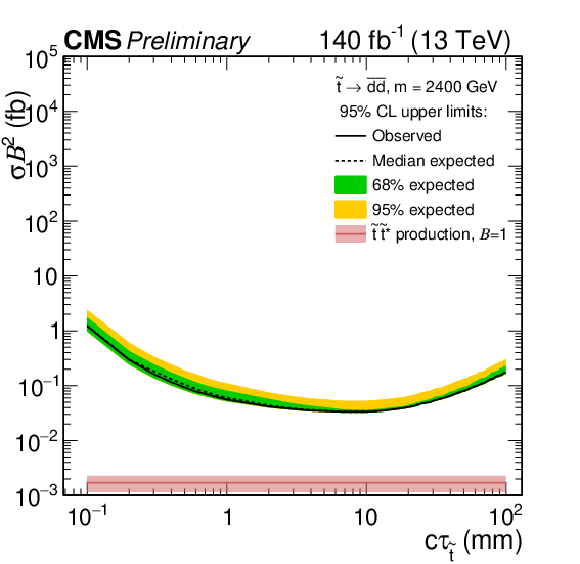
Figure 9-f:
Observed and expected 95% CL upper limits on cross section times branching fraction squared as a function of $c\tau $ for multijet dijet signal, for a fixed mass of 2400 GeV in the full Run 2 data set. The neutralino and gluino pair production cross sections are overlaid for the multijet signals, and the top squark pair production cross section is overlaid for the dijet signals. |
| Tables | |
png pdf |
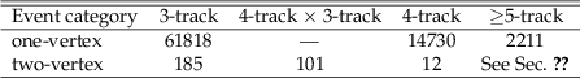
Table 1:
Event yields in the control regions in 101 fb$^{-1}$ of data. The "one-vertex'' events correspond to events containing exactly one vertex with the specified number of tracks. The "two-vertex'' events have two or more vertices containing the specified numbers of tracks. We seek the signal in the $\geq $5-track two-vertex sample. |
png pdf |
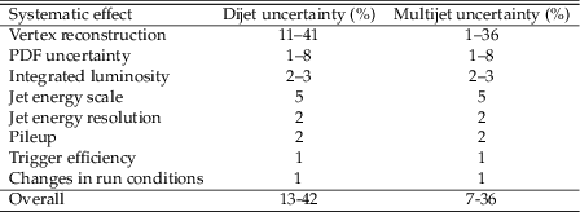
Table 2:
Signal-related systematic uncertainties for dijet and multijet signal models. The overall uncertainty is the sum in quadrature of the individual uncertainties. |
png pdf |
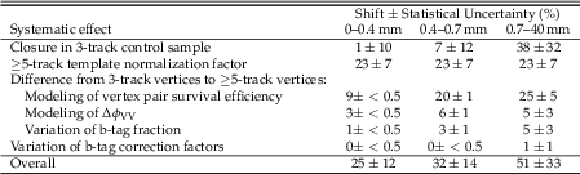
Table 3:
Systematic shifts and the statistical uncertainties on them in the background prediction in each ${d_{\mathrm {VV}}^{\,\mathrm {C}}}$ bin arising from varying the construction of the ${d_{\mathrm {VV}}^{\,\mathrm {C}}}$ template. The overall systematic uncertainty and its statistical uncertainty in each bin is the sum in quadrature of the shifts assuming no correlations among the sources. |

png pdf |
Table 4:
Predicted yields for the background-only normalized template, the predicted yields for multijet signals with mass of 800 GeV, production cross section of 0.3 fb, and $c\tau =$ 0.3, 1.0, and 10 mm, and the observed yield in each ${d_{\mathrm {VV}}}$ bin. The uncertainty in the signal yields and the systematic uncertainty in the background prediction reflect the systematic uncertainties given in Tables 2 and 3, respectively. |
| Summary |
| We present a search for pair-produced long-lived particles decaying into multijet and dijet final states using proton-proton collision events collected with the CMS detector at a center-of-mass energy of 13 TeV during the full Run 2 data collection period, corresponding to an integrated luminosity of 140 fb$^{-1}$. No events were observed in the signal region in the 2017 and 2018 datasets, and no excess yield beyond the standard model prediction is observed in the full Run 2 dataset. At 95% CL, upper limits are set for an RPV SUSY model in which a long-lived neutralino or gluino decays into a multijet final state with top, bottom, and strange antiquarks. Signal pair-production cross sections larger than 0.08 fb are excluded for long-lived neutralino, gluino, and top squark masses between 800 and 3000 GeV and mean proper decay lengths between 1 mm and 25 mm. For the range of mean proper decay lengths between 0.6 and 90 mm, the data exclude gluino masses up to 2500 GeV. For a neutralino LSP, the data exclude neutralino masses up to 1100 GeV for mean proper decay lengths between 0.6 and 70 mm. Additionally, limits are placed for an RPV SUSY model in which a long-lived top squark decays into a dijet final state with two down antiquarks. The data exclude top squark masses up to 1600 GeV for mean proper decay lengths between 0.4 and 80 mm. These are the most stringent bounds on these models for $c\tau$ between 100 $\mu$m and 15 mm for all masses considered, complementing the results of the CMS displaced jet search [29]. While the search directly constrains these two RPV SUSY models, the techniques and methodology are generic and the results are applicable to other models of pair-produced long-lived particles that decay into jets. A method is provided in Appendix A1 to facilitate the reinterpretation of these results for alternative models. |
| References | ||||
| 1 | R. Barbier et al. | $ R $-parity violating supersymmetry | PR 420 (2005) 1 | hep-ph/0406039 |
| 2 | C. Cs\'aki, Y. Grossman, and B. Heidenreich | Minimal flavor violation supersymmetry: a natural theory for $ R $-parity violation | PRD 85 (2012) 095009 | 1111.1239 |
| 3 | C. Cs\'aki et al. | Phenomenology of a long-lived LSP with $ R $-parity violation | JHEP 08 (2015) 016 | 1505.00784 |
| 4 | L. J. Hall and M. Suzuki | Explicit $ R $-parity breaking in supersymmetric models | NPB 231 (1984) 419 | |
| 5 | N. Arkani-Hamed and S. Dimopoulos | Supersymmetric unification without low energy supersymmetry and signatures for fine-tuning at the LHC | JHEP 06 (2005) 073 | hep-th/0405159 |
| 6 | N. Arkani-Hamed, S. Dimopoulos, G. F. Giudice, and A. Romanino | Aspects of split supersymmetry | NPB 709 (2005) 3 | hep-ph/0409232 |
| 7 | N. Arkani-Hamed et al. | Simply unnatural supersymmetry | 2012 | |
| 8 | A. Arvanitaki, N. Craig, S. Dimopoulos, and G. Villadoro | Mini-split | JHEP 02 (2013) 126 | 1210.0555 |
| 9 | P. Gambino, G. F. Giudice, and P. Slavich | Gluino decays in split supersymmetry | NPB 726 (2005) 35 | hep-ph/0506214 |
| 10 | G. F. Giudice and A. Romanino | Split supersymmetry | NPB 699 (2004) 65 | hep-ph/0406088 |
| 11 | J. L. Hewett, B. Lillie, M. Masip, and T. G. Rizzo | Signatures of long-lived gluinos in split supersymmetry | JHEP 09 (2004) 070 | hep-ph/0408248 |
| 12 | T. Han, Z. Si, K. M. Zurek, and M. J. Strassler | Phenomenology of hidden valleys at hadron colliders | JHEP 07 (2008) 008 | 0712.2041 |
| 13 | M. J. Strassler and K. M. Zurek | Echoes of a hidden valley at hadron colliders | PLB 651 (2007) 374 | hep-ph/0604261 |
| 14 | M. J. Strassler and K. M. Zurek | Discovering the Higgs through highly-displaced vertices | PLB 661 (2008) 263 | hep-ph/0605193 |
| 15 | J. Fan, M. Reece, and J. T. Ruderman | Stealth supersymmetry | JHEP 11 (2011) 012 | 1105.5135 |
| 16 | J. Fan, M. Reece, and J. T. Ruderman | A stealth supersymmetry sampler | JHEP 07 (2012) 196 | 1201.4875 |
| 17 | L. Calibbi, L. Lopez-Honorez, S. Lowette, and A. Mariotti | Singlet-Doublet dark matter freeze-in: LHC displaced signatures versus cosmology | JHEP 09 (2018) 037 | 1805.04423 |
| 18 | R. T. Co, F. D'Eramo, L. J. Hall, and D. Pappadopulo | Freeze-In dark matter with displaced signatures at colliders | JCAP 1512 (2015) 024 | 1506.07532 |
| 19 | Y. Cui, L. Randall, and B. Shuve | A WIMPy baryogenesis miracle | JHEP 04 (2012) 075 | 1112.2704 |
| 20 | Y. Cui and R. Sundrum | Baryogenesis for weakly interacting massive particles | PRD 87 (2013) 116013 | 1212.2973 |
| 21 | Y. Cui and B. Shuve | Probing baryogenesis with displaced vertices at the LHC | JHEP 02 (2015) 049 | 1409.6729 |
| 22 | L. J. Hall, K. Jedamzik, J. March-Russell, and S. M. West | Freeze-in production of FIMP dark matter | JHEP 03 (2010) 080 | 0911.1120 |
| 23 | D. E. Kaplan, M. A. Luty, and K. M. Zurek | Asymmetric dark matter | PRD 79 (2009) 115016 | 0901.4117 |
| 24 | I.-W. Kim and K. M. Zurek | Flavor and collider signatures of asymmetric dark matter | PRD 89 (2014) 035008 | 1310.2617 |
| 25 | CMS Collaboration | Search for long-lived particles with displaced vertices in multijet events in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | PRD 98 (2018) 092011 | CMS-EXO-17-018 1808.03078 |
| 26 | CMS Collaboration | CMS Technical Design Report for the Pixel Detector Upgrade | ||
| 27 | ATLAS Collaboration | Search for long-lived, massive particles in events with displaced vertices and missing transverse momentum in $ \sqrt{s} = $ 13 TeV pp collisions with the ATLAS detector | PRD 97 (2018) 052012 | 1710.04901 |
| 28 | ATLAS Collaboration | Search for long-lived, massive particles in events with a displaced vertex and a muon with large impact parameter in $ pp $ collisions at $ \sqrt{s} = $ 13 TeV with the ATLAS detector | 2003.11956 | |
| 29 | CMS Collaboration | Search for long-lived particles decaying into displaced jets | CMS-PAS-EXO-19-021 | CMS-PAS-EXO-19-021 |
| 30 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | CMS-00-001 |
| 31 | CMS Collaboration | Track impact parameter resolution in the 2017 dataset with the CMS Phase-1 Pixel detector | CDS | |
| 32 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 33 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 34 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_t $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 35 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 36 | CMS Collaboration | Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV | JINST 12 (2017) P02014 | CMS-JME-13-004 1607.03663 |
| 37 | T. Sjostrand et al. | An Introduction to PYTHIA 8.2 | CPC 191 (2015) 159 | 1410.3012 |
| 38 | NNPDF Collaboration | Parton distributions from high-precision collider data | EPJC77 (2017) 663 | 1706.00428 |
| 39 | CMS Collaboration | Extraction and validation of a new set of CMS PYTHIA8 tunes from underlying-event measurements | EPJC 80 (2020) 4 | CMS-GEN-17-001 1903.12179 |
| 40 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 41 | NNPDF Collaboration | Parton distributions for the LHC Run II | JHEP 04 (2015) 040 | 1410.8849 |
| 42 | J. Alwall et al. | Comparative study of various algorithms for the merging of parton showers and matrix elements in hadronic collisions | EPJC 53 (2008) 473 | 0706.2569 |
| 43 | \GEANTfour Collaboration | GEANT4--a simulation toolkit | NIMA 506 (2003) 250 | |
| 44 | CMS Collaboration | Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV | JINST 13 (2018) P05011 | CMS-BTV-16-002 1712.07158 |
| 45 | J. Butterworth et al. | PDF4LHC recommendations for LHC Run II | JPG 43 (2016) 023001 | 1510.03865 |
| 46 | CMS Collaboration | CMS luminosity measurement for the 2017 data-taking period at $ \sqrt{s}= $ 13 TeV | CMS-PAS-LUM-17-004 | CMS-PAS-LUM-17-004 |
| 47 | CMS Collaboration | CMS luminosity measurement for the 2018 data-taking period at $ \sqrt{s}= $ 13 TeV | CMS-PAS-LUM-18-002 | CMS-PAS-LUM-18-002 |
| 48 | C. Borschensky et al. | Squark and gluino production cross sections in pp collisions at $ \sqrt{s}= $ 13, 14, 33 and 100 TeV | EPJC 74 (2014) 3174 | 1407.5066 |
| 49 | W. Beenakker et al. | NNLL resummation for stop pair-production at the LHC | JHEP 05 (2016) 153 | 1601.02954 |
| 50 | M. Beneke, J. Piclum, C. Schwinn, and C. Wever | NNLL soft and Coulomb resummation for squark and gluino production at the LHC | JHEP 10 (2016) 054 | 1607.07574 |
| 51 | B. Fuks, M. Klasen, D. R. Lamprea, and M. Rothering | Gaugino production in proton-proton collisions at a center-of-mass energy of 8 TeV | JHEP 10 (2012) 081 | 1207.2159 |
| 52 | B. Fuks, M. Klasen, D. R. Lamprea, and M. Rothering | Precision predictions for electroweak superpartner production at hadron colliders with Resummino | EPJC 73 (2013) 2480 | 1304.0790 |
| 53 | CMS Collaboration | Search for pair-produced resonances decaying to quark pairs in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | PRD 98 (2018), no. 11, 112014 | CMS-EXO-17-021 1808.03124 |
| 54 | CMS Collaboration | Search for pair-produced three-jet resonances in proton-proton collisions at $ \sqrt s = $ 13 TeV | PRD 99 (2019), no. 1, 012010 | CMS-EXO-17-030 1810.10092 |

|
Compact Muon Solenoid LHC, CERN |

|

|

|

|

|

|