Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-SMP-21-008 ; CERN-EP-2023-257 | ||
| Measurement of multidifferential cross sections for dijet production in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | ||
| CMS Collaboration | ||
| 28 December 2023 | ||
| Accepted for publication in Eur. Phys. J. C | ||
| Abstract: A measurement of the dijet production cross section is reported based on proton-proton collision data collected in 2016 at $ \sqrt{s}= $ 13 TeV by the CMS experiment at the CERN LHC, corresponding to an integrated luminosity of up to 36.3 fb$ ^{-1} $. Jets are reconstructed with the anti-$ k_{\mathrm{T}} $ algorithm for distance parameters of $ R= $ 0.4 and 0.8. Cross sections are measured double-differentially (2D) as a function of the largest absolute rapidity $ |y|_{\text{max}} $ of the two jets with the highest transverse momenta $ p_{\mathrm{T}} $ and their invariant mass $ m_{1,2} $, and triple-differentially (3D) as a function of the rapidity separation $ y^{*} $, the total boost $ y_{\text{b}} $, and either $ m_{1,2} $ or the average $ p_{\mathrm{T}} $ of the two jets. The cross sections are unfolded to correct for detector effects and are compared with fixed-order calculations derived at next-to-next-to-leading order in perturbative quantum chromodynamics. The impact of the measurements on the parton distribution functions and the strong coupling constant at the mass of the Z boson is investigated, yielding a value of $ \alpha_\mathrm{S}(m_{\mathrm{Z}})= $ 0.1179 $ \pm $ 0.0019. | ||
| Links: e-print arXiv:2312.16669 [hep-ex] (PDF) ; CDS record ; inSPIRE record ; HepData record ; CADI line (restricted) ; | ||
| Figures & Tables | Summary | Additional Figures | References | CMS Publications |
|---|
| Figures | |
png pdf |
Figure 1:
Illustration of the dijet rapidity phase space, highlighting the relationship between the variables used for the 2D and 3D measurements. The colored triangles are suggestive of the orientation of the two jets in the different phase space regions in the laboratory frame, assuming that the beam line runs horizontally. |
png pdf |
Figure 2:
Response matrix for the 2D measurement as a function of $ m_{1,2} $ using jets with $ R = $ 0.8. The entries represent the probability for a dijet event generated in the phase space region ($ m_{1,2}^\text{gen} $, $ |y|_{\text{max}}^\text{gen} $) indicated on the $ x $ axis to be reconstructed in the phase space region ($ m_{1,2}^\text{rec} $, $ |y|_{\text{max}}^\text{rec} $) indicated on the $ y $ axis. Response matrices for all other jet sizes and observables can be found in Appendix app:supplementary-material. |
png pdf |
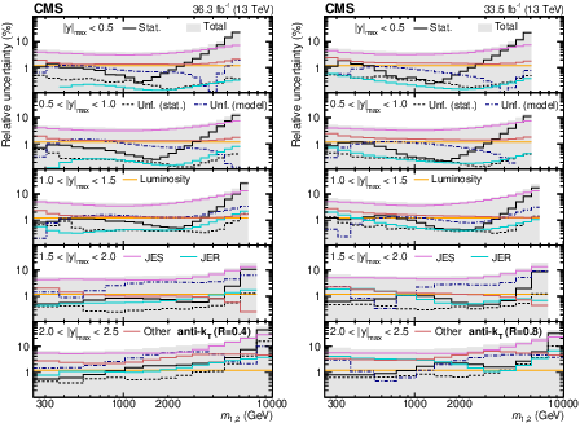
Figure 3:
Breakdown of the experimental uncertainty for the 2D measurements as a function of $ m_{1,2} $ using jets with $ R = $ 0.4 (left) and 0.8 (right). The individual components are discussed in Section 7, and the abbreviation "Unf." refers to the unfolding uncertainties. The shaded area represents the sum in quadrature of all statistical and systematic uncertainty components. |
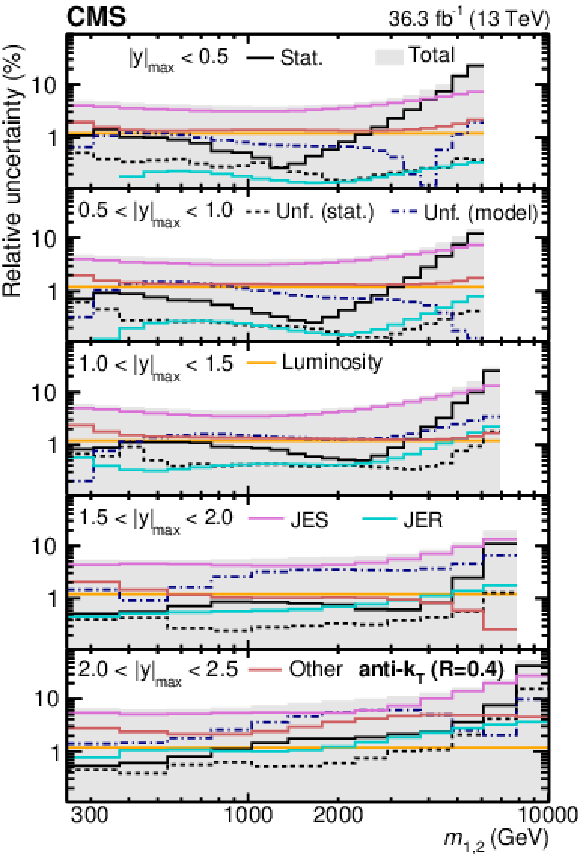
png pdf |
Figure 3-a:
Breakdown of the experimental uncertainty for the 2D measurements as a function of $ m_{1,2} $ using jets with $ R = $ 0.4 (left) and 0.8 (right). The individual components are discussed in Section 7, and the abbreviation "Unf." refers to the unfolding uncertainties. The shaded area represents the sum in quadrature of all statistical and systematic uncertainty components. |
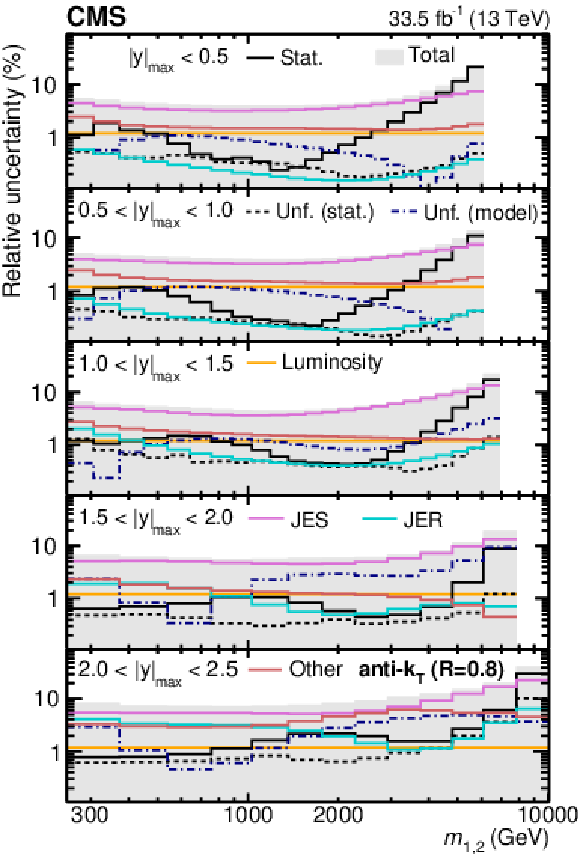
png pdf |
Figure 3-b:
Breakdown of the experimental uncertainty for the 2D measurements as a function of $ m_{1,2} $ using jets with $ R = $ 0.4 (left) and 0.8 (right). The individual components are discussed in Section 7, and the abbreviation "Unf." refers to the unfolding uncertainties. The shaded area represents the sum in quadrature of all statistical and systematic uncertainty components. |
png pdf |
Figure 4:
Breakdown of the experimental uncertainty for the 3D measurement as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $\ using jets with $ R = $ 0.4. The individual components are discussed in Section 7. The shaded area represents the sum in quadrature of all statistical and systematic uncertainty components. Similar plots for all other jet sizes and observables can be found in Appendix app:supplementary-material. |
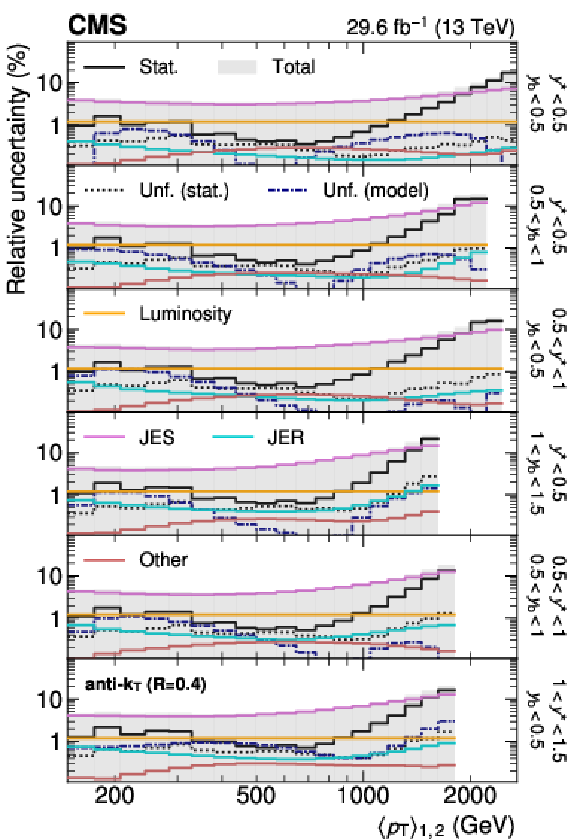
png pdf |
Figure 4-a:
Breakdown of the experimental uncertainty for the 3D measurement as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $\ using jets with $ R = $ 0.4. The individual components are discussed in Section 7. The shaded area represents the sum in quadrature of all statistical and systematic uncertainty components. Similar plots for all other jet sizes and observables can be found in Appendix app:supplementary-material. |
png pdf |
Figure 4-b:
Breakdown of the experimental uncertainty for the 3D measurement as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $\ using jets with $ R = $ 0.4. The individual components are discussed in Section 7. The shaded area represents the sum in quadrature of all statistical and systematic uncertainty components. Similar plots for all other jet sizes and observables can be found in Appendix app:supplementary-material. |
png pdf |
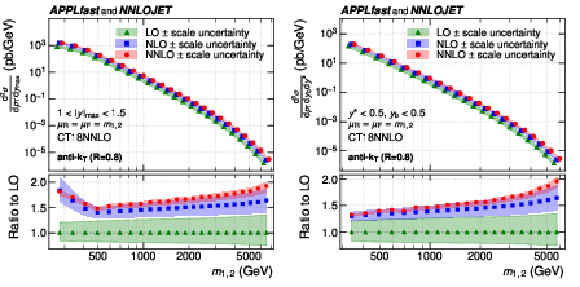
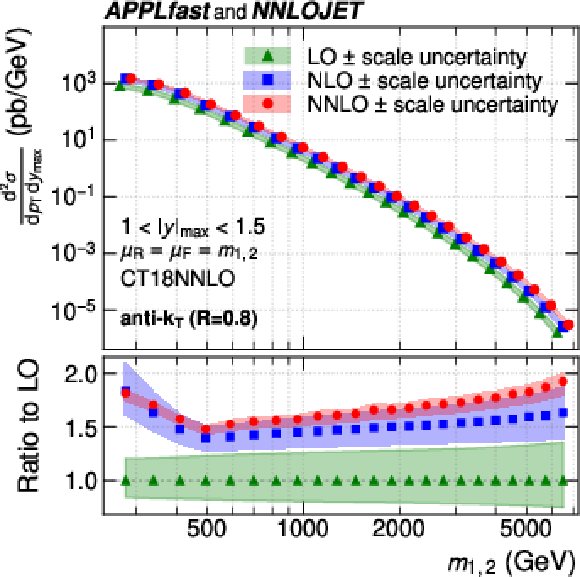
Figure 5:
Theoretical predictions for the 2D (left) and 3D (right) cross sections, as a function of $ m_{1,2} $, illustrated here in the rapidity regions 1.0 $ < |y|_{\text{max}} < $ 1.5 and $ y_{\text{b}} < $ 0.5, $ y^{*} < $ 0.5, together with the corresponding six-point scale uncertainty for $ \mu_{\mathrm{R}}=\mu_{\mathrm{F}}=m_{1,2} $ using the CT18 NNLO PDF set. In the upper panels, the curves and symbols are slightly shifted for better visibility. The lower panels show the ratio to the respective prediction at LO\@. The fluctuations in the NNLO predictions are due to the limited statistical precision of the calculation. |
png pdf |
Figure 5-a:
Theoretical predictions for the 2D (left) and 3D (right) cross sections, as a function of $ m_{1,2} $, illustrated here in the rapidity regions 1.0 $ < |y|_{\text{max}} < $ 1.5 and $ y_{\text{b}} < $ 0.5, $ y^{*} < $ 0.5, together with the corresponding six-point scale uncertainty for $ \mu_{\mathrm{R}}=\mu_{\mathrm{F}}=m_{1,2} $ using the CT18 NNLO PDF set. In the upper panels, the curves and symbols are slightly shifted for better visibility. The lower panels show the ratio to the respective prediction at LO\@. The fluctuations in the NNLO predictions are due to the limited statistical precision of the calculation. |
png pdf |
Figure 5-b:
Theoretical predictions for the 2D (left) and 3D (right) cross sections, as a function of $ m_{1,2} $, illustrated here in the rapidity regions 1.0 $ < |y|_{\text{max}} < $ 1.5 and $ y_{\text{b}} < $ 0.5, $ y^{*} < $ 0.5, together with the corresponding six-point scale uncertainty for $ \mu_{\mathrm{R}}=\mu_{\mathrm{F}}=m_{1,2} $ using the CT18 NNLO PDF set. In the upper panels, the curves and symbols are slightly shifted for better visibility. The lower panels show the ratio to the respective prediction at LO\@. The fluctuations in the NNLO predictions are due to the limited statistical precision of the calculation. |
png pdf |
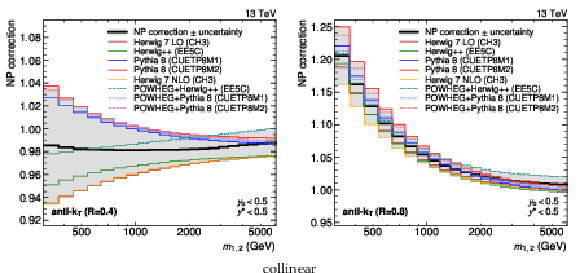
Figure 6:
Nonperturbative correction factors obtained for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $, illustrated here in the rapidity region ($ y_{\text{b}} < $ 0.5, $ y^{*} < $ 0.5). Individual correction factors are first derived from simulation using eight different MC configurations. The largest and smallest value obtained in each observable bin is then used to define the final correction factor and its associated uncertainty. The correction values are larger for jets with $ R = $ 0.8, increasing to over 20% in the lowest $ m_{1,2} $ bin. |
png pdf |
Figure 6-a:
Nonperturbative correction factors obtained for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $, illustrated here in the rapidity region ($ y_{\text{b}} < $ 0.5, $ y^{*} < $ 0.5). Individual correction factors are first derived from simulation using eight different MC configurations. The largest and smallest value obtained in each observable bin is then used to define the final correction factor and its associated uncertainty. The correction values are larger for jets with $ R = $ 0.8, increasing to over 20% in the lowest $ m_{1,2} $ bin. |
png pdf |
Figure 6-b:
Nonperturbative correction factors obtained for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $, illustrated here in the rapidity region ($ y_{\text{b}} < $ 0.5, $ y^{*} < $ 0.5). Individual correction factors are first derived from simulation using eight different MC configurations. The largest and smallest value obtained in each observable bin is then used to define the final correction factor and its associated uncertainty. The correction values are larger for jets with $ R = $ 0.8, increasing to over 20% in the lowest $ m_{1,2} $ bin. |
png pdf |
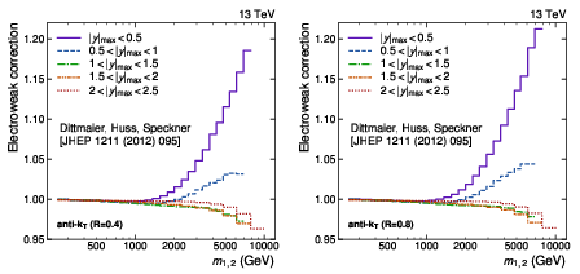
Figure 7:
Electroweak correction factors obtained for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ in the five different $ |y|_{\text{max}} $ regions. The corrections depend strongly on the kinematic properties of the jets and are observed to be largest at central rapidities for $ m_{1,2} > $ 1 TeV. |
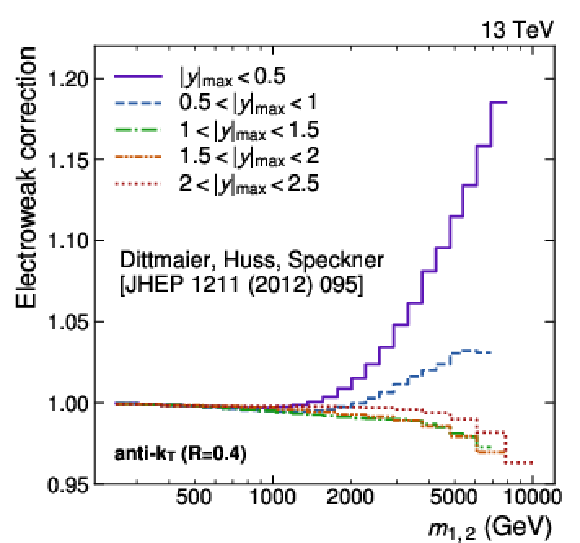
png pdf |
Figure 7-a:
Electroweak correction factors obtained for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ in the five different $ |y|_{\text{max}} $ regions. The corrections depend strongly on the kinematic properties of the jets and are observed to be largest at central rapidities for $ m_{1,2} > $ 1 TeV. |
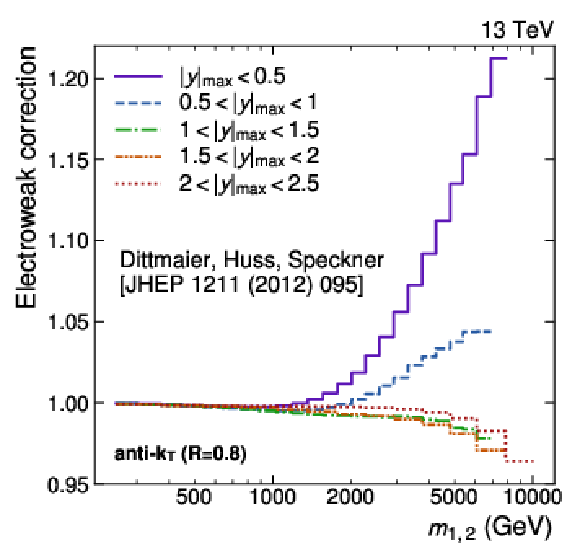
png pdf |
Figure 7-b:
Electroweak correction factors obtained for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ in the five different $ |y|_{\text{max}} $ regions. The corrections depend strongly on the kinematic properties of the jets and are observed to be largest at central rapidities for $ m_{1,2} > $ 1 TeV. |
png pdf |
Figure 8:
Differential dijet cross sections, illustrated here for the 2D measurement as a function of $ m_{1,2} $ using jets with $ R = $ 0.8 (left), and the 3D measurement as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ using jets with $ R = $ 0.4 (right). The markers and lines indicate the measured unfolded cross sections and the corresponding NNLO predictions, respectively. For better visibility, the values are scaled by a factor depending on the rapidity region, as indicated in the legend. Analogous plots for all other jet sizes and observables can be found in Appendix app:supplementary-material. |
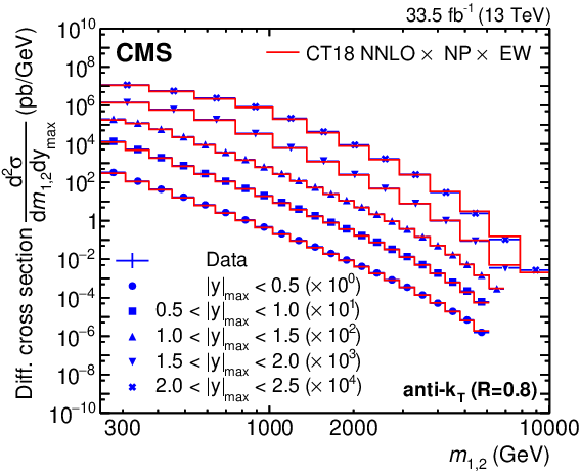
png pdf |
Figure 8-a:
Differential dijet cross sections, illustrated here for the 2D measurement as a function of $ m_{1,2} $ using jets with $ R = $ 0.8 (left), and the 3D measurement as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ using jets with $ R = $ 0.4 (right). The markers and lines indicate the measured unfolded cross sections and the corresponding NNLO predictions, respectively. For better visibility, the values are scaled by a factor depending on the rapidity region, as indicated in the legend. Analogous plots for all other jet sizes and observables can be found in Appendix app:supplementary-material. |
png pdf |
Figure 8-b:
Differential dijet cross sections, illustrated here for the 2D measurement as a function of $ m_{1,2} $ using jets with $ R = $ 0.8 (left), and the 3D measurement as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ using jets with $ R = $ 0.4 (right). The markers and lines indicate the measured unfolded cross sections and the corresponding NNLO predictions, respectively. For better visibility, the values are scaled by a factor depending on the rapidity region, as indicated in the legend. Analogous plots for all other jet sizes and observables can be found in Appendix app:supplementary-material. |
png pdf |
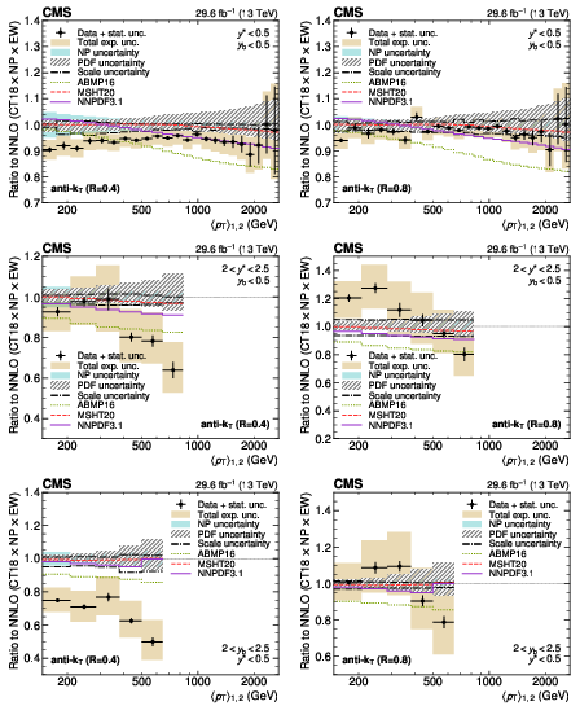
Figure 9:
Comparison of the 2D dijet cross section as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right). Shown are the ratios of the measured cross sections (markers) to the predictions obtained using the CT18 NNLO PDF set. The error bars and shaded yellow regions indicate the statistical and the total experimental uncertainties of the data, respectively, and the hatched teal band indicates the sum in quadrature of the PDF, NP, and scale uncertainties. Alternative theoretical predictions obtained using other global PDF sets are shown as colored lines. Similar plots for the individual rapidity regions can be found in Appendix app:supplementary-material. |
png pdf |
Figure 9-a:
Comparison of the 2D dijet cross section as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right). Shown are the ratios of the measured cross sections (markers) to the predictions obtained using the CT18 NNLO PDF set. The error bars and shaded yellow regions indicate the statistical and the total experimental uncertainties of the data, respectively, and the hatched teal band indicates the sum in quadrature of the PDF, NP, and scale uncertainties. Alternative theoretical predictions obtained using other global PDF sets are shown as colored lines. Similar plots for the individual rapidity regions can be found in Appendix app:supplementary-material. |
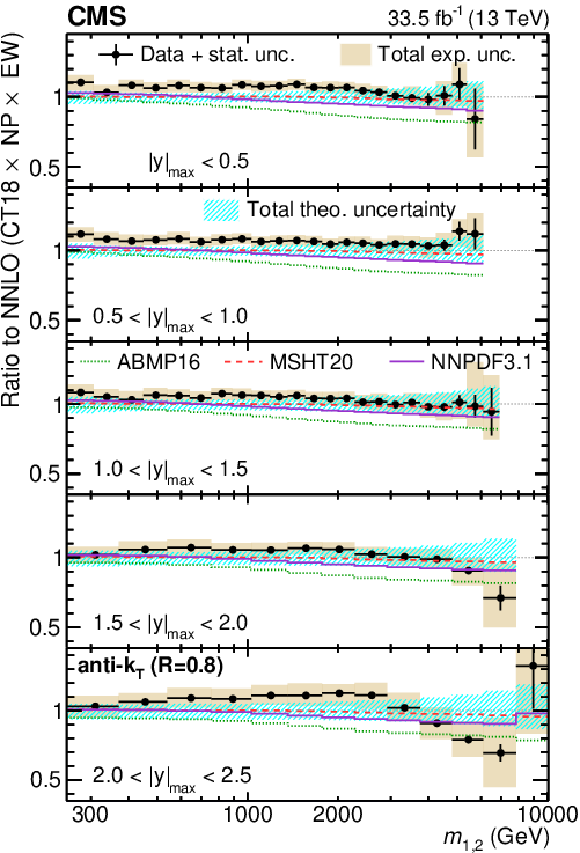
png pdf |
Figure 9-b:
Comparison of the 2D dijet cross section as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right). Shown are the ratios of the measured cross sections (markers) to the predictions obtained using the CT18 NNLO PDF set. The error bars and shaded yellow regions indicate the statistical and the total experimental uncertainties of the data, respectively, and the hatched teal band indicates the sum in quadrature of the PDF, NP, and scale uncertainties. Alternative theoretical predictions obtained using other global PDF sets are shown as colored lines. Similar plots for the individual rapidity regions can be found in Appendix app:supplementary-material. |
png pdf |
Figure 10:
Comparison of the 3D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three out of the total of 15 rapidity regions. The data points and predictions for alternative PDFs are analogous to those in Fig. 9. In addition, the separate contributions to the theory uncertainty due to the CT18 PDFs, NP corrections, and six-point scale variations are shown explicitly. Similar plots for all rapidity regions and observables can be found in Appendix app:supplementary-material. |
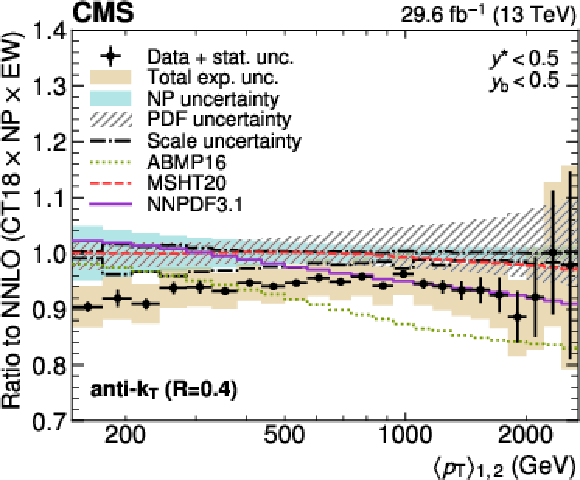
png pdf |
Figure 10-a:
Comparison of the 3D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three out of the total of 15 rapidity regions. The data points and predictions for alternative PDFs are analogous to those in Fig. 9. In addition, the separate contributions to the theory uncertainty due to the CT18 PDFs, NP corrections, and six-point scale variations are shown explicitly. Similar plots for all rapidity regions and observables can be found in Appendix app:supplementary-material. |
png pdf |
Figure 10-b:
Comparison of the 3D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three out of the total of 15 rapidity regions. The data points and predictions for alternative PDFs are analogous to those in Fig. 9. In addition, the separate contributions to the theory uncertainty due to the CT18 PDFs, NP corrections, and six-point scale variations are shown explicitly. Similar plots for all rapidity regions and observables can be found in Appendix app:supplementary-material. |
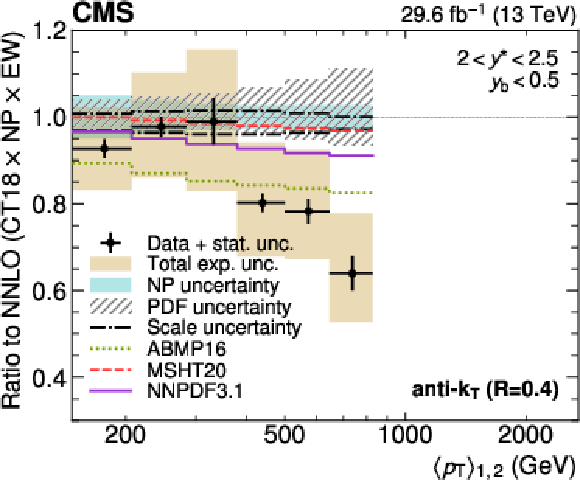
png pdf |
Figure 10-c:
Comparison of the 3D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three out of the total of 15 rapidity regions. The data points and predictions for alternative PDFs are analogous to those in Fig. 9. In addition, the separate contributions to the theory uncertainty due to the CT18 PDFs, NP corrections, and six-point scale variations are shown explicitly. Similar plots for all rapidity regions and observables can be found in Appendix app:supplementary-material. |
png pdf |
Figure 10-d:
Comparison of the 3D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three out of the total of 15 rapidity regions. The data points and predictions for alternative PDFs are analogous to those in Fig. 9. In addition, the separate contributions to the theory uncertainty due to the CT18 PDFs, NP corrections, and six-point scale variations are shown explicitly. Similar plots for all rapidity regions and observables can be found in Appendix app:supplementary-material. |
png pdf |
Figure 10-e:
Comparison of the 3D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three out of the total of 15 rapidity regions. The data points and predictions for alternative PDFs are analogous to those in Fig. 9. In addition, the separate contributions to the theory uncertainty due to the CT18 PDFs, NP corrections, and six-point scale variations are shown explicitly. Similar plots for all rapidity regions and observables can be found in Appendix app:supplementary-material. |
png pdf |
Figure 10-f:
Comparison of the 3D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three out of the total of 15 rapidity regions. The data points and predictions for alternative PDFs are analogous to those in Fig. 9. In addition, the separate contributions to the theory uncertainty due to the CT18 PDFs, NP corrections, and six-point scale variations are shown explicitly. Similar plots for all rapidity regions and observables can be found in Appendix app:supplementary-material. |
png pdf |
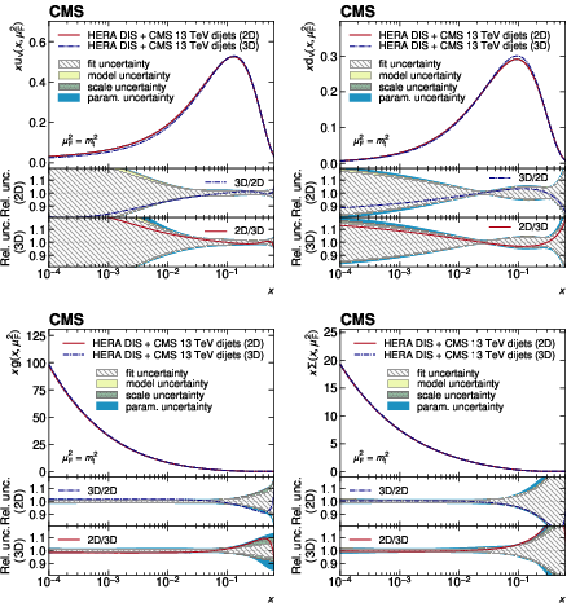
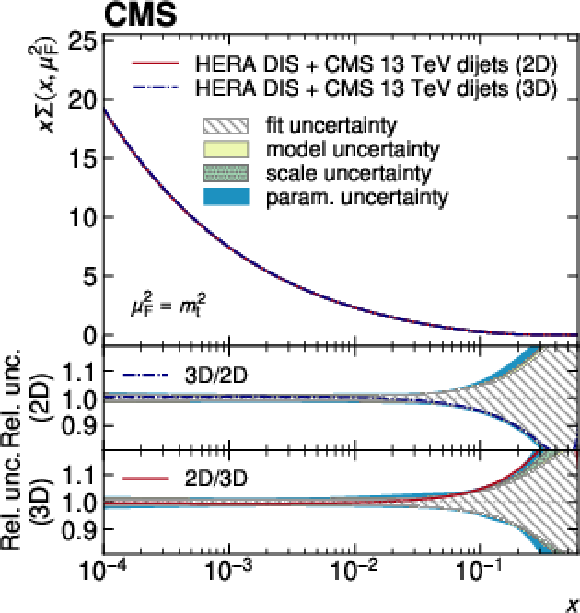
Figure 11:
Parton distributions obtained in a fit to HERA DIS data together with the CMS 2D or 3D dijet measurements. The top panels show the PDFs of the up and down valence quarks (upper row), of the gluon (lower left), and of the total sea quarks (lower right) as a function of the fractional parton momentum $ x $ at a factorization scale equal to the top quark mass. The middle (lower) panels show the relative uncertainty contributions obtained for the 2D (3D) fit, as well as the ratios of the fitted central values. |
png pdf |
Figure 11-a:
Parton distributions obtained in a fit to HERA DIS data together with the CMS 2D or 3D dijet measurements. The top panels show the PDFs of the up and down valence quarks (upper row), of the gluon (lower left), and of the total sea quarks (lower right) as a function of the fractional parton momentum $ x $ at a factorization scale equal to the top quark mass. The middle (lower) panels show the relative uncertainty contributions obtained for the 2D (3D) fit, as well as the ratios of the fitted central values. |
png pdf |
Figure 11-b:
Parton distributions obtained in a fit to HERA DIS data together with the CMS 2D or 3D dijet measurements. The top panels show the PDFs of the up and down valence quarks (upper row), of the gluon (lower left), and of the total sea quarks (lower right) as a function of the fractional parton momentum $ x $ at a factorization scale equal to the top quark mass. The middle (lower) panels show the relative uncertainty contributions obtained for the 2D (3D) fit, as well as the ratios of the fitted central values. |
png pdf |
Figure 11-c:
Parton distributions obtained in a fit to HERA DIS data together with the CMS 2D or 3D dijet measurements. The top panels show the PDFs of the up and down valence quarks (upper row), of the gluon (lower left), and of the total sea quarks (lower right) as a function of the fractional parton momentum $ x $ at a factorization scale equal to the top quark mass. The middle (lower) panels show the relative uncertainty contributions obtained for the 2D (3D) fit, as well as the ratios of the fitted central values. |
png pdf |
Figure 11-d:
Parton distributions obtained in a fit to HERA DIS data together with the CMS 2D or 3D dijet measurements. The top panels show the PDFs of the up and down valence quarks (upper row), of the gluon (lower left), and of the total sea quarks (lower right) as a function of the fractional parton momentum $ x $ at a factorization scale equal to the top quark mass. The middle (lower) panels show the relative uncertainty contributions obtained for the 2D (3D) fit, as well as the ratios of the fitted central values. |
png pdf |
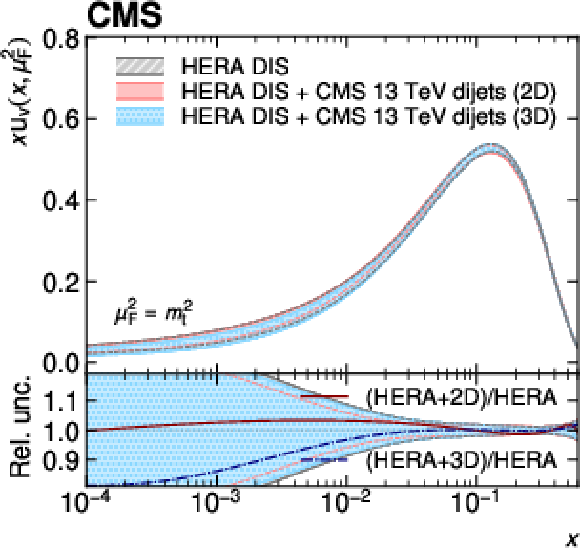
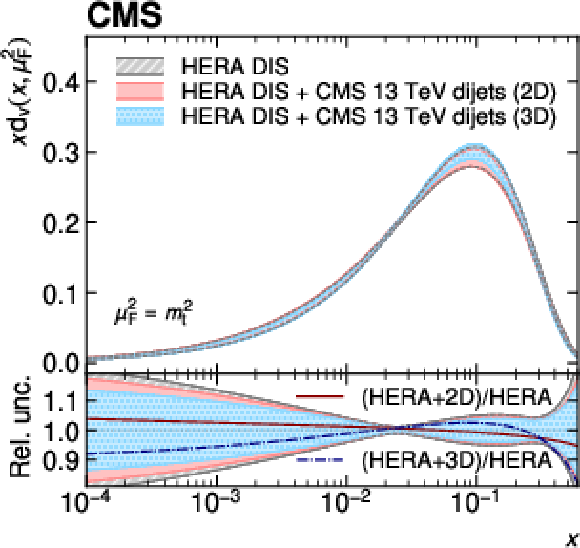
Figure 12:
Parton distributions obtained in a fit to HERA DIS data together with the CMS dijet data, compared to a fit to HERA DIS data alone. Shown are the PDFs of the up and down valence quarks (upper row), of the gluon (lower left), and of the total sea quarks (lower right) as a function of the fractional parton momentum $ x $ at a factorization scale equal to the top quark mass. The bands indicate the fit uncertainty and are shown in the lower panels as a relative uncertainty with respect to the corresponding central values. The lines in the lower panels show the ratios between the fitted central values. |
png pdf |
Figure 12-a:
Parton distributions obtained in a fit to HERA DIS data together with the CMS dijet data, compared to a fit to HERA DIS data alone. Shown are the PDFs of the up and down valence quarks (upper row), of the gluon (lower left), and of the total sea quarks (lower right) as a function of the fractional parton momentum $ x $ at a factorization scale equal to the top quark mass. The bands indicate the fit uncertainty and are shown in the lower panels as a relative uncertainty with respect to the corresponding central values. The lines in the lower panels show the ratios between the fitted central values. |
png pdf |
Figure 12-b:
Parton distributions obtained in a fit to HERA DIS data together with the CMS dijet data, compared to a fit to HERA DIS data alone. Shown are the PDFs of the up and down valence quarks (upper row), of the gluon (lower left), and of the total sea quarks (lower right) as a function of the fractional parton momentum $ x $ at a factorization scale equal to the top quark mass. The bands indicate the fit uncertainty and are shown in the lower panels as a relative uncertainty with respect to the corresponding central values. The lines in the lower panels show the ratios between the fitted central values. |
png pdf |
Figure 12-c:
Parton distributions obtained in a fit to HERA DIS data together with the CMS dijet data, compared to a fit to HERA DIS data alone. Shown are the PDFs of the up and down valence quarks (upper row), of the gluon (lower left), and of the total sea quarks (lower right) as a function of the fractional parton momentum $ x $ at a factorization scale equal to the top quark mass. The bands indicate the fit uncertainty and are shown in the lower panels as a relative uncertainty with respect to the corresponding central values. The lines in the lower panels show the ratios between the fitted central values. |
png pdf |
Figure 12-d:
Parton distributions obtained in a fit to HERA DIS data together with the CMS dijet data, compared to a fit to HERA DIS data alone. Shown are the PDFs of the up and down valence quarks (upper row), of the gluon (lower left), and of the total sea quarks (lower right) as a function of the fractional parton momentum $ x $ at a factorization scale equal to the top quark mass. The bands indicate the fit uncertainty and are shown in the lower panels as a relative uncertainty with respect to the corresponding central values. The lines in the lower panels show the ratios between the fitted central values. |
| Tables | |
png pdf |
Table 1:
Overview of the single-jet (dijet) triggers deployed for the different $ p_{\mathrm{T}} $ ($ \langle p_{\mathrm{T}} \rangle $) thresholds at the HLT, and the corresponding integrated luminosities. |
png pdf |
Table 2:
Nominal values and variations of parameters used to determine the PDF model uncertainty. Variations marked with an asterisk are in conflict with the requirement $ \mu_{\mathrm{F},\,0} < m_\mathrm{c} $ and thus cannot be used directly for the uncertainty estimation. Following Ref. [63], the results obtained for the opposite variation are symmetrized in these cases. |
png pdf |
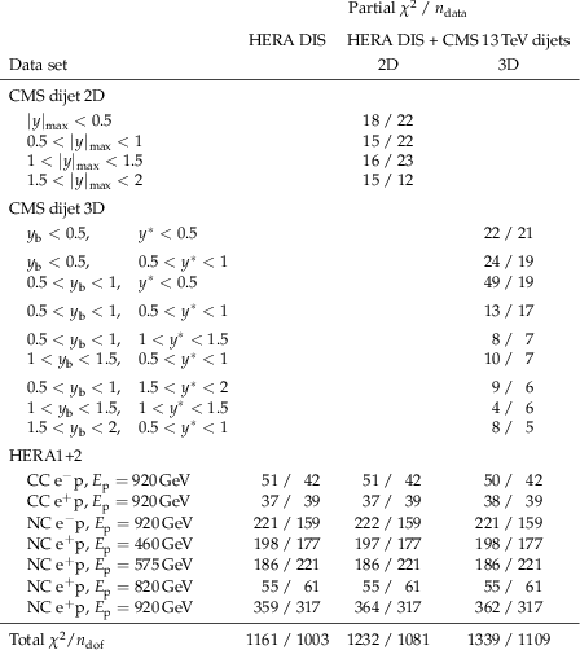
Table 3:
Goodness-of-fit values for the fits to the HERA DIS data alone, and together with the CMS dijet measurements, using the PDF parametrization given in Eq. 8. The table shows the partial $ \chi^2 $ values divided by the number of data points for the HERA DIS datasets and each of the dijet rapidity regions. The total $ \chi^2 $ value, divided by the number of degrees of freedom, is given at the bottom of the table. |
png pdf |
Table 4:
Goodness-of-fit values for the fits to the HERA DIS data alone, and together with the CMS dijet measurements, including all rapidity regions. The table shows the partial $ \chi^2 $ values divided by the number of data points for the HERA DIS datasets and each of the dijet rapidity regions. The total $ \chi^2 $ value, divided by the number of degrees of freedom, is given at the bottom of the table. |
| Summary |
| The dijet production cross section is measured based on pp collision data recorded by the CMS detector in 2016 at $ \sqrt{s} = $ 13 TeV, corresponding to an integrated luminosity of up to 36.3 fb$ ^{-1} $. The measurements are performed double-differentially (2D) as a function of the dijet invariant mass $ m_{1,2} $ in five regions of the maximal absolute rapidity $ |y|_{\text{max}} $\ of the two jets with the largest transverse momenta, and triple-differentially (3D) as a function of either $ m_{1,2} $ or the average transverse momentum $ \langle p_{\mathrm{T}} \rangle_{1,2} $ in 15 bins of the rapidity variables $ y^{*} $\ and $ y_{\text{b}} $. The latter two variables correspond to the rapidity separation of the two jets, and the total boost of the dijet system, respectively. All measurements are performed for jets clustered using the anti-$ k_{\mathrm{T}} $ jet algorithm with distance parameters $ R= $ 0.4 and 0.8, and the cross sections are unfolded in all measurement dimensions simultaneously to correct for detector effects. This is the first time that such a large set of multidifferential dijet measurements for two observables, $ \langle p_{\mathrm{T}} \rangle_{1,2} $ and $ m_{1,2} $, and two jet distance parameters, $ R = $ 0.4 and 0.8, is made available for comparison to theory and use in fits of the parton distribution functions (PDFs) of the proton. Predictions at next-to-next-to-leading order (NNLO) in perturbative quantum chromodynamics, supplemented with electroweak and nonperturbative corrections are observed to describe the data better for $ R = $ 0.8. Using the measurement of $ m_{1,2} $ for $ R = $ 0.8, the PDFs of the proton are determined simultaneously in fits to the dijet measurements together with deep-inelastic scattering data from the HERA experiments following the approach described in earlier HERAPDF analyses [1,2,63]. The results obtained from the double- and triple-differential measurements are compatible within the estimated uncertainties. The inclusion of either of the dijet measurements leads to an improved determination of the PDFs compared to fits to HERA data alone. In particular, the uncertainty in the gluon distribution at fractional proton momenta $ x > $ 0.1 is reduced, with the 3D dijet data providing tighter constraints at higher values of $ x $ compared to the 2D data. The strong coupling constant at the Z boson mass is determined simultaneously with the PDFs, yielding consistent results between the 2D and 3D dijet measurements, with the former resulting in the slightly more precise value of $ \alpha_\mathrm{S}(m_{\mathrm{Z}}) = $ 0.1179 $ \pm $ 0.0019 at NNLO. The impact of subleading-color contributions to the leading-color NNLO calculation used here is not yet known [42]. Apart from being useful as inputs to PDF fits or studies of jet size dependence, the present 2D and 3D measurements for two jet size parameters, $ R= $ 0.4 and 0.8, and for the two dijet observables $ m_{1,2} $ and $ \langle p_{\mathrm{T}} \rangle_{1,2} $, provide an ideal testing ground for further investigations. |
| Additional Figures | |
png pdf |
Additional Figure 1:
Response matrices for the 2D measurements as a function of $ |y|_{\text{max}} $ and $ m_{1,2} $ for jets with $ R = $ 0.4. The details correspond to those of Fig. 2. |
png pdf |
Additional Figure 2:
Partial response matrices for the 3D measurements as a function of $ m_{1,2} $ using jets with $ R = $ 0.4 (upper) and 0.8 (lower), shown here for the five rapidity regions with $ y_{\text{b}} < $ 0.5. The details correspond to those of Fig. 2. |
png pdf |
Additional Figure 2-a:
Partial response matrices for the 3D measurements as a function of $ m_{1,2} $ using jets with $ R = $ 0.4 (upper) and 0.8 (lower), shown here for the five rapidity regions with $ y_{\text{b}} < $ 0.5. The details correspond to those of Fig. 2. |
png pdf |
Additional Figure 2-b:
Partial response matrices for the 3D measurements as a function of $ m_{1,2} $ using jets with $ R = $ 0.4 (upper) and 0.8 (lower), shown here for the five rapidity regions with $ y_{\text{b}} < $ 0.5. The details correspond to those of Fig. 2. |
png pdf |
Additional Figure 3:
Partial response matrices for the 3D measurements as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ using jets with $ R = $ 0.4 (upper) and 0.8 (lower), shown here for the five rapidity regions with $ y_{\text{b}} < $ 0.5. The details correspond to those of Fig. 2. |
png pdf |
Additional Figure 3-a:
Partial response matrices for the 3D measurements as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ using jets with $ R = $ 0.4 (upper) and 0.8 (lower), shown here for the five rapidity regions with $ y_{\text{b}} < $ 0.5. The details correspond to those of Fig. 2. |
png pdf |
Additional Figure 3-b:
Partial response matrices for the 3D measurements as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ using jets with $ R = $ 0.4 (upper) and 0.8 (lower), shown here for the five rapidity regions with $ y_{\text{b}} < $ 0.5. The details correspond to those of Fig. 2. |
png pdf |
Additional Figure 4:
Overview of the 2D dijet cross section as a function of $ m_{1,2} $ in all 5 $ |y|_{\text{max}} $ regions, using jets with $ R = $ 0.4. The details correspond to those of Fig. 8. |
png pdf |
Additional Figure 5:
Overview of the 3D dijet cross section as a function of $ m_{1,2} $ in all 15 $ {(y^{*}\!, y_{\text{b}})} $ regions, using jets with $ R = $ 0.4 (left) and 0.8 (right). The details correspond to those of Fig. 8. |
png pdf |
Additional Figure 5-a:
Overview of the 3D dijet cross section as a function of $ m_{1,2} $ in all 15 $ {(y^{*}\!, y_{\text{b}})} $ regions, using jets with $ R = $ 0.4 (left) and 0.8 (right). The details correspond to those of Fig. 8. |
png pdf |
Additional Figure 5-b:
Overview of the 3D dijet cross section as a function of $ m_{1,2} $ in all 15 $ {(y^{*}\!, y_{\text{b}})} $ regions, using jets with $ R = $ 0.4 (left) and 0.8 (right). The details correspond to those of Fig. 8. |
png pdf |
Additional Figure 6:
Overview of the 3D dijet cross section as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ in all 15 $ {(y^{*}\!, y_{\text{b}})} $ regions, using jets with $ R = $ 0.8. The details correspond to those of Fig. 8. |
png pdf |
Additional Figure 7:
Breakdown of the experimental uncertainty for the 3D measurements as a function of $ m_{1,2} $ using jets with $ R = $ 0.4 (left) and 0.8 (right), in six out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 4. |
png pdf |
Additional Figure 7-a:
Breakdown of the experimental uncertainty for the 3D measurements as a function of $ m_{1,2} $ using jets with $ R = $ 0.4 (left) and 0.8 (right), in six out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 4. |
png pdf |
Additional Figure 7-b:
Breakdown of the experimental uncertainty for the 3D measurements as a function of $ m_{1,2} $ using jets with $ R = $ 0.4 (left) and 0.8 (right), in six out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 4. |
png pdf |
Additional Figure 8:
(continuation of Fig. 7) Breakdown of the experimental uncertainty for the 3D measurements as a function of $ m_{1,2} $ using jets with $ R = $ 0.4 (left) and 0.8 (right), in the remaining nine out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 4. |
png pdf |
Additional Figure 8-a:
(continuation of Fig. 7) Breakdown of the experimental uncertainty for the 3D measurements as a function of $ m_{1,2} $ using jets with $ R = $ 0.4 (left) and 0.8 (right), in the remaining nine out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 4. |
png pdf |
Additional Figure 8-b:
(continuation of Fig. 7) Breakdown of the experimental uncertainty for the 3D measurements as a function of $ m_{1,2} $ using jets with $ R = $ 0.4 (left) and 0.8 (right), in the remaining nine out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 4. |
png pdf |
Additional Figure 9:
Breakdown of the experimental uncertainty for the 3D measurements as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ using jets with $ R = $ 0.8, in six out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 4. |
png pdf |
Additional Figure 10:
(continuation of Fig. 9) Breakdown of the experimental uncertainty for the 3D measurements as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ using jets with $ R = $ 0.8, in the remaining nine out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 4. |
png pdf |
Additional Figure 11:
Comparison of the 2D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three inner $ |y|_{\text{max}} $ regions. The details correspond to those of Fig. 9. |
png pdf |
Additional Figure 11-a:
Comparison of the 2D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three inner $ |y|_{\text{max}} $ regions. The details correspond to those of Fig. 9. |
png pdf |
Additional Figure 11-b:
Comparison of the 2D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three inner $ |y|_{\text{max}} $ regions. The details correspond to those of Fig. 9. |
png pdf |
Additional Figure 11-c:
Comparison of the 2D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three inner $ |y|_{\text{max}} $ regions. The details correspond to those of Fig. 9. |
png pdf |
Additional Figure 11-d:
Comparison of the 2D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three inner $ |y|_{\text{max}} $ regions. The details correspond to those of Fig. 9. |
png pdf |
Additional Figure 11-e:
Comparison of the 2D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three inner $ |y|_{\text{max}} $ regions. The details correspond to those of Fig. 9. |
png pdf |
Additional Figure 11-f:
Comparison of the 2D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for three inner $ |y|_{\text{max}} $ regions. The details correspond to those of Fig. 9. |
png pdf |
Additional Figure 12:
(continuation of Fig. 11) Comparison of the 2D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for two outermost $ |y|_{\text{max}} $ regions. The details correspond to those of Fig. 9. |
png pdf |
Additional Figure 12-a:
(continuation of Fig. 11) Comparison of the 2D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for two outermost $ |y|_{\text{max}} $ regions. The details correspond to those of Fig. 9. |
png pdf |
Additional Figure 12-b:
(continuation of Fig. 11) Comparison of the 2D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for two outermost $ |y|_{\text{max}} $ regions. The details correspond to those of Fig. 9. |
png pdf |
Additional Figure 12-c:
(continuation of Fig. 11) Comparison of the 2D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for two outermost $ |y|_{\text{max}} $ regions. The details correspond to those of Fig. 9. |
png pdf |
Additional Figure 12-d:
(continuation of Fig. 11) Comparison of the 2D dijet cross section for jets with $ R = $ 0.4 (left) and 0.8 (right) as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, shown here for two outermost $ |y|_{\text{max}} $ regions. The details correspond to those of Fig. 9. |
png pdf |
Additional Figure 13:
Comparison of the 3D dijet cross section as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right), in six out of the total 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 10. |
png pdf |
Additional Figure 13-a:
Comparison of the 3D dijet cross section as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right), in six out of the total 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 10. |
png pdf |
Additional Figure 13-b:
Comparison of the 3D dijet cross section as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right), in six out of the total 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 10. |
png pdf |
Additional Figure 14:
(continuation of Fig. 13) Comparison of the 3D dijet cross section as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right), in the remaining nine out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 10. |
png pdf |
Additional Figure 14-a:
(continuation of Fig. 13) Comparison of the 3D dijet cross section as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right), in the remaining nine out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 10. |
png pdf |
Additional Figure 14-b:
(continuation of Fig. 13) Comparison of the 3D dijet cross section as a function of $ m_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right), in the remaining nine out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 10. |
png pdf |
Additional Figure 15:
Comparison of the 3D dijet cross section as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right), in six out of the total 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 10. |
png pdf |
Additional Figure 15-a:
Comparison of the 3D dijet cross section as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right), in six out of the total 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 10. |
png pdf |
Additional Figure 15-b:
Comparison of the 3D dijet cross section as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right), in six out of the total 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 10. |
png pdf |
Additional Figure 16:
(continuation of Fig. 15) Comparison of the 3D dijet cross section as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right), in the remaining nine out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 10. |
png pdf |
Additional Figure 16-a:
(continuation of Fig. 15) Comparison of the 3D dijet cross section as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right), in the remaining nine out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 10. |
png pdf |
Additional Figure 16-b:
(continuation of Fig. 15) Comparison of the 3D dijet cross section as a function of $ \langle p_{\mathrm{T}} \rangle_{1,2} $ to fixed-order theoretical calculations at NNLO, using jets with $ R = $ 0.4 (left) and 0.8 (right), in the remaining nine out of 15 $ {(y^{*}\!, y_{\text{b}})} $ bins. The details correspond to those of Fig. 10. |
| References | ||||
| 1 | H1 and ZEUS Collaborations | Combined measurement and QCD analysis of the inclusive $ \mathrm{e}^{\pm}\mathrm{p} $ scattering cross sections at HERA | JHEP 01 (2010) 109 | 0911.0884 |
| 2 | H1 and ZEUS Collaborations | Combination of measurements of inclusive deep inelastic $ \mathrm{e}^{\pm}\mathrm{p} $ scattering cross sections and QCD analysis of HERA data | EPJC 75 (2015) 580 | 1506.06042 |
| 3 | J. Currie et al. | Precise predictions for dijet production at the LHC | PRL 119 (2017) 152001 | 1705.10271 |
| 4 | A. Gehrmann-De Ridder et al. | Triple differential dijet cross section at the LHC | PRL 123 (2019) 102001 | 1905.09047 |
| 5 | CMS Collaboration | Measurement of the triple-differential dijet cross section in proton-proton collisions at $ \sqrt{s}= $ 8 TeV and constraints on parton distribution functions | EPJC 77 (2017) 746 | CMS-SMP-16-011 1705.02628 |
| 6 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_{\mathrm{T}} $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 7 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 8 | ATLAS Collaboration | Measurement of inclusive jet and dijet cross sections in proton-proton collisions at 7 TeV centre-of-mass energy with the ATLAS detector | EPJC 71 (2011) 1512 | 1009.5908 |
| 9 | CMS Collaboration | Measurement of the differential dijet production cross section in proton-proton collisions at $ \sqrt{s}= $ 7 TeV | PLB 700 (2011) 187 | CMS-QCD-10-025 1104.1693 |
| 10 | CMS Collaboration | Measurements of differential jet cross sections in proton-proton collisions at $ \sqrt{s}= $ 7 TeV with the CMS detector | PRD 87 (2013) 112002 | CMS-QCD-11-004 1212.6660 |
| 11 | ATLAS Collaboration | Measurement of dijet cross sections in pp collisions at 7 TeV centre-of-mass energy using the ATLAS detector | JHEP 05 (2014) 059 | 1312.3524 |
| 12 | ATLAS Collaboration | Measurement of inclusive jet and dijet cross-sections in proton-proton collisions at $ \sqrt{s}= $ 13 TeV with the ATLAS detector | JHEP 05 (2018) 195 | 1711.02692 |
| 13 | CMS Collaboration | HEPData record for this analysis | link | |
| 14 | CMS Collaboration | The CMS trigger system | JINST 12 (2017) P01020 | CMS-TRG-12-001 1609.02366 |
| 15 | CMS Collaboration | Performance of the CMS Level-1 trigger in proton-proton collisions at $ \sqrt{s}= $ 13 TeV | JINST 15 (2020) P10017 | CMS-TRG-17-001 2006.10165 |
| 16 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | |
| 17 | T. Sjöstrand et al. | An introduction to PYTHIA 8.2 | Comput. Phys. Commun. 191 (2015) 159 | 1410.3012 |
| 18 | CMS Collaboration | Event generator tunes obtained from underlying event and multiparton scattering measurements | EPJC 76 (2016) 155 | CMS-GEN-14-001 1512.00815 |
| 19 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 20 | GEANT4 Collaboration | GEANT 4---a simulation toolkit | NIM A 506 (2003) 250 | |
| 21 | CMS Collaboration | Measurement of the inelastic proton-proton cross section at $ \sqrt{s} = $ 13 TeV | JHEP 07 (2018) 161 | CMS-FSQ-15-005 1802.02613 |
| 22 | CMS Collaboration | Pileup mitigation at CMS in 13 TeV data | JINST 15 (2020) P09018 | CMS-JME-18-001 2003.00503 |
| 23 | CMS Collaboration | Particle-flow reconstruction and global event description with the CMS detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 24 | CMS Collaboration | Technical proposal for the Phase-II upgrade of the Compact Muon Solenoid | CMS Technical Proposal CERN-LHCC-2015-010, CMS-TDR-15-02, 2015 CDS |
|
| 25 | CMS Collaboration | Study of pileup removal algorithms for jets | CMS Physics Analysis Summary, 2014 CMS-PAS-JME-14-001 |
CMS-PAS-JME-14-001 |
| 26 | CMS Collaboration | Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV | JINST 12 (2017) P02014 | CMS-JME-13-004 1607.03663 |
| 27 | CMS Collaboration | Jet algorithms performance in 13 TeV data | CMS Physics Analysis Summary, 2017 CMS-PAS-JME-16-003 |
CMS-PAS-JME-16-003 |
| 28 | CMS Collaboration | Performance of missing transverse momentum reconstruction in proton-proton collisions at $ \sqrt{s}= $ 13 TeV using the CMS detector | JINST 14 (2019) P07004 | CMS-JME-17-001 1903.06078 |
| 29 | S. Schmitt | TUnfold: an algorithm for correcting migration effects in high energy physics | JINST 7 (2012) T10003 | 1205.6201 |
| 30 | CMS Collaboration | Jet energy scale and resolution performance with 13 TeV data collected by CMS in 2016--2018 | CMS Detector Performance Note CMS-DP-2020-019, 2020 CDS |
|
| 31 | CMS Collaboration | Precision luminosity measurement in proton-proton collisions at $ \sqrt{s} = $ 13 TeV in 2015 and 2016 at CMS | EPJC 81 (2021) 800 | CMS-LUM-17-003 2104.01927 |
| 32 | T. Gehrmann et al. | Jet cross sections and transverse momentume distributions with NNLOJET | in Proceedings of 13th International Symposium on Radiative Corrections (Applications of Quantum Field Theory to Phenomenology), 2017 PoS(RADCOR) 290 (2017) 074 |
1801.06415 |
| 33 | T. Kluge, K. Rabbertz, and M. Wobisch | fastNLO: Fast pQCD calculations for PDF fits | in Proc. 14th Intern. Workshop on Deep-Inelastic Scattering (DIS 2006), Tsukuba, Japan, 2006 link |
hep-ph/0609285 |
| 34 | D. Britzger, K. Rabbertz, F. Stober, and M. Wobisch | New features in version 2 of the fastNLO project | in Proc. 20th Intern. Workshop on Deep-Inelastic Scattering and Related Subjects, 2012 link |
1208.3641 |
| 35 | D. Britzger et al. | Calculations for deep inelastic scattering using fast interpolation grid techniques at NNLO in QCD and the extraction of $ \alpha_\text{s} $ from HERA data | EPJC 79 (2019) 845 | 1906.05303 |
| 36 | D. Britzger et al. | NNLO interpolation grids for jet production at the LHC | EPJC 82 (2022) 930 | 2207.13735 |
| 37 | J. Currie et al. | Jet cross sections at the LHC with NNLOJET | in Proceedings of Loops and Legs in Quantum Field Theory PoS(LL) 303 (2018) 001 |
1807.06057 |
| 38 | M. Cacciari et al. | The $ \mathrm{t} \overline{\mathrm{t}} $ cross-section at 1.8 TeV and 1.96 TeV: a study of the systematics due to parton densities and scale dependence | JHEP 04 (2004) 068 | hep-ph/0303085 |
| 39 | S. Catani, D. de Florian, M. Grazzini, and P. Nason | Soft-gluon resummation for Higgs boson production at hadron colliders | JHEP 07 (2003) 028 | hep-ph/0306211 |
| 40 | A. Banfi, G. P. Salam, and G. Zanderighi | Phenomenology of event shapes at hadron colliders | JHEP 06 (2010) 038 | 1001.4082 |
| 41 | J. Currie, E. W. N. Glover, and J. Pires | Next-to-next-to leading order QCD predictions for single jet inclusive production at the LHC | PRL 118 (2017) 072002 | 1611.01460 |
| 42 | X. Chen et al. | NNLO QCD corrections in full colour for jet production observables at the LHC | JHEP 09 (2022) 025 | 2204.10173 |
| 43 | CMS Collaboration | Measurement of the double-differential inclusive jet cross section in proton-proton collisions at $ \sqrt{s} = $ 13 TeV | EPJC 76 (2016) 451 | CMS-SMP-15-007 1605.04436 |
| 44 | CMS Collaboration | Investigations of the impact of the parton shower tuning in PYTHIA 8 in the modelling of $ \mathrm{t\overline{t}} $ at $ \sqrt{s}= $ 8 and 13 TeV | CMS Physics Analysis Summary, 2016 CMS-PAS-TOP-16-021 |
CMS-PAS-TOP-16-021 |
| 45 | M. Bähr et al. | HERWIG++ physics and manual | EPJC 58 (2008) 639 | 0803.0883 |
| 46 | M. H. Seymour and A. Siódmok | Constraining MPI models using $ \sigma_{\text{eff}} $ and recent Tevatron and LHC underlying event data | JHEP 10 (2013) 113 | 1307.5015 |
| 47 | P. Nason | A new method for combining NLO QCD with shower Monte Carlo algorithms | JHEP 11 (2004) 040 | hep-ph/0409146 |
| 48 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with parton shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 49 | S. Alioli, P. Nason, C. Oleari, and E. Re | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX | JHEP 06 (2010) 043 | 1002.2581 |
| 50 | S. Alioli et al. | Jet pair production in POWHEG | JHEP 04 (2011) 081 | 1012.3380 |
| 51 | J. Bellm et al. | HERWIG 7.0/ HERWIG++ 3.0 release note | EPJC 76 (2016) 196 | 1512.01178 |
| 52 | CMS Collaboration | Development and validation of HERWIG 7 tunes from CMS underlying-event measurements | EPJC 81 (2021) 312 | CMS-GEN-19-001 2011.03422 |
| 53 | S. Dittmaier, A. Huss, and C. Speckner | Weak radiative corrections to dijet production at hadron colliders | JHEP 11 (2012) 095 | 1210.0438 |
| 54 | A. Buckley et al. | LHAPDF6: parton density access in the LHC precision era | EPJC 75 (2015) 132 | 1412.7420 |
| 55 | S. Alekhin, J. Blümlein, S. Moch, and R. Plačakytė | Parton distribution functions, $ \alpha_\text{s} $, and heavy-quark masses for LHC Run II | PRD 96 (2017) 014011 | 1701.05838 |
| 56 | T.-J. Hou et al. | New CTEQ global analysis of quantum chromodynamics with high-precision data from the LHC | PRD 103 (2021) 014013 | 1912.10053 |
| 57 | S. Bailey et al. | Parton distributions from LHC, HERA, Tevatron and fixed target data: MSHT20 PDFs | EPJC 81 (2021) 341 | 2012.04684 |
| 58 | NNPDF Collaboration | Parton distributions from high-precision collider data | EPJC 77 (2017) 663 | 1706.00428 |
| 59 | CMS Collaboration | Measurement of the ratio of inclusive jet cross sections using the anti-$ k_{\mathrm{T}} $ algorithm with radius parameters $ {R} = $ 0.5 and 0.7 in pp collisions at $ \sqrt{s} $ = 7 TeV | PRD 90 (2014) 072006 | CMS-SMP-13-002 1406.0324 |
| 60 | ATLAS Collaboration | Measurement of the inclusive jet cross-sections in proton--proton collisions at $ \sqrt{s} = $ 8 TeV with the ATLAS detector | JHEP 09 (2017) 020 | 1706.03192 |
| 61 | CMS Collaboration | Measurement and QCD analysis of double-differential inclusive jet cross sections in proton-proton collisions at $ \sqrt{s} $ = 13 TeV | JHEP 02 (2022) 142 | CMS-SMP-20-011 2111.10431 |
| 62 | CMS Collaboration | Addendum to: Measurement and QCD analysis of double-differential inclusive jet cross sections in proton-proton collisions at $ \sqrt{s} $ = 13 TeV | JHEP 12 (2022) 035 | CMS-SMP-20-011 2111.10431 |
| 63 | H1 and ZEUS Collaborations | Impact of jet-production data on the next-to-next-to-leading-order determination of HERAPDF2.0 parton distributions | EPJC 82 (2022) 243 | 2112.01120 |
| 64 | J. Bellm et al. | Jet cross sections at the LHC and the quest for higher precision | EPJC 80 (2020) 93 | 1903.12563 |
| 65 | S. Alekhin et al. | HERAFitter: open source QCD fit project | EPJC 75 (2015) 304 | 1410.4412 |
| 66 | V. Bertone et al. | xFitter 2.0.0: An open source QCD fit framework | in Proceedings of XXV International Workshop on Deep-Inelastic Scattering and Related Subjects PoS(DIS) 297 (2017) 203 |
1709.01151 |
| 67 | Y. L. Dokshitzer | Calculation of the structure functions for deep inelastic scattering and e$ ^{+} $e$ ^{-} $ annihilation by perturbation theory in quantum chromodynamics | Sov. Phys. JETP 46 (1977) 641 | |
| 68 | V. N. Gribov and L. N. Lipatov | Deep inelastic ep scattering in perturbation theory | Sov. J. Nucl. Phys. 15 (1972) 438 | |
| 69 | G. Altarelli and G. Parisi | Asymptotic freedom in parton language | NPB 126 (1977) 298 | |
| 70 | M. Botje | QCDNUM: Fast QCD evolution and convolution | Comput. Phys. Commun. 182 (2011) 490 | 1005.1481 |
| 71 | R. S. Thorne and R. G. Roberts | Ordered analysis of heavy flavor production in deep inelastic scattering | PRD 57 (1998) 6871 | hep-ph/9709442 |
| 72 | R. S. Thorne | Variable-flavor number scheme for next-to-next-to-leading order | PRD 73 (2006) 054019 | hep-ph/0601245 |
| 73 | R. S. Thorne | Effect of changes of variable flavor number scheme on parton distribution functions and predicted cross sections | PRD 86 (2012) 074017 | 1201.6180 |
| 74 | W. T. Giele and S. Keller | Implications of hadron collider observables on parton distribution function uncertainties | PRD 58 (1998) 094023 | hep-ph/9803393 |
| 75 | W. T. Giele, S. A. Keller, and D. A. Kosower | Parton distribution function uncertainties | hep-ph/0104052 | |
| 76 | J. Pumplin et al. | Uncertainties of predictions from parton distribution functions. II. The Hessian method | PRD 65 (2001) 014013 | hep-ph/0101032 |
| 77 | Particle Data Group , R. L. Workman et al. | Review of particle physics | Prog. Theor. Exp. Phys. 2022 (2022) 083C01 | |
| 78 | CMS Collaboration | Measurement and QCD analysis of double-differential inclusive jet cross sections in pp collisions at $ \sqrt{s}= $ 8 TeV and cross section ratios to 2.76 and 7 TeV | JHEP 03 (2017) 156 | CMS-SMP-14-001 1609.05331 |
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|