Compact Muon Solenoid
LHC, CERN

Visit us: CMS Public Website, CMS Physics ; Contact us: CMS Publications Committee

CMS-HIG-20-018 ; CERN-EP-2022-010

Search for light Higgs bosons from supersymmetric cascade decays in pp collisions at $\sqrt s = $ 13 TeV

CMS Collaboration

28 April 2022

Eur. Phys. J. C 83 (2023) 571

Abstract: A search is reported for pairs of light Higgs bosons (H$_{1}$) produced in supersymmetric cascade decays in final states with small missing transverse momentum. A data set of LHC pp collisions collected with the CMS detector at $\sqrt s = $ 13 TeV and corresponding to an integrated luminosity of 138 fb$^{-1}$ is used. The search targets events where both H$_{1}$ bosons decay into $\mathrm{b\bar{b}}$ pairs that are reconstructed as large-radius jets using substructure techniques. No evidence is found for an excess of events beyond the background expectations of the standard model (SM). Results from the search are interpreted in the next-to-minimal supersymmetric extension of the SM, where a "singlino'' of small mass leads to squark and gluino cascade decays that can predominantly end in a highly Lorentz-boosted singlet-like H$_{1}$ and a singlino-like neutralino of small transverse momentum. Upper limits are set on the product of the squark or gluino pair production cross section and the square of the $\mathrm{b\bar{b}}$ branching fraction of the H$_{1}$ in a benchmark model containing almost mass-degenerate gluinos and light-flavour squarks. Under the assumption of an SM-like H$_{1}$ $\to\mathrm{b\bar{b}}$ branching fraction, H$_{1}$ bosons with masses in the range 40-120 GeV arising from the decays of squarks or gluinos with a mass of 1200 to 2500 GeV are excluded at 95% confidence level.

Links: e-print arXiv:2204.13532 [hep-ex] (PDF) ; CDS record ; inSPIRE record ; HepData record ; CADI line (restricted) ;

Figures
png pdf	Figure 1: Diagram of squark pair production and subsequent cascade decay in the benchmark signal model. The particle $\tilde{\chi}^{0}_{2}$ is the next-to-LSP, ${\tilde{\chi} _{\text {S}}^0}$ is the singlino-like LSP, and H$_{1}$ is the CP-even singlet-like Higgs boson.
png pdf	Figure 2: The ${H_{\mathrm {T}}}$ distribution in signal events with different values of ${m_{\text {SUSY}}}$, and in the simulated SM backgrounds, normalised to unit area. The uncertainties are statistical. All events satisfy the preselection.
png pdf	Figure 3: Distributions of simulated signal and multijet events in the 2D double-b tag discriminant plane, where the fractions of events in each bin are represented by the areas of the filled red and open blue squares, respectively. The signal parameters are $ {m_{\mathrm{H} _1}} = $ 70 GeV and $ {m_{\text {SUSY}}} = $ 2000 GeV. The kinematic selection is implemented with the masses of the two AK8 jets required to be within the set of signal and sideband mass regions defined in Section 5.2. The green, yellow, and orange shaded areas represent the tag region (TR), control region (CR), and validation region (VR), respectively. Of the plotted signal events, 65% fall within the TR.
png pdf	Figure 4: Map of mass regions used in the 2D soft-drop mass plane. The regions labelled $S_i$ are the signal mass regions, and the disjoint regions ${U_{i}}$ form the corresponding sidebands.
png pdf	Figure 5: The normalised distribution of events in the 2D soft-drop mass plane overlaid by the map of mass regions. The upper left, upper right, and middle left panels correspond to signal events for $ {m_{\text {SUSY}}} = $ 2000 GeV and ${m_{\mathrm{H} _1}}$ values of 40, 70, and 125 GeV, respectively. The panels at middle right, lower left, and lower right correspond to simulated multijet, ${\mathrm{t} {}\mathrm{\bar{t}}}$, and vector boson backgrounds, respectively. All events satisfy the TR requirement and the kinematic selection.
png pdf	Figure 5-a: The normalised distribution of events in the 2D soft-drop mass plane overlaid by the map of mass regions. The panel corresponds to signal events for $ {m_{\text {SUSY}}} = $ 2000 GeV and ${m_{\mathrm{H} _1}}$ values of 40 GeV. All events satisfy the TR requirement and the kinematic selection.
png pdf	Figure 5-b: The normalised distribution of events in the 2D soft-drop mass plane overlaid by the map of mass regions. The panel corresponds to signal events for $ {m_{\text {SUSY}}} = $ 2000 GeV and ${m_{\mathrm{H} _1}}$ values of 70 GeV. All events satisfy the TR requirement and the kinematic selection.
png pdf	Figure 5-c: The normalised distribution of events in the 2D soft-drop mass plane overlaid by the map of mass regions. The panel corresponds to signal events for $ {m_{\text {SUSY}}} = $ 2000 GeV and ${m_{\mathrm{H} _1}}$ values of 125 GeV. All events satisfy the TR requirement and the kinematic selection.
png pdf	Figure 5-d: The normalised distribution of events in the 2D soft-drop mass plane overlaid by the map of mass regions. The panel corresponds to the simulated multijet background. All events satisfy the TR requirement and the kinematic selection.
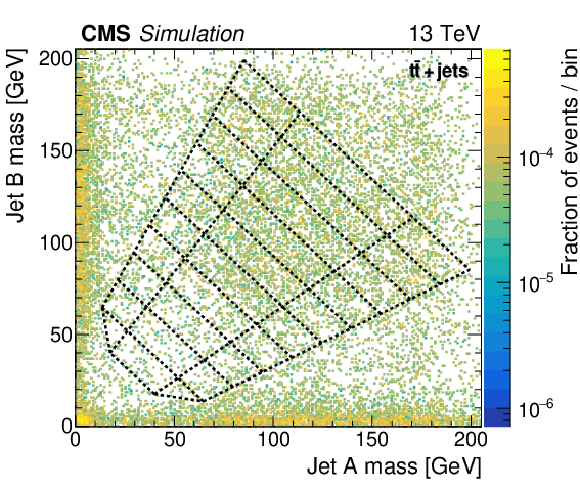
png pdf	Figure 5-e: The normalised distribution of events in the 2D soft-drop mass plane overlaid by the map of mass regions. The panel corresponds to the ${\mathrm{t} {}\mathrm{\bar{t}}}$ background. All events satisfy the TR requirement and the kinematic selection.
png pdf	Figure 5-f: The normalised distribution of events in the 2D soft-drop mass plane overlaid by the map of mass regions. The panel corresponds to the vector boson background. All events satisfy the TR requirement and the kinematic selection.
png pdf	Figure 6: Observed and expected yields in the TR for each of the 30 search regions, summed over the three data-taking years. The multijet background is estimated from data using the method described in Section 6, while the other backgrounds are simulated. Example signal distributions are shown for $ {m_{\mathrm{H} _1}} = $ 70 GeV and $ {m_{\text {SUSY}}} =$ 1200, 2000, and 2800 GeV. The error bars represent the statistical uncertainties and the hatched bands the systematic uncertainties.
png pdf	Figure 7: A comparison of the predicted and observed multijet yields in the validation region (VR), after subtraction of the other simulated backgrounds. The prediction is made separately for the three data-taking years, and the results are summed. The error bars on the data points represent their statistical uncertainties. The uncertainties in the predicted yields (statistical and systematic) are indicated by the hatched bands.
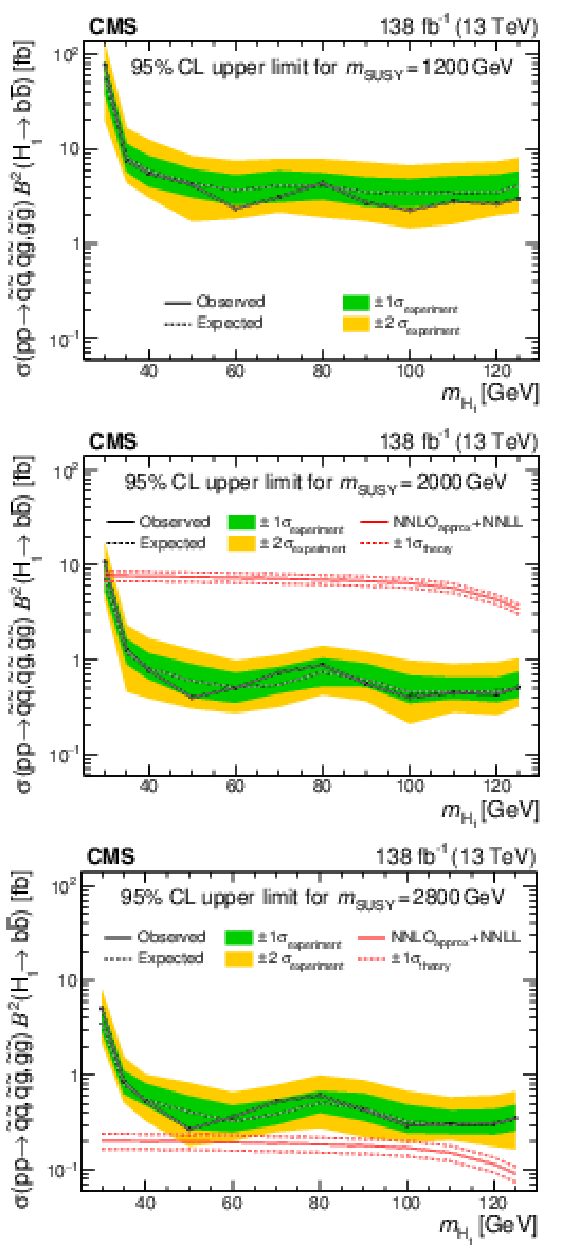
png pdf	Figure 8: Yields in all search regions after the background-only fit, summed over the three data-taking years. Example signal contributions used in the signal+background fits are shown for $ {m_{\text {SUSY}}} = $ 2200 GeV, and $ {m_{\mathrm{H} _1}} =$ 50, 90, and 125 GeV. The error bars represent the statistical uncertainties and the hatched bands the systematic uncertainties.
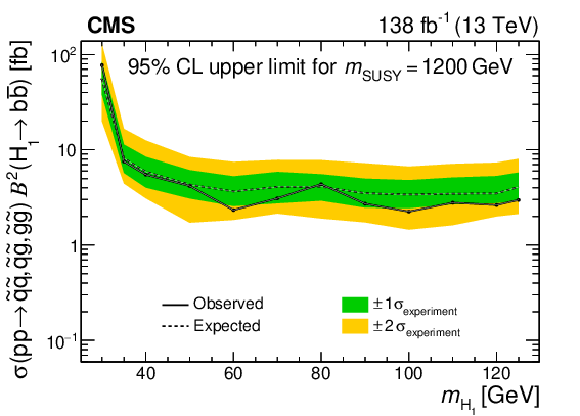
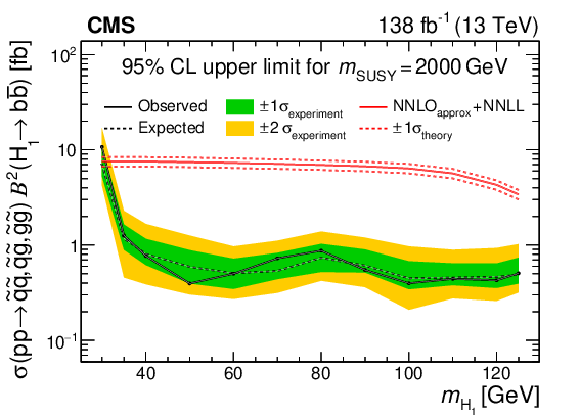
png pdf	Figure 9: Upper limits at 95% CL on $\sigma {\mathcal {B}} ^2$ as a function of ${m_{\mathrm{H} _1}}$, for ${m_{\text {SUSY}}}$ values of 1200 (upper), 2000 (middle), and 2800 GeV (lower). The solid and dashed black lines indicate the observed and median expected limits, respectively. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The solid and dashed red lines show the theoretical value of $\sigma {\mathcal {B}} ^2$ and its uncertainty [21-30]. In the upper plot, these $\sigma {\mathcal {B}} ^2$ values are beyond the maximum of the vertical axis.
png pdf	Figure 9-a: Upper limits at 95% CL on $\sigma {\mathcal {B}} ^2$ as a function of ${m_{\mathrm{H} _1}}$, for ${m_{\text {SUSY}}}$ values of 1200 GeV. The solid and dashed black lines indicate the observed and median expected limits, respectively. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The solid and dashed red lines show the theoretical value of $\sigma {\mathcal {B}} ^2$ and its uncertainty [21-30]. These $\sigma {\mathcal {B}} ^2$ values are beyond the maximum of the vertical axis.
png pdf	Figure 9-b: Upper limits at 95% CL on $\sigma {\mathcal {B}} ^2$ as a function of ${m_{\mathrm{H} _1}}$, for ${m_{\text {SUSY}}}$ values of 2000 GeV. The solid and dashed black lines indicate the observed and median expected limits, respectively. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The solid and dashed red lines show the theoretical value of $\sigma {\mathcal {B}} ^2$ and its uncertainty [21-30].
png pdf	Figure 9-c: Upper limits at 95% CL on $\sigma {\mathcal {B}} ^2$ as a function of ${m_{\mathrm{H} _1}}$, for ${m_{\text {SUSY}}}$ values of 2800 GeV. The solid and dashed black lines indicate the observed and median expected limits, respectively. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The solid and dashed red lines show the theoretical value of $\sigma {\mathcal {B}} ^2$ and its uncertainty [21-30].
png pdf	Figure 10: The observed 95% CL upper limit on $\sigma {\mathcal {B}} ^2 / (\sigma {\mathcal {B}} ^2)_{\textrm {theory}}$, quantified by the colour scale as a function of ${m_{\mathrm{H} _1}}$ and ${m_{\text {SUSY}}}$. The solid and dashed red lines indicate the observed excluded region and its theoretical uncertainty, respectively. The solid and dashed black lines respectively represent the expected excluded region and its 68% CL interval, under the background-only hypothesis.
png pdf	Figure A1: The observed and expected 95% CL upper limit on the product of $\sigma {\mathcal {B}} ^2$ and ${A_{\text {kin}}}$, the kinematic acceptance and efficiency for the $ {H_{\mathrm {T}}} > $ 3500 GeV region, as a function of ${m_{\mathrm{H} _1}}$. The results are independent of ${m_{\text {SUSY}}}$ within 10% in the range 1600 $ < {m_{\text {SUSY}}} < $ 2800 GeV. The solid and dashed black lines indicate the observed and median expected limits, respectively. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis.

Tables
png pdf	Table 1: Inclusive pair-production cross sections calculated at approximately NNLO and NNLL in ${\alpha _\mathrm {S}}$ [21-29] for squark mass ${m_{\text {SUSY}}}$ and gluino mass 1% larger. The quoted uncertainty is obtained from variations in the choice of scales, parton distribution functions, and ${\alpha _\mathrm {S}}$.
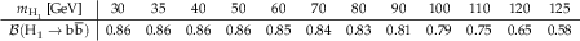
png pdf	Table 2: The ${m_{\mathrm{H} _1}}$ values in this search and corresponding H$_{1}$ $\to\mathrm{b\bar{b}}$ branching fractions.
png pdf	Table A1: Reference values of the product of kinematic acceptance and efficiency (${A_{\text {kin}}}$) for the $ {H_{\mathrm {T}}} > $ 3500 GeV region for the benchmark signal model with different values of ${m_{\text {SUSY}}}$. These values are independent of ${m_{\mathrm{H} _1}}$ within 2% in the range 30 $ < {m_{\mathrm{H} _1}} < $ 125 GeV.

Summary

This paper presents a search for pairs of light Higgs bosons (H$_{1}$) produced in supersymmetric cascade decays. The targeted final states have small amounts of missing transverse momentum and two H$_{1}$ $\to\mathrm{b\bar{b}}$ decays that are reconstructed as large-radius jets using substructure techniques. The search is based on data from pp collisions collected by the CMS experiment at $\sqrt s = $ 13 TeV during 2016-2018, corresponding to an integrated luminosity of 138 fb$^{-1}$. With no evidence found for an excess of events beyond the background expectations of the standard model (SM), the results are interpreted in the next-to-minimal supersymmetric extension of the SM (NMSSM), where a "singlino'' of small mass leads to squark and gluino cascade decays that can predominantly end in a highly Lorentz-boosted singlet-like H$_{1}$ and a singlino-like neutralino of small transverse momentum. Upper limits are set on the product of the production cross section and the square of the $\mathrm{b\bar{b}}$ branching fraction of the H$_{1}$ for an NMSSM benchmark model with almost mass-degenerate gluinos and light-flavour squarks and branching fractions of unity for the cascade decays ending with the H$_{1}$. Under the assumption of an SM-like H$_{1}$ $\to\mathrm{b\bar{b}}$ branching fraction, H$_{1}$ bosons with masses in the range 40-120 GeV, arising from the decays of squarks or gluinos with a mass from 1200 to 2500 GeV, are excluded at 95% confidence level.

Additional Figures
png pdf	Additional Figure 1: The normalised distribution of signal events in the 2D soft-drop mass plane, overlaid by the map of mass regions. The signal parameters are $ {m_{\text {SUSY}}} = $ 2000 GeV and $ {m_{\mathrm{H} _1}} = $ 30 GeV. All events satisfy the TR requirement and the kinematic selection.
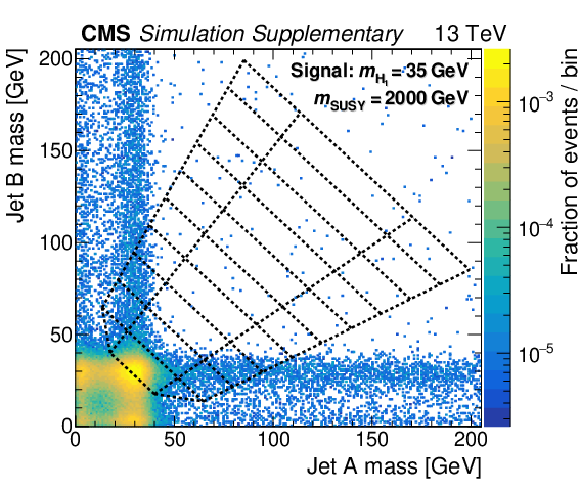
png pdf	Additional Figure 2: The normalised distribution of signal events in the 2D soft-drop mass plane, overlaid by the map of mass regions. The signal parameters are $ {m_{\text {SUSY}}} = $ 2000 GeV and $ {m_{\mathrm{H} _1}} = $ 35 GeV. All events satisfy the TR requirement and the kinematic selection.
png pdf	Additional Figure 3: The normalised distribution of signal events in the 2D soft-drop mass plane, overlaid by the map of mass regions. The signal parameters are $ {m_{\text {SUSY}}} = $ 2000 GeV and $ {m_{\mathrm{H} _1}} = $ 50 GeV. All events satisfy the TR requirement and the kinematic selection.
png pdf	Additional Figure 4: The normalised distribution of signal events in the 2D soft-drop mass plane, overlaid by the map of mass regions. The signal parameters are $ {m_{\text {SUSY}}} = $ 2000 GeV and $ {m_{\mathrm{H} _1}} = $ 90 GeV. All events satisfy the TR requirement and the kinematic selection.
png pdf	Additional Figure 5: The normalised distribution of signal events in the 2D soft-drop mass plane, overlaid by the map of mass regions. The signal parameters are $ {m_{\text {SUSY}}} = $ 2000 GeV and $ {m_{\mathrm{H} _1}} = $ 110 GeV. All events satisfy the TR requirement and the kinematic selection.
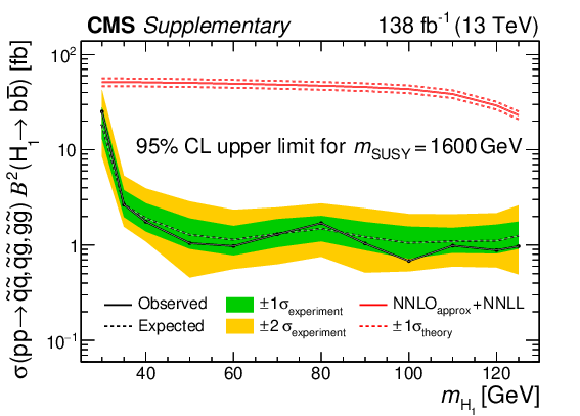
png pdf	Additional Figure 6: Upper limits at 95% CL on $\sigma \mathcal {B}^2$ as a function of ${m_{\mathrm{H} _1}}$, for $ {m_{\text {SUSY}}} = $ 1600 GeV. The solid and dashed black lines indicate the observed and median expected limits, respectively. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The solid and dashed red lines show the theoretical value of $\sigma \mathcal {B}^2$ and its uncertainty.
png pdf	Additional Figure 7: Upper limits at 95% CL on $\sigma \mathcal {B}^2$ as a function of ${m_{\mathrm{H} _1}}$, for $ {m_{\text {SUSY}}} = $ 2200 GeV. The solid and dashed black lines indicate the observed and median expected limits, respectively. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The solid and dashed red lines show the theoretical value of $\sigma \mathcal {B}^2$ and its uncertainty.
png pdf	Additional Figure 8: Upper limits at 95% CL on $\sigma \mathcal {B}^2$ as a function of ${m_{\mathrm{H} _1}}$, for $ {m_{\text {SUSY}}} = $ 2400 GeV. The solid and dashed black lines indicate the observed and median expected limits, respectively. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The solid and dashed red lines show the theoretical value of $\sigma \mathcal {B}^2$ and its uncertainty.
png pdf	Additional Figure 9: Upper limits at 95% CL on $\sigma \mathcal {B}^2$ as a function of ${m_{\mathrm{H} _1}}$, for $ {m_{\text {SUSY}}} = $ 2600 GeV. The solid and dashed black lines indicate the observed and median expected limits, respectively. The inner (green) band and the outer (yellow) band indicate the regions containing 68 and 95%, respectively, of the distribution of limits expected under the background-only hypothesis. The solid and dashed red lines show the theoretical value of $\sigma \mathcal {B}^2$ and its uncertainty.

References
1	P. Ramond	Dual theory for free fermions	PRD 3 (1971) 2415
2	Y. A. Golfand and E. P. Likhtman	Extension of the algebra of Poincaré group generators and violation of P invariance	JEPTL 13 (1971)323
3	A. Neveu and J. H. Schwarz	Factorizable dual model of pions	NPB 31 (1971) 86
4	D. V. Volkov and V. P. Akulov	Possible universal neutrino interaction	JEPTL 16 (1972)438
5	J. Wess and B. Zumino	A Lagrangian model invariant under supergauge transformations	PLB 49 (1974) 52
6	J. Wess and B. Zumino	Supergauge transformations in four dimensions	NPB 70 (1974) 39
7	P. Fayet	Supergauge invariant extension of the Higgs mechanism and a model for the electron and its neutrino	NPB 90 (1975) 104
8	H. P. Nilles	Supersymmetry, supergravity and particle physics	Phys. Rep. 110 (1984) 1
9	U. Ellwanger, C. Hugonie, and A. M. Teixeira	The Next-to-Minimal Supersymmetric Standard Model	Phys. Repts. 496 (2010) 1	0910.1785
10	U. Ellwanger and A. Teixeira	NMSSM with a singlino LSP: possible challenges for searches for supersymmetry at the LHC	JHEP 10 (2014) 113	1406.7221
11	U. Ellwanger and A. Teixeira	Excessive Higgs pair production with little MET from squarks and gluinos in the NMSSM	JHEP 04 (2015) 172	1412.6394
12	A. Titterton et al.	Exploring sensitivity to NMSSM signatures with low missing transverse energy at the LHC	JHEP 10 (2018) 064	1807.10672
13	CMS Collaboration	Search for physics beyond the standard model in events with high-momentum Higgs bosons and missing transverse momentum in proton-proton collisions at 13 TeV	PRL 120 (2018) 241801	CMS-SUS-17-006 1712.08501
14	CMS Collaboration	Search for higgsinos in channels with two Higgs bosons and missing transverse momentum in proton-proton collisions at $ \sqrt{s} = $ 13 TeV	2021. Submitted to JHEP	CMS-SUS-20-004 2201.04206
15	ATLAS Collaboration	Search for pair production of higgsinos in final states with at least three $ b $-tagged jets in $ \sqrt{s} = $ 13 TeV pp collisions using the ATLAS detector	PRD 98 (2018) 092002	1806.04030
16	CMS Collaboration	Searches for electroweak neutralino and chargino production in channels with Higgs, Z, and W bosons in pp collisions at 8 TeV	PRD 90 (2014) 092007	CMS-SUS-14-002 1409.3168
17	CMS Collaboration	Search for higgsino pair production in $ {\mathrm{p}}{\mathrm{p}} $ collisions at $ \sqrt{s} = $ 13 TeV in final states with large missing transverse momentum and two Higgs bosons decaying via $ \mathrm{H} \to \mathrm{b\bar{b}} $	PRD 97 (2018) 032007	CMS-SUS-16-044 1709.04896
18	CMS Collaboration	Precision luminosity measurement in proton-proton collisions at $ \sqrt{s} = $ 13 TeV in 2015 and 2016 at CMS	EPJC 81 (2021) 800	CMS-LUM-17-003 2104.01927
19	CMS Collaboration	CMS luminosity measurement for the 2017 data-taking period at $ \sqrt{s} = $ 13 TeV	CMS-PAS-LUM-17-004	CMS-PAS-LUM-17-004
20	CMS Collaboration	CMS luminosity measurement for the 2018 data-taking period at $ \sqrt{s} = $ 13 TeV	CMS-PAS-LUM-18-002	CMS-PAS-LUM-18-002
21	W. Beenakker, R. Hopker, M. Spira, and P. M. Zerwas	Squark and gluino production at hadron colliders	NPB 492 (1997) 51	hep-ph/9610490
22	A. Kulesza and L. Motyka	Threshold resummation for squark-antisquark and gluino-pair production at the LHC	PRL 102 (2009) 111802	0807.2405
23	A. Kulesza and L. Motyka	Soft gluon resummation for the production of gluino-gluino and squark-antisquark pairs at the LHC	PRD 80 (2009) 095004	0905.4749
24	W. Beenakker et al.	Soft-gluon resummation for squark and gluino hadroproduction	JHEP 12 (2009) 041	0909.4418
25	W. Beenakker et al.	Squark and gluino hadroproduction	Int. J. Mod. Phys. A 26 (2011) 2637	1105.1110
26	W. Beenakker et al.	NNLL resummation for squark-antisquark pair production at the LHC	JHEP 01 (2012) 076	1110.2446
27	W. Beenakker et al.	Towards NNLL resummation: hard matching coefficients for squark and gluino hadroproduction	JHEP 10 (2013) 120	1304.6354
28	W. Beenakker et al.	NNLL resummation for squark and gluino production at the LHC	JHEP 12 (2014) 023	1404.3134
29	W. Beenakker et al.	NNLL-fast: predictions for coloured supersymmetric particle production at the LHC with threshold and Coulomb resummation	JHEP 12 (2016) 133	1607.07741
30	A. Djouadi, J. Kalinowski, and M. Spira	HDECAY: a program for Higgs boson decays in the standard model and its supersymmetric extension	CPC 108 (1998) 56	hep-ph/9704448
31	A. Djouadi, J. Kalinowski, M. Muhlleitner, and M. Spira	HDECAY: Twenty++ years after	CPC 238 (2019) 214	1801.09506
32	CMS Collaboration	Performance of the CMS Level-1 trigger in proton-proton collisions at $ \sqrt{s} = $ 13 TeV	JINST 15 (2020) P10017	CMS-TRG-17-001 2006.10165
33	CMS Collaboration	The CMS trigger system	JINST 12 (2017) P01020	CMS-TRG-12-001 1609.02366
34	CMS Collaboration	The CMS experiment at the CERN LHC	JINST 3 (2008) S08004	CMS-00-001
35	J. Alwall et al.	The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations	JHEP 07 (2014) 079	1405.0301
36	J. Alwall et al.	Comparative study of various algorithms for the merging of parton showers and matrix elements in hadronic collisions	EPJC 53 (2008) 473	0706.2569
37	S. Frixione, P. Nason, and G. Ridolfi	A positive-weight next-to-leading-order Monte Carlo for heavy flavour hadroproduction	JHEP 09 (2007) 126	0707.3088
38	P. Nason	A new method for combining NLO QCD with shower Monte Carlo algorithms	JHEP 11 (2004) 040	hep-ph/0409146
39	S. Frixione, P. Nason, and C. Oleari	Matching NLO QCD computations with parton shower simulations: the POWHEG method	JHEP 11 (2007) 070	0709.2092
40	S. Alioli, P. Nason, C. Oleari, and E. Re	A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX	JHEP 06 (2010) 043	1002.2581
41	NNPDF Collaboration	Unbiased global determination of parton distributions and their uncertainties at NNLO and LO	NPB 855 (2012) 153	1107.2652
42	NNPDF Collaboration	Parton distributions for the LHC Run II	JHEP 04 (2015) 040	1410.8849
43	NNPDF Collaboration	Parton distributions with QED corrections	NPB 877 (2013) 290	1308.0598
44	NNPDF Collaboration	Parton distributions from high-precision collider data	EPJC 77 (2017) 663	1706.00428
45	T. Sjostrand et al.	An introduction to PYTHIA 8.2	CPC 191 (2015) 159	1410.3012
46	CMS Collaboration	Event generator tunes obtained from underlying event and multiparton scattering measurements	EPJC 76 (2016) 155	CMS-GEN-14-001 1512.00815
47	P. Skands, S. Carrazza, and J. Rojo	Tuning PYTHIA 8.1: the Monash 2013 tune	EPJC 74 (2014) 3024	1404.5630
48	CMS Collaboration	Extraction and validation of a new set of CMS PYTHIA 8 tunes from underlying-event measurements	EPJC 80 (2020) 4	CMS-GEN-17-001 1903.12179
49	M. Czakon and A. Mitov	TOP++: a program for the calculation of the top-pair cross-section at hadron colliders	CPC 185 (2014) 2930	1112.5675
50	R. Gavin, Y. Li, F. Petriello, and S. Quackenbush	FEWZ 2.0: A code for hadronic Z production at next-to-next-to-leading order	CPC 182 (2011) 2388	1011.3540
51	R. Gavin, Y. Li, F. Petriello, and S. Quackenbush	W physics at the LHC with FEWZ 2.1	CPC 184 (2013) 208	1201.5896
52	Y. Li and F. Petriello	Combining QCD and electroweak corrections to dilepton production in FEWZ	PRD 86 (2012) 094034	1208.5967
53	CMS Collaboration	Measurement of the inelastic proton-proton cross section at $ \sqrt{s}= $ 13 TeV	JHEP 07 (2018) 161	CMS-FSQ-15-005 1802.02613
54	GEANT4 Collaboration	GEANT4--a simulation toolkit	NIMA 506 (2003) 250
55	CMS Collaboration	Particle-flow reconstruction and global event description with the CMS detector	JINST 12 (2017) P10003	CMS-PRF-14-001 1706.04965
56	M. Cacciari, G. P. Salam, and G. Soyez	The anti-$ {k_{\mathrm{T}}} $ jet clustering algorithm	JHEP 04 (2008) 063	0802.1189
57	M. Cacciari, G. P. Salam, and G. Soyez	FastJet user manual	EPJC 72 (2012) 1896	1111.6097
58	CMS Collaboration	Technical proposal for the Phase-II upgrade of the Compact Muon Solenoid	CMS-PAS-TDR-15-002	CMS-PAS-TDR-15-002
59	CMS Collaboration	Pileup mitigation at CMS in 13 TeV data	JINST 15 (2020) P09018	CMS-JME-18-001 2003.00503
60	D. Bertolini, P. Harris, M. Low, and N. Tran	Pileup Per Particle Identification	JHEP 10 (2014) 059	1407.6013
61	CMS Collaboration	Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV	JINST 12 (2017) P02014	CMS-JME-13-004 1607.03663
62	CMS Collaboration	Jet energy scale and resolution performance with 13 TeV data collected by CMS in 2016	CDS
63	CMS Collaboration	Jet performance in pp collisions at $ \sqrt{s}= $ 7 TeV	CMS-PAS-JME-10-003
64	CMS Collaboration	Jet algorithms performance in 13 TeV data	CMS-PAS-JME-16-003	CMS-PAS-JME-16-003
65	CMS Collaboration	Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV	JINST 13 (2018) P05011	CMS-BTV-16-002 1712.07158
66	T. Adams et al.	Beam test evaluation of electromagnetic calorimeter modules made from proton-damaged PbWO$ _4 $ crystals	JINST 11 (2016) P04012
67	A. J. Larkoski, S. Marzani, G. Soyez, and J. Thaler	Soft Drop	JHEP 05 (2014) 146	1402.2657
68	ATLAS and CMS Collaborations	Procedure for the LHC Higgs boson search combination in summer 2011	CMS-NOTE-2011-005
69	T. Junk	Confidence level computation for combining searches with small statistics	NIMA 434 (1999) 435	hep-ex/9902006
70	A. L. Read	Presentation of search results: The CLs technique	JPG 28 (2002) 2693
71	CMS Collaboration	HEPData record for this analysis	link

Compact Muon Solenoid LHC, CERN

© Copyright CERN 2024 for the benefit of the CMS Collaboration