

Compact Muon Solenoid
LHC, CERN
| CMS-EXO-17-016 ; CERN-EP-2018-272 | ||
| Search for heavy neutrinos and third-generation leptoquarks in hadronic states of two τ leptons and two jets in proton-proton collisions at √s= 13 TeV | ||
| CMS Collaboration | ||
| 2 November 2018 | ||
| JHEP 03 (2019) 170 | ||
| Abstract: A search for new particles has been conducted using events with two high transverse momentum τ leptons that decay hadronically and at least two energetic jets. The analysis is performed using data from proton-proton collisions at √s= 13 TeV, collected by the CMS experiment at the LHC in 2016 and corresponding to an integrated luminosity of 35.9 fb−1. The observed data are consistent with standard model expectations. The results are interpreted in the context of two physics models. The first model involves right-handed charged bosons, WR, that decay to heavy right-handed Majorana neutrinos, Nℓ (ℓ=e, μ, τ), arising in a left-right symmetric extension of the standard model. The model considers that Ne and Nμ are too heavy to be detected at the LHC. Assuming that the Nτ mass is half of the WR mass, masses of the WR boson below 3.50 TeV are excluded at 95% confidence level. Exclusion limits are also presented considering different scenarios for the mass ratio between Nτ and WR, as a function of WR mass. In the second model, pair production of third-generation scalar leptoquarks that decay into ττbb is considered, resulting in an observed exclusion region with leptoquark masses below 1.02 TeV, assuming a 100% branching fraction for the leptoquark decay to a τ lepton and a bottom quark. These results represent the most stringent limits to date on these models. | ||
| Links: e-print arXiv:1811.00806 [hep-ex] (PDF) ; CDS record ; inSPIRE record ; CADI line (restricted) ; | ||
| Figures | |
png pdf |
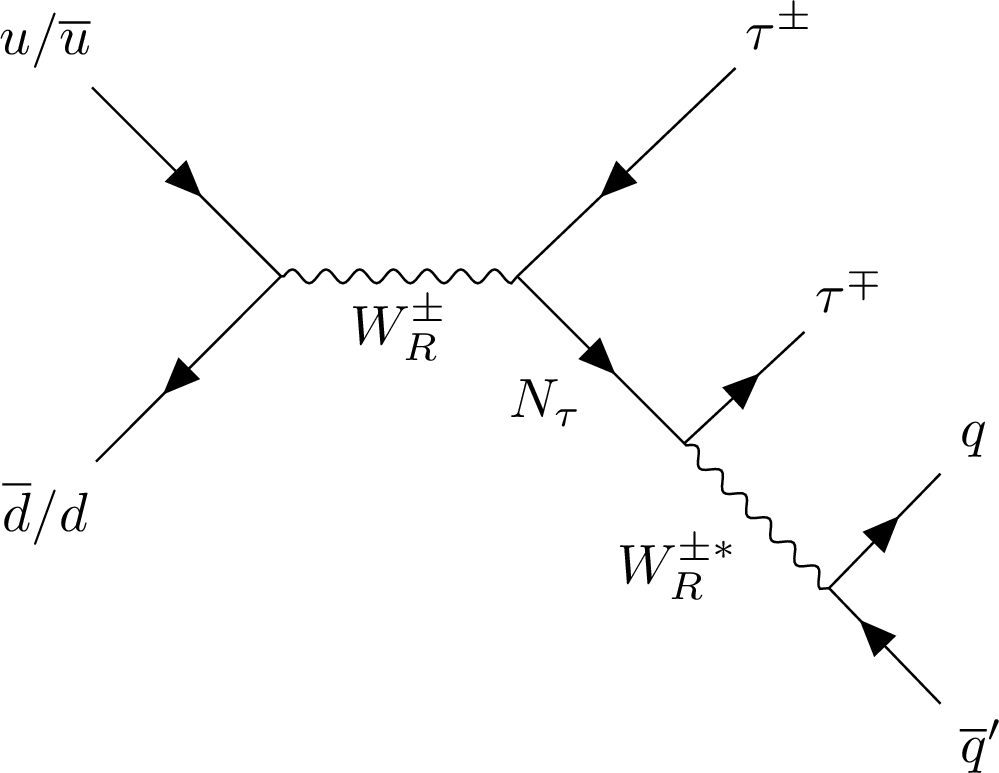
Figure 1:
Leading order Feynman diagram for the production of a right-handed WR that decays to a heavy neutrino Nτ, with a final state of two τ leptons and two jets. |
png pdf |
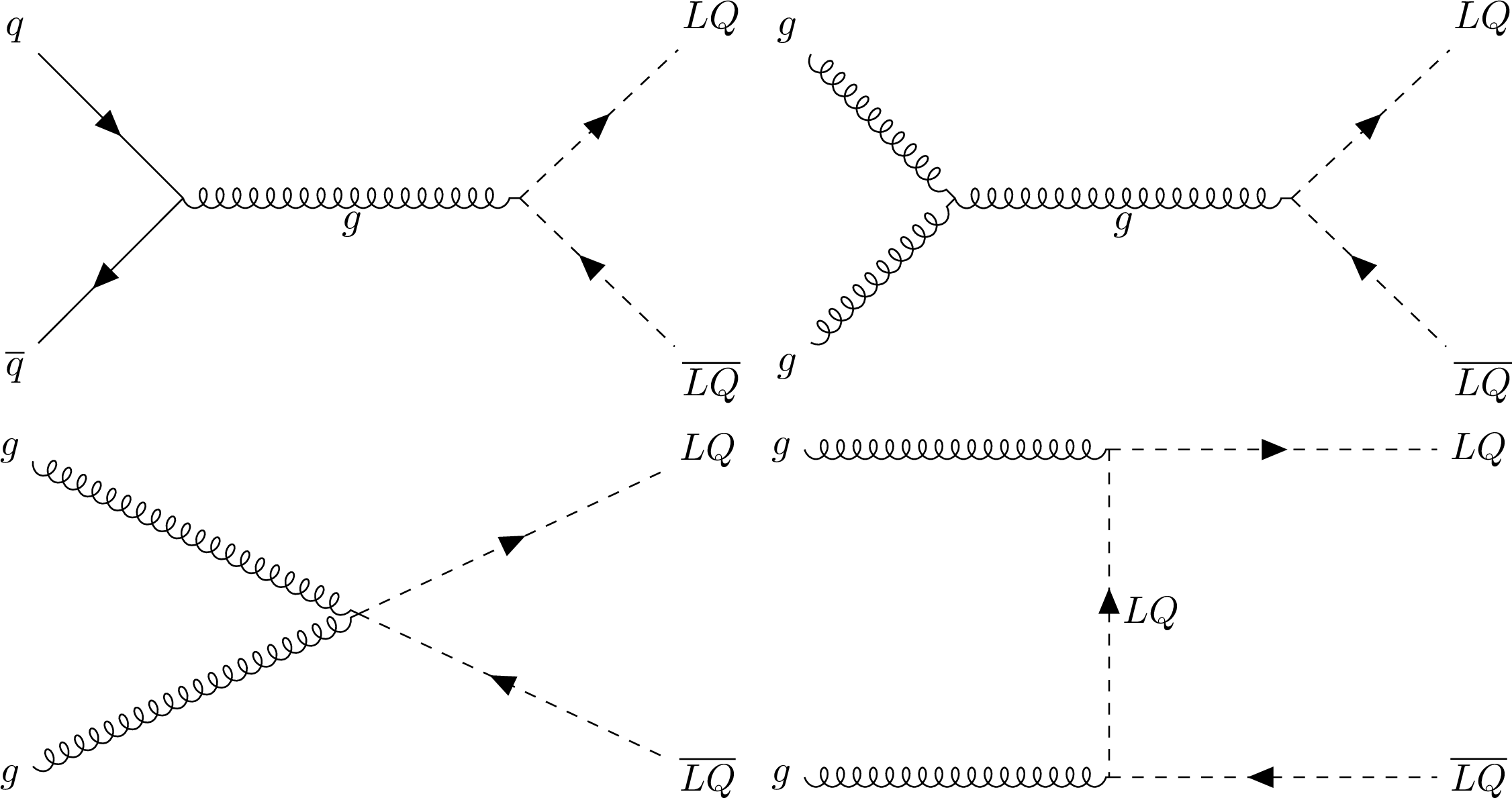
Figure 2:
Leading order Feynman diagrams for the pair-production of LQs, leading to final states with two τ leptons and two b quarks. |

png pdf |
Figure 2-a:
Leading order Feynman diagram for the pair-production of LQs, leading to final states with two τ leptons and two b quarks. |
png pdf |
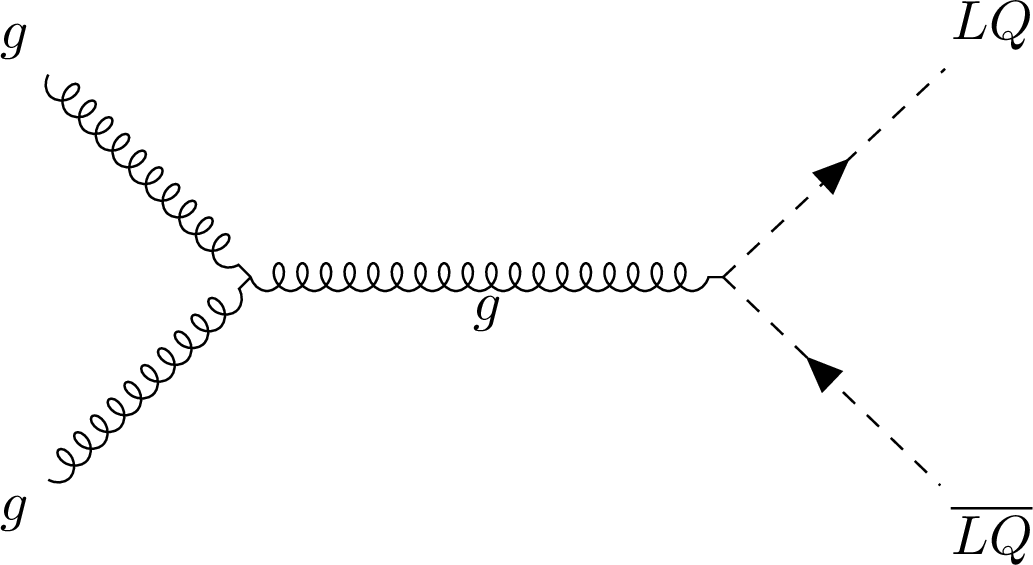
Figure 2-b:
Leading order Feynman diagram for the pair-production of LQs, leading to final states with two τ leptons and two b quarks. |
png pdf |
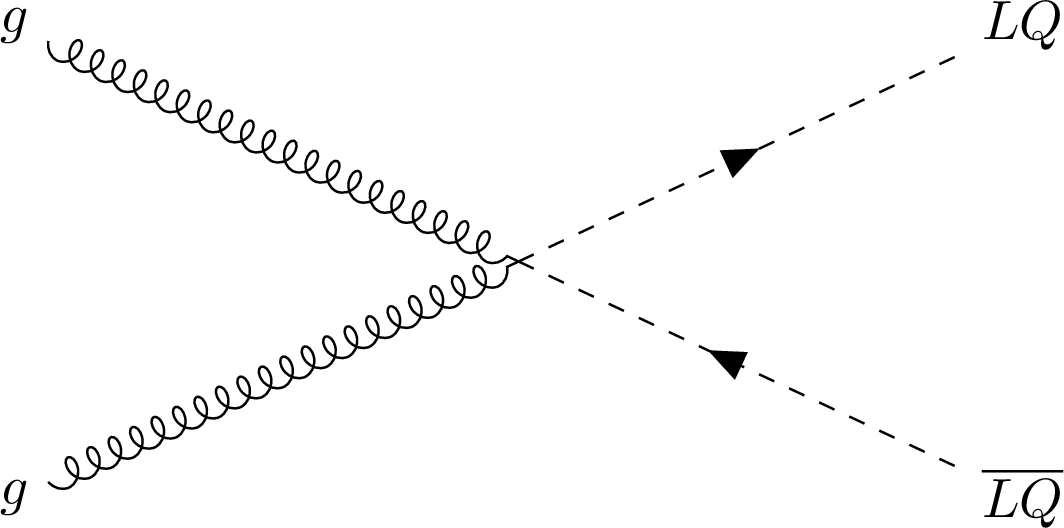
Figure 2-c:
Leading order Feynman diagram for the pair-production of LQs, leading to final states with two τ leptons and two b quarks. |
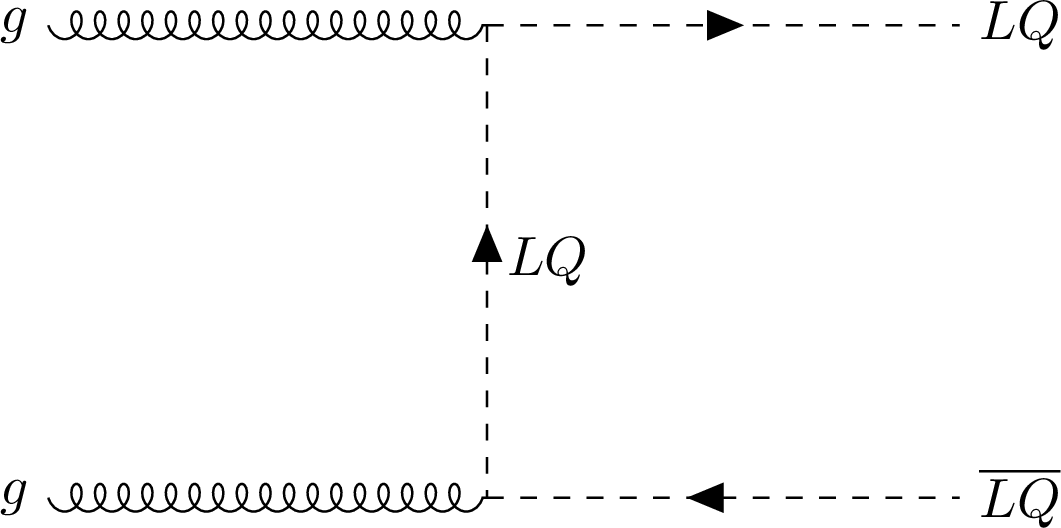
png pdf |
Figure 2-d:
Leading order Feynman diagram for the pair-production of LQs, leading to final states with two τ leptons and two b quarks. |
png pdf |
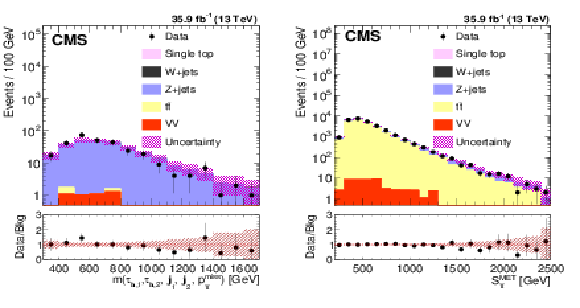
Figure 3:
Distributions in m(τh,1,τh,2,j1,j2,pTmiss) (left), for the Z(ττ) control sample with relaxed τh candidate pT thresholds and m(τh,1,τh,2)< 100 GeV, and SMETT (right), for the t¯t(μμjj) control sample. The bottom frames show the ratio between the observed data in the control samples and the total background (Bkg) predictions. The bands correspond to the statistical uncertainty for the background. |
png pdf |
Figure 3-a:
Distribution in m(τh,1,τh,2,j1,j2,pTmiss), for the Z(ττ) control sample with relaxed τh candidate pT thresholds and m(τh,1,τh,2)< 100 GeV. The bottom frame shows the ratio between the observed data in the control samples and the total background (Bkg) predictions. The band corresponds to the statistical uncertainty for the background. |

png pdf |
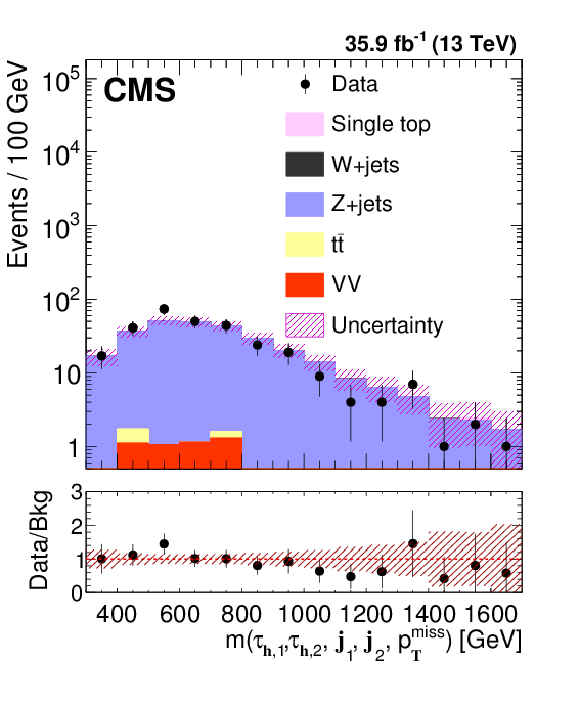
Figure 3-b:
Distribution in SMETT, for the t¯t(μμjj) control sample. The bottom frame shows the ratio between the observed data in the control samples and the total background (Bkg) predictions. The band corresponds to the statistical uncertainty for the background. |
png pdf |
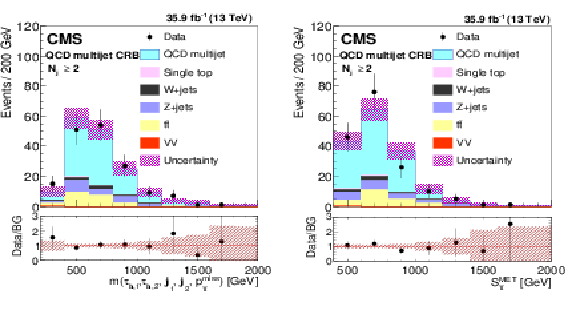
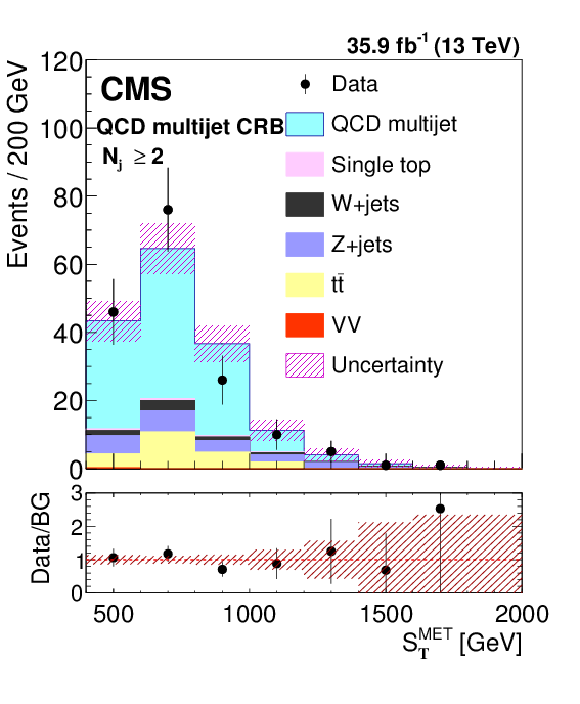
Figure 4:
QCD multijet background validation test, using the distributions in CR B m(τh,1,τh,2,j1,j2,pTmiss) (left) and SMETT (right). The shape of the QCD background is found from data in the loose τh region, CR A and then applied to CR B, defined by pTmiss< 50 GeV and tight τh isolation. For both samples, the non-QCD contributions are estimated from simulation. Note that the normalizations match by construction. The bottom frame shows the ratio between the observed data in CR B and the total background estimation. |

png pdf |
Figure 4-a:
QCD multijet background validation test, using the distribution in CR B m(τh,1,τh,2,j1,j2,pTmiss). shape of the QCD background is found from data in the loose τh region, CR A and then applied to CR B, defined by pTmiss< 50 GeV and tight τh isolation. The non-QCD contributions are estimated from simulation. Note that the normalizations match by construction. The bottom frame shows the ratio between the observed data in CR B and the total background estimation. |
png pdf |
Figure 4-b:
QCD multijet background validation test, using the distribution in CR B SMETT. The shape of the QCD background is found from data in the loose τh region, CR A and then applied to CR B, defined by pTmiss< 50 GeV and tight τh isolation. The non-QCD contributions are estimated from simulation. Note that the normalizations match by construction. The bottom frame shows the ratio between the observed data in CR B and the total background estimation. |

png pdf |
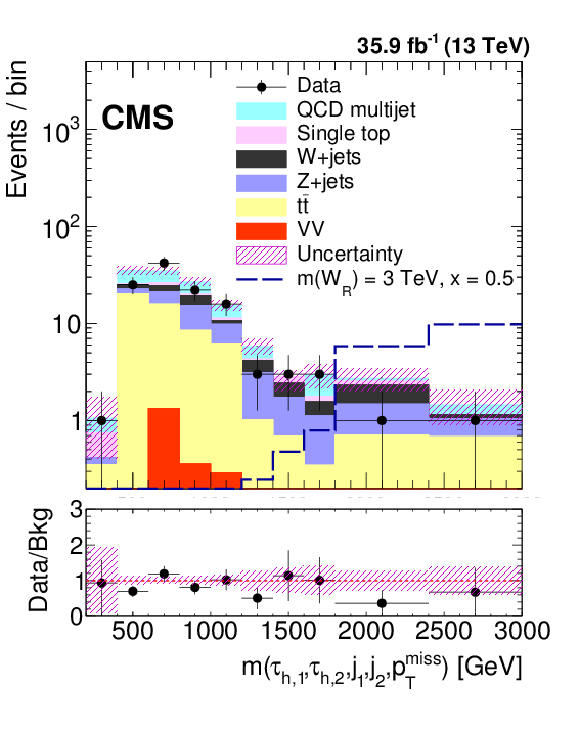
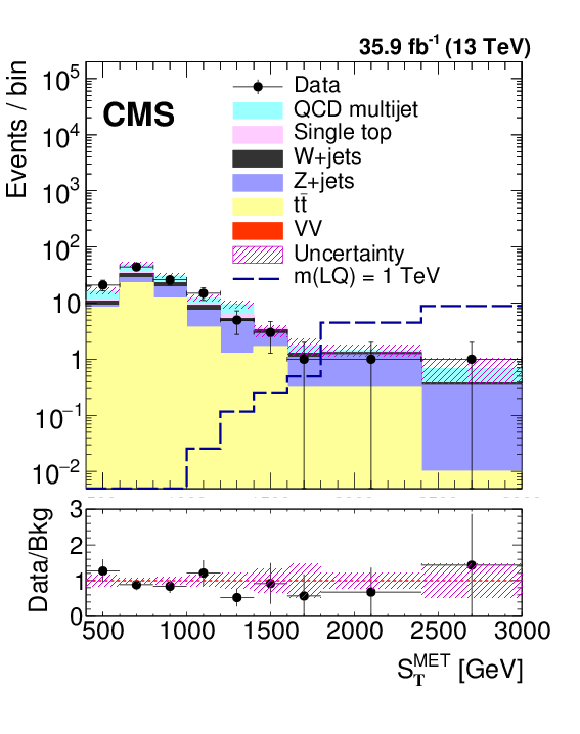
Figure 5:
Distributions in m(τh,1,τh,2,j1,j2,pTmiss) (left) and SMETT (right) for the estimated background in the signal region. The heavy neutrino model with m(WR)= 3 TeV and m(Nτ)= 1.5 TeV is used as a benchmark in the m(τh,1,τh,2,j1,j2,pTmiss) distribution, while the leptoquark model with m(LQ)= 1 TeV is used as a benchmark in the SMETT distribution. The bottom frame shows the ratio between the observed data and the background estimation; the band corresponds to the statistical uncertainty in the background. The t¯t, QCD multijet, and Z+jets contributions are estimated employing control regions in data and simulation, while the other contributions are obtained fully from the simulation. |
png pdf |
Figure 5-a:
Distribution in m(τh,1,τh,2,j1,j2,pTmiss) for the estimated background in the signal region. The heavy neutrino model with m(WR)= 3 TeV and m(Nτ)= 1.5 TeV is used as a benchmark in the m(τh,1,τh,2,j1,j2,pTmiss) distribution, while the leptoquark model with m(LQ)= 1 TeV is used as a benchmark in the SMETT distribution. The bottom frame shows the ratio between the observed data and the background estimation; the band corresponds to the statistical uncertainty in the background. The t¯t, QCD multijet, and Z+jets contributions are estimated employing control regions in data and simulation, while the other contributions are obtained fully from the simulation. |
png pdf |
Figure 5-b:
Distribution in SMETT for the estimated background in the signal region. The heavy neutrino model with m(WR)= 3 TeV and m(Nτ)= 1.5 TeV is used as a benchmark in the m(τh,1,τh,2,j1,j2,pTmiss) distribution, while the leptoquark model with m(LQ)= 1 TeV is used as a benchmark in the SMETT distribution. The bottom frame shows the ratio between the observed data and the background estimation; the band corresponds to the statistical uncertainty in the background. The t¯t, QCD multijet, and Z+jets contributions are estimated employing control regions in data and simulation, while the other contributions are obtained fully from the simulation. |
png pdf |
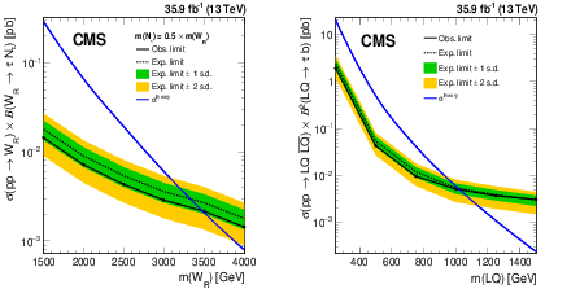
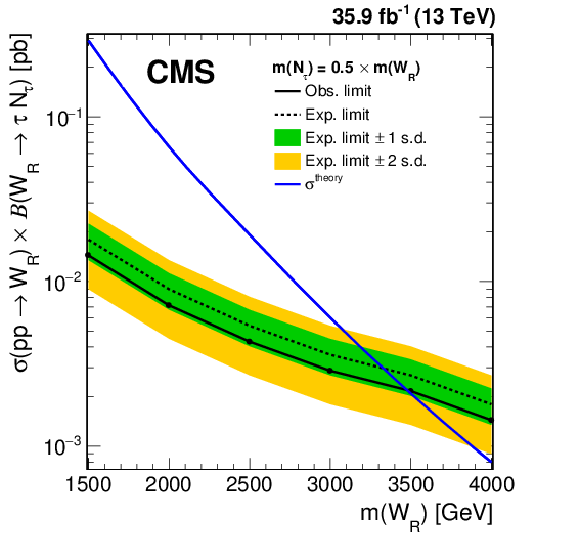
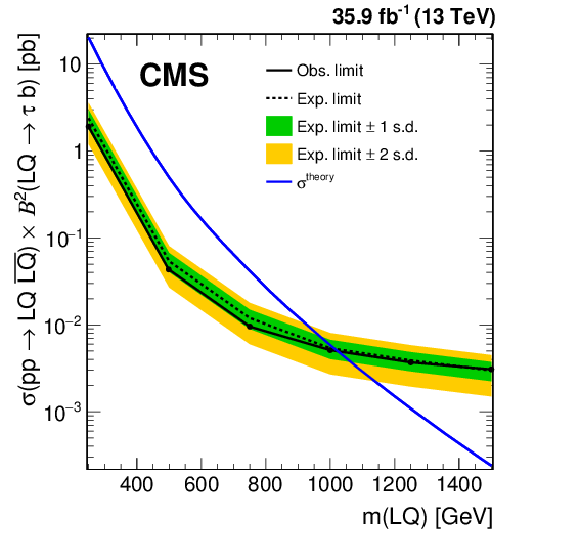
Figure 6:
Upper limits at 95% CL on the product of the cross section and the branching fraction for the production of WR (left) decaying to Nτ and for a pair of leptoquarks each decaying to τb (right), as functions of the produced particle mass. The observed limits are shown as solid black lines. Expected limits and their one- (two-) standard deviation limits are shown by dashed lines with green (yellow) bands. The theoretical cross sections are indicated by the solid blue lines. |
png pdf |
Figure 6-a:
Upper limit at 95% CL on the product of the cross section and the branching fraction for the production of WR decaying to Nτ, as function of the produced WR mass. The observed limit is shown as a solid black line. The expected limit and its one- (two-) standard deviation limits are shown by a dashed line with green (yellow) bands. The theoretical cross section is indicated by the solid blue line. |
png pdf |
Figure 6-b:
Upper limit at 95% CL on the product of the cross section and the branching fraction for the production a pair of leptoquarks each decaying to τb, as function of the produced leptoquark mass. The observed limit is shown as a solid black line. The expected limits and its one- (two-) standard deviation limits are shown by a dashed line with green (yellow) bands. The theoretical cross section is indicated by the solid blue line. |
png pdf |
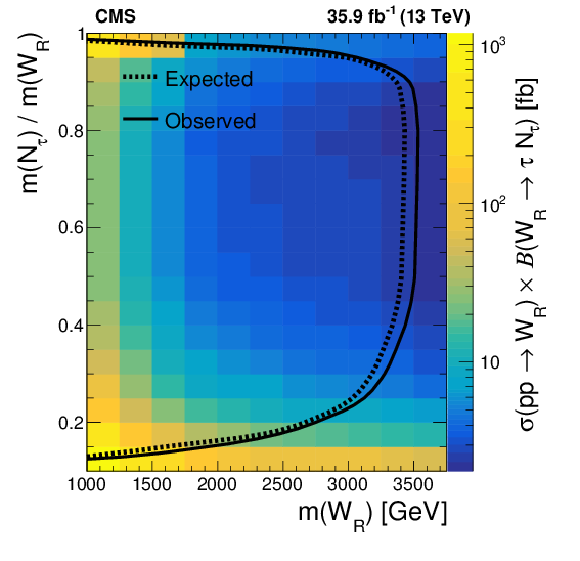
Figure 7:
Expected and observed limits at 95% CL on the product of the cross section and the branching fraction (WR→τNτ) as a function of m(WR) and m(Nτ)/m(WR). |
| Tables | |
png pdf |
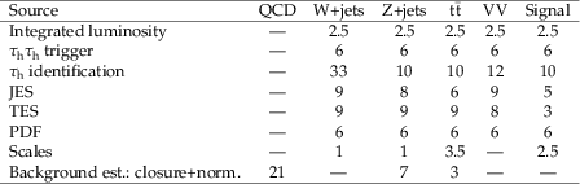
Table 1:
Summary of systematic uncertainties, given in percent. The τh identification, JES, and TES uncertainties are also considered as uncertainties in the shapes of the m(τh,1,τh,2,j1,j2,pTmiss) and SMETT distributions. Not included in the table are the bin-by-bin statistical uncertainties, which increase with larger values of mass and SMETT. |
png pdf |
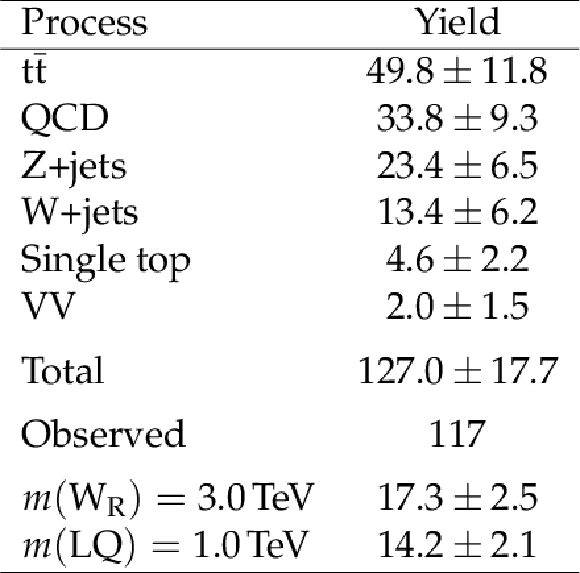
Table 2:
Estimated background and signal yields in the SR and their total uncertainties. The expected number of events for the WR signal sample assumes m(Nτ)=m(WR)/2. |
| Summary |
| A search is performed for physics beyond the standard model in events with two energetic τ leptons and two energetic jets, using data corresponding to an integrated luminosity of 35.9 fb−1 collected in 2016 with the CMS detector in proton-proton collisions at √s= 13 TeV. The search focuses on two benchmark scenarios: (1) the production of heavy right-handed Majorana neutrinos, Nℓ, and right-handed WR bosons, which arise in the left-right symmetric extensions of the standard model and where the WR and Nℓ decay chains result in a pair of high transverse momentum τ leptons; and (2) the pair production of third-generation scalar leptoquarks that decay to ττbb. The observed m(τh,1,τh,2,j1,j2,pmissT) and SMETT distributions do not reveal any evidence for physics beyond the standard model. Assuming that only the Nτ flavor contributes significantly to the WR decay width, WR masses below 3.52 (2.75) TeV are excluded at 95% confidence level, assuming the Nτ mass is 0.8 (0.2) times the mass of the WR boson. In the second beyond the standard model scenario, leptoquarks with a mass less than 1.02 TeV are excluded at 95% confidence level, to be compared with an expected mass limit of 1.00 TeV. Both of these results represent the most stringent limits to date for ττjj final states. |
| References | ||||
| 1 | Particle Data Group, C. Patrignani et al. | Review of particle physics | CPC 40 (2016) 100001 | |
| 2 | M. Lindner, T. Ohlsson, and G. Seidl | Seesaw mechanisms for Dirac and Majorana neutrino masses | PRD 65 (2002) 053014 | hep-ph/0109264 |
| 3 | P. Minkowski | μ→eγ at a rate of one out of 109 muon decays? | PLB 67 (1977) 421 | |
| 4 | R. N. Mohapatra and G. Senjanovic | Neutrino mass and spontaneous parity nonconservation | PRL 44 (1980) 912 | |
| 5 | M. Gell-Mann, P. Ramond, and R. Slansky | Complex spinors and unified theories | in Supergravity, p. 315 Elsevier Science Ltd, New York, September, 1979 Conference number C79-09-27 | 1306.4669 |
| 6 | T. Yanagida | Horizontal symmetry and masses of neutrinos | Prog. Theor. Phys. 64 (1980) 1103 | |
| 7 | R. N. Mohapatra and R. E. Marshak | Local B-L Symmetry of electroweak interactions, Majorana neutrinos and neutron oscillations | PRL 44 (1980) 1316, .[Erratum: \DOI10.1103/PhysRevLett.44.1316] | |
| 8 | J. C. Pati and A. Salam | Unified lepton-hadron symmetry and a gauge theory of the basic interactions | PRD 8 (1973) 1240 | |
| 9 | J. C. Pati and A. Salam | Lepton number as the fourth color | PRD 10 (1974) 275, . [Erratum: \DOI10.1103/PhysRevD.11.703.2] | |
| 10 | H. Georgi and S. L. Glashow | Unity of all elementary particle forces | PRL 32 (1974) 438 | |
| 11 | H. Fritzsch and P. Minkowski | Unified interactions of leptons and hadrons | Ann. Phys. 93 (1975) 193 | |
| 12 | S. Dimopoulos and L. Susskind | Mass without scalars | NPB 155 (1979) 237 | |
| 13 | S. Dimopoulos | Technicolored signatures | NPB 168 (1980) 69 | |
| 14 | E. Farhi and L. Susskind | Technicolor | PR 74 (1981) 277 | |
| 15 | K. D. Lane and M. V. Ramana | Walking technicolor signatures at hadron colliders | PRD 44 (1991) 2678 | |
| 16 | B. Schrempp and F. Schrempp | Light leptoquarks | PLB 153 (1985) 101 | |
| 17 | B. Gripaios | Composite leptoquarks at the LHC | JHEP 02 (2010) 045 | 0910.1789 |
| 18 | G. R. Farrar and P. Fayet | Phenomenology of the production, decay, and detection of new hadronic states associated with supersymmetry | PLB 76 (1978) 575 | |
| 19 | P. Ramond | Dual theory for free fermions | PRD 3 (1971) 2415 | |
| 20 | Y. A. Golfand and E. P. Likhtman | Extension of the algebra of Poincare group generators and violation of p invariance | JEPTL 13 (1971)323 | |
| 21 | A. Neveu and J. H. Schwarz | Factorizable dual model of pions | NPB 31 (1971) 86 | |
| 22 | D. V. Volkov and V. P. Akulov | Possible universal neutrino interaction | JEPTL 16 (1972)438 | |
| 23 | J. Wess and B. Zumino | A Lagrangian model invariant under supergauge transformations | PLB 49 (1974) 52 | |
| 24 | J. Wess and B. Zumino | Supergauge transformations in four dimensions | NPB 70 (1974) 39 | |
| 25 | P. Fayet | Supergauge invariant extension of the Higgs mechanism and a model for the electron and its neutrino | NPB 90 (1975) 104 | |
| 26 | H. P. Nilles | Supersymmetry, supergravity and particle physics | PR 110 (1984) 1 | |
| 27 | R. Barbier et al. | R-parity violating supersymmetry | PR 420 (2005) 1 | hep-ph/0406039 |
| 28 | W. Buchmuller and D. Wyler | Constraints on SU(5)-type leptoquarks | PLB 177 (1986) 377 | |
| 29 | O. U. Shanker | πℓ2, Kℓ3 and K0−ˉK0 constraints on leptoquarks and supersymmetric particles | NPB 204 (1982) 375 | |
| 30 | BaBar Collaboration | Evidence for an excess of ˉB→D(∗)τ−ˉντ decays | PRL 109 (2012) 101802 | 1205.5442 |
| 31 | BaBar Collaboration | Measurement of an excess of ˉB→D(∗)τ−ˉντ decays and implications for charged Higgs bosons | PRD 88 (2013) 072012 | 1303.0571 |
| 32 | Belle Collaboration | Observation of B0→D∗−τ+ντ decay at Belle | PRL 99 (2007) 191807 | 0706.4429 |
| 33 | Belle Collaboration | Observation of B+→ˉD∗0τ+ντ and evidence for B+→ˉD0τ+ντ at Belle | PRD 82 (2010) 072005 | 1005.2302 |
| 34 | Belle Collaboration | Measurement of the branching ratio of ˉB→D(∗)τ−ˉντ relative to ˉB→D(∗)ℓ−ˉνℓ decays with hadronic tagging at Belle | PRD 92 (2015) 072014 | 1507.03233 |
| 35 | Belle Collaboration | Measurement of the τ lepton polarization and R(D∗) in the decay ˉB→D∗τ−ˉντ | PRL 118 (2017) 211801 | 1612.00529 |
| 36 | LHCb Collaboration | Measurement of form-factor-independent observables in the decay B0→K∗0μ+μ− | PRL 111 (2013) 191801 | 1308.1707 |
| 37 | LHCb Collaboration | Test of lepton universality using B+→K+ℓ+ℓ− decays | PRL 113 (2014) 151601 | 1406.6482 |
| 38 | LHCb Collaboration | Measurement of the ratio of branching fractions B(ˉB0→D∗+τ−ˉντ)/B(ˉB0→D∗+μ−ˉνμ) | PRL 115 (2015) 111803 | 1506.08614 |
| 39 | LHCb Collaboration | Angular analysis of the B0→K∗0μ+μ− decay using 3 fb−1 of integrated luminosity | JHEP 02 (2016) 104 | 1512.04442 |
| 40 | LHCb Collaboration | Test of lepton universality with B0→K∗0ℓ+ℓ− decays | JHEP 08 (2017) 055 | 1705.05802 |
| 41 | M. Tanaka and R. Watanabe | New physics in the weak interaction of ˉB→D(∗)τˉν | PRD 87 (2013) 034028 | 1212.1878 |
| 42 | Y. Sakaki, M. Tanaka, A. Tayduganov, and R. Watanabe | Testing leptoquark models in ˉB→D(∗)τˉν | PRD 88 (2013) 094012 | 1309.0301 |
| 43 | I. Dorsner, S. Fajfer, N. Kosnik, and I. Nisandzic | Minimally flavored colored scalar in ˉB→D(∗)τˉν and the mass matrices constraints | JHEP 11 (2013) 084 | 1306.6493 |
| 44 | B. Gripaios, M. Nardecchia, and S. A. Renner | Composite leptoquarks and anomalies in B-meson decays | JHEP 05 (2015) 006 | 1412.1791 |
| 45 | ATLAS Collaboration | Search for heavy neutrinos and right-handed W bosons in events with two leptons and jets in pp collisions at √s= 7 TeV with the ATLAS detector | EPJC 72 (2012) 2056 | 1203.5420 |
| 46 | ATLAS Collaboration | Search for heavy Majorana neutrinos with the ATLAS detector in pp collisions at √s= 8 TeV | JHEP 07 (2015) 162 | 1506.06020 |
| 47 | ATLAS Collaboration | Search for third generation scalar leptoquarks in pp collisions at √s= 7 TeV with the ATLAS detector | JHEP 06 (2013) 033 | 1303.0526 |
| 48 | CMS Collaboration | Search for a heavy right-handed W boson and a heavy neutrino in events with two same-flavor leptons and two jets at √s= 13 TeV | JHEP 05 (2018) 148 | CMS-EXO-17-011 1803.11116 |
| 49 | CMS Collaboration | Search for a heavy composite Majorana neutrino in the final state with two leptons and two quarks at √s= 13 TeV | PLB 775 (2017) 315 | CMS-EXO-16-026 1706.08578 |
| 50 | CMS Collaboration | Search for third-generation scalar leptoquarks and heavy right-handed neutrinos in final states with two tau leptons and two jets in proton-proton collisions at √s= 13 TeV | JHEP 07 (2017) 121 | CMS-EXO-16-023 1703.03995 |
| 51 | CMS Collaboration | Search for heavy neutrinos or third-generation leptoquarks in final states with two hadronically decaying τ leptons and two jets in proton-proton collisions at √s= 13 TeV | JHEP 03 (2017) 077 | CMS-EXO-16-016 1612.01190 |
| 52 | CMS Collaboration | Search for heavy neutrinos and W bosons with right-handed couplings in proton-proton collisions at √s= 8 TeV | EPJC 74 (2014) 3149 | CMS-EXO-13-008 1407.3683 |
| 53 | CMS Collaboration | Search for third-generation scalar leptoquarks decaying to a top quark and a τ lepton at √s= 13 TeV | EPJC 78 (2018) 707 | CMS-B2G-16-028 1803.02864 |
| 54 | CMS Collaboration | Constraints on models of scalar and vector leptoquarks decaying to a quark and a neutrino at √s= 13 TeV | PRD 98 (2018) 032005 | CMS-SUS-18-001 1805.10228 |
| 55 | CMS Collaboration | Search for a singly produced third-generation scalar leptoquark decaying to a τ lepton and a bottom quark in proton-proton collisions at √s= 13 TeV | JHEP 07 (2018) 115 | CMS-EXO-17-029 1806.03472 |
| 56 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 03 (2008) S08004 | CMS-00-001 |
| 57 | CMS Collaboration | Particle-flow reconstruction and global event description with the cms detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 58 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-kT jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 59 | CMS Collaboration | Jet algorithms performance in 13 TeV data | CMS-PAS-JME-16-003 | CMS-PAS-JME-16-003 |
| 60 | CMS Collaboration | Determination of jet energy calibration and transverse momentum resolution in CMS | JINST 6 (2011) 11002 | CMS-JME-10-011 1107.4277 |
| 61 | CMS Collaboration | Study of pileup removal algorithms for jets | CMS-PAS-JME-14-001 | CMS-PAS-JME-14-001 |
| 62 | CMS Collaboration | Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV | JINST 13 (2018) P05011 | CMS-BTV-16-002 1712.07158 |
| 63 | CMS Collaboration | Performance of electron reconstruction and selection with the CMS detector in proton-proton collisions at √s= 8 TeV | JINST 10 (2015) P06005 | CMS-EGM-13-001 1502.02701 |
| 64 | CMS Collaboration | Performance of the CMS muon detector and muon reconstruction with proton-proton collisions at √s= 13 TeV | JINST 13 (2018) P06015 | CMS-MUO-16-001 1804.04528 |
| 65 | M. Cacciari and G. P. Salam | Pileup subtraction using jet areas | PLB 659 (2008) 119 | 0707.1378 |
| 66 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 67 | CMS Collaboration | Performance of reconstruction and identification of τ leptons decaying to hadrons and ντ in pp collisions at √s= 13 TeV | JINST 13 (2018) P10005 | CMS-TAU-16-003 1809.02816 |
| 68 | CMS Collaboration | Performance of missing transverse momentum in proton-proton collisions at √s= 13 TeV using the CMS detector | CMS-PAS-JME-17-001 | CMS-PAS-JME-17-001 |
| 69 | J. Alwall et al. | The automated computation of tree-level and next-to-leading order differential cross sections, and their matching to parton shower simulations | JHEP 07 (2014) 079 | 1405.0301 |
| 70 | T. Sjostrand et al. | An introduction to PYTHIA 8.2 | CPC 191 (2015) 159 | 1410.3012 |
| 71 | CMS Collaboration | Event generator tunes obtained from underlying event and multiparton scattering measurements | EPJC 76 (2016) 155 | CMS-GEN-14-001 1512.00815 |
| 72 | M. Kramer, T. Plehn, M. Spira, and P. M. Zerwas | Pair production of scalar leptoquarks at the CERN LHC | PRD 71 (2005) 057503 | hep-ph/0411038 |
| 73 | F. del Aguila, J. A. Aguilar-Saavedra, and R. Pittau | Heavy neutrino signals at large hadron colliders | JHEP 10 (2007) 047 | hep-ph/0703261 |
| 74 | J. Pumplin et al. | New generation of parton distributions with uncertainties from global QCD analysis | JHEP 07 (2002) 012 | hep-ph/0201195 |
| 75 | CMS Collaboration | Measurement of the inclusive Z cross section via decays to tau pairs in pp collisions at √s= 7 TeV | JHEP 08 (2011) 117 | CMS-EWK-10-013 1104.1617 |
| 76 | CMS Collaboration | CMS luminosity measurements for the 2016 data taking period | CMS-PAS-LUM-17-001 | CMS-PAS-LUM-17-001 |
| 77 | J. Butterworth et al. | PDF4LHC recommendations for LHC run II | JPG 43 (2016) 023001 | 1510.03865 |
| 78 | S. Alekhin et al. | The PDF4LHC working group interim report | 1101.0536 | |
| 79 | M. Botje et al. | The PDF4LHC working group interim recommendations | 1101.0538 | |
| 80 | T. Junk | Confidence level computation for combining searches with small statistics | NIMA 434 (1999) 435 | hep-ex/9902006 |
| 81 | A. L. Read | Presentation of search results: the CLs technique | JPG 28 (2002) 2693 | |
| 82 | ATLAS and CMS Collaborations | Procedure for the LHC Higgs boson search combination in Summer 2011 | CMS-NOTE-2011-005 | |
| 83 | R. J. Barlow and C. Beeston | Fitting using finite Monte Carlo samples | CPC 77 (1993) 219 | |

|
Compact Muon Solenoid LHC, CERN |

|

|

|

|

|

|