

Compact Muon Solenoid
LHC, CERN
| CMS-PAS-HIG-18-001 | ||
| Measurements of properties of the Higgs boson in the four-lepton final state at $\sqrt{s}= $ 13 TeV | ||
| CMS Collaboration | ||
| June 2018 | ||
| Abstract: Properties of the Higgs boson are measured in the $\mathrm{H}\rightarrow{\mathrm{Z}}{\mathrm{Z}}\rightarrow4\ell$ ($\ell={\mathrm{e}},\mu$) decay channel. A data sample of proton-proton collisions at a center-of-mass energy of 13 TeV is used, corresponding to an integrated luminosity of 41.5 fb$^{-1}$ recorded in 2017 by the CMS detector at the LHC. The signal-strength modifier $\mu$, defined as the ratio of the observed Higgs boson rate in the $\mathrm{H}\rightarrow{\mathrm{Z}}{\mathrm{Z}}\rightarrow4\ell$ decay channel to the standard model expectation, is measured to be $\mu= $ 1.10$^{+0.19}_{-0.17}$ at $m_{\mathrm{H}}= $ 125.09 GeV, the combined ATLAS and CMS measurement of the Higgs boson mass. The signal-strength modifiers for the main Higgs boson production modes are also constrained. Combination with data recorded in 2016 by the CMS detector at a center-of-mass energy of 13 TeV corresponding to an integrated luminosity of 35.9 fb$^{-1}$ is reported. All results are found to be compatible with the standard model predictions. | ||
| Links: CDS record (PDF) ; inSPIRE record ; CADI line (restricted) ; | ||
| Figures | |
png pdf |
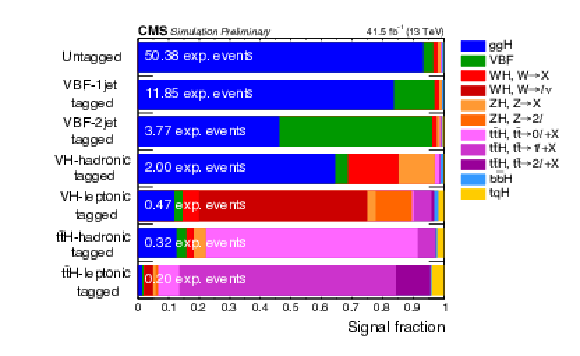
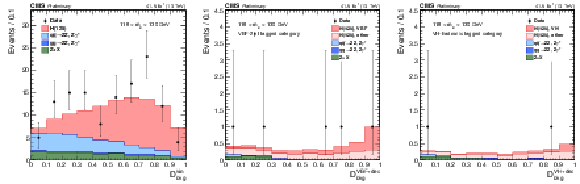
Figure 1:
Signal relative purity of the seven event categories in terms of the seven main production mechanisms of the Higgs boson in a 118 $ < {m_{4\ell}} < $ 130 GeV mass window. The $ {{\mathrm {W}} {\mathrm {H}}}$, $ {{\mathrm {Z}} {\mathrm {H}}}$ and $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}}$ processes are split according to the decay of associated objects, whereby X denotes anything other than an electron or muon. |

png pdf |
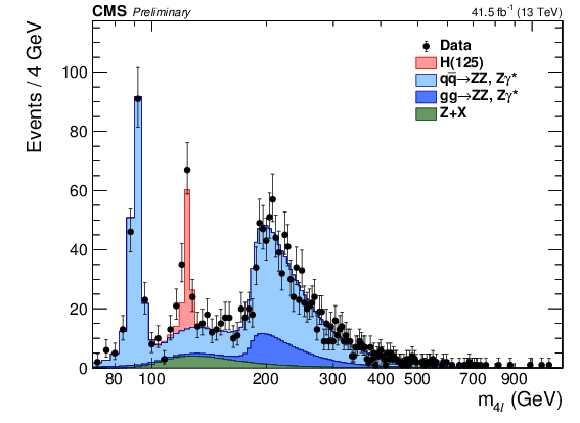
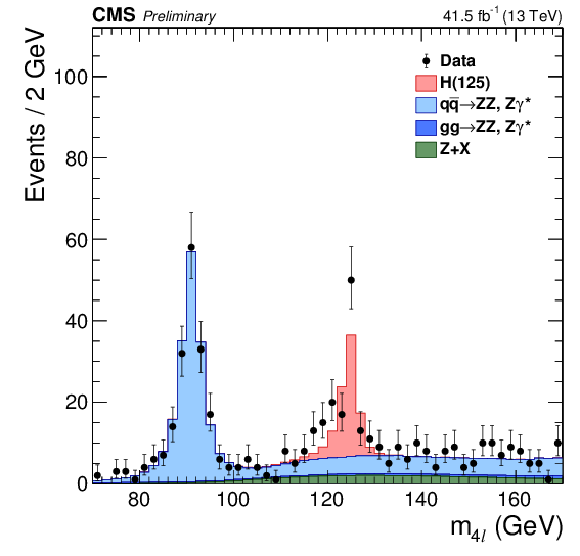
Figure 2:
Distribution of the four-lepton reconstructed invariant mass $ {m_{4\ell}}$ in the full mass range (left) and the low-mass range (right). Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The order in perturbation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. No events are observed with $ {m_{4\ell}} > $ 1.1 TeV. |
png pdf |
Figure 2-a:
Distribution of the four-lepton reconstructed invariant mass $ {m_{4\ell}}$ in the full mass range (left) and the low-mass range (right). Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The order in perturbation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. No events are observed with $ {m_{4\ell}} > $ 1.1 TeV. |
png pdf |
Figure 2-b:
Distribution of the four-lepton reconstructed invariant mass $ {m_{4\ell}}$ in the full mass range (left) and the low-mass range (right). Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The order in perturbation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. No events are observed with $ {m_{4\ell}} > $ 1.1 TeV. |
png pdf |
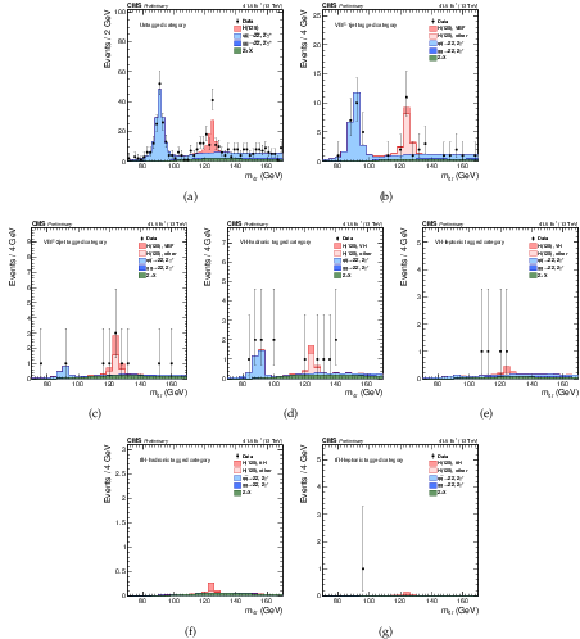
Figure 3:
Distribution of the four-lepton reconstructed mass in the seven event categories for the low-mass range. (a) untagged category (b) VBF-1jet-tagged category (c) VBF-2jet-tagged category (d) VH-hadronic-tagged category (e) VH-leptonic-tagged category (f) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-hadronic-tagged category (g) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-leptonic-tagged. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. For the categories other than the untagged category, the SM Higgs boson signal is separated into two components: the production mode that is targeted by the specific category, and other production modes, where the gluon fusion process dominates. The order in pertubation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |

png pdf |
Figure 3-a:
Distribution of the four-lepton reconstructed mass in the seven event categories for the low-mass range. (a) untagged category (b) VBF-1jet-tagged category (c) VBF-2jet-tagged category (d) VH-hadronic-tagged category (e) VH-leptonic-tagged category (f) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-hadronic-tagged category (g) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-leptonic-tagged. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. For the categories other than the untagged category, the SM Higgs boson signal is separated into two components: the production mode that is targeted by the specific category, and other production modes, where the gluon fusion process dominates. The order in pertubation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |
png pdf |
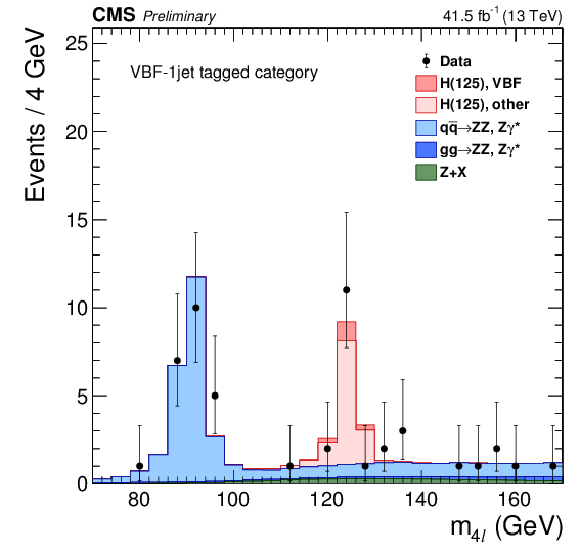
Figure 3-b:
Distribution of the four-lepton reconstructed mass in the seven event categories for the low-mass range. (a) untagged category (b) VBF-1jet-tagged category (c) VBF-2jet-tagged category (d) VH-hadronic-tagged category (e) VH-leptonic-tagged category (f) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-hadronic-tagged category (g) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-leptonic-tagged. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. For the categories other than the untagged category, the SM Higgs boson signal is separated into two components: the production mode that is targeted by the specific category, and other production modes, where the gluon fusion process dominates. The order in pertubation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |

png pdf |
Figure 3-c:
Distribution of the four-lepton reconstructed mass in the seven event categories for the low-mass range. (a) untagged category (b) VBF-1jet-tagged category (c) VBF-2jet-tagged category (d) VH-hadronic-tagged category (e) VH-leptonic-tagged category (f) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-hadronic-tagged category (g) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-leptonic-tagged. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. For the categories other than the untagged category, the SM Higgs boson signal is separated into two components: the production mode that is targeted by the specific category, and other production modes, where the gluon fusion process dominates. The order in pertubation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |

png pdf |
Figure 3-d:
Distribution of the four-lepton reconstructed mass in the seven event categories for the low-mass range. (a) untagged category (b) VBF-1jet-tagged category (c) VBF-2jet-tagged category (d) VH-hadronic-tagged category (e) VH-leptonic-tagged category (f) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-hadronic-tagged category (g) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-leptonic-tagged. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. For the categories other than the untagged category, the SM Higgs boson signal is separated into two components: the production mode that is targeted by the specific category, and other production modes, where the gluon fusion process dominates. The order in pertubation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |
png pdf |
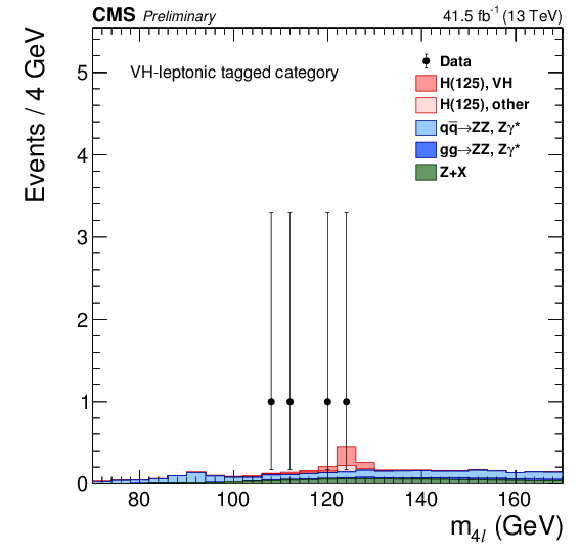
Figure 3-e:
Distribution of the four-lepton reconstructed mass in the seven event categories for the low-mass range. (a) untagged category (b) VBF-1jet-tagged category (c) VBF-2jet-tagged category (d) VH-hadronic-tagged category (e) VH-leptonic-tagged category (f) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-hadronic-tagged category (g) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-leptonic-tagged. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. For the categories other than the untagged category, the SM Higgs boson signal is separated into two components: the production mode that is targeted by the specific category, and other production modes, where the gluon fusion process dominates. The order in pertubation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |
png pdf |
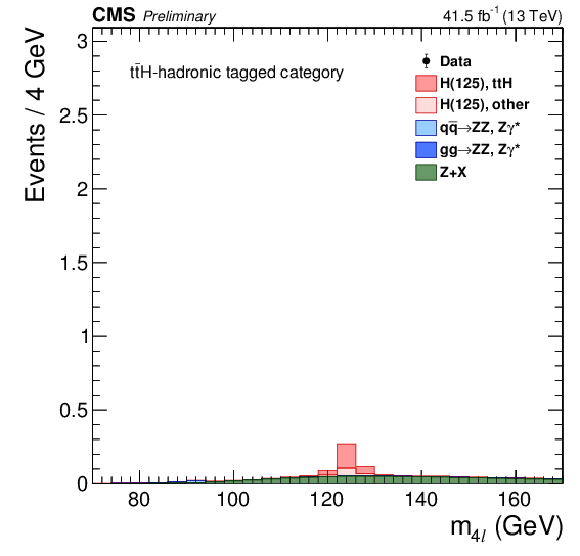
Figure 3-f:
Distribution of the four-lepton reconstructed mass in the seven event categories for the low-mass range. (a) untagged category (b) VBF-1jet-tagged category (c) VBF-2jet-tagged category (d) VH-hadronic-tagged category (e) VH-leptonic-tagged category (f) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-hadronic-tagged category (g) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-leptonic-tagged. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. For the categories other than the untagged category, the SM Higgs boson signal is separated into two components: the production mode that is targeted by the specific category, and other production modes, where the gluon fusion process dominates. The order in pertubation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |
png pdf |
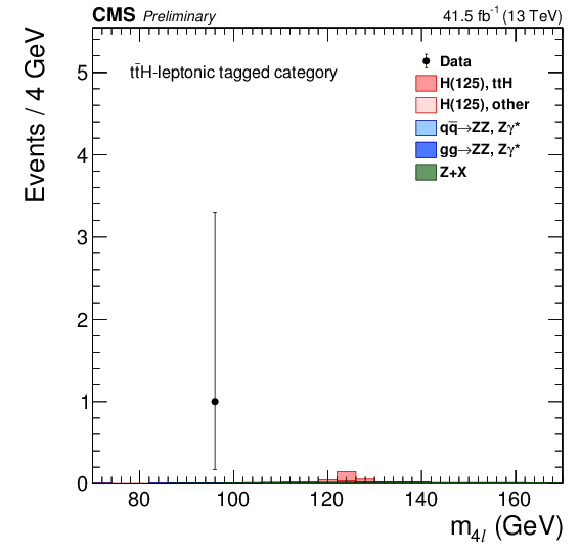
Figure 3-g:
Distribution of the four-lepton reconstructed mass in the seven event categories for the low-mass range. (a) untagged category (b) VBF-1jet-tagged category (c) VBF-2jet-tagged category (d) VH-hadronic-tagged category (e) VH-leptonic-tagged category (f) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-hadronic-tagged category (g) $ {{\mathrm {t}}\bar{{\mathrm {t}}} {\mathrm {H}}} $-leptonic-tagged. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. For the categories other than the untagged category, the SM Higgs boson signal is separated into two components: the production mode that is targeted by the specific category, and other production modes, where the gluon fusion process dominates. The order in pertubation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |

png pdf |
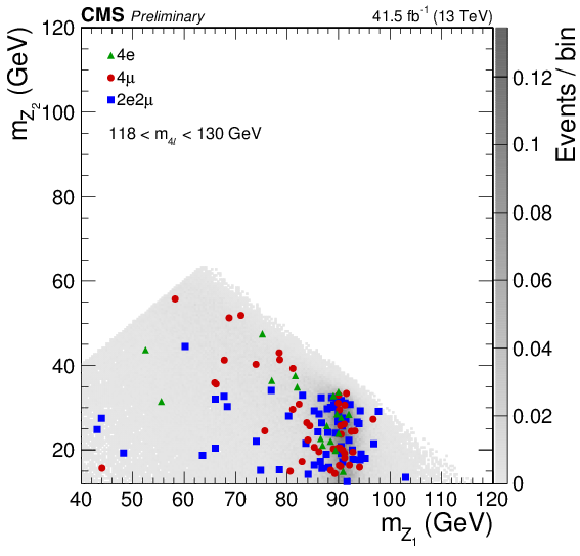
Figure 4:
Distribution of the $ {\mathrm {Z}}_1$ (left) and $ {\mathrm {Z}}_2$ (center) reconstructed invariant masses and correlation between the two (right) in the mass region 118 $ < {m_{4\ell}} < $ 130 GeV. The stacked histograms and the gray scale represent expected distributions of the signal and background processes, and points represent the data. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The order in perturbation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |

png pdf |
Figure 4-a:
Distribution of the $ {\mathrm {Z}}_1$ (left) and $ {\mathrm {Z}}_2$ (center) reconstructed invariant masses and correlation between the two (right) in the mass region 118 $ < {m_{4\ell}} < $ 130 GeV. The stacked histograms and the gray scale represent expected distributions of the signal and background processes, and points represent the data. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The order in perturbation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |

png pdf |
Figure 4-b:
Distribution of the $ {\mathrm {Z}}_1$ (left) and $ {\mathrm {Z}}_2$ (center) reconstructed invariant masses and correlation between the two (right) in the mass region 118 $ < {m_{4\ell}} < $ 130 GeV. The stacked histograms and the gray scale represent expected distributions of the signal and background processes, and points represent the data. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The order in perturbation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |
png pdf |
Figure 4-c:
Distribution of the $ {\mathrm {Z}}_1$ (left) and $ {\mathrm {Z}}_2$ (center) reconstructed invariant masses and correlation between the two (right) in the mass region 118 $ < {m_{4\ell}} < $ 130 GeV. The stacked histograms and the gray scale represent expected distributions of the signal and background processes, and points represent the data. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The order in perturbation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |

png pdf |
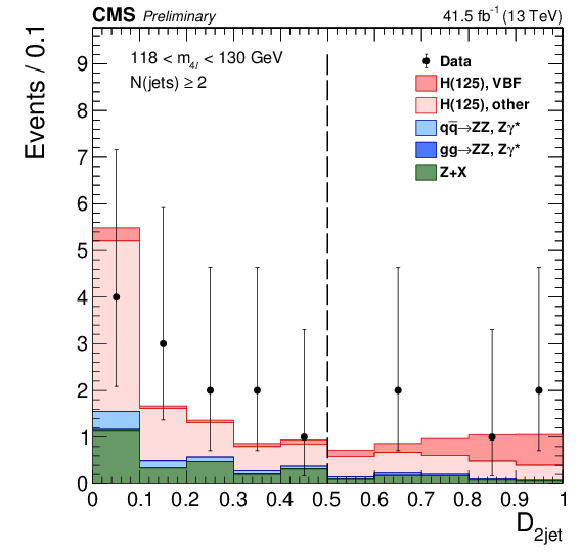
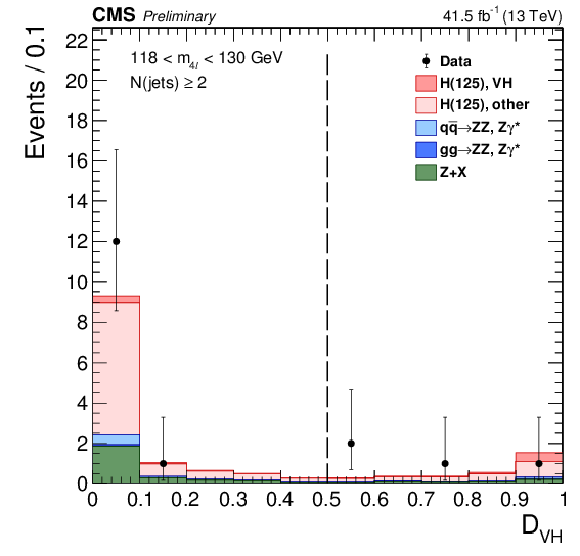
Figure 5:
Distribution of categorization discriminants in the mass region 118 $ < {m_{4\ell}} < $ 130 GeV: (left) $ {{\mathcal D}_{\mathrm 2jet}} $, (middle) ${{\mathcal D}_{\mathrm 1jet}}$, (right) ${{\mathcal D}_{\mathrm VH}} = max({{\mathcal D}_{\mathrm {{\mathrm {W}} {\mathrm {H}}}}}$, ${{\mathcal D}_{\mathrm {{\mathrm {Z}} {\mathrm {H}}}}})$. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The vertical gray dashed lines denote the working points used in the event categorization. The SM Higgs boson signal is separated into two components: the production mode which is targeted by the specific discriminant, and other production modes, where the gluon fusion process dominates. The order in perturbation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |
png pdf |
Figure 5-a:
Distribution of categorization discriminants in the mass region 118 $ < {m_{4\ell}} < $ 130 GeV: (left) $ {{\mathcal D}_{\mathrm 2jet}} $, (middle) ${{\mathcal D}_{\mathrm 1jet}}$, (right) ${{\mathcal D}_{\mathrm VH}} = max({{\mathcal D}_{\mathrm {{\mathrm {W}} {\mathrm {H}}}}}$, ${{\mathcal D}_{\mathrm {{\mathrm {Z}} {\mathrm {H}}}}})$. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The vertical gray dashed lines denote the working points used in the event categorization. The SM Higgs boson signal is separated into two components: the production mode which is targeted by the specific discriminant, and other production modes, where the gluon fusion process dominates. The order in perturbation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |

png pdf |
Figure 5-b:
Distribution of categorization discriminants in the mass region 118 $ < {m_{4\ell}} < $ 130 GeV: (left) $ {{\mathcal D}_{\mathrm 2jet}} $, (middle) ${{\mathcal D}_{\mathrm 1jet}}$, (right) ${{\mathcal D}_{\mathrm VH}} = max({{\mathcal D}_{\mathrm {{\mathrm {W}} {\mathrm {H}}}}}$, ${{\mathcal D}_{\mathrm {{\mathrm {Z}} {\mathrm {H}}}}})$. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The vertical gray dashed lines denote the working points used in the event categorization. The SM Higgs boson signal is separated into two components: the production mode which is targeted by the specific discriminant, and other production modes, where the gluon fusion process dominates. The order in perturbation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |
png pdf |
Figure 5-c:
Distribution of categorization discriminants in the mass region 118 $ < {m_{4\ell}} < $ 130 GeV: (left) $ {{\mathcal D}_{\mathrm 2jet}} $, (middle) ${{\mathcal D}_{\mathrm 1jet}}$, (right) ${{\mathcal D}_{\mathrm VH}} = max({{\mathcal D}_{\mathrm {{\mathrm {W}} {\mathrm {H}}}}}$, ${{\mathcal D}_{\mathrm {{\mathrm {Z}} {\mathrm {H}}}}})$. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The vertical gray dashed lines denote the working points used in the event categorization. The SM Higgs boson signal is separated into two components: the production mode which is targeted by the specific discriminant, and other production modes, where the gluon fusion process dominates. The order in perturbation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. |
png pdf |
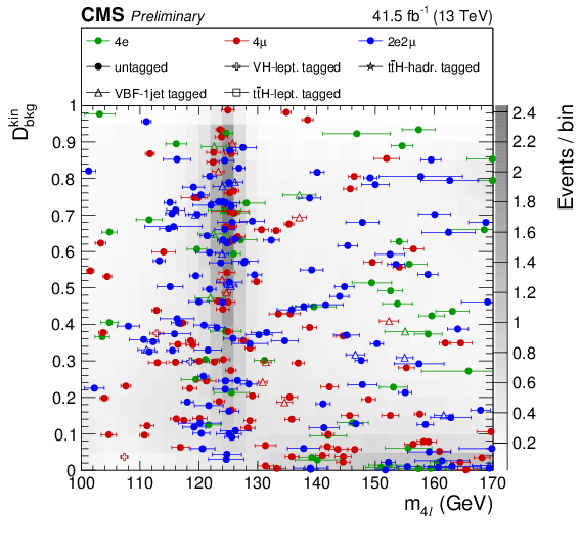
Figure 6:
Distribution of three different kinematic discriminants versus $ {m_{4\ell}}$: $ {{\cal D}^{\mathrm kin}_{\mathrm bkg}} $ (left), $ {{\mathcal {D}}^{\mathrm {VBF}+\mathrm {dec}}_{\mathrm {bkg}}} $ (middle) and $ {{\mathcal {D}}^{\mathrm {VH}+\mathrm {dec}}_{\mathrm {bkg}}} $ (right) shown in the mass region 100 $ < {m_{4\ell}} < $ 170 GeV. The gray scale represents the expected total number of $ {\mathrm {Z}} {\mathrm {Z}}$ and $ {\mathrm {Z}}$+X background and SM Higgs boson signal events for $ {m_{{\mathrm {H}}}} = $ 125 GeV. The points show the data and the horizontal bars represent the measured event-by-event mass uncertainties. Different marker styles are used to denote the categorization of the events. |
png pdf |
Figure 6-a:
Distribution of three different kinematic discriminants versus $ {m_{4\ell}}$: $ {{\cal D}^{\mathrm kin}_{\mathrm bkg}} $ (left), $ {{\mathcal {D}}^{\mathrm {VBF}+\mathrm {dec}}_{\mathrm {bkg}}} $ (middle) and $ {{\mathcal {D}}^{\mathrm {VH}+\mathrm {dec}}_{\mathrm {bkg}}} $ (right) shown in the mass region 100 $ < {m_{4\ell}} < $ 170 GeV. The gray scale represents the expected total number of $ {\mathrm {Z}} {\mathrm {Z}}$ and $ {\mathrm {Z}}$+X background and SM Higgs boson signal events for $ {m_{{\mathrm {H}}}} = $ 125 GeV. The points show the data and the horizontal bars represent the measured event-by-event mass uncertainties. Different marker styles are used to denote the categorization of the events. |

png pdf |
Figure 6-b:
Distribution of three different kinematic discriminants versus $ {m_{4\ell}}$: $ {{\cal D}^{\mathrm kin}_{\mathrm bkg}} $ (left), $ {{\mathcal {D}}^{\mathrm {VBF}+\mathrm {dec}}_{\mathrm {bkg}}} $ (middle) and $ {{\mathcal {D}}^{\mathrm {VH}+\mathrm {dec}}_{\mathrm {bkg}}} $ (right) shown in the mass region 100 $ < {m_{4\ell}} < $ 170 GeV. The gray scale represents the expected total number of $ {\mathrm {Z}} {\mathrm {Z}}$ and $ {\mathrm {Z}}$+X background and SM Higgs boson signal events for $ {m_{{\mathrm {H}}}} = $ 125 GeV. The points show the data and the horizontal bars represent the measured event-by-event mass uncertainties. Different marker styles are used to denote the categorization of the events. |
png pdf |
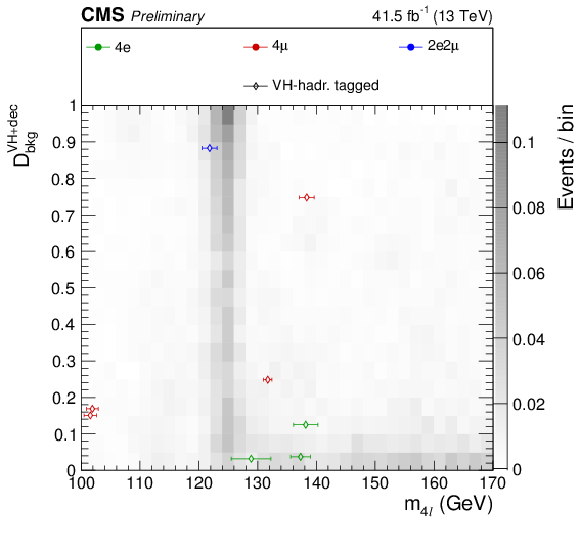
Figure 6-c:
Distribution of three different kinematic discriminants versus $ {m_{4\ell}}$: $ {{\cal D}^{\mathrm kin}_{\mathrm bkg}} $ (left), $ {{\mathcal {D}}^{\mathrm {VBF}+\mathrm {dec}}_{\mathrm {bkg}}} $ (middle) and $ {{\mathcal {D}}^{\mathrm {VH}+\mathrm {dec}}_{\mathrm {bkg}}} $ (right) shown in the mass region 100 $ < {m_{4\ell}} < $ 170 GeV. The gray scale represents the expected total number of $ {\mathrm {Z}} {\mathrm {Z}}$ and $ {\mathrm {Z}}$+X background and SM Higgs boson signal events for $ {m_{{\mathrm {H}}}} = $ 125 GeV. The points show the data and the horizontal bars represent the measured event-by-event mass uncertainties. Different marker styles are used to denote the categorization of the events. |
png pdf |
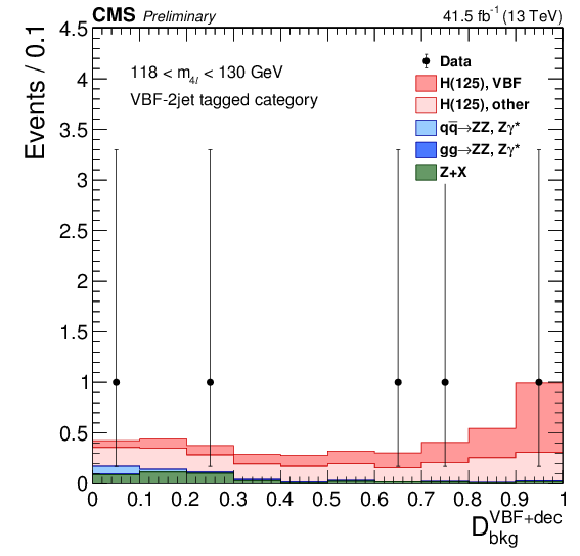
Figure 7:
Distribution of kinematic discriminants in the mass region 118 $ < {m_{4\ell}} < $ 130 GeV: (left) ${{\cal D}^{\mathrm kin}_{\mathrm bkg}}$, (middle) ${{\mathcal {D}}^{\mathrm {VBF}+\mathrm {dec}}_{\mathrm {bkg}}}$, (right) ${{\mathcal {D}}^{\mathrm {VH}+\mathrm {dec}}_{\mathrm {bkg}}}$. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The SM Higgs boson signal is separated into two components: the production mode which is targeted by the specific discriminant, and other production modes, where the gluon fusion process dominates. |

png pdf |
Figure 7-a:
Distribution of kinematic discriminants in the mass region 118 $ < {m_{4\ell}} < $ 130 GeV: (left) ${{\cal D}^{\mathrm kin}_{\mathrm bkg}}$, (middle) ${{\mathcal {D}}^{\mathrm {VBF}+\mathrm {dec}}_{\mathrm {bkg}}}$, (right) ${{\mathcal {D}}^{\mathrm {VH}+\mathrm {dec}}_{\mathrm {bkg}}}$. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The SM Higgs boson signal is separated into two components: the production mode which is targeted by the specific discriminant, and other production modes, where the gluon fusion process dominates. |
png pdf |
Figure 7-b:
Distribution of kinematic discriminants in the mass region 118 $ < {m_{4\ell}} < $ 130 GeV: (left) ${{\cal D}^{\mathrm kin}_{\mathrm bkg}}$, (middle) ${{\mathcal {D}}^{\mathrm {VBF}+\mathrm {dec}}_{\mathrm {bkg}}}$, (right) ${{\mathcal {D}}^{\mathrm {VH}+\mathrm {dec}}_{\mathrm {bkg}}}$. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The SM Higgs boson signal is separated into two components: the production mode which is targeted by the specific discriminant, and other production modes, where the gluon fusion process dominates. |

png pdf |
Figure 7-c:
Distribution of kinematic discriminants in the mass region 118 $ < {m_{4\ell}} < $ 130 GeV: (left) ${{\cal D}^{\mathrm kin}_{\mathrm bkg}}$, (middle) ${{\mathcal {D}}^{\mathrm {VBF}+\mathrm {dec}}_{\mathrm {bkg}}}$, (right) ${{\mathcal {D}}^{\mathrm {VH}+\mathrm {dec}}_{\mathrm {bkg}}}$. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The SM Higgs boson signal is separated into two components: the production mode which is targeted by the specific discriminant, and other production modes, where the gluon fusion process dominates. |

png pdf |
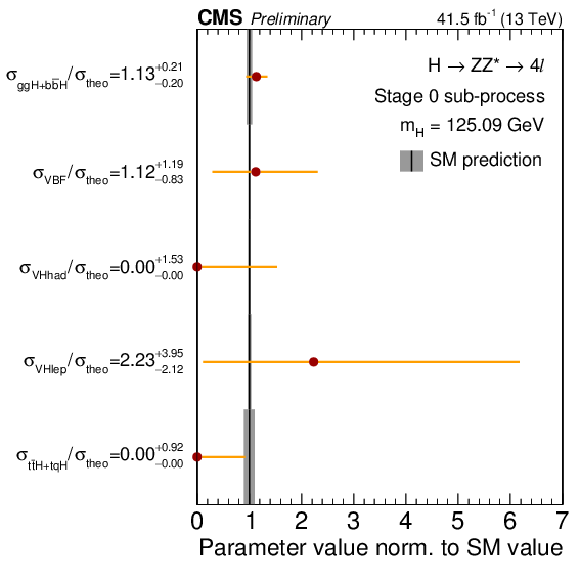
Figure 8:
(Top left) Observed values of the signal strength $\mu =\sigma /\sigma _{SM}$ for the seven event categories, compared to the combined $\mu $ shown as a vertical line with a filled band representing the uncertainty. The horizontal bars indicate the one standard deviation uncertainties. (Top right) Results of likelihood scans for the signal-strength modifiers corresponding to the main SM Higgs boson production modes, compared to the combined $\mu $ shown as a vertical line. The horizontal bars and the filled band indicate the $\pm $ 1$\sigma $ uncertainties. The uncertainties include both statistical and systematic sources. (Bottom left) Result of the 2D likelihood scan for the $ {\mu _{{\mathrm {g}} {\mathrm {g}} {\mathrm {H}},\, {{\mathrm {t}\overline {\mathrm {t}}}} {\mathrm {H}}, {{\mathrm {b}}\bar{{\mathrm {b}}} {\mathrm {H}}}, {{\mathrm {t}} {\mathrm {q}} {\mathrm {H}}}}} $ and $ {\mu _{\mathrm {VBF},\mathrm {V {\mathrm {H}}}}} $ signal-strength modifiers. The solid and dashed contours show the 68% and 95% CL regions, respectively. The cross indicates the best-fit value, and the diamond represents the expected value for the SM Higgs boson. (Bottom right) Results of the fit for simplified template cross sections for the stage 0 sub-processes, normalized to the SM prediction. |

png pdf |
Figure 8-a:
(Top left) Observed values of the signal strength $\mu =\sigma /\sigma _{SM}$ for the seven event categories, compared to the combined $\mu $ shown as a vertical line with a filled band representing the uncertainty. The horizontal bars indicate the one standard deviation uncertainties. (Top right) Results of likelihood scans for the signal-strength modifiers corresponding to the main SM Higgs boson production modes, compared to the combined $\mu $ shown as a vertical line. The horizontal bars and the filled band indicate the $\pm $ 1$\sigma $ uncertainties. The uncertainties include both statistical and systematic sources. (Bottom left) Result of the 2D likelihood scan for the $ {\mu _{{\mathrm {g}} {\mathrm {g}} {\mathrm {H}},\, {{\mathrm {t}\overline {\mathrm {t}}}} {\mathrm {H}}, {{\mathrm {b}}\bar{{\mathrm {b}}} {\mathrm {H}}}, {{\mathrm {t}} {\mathrm {q}} {\mathrm {H}}}}} $ and $ {\mu _{\mathrm {VBF},\mathrm {V {\mathrm {H}}}}} $ signal-strength modifiers. The solid and dashed contours show the 68% and 95% CL regions, respectively. The cross indicates the best-fit value, and the diamond represents the expected value for the SM Higgs boson. (Bottom right) Results of the fit for simplified template cross sections for the stage 0 sub-processes, normalized to the SM prediction. |

png pdf |
Figure 8-b:
(Top left) Observed values of the signal strength $\mu =\sigma /\sigma _{SM}$ for the seven event categories, compared to the combined $\mu $ shown as a vertical line with a filled band representing the uncertainty. The horizontal bars indicate the one standard deviation uncertainties. (Top right) Results of likelihood scans for the signal-strength modifiers corresponding to the main SM Higgs boson production modes, compared to the combined $\mu $ shown as a vertical line. The horizontal bars and the filled band indicate the $\pm $ 1$\sigma $ uncertainties. The uncertainties include both statistical and systematic sources. (Bottom left) Result of the 2D likelihood scan for the $ {\mu _{{\mathrm {g}} {\mathrm {g}} {\mathrm {H}},\, {{\mathrm {t}\overline {\mathrm {t}}}} {\mathrm {H}}, {{\mathrm {b}}\bar{{\mathrm {b}}} {\mathrm {H}}}, {{\mathrm {t}} {\mathrm {q}} {\mathrm {H}}}}} $ and $ {\mu _{\mathrm {VBF},\mathrm {V {\mathrm {H}}}}} $ signal-strength modifiers. The solid and dashed contours show the 68% and 95% CL regions, respectively. The cross indicates the best-fit value, and the diamond represents the expected value for the SM Higgs boson. (Bottom right) Results of the fit for simplified template cross sections for the stage 0 sub-processes, normalized to the SM prediction. |
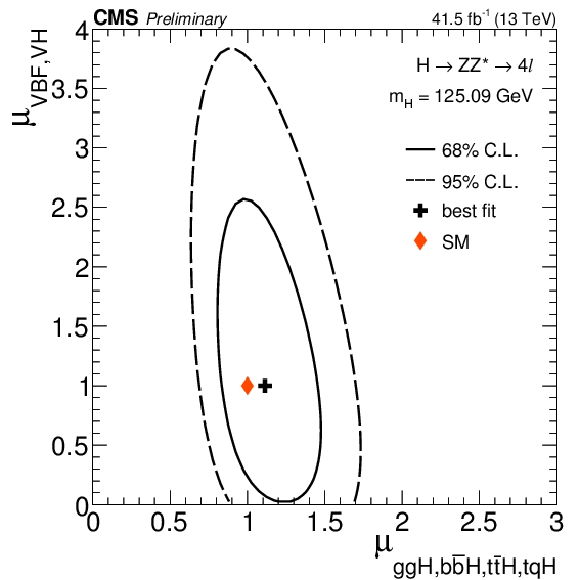
png pdf |
Figure 8-c:
(Top left) Observed values of the signal strength $\mu =\sigma /\sigma _{SM}$ for the seven event categories, compared to the combined $\mu $ shown as a vertical line with a filled band representing the uncertainty. The horizontal bars indicate the one standard deviation uncertainties. (Top right) Results of likelihood scans for the signal-strength modifiers corresponding to the main SM Higgs boson production modes, compared to the combined $\mu $ shown as a vertical line. The horizontal bars and the filled band indicate the $\pm $ 1$\sigma $ uncertainties. The uncertainties include both statistical and systematic sources. (Bottom left) Result of the 2D likelihood scan for the $ {\mu _{{\mathrm {g}} {\mathrm {g}} {\mathrm {H}},\, {{\mathrm {t}\overline {\mathrm {t}}}} {\mathrm {H}}, {{\mathrm {b}}\bar{{\mathrm {b}}} {\mathrm {H}}}, {{\mathrm {t}} {\mathrm {q}} {\mathrm {H}}}}} $ and $ {\mu _{\mathrm {VBF},\mathrm {V {\mathrm {H}}}}} $ signal-strength modifiers. The solid and dashed contours show the 68% and 95% CL regions, respectively. The cross indicates the best-fit value, and the diamond represents the expected value for the SM Higgs boson. (Bottom right) Results of the fit for simplified template cross sections for the stage 0 sub-processes, normalized to the SM prediction. |
png pdf |
Figure 8-d:
(Top left) Observed values of the signal strength $\mu =\sigma /\sigma _{SM}$ for the seven event categories, compared to the combined $\mu $ shown as a vertical line with a filled band representing the uncertainty. The horizontal bars indicate the one standard deviation uncertainties. (Top right) Results of likelihood scans for the signal-strength modifiers corresponding to the main SM Higgs boson production modes, compared to the combined $\mu $ shown as a vertical line. The horizontal bars and the filled band indicate the $\pm $ 1$\sigma $ uncertainties. The uncertainties include both statistical and systematic sources. (Bottom left) Result of the 2D likelihood scan for the $ {\mu _{{\mathrm {g}} {\mathrm {g}} {\mathrm {H}},\, {{\mathrm {t}\overline {\mathrm {t}}}} {\mathrm {H}}, {{\mathrm {b}}\bar{{\mathrm {b}}} {\mathrm {H}}}, {{\mathrm {t}} {\mathrm {q}} {\mathrm {H}}}}} $ and $ {\mu _{\mathrm {VBF},\mathrm {V {\mathrm {H}}}}} $ signal-strength modifiers. The solid and dashed contours show the 68% and 95% CL regions, respectively. The cross indicates the best-fit value, and the diamond represents the expected value for the SM Higgs boson. (Bottom right) Results of the fit for simplified template cross sections for the stage 0 sub-processes, normalized to the SM prediction. |

png pdf |
Figure 9:
Distribution of the four-lepton reconstructed invariant mass $ {m_{4\ell}}$ in the full mass range combining 2016 and 2017. Points with error bars represent the data and stacked histograms represent expected distributions of the signal and background processes. The SM Higgs boson signal with $ {m_{{\mathrm {H}}}} = $ 125 GeV, denoted as ${\mathrm H}(125)$, and the $ {\mathrm {Z}} {\mathrm {Z}}$ backgrounds are normalized to the SM expectation, the $ {\mathrm {Z}}$+X background to the estimation from data. The order in perturbation theory used for the normalization of the irreducible backgrounds is described in Section 7.1. No events are observed with $ {m_{4\ell}} > $ 1.1 TeV. |
png pdf |
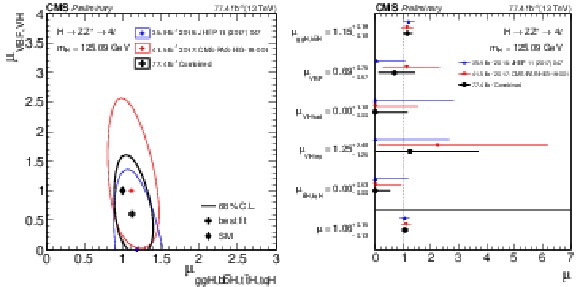
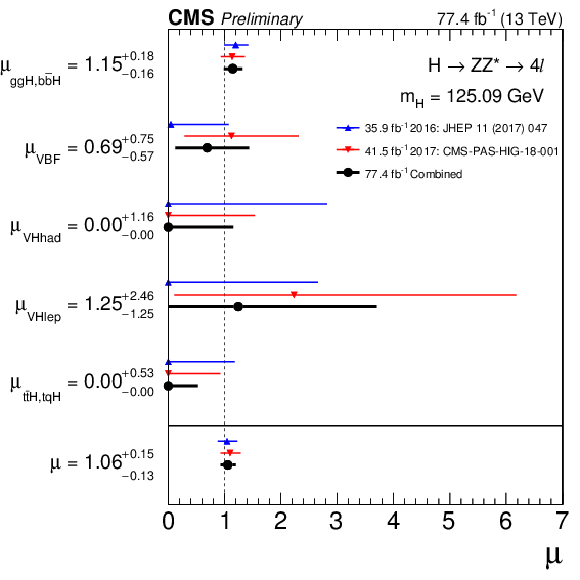
Figure 10:
(Left) Result of the 2D likelihood scan for the $ {\mu _{{\mathrm {g}} {\mathrm {g}} {\mathrm {H}},\, {{\mathrm {t}\overline {\mathrm {t}}}} {\mathrm {H}}, {{\mathrm {b}}\bar{{\mathrm {b}}} {\mathrm {H}}}, {{\mathrm {t}} {\mathrm {q}} {\mathrm {H}}}}} $ and $ {\mu _{\mathrm {VBF},\mathrm {V {\mathrm {H}}}}} $ signal-strength modifiers. The solid contours show the 68% CL regions. The cross indicates the best-fit value, and the diamond represents the expected value for the SM Higgs boson. (Right) Results of likelihood scans for the signal-strength modifiers corresponding to the main SM Higgs boson production modes, compared to the SM expectation shown as a vertical dashed line. The horizontal bars indicate the $\pm $1$\sigma $ uncertainties. The uncertainties include both statistical and systematic sources. The measurements of the global signal strength $\mu $ are also shown. |

png pdf |
Figure 10-a:
(Left) Result of the 2D likelihood scan for the $ {\mu _{{\mathrm {g}} {\mathrm {g}} {\mathrm {H}},\, {{\mathrm {t}\overline {\mathrm {t}}}} {\mathrm {H}}, {{\mathrm {b}}\bar{{\mathrm {b}}} {\mathrm {H}}}, {{\mathrm {t}} {\mathrm {q}} {\mathrm {H}}}}} $ and $ {\mu _{\mathrm {VBF},\mathrm {V {\mathrm {H}}}}} $ signal-strength modifiers. The solid contours show the 68% CL regions. The cross indicates the best-fit value, and the diamond represents the expected value for the SM Higgs boson. (Right) Results of likelihood scans for the signal-strength modifiers corresponding to the main SM Higgs boson production modes, compared to the SM expectation shown as a vertical dashed line. The horizontal bars indicate the $\pm $1$\sigma $ uncertainties. The uncertainties include both statistical and systematic sources. The measurements of the global signal strength $\mu $ are also shown. |
png pdf |
Figure 10-b:
(Left) Result of the 2D likelihood scan for the $ {\mu _{{\mathrm {g}} {\mathrm {g}} {\mathrm {H}},\, {{\mathrm {t}\overline {\mathrm {t}}}} {\mathrm {H}}, {{\mathrm {b}}\bar{{\mathrm {b}}} {\mathrm {H}}}, {{\mathrm {t}} {\mathrm {q}} {\mathrm {H}}}}} $ and $ {\mu _{\mathrm {VBF},\mathrm {V {\mathrm {H}}}}} $ signal-strength modifiers. The solid contours show the 68% CL regions. The cross indicates the best-fit value, and the diamond represents the expected value for the SM Higgs boson. (Right) Results of likelihood scans for the signal-strength modifiers corresponding to the main SM Higgs boson production modes, compared to the SM expectation shown as a vertical dashed line. The horizontal bars indicate the $\pm $1$\sigma $ uncertainties. The uncertainties include both statistical and systematic sources. The measurements of the global signal strength $\mu $ are also shown. |
| Tables | |
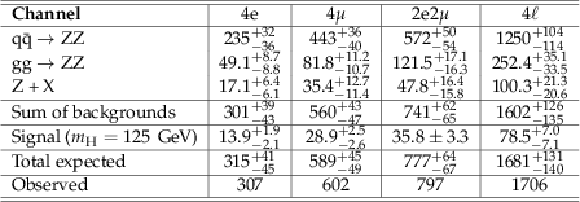
png pdf |
Table 1:
The number of expected background and signal events and number of observed candidates after full analysis selection, for each final state, for the full mass range $ {m_{4\ell}} > $ 70 GeV and for an integrated luminosity of 41.5 fb$^{-1}$. Signal and ZZ backgrounds are estimated from Monte Carlo simulation, $ {\mathrm {Z}}$+X is estimated from data. The uncertainties include both statistical and systematic sources. |

png pdf |
Table 2:
The number of expected background and signal events and number of observed candidates after full analysis selection, for each event category, for the mass range 118 $ < {m_{4\ell}} < $ 130 GeV and for an integrated luminosity of 41.5 fb$^{-1}$. The yields are given for the different production modes. Signal and ZZ backgrounds are estimated from Monte Carlo simulation, $ {\mathrm {Z}}$+X is estimated from data. The uncertainties include both statistical and systematic sources. |

png pdf |
Table 3:
Expected and observed signal-strength modifiers with 2017 data. |

png pdf |
Table 4:
Expected and observed signal-strength modifiers for combined 2016 and 2017 data. |
| Summary |
| Several measurements of Higgs boson production in the four-lepton final state at $\sqrt{s} = $ 13 TeV have been presented, using data samples corresponding to an integrated luminosity of 41.5 fb$^{-1}$. The measured signal strength modifier is $\mu = $ 1.10$^{+0.19}_{-0.17} $, and the measured signal strength modifiers associated with fermions and vector bosons are ${\mu_{\mathrm{g}\mathrm{g}\mathrm{H},\,\mathrm{t\bar{t}}\mathrm{H},{{\mathrm{b}}\bar{{\mathrm{b}}}\mathrm{H}},{{\mathrm{t}}{\mathrm{q}}\mathrm{H}}}} = $ 1.11$^{+0.23}_{-0.21}$ and ${\mu_{\mathrm{VBF},\mathrm{V\mathrm{H}}}} = $ 1.00$^{+0.96}_{-0.71}$, respectively. Results based on data collected in 2016 and 2017 are combined and the measured signal strength modifier is $\mu = $ 1.06$^{+0.15}_{-0.13}$. All results are consistent, within their uncertainties, with the expectations for the SM Higgs boson. |
| References | ||||
| 1 | CMS Collaboration | Observation of a new boson at a mass of 125 GeV with the CMS experiment at the LHC | PLB 716 (2012) 30 | CMS-HIG-12-028 1207.7235 |
| 2 | ATLAS Collaboration | Observation of a new particle in the search for the Standard Model Higgs boson with the ATLAS detector at the LHC | PLB 716 (2012) 1 | 1207.7214 |
| 3 | F. Englert and R. Brout | Broken Symmetry and the Mass of Gauge Vector Mesons | PRL 13 (1964) 321 | |
| 4 | P. W. Higgs | Broken symmetries, massless particles and gauge fields | PL12 (1964) 132 | |
| 5 | P. W. Higgs | Broken Symmetries and the Masses of Gauge Bosons | PRL 13 (1964) 508 | |
| 6 | G. Guralnik, C. Hagen, and T. Kibble | Global Conservation Laws and Massless Particles | PRL 13 (1964) 585 | |
| 7 | P. W. Higgs | Spontaneous Symmetry Breakdown without Massless Bosons | PR145 (1966) 1156 | |
| 8 | T. Kibble | Symmetry breaking in nonAbelian gauge theories | PR155 (1967) 1554 | |
| 9 | CMS Collaboration | Precise determination of the mass of the Higgs boson and tests of compatibility of its couplings with the standard model predictions using proton collisions at 7 and 8 TeV | EPJC 75 (2015) 212 | CMS-HIG-14-009 1412.8662 |
| 10 | ATLAS Collaboration | Measurements of the Higgs boson production and decay rates and coupling strengths using $ pp $ collision data at $ \sqrt{s}= $ 7 and 8 TeV in the ATLAS experiment | EPJC 76 (2016) | 1507.04548 |
| 11 | ATLAS and CMS Collaborations | Combined Measurement of the Higgs Boson Mass in $ pp $ Collisions at $ \sqrt{s}= $ 7 and 8 TeV with the ATLAS and CMS Experiments | PRL 114 (2015) 191803 | 1503.07589 |
| 12 | ATLAS and CMS Collaborations | Measurements of the Higgs boson production and decay rates and constraints on its couplings from a combined ATLAS and CMS analysis of the LHC pp collision data at $ \sqrt{s}= $ 7 and 8 TeV | JHEP 08 (2016) 45 | 1606.02266 |
| 13 | CMS Collaboration | Combined meaurements of the Higgs boson's couplings at $ \sqrt{s}= $ 13 TeV | ||
| 14 | CMS Collaboration | Measurement of the properties of a Higgs boson in the four-lepton final state | PRD 89 (2014) 092007 | CMS-HIG-13-002 1312.5353 |
| 15 | CMS Collaboration | Study of the Mass and Spin-Parity of the Higgs Boson Candidate via Its Decays to $ Z $ Boson Pairs | PRL 110 (2013) 081803 | CMS-HIG-12-041 1212.6639 |
| 16 | CMS Collaboration | Constraints on the spin-parity and anomalous HVV couplings of the Higgs boson in proton collisions at 7 and 8 TeV | PRD 92 (2015) 012004 | CMS-HIG-14-018 1411.3441 |
| 17 | CMS Collaboration | Constraints on the Higgs boson width from off-shell production and decay to $ \mathrm{Z} $-boson pairs | PLB 736 (2014) 64 | CMS-HIG-14-002 1405.3455 |
| 18 | CMS Collaboration | Limits on the Higgs boson lifetime and width from its decay to four charged leptons | PRD 92 (2015) 072010 | CMS-HIG-14-036 1507.06656 |
| 19 | CMS Collaboration | Measurement of differential and integrated fiducial cross sections for Higgs boson production in the four-lepton decay channel in pp collisions at $ \sqrt{s}= $ 7 and 8 TeV | JHEP 04 (2016) 005 | CMS-HIG-14-028 1512.08377 |
| 20 | CMS Collaboration | Measurements of properties of the Higgs boson decaying into the four-lepton final state in pp collisions at $ \sqrt{s}= $ 13 TeV | JHEP 11 (2017) 047 | CMS-HIG-16-041 1706.09936 |
| 21 | CMS Collaboration | Constraints on anomalous Higgs boson couplings using production and decay information in the four-lepton final state | PLB775 (2017) 1--24 | CMS-HIG-17-011 1707.00541 |
| 22 | A. Dominguez et al. | CMS Technical Design Report for the Pixel Detector Upgrade | CERN-LHCC-2012-016. CMS-TDR-11, Sep, 2012 , Additional contacts: Jeffrey Spalding, Fermilab, Jeffrey.Spalding@cern.ch Didier Contardo, Universite Claude Bernard-Lyon I, didier.claude.contardo@cern.ch | |
| 23 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | CMS-00-001 |
| 24 | S. Alioli, P. Nason, C. Oleari, and E. Re | NLO vector-boson production matched with shower in POWHEG | JHEP 07 (2008) 060 | 0805.4802 |
| 25 | P. Nason | A new method for combining NLO QCD with shower Monte Carlo algorithms | JHEP 11 (2004) 040 | hep-ph/0409146 |
| 26 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with parton shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 27 | G. Luisoni, P. Nason, C. Oleari, and F. Tramontano | HW$ ^{\pm} $/HZ + 0 and 1 jet at NLO with the POWHEG BOX interfaced to GoSam and their merging within MiNLO | JHEP 10 (2013) 1 | 1306.2542 |
| 28 | C. Anastasiou et al. | High precision determination of the gluon fusion Higgs boson cross-section at the LHC | JHEP 2016 (2016), no. 5, 1 | 1602.00695 |
| 29 | Y. Gao et al. | Spin determination of single-produced resonances at hadron colliders | PRD 81 (2010) 075022 | 1001.3396 |
| 30 | S. Bolognesi et al. | On the spin and parity of a single-produced resonance at the LHC | PRD 86 (2012) 095031 | 1208.4018 |
| 31 | I. Anderson et al. | Constraining anomalous $ HVV $ interactions at proton and lepton colliders | PRD 89 (2014) 035007 | 1309.4819 |
| 32 | A. V. Gritsan, R. Roentsch, M. Schulze, and M. Xiao | Constraining anomalous Higgs boson couplings to the heavy flavor fermions using matrix element techniques | PRD94 (2016), no. 5, 055023 | 1606.03107 |
| 33 | J. M. Campbell and R. K. Ellis | MCFM for the Tevatron and the LHC | NPPS 205 (2010) 10 | 1007.3492 |
| 34 | NNPDF Collaboration | Parton distributions from high-precision collider data | EPJC 77 (2017) 663 | 1706.00428 |
| 35 | T. Sjostrand et al. | An introduction to pythia 8.2 | Computer Physics Communications 191 (2015) 159 | |
| 36 | CMS Collaboration | Event generator tunes obtained from underlying event and multiparton scattering measurements | EPJC 76 (2016) 155 | CMS-GEN-14-001 1512.00815 |
| 37 | GEANT4 Collaboration | GEANT4: a simulation toolkit | NIMA 506 (2003) 250 | |
| 38 | J. Allison et al. | Geant4 developments and applications | IEEE Trans. Nucl. Sci. 53 (2006) 270 | |
| 39 | CMS Collaboration | Particle-flow reconstruction and global event description with the cms detector | JINST 12 (2017) P10003 | CMS-PRF-14-001 1706.04965 |
| 40 | CMS Collaboration | Performance of CMS muon reconstruction in $ pp $ collision events at $ \sqrt{s} = $ 7 TeV | JINST 7 (2012) P10002 | CMS-MUO-10-004 1206.4071 |
| 41 | CMS Collaboration | Performance of electron reconstruction and selection with the CMS detector in proton-proton collisions at $ \sqrt{s} = $ 8 TeV | JINST 10 (2015) P06005 | CMS-EGM-13-001 1502.02701 |
| 42 | CMS Collaboration | Electron and Photon performance in CMS with the full 2017 data sample and additional highlights for the CALOR 2018 conference | CMS Detector Performance Summary CMS-DPS-2018/2017 | |
| 43 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_t $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 44 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet user manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 45 | CMS Collaboration | Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV | JINST 12 (2017), no. 02, P02014 | CMS-JME-13-004 1607.03663 |
| 46 | CMS Collaboration | Jet algorithms performance in 13 TeV data | CMS-PAS-JME-16-003 | CMS-PAS-JME-16-003 |
| 47 | CMS Collaboration | Identification of heavy-flavour jets with the CMS detector in pp collisions at 13 TeV | JINST 13 (2018), no. 05, P05011 | CMS-BTV-16-002 1712.07158 |
| 48 | Particle Data Group Collaboration | Review of Particle Physics | CPC40 (2016), no. 10, 100001 | |
| 49 | CMS Collaboration | Search for a Higgs Boson in the Mass Range from 145 to 1000 GeV Decaying to a Pair of W or Z Bosons | JHEP 10 (2015) 144 | CMS-HIG-13-031 1504.00936 |
| 50 | M. Grazzini, S. Kallweit, and D. Rathlev | ZZ production at the LHC: Fiducial cross sections and distributions in NNLO QCD | PLB 750 (2015) 407 -- 410 | 1507.06257 |
| 51 | A. Bierweiler, T. Kasprzik, and J. H. Kuhn | Vector-boson pair production at the LHC to $ \mathcal{O}(\alpha^3) $ accuracy | JHEP 12 (2013) 071 | 1305.5402 |
| 52 | M. Bonvini et al. | Signal-background interference effects in $ gg \to H \to WW $ beyond leading order | PRD 88 (2013) 034032 | 1304.3053 |
| 53 | K. Melnikov and M. Dowling | Production of two Z-bosons in gluon fusion in the heavy top quark approximation | PLB 744 (2015) 43 | 1503.01274 |
| 54 | C. S. Li, H. T. Li, D. Y. Shao, and J. Wang | Soft gluon resummation in the signal-background interference process of $ gg(\to h^*) \to ZZ $~ | 1504.02388 | |
| 55 | G. Passarino | Higgs CAT | EPJC 74 (2014) 2866 | 1312.2397 |
| 56 | S. Catani and M. Grazzini | An NNLO subtraction formalism in hadron collisions and its application to Higgs boson production at the LHC | PRL 98 (2007) 222002 | hep-ph/0703012 |
| 57 | M. Grazzini | NNLO predictions for the Higgs boson signal in the H $ \to $ WW $ \to\ell\nu\ell\nu $ and H$ \to $ ZZ $ \to4\ell $ decay channels | JHEP 02 (2008) 043 | 0801.3232 |
| 58 | M. Grazzini and H. Sargsyan | Heavy-quark mass effects in Higgs boson production at the LHC | JHEP 09 (2013) 129 | 1306.4581 |
| 59 | L. Landau | On the energy loss of fast particles by ionization | J. Phys. (USSR) 8 (1944)201 | |
| 60 | D. de Florian, G. Ferrera, M. Grazzini, and D. Tommasini | Higgs boson production at the LHC: transverse momentum resummation effects in the $ H \to \gamma \gamma $, $ H \to WW \to \ell\nu\ell\nu $ and $ H \to ZZ \to 4\ell $ decay modes | JHEP 06 (2012) 132 | 1203.6321 |
| 61 | E. Bagnaschi, G. Degrassi, P. Slavich, and A. Vicini | Higgs production via gluon fusion in the POWHEG approach in the SM and in the MSSM | JHEP 02 (2012) 088 | 1111.2854 |
| 62 | K. Hamilton, P. Nason, E. Re, and G. Zanderighi | NNLOPS simulation of Higgs boson production | JHEP 10 (2013) 222 | 1309.0017 |
| 63 | LHC Higgs Cross Section Working Group | Handbook of LHC Higgs Cross Sections: 4. Deciphering the Nature of the Higgs Sector | CERN-2016-XXX (CERN, Geneva, 2016) | 1610.07922 |
| 64 | F. Garwood | Fiducial Limits for the Poisson Distribution | Biometrika 28 (1936), no. 3-4, 437--442 | |

|
Compact Muon Solenoid LHC, CERN |

|

|

|

|

|

|