Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-PAS-TOP-14-013 | ||
| Measurement of double differential cross sections for top quark pair production in pp collisions at $\sqrt{s} =$ 8 TeV | ||
| CMS Collaboration | ||
| July 2016 | ||
| Abstract: Normalized double differential cross sections for top quark pair ($\rm{t\bar{t}}$) production are measured in pp collisions at a centre-of-mass energy of 8 TeV with the CMS experiment at the LHC. The analyzed data correspond to an integrated luminosity of 19.7 fb$^{-1}$. The measurement is performed using the dilepton ${\rm e}^{\pm}\mu^{\mp}$ decay mode of the $\rm{t\bar{t}}$ system. The $\rm{t\bar{t}}$ cross section is measured as a function of various combinations of two observables characterizing the kinematics of the top quark and the $\rm{t\bar{t}}$ system. The data are compared to calculations in perturbative QCD at next-to-leading order and approximate next-to-next-to-leading order, and to predictions of Monte Carlo event generators that complement fixed-order computations with parton showers, hadronization, and multiple-parton interactions. Overall, a reasonable agreement is observed which is improved when the latest global sets of proton parton distribution functions are used in the predictions. The impact of the measured cross sections constraining the gluon distribution in the proton is demonstrated in a fit of parametrized parton distribution functions to the data. | ||
|
Links:
CDS record (PDF) ;
CADI line (restricted) ;
These preliminary results are superseded in this paper, EPJC 77 (2017) 459. The superseded preliminary plots can be found here. |
||
| Figures | |
png pdf |
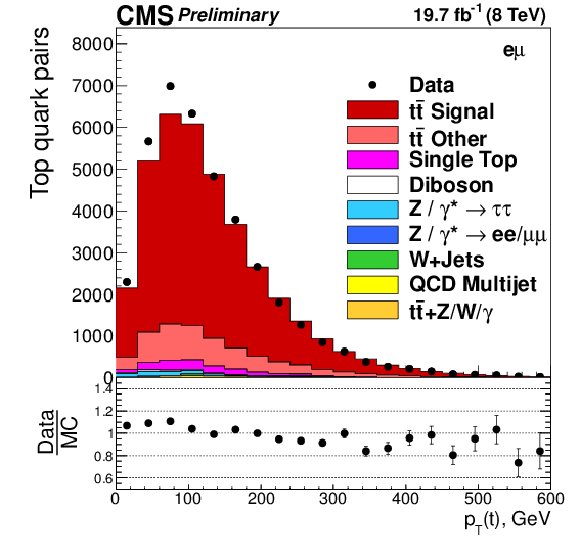
Figure 1-a:
Distributions of $p_{T}(\rm t)$ (a), $|y(\rm t)|$ (b), $p_{T}({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ (c), $|y({\mathrm{ t } {}\mathrm{ \bar{t} } } )|$ (d) and $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ (e) in events after the kinematic reconstruction. The experimental data with the vertical bars corresponding to the data statistical uncertainties are plotted together with distributions of simulated signal and different background processes. The lower panel in each plot shows the ratio of the observed data event yields to those expected in the simulation. |
png pdf |
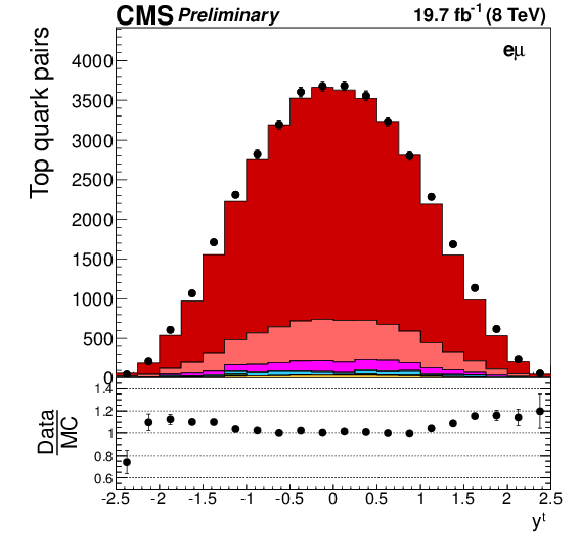
Figure 1-b:
Distributions of $p_{T}(\rm t)$ (a), $|y(\rm t)|$ (b), $p_{T}({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ (c), $|y({\mathrm{ t } {}\mathrm{ \bar{t} } } )|$ (d) and $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ (e) in events after the kinematic reconstruction. The experimental data with the vertical bars corresponding to the data statistical uncertainties are plotted together with distributions of simulated signal and different background processes. The lower panel in each plot shows the ratio of the observed data event yields to those expected in the simulation. |
png pdf |
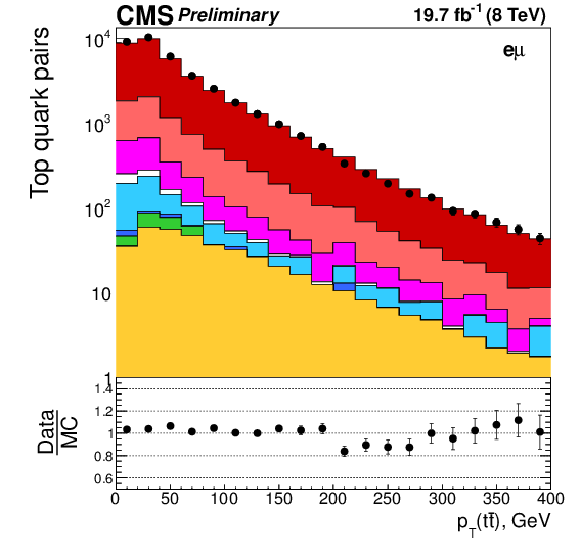
Figure 1-c:
Distributions of $p_{T}(\rm t)$ (a), $|y(\rm t)|$ (b), $p_{T}({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ (c), $|y({\mathrm{ t } {}\mathrm{ \bar{t} } } )|$ (d) and $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ (e) in events after the kinematic reconstruction. The experimental data with the vertical bars corresponding to the data statistical uncertainties are plotted together with distributions of simulated signal and different background processes. The lower panel in each plot shows the ratio of the observed data event yields to those expected in the simulation. |
png pdf |
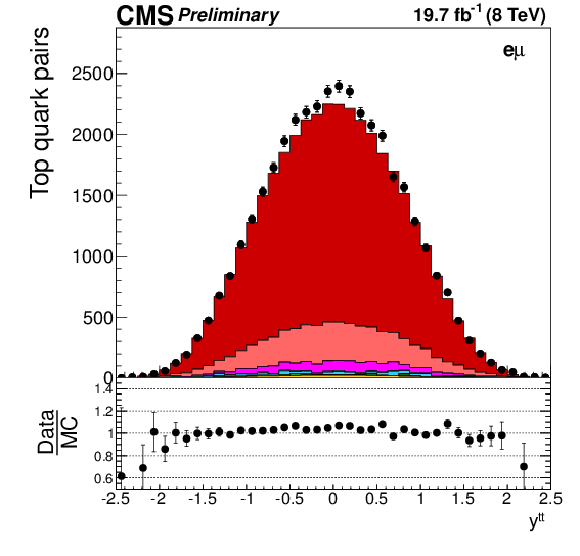
Figure 1-d:
Distributions of $p_{T}(\rm t)$ (a), $|y(\rm t)|$ (b), $p_{T}({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ (c), $|y({\mathrm{ t } {}\mathrm{ \bar{t} } } )|$ (d) and $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ (e) in events after the kinematic reconstruction. The experimental data with the vertical bars corresponding to the data statistical uncertainties are plotted together with distributions of simulated signal and different background processes. The lower panel in each plot shows the ratio of the observed data event yields to those expected in the simulation. |
png pdf |
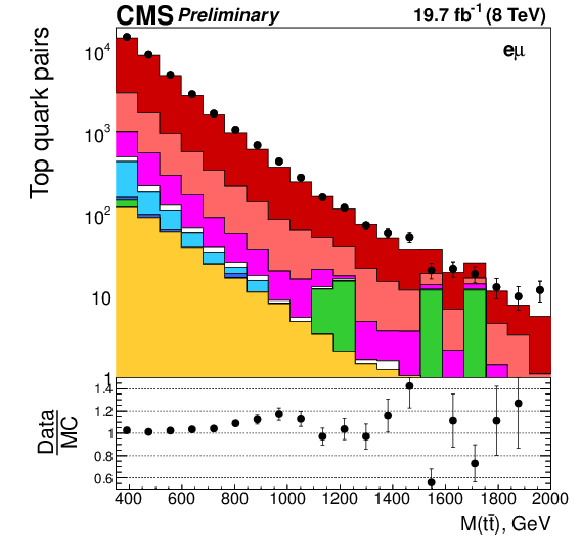
Figure 1-e:
Distributions of $p_{T}(\rm t)$ (a), $|y(\rm t)|$ (b), $p_{T}({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ (c), $|y({\mathrm{ t } {}\mathrm{ \bar{t} } } )|$ (d) and $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ (e) in events after the kinematic reconstruction. The experimental data with the vertical bars corresponding to the data statistical uncertainties are plotted together with distributions of simulated signal and different background processes. The lower panel in each plot shows the ratio of the observed data event yields to those expected in the simulation. |
png pdf |
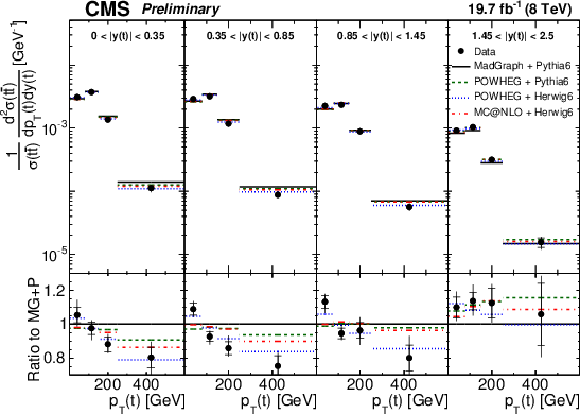
Figure 2:
Comparison of the measured normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ differential cross sections as a function of ${ {p_{\mathrm {T}}} ({\rm t})}$ in different ${y({\rm t})}$ ranges to MC predictions calculated using MadGraph+Pythia6 , Powheg+Pythia6 , Powheg+Herwig6 and mc@nlo+Herwig6. The inner vertical bars on the data points represent the statistical uncertainties and the full bars include also the systematic uncertainties added in quadrature. In the bottom panel the ratios to the MadGraph+Pythia6 (MG+P) predictions are shown. |
png pdf |
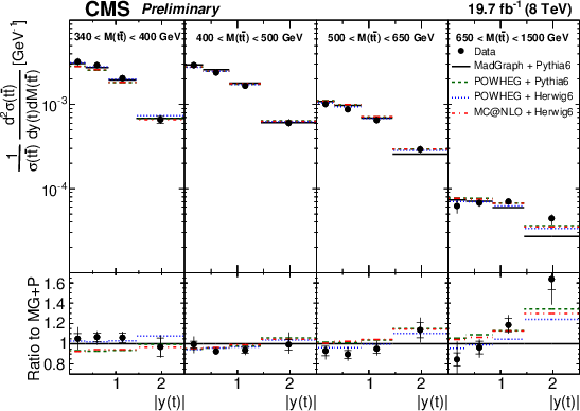
Figure 3:
Comparison of the measured normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ differential cross sections as a function of ${y({\rm t})}$ in different $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ ranges to MC predictions. Details can be found in the caption of Fig. 2. |
png pdf |
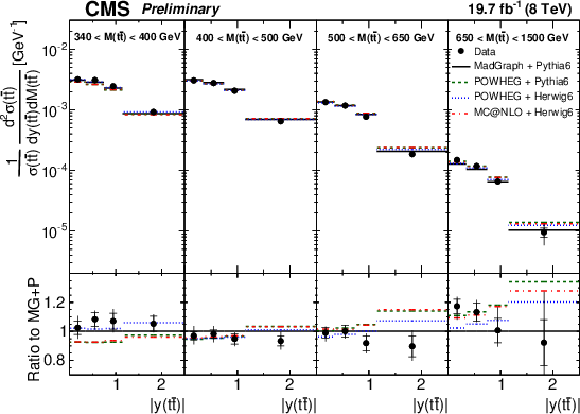
Figure 4:
Comparison of the measured normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ differential cross sections as a function of ${y({\mathrm{ t } {}\mathrm{ \bar{t} } } )}$ in different of $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ ranges to MC predictions. Details can be found in the caption of Fig. 2. |
png pdf |
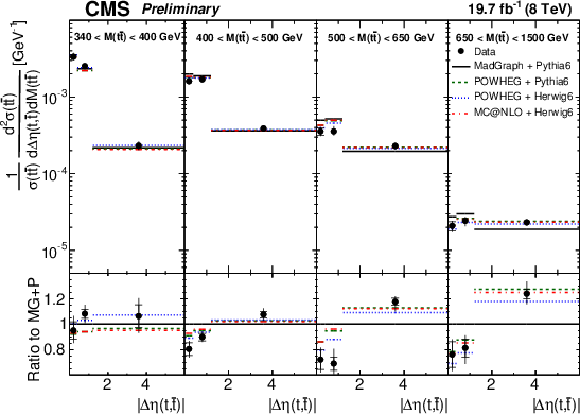
Figure 5:
Comparison of the measured normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ differential cross sections as a function of ${\Delta \eta (\mathrm{t},\mathrm{\bar{t}})}$ in different $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ ranges to MC predictions. Details can be found in the caption of Fig. 2. |
png pdf |
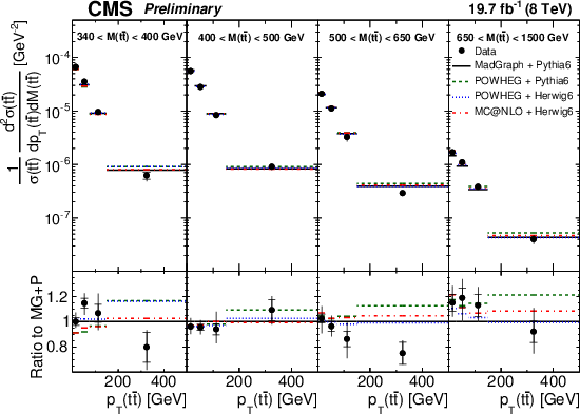
Figure 6:
Comparison of the measured normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ differential cross sections as a function of ${ {p_{\mathrm {T}}} ({\mathrm{ t } {}\mathrm{ \bar{t} } } )}$ in different $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ ranges to MC predictions. Details can be found in the caption of Fig. 2. |
png pdf |
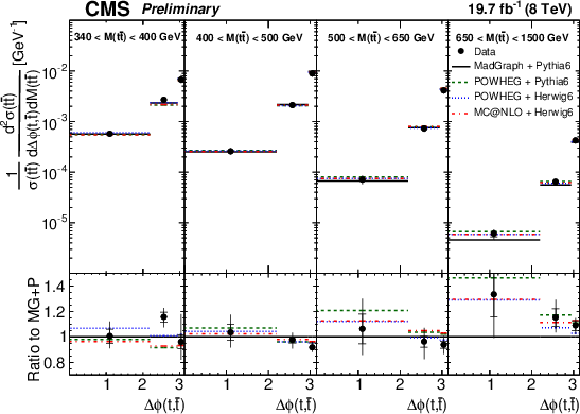
Figure 7:
Comparison of the measured normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ differential cross sections as a function of ${\Delta \phi (\mathrm{t}, \mathrm{\bar{t}})}$ in different $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ ranges to MC predictions. Details can be found in the caption of Fig. 2. |
png pdf |
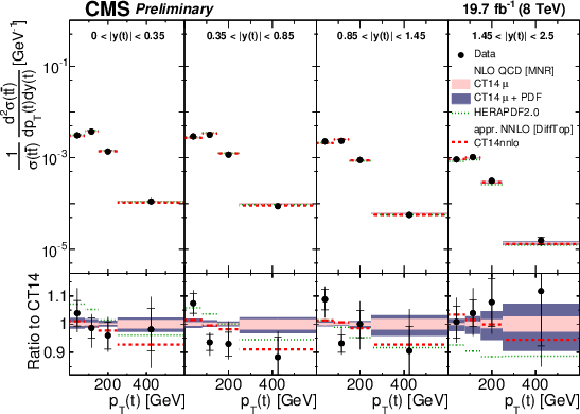
Figure 8:
Comparison of the measured normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ differential cross sections as a function of ${ {p_{\mathrm {T}}} ({\rm t})}$ in different ${y({\rm t})}$ ranges to NLO $O(\alpha _s^3)$ and approximate NNLO $O(\alpha _s^4)$ predictions calculated with CT14 and HERAPDF2.0. The inner vertical bars on the data points represent the statistical uncertainties and the full bars include also the systematic uncertainties added in quadrature. The light band on the CT14 theoretical predictions represents the scale uncertainties of the NLO predictions and the dark band shows the scale and PDF uncertainties added in quadrature. The dotted line shows NLO predictions calculated with HERAPDF2.0 . The dashed line shows approximate NNLO predictions calculated with CT14 . In the bottom panel the ratios to the CT14 predictions are shown. |
png pdf |
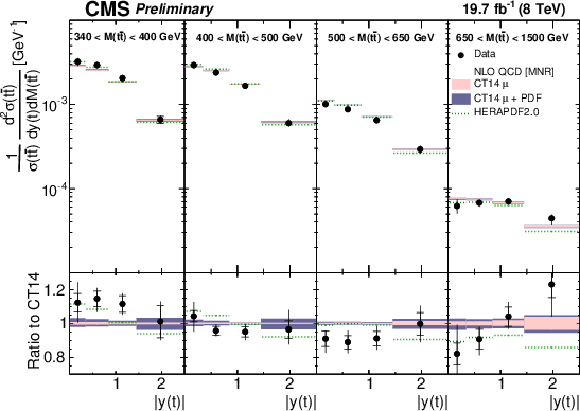
Figure 9:
Comparison of the measured normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } } $ differential cross sections as a function of ${y({\rm t})}$ in different $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ ranges to NLO $O(\alpha _s^3)$ predictions. Details can be found in the caption of Fig. 8. Approximate NNLO $O(\alpha _s^4)$ predictions are not available for this distribution. |
png pdf |
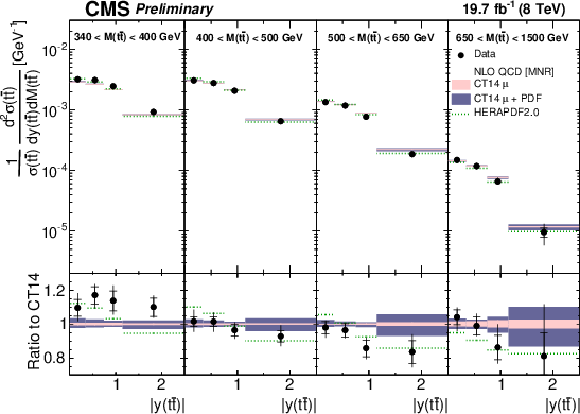
Figure 10:
Comparison of the measured normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ differential cross sections as a function of ${y({\mathrm{ t } {}\mathrm{ \bar{t} } } )}$ in different $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ ranges to NLO $O(\alpha _s^3)$ predictions. Details can be found in the caption of Fig. 8. Approximate NNLO $O(\alpha _s^4)$ predictions are not available for this distribution. |
png pdf |
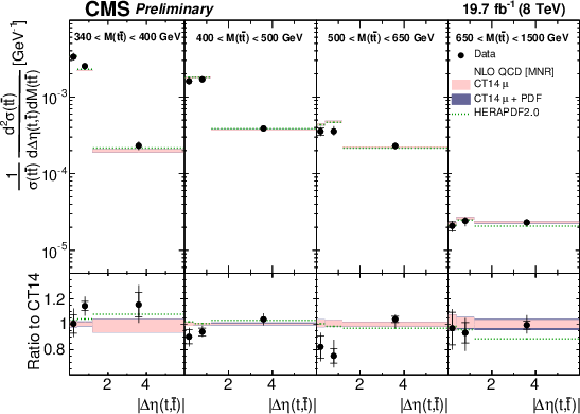
Figure 11:
Comparison of the measured normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ differential cross sections as a function of ${\Delta \eta (\mathrm{t}, \mathrm{\bar{t}})}$ in different $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ ranges to NLO $O(\alpha _s^3)$ predictions. Details can be found in the caption of Fig. 8. Approximate NNLO $O(\alpha _s^4)$ predictions are not available for this distribution. |
png pdf |
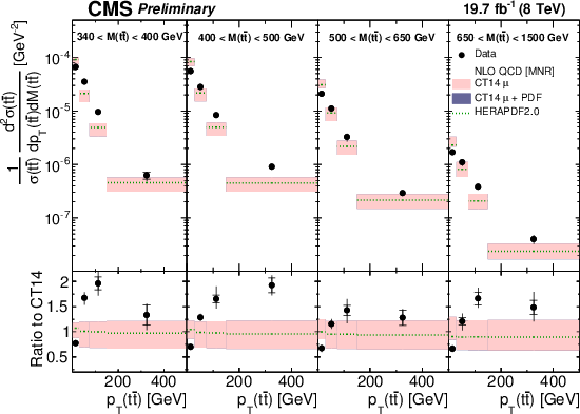
Figure 12:
Comparison of the measured normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ differential cross sections as a function of ${ {p_{\mathrm {T}}} ({\mathrm{ t } {}\mathrm{ \bar{t} } } )}$ in different $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ ranges to NLO $O(\alpha _s^3)$ predictions. Details can be found in the caption of Fig. 8. Approximate NNLO $O(\alpha _s^4)$ predictions are not available for this distribution. |
png pdf |
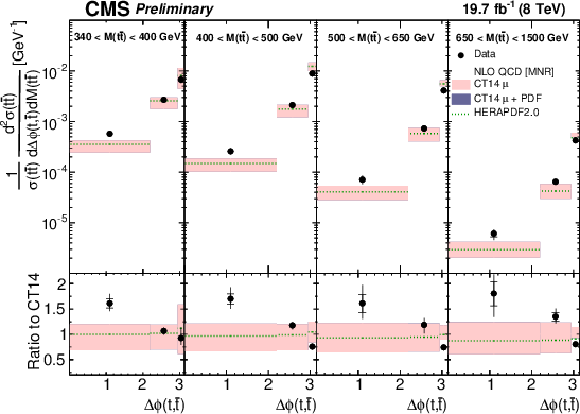
Figure 13:
Comparison of the measured normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ differential cross sections as a function of ${\Delta \phi (\mathrm{t}, \mathrm{\bar{t}})}$ in different $M({\mathrm{ t } {}\mathrm{ \bar{t} } } )$ ranges to NLO $O(\alpha _s^3)$ predictions. Details can be found in the caption of Fig. 8. Approximate NNLO $O(\alpha _s^4)$ predictions are not available for this distribution. |
png pdf |
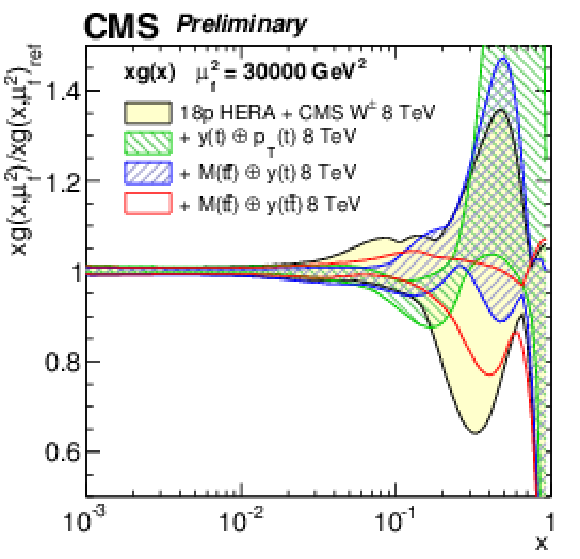
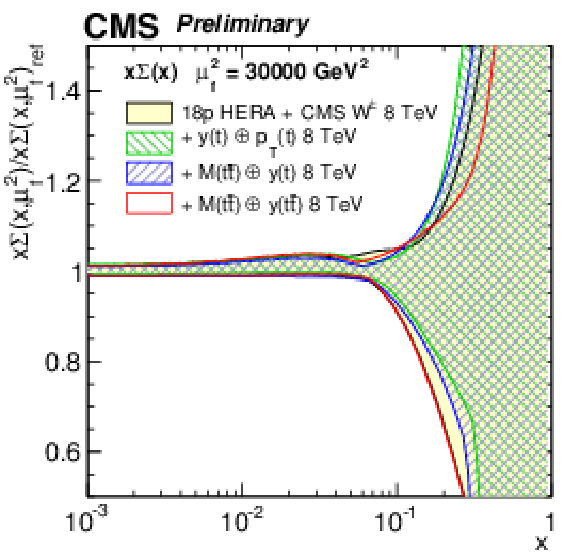
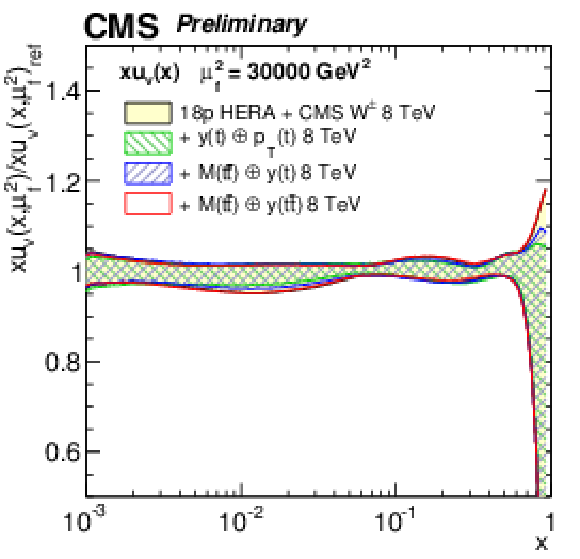
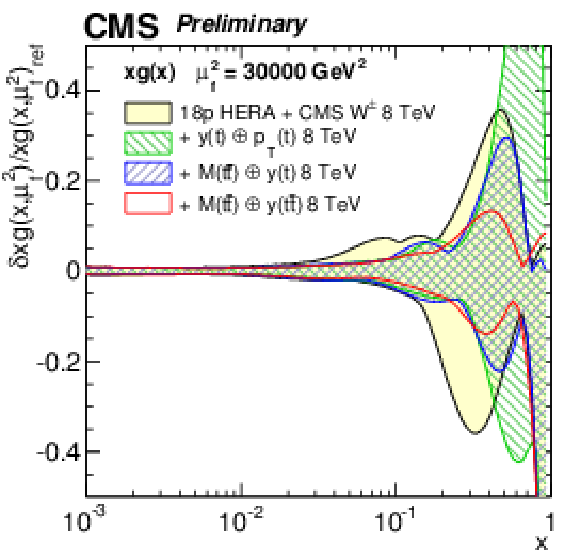
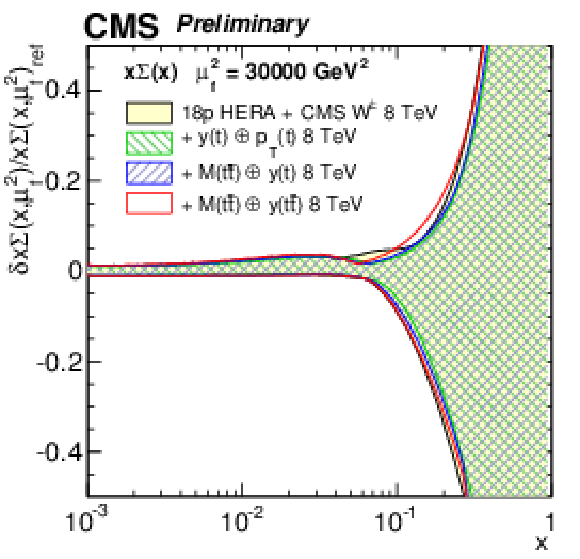
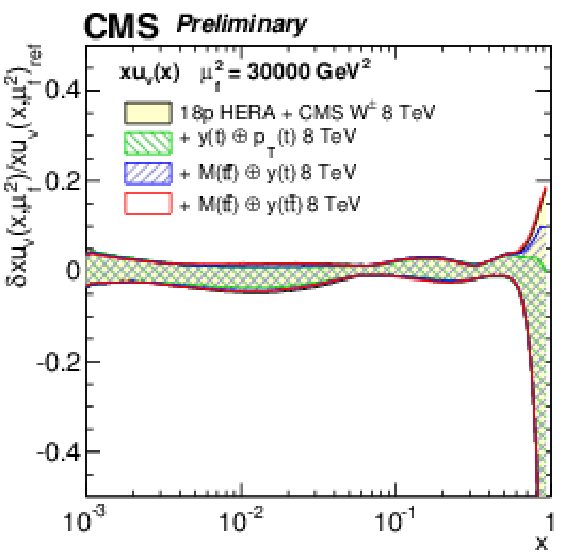
Figure 14-a:
The gluon (a), sea quark (b), u valence (c) and d valence quark (d) distributions at $\mu _f^2=$ 30 000 GeV$^2$, as obtained in all variants of the PDF fit, normalized to the results from the fit using the DIS and ${\rm W}^{\pm }$ boson charge asymmetry data only. The total uncertainty of each distribution is shown by a shaded (or hatched) band. 18p stands for the 18-parameter fit as described in the text. |
png pdf |
Figure 14-b:
The gluon (a), sea quark (b), u valence (c) and d valence quark (d) distributions at $\mu _f^2=$ 30 000 GeV$^2$, as obtained in all variants of the PDF fit, normalized to the results from the fit using the DIS and ${\rm W}^{\pm }$ boson charge asymmetry data only. The total uncertainty of each distribution is shown by a shaded (or hatched) band. 18p stands for the 18-parameter fit as described in the text. |
png pdf |
Figure 14-c:
The gluon (a), sea quark (b), u valence (c) and d valence quark (d) distributions at $\mu _f^2=$ 30 000 GeV$^2$, as obtained in all variants of the PDF fit, normalized to the results from the fit using the DIS and ${\rm W}^{\pm }$ boson charge asymmetry data only. The total uncertainty of each distribution is shown by a shaded (or hatched) band. 18p stands for the 18-parameter fit as described in the text. |
png pdf |
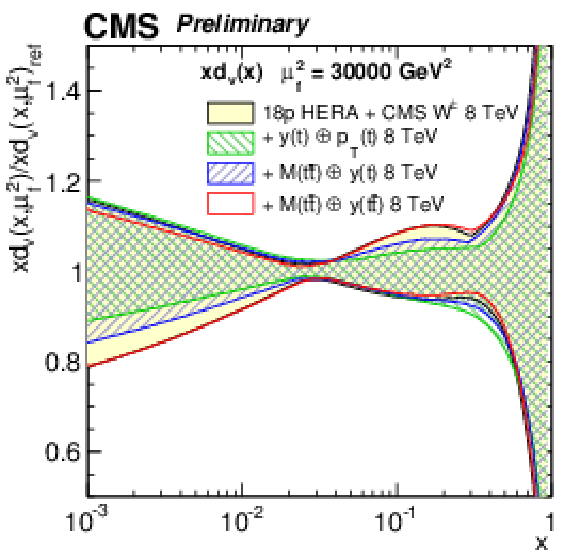
Figure 14-d:
The gluon (a), sea quark (b), u valence (c) and d valence quark (d) distributions at $\mu _f^2=$ 30 000 GeV$^2$, as obtained in all variants of the PDF fit, normalized to the results from the fit using the DIS and ${\rm W}^{\pm }$ boson charge asymmetry data only. The total uncertainty of each distribution is shown by a shaded (or hatched) band. 18p stands for the 18-parameter fit as described in the text. |
png pdf |
Figure 15-a:
Fractional total uncertainties of the gluon (a), sea quark (b), u valence (c) and d valence quark (d) distributions at $\mu _f^2=$ 30 000 GeV$^2$, shown by shaded (or hatched) bands, as obtained in all variants of the PDF fit. 18p stands for the 18-parameter fit as described in the text. |
png pdf |
Figure 15-b:
Fractional total uncertainties of the gluon (a), sea quark (b), u valence (c) and d valence quark (d) distributions at $\mu _f^2=$ 30 000 GeV$^2$, shown by shaded (or hatched) bands, as obtained in all variants of the PDF fit. 18p stands for the 18-parameter fit as described in the text. |
png pdf |
Figure 15-c:
Fractional total uncertainties of the gluon (a), sea quark (b), u valence (c) and d valence quark (d) distributions at $\mu _f^2=$ 30 000 GeV$^2$, shown by shaded (or hatched) bands, as obtained in all variants of the PDF fit. 18p stands for the 18-parameter fit as described in the text. |
png pdf |
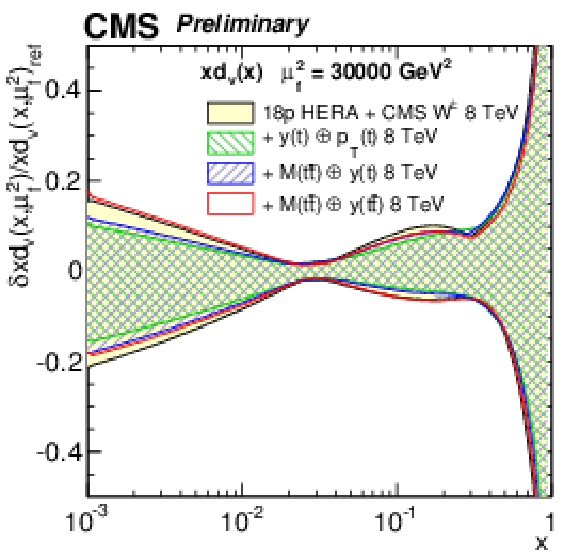
Figure 15-d:
Fractional total uncertainties of the gluon (a), sea quark (b), u valence (c) and d valence quark (d) distributions at $\mu _f^2=$ 30 000 GeV$^2$, shown by shaded (or hatched) bands, as obtained in all variants of the PDF fit. 18p stands for the 18-parameter fit as described in the text. |
png pdf |
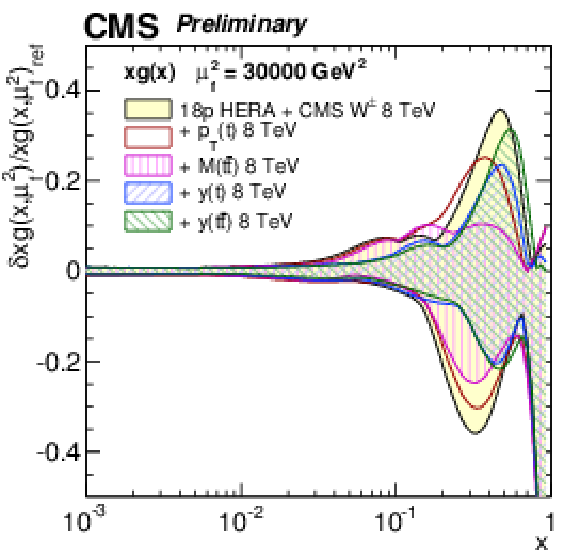
Figure 16-a:
Same as in Fig. 15 for the variants of the PDF fit using the single differential ${\mathrm {t}\overline {\mathrm {t}}}$ cross sections. |
png pdf |
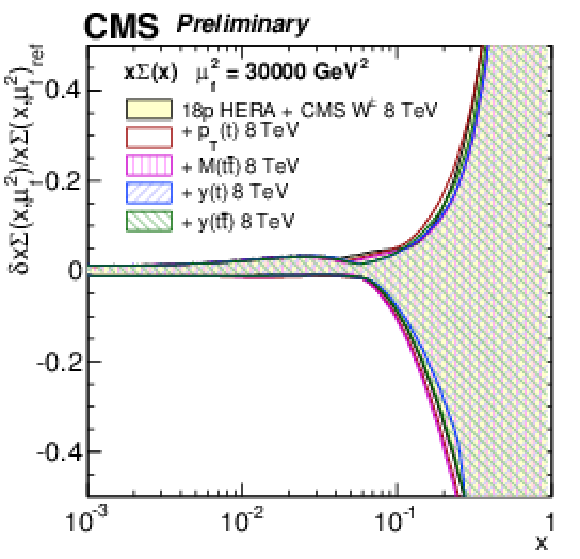
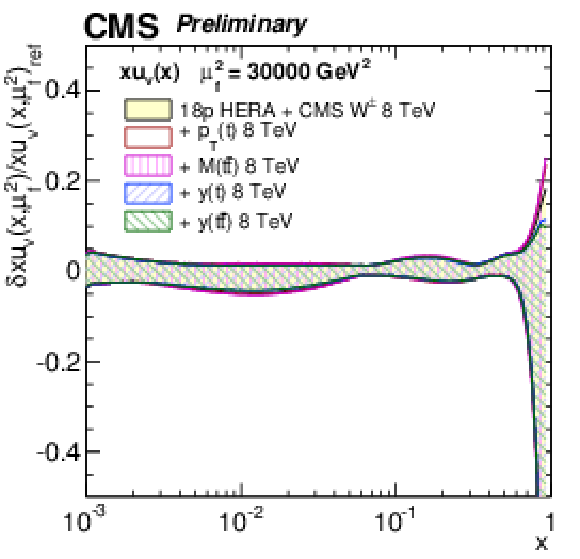
Figure 16-b:
Same as in Fig. 15 for the variants of the PDF fit using the single differential ${\mathrm {t}\overline {\mathrm {t}}}$ cross sections. |
png pdf |
Figure 16-c:
Same as in Fig. 15 for the variants of the PDF fit using the single differential ${\mathrm {t}\overline {\mathrm {t}}}$ cross sections. |
png pdf |
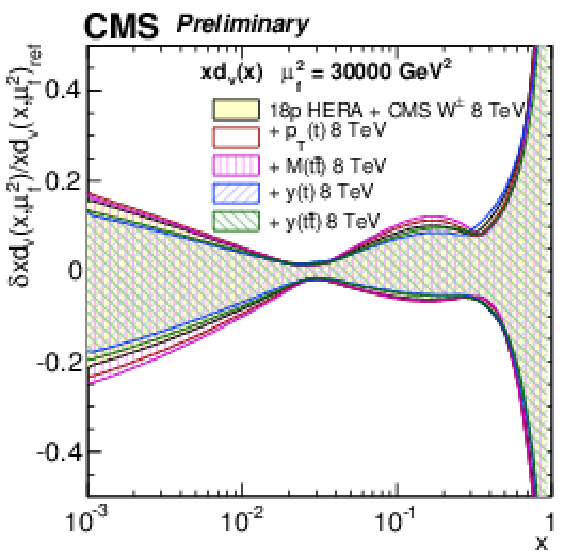
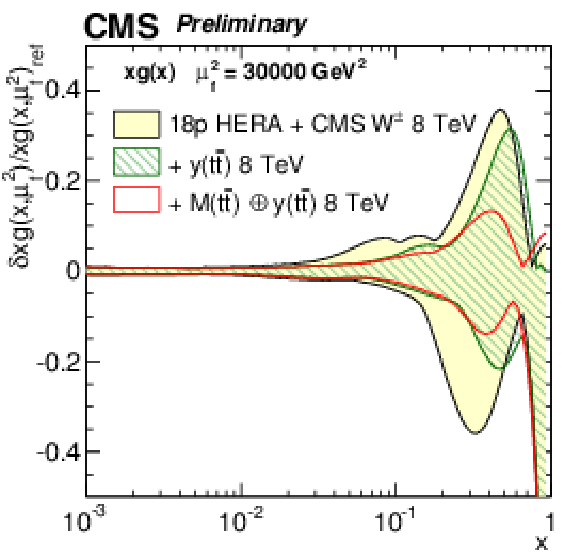
Figure 16-d:
Same as in Fig. 15 for the variants of the PDF fit using the single differential ${\mathrm {t}\overline {\mathrm {t}}}$ cross sections. |
png pdf |
Figure 17:
Fractional total uncertainties of the gluon distribution at $\mu _f^2=$ 30 000 GeV$^2$, shown by shaded (or hatched) bands, as obtained in the PDF fit using the DIS and ${\rm W}^{\pm }$ boson charge asymmetry data only, as well as single and double differential ${\mathrm {t}\overline {\mathrm {t}}}$ cross sections. 18p stands for the 18-parameter fit as described in the text. |
| Tables | |
png pdf |
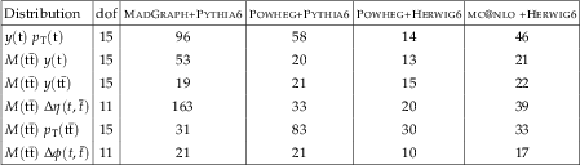
Table 1:
The ${\chi ^2}$ values of double differential normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ cross sections with respect to MC calculations. |
png pdf |
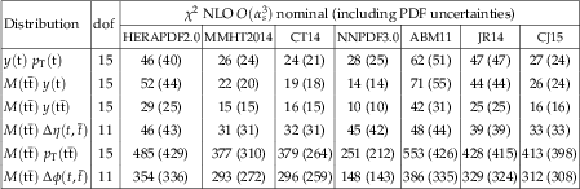
Table 2:
The ${\chi ^2}$ values of double differential normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ cross sections with respect to NLO $O(\alpha _s^3)$ theoretical calculations [17] using different PDF sets. The ${\chi ^2}$ values that include PDF uncertainties are shown in parenthesis. |
png pdf |
Table 3:
The ${\chi ^2}$ values of double differential normalized ${\mathrm{ t } {}\mathrm{ \bar{t} } }$ cross sections with respect to approximate NNLO $O(\alpha _s^4)$ theoretical calculations [4,18,65,66] using different PDF sets. |
png pdf |
Table 4:
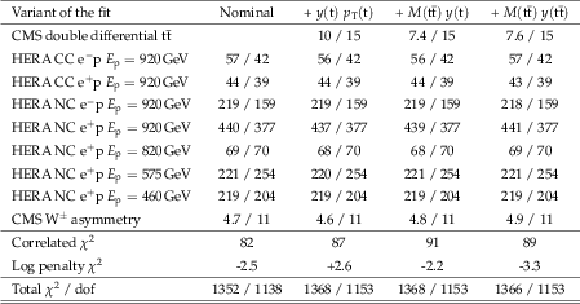
The global and partial ${\chi ^2}$/dof values for the data sets in all variants of the PDF fit. The variant of the fit which uses the DIS and ${\rm W}^{\pm }$ boson charge asymmetry data only is denoted as `Nominal', Every double differential ${\mathrm {t}\overline {\mathrm {t}}}$ distribution is added ($+$) to the nominal data, one at a time. For HERA measurements, the energy of the proton beam, $E_{ {\mathrm {p}}}$, is listed for each data set, with electron energy being $E_{ {\mathrm {e}}}=$ 27.5 GeV. The `Correlated ${\chi ^2} $' and `Log penalty $\chi ^2$' entries refer to the ${\chi ^2}$ contributions from the nuisance parameters and from the logarithmic term, respectively, described in the text. |
| Summary |
| The first measurement of normalized double differential $\mathrm{ t \bar{t} }$ production cross sections in pp collisions at $ \sqrt{s} = $ 8 TeV is presented. The measurement is performed for the ${\rm e}\mu$ final state, using 19.7 fb$^{-1}$ of collision data collected by the CMS detector. The normalized $\mathrm{ t \bar{t} }$ cross section is measured in the full phase space as a function of six different combinations of variables, which describe the top quark or $\mathrm{ t \bar{t} }$ kinematics. The observed trends support the findings reported in the previous single differential $\mathrm{ t \bar{t} }$ measurement, in particular the tendency of a softer distribution of $p_{\mathrm{T}}(\mathrm{t})$ in data compared to the MC predictions. The presented double differential studies reveal a broader distribution of $y(\mathrm{t})$ at high $M(\mathrm{ t \bar{t} })$ in data than in MC and a larger pseudorapidity separation $\Delta \eta (\mathrm{t}, \mathrm{\bar{t}})$ at moderate $M(\mathrm{ t \bar{t} })$ in data compared to MC. The data are in agreement with NLO QCD predictions based on modern PDF sets. The measured double differential cross sections have been incorporated into a PDF fit together with other data from HERA and LHC. A significant impact on the gluon distributions at large values of $x$ is observed, in particular when the distribution of $y({\mathrm{t \bar{t}}})$ in the $M(\mathrm{ t \bar{t} })$ ranges is used. It exceeds the impact of single differential normalized $\mathrm{ t \bar{t} }$ cross sections, thus strongly suggests the use of these measurements in future PDF fits. |
| References | ||||
| 1 | D0 Collaboration | Observation of the top quark | PRL 74 (1995) 2632--2637 | hep-ex/9503003 |
| 2 | CDF Collaboration | Observation of top quark production in $ \bar{p}p $ collisions | PRL 74 (1995) 2626--2631 | hep-ex/9503002 |
| 3 | M. Czakon, M. L. Mangano, A. Mitov, and J. Rojo | Constraints on the gluon PDF from top quark pair production at hadron colliders | JHEP 07 (2013) 167 | 1303.7215 |
| 4 | M. Guzzi, K. Lipka, and S.-O. Moch | Top-quark pair production at hadron colliders: differential cross section and phenomenological applications with DiffTop | JHEP 01 (2015) 082 | 1406.0386 |
| 5 | CMS Collaboration | Constraints on parton distribution functions and extraction of the strong coupling constant from the inclusive jet cross section in pp collisions at $ \sqrt{s} = 7 $ $ \,\text {TeV} $ | EPJC 75 (2015), no. 6, 288 | CMS-SMP-12-028 1410.6765 |
| 6 | CMS Collaboration | Measurements of differential jet cross sections in proton-proton collisions at $ \sqrt{s}=7 $ TeV with the CMS detector | PRD 87 (2013), no. 11, 112002, , [Erratum: Phys. Rev. D \bf 87 (2013), no. 11, 119902] | CMS-QCD-11-004 1212.6660 |
| 7 | CDF Collaboration | First Measurement of the $ t{\bar t} $ Differential Cross Section $ d\sigma/dM_{t{\bar t}} $ in $ p{\bar p} $ Collisions at $ \sqrt{s} $ = 1.96 TeV | PRL 102 (2009) 222003 | 0903.2850 |
| 8 | D0 Collaboration | Measurement of differential $ t\bar{t} $ production cross sections in $ p\bar{p} $ collisions | PRD 90 (2014), no. 9, 092006 | 1401.5785 |
| 9 | ATLAS Collaboration | Measurements of top quark pair relative differential cross-sections with ATLAS in pp collisions at $ \sqrt{s} $ = 7 TeV | EPJC 73 (2013) 2261 | 1207.5644 |
| 10 | CMS Collaboration | Measurement of differential top-quark pair production cross sections in pp collisions at $ \sqrt{s} = $ 7 TeV | EPJC 73 (2013) 2339 | CMS-TOP-11-013 1211.2220 |
| 11 | ATLAS Collaboration | Measurements of normalized differential cross-sections for $ t{\bar t} $ production in $ pp $ collisions at $ \sqrt{s} $ = 7 TeV using the ATLAS detector | PRD 90 (2014) 072004 | 1407.0371 |
| 12 | ATLAS Collaboration | Measurement of top quark pair differential cross-sections in the dilepton channel in $ pp $ collisions at $ \sqrt{s} $ = 7 and 8 TeV with ATLAS | Submitted to PRD | 1607.07281 |
| 13 | CMS Collaboration | Measurement of the differential cross section for top quark pair production in pp collisions at $ \sqrt{s} = 8\,\text {TeV} $ | EPJC 75 (2015), no. 11, 542 | CMS-TOP-12-028 1505.04480 |
| 14 | ATLAS Collaboration | Measurements of top-quark pair differential cross-sections in the lepton+jets channel in $ pp $ collisions at $ \sqrt{s}=8 $ TeV using the ATLAS detector | 1511.04716 | |
| 15 | CMS Collaboration | Measurement of the differential cross section for $ \mathrm{t \bar t} $ production in the dilepton final state at $ \sqrt{s}=13 \mathrm{TeV} $ | ||
| 16 | CMS Collaboration | Measurement of the inclusive and differential tt production cross sections in lepton + jets final states at 13 TeV | ||
| 17 | M. L. Mangano, P. Nason, and G. Ridolfi | Heavy quark correlations in hadron collisions at next-to-leading order | Nucl. Phys. B 373 (1992) 295--345 | |
| 18 | N. Kidonakis, E. Laenen, S. Moch, and R. Vogt | Sudakov resummation and finite order expansions of heavy quark hadroproduction cross-sections | PRD 64 (2001) 114001 | hep-ph/0105041 |
| 19 | J. Alwall et al. | MadGraph 5 : Going Beyond | JHEP 06 (2011) 128 | 1106.0522 |
| 20 | P. Artoisenet et al. | Automatic spin-entangled decays of heavy resonances in Monte Carlo simulations | JHEP 03 (2013) 015 | 1212.3460 |
| 21 | T. Sj\"ostrand, S. Mrenna, and P. Z. Skands | PYTHIA 6.4 Physics and Manual | JHEP 05 (2006) 026 | hep-ph/0603175 |
| 22 | J. Pumplin et al. | New generation of parton distributions with uncertainties from global QCD analysis | JHEP 07 (2002) 012 | hep-ph/0201195 |
| 23 | S. Alioli et al. | NLO single-top production matched with shower in POWHEG: $ s $- and $ t $-channel contributions | JHEP 09 (2009) 111 | 0907.4076 |
| 24 | S. Alioli et al. | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX | JHEP 06 (2010) 043 | 1002.2581 |
| 25 | E. Re | Single-top Wt-channel production matched with parton showers using the POWHEG method | EPJC 71 (2011) 1547 | 1009.2450 |
| 26 | G. Corcella et al. | HERWIG 6.5: An event generator for hadron emission reactions with interfering gluons (including supersymmetric processes) | JHEP 01 (2001) 010 | hep-ph/0011363 |
| 27 | S. Frixione and B. R. Webber | Matching NLO QCD computations and parton shower simulations | JHEP 06 (2002) 29 | hep-ph/0204244 |
| 28 | H.-L. Lai et al. | New parton distributions for collider physics | PRD 82 (2010) 074024 | 1007.2241 |
| 29 | GEANT4 Collaboration | GEANT4: A Simulation toolkit | NIMA 506 (2003) 250--303 | |
| 30 | CMS Collaboration | Particle-Flow Event Reconstruction in CMS and Performance for Jets, Taus, and $ E_{\mathrm{T}}^{\text{miss}} $ | CDS | |
| 31 | CMS Collaboration | Commissioning of the particle-flow event reconstruction with the first LHC collisions recorded in the CMS detector | CDS | |
| 32 | M. Cacciari, G. P. Salam, and G. Soyez | The catchment area of jets | JHEP 04 (2008) 005 | 0802.1188 |
| 33 | CMS Collaboration | Performance of Electron Reconstruction and Selection with the CMS Detector in Proton-Proton Collisions at $ \sqrt{s} = 8 $ TeV | JINST 10 (2015), no. 06, P06005 | CMS-EGM-13-001 1502.02701 |
| 34 | CMS Collaboration | Measurement of the Drell-Yan Cross Section in $ pp $ Collisions at $ \sqrt{s}=7 $ TeV | JHEP 10 (2011) 007 | CMS-EWK-10-007 1108.0566 |
| 35 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_t $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 36 | M. Cacciari, G. P. Salam, and G. Soyez | FastJet User Manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 37 | CMS Collaboration | Identification of b-quark jets with the CMS experiment | JINST 8 (2013) P04013 | CMS-BTV-12-001 1211.4462 |
| 38 | CMS Collaboration | Missing transverse energy performance of the CMS detector | JINST 6 (2011) P09001 | CMS-JME-10-009 1106.5048 |
| 39 | CMS Collaboration | Performance of missing transverse energy reconstruction by the CMS experiment in $ \sqrt{s}= $ 8 TeV pp data | JINST 10 (2015) P02006 | CMS-JME-13-003 1411.0511 |
| 40 | D0 Collaboration | Measurement of the top quark mass using dilepton events. D$ \O\ $ Collaboration | PRL 80 (1998) 2063--2068 | hep-ex/9706014 |
| 41 | I. Korol | Measurement of Double Differential $\mathrm{t \bar{t}}$ Production Cross Sections with the CMS Detector | PhD thesis, Universit\"at Hamburg (2016) DESY-THESIS-2016-011 | |
| 42 | S. Schmitt | TUnfold: an algorithm for correcting migration effects in high energy physics | JINST 7 (2012) T10003 | 1205.6201 |
| 43 | A. N. Tikhonov | Solution of incorrectly formulated problems and the regularization method | Soviet Math. Dokl. 4 (1963) 1035--1038 | |
| 44 | V. Blobel | Data Unfolding | Terascale statistics school Hamburg (2010) | |
| 45 | Particle Data Group Collaboration | Review of Particle Physics | CPC 38 (2014) 090001 | |
| 46 | TOTEM Collaboration | First measurement of the total proton-proton cross section at the LHC energy of $ \sqrt{s} $ = 7 TeV | Europhys. Lett. 96 (2011) 21002 | 1110.1395 |
| 47 | CMS Collaboration | Performance of b tagging at $ \sqrt{s}=8 $ TeV in multijet, ttbar and boosted topology events | Technical Report CMS-PAS-BTV-13-001, CERN, Geneva (2013) | |
| 48 | CMS Collaboration | Jet energy scale and resolution in the CMS experiment in pp collisions at 8 TeV | Submitted to JINST | CMS-JME-13-004 1607.03663 |
| 49 | CMS Collaboration | Determination of jet energy calibration and transverse momentum resolution in CMS | JINST 6 (2011) P11002 | CMS-JME-10-011 1107.4277 |
| 50 | CMS Collaboration | CMS Luminosity Based on Pixel Cluster Counting - Summer 2013 Update | CMS-PAS-LUM-13-001 | CMS-PAS-LUM-13-001 |
| 51 | CMS Collaboration | Measurement of the top quark mass using proton-proton data at $ {\sqrt{s}} $ = 7 and 8 TeV | PRD 93 (2016), no. 7, 072004 | CMS-TOP-14-022 1509.04044 |
| 52 | N. Kidonakis | NNNLO soft-gluon corrections for the top-quark $ p_T $ and rapidity distributions | PRD 91 (2015), no. 3, 031501 | 1411.2633 |
| 53 | M. Czakon, D. Heymes, and A. Mitov | High-precision differential predictions for top-quark pairs at the LHC | PRL 116 (2016), no. 8, 082003 | 1511.00549 |
| 54 | M. Czakon, D. Heymes, and A. Mitov | Dynamical scales for multi-TeV top-pair production at the LHC | 1606.03350 | |
| 55 | J. M. Campbell and R. K. Ellis | MCFM for the Tevatron and the LHC | NPPS 205-206 (2010) 10--15 | 1007.3492 |
| 56 | S. Alekhin, J. Blumlein, and S. Moch | Parton Distribution Functions and Benchmark Cross Sections at NNLO | PRD 86 (2012) 054009 | 1202.2281 |
| 57 | A. Accardi et al. | Constraints on large-$ x $ parton distributions from new weak boson production and deep-inelastic scattering data | PRD 93 (2016), no. 11, 114017 | 1602.03154 |
| 58 | S. Dulat et al. | New parton distribution functions from a global analysis of quantum chromodynamics | PRD 93 (2016), no. 3, 033006 | 1506.07443 |
| 59 | ZEUS, H1 Collaboration | Combination of measurements of inclusive deep inelastic $ {e^{\pm }p} $ scattering cross sections and QCD analysis of HERA data | EPJC 75 (2015), no. 12, 580 | 1506.06042 |
| 60 | P. Jimenez-Delgado and E. Reya | Delineating parton distributions and the strong coupling | PRD 89 (2014), no. 7, 074049 | 1403.1852 |
| 61 | L. A. Harland-Lang, A. D. Martin, P. Motylinski, and R. S. Thorne | Parton distributions in the LHC era: MMHT 2014 PDFs | EPJC 75 (2015), no. 5, 204 | 1412.3989 |
| 62 | NNPDF Collaboration | Parton distributions for the LHC Run II | JHEP 04 (2015) 040 | 1410.8849 |
| 63 | A. Buckley et al. | LHAPDF6: parton density access in the LHC precision era | EPJC 75 (2015) 132 | 1412.7420 |
| 64 | M. Cacciari et al. | Updated predictions for the total production cross sections of top and of heavier quark pairs at the Tevatron and at the LHC | JHEP 09 (2008) 127 | 0804.2800 |
| 65 | M. Guzzi, K. Lipka, and S.-O. Moch | Differential cross sections for top pair production at the LHC | Nuclear and Particle Physics Proceedings (2015) NPPP601, , [PoSDIS2014,052(2014)] | 1409.0444 |
| 66 | M. Guzzi, K. Lipka, and S.-O. Moch | Top-quark production at the LHC: differential cross section and phenomenological applications | PoS DIS2013 (2013) 049 | 1308.1635 |
| 67 | S. Alekhin, J. Blumlein, and S. Moch | The ABM parton distributions tuned to LHC data | PRD 89 (2014), no. 5, 054028 | 1310.3059 |
| 68 | CMS Collaboration | Measurement of the differential cross section and charge asymmetry for inclusive pp to W + X production at $ \sqrt{s}=8 $ TeV | Submitted to EPJC | CMS-SMP-14-022 1603.01803 |
| 69 | S. Alekhin et al. | HERAFitter | EPJC 75 (2015), no. 7, 304 | 1410.4412 |
| 70 | xFitter group | xFitter | xFitter web site | |
| 71 | V. Gribov and L. Lipatov | Deep inelastic $ ep $ scattering in perturbation theory | Sov.J.Nucl.Phys. 15 (1972)438--450 | |
| 72 | G. Altarelli and G. Parisi | Asymptotic Freedom in Parton Language | Nucl. Phys. B 126 (1977) 298 | |
| 73 | G. Curci, W. Furmanski, and R. Petronzio | Evolution of Parton Densities Beyond Leading Order: The Nonsinglet Case | Nucl. Phys. B 175 (1980) 27 | |
| 74 | W. Furmanski and R. Petronzio | Singlet Parton Densities Beyond Leading Order | PLB 97 (1980) 437 | |
| 75 | S. Moch, J. Vermaseren, and A. Vogt | The Three-Loop Splitting Functions in QCD: The Nonsinglet Case | Nucl. Phys. B 688 (2004) 101--134 | hep-ph/0403192 |
| 76 | A. Vogt, S. Moch, and J. Vermaseren | The Three-Loop Splitting Functions in QCD: The Singlet Case | Nucl. Phys. B 691 (2004) 129--181 | hep-ph/0404111 |
| 77 | M. Botje | QCDNUM: Fast QCD Evolution and Convolution | CPC 182 (2011) 490--532 | 1005.1481 |
| 78 | R. S. Thorne and R. G. Roberts | An Ordered analysis of heavy flavor production in deep inelastic scattering | PRD 57 (1998) 6871--6898 | hep-ph/9709442 |
| 79 | R. S. Thorne | A Variable-flavor number scheme for NNLO | PRD 73 (2006) 054019 | hep-ph/0601245 |
| 80 | R. S. Thorne | Effect of changes of variable flavor number scheme on parton distribution functions and predicted cross sections | PRD 86 (2012) 074017 | 1201.6180 |
| 81 | J. M. Campbell and R. K. Ellis | An Update on vector boson pair production at hadron colliders | PRD 60 (1999) 113006 | hep-ph/9905386 |
| 82 | T. Carli et al. | A posteriori inclusion of parton density functions in NLO QCD final-state calculations at hadron colliders: The APPLGRID Project | EPJC 66 (2010) 503--524 | 0911.2985 |
| 83 | A. D. Martin, W. J. Stirling, R. S. Thorne, and G. Watt | Parton distributions for the LHC | EPJC 63 (2009) 189--285 | 0901.0002 |
| 84 | CMS Collaboration | Measurement of the muon charge asymmetry in inclusive $ pp \to W+X $ production at $ \sqrt s = $ 7 TeV and an improved determination of light parton distribution functions | PRD 90 (2014), no. 3, 032004 | CMS-SMP-12-021 1312.6283 |
| 85 | H1 Collaboration | Inclusive Deep Inelastic Scattering at High $ Q^2 $ with Longitudinally Polarised Lepton Beams at HERA | JHEP 09 (2012) 061 | 1206.7007 |
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|