CMS-PAS-HIN-16-008

CMS-PAS-HIN-16-008
Strong suppression of $\Upsilon$ excited states in PbPb collisions at $\sqrt{s_{NN}} = $ 5.02 TeV
CMS Collaboration
September 2016

Abstract: Measurements of $\Upsilon$ mesons, produced in pp and PbPb collisions at $\sqrt{s_{NN}} = $ 5.02 TeV and reconstructed via their dimuon decay channel, are reported using data collected by the CMS experiment. Double ratios are formed by comparing the yields of the excited states to the ground state in both PbPb and pp collisions at the same energy. The ${\Upsilon (\textrm {2S})}$ double ratio is studied as a function of centrality, dimuon transverse momentum, and rapidity. We find suppression in all centrality bins and no variation with kinematics. For the ${\Upsilon (\textrm {3S})}$, no significant signal is found in the PbPb data. A search in centrality bins shows that the double ratio is significantly below unity at all centralities, an observation that indicates strong suppression in heavy-ion collisions. The centrality-integrated double ratios are 0.308 $\pm$ 0.055 (stat) $\pm$ 0.017 (syst) for the ${\Upsilon (\textrm {2S})}$ and less than 0.26 at 95% confidence level for the ${\Upsilon (\textrm {3S})}$.
Links: CDS record (PDF) ; CADI line (restricted) ; These preliminary results are superseded in this paper, PRL 120 (2018) 142301. The superseded preliminary plots can be found here.

Figures	Summary	Additional Figures	References	CMS Publications

Figures
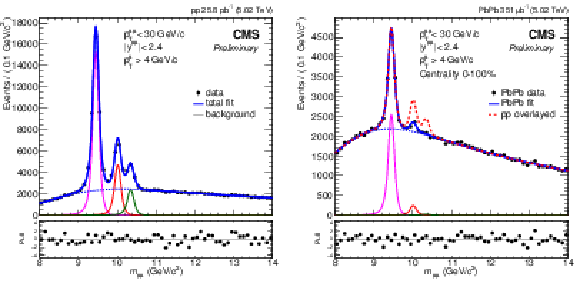
png pdf	Figure 1: Dimuon invariant mass distributions (black circles) for pp data (left) and centrality-integrated PbPb data (right). The fit is shown as a solid blue line and the background component as a dashed blue line. ${\Upsilon (\textrm {1S})}$, ${\Upsilon (\textrm {2S})}$, and ${\Upsilon (\textrm {3S})}$ are also depicted as solid magenta, red and green lines, respectively. The dashed red line in the PbPb panel represents the pp ${\Upsilon }$ signal fit normalized at the ${\Upsilon (\textrm {1S})}$ mass. The lower panels show the pull distributions.
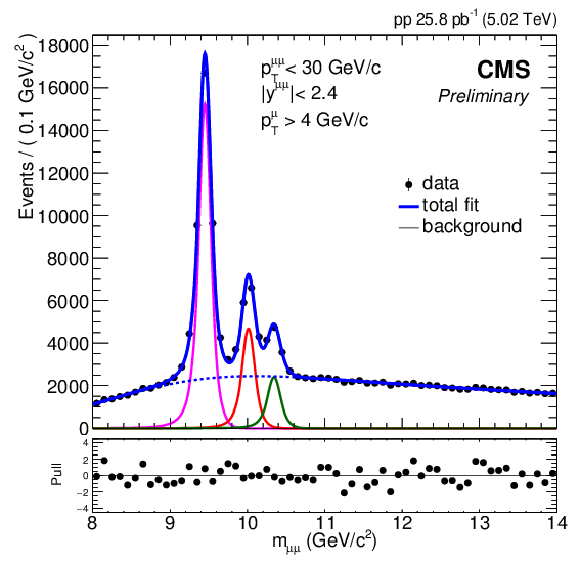
png pdf	Figure 1-a: Dimuon invariant mass distributions (black circles) for pp data. The fit is shown as a solid blue line and the background component as a dashed blue line. ${\Upsilon (\textrm {1S})}$, ${\Upsilon (\textrm {2S})}$, and ${\Upsilon (\textrm {3S})}$ are also depicted as solid magenta, red and green lines, respectively. The lower panel shows the pull distributions.
png pdf	Figure 1-b: Dimuon invariant mass distributions (black circles) for centrality-integrated PbPb data. The fit is shown as a solid blue line and the background component as a dashed blue line. ${\Upsilon (\textrm {1S})}$, ${\Upsilon (\textrm {2S})}$, and ${\Upsilon (\textrm {3S})}$ are also depicted as solid magenta, red and green lines, respectively. The dashed red line represents the pp ${\Upsilon }$ signal fit normalized at the ${\Upsilon (\textrm {1S})}$ mass. The lower panel shows the pull distributions.
png pdf	Figure 2: Double ratio of $ {\Upsilon (\textrm {2S})} $ as a function of centrality. The centrality-integrated value is shown in the right sub-panel. The error bars represent the statistical uncertainty from the PbPb data, while the boxes represent the systematic uncertainty. The box drawn around the line at unity depicts the systematic and statistical uncertainties from pp as well as the systematic uncertainties due to the combined detection efficiency, which are applicable to all points.
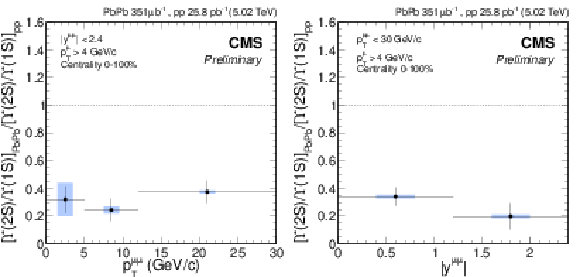
png pdf	Figure 3: Double ratio of the $ {\Upsilon (\textrm {2S})} $ as a function of ${p_{\mathrm {T}}} $ (left) and rapidity (right). The error bars depict the statistical uncertainty while the boxes represent the systematic uncertainties (signal PDF, background PDF, and combined detection efficiency). The contributions from both PbPb and pp are incorporated in the uncertainties.
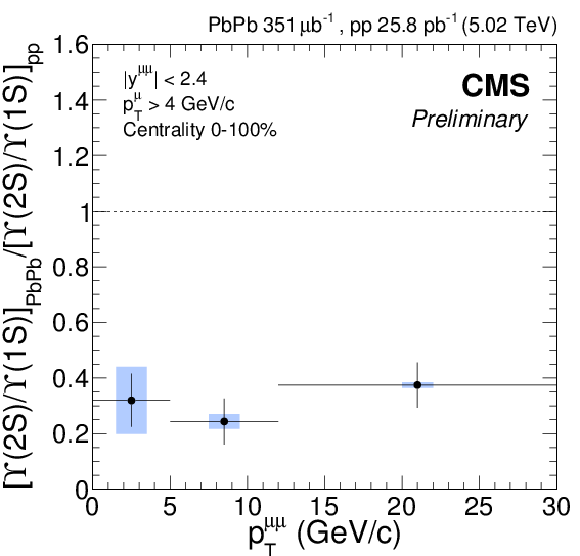
png pdf	Figure 3-a: Double ratio of the $ {\Upsilon (\textrm {2S})} $ as a function of ${p_{\mathrm {T}}} $. The error bars depict the statistical uncertainty while the boxes represent the systematic uncertainties (signal PDF, background PDF, and combined detection efficiency). The contributions from both PbPb and pp are incorporated in the uncertainties.
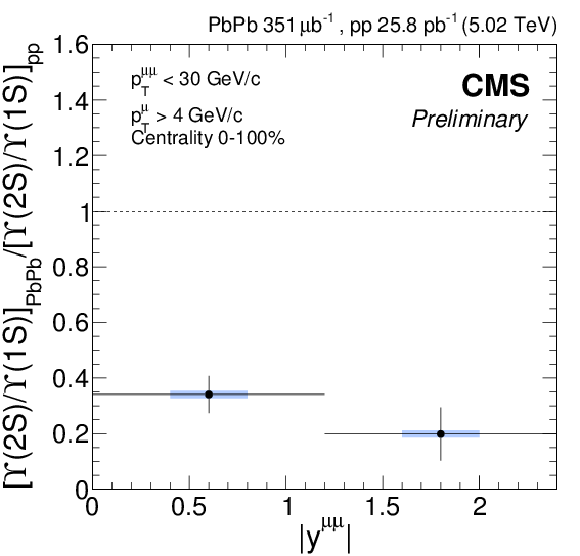
png pdf	Figure 3-b: Double ratio of the $ {\Upsilon (\textrm {2S})} $ as a function of rapidity. The error bars depict the statistical uncertainty while the boxes represent the systematic uncertainties (signal PDF, background PDF, and combined detection efficiency). The contributions from both PbPb and pp are incorporated in the uncertainties.
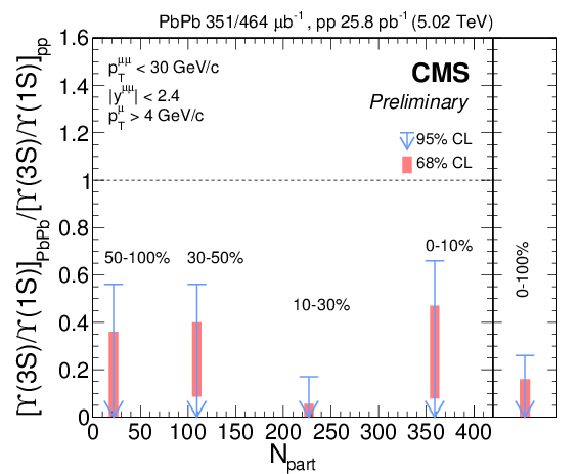
png pdf	Figure 4: Upper limits at 95% CL (blue arrows) and 68% CL (red boxes) of the $ {\Upsilon (\textrm {3S})}$ double ratio as a function of centrality. The centrality-integrated limit is shown in the right sub-panel.

Summary

In summary, we presented measurements of the ${\Upsilon (\textrm {2S})}$ and ${\Upsilon (\textrm {3S})}$ double ratios, at 5.02 TeV, using pp and PbPb data samples significantly larger than those used in the measurement reported in Ref. [16], devoted to the 2.76 TeV data. Indeed, the integrated luminosities increased by a factor of 2.3 for the PbPb sample and by more than a factor 100 for the pp sample. Furthermore, the ${\Upsilon}$ production cross sections should increase with the collision energy by around a factor of 1.8, from 2.76 to 5.02 TeV. The centrality-integrated double ratios are $DR_{21} =$ 0.308 $\pm$ 0.055(stat) $\pm$ 0.017(syst) for the ${\Upsilon (\textrm {2S})}$ and $DR_{31} < $ 0.26 at 95% CL for the ${\Upsilon (\textrm {3S})}$. The large relative suppression of the ${\Upsilon (\textrm {2S})}$ does not show significant variations with $p_{\mathrm{T}}$ and $| y | $, within the explored phase space window of $p_{\mu\mu} < $ 30 GeV/$c$ and $ | y_{\mu\mu} | < $ 2.4. In the most peripheral bin (70-100%), the ${\Upsilon (\textrm {2S})}$ double ratio is consistent with unity. For the most central bin (0-5%), it is consistent with zero and the upper limit on the double ratio is evaluated to be 0.36 at 95% CL. The ${\Upsilon (\textrm {3S})}$ double ratio is significantly lower than unity in all centrality bins of the analysis and there is no indication that the suppression is weaker in the most peripheral events probed in our study.

Additional Figures

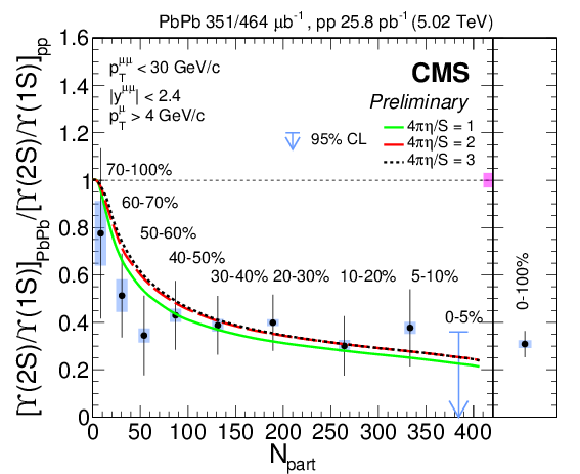
png pdf

Additional Figure 1:
Double ratio of ${\Upsilon (\textrm {2S})}$ as a function of centrality. The centrality-integrated value is shown in the right sub-panel. The error bars represent the statistical uncertainty from the PbPb data, while the boxes represent the systematic uncertainty. The box drawn around the line at unity depicts the systematic and statistical uncertainties from pp as well as the systematic uncertainties due to the combined detection efficiency, which are applicable to all points. Theory curves are also shown, for different shear viscosity over entropy ratios [1].

References
1	T. Matsui and H. Satz	$ \mathrm{J}/\psi $ Suppression by Quark-Gluon Plasma Formation	PLB 178 (1986) 416
2	N. Brambilla et al.	Heavy quarkonium: progress, puzzles, and opportunities	EPJC 71 (2011) 1534	1010.5827
3	A. Andronic et al.	Heavy-flavour and quarkonium production in the LHC era: from proton–proton to heavy-ion collisions	EPJC 76 (2016) 107	1506.03981
4	M. Cheng et al.	Equation of State for physical quark masses	PRD 81 (2010) 054504	0911.2215
5	Wuppertal-Budapest Collaboration	Is there still any $ T_c $ mystery in lattice QCD? Results with physical masses in the continuum limit III	JHEP 09 (2010) 073	1005.3508
6	S. Digal, P. Petreczky, and H. Satz	Quarkonium feed down and sequential suppression	PRD 64 (2001) 094015	hep-ph/0106017
7	A. Mocsy and P. Petreczky	Color screening melts quarkonium	PRL 99 (2007) 211602	0706.2183
8	M. Laine, O. Philipsen, P. Romatschke, and M. Tassler	Real-time static potential in hot QCD	JHEP 03 (2007) 054	hep-ph/0611300
9	A. Dumitru, Y. Guo, and M. Strickland	The Imaginary part of the static gluon propagator in an anisotropic (viscous) QCD plasma	PRD 79 (2009) 114003	0903.4703
10	A. Rothkopf, T. Hatsuda, and S. Sasaki	Complex Heavy-Quark Potential at Finite Temperature from Lattice QCD	PRL 108 (2012) 162001	1108.1579
11	Y. Burnier, O. Kaczmarek, and A. Rothkopf	Quarkonium at finite temperature: Towards realistic phenomenology from first principles	JHEP 12 (2015) 101	1509.07366
12	R. L. Thews, M. Schroedter, and J. Rafelski	Enhanced $ \mathrm{J}/\psi $ production in deconfined quark matter	PRC 63 (2001) 054905	hep-ph/0007323
13	M. I. Gorenstein, A. P. Kostyuk, H. Stoecker, and W. Greiner	Statistical coalescence model with exact charm conservation	PLB 509 (2001) 277	hep-ph/0010148
14	A. Andronic, P. Braun-Munzinger, K. Redlich, and J. Stachel	Evidence for charmonium generation at the phase boundary in ultra-relativistic nuclear collisions	PLB 652 (2007) 259	nucl-th/0701079
15	ALICE Collaboration	Centrality, rapidity and transverse momentum dependence of $ \mathrm{J}/\psi $ suppression in Pb-Pb collisions at $ \sqrt{s_{\rm NN}} $ = 2.76 TeV	PLB 734 (2014) 314	1311.0214
16	CMS Collaboration	Observation of sequential Upsilon suppression in PbPb collisions	PRL 109 (2012) 222301	CMS-HIN-11-011 1208.2826
17	B. Krouppa and M. Strickland	Predictions for bottomonia suppression in 5.023 TeV Pb-Pb collisions		1605.03561
18	CMS Collaboration	The CMS experiment at the CERN LHC	JINST 3 (2008) S08004	CMS-00-001
19	CMS Collaboration	Performance of CMS muon reconstruction in $ pp $ collision events at $ \sqrt{s}=7 $ TeV	JINST 7 (2012) P10002	CMS-MUO-10-004 1206.4071
20	CMS Collaboration	Dependence on pseudorapidity and centrality of charged hadron production in PbPb collisions at a nucleon-nucleon centre-of-mass energy of 2.76 TeV	JHEP 08 (2011) 141	CMS-HIN-10-001 1107.4800
21	C. Loizides, J. Nagle, and P. Steinberg	Improved version of the PHOBOS Glauber Monte Carlo	SoftwareX 1-2 (2015) 13	1408.2549
22	M. J. Oreglia	A study of the reactions $\psi' \to \gamma\gamma \psi$	PhD thesis, Stanford University, 1980 SLAC Report SLAC-R-236, see A
23	Particle Data Group Collaboration	Review of Particle Physics	CPC 38 (2014) 090001
24	H. Akaike	A new look at the statistical model identification	IEEE Trans. Autom. Control 19 (1974) 716
25	K. Burnham and D. Anderson	Multimodel inference: understanding AIC and BIC in model selection	Sociol. Methods Res. 33 (2004) 261
26	T. Sjostrand, S. Mrenna, and P. Skands	PYTHIA 6.4 physics and manual	JHEP 05 (2006) 026	hep-ph/0603175
27	CMS Collaboration	Indications of suppression of excited $ \varUpsilon $ states in PbPb collisions at $ \sqrt{S_{NN}} $ = 2.76 TeV	PRL 107 (2011) 052302	CMS-HIN-11-007 1105.4894
28	CMS Collaboration	Event activity dependence of Y(nS) production in $ \sqrt{s_{NN}} $=5.02 TeV pPb and $ \sqrt{s} $=2.76 TeV pp collisions	JHEP 04 (2014) 103	CMS-HIN-13-003 1312.6300
29	CMS Collaboration	Measurement of Prompt $ \psi(2S) \to \mathrm{J}/\psi $ Yield Ratios in PbPb and pp Collisions at $ \sqrt {s_{NN}} = $ 2.76 TeV	PRL 113 (2014) 262301	CMS-HIN-12-007 1410.1804
30	G. J. Feldman and R. D. Cousins	A Unified approach to the classical statistical analysis of small signals	PRD 57 (1998) 3873	physics/9711021