CMS-FSQ-15-009

CMS-FSQ-15-009 ; CERN-EP-2019-151
Bose-Einstein correlations of charged hadrons in proton-proton collisions at $\sqrt{s} = $ 13 TeV
CMS Collaboration
20 October 2019
JHEP 03 (2020) 014
Abstract: Bose-Einstein correlations of charged hadrons are measured over a broad multiplicity range, from a few particles up to about 250 reconstructed charged hadrons in proton-proton collisions at $\sqrt{s} = $ 13 TeV. The results are based on data collected using the CMS detector at the LHC during runs with a special low-pileup configuration. Three analysis techniques with different degrees of dependence on simulations are used to remove the non-Bose-Einstein background from the correlation functions. All three methods give consistent results. The measured lengths of homogeneity are studied as functions of particle multiplicity as well as average pair transverse momentum and mass. The results are compared with data from both CMS and ATLAS at $\sqrt{s} = $ 7 TeV, as well as with theoretical predictions.
Links: e-print arXiv:1910.08815 [hep-ex] (PDF) ; CDS record ; inSPIRE record ; HepData record ; CADI line (restricted) ;

Figures & Tables	Summary	Additional Figures	References	CMS Publications

Figures
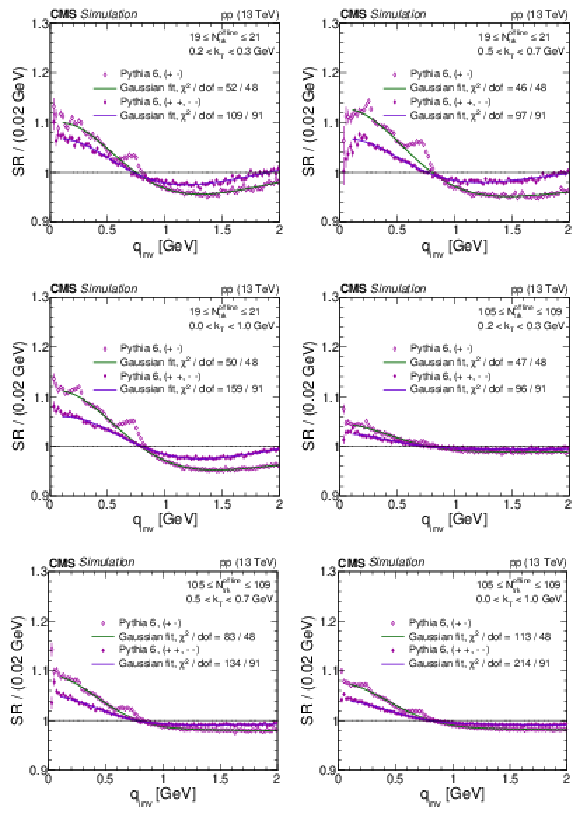
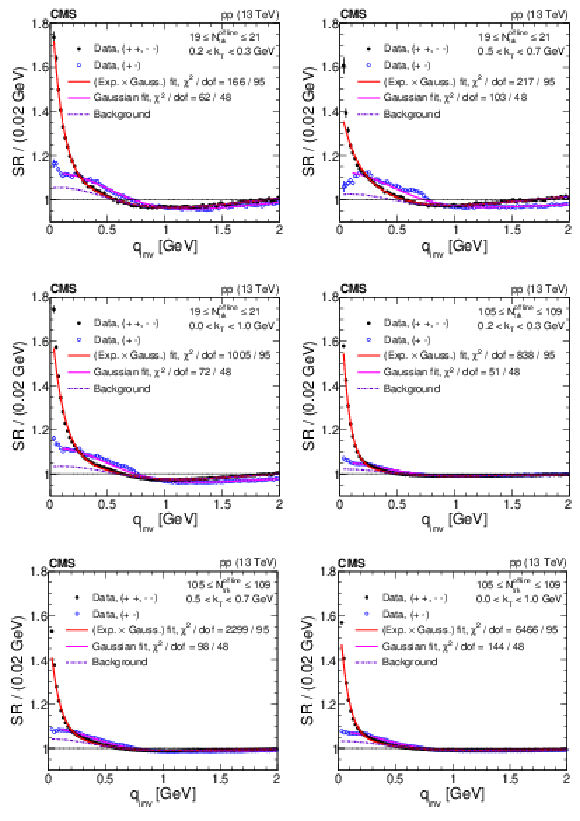
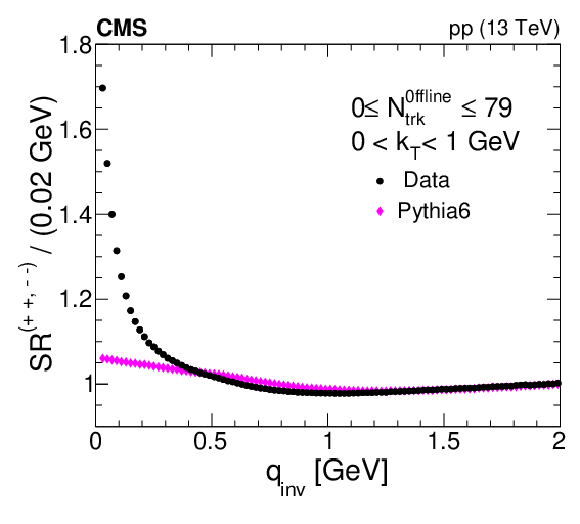
png pdf	Figure 1: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios employing PYTHIA 6 (Z2* tune) in different bins of $N^\text {offline}_\text {trk}$ and $ {k_{\mathrm {T}}} $ with the respective Gaussian fit from Eq. (4). The following $ {q_\text {inv}} $ ranges are excluded from the fits: 0.2 $ < {q_\text {inv}} < $ 0.3, 0.4 $ < {q_\text {inv}} < $ 0.9, and 0.95 $ < {q_\text {inv}} < $ 1.2 GeV. Coulomb interactions are not included in the simulation. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Figure 1-a: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios employing PYTHIA 6 (Z2* tune) in bin 19 $ \leq N^\text {offline}_\text {trk} \leq $ 21 and 0.2 $ < {k_{\mathrm {T}}} \leq $ 0.3 GeV with the Gaussian fit from Eq. (4). The following $ {q_\text {inv}} $ ranges are excluded from the fits: 0.2 $ < {q_\text {inv}} < $ 0.3, 0.4 $ < {q_\text {inv}} < $ 0.9, and 0.95 $ < {q_\text {inv}} < $ 1.2 GeV. Coulomb interactions are not included in the simulation. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Figure 1-b: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios employing PYTHIA 6 (Z2* tune) in bin 19 $ \leq N^\text {offline}_\text {trk} \leq $ 21 and 0.5 $ < {k_{\mathrm {T}}} \leq $ 0.7 GeV with the Gaussian fit from Eq. (4). The following $ {q_\text {inv}} $ ranges are excluded from the fits: 0.2 $ < {q_\text {inv}} < $ 0.3, 0.4 $ < {q_\text {inv}} < $ 0.9, and 0.95 $ < {q_\text {inv}} < $ 1.2 GeV. Coulomb interactions are not included in the simulation. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Figure 1-c: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios employing PYTHIA 6 (Z2* tune) in bin 19 $ \leq N^\text {offline}_\text {trk} \leq $ 21 and 0 $ < {k_{\mathrm {T}}} \leq $ 1 GeV with the Gaussian fit from Eq. (4). The following $ {q_\text {inv}} $ ranges are excluded from the fits: 0.2 $ < {q_\text {inv}} < $ 0.3, 0.4 $ < {q_\text {inv}} < $ 0.9, and 0.95 $ < {q_\text {inv}} < $ 1.2 GeV. Coulomb interactions are not included in the simulation. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
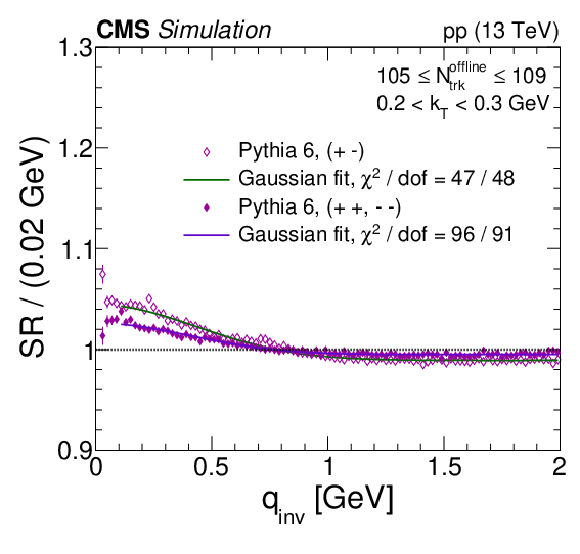
png pdf	Figure 1-d: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios employing PYTHIA 6 (Z2* tune) in bin 105 $ \leq N^\text {offline}_\text {trk} \leq $ 109 and 0.2 $ < {k_{\mathrm {T}}} \leq $ 0.3 GeV with the Gaussian fit from Eq. (4). The following $ {q_\text {inv}} $ ranges are excluded from the fits: 0.2 $ < {q_\text {inv}} < $ 0.3, 0.4 $ < {q_\text {inv}} < $ 0.9, and 0.95 $ < {q_\text {inv}} < $ 1.2 GeV. Coulomb interactions are not included in the simulation. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
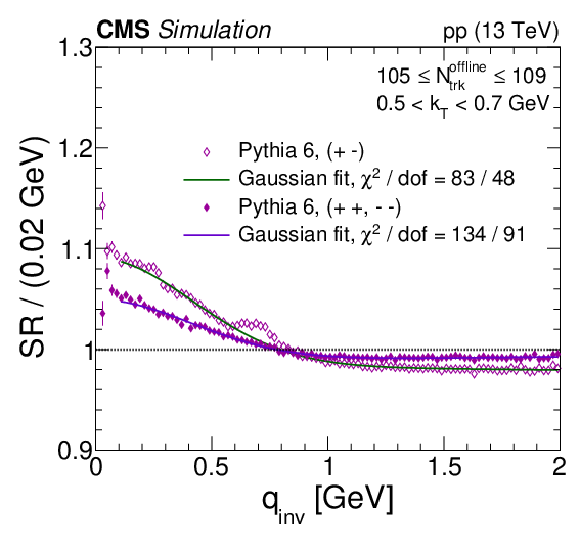
png pdf	Figure 1-e: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios employing PYTHIA 6 (Z2* tune) in bin 105 $ \leq N^\text {offline}_\text {trk} \leq $ 109 and 0.5 $ < {k_{\mathrm {T}}} \leq $ 0.7 GeV with the Gaussian fit from Eq. (4). The following $ {q_\text {inv}} $ ranges are excluded from the fits: 0.2 $ < {q_\text {inv}} < $ 0.3, 0.4 $ < {q_\text {inv}} < $ 0.9, and 0.95 $ < {q_\text {inv}} < $ 1.2 GeV. Coulomb interactions are not included in the simulation. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
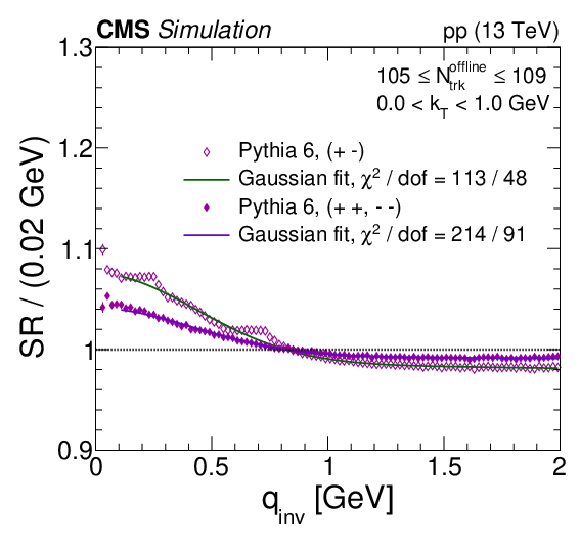
png pdf	Figure 1-f: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios employing PYTHIA 6 (Z2* tune) in bin 105 $ \leq N^\text {offline}_\text {trk} \leq $ 109 and 0 $ < {k_{\mathrm {T}}} \leq $ 1 GeV with the Gaussian fit from Eq. (4). The following $ {q_\text {inv}} $ ranges are excluded from the fits: 0.2 $ < {q_\text {inv}} < $ 0.3, 0.4 $ < {q_\text {inv}} < $ 0.9, and 0.95 $ < {q_\text {inv}} < $ 1.2 GeV. Coulomb interactions are not included in the simulation. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
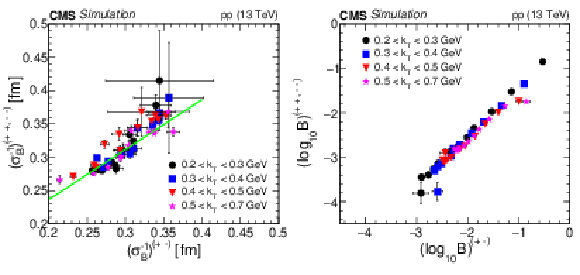
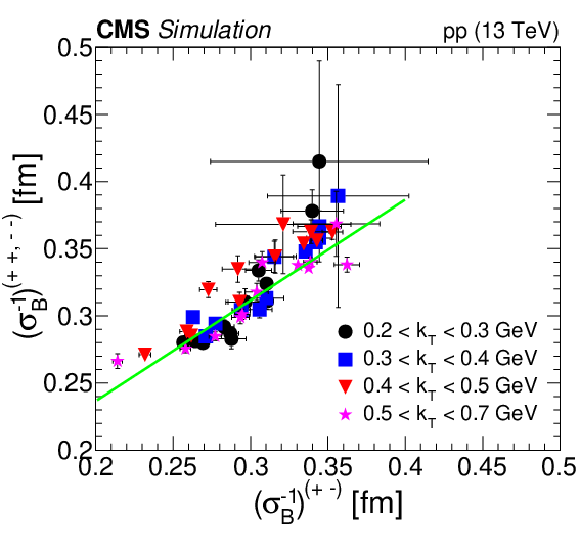
png pdf	Figure 2: Relations between same-sign ($++,-$) and opposite-sign ($+-$) fit parameters from Eq. (4), as a function of $ {k_{\mathrm {T}}} $ and $N^\text {offline}_\text {trk}$ for events in MB (i.e., higher $\sigma _{\text {B}}^{-1}$ and lower $\text {log}_{10}\text {B}$) and HM (i.e., lower $\sigma _{\text {B}}^{-1}$ and higher $\text {log}_{10}\text {B}$) ranges. The fit values found for the parameters corresponding to the peak's width (left) and the amplitude (right) of the same-sign and opposite-sign correlations are shown. For a given $ {k_{\mathrm {T}}} $ range, each point represents an $N^\text {offline}_\text {trk}$ bin. The line in the left plot is a linear fit to all the data. The error bars represent statistical uncertainties.
png pdf	Figure 2-a: Relations between same-sign ($++,-$) and opposite-sign ($+-$) fit parameters from Eq. (4), as a function of $ {k_{\mathrm {T}}} $ and $N^\text {offline}_\text {trk}$ for events in MB (i.e., higher $\sigma _{\text {B}}^{-1}$ and lower $\text {log}_{10}\text {B}$) and HM (i.e., lower $\sigma _{\text {B}}^{-1}$ and higher $\text {log}_{10}\text {B}$) ranges. The fit values found for the parameters corresponding to the peak's width of the same-sign and opposite-sign correlations are shown. For a given $ {k_{\mathrm {T}}} $ range, each point represents an $N^\text {offline}_\text {trk}$ bin. The line is a linear fit to all the data. The error bars represent statistical uncertainties.
png pdf	Figure 2-b: Relations between same-sign ($++,-$) and opposite-sign ($+-$) fit parameters from Eq. (4), as a function of $ {k_{\mathrm {T}}} $ and $N^\text {offline}_\text {trk}$ for events in MB (i.e., higher $\sigma _{\text {B}}^{-1}$ and lower $\text {log}_{10}\text {B}$) and HM (i.e., lower $\sigma _{\text {B}}^{-1}$ and higher $\text {log}_{10}\text {B}$) ranges. The fit values found for the parameters corresponding to the peak's amplitude of the same-sign and opposite-sign correlations are shown. For a given $ {k_{\mathrm {T}}} $ range, each point represents an $N^\text {offline}_\text {trk}$ bin. The error bars represent statistical uncertainties.
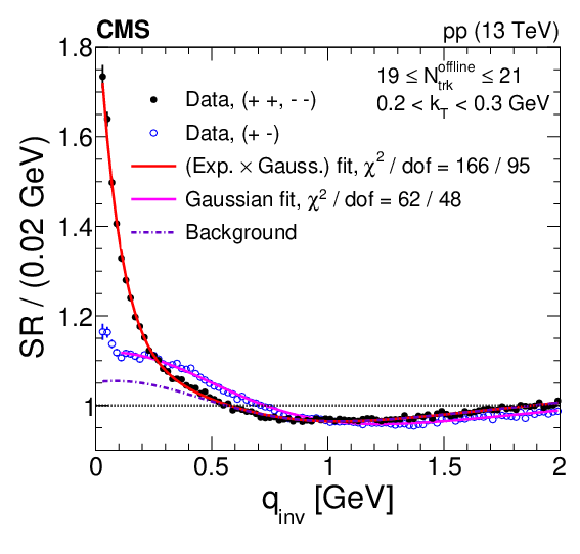
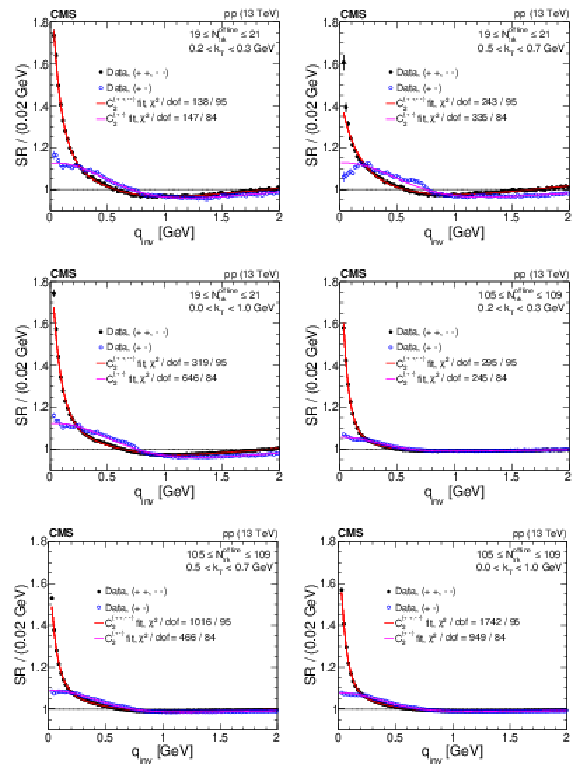
png pdf	Figure 3: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios in data for different bins of $N^\text {offline}_\text {trk}$ and $ {k_{\mathrm {T}}} $, with their respective fits. The label "(Exp. $\times $ Gauss.) fit'' refers to the same-sign data and is given by Eq. (7). The label "Gaussian fit'' corresponds to Eq. (4) applied to opposite-sign data and "Background'' is the component of Eq. (7) that is found from the Gaussian fit using Eqs. (5) and (6) to convert the fit parameters. Coulomb corrections are accounted for using the Gamow factor. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
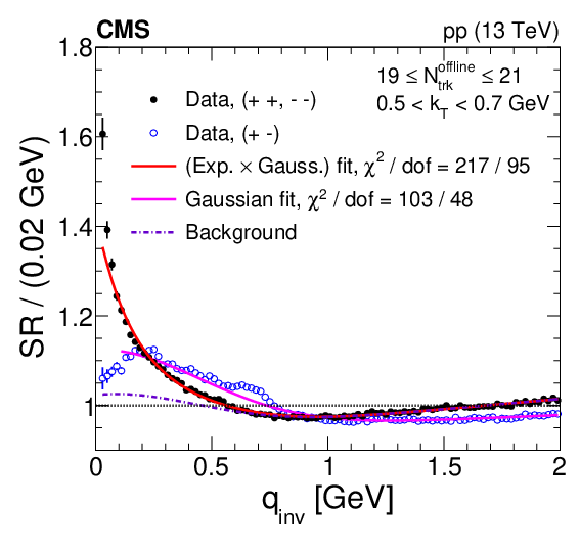
png pdf	Figure 3-a: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios in data for bin 19 $ \leq N^\text {offline}_\text {trk} \leq $ 21 and 0.2 $ < {k_{\mathrm {T}}} \leq $ 0.3 GeV, with fit. The label "(Exp. $\times $ Gauss.) fit'' refers to the same-sign data and is given by Eq. (7). The label "Gaussian fit'' corresponds to Eq. (4) applied to opposite-sign data and "Background'' is the component of Eq. (7) that is found from the Gaussian fit using Eqs. (5) and (6) to convert the fit parameters. Coulomb corrections are accounted for using the Gamow factor. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Figure 3-b: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios in data for bin 19 $ \leq N^\text {offline}_\text {trk} \leq $ 21 and 0.5 $ < {k_{\mathrm {T}}} \leq $ 0.7 GeV, with fit. The label "(Exp. $\times $ Gauss.) fit'' refers to the same-sign data and is given by Eq. (7). The label "Gaussian fit'' corresponds to Eq. (4) applied to opposite-sign data and "Background'' is the component of Eq. (7) that is found from the Gaussian fit using Eqs. (5) and (6) to convert the fit parameters. Coulomb corrections are accounted for using the Gamow factor. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Figure 3-c: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios in data for bin 19 $ \leq N^\text {offline}_\text {trk} \leq $ 21 and 0 $ < {k_{\mathrm {T}}} \leq $ 1 GeV, with fit. The label "(Exp. $\times $ Gauss.) fit'' refers to the same-sign data and is given by Eq. (7). The label "Gaussian fit'' corresponds to Eq. (4) applied to opposite-sign data and "Background'' is the component of Eq. (7) that is found from the Gaussian fit using Eqs. (5) and (6) to convert the fit parameters. Coulomb corrections are accounted for using the Gamow factor. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
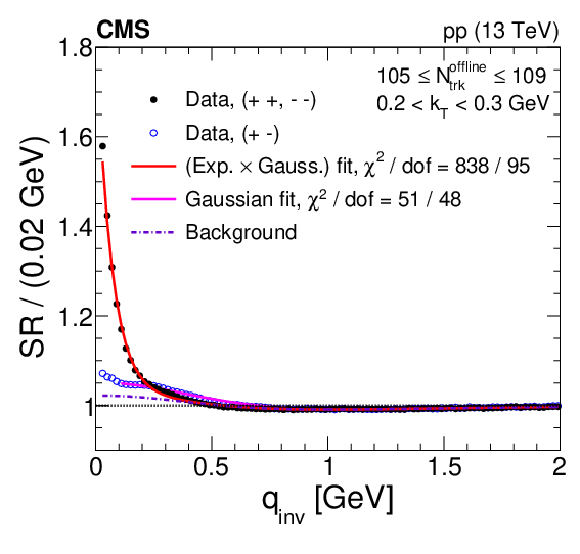
png pdf	Figure 3-d: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios in data for bin 105 $ \leq N^\text {offline}_\text {trk} \leq $ 109 and 0.2 $ < {k_{\mathrm {T}}} \leq $ 0.3 GeV, with fit. The label "(Exp. $\times $ Gauss.) fit'' refers to the same-sign data and is given by Eq. (7). The label "Gaussian fit'' corresponds to Eq. (4) applied to opposite-sign data and "Background'' is the component of Eq. (7) that is found from the Gaussian fit using Eqs. (5) and (6) to convert the fit parameters. Coulomb corrections are accounted for using the Gamow factor. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
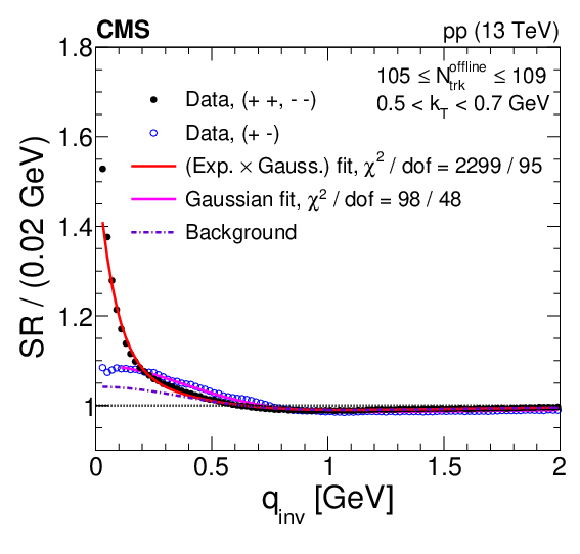
png pdf	Figure 3-e: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios in data for bin 105 $ \leq N^\text {offline}_\text {trk} \leq $ 109 and 0.5 $ < {k_{\mathrm {T}}} \leq $ 0.7 GeV, with fit. The label "(Exp. $\times $ Gauss.) fit'' refers to the same-sign data and is given by Eq. (7). The label "Gaussian fit'' corresponds to Eq. (4) applied to opposite-sign data and "Background'' is the component of Eq. (7) that is found from the Gaussian fit using Eqs. (5) and (6) to convert the fit parameters. Coulomb corrections are accounted for using the Gamow factor. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Figure 3-f: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios in data for bin 105 $ \leq N^\text {offline}_\text {trk} \leq $ 109 and 0 $ < {k_{\mathrm {T}}} \leq $ 1 GeV, with fit. The label "(Exp. $\times $ Gauss.) fit'' refers to the same-sign data and is given by Eq. (7). The label "Gaussian fit'' corresponds to Eq. (4) applied to opposite-sign data and "Background'' is the component of Eq. (7) that is found from the Gaussian fit using Eqs. (5) and (6) to convert the fit parameters. Coulomb corrections are accounted for using the Gamow factor. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Figure 4: Results for $ {R_\text {inv}} $ (left) and $\lambda $ (right) from the three methods as a function of multiplicity (upper) and $ {k_{\mathrm {T}}} $ (lower). In the upper plots, statistical and systematic uncertainties are represented by internal and external error bars, respectively. In the lower plots, statistical and systematic uncertainties are shown as error bars and open boxes, respectively.
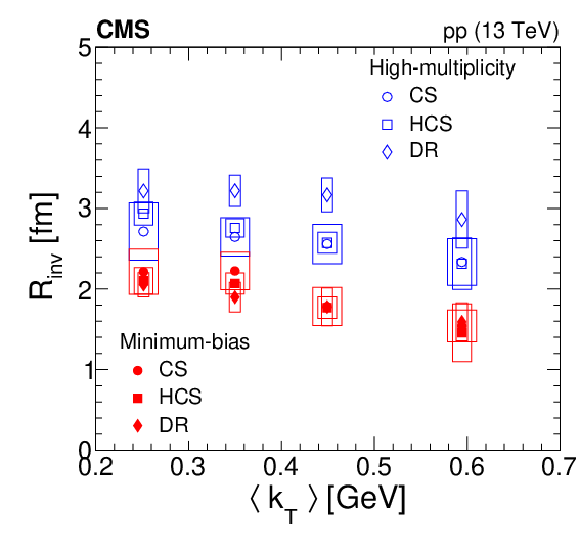
png pdf	Figure 4-a: Result for $ {R_\text {inv}} $ from the three methods as a function of multiplicity. Statistical and systematic uncertainties are represented by internal and external error bars, respectively.
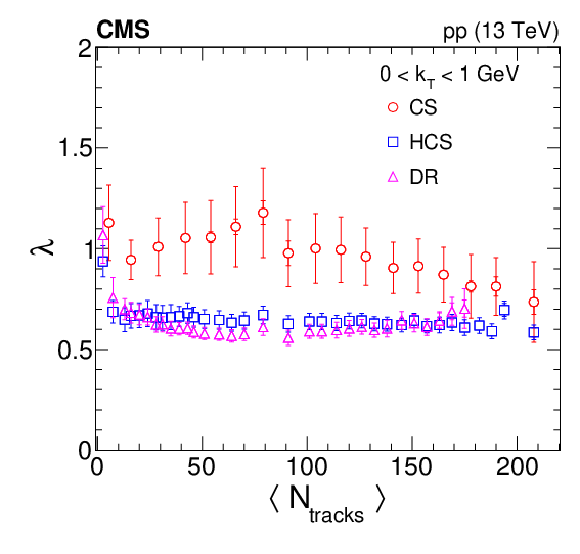
png pdf	Figure 4-b: Result for $\lambda $ from the three methods as a function of $ {k_{\mathrm {T}}} $. Statistical and systematic uncertainties are represented by internal and external error bars, respectively.
png pdf	Figure 4-c: Result for $ {R_\text {inv}} $ from the three methods as a function of multiplicity. Statistical and systematic uncertainties are shown as error bars and open boxes, respectively.
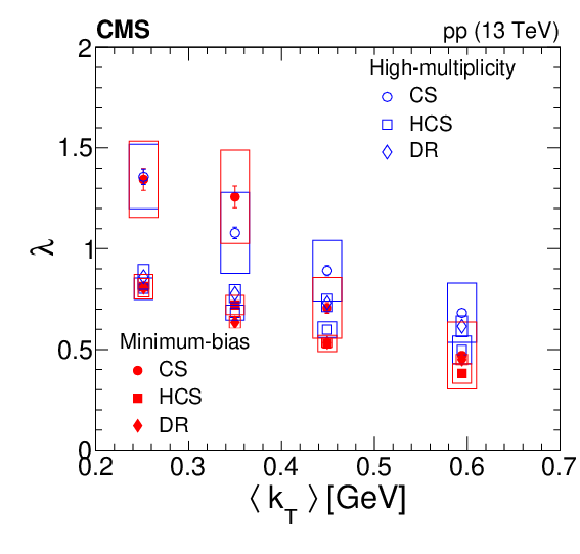
png pdf	Figure 4-d: Result for $\lambda $ from the three methods as a function of $ {k_{\mathrm {T}}} $. Statistical and systematic uncertainties are shown as error bars and open boxes, respectively.
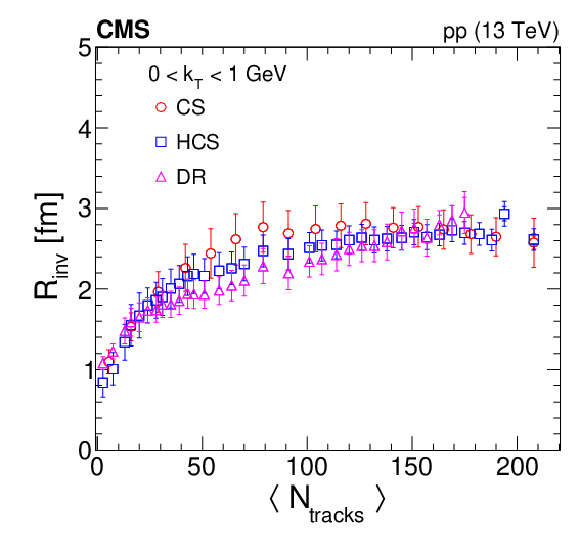
png pdf	Figure 5: The $ {R_\text {inv}} $ fit parameters as a function of particle-level multiplicities using the HCS method in pp collisions at 13 TeV compared to results for pp collisions at 7 TeV from CMS (left) and ATLAS (right). Both the ordinate and abscissa for the CMS data in the right plot have been adjusted for compatibility with the ATLAS analysis procedure, as explained in the text. The error bars in the CMS [5] case represent systematic uncertainties (statistical uncertainties are smaller than the marker size) and in the ATLAS [15] case, statistical and systematic uncertainties added in quadrature.
png pdf	Figure 5-a: The $ {R_\text {inv}} $ fit parameters as a function of particle-level multiplicities using the HCS method in pp collisions at 13 TeV compared to results for pp collisions at 7 TeV from CMS. The error bars represent systematic uncertainties (statistical uncertainties are smaller than the marker size).
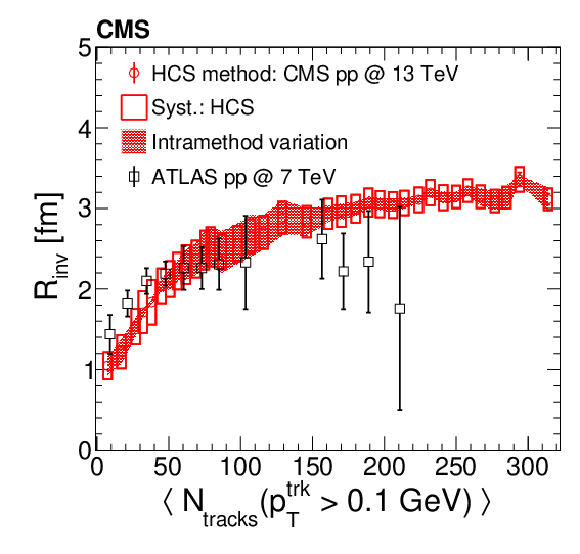
png pdf	Figure 5-b: The $ {R_\text {inv}} $ fit parameters as a function of particle-level multiplicities using the HCS method in pp collisions at 13 TeV compared to results for pp collisions at 7 TeV from ATLAS. Both the ordinate and abscissa for the CMS data have been adjusted for compatibility with the ATLAS analysis procedure, as explained in the text. In the ATLAS [15] case, statistical and systematic uncertainties added in quadrature.
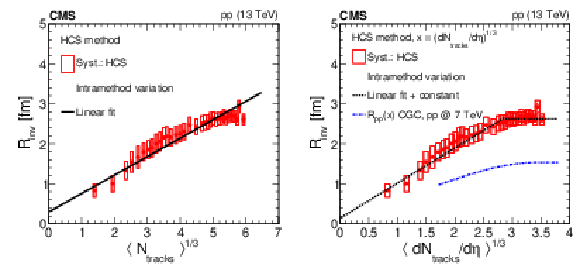
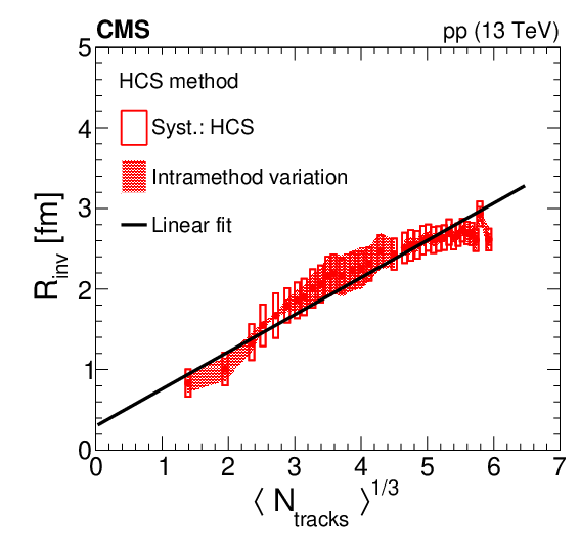
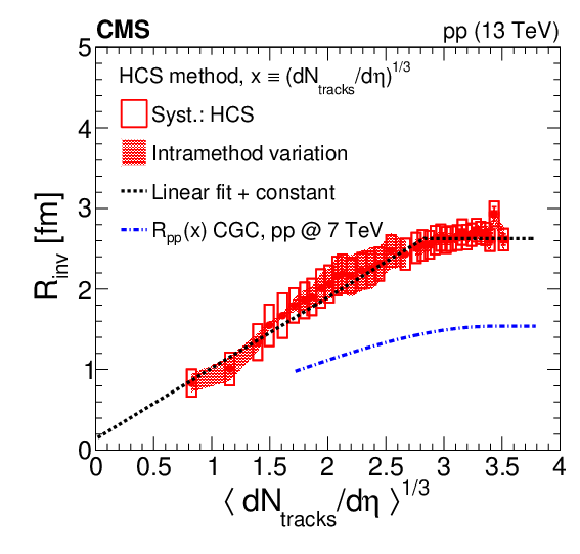
png pdf	Figure 6: Comparison of $ {R_\text {inv}} $ obtained with the HCS method with theoretical expectations. Values of $ {R_\text {inv}} $ as a function of $< N_\text {tracks}> ^{1/3}$ (left) are shown with a linear fit to illustrate the expectation from hydrodynamics. Values of $ {R_\text {inv}} $ are compared with predictions from the CGC as a function of $< {\mathrm {d}}N_\text {tracks}/ {\mathrm {d}}\eta > ^{1/3}$ (right). The dot-dashed blue line is the result of the parameterization in Eq. (8). The linear plus constant function (dashed black lines) for $< {\mathrm {d}}N_\text {tracks}/ {\mathrm {d}}\eta > ^{1/3}$ is shown to illustrate the qualitative behavior suggested by the CGC (the matching point of the two lines is the result of a fit). Only statistical uncertainties are considered.
png pdf	Figure 6-a: Comparison of $ {R_\text {inv}} $ obtained with the HCS method with theoretical expectations. Values of $ {R_\text {inv}} $ as a function of $< N_\text {tracks}> ^{1/3}$ are shown with a linear fit to illustrate the expectation from hydrodynamics.
png pdf	Figure 6-b: Comparison of $ {R_\text {inv}} $ obtained with the HCS method with theoretical expectations. Values of $ {R_\text {inv}} $ are compared with predictions from the CGC as a function of $< {\mathrm {d}}N_\text {tracks}/ {\mathrm {d}}\eta > ^{1/3}$. The dot-dashed blue line is the result of the parameterization in Eq. (8). The linear plus constant function (dashed black lines) for $< {\mathrm {d}}N_\text {tracks}/ {\mathrm {d}}\eta > ^{1/3}$ is shown to illustrate the qualitative behavior suggested by the CGC (the matching point of the two lines is the result of a fit). Only statistical uncertainties are considered.
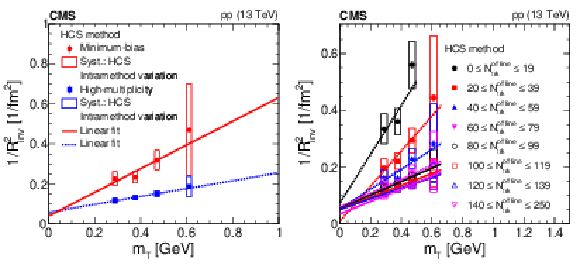
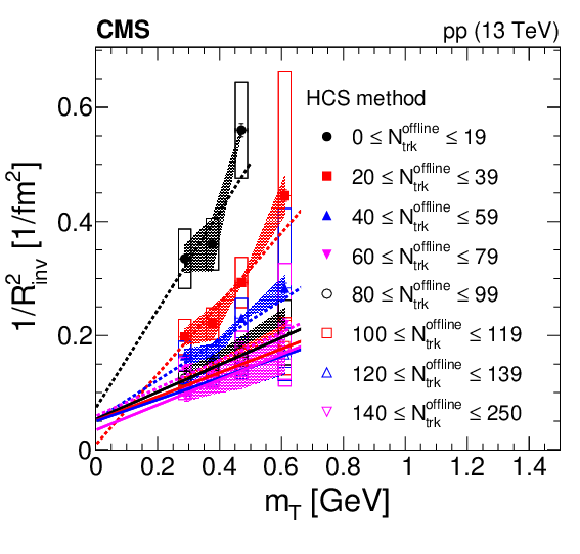
png pdf	Figure 7: The distribution $1/R_{\text {inv}}^2$ as a function of $ {m_{\mathrm {T}}} $ for the HCS method. Results corresponding to the MB range (0 $ \le N_\text {trk}^\text {offline} \le $ 79) and to the HM one (80 $ \le N_\text {trk}^\text {offline} \le $ 250) are shown (left). Results are also shown in more differential bins of multiplicity (right). Statistical uncertainties are represented by the error bars, systematic uncertainties related to the HCS method are shown as open boxes, and the relative uncertainties from the intramethods variation are represented by the shaded bands. Only statistical uncertainties are considered in all the fits.
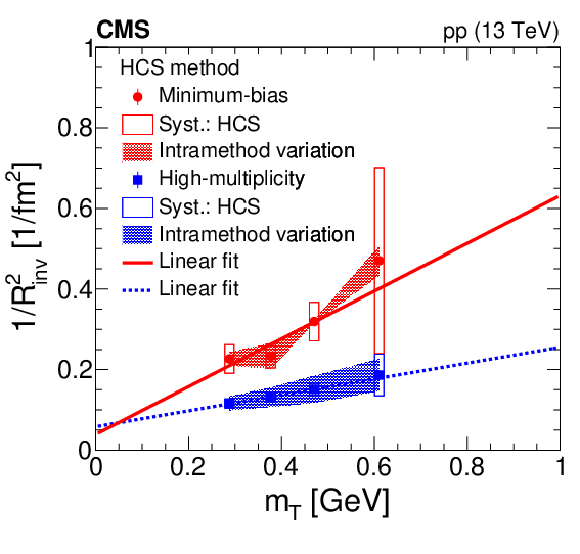
png pdf	Figure 7-a: The distribution $1/R_{\text {inv}}^2$ as a function of $ {m_{\mathrm {T}}} $ for the HCS method. Results corresponding to the MB range (0 $ \le N_\text {trk}^\text {offline} \le $ 79) and to the HM one (80 $ \le N_\text {trk}^\text {offline} \le $ 250) are shown. Statistical uncertainties are represented by the error bars, systematic uncertainties related to the HCS method are shown as open boxes, and the relative uncertainties from the intramethods variation are represented by the shaded bands. Only statistical uncertainties are considered in all the fits.
png pdf	Figure 7-b: The distribution $1/R_{\text {inv}}^2$ as a function of $ {m_{\mathrm {T}}} $ for the HCS method. Results are shown in bins of multiplicity. Statistical uncertainties are represented by the error bars, systematic uncertainties related to the HCS method are shown as open boxes, and the relative uncertainties from the intramethods variation are represented by the shaded bands. Only statistical uncertainties are considered in all the fits.
png pdf	Figure 8: Illustration of the steps in the double ratio method. The single ratio in data is constructed (left), followed by a similar procedure with simulated events (PYTHIA 6, Z2* tune). The ratio of the two curves on the left defines the double ratio (right). The reference sample is obtained with the $\eta $-mixing procedure. All results correspond to integrated values in $N^\text {offline}_\text {trk}$ and $ {k_{\mathrm {T}}} $.
png pdf	Figure 8-a: Illustration of the steps in the double ratio method. The single ratio in data is constructed, followed by a similar procedure with simulated events (PYTHIA 6, Z2* tune). The reference sample is obtained with the $\eta $-mixing procedure. All results correspond to integrated values in $N^\text {offline}_\text {trk}$ and $ {k_{\mathrm {T}}} $.
png pdf	Figure 8-b: Illustration of the steps in the double ratio method. The ratio of the two curves in Fig. 8-a defines the double ratio. The reference sample is obtained with the $\eta $-mixing procedure. All results correspond to integrated values in $N^\text {offline}_\text {trk}$ and $ {k_{\mathrm {T}}} $.
png pdf	Figure 9: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios are shown in different $N^\text {offline}_\text {trk}$ and $ {k_{\mathrm {T}}} $ bins, together with the full fits (continuous curves) given in Eqs. (10) and (13), for minimum-bias (upper row) and HM (lower row) events. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
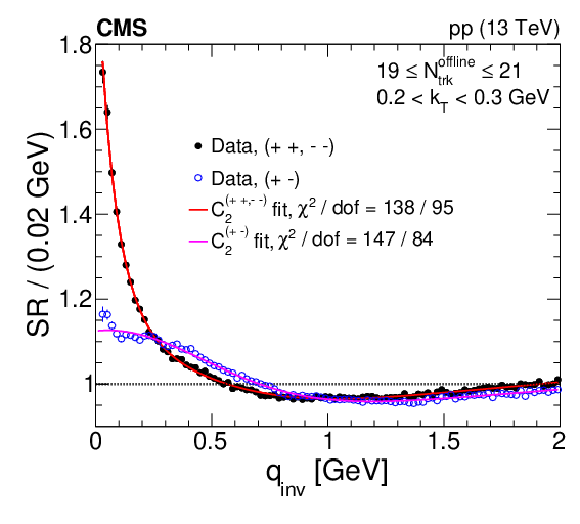
png pdf	Figure 9-a: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios are shown in bin 19 $ \leq N^\text {offline}_\text {trk} \leq $ 21 and 0.2 $ < {k_{\mathrm {T}}} \leq $ 0.3 GeV, together with the full fit (continuous curve) given in Eqs. (10) and (13), for minimum-bias events. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Figure 9-b: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios are shown in bin 19 $ \leq N^\text {offline}_\text {trk} \leq $ 21 and 0.5 $ < {k_{\mathrm {T}}} \leq $ 0.7 GeV, together with the full fit (continuous curve) given in Eqs. (10) and (13), for minimum-bias events. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
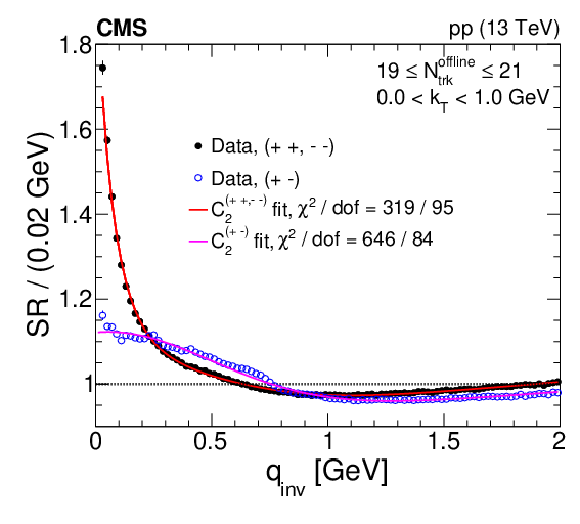
png pdf	Figure 9-c: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios are shown in bin 19 $ \leq N^\text {offline}_\text {trk} \leq $ 21 and 0 $ < {k_{\mathrm {T}}} \leq $ 1 GeV, together with the full fit (continuous curve) given in Eqs. (10) and (13), for minimum-bias events. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
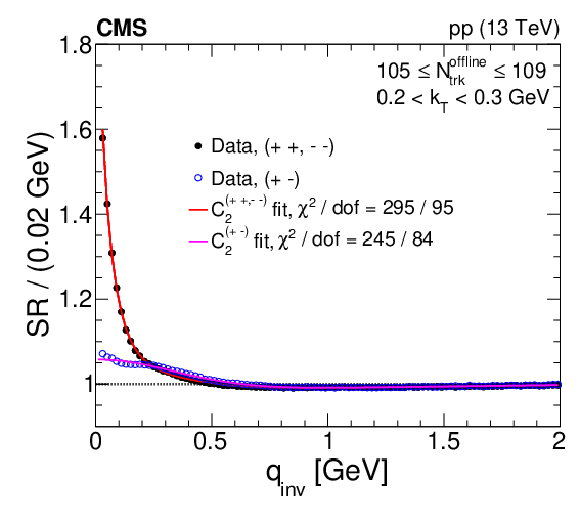
png pdf	Figure 9-d: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios are shown in bin 105 $ \leq N^\text {offline}_\text {trk} \leq $ 109 and 0.2 $ < {k_{\mathrm {T}}} \leq $ 0.3 GeV, together with the full fit (continuous curve) given in Eqs. (10) and (13), for HM events. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Figure 9-e: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios are shown in bin 105 $ \leq N^\text {offline}_\text {trk} \leq $ 109 and 0.5 $ < {k_{\mathrm {T}}} \leq $ 0.7 GeV, together with the full fit (continuous curve) given in Eqs. (10) and (13), for HM events. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
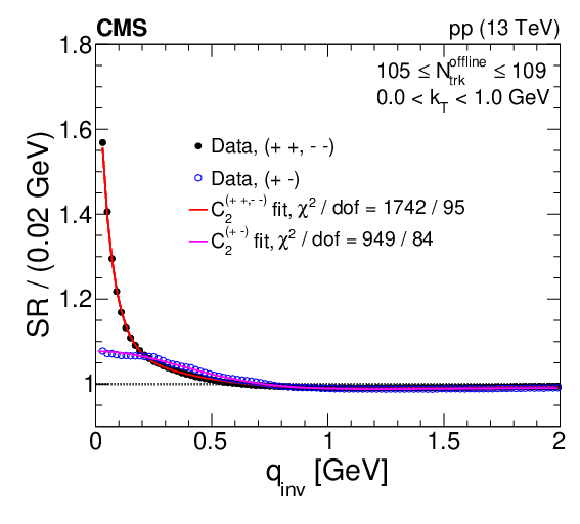
png pdf	Figure 9-f: The same-sign ($++,-$) and opposite-sign ($+-$) single ratios are shown in bin 105 $ \leq N^\text {offline}_\text {trk} \leq $ 109 and 0 $ < {k_{\mathrm {T}}} \leq $ 1 GeV, together with the full fit (continuous curve) given in Eqs. (10) and (13), for HM events. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
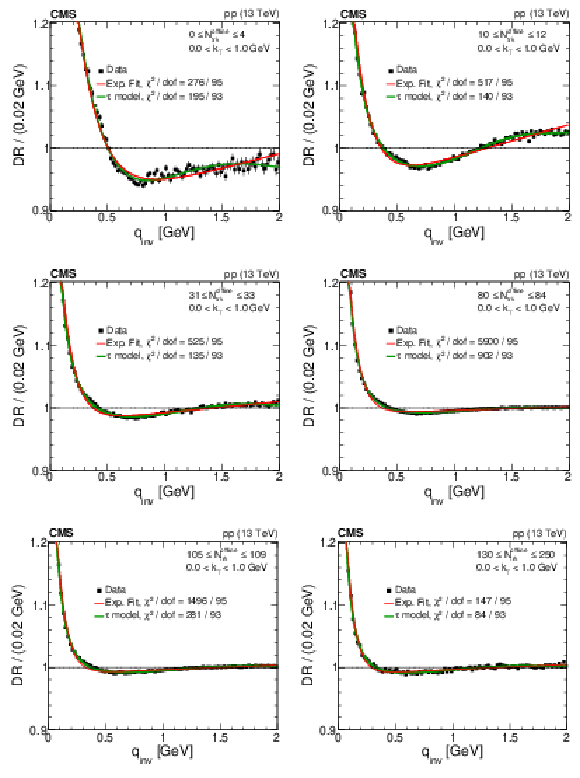
png pdf	Figure 10: Correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in six multiplicity bins. The results are zoomed along the vertical axis. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Figure 10-a: Correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bin 0 $ \leq N^\text {offline}_\text {trk} \leq $ 4. The results are zoomed along the vertical axis. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Figure 10-b: Correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bin 10 $ \leq N^\text {offline}_\text {trk} \leq $ 12. The results are zoomed along the vertical axis. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
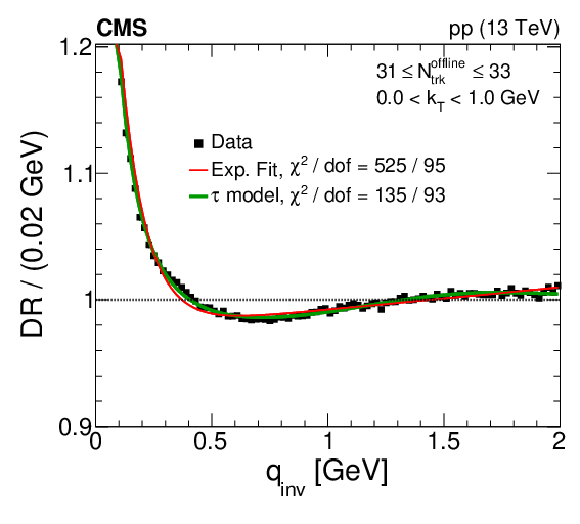
png pdf	Figure 10-c: Correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bin 31 $ \leq N^\text {offline}_\text {trk} \leq $ 33. The results are zoomed along the vertical axis. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
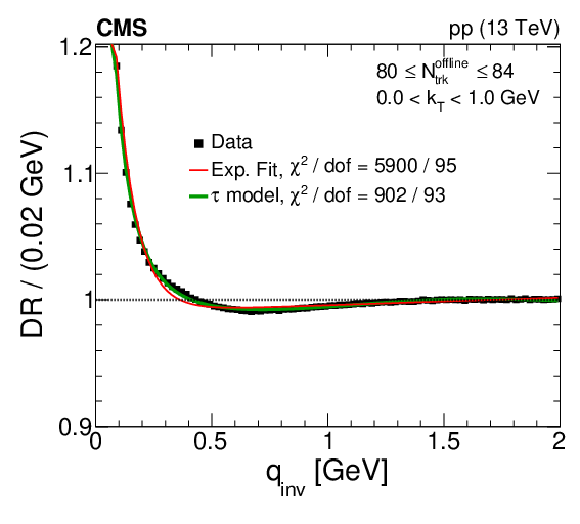
png pdf	Figure 10-d: Correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bin 80 $ \leq N^\text {offline}_\text {trk} \leq $ 84. The results are zoomed along the vertical axis. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
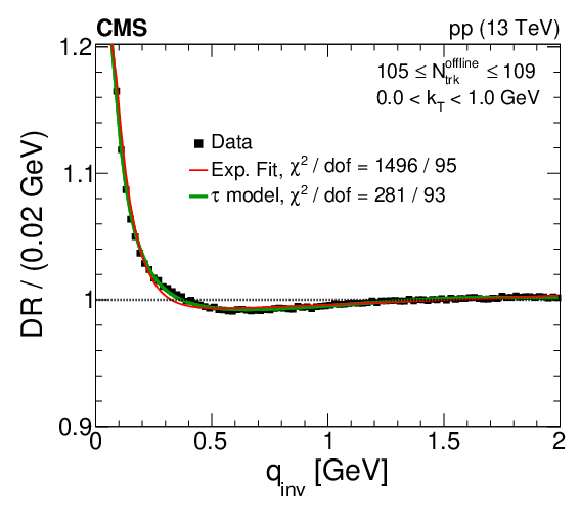
png pdf	Figure 10-e: Correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bin 105 $ \leq N^\text {offline}_\text {trk} \leq $ 109. The results are zoomed along the vertical axis. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
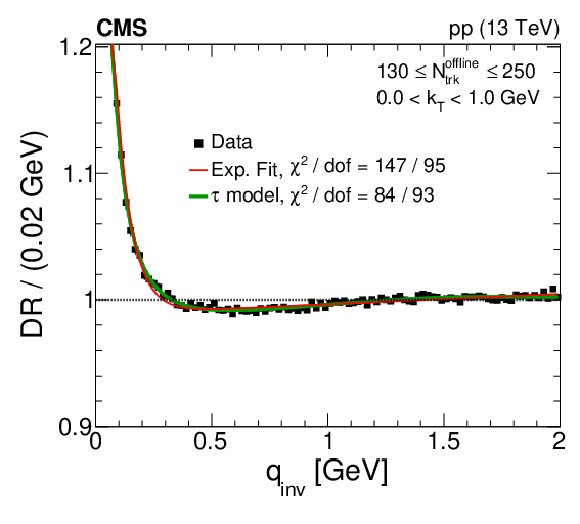
png pdf	Figure 10-f: Correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bin 130 $ \leq N^\text {offline}_\text {trk} \leq $ 250. The results are zoomed along the vertical axis. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
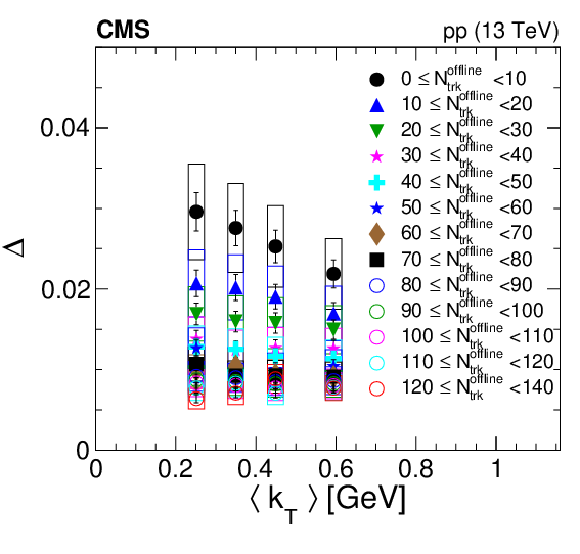
png pdf	Figure 11: The depth of the anticorrelation $\Delta $ is shown as a function of multiplicity (left) for $ {k_{\mathrm {T}}} $-integrated values. The fit parameter $\Delta $ is also shown in finer bins of $N_\text {tracks}$ and $ {k_{\mathrm {T}}} $ (right). The statistical uncertainties are represented by the error bars, while the systematic ones are represented by the open boxes.
png pdf	Figure 11-a: The depth of the anticorrelation $\Delta $ is shown as a function of multiplicity for $ {k_{\mathrm {T}}} $-integrated values. The statistical uncertainties are represented by the error bars, while the systematic ones are represented by the open boxes.
png pdf	Figure 11-b: The fit parameter $\Delta $ is shown in fine bins of $N_\text {tracks}$ and $ {k_{\mathrm {T}}} $. The statistical uncertainties are represented by the error bars, while the systematic ones are represented by the open boxes.

Tables
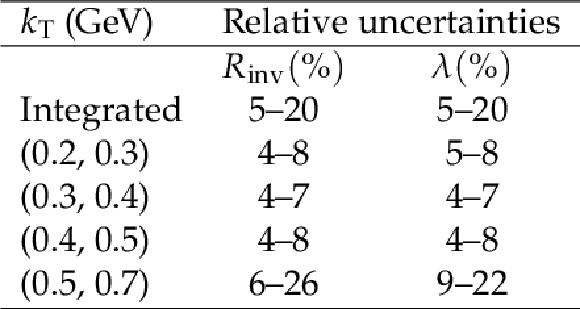
png pdf	Table 1: Total systematic uncertainties in different $ {k_{\mathrm {T}}} $ bins for the hybrid cluster subtraction technique. The ranges in the uncertainties indicate the minimum and maximum values found for all multiplicity bins.
png pdf	Table 2: Values of the fit parameters from Eqs. (11) and (12), describing the cluster contribution in the data OS correlation function. The estimated uncertainty in the parameters is about 10%.

Summary

A Bose-Einstein correlation measurement is reported using data collected with the CMS detector at the LHC in proton-proton collisions at $\sqrt{s} = $ 13 TeV, covering a broad range of charged particle multiplicity, from a few particles up to 250 reconstructed charged hadrons. Three analysis methods, each with a different dependence on Monte Carlo simulations, are used to generate correlation functions, which are found to give consistent results. One dimensional studies of the radius fit parameter, $R_{\text{inv}}$, and the intercept parameter, $\lambda$, have been carried out for both inclusive events and high multiplicity events selected using a dedicated online trigger. For multiplicities in the range 0 $ < N_{\text{trk}}^{\text{offline}} < $ 250 and average pair transverse momentum 0 $ < {k_{\mathrm{T}}} < $ 1 GeV, values of the radius fit parameter and intercept are in the ranges 0.8 $ < R_{\text{inv}} < $ 3.0 fm and 0.5 $ < \lambda < $ 1.0, respectively.

Over most of the multiplicity range studied, the value of $R_{\text{inv}}$ increases with increasing event multiplicities and is proportional to $ < N_{\text{tracks}} > ^{1/3} $, a trend which is predicted by hydrodynamical calculations. For events with more than ${\sim}$ 100 charged particles, the observed dependence of $R_{\text{inv}}$ suggests a possible saturation, with the lengths of homogeneity also consistent with a constant value. Comparisons of the multiplicity dependence are made with predictions of the color glass condensate effective theory, by means of a parameterization of the radius of the system formed in pp collisions. The values of the radius parameters in the model are much lower than those in the data, although the general shape of the dependence on multiplicity is similar in both cases. The radius fit parameter $R_{\text{inv}}$ is also observed to decrease with increasing ${k_{\mathrm{T}}}$, a behavior that is consistent with emission from a system that is expanding prior to its decoupling.

Inspired by hydrodynamic models, the dependence of $R_{\text{inv}}^{-2}$ on the average pair transverse mass was investigated and the two are observed to be proportional, a behavior similar to that seen in nucleus-nucleus collisions. The proportionality constant between $R_{\text{inv}}^{-2}$ and transverse mass can be related to the flow parameter of a Hubble-type expansion of the system. For pp collisions at 13 TeV, this expansion is slower for larger event multiplicity, a dependence that was also found in nucleus-nucleus collisions. Therefore, the present analysis reveals additional similarities between the systems produced in high multiplicity pp collisions and those found using data for larger initial systems. These results may provide additional constraints on future attempts using hydrodynamical models and/or the color glass condensate framework to explain the entire range of similarities between pp and heavy ion interactions.

Additional Figures
png pdf	Additional Figure 1: Unzoomed correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bins. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Additional Figure 1-a: Unzoomed correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bin 0 $ \leq N^\text {offline}_\text {trk} \leq $ 4. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
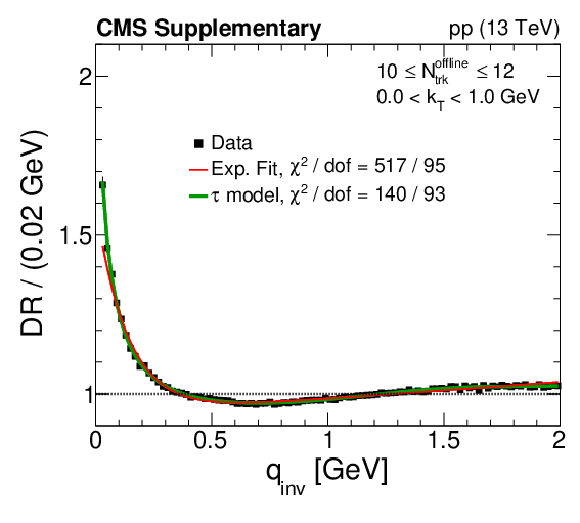
png pdf	Additional Figure 1-b: Unzoomed correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bin 10 $ \leq N^\text {offline}_\text {trk} \leq $ 12. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
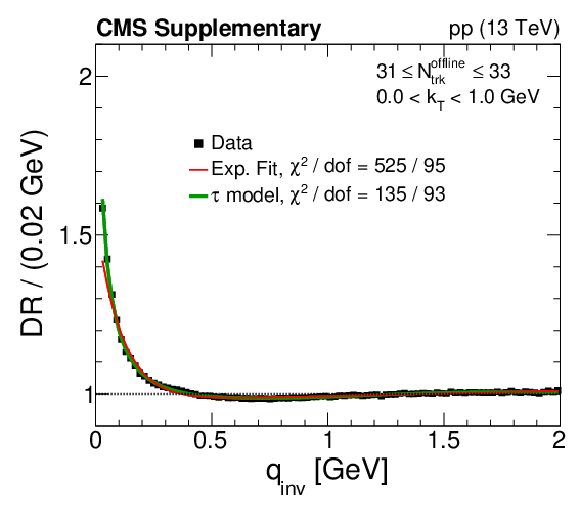
png pdf	Additional Figure 1-c: Unzoomed correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bin 31 $ \leq N^\text {offline}_\text {trk} \leq $ 33. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Additional Figure 1-d: Unzoomed correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bin 80 $ \leq N^\text {offline}_\text {trk} \leq $ 84. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
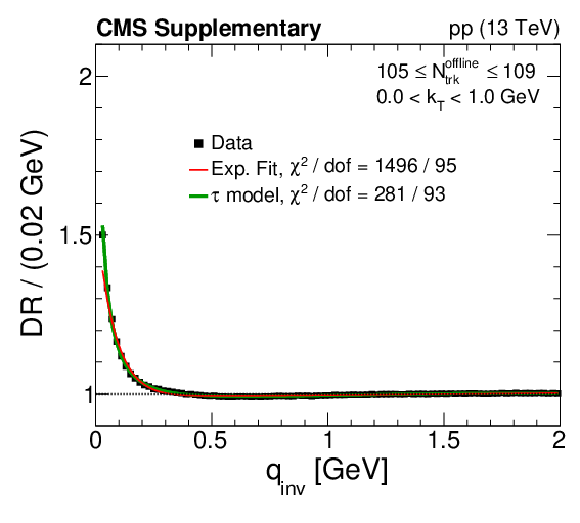
png pdf	Additional Figure 1-e: Unzoomed correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bin 105 $ \leq N^\text {offline}_\text {trk} \leq $ 109. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.
png pdf	Additional Figure 1-f: Unzoomed correlation functions from the double ratio technique, integrated in the range 0 $ < {k_{\mathrm {T}}} < $ 1 GeV, in multiplicity bin 130 $ \leq N^\text {offline}_\text {trk} \leq $ 250. The error bars represent statistical uncertainties and in most cases are smaller than the marker size.

References
1	G. Goldhaber, S. Goldhaber, W. Lee and A. Pais	Influence of Bose-Einstein statistics in the antiproton-proton annihilation process	PR120 (1960) 300
2	M. A. Lisa, S. Pratt, R. Soltz, and U. Wiedemann	Femtoscopy in relativistic heavy ion collisions: two decades of progress	Annu. Rev. Nucl. Part. Sci. 55 (2005) 357	nucl-ex/0505014
3	CMS Collaboration	First measurement of Bose-Einstein correlations in proton-proton collisions at $ \sqrt{s} = $ 0.9 and 2.36 TeV at the LHC	PRL 105 (2010) 032001	CMS-QCD-10-003 1005.3294
4	CMS Collaboration	Measurement of Bose-Einstein correlations in pp collisions at $ \sqrt{s}= $ 0.9 and 7 TeV	JHEP 05 (2011) 029	CMS-QCD-10-023 1101.3518
5	CMS Collaboration	Bose-Einstein correlations in pp, pPb, and PbPb collisions at $ \sqrt{s} = $ 0.9--7 TeV	PRC 97 (2018) 064912	CMS-FSQ-14-002 1712.07198
6	AFS Collaboration	Bose-Esinstein correlations in $ \alpha \alpha $, $\mathrm{pp}$ and $ \mathrm{p}\overline{\mathrm{p}} $ interactions	PLB 129 (1983) 269
7	AFS Collaboration	Bose-Einstein correlations between kaons	PLB 155 (1985) 128
8	AFS Collaboration	Evidence of a directional dependence of Bose-Einstein correlations at the CERN Intersecting Storage Rings	PLB 187 (1987) 420
9	UA1 Collaboration	Bose-Einstein correlations in $ \mathrm{p}\overline{\mathrm{p}} $ interactions at $ \sqrt{s} = $ 0.2 to 0.9 TeV	PLB 226 (1989) 410
10	E735 Collaboration	Study of the source size in $ \mathrm{p \bar{p}} $ collisions at 1.8 TeV using pion interferometry	PRD 48 (1993) 1931
11	PHOBOS Collaboration	Transverse momentum and rapidity dependence of HBT correlations in Au+Au collisions at $ \sqrt{s_{\text{NN}}} = $ 62.4 GeV and 200 GeV	PRC 73 (2006) 031901	nucl-ex/0409001
12	STAR Collaboration	Pion interferometry in Au+Au collisions at $ \sqrt{s_{\text{NN}}} = $ 200 GeV	PRC 71 (2005) 044906	nucl-ex/0411036
13	PHENIX Collaboration	Source breakup dynamics in Au+Au collisions at $ \sqrt{s_{\text{NN}}} = $ 200 GeV via three-dimensional two-pion source imaging	PRL 100 (2008) 232301	0712.4372
14	ALICE Collaboration	Two-pion Bose-Einstein correlations in pp collisions at $ \sqrt{s} = $ 900 GeV	PRD 82 (2010) 052001	1007.0516
15	ATLAS Collaboration	Two-particle Bose-Einstein correlations in pp collisions at $ \sqrt{s}= $ 0.9 and 7 TeV with the ATLAS detector	EPJC 75 (2015) 466	1502.07947
16	LHCb Collaboration	Bose-Einstein correlations of same-sign charged pions in the forward region in pp collisions at $ \sqrt{s} = $ 7 TeV	JHEP 12 (2017) 025	1709.01769
17	CMS Collaboration	Observation of long-range near-side angular correlations in proton-proton collisions at the LHC	JHEP 09 (2010) 091	CMS-QCD-10-002 1009.4122
18	ATLAS Collaboration	Observation of long-range elliptic azimuthal anisotropies in $ \sqrt{s}= $ 13 and 2.76 TeV pp collisions with the ATLAS detector	PRL 116 (2016) 172301	1509.04776
19	CMS Collaboration	Observation of long-range near-side angular correlations in pPb collisions at the LHC	PLB 718 (2013) 795	CMS-HIN-12-015 1210.5482
20	ALICE Collaboration	Long-range angular correlations on the near and away side in p-Pb collisions at $ \sqrt{s_{\text{NN}}}= $ 5.02 TeV	PLB 719 (2013) 29	1212.2001
21	ATLAS Collaboration	Observation of associated near-side and away-side long-range correlations in $ \sqrt{s_{\text{NN}}}= $ 5.02 TeV proton-lead collisions with the ATLAS detector	PRL 110 (2013) 182302	1212.5198
22	LHCb Collaboration	Measurements of long-range near-side angular correlations in $ \sqrt{s_{\text{\text{NN}}}}= $ 5 TeV proton-lead collisions in the forward region	PLB 762 (2016) 473	1512.00439
23	CMS Collaboration	Evidence for collectivity in pp collisions at the LHC	PLB 765 (2017) 193	CMS-HIN-16-010 1606.06198
24	CMS Collaboration	Measurement of long-range near-side two-particle angular correlations in pp collisions at $ \sqrt s = $ 13 TeV	PRL 116 (2016) 172302	CMS-FSQ-15-002 1510.03068
25	K. Dusling, W. Li, and B. Schenke	Novel collective phenomena in high-energy proton-proton and proton-nucleus collisions	Int. J. Mod. Phys. E 25 (2016) 1630002	1509.07939
26	A. N. Makhlin and Y. M. Sinyukov	The hydrodynamics of hadron matter under a pion interferometric microscope	Z. Phys. C 39 (1988) 69
27	CMS Collaboration	Description and performance of track and primary-vertex reconstruction with the CMS tracker	JINST 9 (2014) P10009	CMS-TRK-11-001 1405.6569
28	CMS Collaboration	The CMS trigger system	JINST 12 (2017) P01020	CMS-TRG-12-001 1609.02366
29	CMS Collaboration	The CMS experiment at the CERN LHC	JINST 3 (2008) S08004	CMS-00-001
30	T. Sjostrand, S. Mrenna, and P. Skands	PYTHIA 6.4 physics and manual	JHEP 05 (2006) 026	hep-ph/0603175
31	T. Sjostrand, S. Mrenna, and P. Z. Skands	A brief introduction to PYTHIA 8.1	CPC 178 (2008) 852	0710.3820
32	CMS Collaboration	Study of the underlying event at forward rapidity in pp collisions at $ \sqrt{s} = $ 0.9, 2.76, and 7 TeV	JHEP 04 (2013) 072	CMS-FWD-11-003 1302.2394
33	CMS Collaboration	Event generator tunes obtained from underlying event and multiparton scattering measurements	EPJC 76 (2016) 155	CMS-GEN-14-001 1512.00815
34	R. Corke and T. Sjöstrand	Interleaved parton showers and tuning prospects	JHEP 03 (2011) 032	1011.1759
35	I. Pierog, T. Karpenko, E. Katzy, J. M. Yatsenko, and K. Werner	EPOS LHC: Test of collective hadronization with data measured at the CERN Large Hadron Collider	PRC 92 (2015) 034906	1306.0121
36	Geant4 Collaboration	GEANT4--a simulation toolkit	NIMA 506 (2003) 250
37	CMS Collaboration	Measurement of transverse momentum relative to dijet systems in PbPb and pp collisions at $ \sqrt{s_{\mathrm{\text{NN}}}}= $ 2.76 TeV	JHEP 01 (2016) 006	CMS-HIN-14-010 1509.09029
38	CMS Collaboration	Study of the inclusive production of charged pions, kaons, and protons in pp collisions at $ \sqrt{s}= $ 0.9 , 2.76, and 7~TeV	EPJC 72 (2012) 2164	CMS-FSQ-12-014 1207.4724
39	CMS Collaboration	Measurement of charged pion, kaon, and proton production in proton-proton collisions at $ \sqrt{s}= $ 13 TeV	PRD 96 (2017) 112003	CMS-FSQ-16-004 1706.10194
40	Y. Sinyukov et al.	Coulomb corrections for interferometry analysis of expanding hadron systems	PLB 432 (1998) 248
41	M. Gyulassy, S. K. Kaufmann, and L. W. Wilson	Pion interferometry of nuclear collisions. I. Theory	PRC 20 (1979) 2267
42	S. Pratt	Coherence and Coulomb effects on pion interferometry	PRD 33 (1986) 72
43	M. Biyajima and T. Mizoguchi	Coulomb wave function correction to Bose-Einstein correlations	SULDP-1994-9
44	M. G. Bowler	Coulomb corrections to Bose-Einstein correlations have been greatly exaggerated	PLB 270 (1991) 69
45	T. Csörgo, and S. Hegyi and Zajc, W. A.	Bose-Einstein correlations for L$ \'evy $ stable source distributions	EPJC 36 (2004) 67	nucl-th/0310042
46	T. Csörgo, A. T. Szerzo and S. Hegyi	Model independent shape analysis of correlations in 1, 2 or 3 dimensions	PLB 489 (2000) 15	hep-ph/9912220
47	E802 Collaboration	System, centrality, and transverse mass dependence of two pion correlation radii in heavy ion collisions at 11.6~A and 14.6 A~$ GeV /c $	PRC 66 (2002) 054906	nucl-ex/0204001
48	ATLAS Collaboration	Femtoscopy with identified charged pions in proton-lead collisions at $ \sqrt{s_{\text{NN}}} = $ 5.02 TeV with ATLAS	PRC 96 (2017) 064908	1704.01621
49	PHOBOS Collaboration	System size dependence of cluster properties from two-particle angular correlations in Cu+Cu and Au+Au collisions at $ \sqrt{s_{\text{NN}}} = $ 200 GeV	PRC 81 (2010) 024904	0812.1172
50	Y. Hama and Sandra S. Padula	Bose-Einstein correlation of particles produced by expanding sources	PRD 37 (1988) 3237
51	P. Bozek and W. Broniowski	Size of the emission source and collectivity in ultra-relativistic p-Pb collisions	PLB 720 (2013) 250	1301.3314
52	V. M. Shapoval, P. Braun-Munzinger, I. A. Karpenko, and Yu. M. Sinyukov	Femtoscopic scales in $ p+p $ and $ p $ + Pb collisions in view of the uncertainty principle	PLB 725 (2013) 139	1304.3815
53	J. C. Collins and M. J. Perry	Superdense matter: neutrons or asymptotically free quarks?	PRL 34 (1975) 1353
54	N. Cabibbo and G. Parisi	Exponential hadronic spectrum and quark liberation	PLB 59 (1975) 67
55	B. A. Freedman and L. D. McLerran	Fermions and gauge vector mesons at finite temperature and density. 1. Formal techniques	PRD 16 (1977) 1130
56	E. V. Shuryak	Theory of hadronic plasma	Sov. Phys. JETP 47 (1978) 212.[Zh. Eksp. Teor. Fiz. 74 (1978) 408]
57	M. I. Khalatnikov	Some questions onf the relativistic hydrodynamic	Zh. Eksp. Teor. Fiz. 26 (1954) 529
58	L. D. Landau	On the multiple production of particles in high energy collisions	Izv. Adak. Nauk SSSR Ser. Fiz. 17 (1953) 51
59	R. Campanini and G. Ferri	Experimental equation of state in $\mathrm{pp}$ and $ \mathrm{p}\overline{\mathrm{p}} $ collisions and phase transition to quark gluon plasma	PLB 703 (2011) 237	1106.2008
60	L. McLerran, M. Praszalowicz, and B. Schenke	Transverse momentum of protons, pions and kaons in high multiplicity pp and pa collisions: evidence for the color glass condensate?	NP A 916 (2013) 210	1306.2350
61	A. Bzdak, B. Schenke, P. Tribedy, and R. Venugopalan	Initial-state geometry and the role of hydrodynamics in proton-proton, proton-nucleus, and deuteron-nucleus collisions	PRC 87 (2013) 064906	1304.3403
62	M. Chojnacki, W. Florkowski, and T. Csörgo	Formation of Hubble-like flow in little bangs	PRC 71 (2005) 044902	nucl-th/0410036
63	T. Csörgo and B. Lörstad	Bose-Einstein correlations for three-dimensionally expanding, cylindrically symmetric, finite systems	PRC 54 (1996) 1390	hep-ph/9509213
64	PHENIX Collaboration	Bose-Einstein correlations of charged pion pairs in Au+Au collisions at $ \sqrt{s_{\text{NN}}} = $ 200 GeV	PRL 93 (2004) 152302	nucl-ex/0401003
65	STAR Collaboration	Pion interferometry in Au+Au and Cu+Cu collisions at $ \sqrt{s_{\text{NN}}} = $ 62.4 and 200 GeV	PRC 80 (2009) 024905	0903.1296
66	PHENIX Collaboration	Systematic study of charged-pion and kaon femtoscopy in Au+Au collisions at $ \sqrt{s_{\text{NN}}} = $ 200 GeV	PRC 92 (2015) 034914	1504.05168
67	ZEUS Collaboration	Bose-Einstein correlations in one and two-dimensions in deep inelastic scattering	PLB 583 (2004) 231	hep-ex/0311030
68	L3 Collaboration	Test of the $ \tau $-model of Bose-Einstein correlations and reconstruction of the source function in hadronic Z-boson decay at LEP	EPJC 71 (2011) 1648	1105.4788
69	T. Csörgo and J. Zimànyi	Pion interferometry for strongly correlated space-time and momentum space	NP A 517 (1990) 588