Compact Muon Solenoid
LHC, CERN
Visit us: CMS Public Website, CMS Physics ;
Contact us: CMS Publications Committee
| CMS-PAS-B2G-12-016 | ||
| Search for exotic light-flavor quark partners in pp collisions at $\sqrt{s} = $ 8 TeV | ||
| CMS Collaboration | ||
| December 2016 | ||
| Abstract: A search is presented for vector-like quarks (VLQ) that only couple to light generation quarks in proton-proton collisions at $\sqrt{s}= $ 8 TeV at the Large Hadron Collider (LHC). The data were collected by the CMS experiment during the 2012 LHC run, corresponding to an integrated luminosity of 19.7 fb$^{-1}$. Both single and pair production of VLQ are considered. The single production search is restricted to down-type vector-like quarks (electric charge $-1/3$), while the pair production search is sensitive to up-type (charge $+2/3$) and down-type heavy quarks. Final states with at least one muon or one electron are considered. No significant excess over standard model expectations is observed, and lower limits on the mass of VLQ are derived. Combining all processes together (including single production), the lower mass limits range from 400 GeV to 1800 GeV, depending on the VLQ branching fractions (BF) to W, Z or H bosons and the electroweak single-production strength. For pair production only, at the usual benchmark assuming $\mathrm{BF(W)}= $ 0.5, $\mathrm{BF(Z)}= $ 0.25 and $\mathrm{BF(H)}=$ 0.25, vector-like quarks with a mass below 685 GeV are excluded at 95% confidence level (with an expected limit of 720 GeV). For $\mathrm{BF(W)}=$ 1.0 the observed lower limit is 845 GeV (expected limit 825 GeV). The results are more stringent than those previously obtained in single and pair production of VLQ coupling to light generation quarks at 7 and 8 TeV. | ||
|
Links:
CDS record (PDF) ;
inSPIRE record ;
CADI line (restricted) ;
These preliminary results are superseded in this paper, PRD 97 (2018) 072008. The superseded preliminary plots can be found here. |
||
| Figures | |
png pdf |
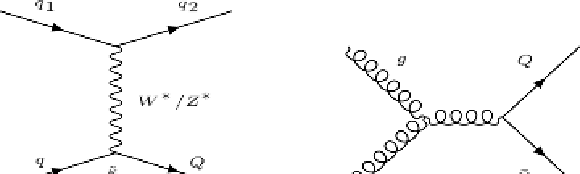
Figure 1:
Vector-like quarks (denoted Q) can be produced in proton-proton collisions either (left) singly through electroweak interactions (here the t-channel mode is shown as an example) or (right) in pairs via the strong interaction. |
png pdf |
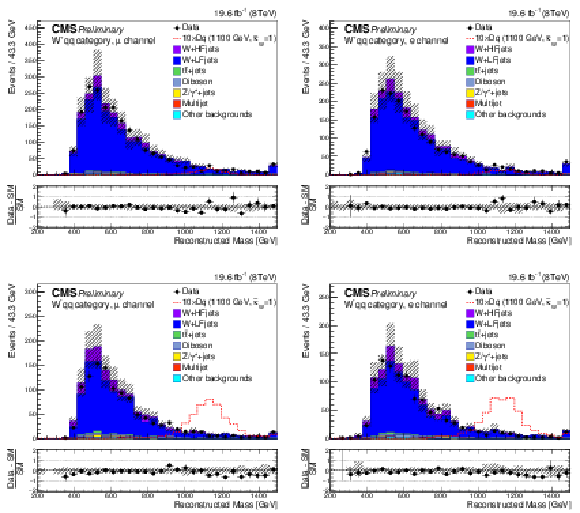
Figure 2:
The reconstructed mass of the vector-like quark in the $\mathrm{W^{+}qq}$ event category (top) and the $\mathrm{W^{-}qq}$ event category (bottom), in the muon channel (left) and the electron channel (right). The enhanced vector-like $D$ quark signal contribution in the $\mathrm{W^{-}qq}$ event category in comparison to the $\mathrm{W^{+}qq}$ event category is clearly shown. The distribution for a heavy vector-like quark signal of mass 1100 GeV and $\tilde{\kappa }_\mathrm {W} = 1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled up by a factor 10 for visibility. |
png pdf |
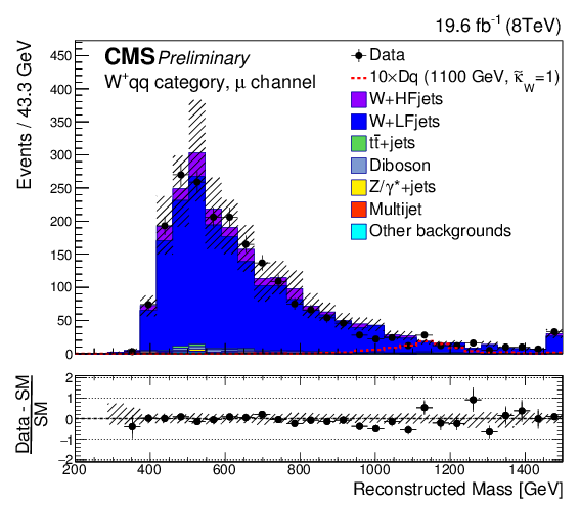
Figure 2-a:
The reconstructed mass of the vector-like quark in the $\mathrm{W^{+}qq}$ event category (top) and the $\mathrm{W^{-}qq}$ event category (bottom), in the muon channel (left) and the electron channel (right). The enhanced vector-like $D$ quark signal contribution in the $\mathrm{W^{-}qq}$ event category in comparison to the $\mathrm{W^{+}qq}$ event category is clearly shown. The distribution for a heavy vector-like quark signal of mass 1100 GeV and $\tilde{\kappa }_\mathrm {W} = 1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled up by a factor 10 for visibility. |
png pdf |
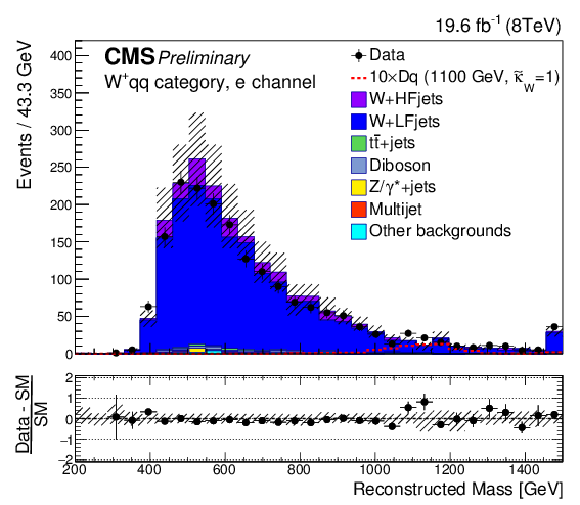
Figure 2-b:
The reconstructed mass of the vector-like quark in the $\mathrm{W^{+}qq}$ event category (top) and the $\mathrm{W^{-}qq}$ event category (bottom), in the muon channel (left) and the electron channel (right). The enhanced vector-like $D$ quark signal contribution in the $\mathrm{W^{-}qq}$ event category in comparison to the $\mathrm{W^{+}qq}$ event category is clearly shown. The distribution for a heavy vector-like quark signal of mass 1100 GeV and $\tilde{\kappa }_\mathrm {W} = 1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled up by a factor 10 for visibility. |
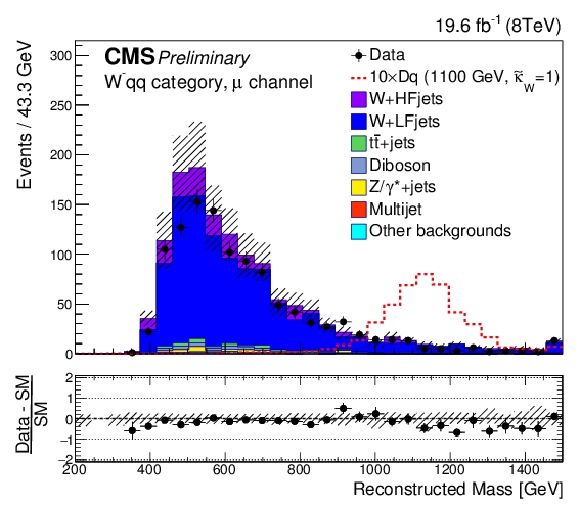
png pdf |
Figure 2-c:
The reconstructed mass of the vector-like quark in the $\mathrm{W^{+}qq}$ event category (top) and the $\mathrm{W^{-}qq}$ event category (bottom), in the muon channel (left) and the electron channel (right). The enhanced vector-like $D$ quark signal contribution in the $\mathrm{W^{-}qq}$ event category in comparison to the $\mathrm{W^{+}qq}$ event category is clearly shown. The distribution for a heavy vector-like quark signal of mass 1100 GeV and $\tilde{\kappa }_\mathrm {W} = 1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled up by a factor 10 for visibility. |
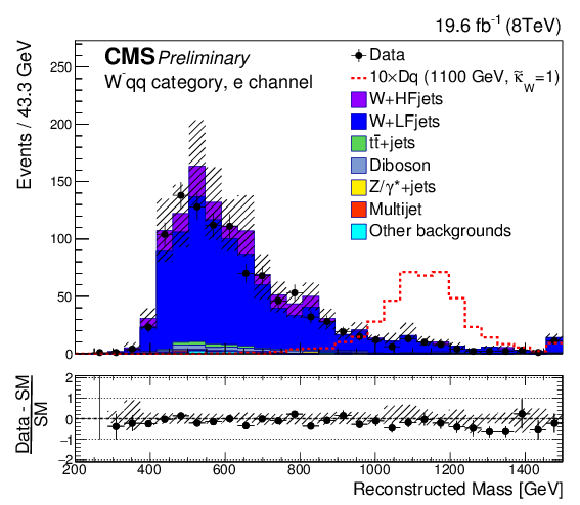
png pdf |
Figure 2-d:
The reconstructed mass of the vector-like quark in the $\mathrm{W^{+}qq}$ event category (top) and the $\mathrm{W^{-}qq}$ event category (bottom), in the muon channel (left) and the electron channel (right). The enhanced vector-like $D$ quark signal contribution in the $\mathrm{W^{-}qq}$ event category in comparison to the $\mathrm{W^{+}qq}$ event category is clearly shown. The distribution for a heavy vector-like quark signal of mass 1100 GeV and $\tilde{\kappa }_\mathrm {W} = 1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled up by a factor 10 for visibility. |
png pdf |
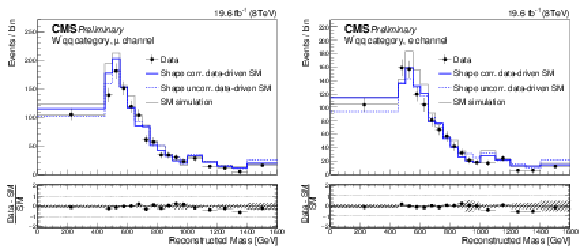
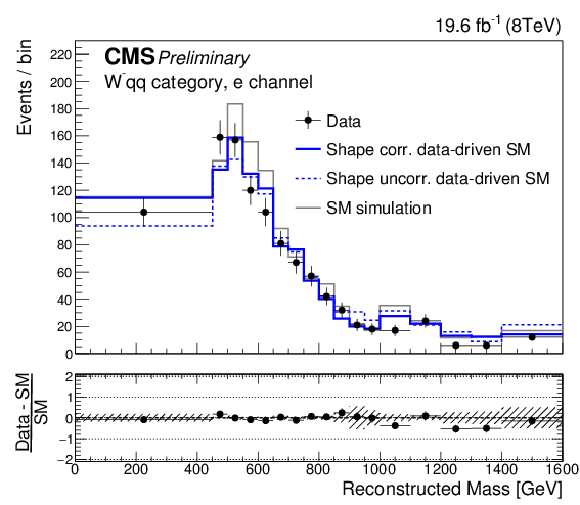
Figure 3:
The reconstructed vector-like quark mass in the $\mathrm {W}^-$qq category for the muon channel (left) and the electron channel (right). The solid bold (blue) line is the data-driven background distribution with shape correction, the dashed (blue) line is the same but without the shape correction, and the grey line represents the SM simulation. |
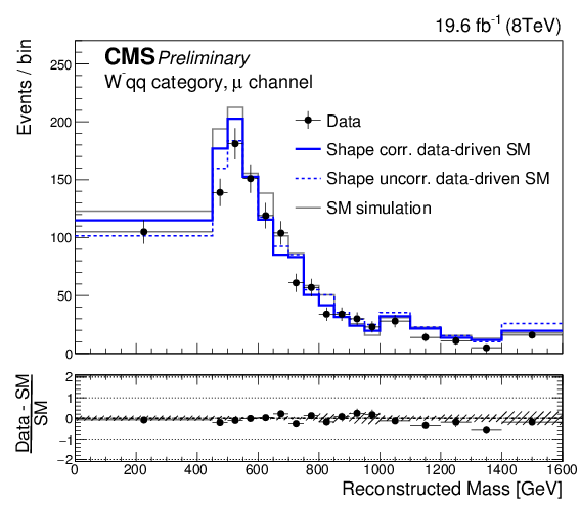
png pdf |
Figure 3-a:
The reconstructed vector-like quark mass in the $\mathrm {W}^-$qq category for the muon channel (left) and the electron channel (right). The solid bold (blue) line is the data-driven background distribution with shape correction, the dashed (blue) line is the same but without the shape correction, and the grey line represents the SM simulation. |
png pdf |
Figure 3-b:
The reconstructed vector-like quark mass in the $\mathrm {W}^-$qq category for the muon channel (left) and the electron channel (right). The solid bold (blue) line is the data-driven background distribution with shape correction, the dashed (blue) line is the same but without the shape correction, and the grey line represents the SM simulation. |
png pdf |
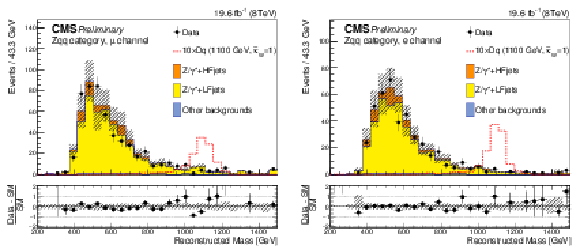
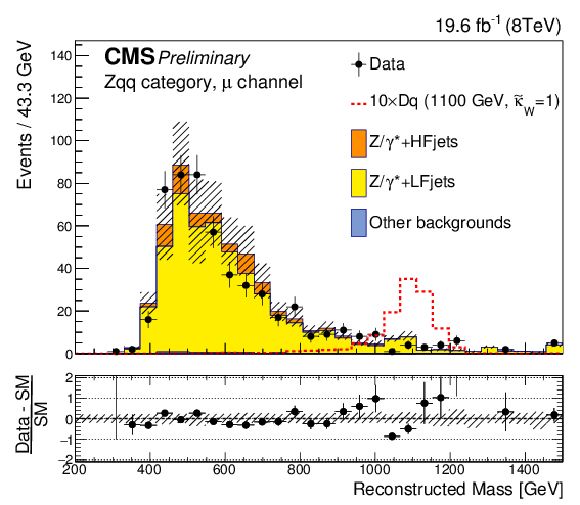
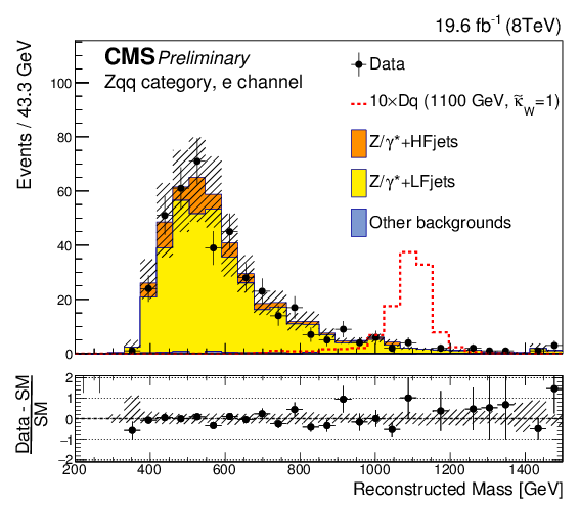
Figure 4:
The reconstructed mass of the vector-like quark in the Zqq event category, in the muon channel (left) and the electron channel (right). The distribution for a heavy vector-like quark signal of mass 1100 GeV and $\tilde{\kappa }_\mathrm {W} = 1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled by a factor 10 for better visibility. |
png pdf |
Figure 4-a:
The reconstructed mass of the vector-like quark in the Zqq event category, in the muon channel (left) and the electron channel (right). The distribution for a heavy vector-like quark signal of mass 1100 GeV and $\tilde{\kappa }_\mathrm {W} = 1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled by a factor 10 for better visibility. |
png pdf |
Figure 4-b:
The reconstructed mass of the vector-like quark in the Zqq event category, in the muon channel (left) and the electron channel (right). The distribution for a heavy vector-like quark signal of mass 1100 GeV and $\tilde{\kappa }_\mathrm {W} = 1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled by a factor 10 for better visibility. |
png pdf |
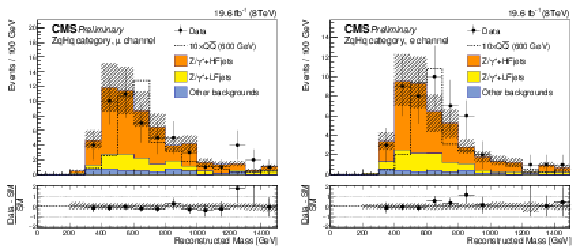
Figure 5:
The reconstructed mass of the vector-like quark candidate in the ZqHq event category, in the muon channel (left) and the electron channel (right). The distribution for a heavy vector-like quark signal of mass 600 GeV and $\tilde{\kappa }_\mathrm {W} = 0.1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=0.25$) is scaled up by a factor 10 for visibility. |
png pdf |
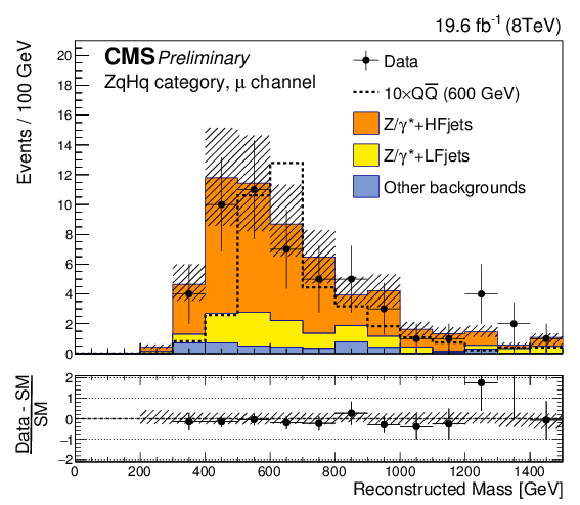
Figure 5-a:
The reconstructed mass of the vector-like quark candidate in the ZqHq event category, in the muon channel (left) and the electron channel (right). The distribution for a heavy vector-like quark signal of mass 600 GeV and $\tilde{\kappa }_\mathrm {W} = 0.1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=0.25$) is scaled up by a factor 10 for visibility. |
png pdf |
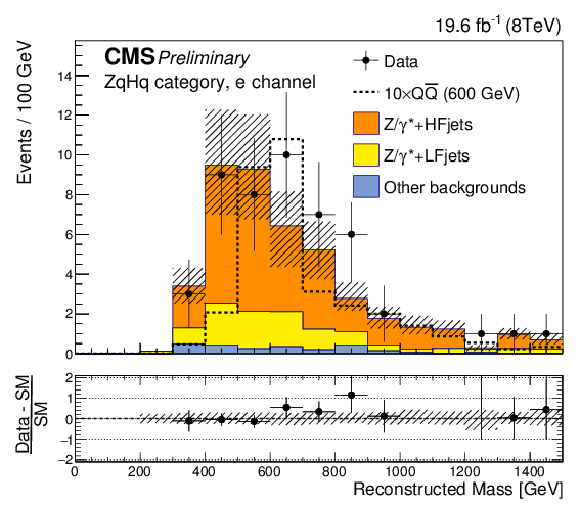
Figure 5-b:
The reconstructed mass of the vector-like quark candidate in the ZqHq event category, in the muon channel (left) and the electron channel (right). The distribution for a heavy vector-like quark signal of mass 600 GeV and $\tilde{\kappa }_\mathrm {W} = 0.1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=0.25$) is scaled up by a factor 10 for visibility. |
png pdf |
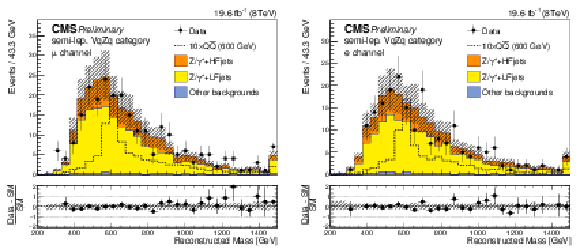
Figure 6:
The reconstructed mass of the vector-like quark candidate in the semi-leptonic VqZq event category, in the muon channel (left) and the electron channel (right). The distribution for a heavy vector-like quark signal of mass 600 GeV and $\tilde{\kappa }_\mathrm {W} = 0.1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled up by a factor 10 for visibility. |
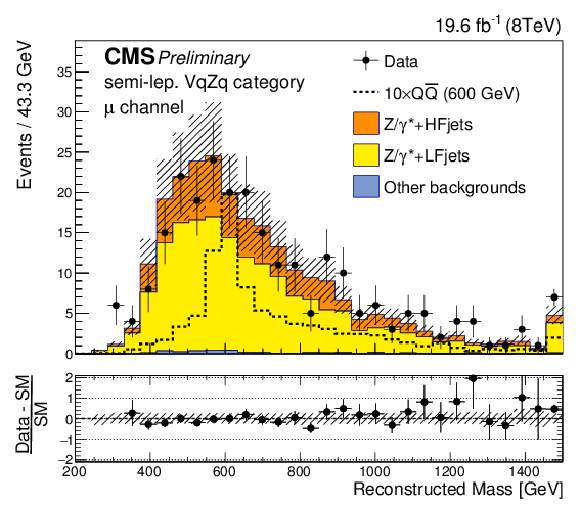
png pdf |
Figure 6-a:
The reconstructed mass of the vector-like quark candidate in the semi-leptonic VqZq event category, in the muon channel (left) and the electron channel (right). The distribution for a heavy vector-like quark signal of mass 600 GeV and $\tilde{\kappa }_\mathrm {W} = 0.1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled up by a factor 10 for visibility. |
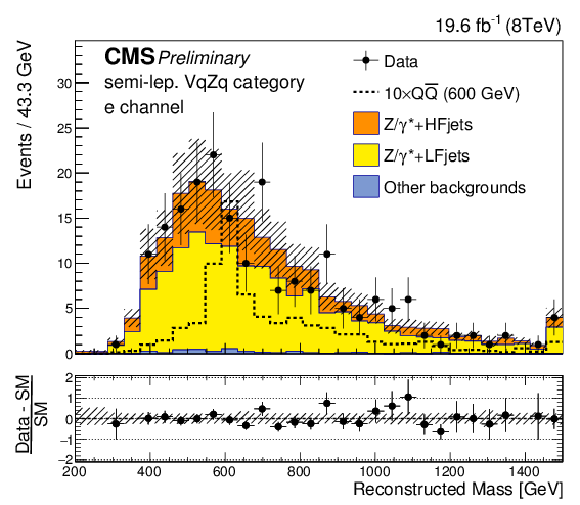
png pdf |
Figure 6-b:
The reconstructed mass of the vector-like quark candidate in the semi-leptonic VqZq event category, in the muon channel (left) and the electron channel (right). The distribution for a heavy vector-like quark signal of mass 600 GeV and $\tilde{\kappa }_\mathrm {W} = 0.1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled up by a factor 10 for visibility. |
png pdf |
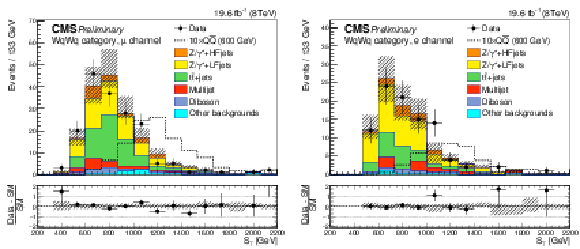
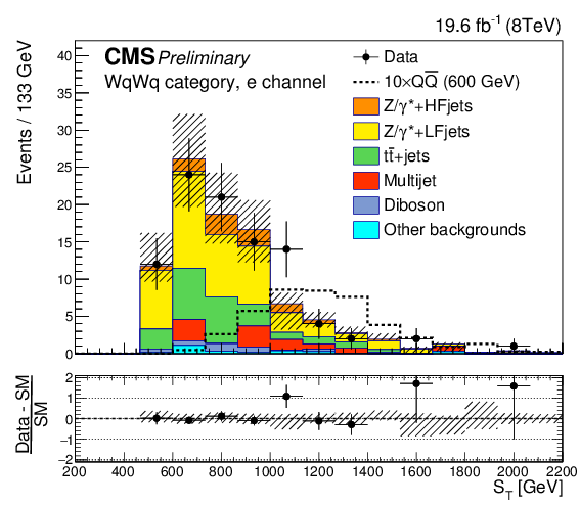
Figure 7:
The $S_T$ variable in the WqWq event category, in the muon channel (left) and in the electron channel (right). The distribution for a heavy vector-like quark signal of mass 600 GeV and $\tilde{\kappa }_\mathrm {W} = 0.1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled up by a factor 10 for visibility. |
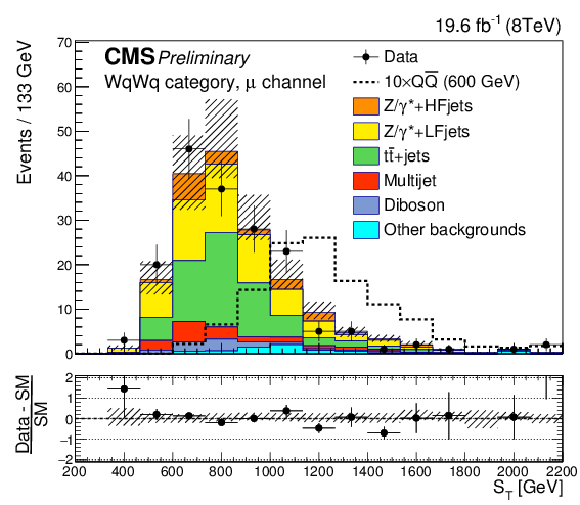
png pdf |
Figure 7-a:
The $S_T$ variable in the WqWq event category, in the muon channel (left) and in the electron channel (right). The distribution for a heavy vector-like quark signal of mass 600 GeV and $\tilde{\kappa }_\mathrm {W} = 0.1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled up by a factor 10 for visibility. |
png pdf |
Figure 7-b:
The $S_T$ variable in the WqWq event category, in the muon channel (left) and in the electron channel (right). The distribution for a heavy vector-like quark signal of mass 600 GeV and $\tilde{\kappa }_\mathrm {W} = 0.1$ (for $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25) is scaled up by a factor 10 for visibility. |
png pdf |
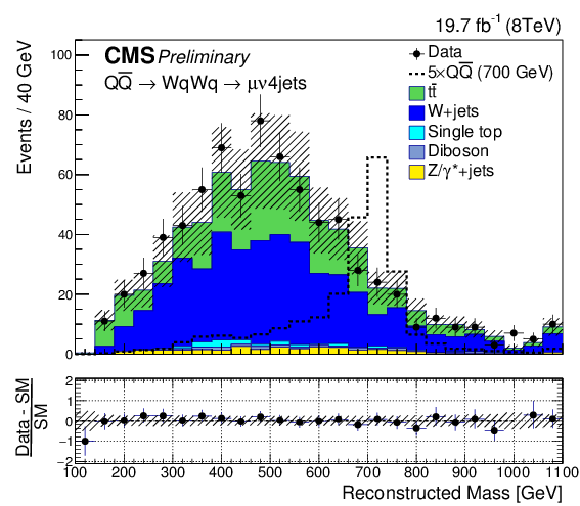
Figure 8:
Reconstructed mass distributions for WqWq (top), WqZq (middle) and WqHq (bottom) reconstruction. The left column is for the $\mu $+jets channel and the right column for the $e$+jets channel. |
png pdf |
Figure 8-a:
Reconstructed mass distributions for WqWq (top), WqZq (middle) and WqHq (bottom) reconstruction. The left column is for the $\mu $+jets channel and the right column for the $e$+jets channel. |
png pdf |
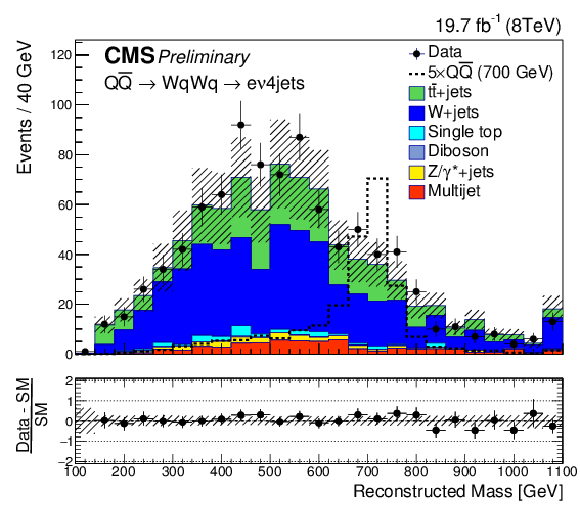
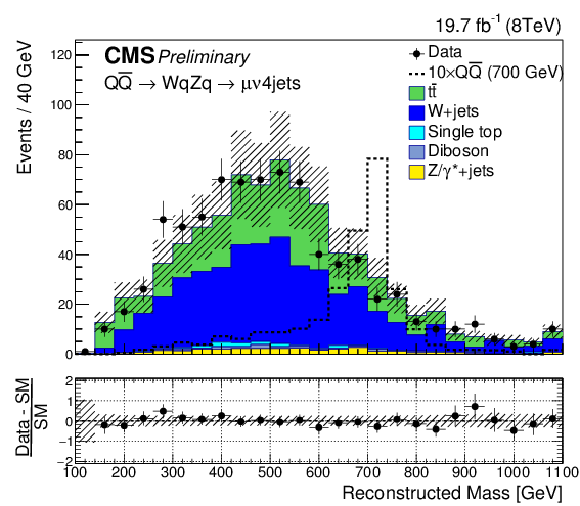
Figure 8-b:
Reconstructed mass distributions for WqWq (top), WqZq (middle) and WqHq (bottom) reconstruction. The left column is for the $\mu $+jets channel and the right column for the $e$+jets channel. |
png pdf |
Figure 8-c:
Reconstructed mass distributions for WqWq (top), WqZq (middle) and WqHq (bottom) reconstruction. The left column is for the $\mu $+jets channel and the right column for the $e$+jets channel. |
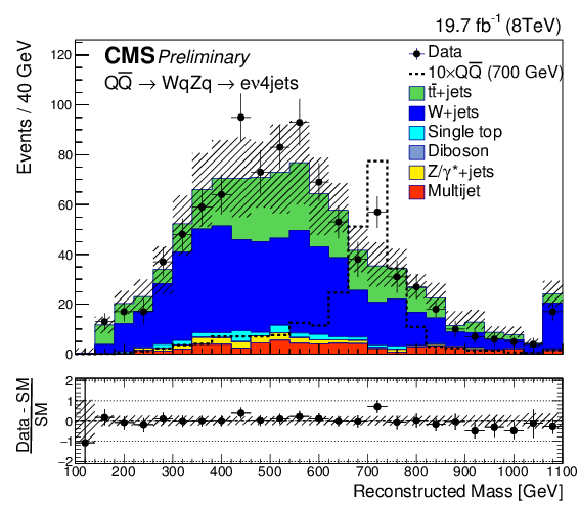
png pdf |
Figure 8-d:
Reconstructed mass distributions for WqWq (top), WqZq (middle) and WqHq (bottom) reconstruction. The left column is for the $\mu $+jets channel and the right column for the $e$+jets channel. |
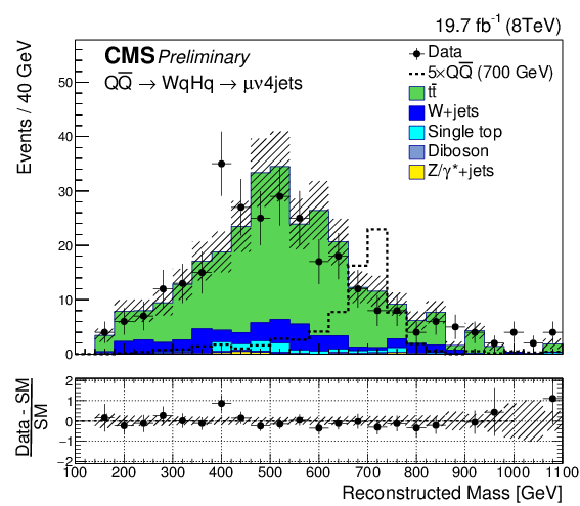
png pdf |
Figure 8-e:
Reconstructed mass distributions for WqWq (top), WqZq (middle) and WqHq (bottom) reconstruction. The left column is for the $\mu $+jets channel and the right column for the $e$+jets channel. |
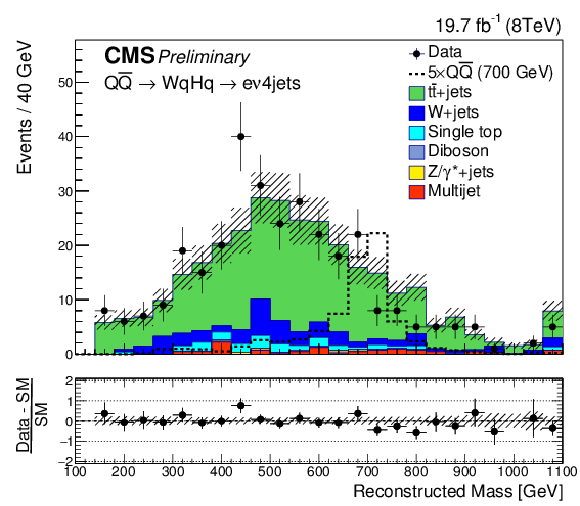
png pdf |
Figure 8-f:
Reconstructed mass distributions for WqWq (top), WqZq (middle) and WqHq (bottom) reconstruction. The left column is for the $\mu $+jets channel and the right column for the $e$+jets channel. |
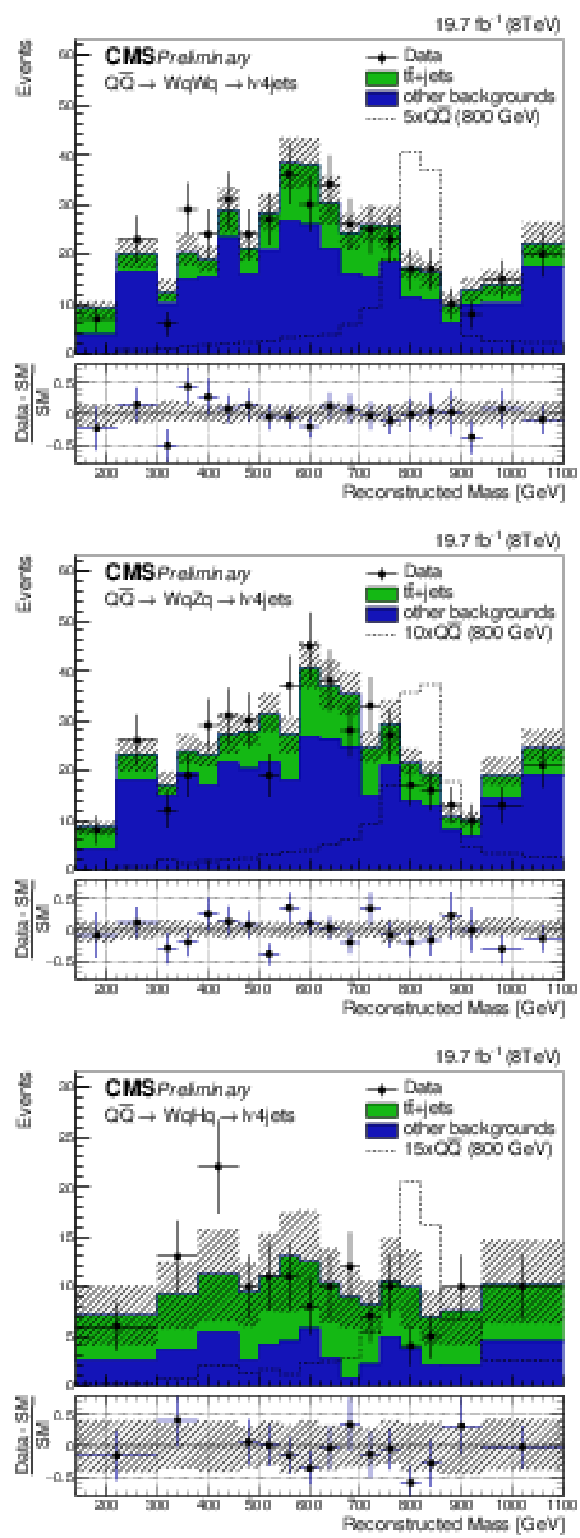
png pdf |
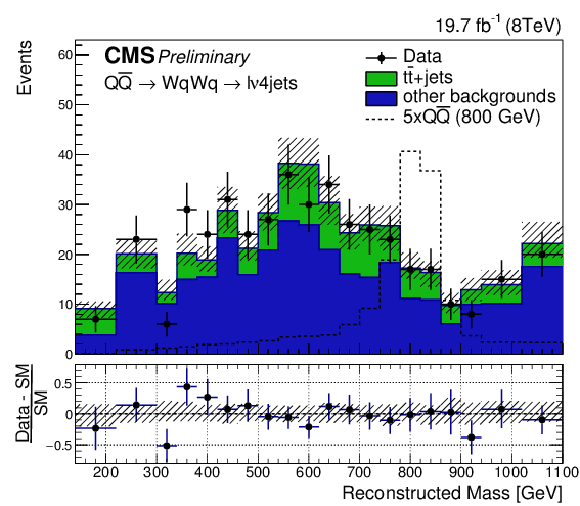
Figure 9:
Mass distributions for WqWq (top), WqZq (middle) and WqHq (bottom) reconstructions for the combination of the $\mu $+jets and $e$+jets channels and for $S_T >$ 1240 GeV. |
png pdf |
Figure 9-a:
Mass distributions for WqWq (top), WqZq (middle) and WqHq (bottom) reconstructions for the combination of the $\mu $+jets and $e$+jets channels and for $S_T >$ 1240 GeV. |
png pdf |
Figure 9-b:
Mass distributions for WqWq (top), WqZq (middle) and WqHq (bottom) reconstructions for the combination of the $\mu $+jets and $e$+jets channels and for $S_T >$ 1240 GeV. |
png pdf |
Figure 9-c:
Mass distributions for WqWq (top), WqZq (middle) and WqHq (bottom) reconstructions for the combination of the $\mu $+jets and $e$+jets channels and for $S_T >$ 1240 GeV. |
png pdf |
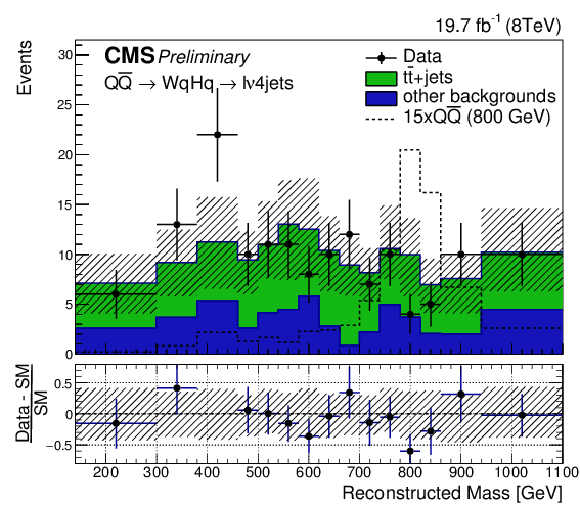
Figure 10:
Mass distribution for WqHq reconstruction, for combined $\mu $+jets and $e$+jets channels and for $S_T >$ 1240 GeV, where events appearing in WqWq sample have been removed. |
png pdf |
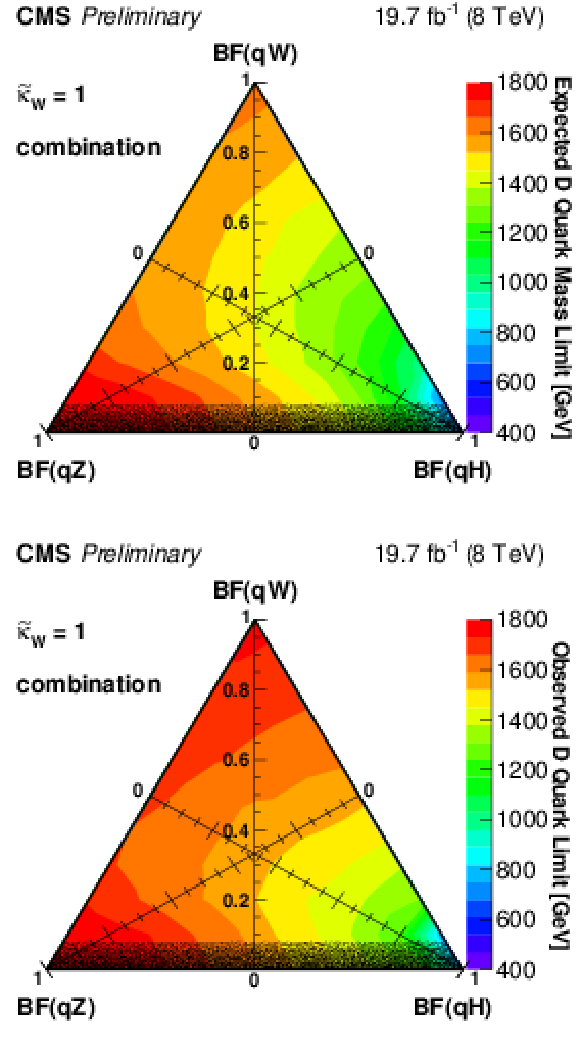
Figure 11:
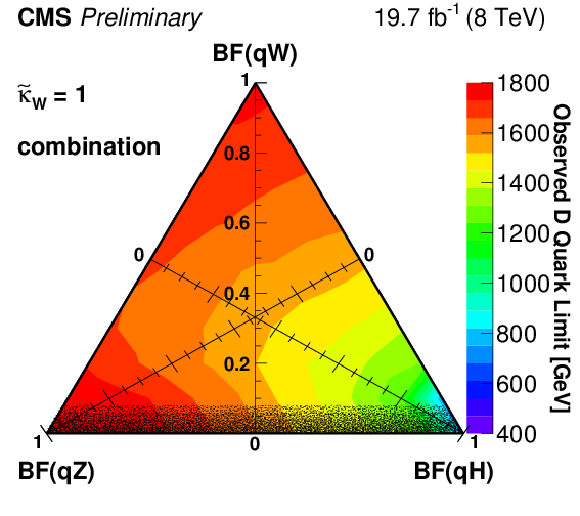
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for the decay of a vector-like quark into a boson and a first-generation quark. The limit contours are determined assuming that $\tilde{\kappa }_\mathrm {W} = 1$, which means that the signal is dominated by electroweak single production. The black shaded band near BF(qW)=0 shows a region where the results can not be reliably interpreted because $\tilde{\kappa }_\mathrm {Z}$ diverges (see text). |
png pdf |
Figure 11-a:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for the decay of a vector-like quark into a boson and a first-generation quark. The limit contours are determined assuming that $\tilde{\kappa }_\mathrm {W} = 1$, which means that the signal is dominated by electroweak single production. The black shaded band near BF(qW)=0 shows a region where the results can not be reliably interpreted because $\tilde{\kappa }_\mathrm {Z}$ diverges (see text). |
png pdf |
Figure 11-b:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for the decay of a vector-like quark into a boson and a first-generation quark. The limit contours are determined assuming that $\tilde{\kappa }_\mathrm {W} = 1$, which means that the signal is dominated by electroweak single production. The black shaded band near BF(qW)=0 shows a region where the results can not be reliably interpreted because $\tilde{\kappa }_\mathrm {Z}$ diverges (see text). |
png pdf |
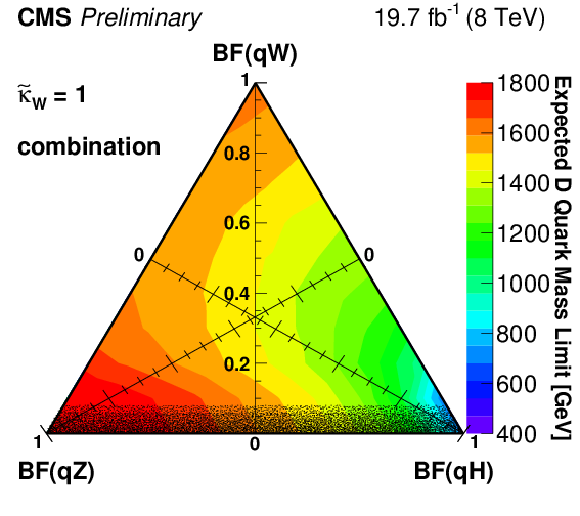
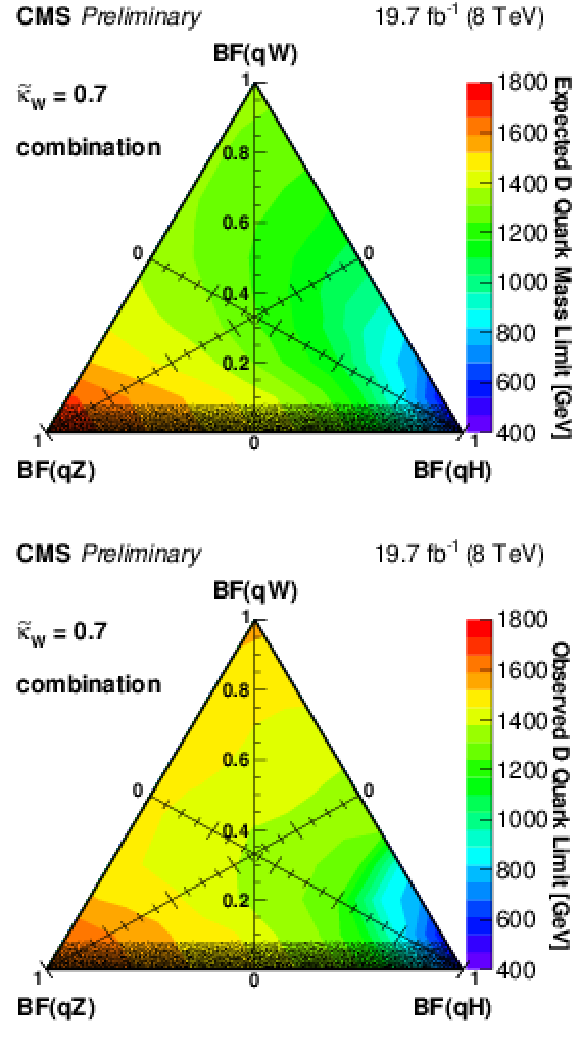
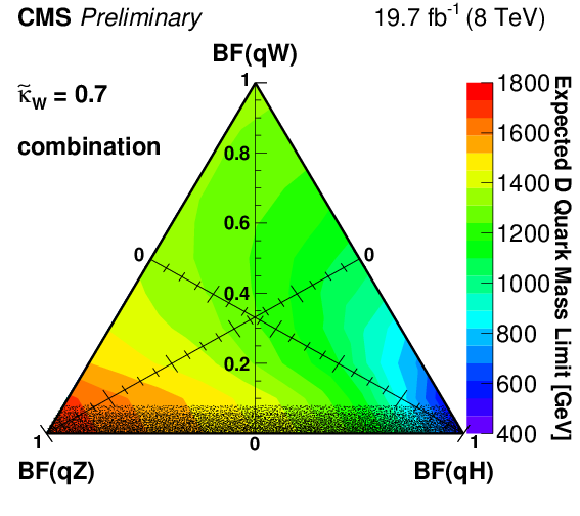
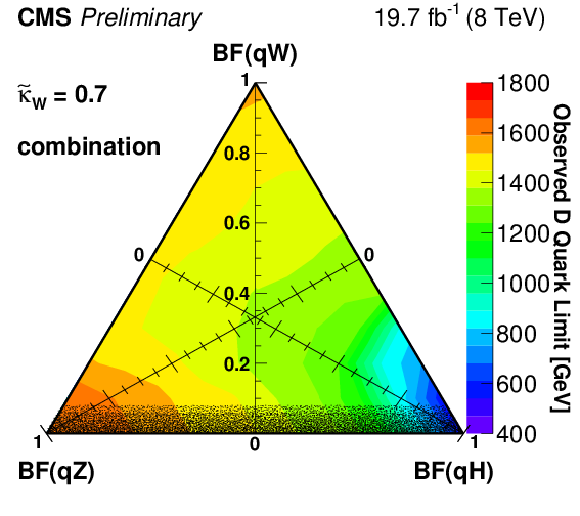
Figure 12:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for a vector-like quark decaying into a boson and a first-generation quark. The limit contours are determined assuming $\tilde{\kappa }_\mathrm {W} = 0.7$, which means that the signal will be dominated by electroweak single production for most of the parameter space represented by the triangles. The black shaded band near BF(qW)=0 represents a region where results can not be reliably interpreted because $\tilde{\kappa }_\mathrm {Z}$ diverges (see text). |
png pdf |
Figure 12-a:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for a vector-like quark decaying into a boson and a first-generation quark. The limit contours are determined assuming $\tilde{\kappa }_\mathrm {W} = 0.7$, which means that the signal will be dominated by electroweak single production for most of the parameter space represented by the triangles. The black shaded band near BF(qW)=0 represents a region where results can not be reliably interpreted because $\tilde{\kappa }_\mathrm {Z}$ diverges (see text). |
png pdf |
Figure 12-b:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for a vector-like quark decaying into a boson and a first-generation quark. The limit contours are determined assuming $\tilde{\kappa }_\mathrm {W} = 0.7$, which means that the signal will be dominated by electroweak single production for most of the parameter space represented by the triangles. The black shaded band near BF(qW)=0 represents a region where results can not be reliably interpreted because $\tilde{\kappa }_\mathrm {Z}$ diverges (see text). |
png pdf |
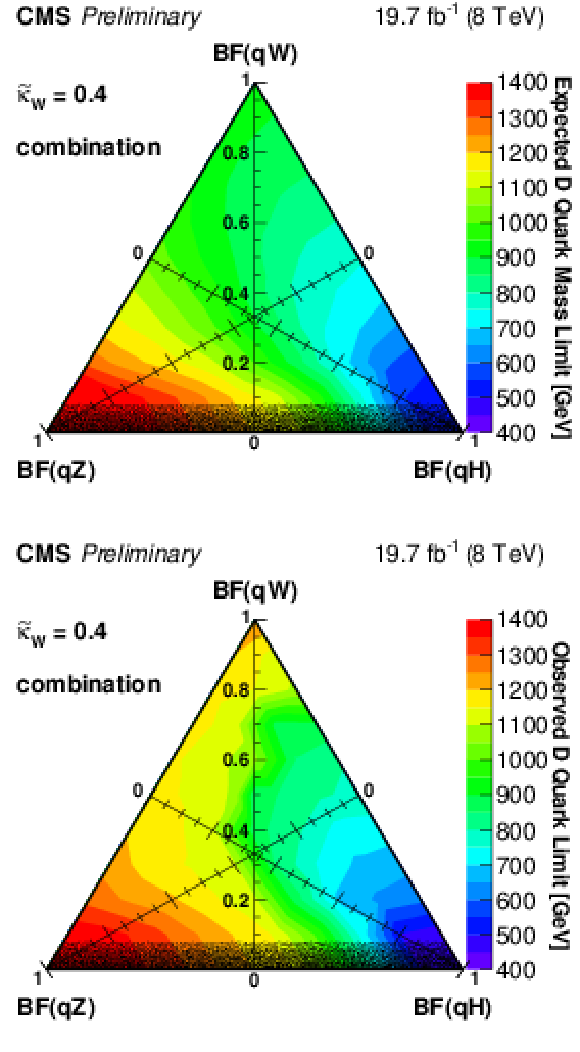
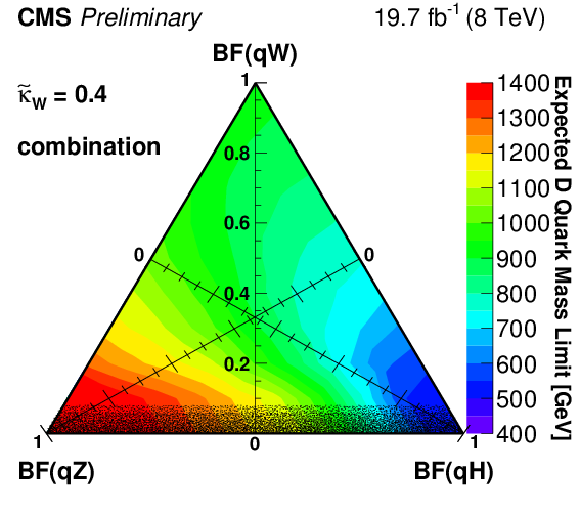
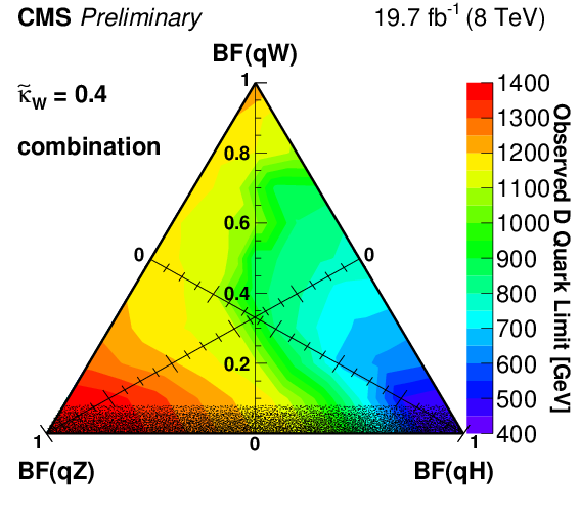
Figure 13:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for a vector-like quark decaying into a boson and a first-generation quark. The limit contours were determined assuming $\tilde{\kappa }_\mathrm {W} = 0.4$, which means that the signal is dominated by electroweak single production in most of the parameter space represented by the triangles, but in which the relative importance of the pair-produced signal has increased. The black shaded band near $\mathrm {BF(qW)}$=0 represents a region where results can not be reliably interpreted because $\tilde{\kappa }_\mathrm {Z}$ diverges (see text). |
png pdf |
Figure 13-a:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for a vector-like quark decaying into a boson and a first-generation quark. The limit contours were determined assuming $\tilde{\kappa }_\mathrm {W} = 0.4$, which means that the signal is dominated by electroweak single production in most of the parameter space represented by the triangles, but in which the relative importance of the pair-produced signal has increased. The black shaded band near $\mathrm {BF(qW)}$=0 represents a region where results can not be reliably interpreted because $\tilde{\kappa }_\mathrm {Z}$ diverges (see text). |
png pdf |
Figure 13-b:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for a vector-like quark decaying into a boson and a first-generation quark. The limit contours were determined assuming $\tilde{\kappa }_\mathrm {W} = 0.4$, which means that the signal is dominated by electroweak single production in most of the parameter space represented by the triangles, but in which the relative importance of the pair-produced signal has increased. The black shaded band near $\mathrm {BF(qW)}$=0 represents a region where results can not be reliably interpreted because $\tilde{\kappa }_\mathrm {Z}$ diverges (see text). |
png pdf |
Figure 14:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for a vector-like quark decaying into a boson and a first-generation quark. The limit contours were determined assuming $\tilde{\kappa }_\mathrm {W} = 0.1$, which means that the signal is dominated by strong pair production for most of the parameter space represented by the triangles. The black shaded band near $\mathrm {BF(qW)}$=0 indicates a region where results can not be reliably interpreted because $\tilde{\kappa }_\mathrm {Z}$ diverges (see text). |
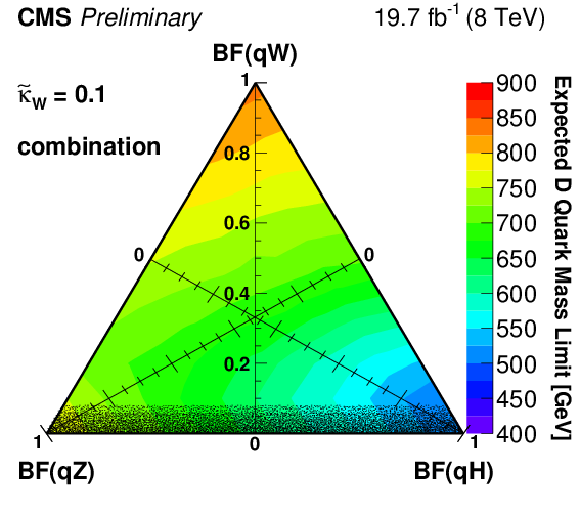
png pdf |
Figure 14-a:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for a vector-like quark decaying into a boson and a first-generation quark. The limit contours were determined assuming $\tilde{\kappa }_\mathrm {W} = 0.1$, which means that the signal is dominated by strong pair production for most of the parameter space represented by the triangles. The black shaded band near $\mathrm {BF(qW)}$=0 indicates a region where results can not be reliably interpreted because $\tilde{\kappa }_\mathrm {Z}$ diverges (see text). |
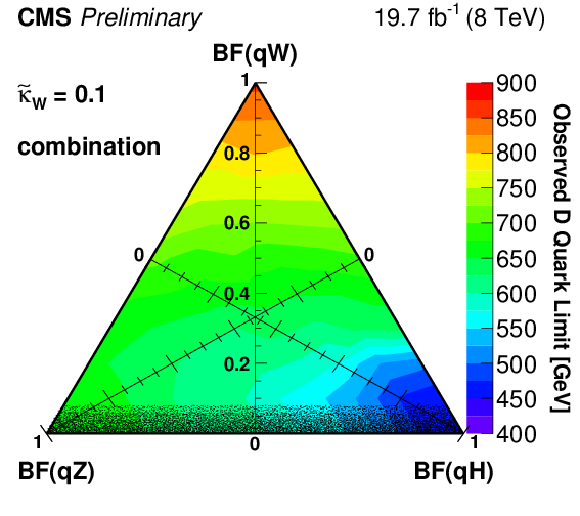
png pdf |
Figure 14-b:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for a vector-like quark decaying into a boson and a first-generation quark. The limit contours were determined assuming $\tilde{\kappa }_\mathrm {W} = 0.1$, which means that the signal is dominated by strong pair production for most of the parameter space represented by the triangles. The black shaded band near $\mathrm {BF(qW)}$=0 indicates a region where results can not be reliably interpreted because $\tilde{\kappa }_\mathrm {Z}$ diverges (see text). |
png pdf |
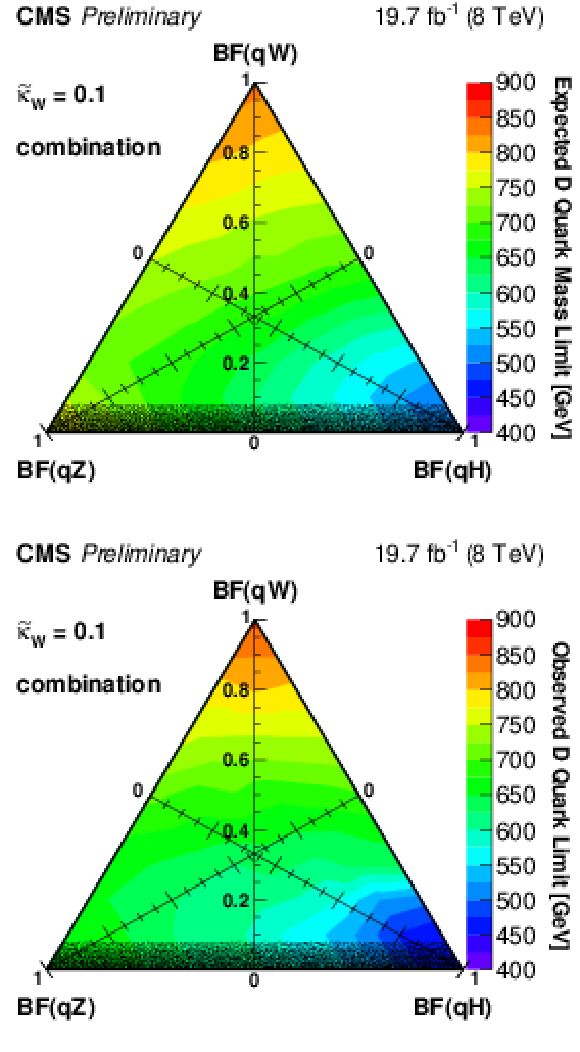
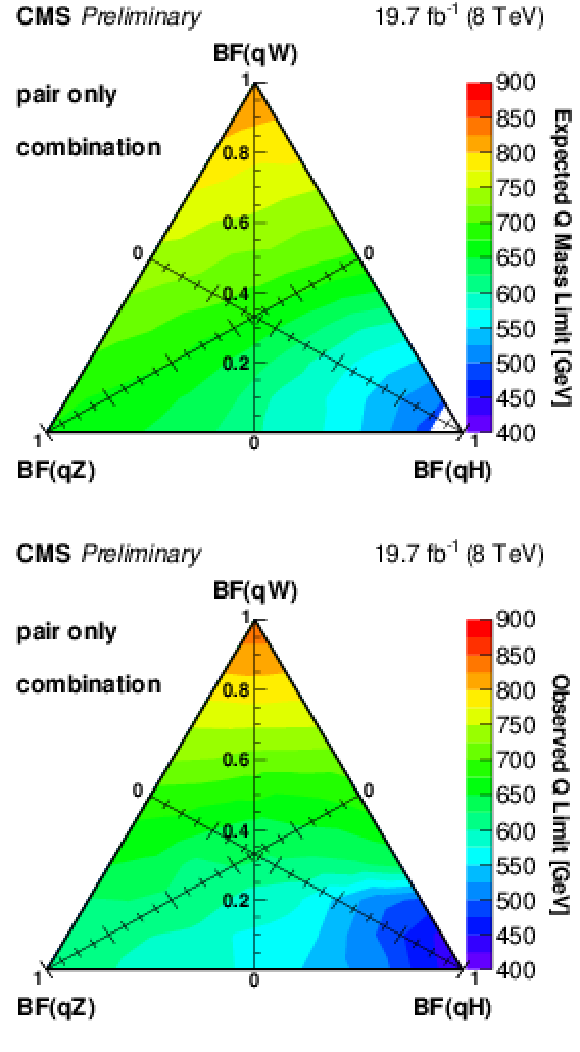
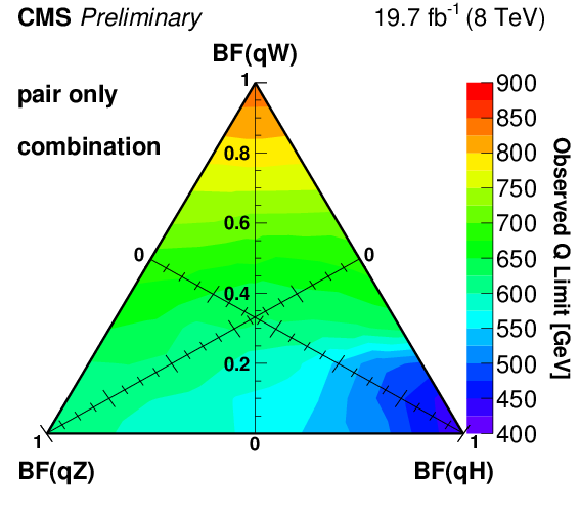
Figure 15:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for a vector-like quark decaying into a boson and a first-generation quark. The limit contours were determined assuming that $\tilde{\kappa }_\mathrm {W}$ and $\tilde{\kappa }_\mathrm {Z}$ are so small that the single production modes can be neglected, and therefore that the heavy quarks can only be produced in pairs via the strong interaction. |
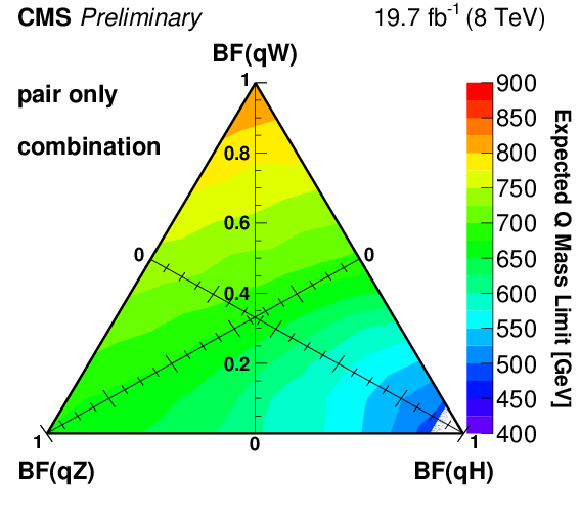
png pdf |
Figure 15-a:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for a vector-like quark decaying into a boson and a first-generation quark. The limit contours were determined assuming that $\tilde{\kappa }_\mathrm {W}$ and $\tilde{\kappa }_\mathrm {Z}$ are so small that the single production modes can be neglected, and therefore that the heavy quarks can only be produced in pairs via the strong interaction. |
png pdf |
Figure 15-b:
The expected (top) and observed (bottom) combined lower mass limits represented in triangular form, where each point of the triangle corresponds to a given set of branching fractions for a vector-like quark decaying into a boson and a first-generation quark. The limit contours were determined assuming that $\tilde{\kappa }_\mathrm {W}$ and $\tilde{\kappa }_\mathrm {Z}$ are so small that the single production modes can be neglected, and therefore that the heavy quarks can only be produced in pairs via the strong interaction. |
png pdf |
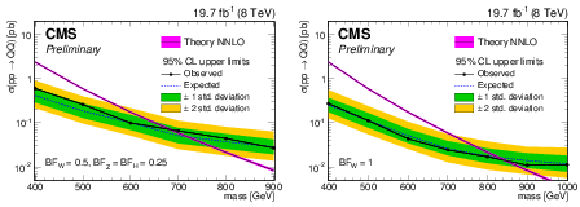
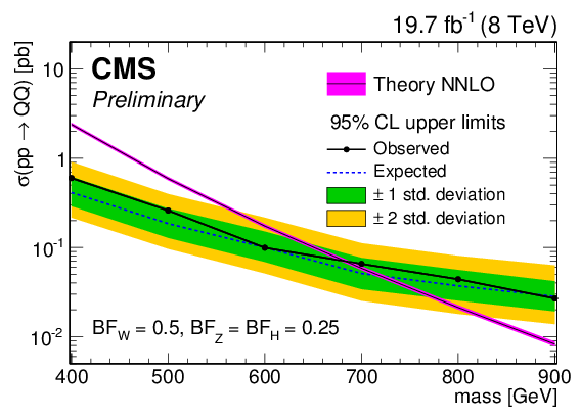
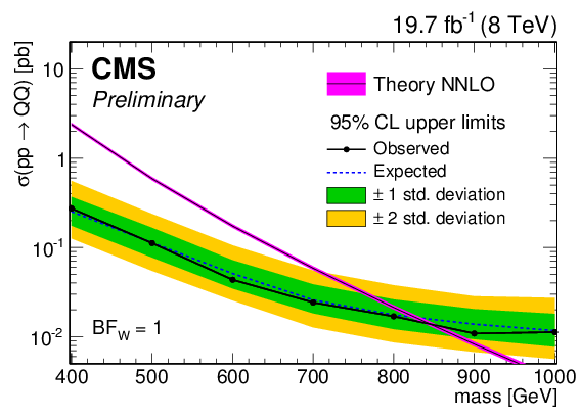
Figure 16:
The 95% CL exclusion limits on the production cross section determined assuming different sets of model parameters ($\mathrm {BF}_\mathrm {W} =$ 0.5, $\mathrm {BF}_\mathrm {Z} =$ 0.25 on the left, and $\mathrm {BF}_\mathrm {W} =$ 1.0 on the right) as a function of the hypothetical heavy quark mass, and for the scenario where only strong pair production of the heavy vector-like quark is considered. The median expected and observed exclusion limits are indicated with a dashed and a solid line, respectively. The ${\pm}$1$\sigma $ and $\pm$2$ \sigma $ expected exclusion limits are represented by the inner (green) and outer (yellow) bands around the median. The cross section from a full NNLO calculation [47], including uncertainties on the PDF and the renormalization and factorization scales, is shown by the magenta band. |
png pdf |
Figure 16-a:
The 95% CL exclusion limits on the production cross section determined assuming different sets of model parameters ($\mathrm {BF}_\mathrm {W} =$ 0.5, $\mathrm {BF}_\mathrm {Z} =$ 0.25 on the left, and $\mathrm {BF}_\mathrm {W} =$ 1.0 on the right) as a function of the hypothetical heavy quark mass, and for the scenario where only strong pair production of the heavy vector-like quark is considered. The median expected and observed exclusion limits are indicated with a dashed and a solid line, respectively. The ${\pm}$1$\sigma $ and $\pm$2$ \sigma $ expected exclusion limits are represented by the inner (green) and outer (yellow) bands around the median. The cross section from a full NNLO calculation [47], including uncertainties on the PDF and the renormalization and factorization scales, is shown by the magenta band. |
png pdf |
Figure 16-b:
The 95% CL exclusion limits on the production cross section determined assuming different sets of model parameters ($\mathrm {BF}_\mathrm {W} =$ 0.5, $\mathrm {BF}_\mathrm {Z} =$ 0.25 on the left, and $\mathrm {BF}_\mathrm {W} =$ 1.0 on the right) as a function of the hypothetical heavy quark mass, and for the scenario where only strong pair production of the heavy vector-like quark is considered. The median expected and observed exclusion limits are indicated with a dashed and a solid line, respectively. The ${\pm}$1$\sigma $ and $\pm$2$ \sigma $ expected exclusion limits are represented by the inner (green) and outer (yellow) bands around the median. The cross section from a full NNLO calculation [47], including uncertainties on the PDF and the renormalization and factorization scales, is shown by the magenta band. |
png pdf |
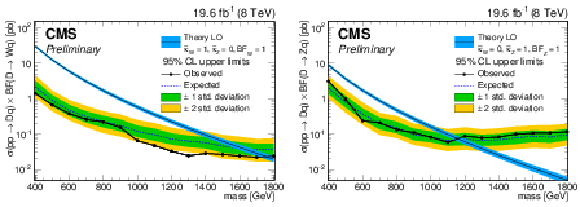
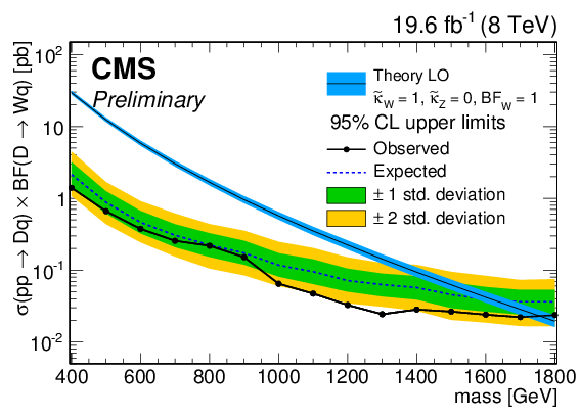
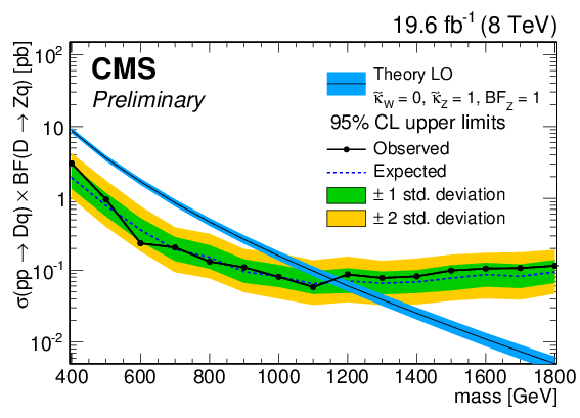
Figure 17:
The 95% CL exclusion limits on the production cross section times branching fraction, assuming a neutral current coupling of zero (left) or a charged current coupling of zero (right). The corresponding leading order theory predictions are superimposed. The predictions are represented by a solid black line centered within a blue band, which shows the uncertainty of the calculation. The uncertainties are determined based on the choice of PDF set along with the renormalization and factorization scales. |
png pdf |
Figure 17-a:
The 95% CL exclusion limits on the production cross section times branching fraction, assuming a neutral current coupling of zero (left) or a charged current coupling of zero (right). The corresponding leading order theory predictions are superimposed. The predictions are represented by a solid black line centered within a blue band, which shows the uncertainty of the calculation. The uncertainties are determined based on the choice of PDF set along with the renormalization and factorization scales. |
png pdf |
Figure 17-b:
The 95% CL exclusion limits on the production cross section times branching fraction, assuming a neutral current coupling of zero (left) or a charged current coupling of zero (right). The corresponding leading order theory predictions are superimposed. The predictions are represented by a solid black line centered within a blue band, which shows the uncertainty of the calculation. The uncertainties are determined based on the choice of PDF set along with the renormalization and factorization scales. |
| Tables | |
png pdf |
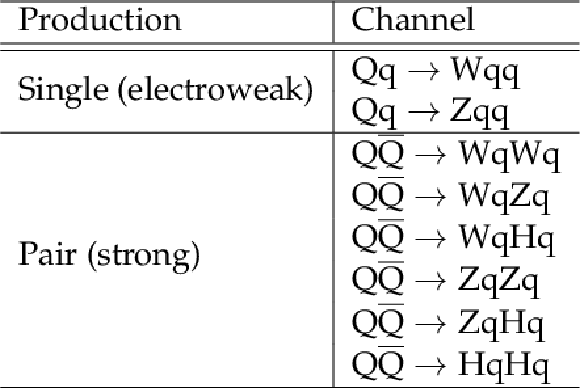
Table 1:
Decay channels of vector-like quarks, Q, considered in the analysis. |
png pdf |
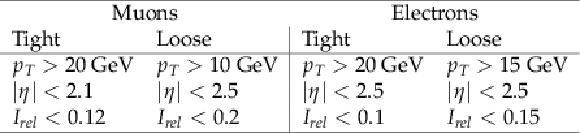
Table 2:
Basic selection requirements for tight and loose leptons. |
png pdf |
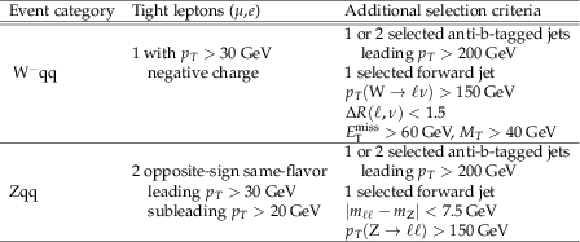
Table 3:
The event categories in this table are optimized for the single production of vector-like quarks. They are defined according to the number of tight muons or electrons present in the event along with additional criteria optimized for specific vector-like quark topologies. In each category, we exclude any events that contain additional loose leptons. |
png pdf |
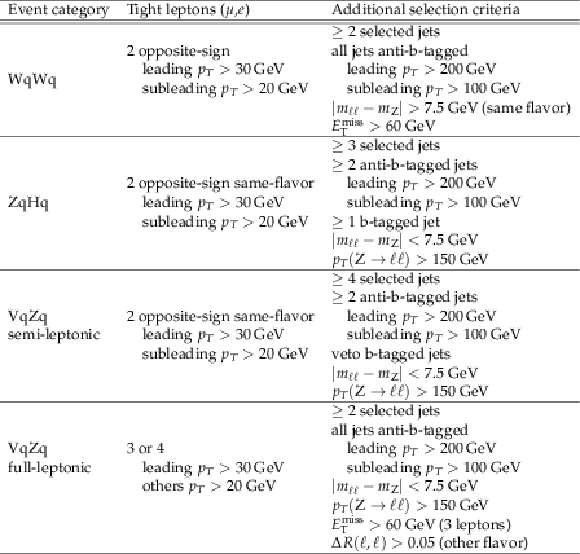
Table 4:
The event categories in this table are optimized for the pair production of vector-like quarks. They are defined according to the number of tight muons or electrons present in the event along with additional criteria optimized for specific vector-like quark topologies. In each event category, we exclude events that contain any additional loose leptons. |
png pdf |
Table 5:
Event yields in the muon channel for the inclusive analysis, for the event categories with one or two isolated leptons. The $\mathrm{W^{+}qq}$ event category is not used in the search, but is shown to demonstrate the expected lepton charge asymmetry. The indicated uncertainties are statistical only. The prediction for the signals is shown assuming branching fractions of $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}= $ 0.25. |
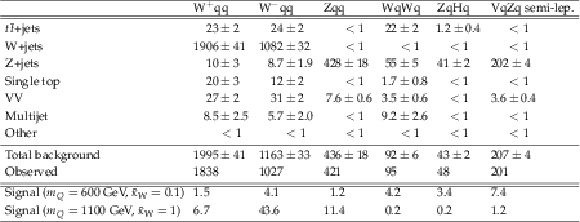
png pdf |
Table 6:
Event yields in the electron channel for the inclusive analysis, for the event categories with one or two isolated leptons. The $\mathrm{W^{+}qq}$ event category is not used in the search, but is shown to demonstrate the expected lepton charge asymmetry. The indicated uncertainties are statistical only. The prediction for the signals is shown assuming branching fractions of $\mathrm {BF}_\mathrm {W} =$ 0.5 and $\mathrm {BF}_\mathrm {Z} = \mathrm {BF}_\mathrm {H}=$ 0.25. |
png pdf |
Table 7:
Discriminating variables used in the definition of the different event categories. |
png pdf |
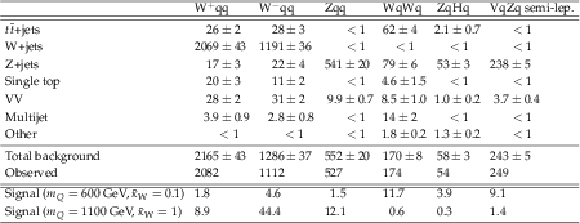
Table 8:
The prediction for the total number of background events compared to the number of observed events in the full-leptonic VqZq event category. The numbers of expected signal events for two different signal hypotheses are shown. The indicated uncertainties are statistical only. |
png pdf |
Table 9:
Combinations of two non-V-boson-decay jets (their b-tag content), which can be accepted to be matched to the quark pair $\mathrm {\{q_l, q_h\}}$. |
png pdf |
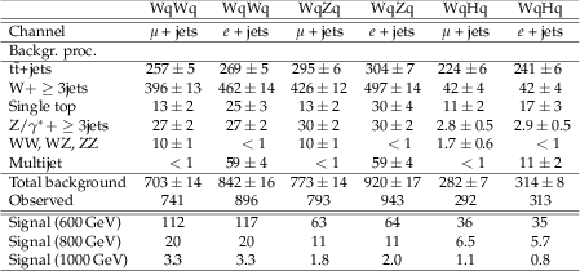
Table 10:
Number of data and expected MC background events for the WqWq, WqZq and WqHq channels after applying the event selection. The uncertainty on the MC prediction is statistical. |
png pdf |
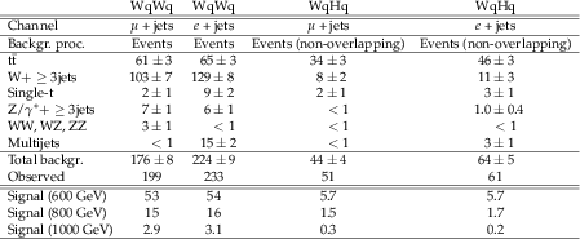
Table 11:
Number of data and expected MC background events in the WqWq and WqHq channels (overlap with WqWq excluded) after the application of the $S_T >$ 1240 GeV requirement. |
png pdf |
Table 12:
Comparison of example expected 95% CL lower mass limits for signal pair production only, illustrating the added sensitivity of the two analyses in the combination. |
png pdf |
Table 13:
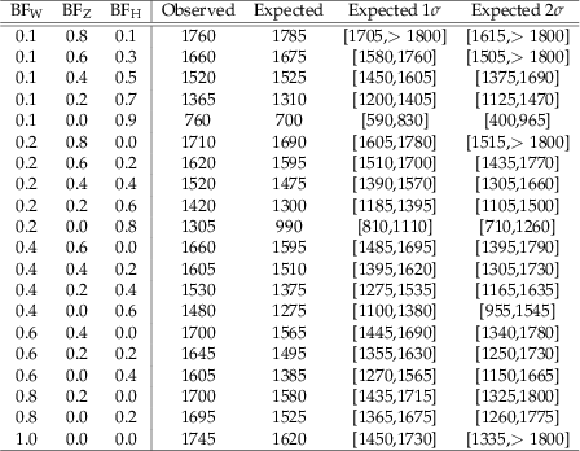
Observed and expected lower limits on the mass (in GeV) of the vector-like quark at 95% CL, for a range of different combinations of decay branching fractions. The $1\sigma $ and $2\sigma $ ranges on the expected limits are also given. The limits are determined assuming $\tilde{\kappa }_\mathrm {W} = 1$. |
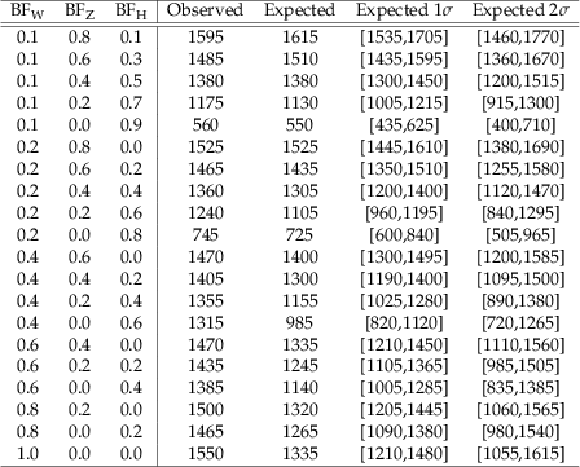
png pdf |
Table 14:
Observed and expected lower limits on the mass (in GeV) of the vector-like quark at 95% CL, for a range of different combinations of decay branching fractions. The $1\sigma $ and $2\sigma $ ranges on the expected limits are also given. The limits were determined using $\tilde{\kappa }_\mathrm {W} = 0.7$. |
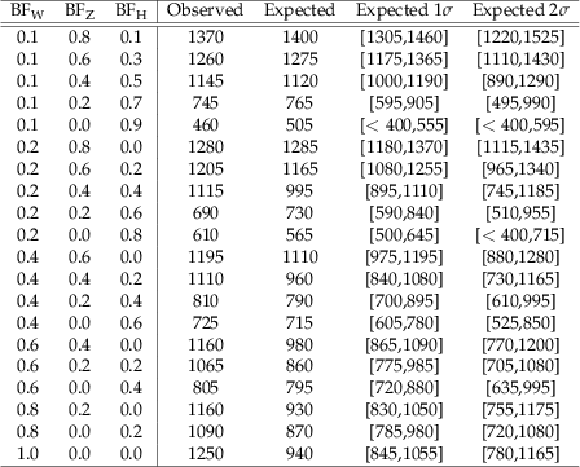
png pdf |
Table 15:
Observed and expected lower limits on the mass (in GeV) of the vector-like quark at 95% CL, for a range of different combinations of decay branching fractions. The $1\sigma $ and $2\sigma $ ranges on the expected limits are also given. The limits are determined assuming $\tilde{\kappa }_\mathrm {W} = 0.4$. |
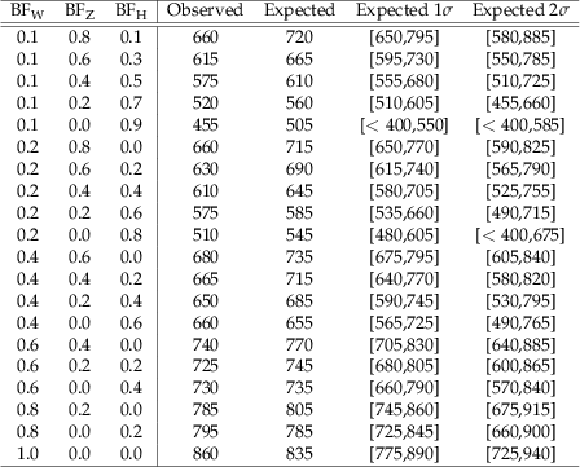
png pdf |
Table 16:
Observed and expected lower limits on the mass (in GeV) of the vector-like quark at 95% CL, for a range of different combinations of decay branching fractions. The $1\sigma $ and $2\sigma $ ranges on the expected limits are also given. The limits were determined assuming $\tilde{\kappa }_\mathrm {W} = 0.1$. |
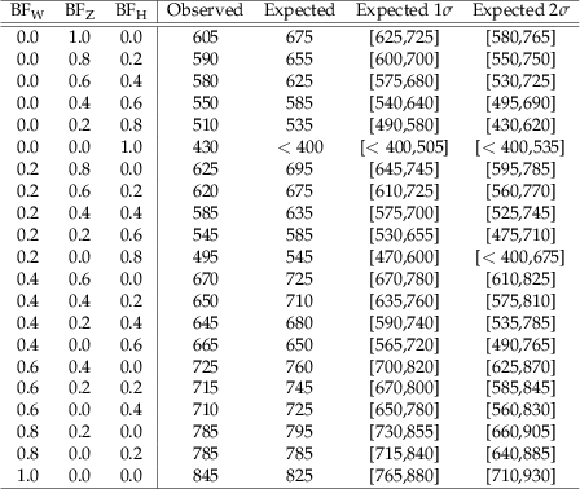
png pdf |
Table 17:
Observed and expected lower limits on the mass (in GeV) of the vector-like quark at 95% CL, for a range of different combinations of decay branching fractions. The $1\sigma $ and $2\sigma $ ranges on the expected limits are also given. The limits are determined under the assumption that pair production is the only available production mechanism for the vector-like quark. |
| Summary |
| A search has been performed for single and pair production of vector-like quarks, coupling to light quarks, in proton-proton collisions at $\sqrt{s} = $ 8 TeV at the LHC. In single production mode the search has been done for down-type quarks (electric charge -1/3), while in the pair production mode the search is sensitive to decays of vector-like quarks into first generation SM quarks. Inclusive and exclusive approaches have been used to perform this search. No significant excess over SM expectations has been observed. Lower limits on the mass of the vector-like quark have been determined by combining the results from both the single and pair production searches. Limits have also been extracted using the data from the pair production search alone. For all processes including single production the lower mass limits range from 400 GeV to 1800 GeV, depending on the vector-like quark branching fractions for decays to W, Z or H bosons and the assumed value of the electroweak single-production strength. When considered pair production data by itself masses between 430 GeV and 845 GeV are excluded. The obtained results are more stringent than those derived by the ATLAS experiment for single and pair production of VLQ coupling to light quarks [12,13]. |
| References | ||||
| 1 | L. Randall and R. Sundrum | A large mass hierarchy from a small extra dimension | PRL 83 (1999) 3370 | hep-ph/9905221 |
| 2 | D. Choudhury, T. Tait, and C. Wagner | Beautiful Mirrors and Precision Electroweak Data | PRD 65 (2002) 053002 | hep-ph/0109097 |
| 3 | N. Arkani-Hamed, A. Cohen, E. Katz, and A. Nelson | The Littlest Higgs | JHEP 0207 (2002) 034 | hep-ph/0206021v2 |
| 4 | M. Schmaltz | Physics beyond the standard model (Theory): Introducing the Little Higgs | NPPS 117 (2003) 40 | hep-ph/0210415 |
| 5 | M. Schmaltz and D. Tucker-Smith | Little Higgs Review | Ann. Rev. Nucl. Part. Sci 55 (2005) 229 | hep-ph/0502182v1 |
| 6 | D. Marzocca, M. Serone, and J. Shu | General Composite Higgs Models | JHEP 08 (2012) 013 | 1205.0770v3 |
| 7 | H.-C. Cheng, B. Dobrescu, and C. Hill | Electroweak Symmetry Breaking and Extra Dimensions | Nucl. Phys. B 589 (2000) 249 | hep-ph/9912343v3 |
| 8 | J. Kang, P. Langacker, and B. Nelson | Theory and Phenomenology of Exotic Isosinglet Quarks and Squarks | PRD 77 (2008) 035003 | 0708.2701v2 |
| 9 | D. Guadagnoli, R. Mohapatra, and I. Sung | Gauged Flavor Group with Left-Right Symmetry | JHEP 1104 (2011) 093 | 1103.4170v3 |
| 10 | A. Atre, G. Azuelos, M. Carena et al. | Model-Independent Searches for New Quarks at the LHC | JHEP 08 (2011) 080 | 1102.1987 |
| 11 | J. Aguilar-Saavedra, R. Benbrik, S. Heinemeyer, and M. Perez-Victoria | A handbook of vector-like quarks: mixing and single production | PRD 88 (2013) 112003 | 1306.0572v3 |
| 12 | ATLAS Collaboration | Search for heavy vector-like quarks coupling to light quarks in proton-proton collisions at $ \sqrt{s} = 7 $ TeV with the ATLAS detector | PLB 712 (2012) 22 | 1112.5755v2 |
| 13 | ATLAS Collaboration | Search for pair production of a new heavy quark that decays into a W boson and a light quark in pp collisions at $ \sqrt{s} = 8 $ TeV with the ATLAS detector | PRD 92 (2015) 112007 | 1509.04261 |
| 14 | M. Buchkremer, G. Cacciapaglia, A. Deandrea, and L. Panizzi | Model Independent Framework for Searches of Top Partners | Nucl.Phys. B 876 (2013) 376 | 1305.4172v3 |
| 15 | CMS Collaboration | The CMS experiment at the CERN LHC | JINST 3 (2008) S08004 | CMS-00-001 |
| 16 | J. Alwall et al. | MadGraph 5: going beyond | JHEP 06 (2011) 128 | 1106.0522 |
| 17 | T. Sj$\stackrel..\text o$strand, S. Mrenna, and P. Z. Skands | PYTHIA 6.4 physics and manual | JHEP 05 (2006) 026 | hep-ph/0603175 |
| 18 | P. Nason | A new method for combining NLO QCD with shower Monte Carlo algorithms | JHEP 11 (2004) 040 | hep-ph/0409146 |
| 19 | S. Frixione, P. Nason, and C. Oleari | Matching NLO QCD computations with parton shower simulations: the POWHEG method | JHEP 11 (2007) 070 | 0709.2092 |
| 20 | S. Alioli et al. | A general framework for implementing NLO calculations in shower Monte Carlo programs: the POWHEG BOX | JHEP 06 (2010) 043 | 1002.2581 |
| 21 | CMS Collaboration | Measurement of the $ \mathrm{ t \bar{t} } $ production cross section in the dilepton channel in pp collisions at $ \sqrt{s}=8 $ TeV | JHEP 02 (2014) 024 | CMS-TOP-12-007 1312.7582 |
| 22 | CMS Collaboration | Observation of the associated production of a single top quark and a W boson in pp collisions at $ \sqrt{s} = 8 $ TeV | PRL 112 (2014) 231802 | CMS-TOP-12-040 1401.2942 |
| 23 | CMS Collaboration | Measurement of $ W^+W^- $ and ZZ production cross sections in pp collisions at $ \sqrt{s} = 8 $ TeV | PLB 721 (2013) 190 | CMS-SMP-12-024 1301.4698 |
| 24 | CMS Collaboration | Measurement of WZ and ZZ production in pp collisions at $ \sqrt{s} = 8 $ TeV in final states with b-tagged jets. | EPJC 74 (2014) 2973 | CMS-SMP-13-011 1403.3047 |
| 25 | GEANT4 Collaboration | GEANT4 -- a simulation toolkit | NIMA 506 (2003) 250 | |
| 26 | CMS Collaboration | Particle-Flow Event Reconstruction in CMS and Performance for Jets, Taus, and MET | ||
| 27 | CMS Collaboration | Commissioning of the particle-flow event reconstruction with the first LHC collisions recorded in the CMS detector | CDS | |
| 28 | CMS Collaboration | Commissioning of the Particle-Flow Reconstruction in Minimum-Bias and Jet Events from pp Collisions at 7 TeV | ||
| 29 | CMS Collaboration | Particle-flow commissioning with muons and electrons from J/Psi and W events at 7 TeV | ||
| 30 | M. Cacciari, G. P. Salam, and G. Soyez | The anti-$ k_t $ jet clustering algorithm | JHEP 04 (2008) 063 | 0802.1189 |
| 31 | Y. L. Dokshitzer, G. Leder, S. Moretti, and B. R. Webber | Better jet clustering algorithms | JHEP 08 (1997) 001 | hep-ph/9707323 |
| 32 | M. Cacciari, G. P. Salam, and G. Soyez | Fastjet User Manual | EPJC 72 (2012) 1896 | 1111.6097 |
| 33 | M. Cacciari and G. P. Salam | Dispelling the $ N^{3} $ myth for the $ k_t $ jet-finder | PLB 641 (2006) 57 | hep-ph/0512210 |
| 34 | CMS Collaboration | Determination of jet energy calibration and transverse momentum resolution in CMS | JINST 6 (2011) P11002 | CMS-JME-10-011 1107.4277 |
| 35 | M. Cacciari, G. P. Salam, and G. Soyez | The catchment area of jets | JHEP 04 (2008) 005 | 0802.1188 |
| 36 | M. Cacciari and G. P. Salam | Pileup subtraction using jet areas | PLB 659 (2008) 119 | 0707.1378 |
| 37 | S. D. Ellis, C. K. Vermilion, and J. R. Walsh | Techniques for improved heavy particle searches with jet substructure | PRD 80 (2009) 051501 | 0903.5081 |
| 38 | S. D. Ellis, C. K. Vermilion, and J. R. Walsh | Recombination algorithms and jet substructure: Pruning as a tool for heavy particle searches | PRD 81 (2010) 094023 | 0912.0033 |
| 39 | CMS Collaboration | Performance of b tagging at $ \sqrt{s} $=8 TeV in multijet, $ t\bar{t} $ and boosted topology events | CMS-PAS-BTV-13-001 | CMS-PAS-BTV-13-001 |
| 40 | CMS Collaboration | Performance of quark/gluon discrimination using pp collision data at $ \sqrt{s} $ = 8 TeV | CMS-PAS-JME-13-002 | CMS-PAS-JME-13-002 |
| 41 | K. Olive et al. | The Review of Particle Physics | CPC 38 (2014) 090001 | |
| 42 | CMS Collaboration | Search for vector-like charge 2/3 T quarks in proton-proton collisions at $ \sqrt{s} $ = 8 TeV | PRD 93 (2016) 012003 | CMS-B2G-13-005 1509.04177 |
| 43 | J. Ott | theta ? theta-auto testing documentation | ||
| 44 | G. Cowan | PDG Review on statistics (chap. 38, page 472) | CPC 38 (2014) 090001 | |
| 45 | CMS Collaboration | Measurement of the differential cross section for top quark pair production in pp collisions at $ \sqrt{s} = 8 $ TeV | EPJC 75 (2015) 542 | CMS-TOP-12-028 1505.04480 |
| 46 | CMS Collaboration | CMS Luminosity Based on Pixel Cluster Counting - Summer 2013 Update | CMS-PAS-LUM-13-001 | CMS-PAS-LUM-13-001 |
| 47 | P. F. M. Czakon and A. Mitov | Total Top-Quark Pair-Production Cross Section at Hadron Colliders Through $ O({\alpha_S}^4) $ | PRL 110 (2013) 252004 | 1303.6254 |
| 48 | P. M. Nadolsky et al. | Implications of CTEQ global analysis for collider observables | PRD 78 (2008) 013004 | 0802.0007 |
|
|
Compact Muon Solenoid LHC, CERN |
|
|
|
|
|
|